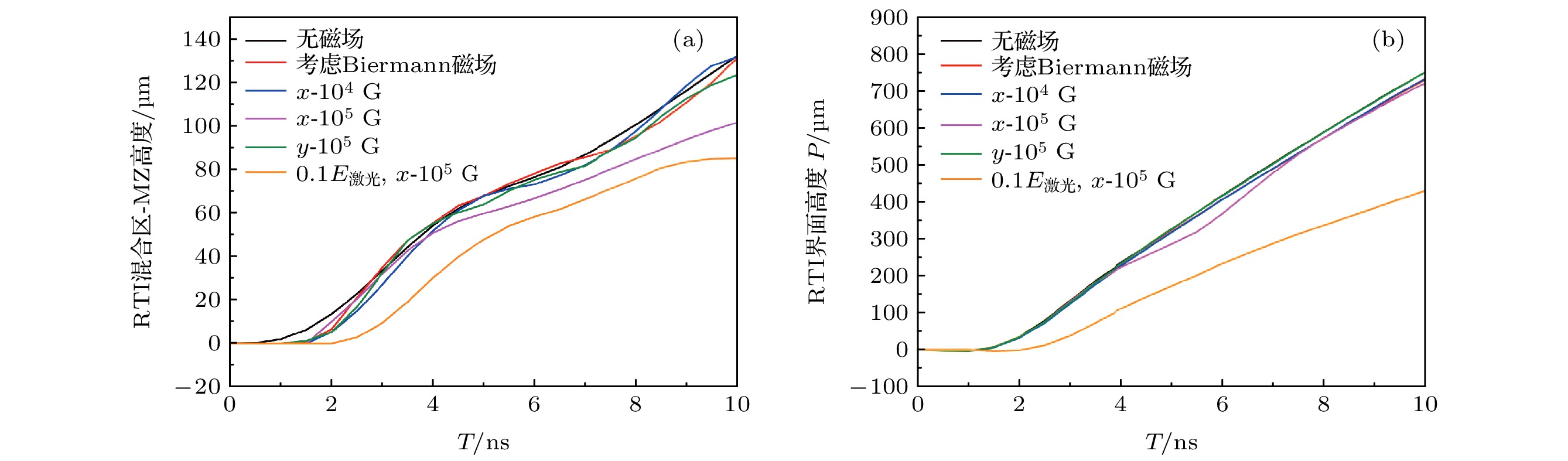
-
Rayleigh-Taylor instability (RTI) is a fundamental physical phenomenon in fluids and plasmas, and plays a significant role in astrophysics, space physics, and engineering. Especially in inertial confinement fusion (ICF) research, numerous experimental and simulation results have identified RTI as one of the most significant barriers to achieving fusion. Understanding the origin and development of RTI will be conducive to formulating mitigation measures to curb the growth of instability, thereby improving the odds of ICF success. Although there have existed many theoretical and experimental studies of RTI under high energy density, there are few experiments to systematically explore the influence of magnetic fields on the evolution of magnetized RTI. Here, a new experimental scheme is proposed based on the Shenguang-II laser facility on which the nanosecond laser beams are used to drive modulation targets of polystyrene (CH) and low-density foam layers. A shock wave is generated after the laser’s CH modulation layer has been ablated, and propagates through CH to low-density foam. Moreover, Richtmyer-Meshkov instability is triggered off when the shock wave accelerates the target. When the laser pulse ends, the shock wave evolves into a blast wave, causing the system to decelerate, resulting in RTI in the reference system of the interface. In this paper the open-source radiation MHD simulation code (FLASH) is used to simulate the RTI generated by a laser-driven modulation target. The evolution of RTI under no magnetic field, under Biermann self-generated magnetic field, and under different applied magnetic fields are systematically investigated and compared with each other. The simulation results show that the Biermann self-generated magnetic field and the applied magnetic field parallel to flow direction do not change the interface dynamics in the evolution process of RTI. Nevertheless, the applied magnetic field perpendicular to flow direction can stabilize RTI and the Kelvin-Helmholtz vortex at the tail of the RTI spike. Magnetic pressure plays a decisive role. The present results provide a reference for the follow-up study of target physics related to ICF and deepen the understanding of the fluid mixing process.
[1] Rayleigh L 1882 Proc. R. Math. Soc. s1–14 170
Google Scholar
[2] Taylor G I 1950 Proc. R. Soc. London, Ser. A 201 192
Google Scholar
[3] Zhou Y, Williams R, Ramaprabhu P, Groom M, Attal N 2021 Physica D 423 132838
Google Scholar
[4] 刘昕悦, 李伟民, 刘永江, 戴黎明, 董昊, 李婧, 赵英利 2019 岩石学报 35 1071
Google Scholar
Liu X Y, Li W M, Liu YJ, Dai L M, Dong H, Li J, Zhao Y L 2019 Acta Petrologica Sin. 35 1071
Google Scholar
[5] Uchiyama Y, Aharonian F, Tanaka T, Takahashi T, Maeda Y 2007 Nature 449 576
Google Scholar
[6] Sano T, Tamatani S, Matsuo K, Law K F F, Morita T, Egashira S, Ota M, Kumar R, Shimogawara H, Hara Y 2021 Phys. Rev. E 104 035206
Google Scholar
[7] Kuramitsu Y, Ohnishi N, Sakawa Y, Morita T, Tanji H, Ide T, Nishio K, Gregory C D, Waugh J N, Booth N 2016 Phys. Plasmas 23 032126
Google Scholar
[8] Nymark T K, Fransson C, Kozma C 2006 Astron. Astrophys. 449 171
Google Scholar
[9] Ma X, Delamere P A, Otto A 2016 J. Geophys. Res. 121 5260
Google Scholar
[10] Matsumoto Y, Hoshino M 2004 Geophys. Res. Lett. 31 1
Google Scholar
[11] Wang L F, Ye W H, Chen Z, Li Y S, Ding Y K, Zhao K G, Zhang J, Li Z Y, Yang Y P, Wu J F, et al. 2021 High Power Laser and Particle Beams 33 012001
Google Scholar
[12] Hammel B A, Haan S W, Clark D S, Edwards M J, Langer S H, Marinak M M, Patel M V, Salmonson J D, Scott H A 2010 High Energy Density Phys. 6 171
Google Scholar
[13] Clark D S, Haan S W, Cook A W, Edwards M J, Hammel B A, Koning J M, Marinak M M 2011 Phys. Plasmas 18 082701
Google Scholar
[14] Zhou Y 2017 Phys. Rep. 723–725 1
Google Scholar
[15] Vandenboomgaerde M, Bonnefille M, Gauthier P 2016 Phys. Plasmas 23 020501
Google Scholar
[16] Capelli D, Schmidt D W, Cardenas T Rivera G, Randolph R B, Fierro F, Merritt E C, Flippo K A, Doss F W, Kline J L 2016 Fusion Sci. Technol. 70 316
Google Scholar
[17] 孙伟, 安维明, 仲佳勇 2020 69 244701
Google Scholar
Sun W, An W M, Zhong J Y 2020 Acta Phys. Sin. 69 244701
Google Scholar
[18] Remington B A, Park H S, Casey D T, Cavalloa R M, Clarka D S, Huntingtona C M, Kuranzb C C, Milesa A R, Nagela S R, Ramana K S, Smalyuk V A 2019 Proc. Natl. Acad. Sci. U.S.A. 116 18233
Google Scholar
[19] Chandrasekhar S 1961 Hydrodynamic and Hydromagnetic Stability (Oxford: Clarendon Press) pp481–512
[20] Sun Y B, Wang C 2020 Phys. Rev. E 101 053110
Google Scholar
[21] 赵凯歌, 薛创, 王立锋, 叶文华, 吴俊峰, 丁永坤, 张维岩, 贺贤土 2018 67 094701
Google Scholar
Zhao K G, Xue C, Wang L F, Ye W H, Wu J F, Ding Y K, Zhang W Y, He X T 2018 Acta Phys. Sin. 67 094701
Google Scholar
[22] Wang L F, Ye W H, Li Y J 2010 Phys. Plasmas 17 052305
Google Scholar
[23] Wang L F, Wu J F, Ye W H, Zhang W Y, He X T 2013 Phys. Plasmas 20 042708
Google Scholar
[24] Reckinger S J, Livescu D, Vasilyev O V 2016 J. Comput. Phys. 313 181
Google Scholar
[25] Wieland S, Reckinger S, Hamlington P E, Livescu D 2017 47th AIAA Fluid Dynamics Conference Denver, Colorado, USA, June 5–9, 2017 p3974
[26] 李源, 罗喜胜 2014 63 085203
Google Scholar
Li Y, Luo X S 2014 Acta Phys. Sin. 63 085203
Google Scholar
[27] Rigon G, Casner A, Albertazzi B, Michel Th, Mabey P, Falize E, Ballet J, Van Box Som L, Pikuz S, Sakawa Y, Sano T, Faenov A, Pikuz T, Ozaki N, Kuramitsu Y, Valdivia M P, Tzeferacos P, Lamb D, Koenig M 2019 Phys. Rev. E 100 021201
Google Scholar
[28] Sauppe J P, Palaniyappan S, Tobias B J, Kline J L, Flippo K A, Landen O L, Shvarts D, Batha S H, Bradley P A, Loomis E N, Vazirani N N, Kawaguchi C F, Kot L, Schmidt D W, Day T H, Zylstra A B, Malka E 2020 Phys. Rev. Lett. 124 185003
Google Scholar
[29] Gao L, Nilson P M, Igumenschev I V, Fiksel G, Yan R, Davies J R, Martinez D, Smalyuk V, Haines M G, Blackman E G, Froula D H, Betti R, Meyerhofer D D 2013 Phys. Rev. Lett. 110 185003
Google Scholar
[30] Khiar B, Revet G, Ciardi A, Burdonov K, Filippov E, Béard J, Cerchez M, Chen S N, Gangolf T, Makarov S S, Ouillé M, Safronova M, Skobelev I Y, Soloviev A, Starodubtsev M, Willi O, Pikuz S, Fuchs J 2019 Phys. Rev. Lett. 12320 205001
Google Scholar
[31] Sun Y B, Gou J N, Wang C 2021 Phys. Plasmas 28 092707
Google Scholar
[32] Barbeau Z, Raman K, Manuel M, Nagel S, Shivamoggi B 2022 Phys. Plasmas 29 012306
Google Scholar
[33] Fryxell B, Olson K, Ricker P, Timmes F X, Zingale M, Lamb D Q, Macneice P, Rosner R, Truran J W, Tufo H M 2000 Astrophys. J. Suppl. Ser. 131 273
Google Scholar
[34] Lei Z, Zhao Z H, Yao W P, Xie Y, Jiao J L, Zhou C T, Zhu S P, He X T, Qiao B 2020 Plasma Phys. Controlled Fusion 62 095020
Google Scholar
[35] Macfarlane J J 1989 Comput. Phys. Commun. 56 259
Google Scholar
[36] Sano T, Inoue T, Nishihara K 2013 Phys. Rev. Lett. 111 205001
Google Scholar
[37] Manuel M J E, Khiar B, Rigon G, Albertazzi B, Klein S R, Kroll F, Brack F E, Michel T, Mabey P, Pikuz S, Williams J C, Koenig M, Casner A, Kuranz C C 2021 Matter Radiat. Extrem. 6 026904
Google Scholar
[38] Shivamoggi B K 1982 Z. Angew. Math. Phys. 33 693
Google Scholar
-
图 1 实验方案初始设置图
Figure 1. Experimental scheme initial setup.
图 2 无磁场情况下, 不同时刻电子密度分布图 (a)
$2.5 \; \rm{ns}$ 时刻; (b)$5 \; \rm{ns}$ 时刻; (c)$7.5 \; \rm{ns}$ 时刻; (d)$10 \; \rm{ns}$ 时刻Figure 2. In the absence of a magnetic field, the electron density distribution at different times: (a)
$2.5 \; \rm{ns}$ ; (b)$5 \; \rm{ns}$ ; (c)$7.5 \; \rm{ns}$ ; (d)$10 \; \rm{ns}$ 
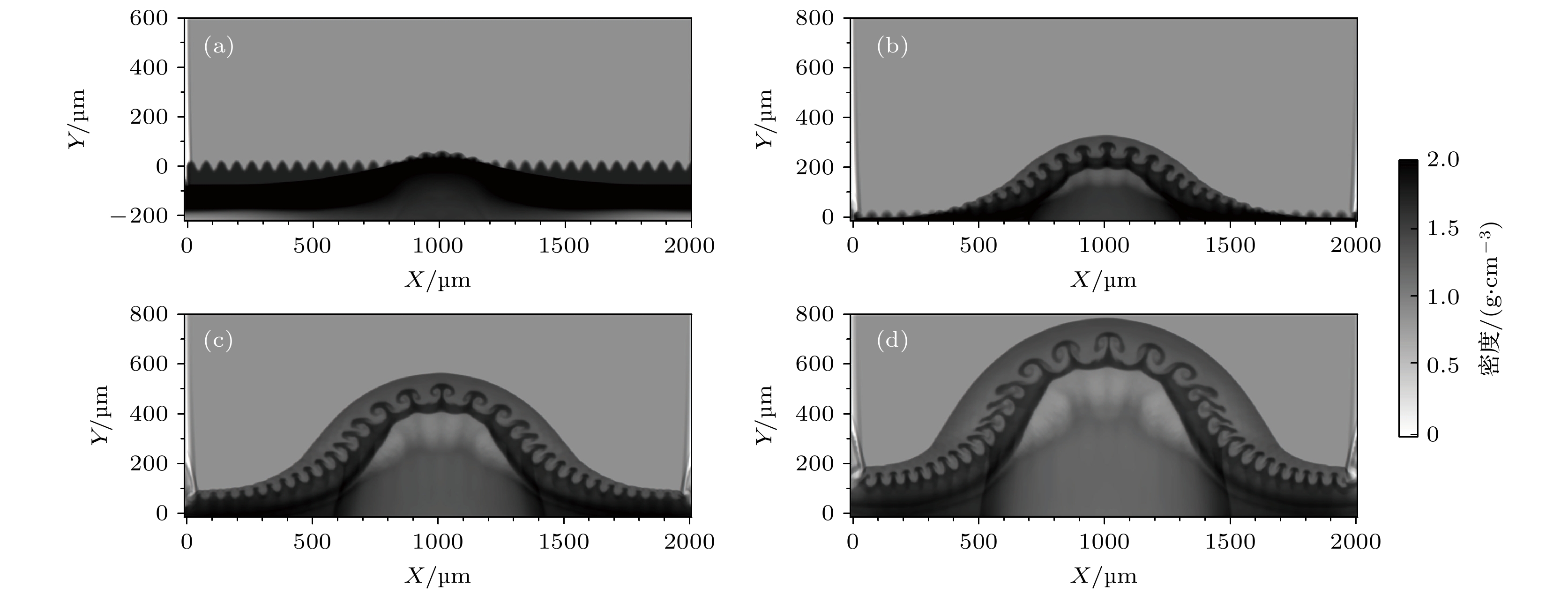
图 3
$10 \; \rm{ns}$ 时刻的电子密度分布图 (a)无磁场情况; (b)考虑Biermann自生磁场情况; (c)考虑Biermann自生磁场、平面靶情况Figure 3. Snapshots of the electron density distribution at the time of
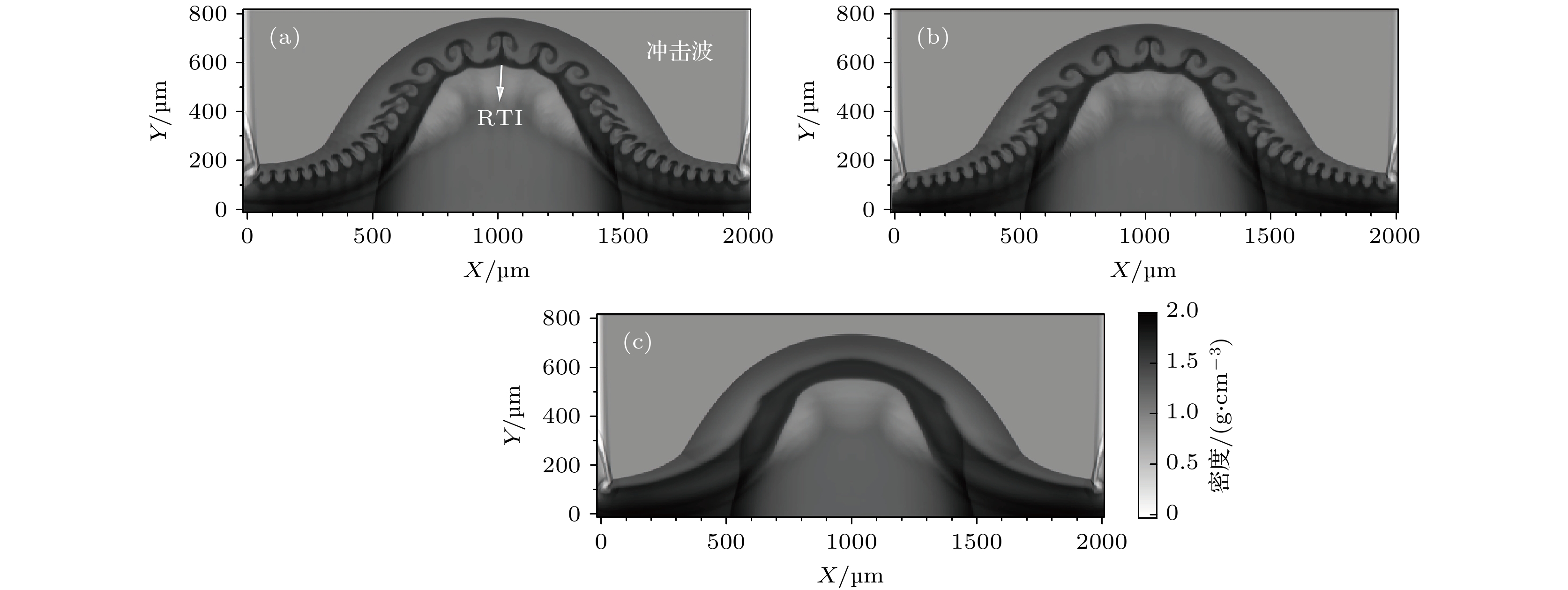
$10 \; \rm{ns}$ : (a) Without magnetic field; (b) considering Biermann self-generated magnetic field; (c) Biermann self-generated magnetic field and plane target
图 4 不同初始外加磁场条件下, 10 ns时刻的电子密度分布图 (a) x方向外加
$10^{4} \; \rm{G}$ 磁场; (b) x方向外加$10^{5} \; \rm{G}$ 磁场; (c) y方向外加$10^{5} \; \rm{G}$ 磁场; (d)激光能量降为原来的10%时, x方向外加$10^{5} \; \rm{G}$ 磁场情况Figure 4. Snapshots of electron density distribution at
$10 \; \rm{ns}$ under different initial applied magnetic field conditions: (a) With$10^{4} \; \rm{G}$ in the x-direction; (b) with$10^{5} \ \rm{G}$ in the x-direction; (c) with$10^{5} \; \rm{G}$ in the y-direction; (d) when the laser energy is reduced to 10%, with$10^{5} \; \rm{G}$ in the x-direction
图 5 不同初始条件下, (a) RTI的MZ高度和(b)界面高度P随时间的发展情况
Figure 5. Development of (a) the RTI MZ height and (b) the RTI interface height with time under different initial conditions
图 6 不同初始条件下, (a) RTI尖钉高度和(b) RTI气泡高度随时间的变化情况
Figure 6. Changes of (a) RTI spike height and (b) RTI bubble height with time under different initial conditions
图 7 不同初始条件下, (a)外围冲击波界面高度和(b) KHI涡旋高度H随时间的发展情况
Figure 7. Development of (a) the peripheral shock wave interface height and (b) KHI vortex height H with time under different initial conditions
图 8 有无磁场时,
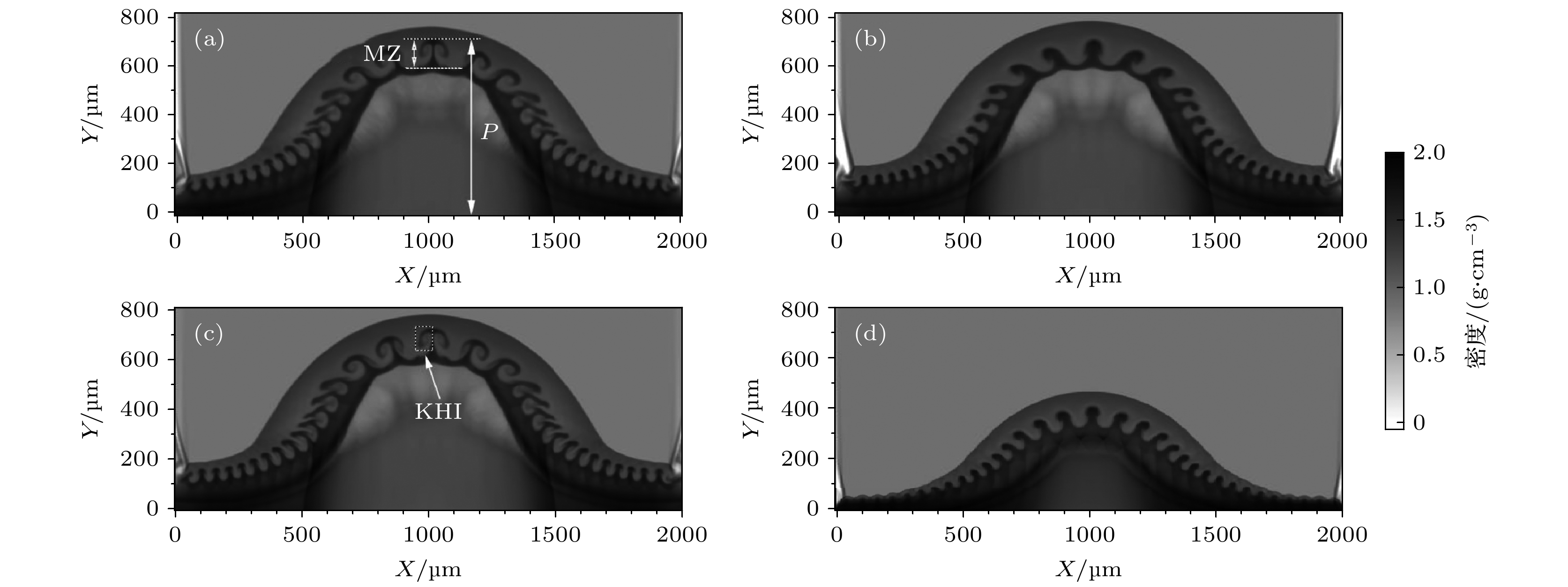
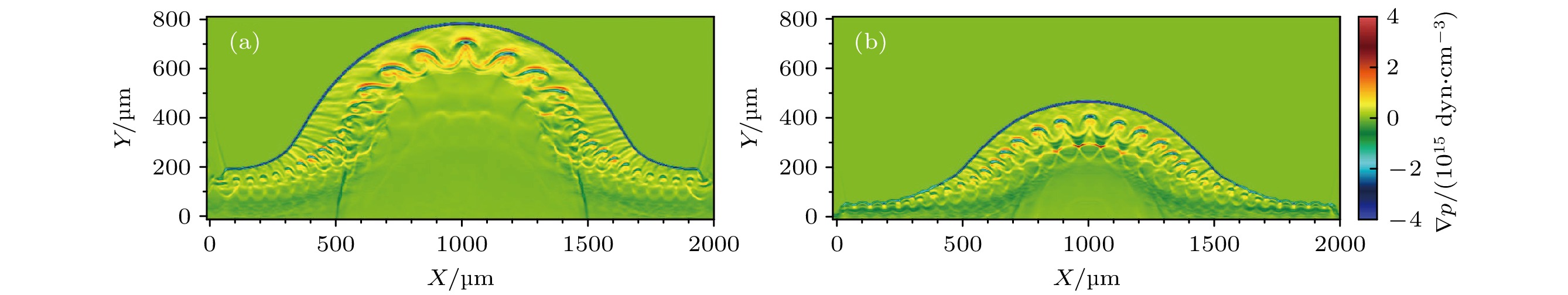
$10 \ \mathrm{ns}$ 时刻RTI演化区域涡度分布情况 (a)无磁场; (b)在x方向施加$10^{5} \; \rm{G}$ 磁场; (c)当相应激光的入射能量降低到10%时, 在x方向外加$10^{5} \; \rm{G}$ 磁场Figure 8. Vorticity distribution in the RTI evolution region at
$10 \; \rm{ns}$ with or without magnetic field: (a) Without the magnetic field; (b) a$10^{5} \; \rm{G}$ magnetic field is applied in the x-direction; (c) when the incident energy of the corresponding laser is reduced to 10%, a$10^{5} \ \rm{G}$ is applied in the x-direction
图 9 x方向初始外加
$10^{5} \; \rm{G}$ 磁场时, 不同激光条件、不同时刻磁场强度分布图 (a), (b)分别对应入射激光能量为$1000 \ \mathrm{J}$ 时,$5$ 和$10 \; \rm{ns}$ 时刻磁场强度分布图; (c), (d)分别对应入射激光$100 \ \mathrm{J}$ 时$5 $ 和$10 \; \rm{ns}$ 时刻磁场强度分布图Figure 9. Magnetic field intensity distribution at different times under different laser conditions, when
$10^{5} \; \rm{G}$ is initially applied in x-direction: (a), (b) The magnetic field intensity distribution at$5 $ and$10 \; \rm{ns}$ corresponding to incident laser$1000 \ \mathrm{J}$ , respectively; (c), (d) the magnetic field intensity distribution at$5$ and$10 \; \rm{ns}$ corresponding to incident laser$100 \; \rm{J}$ , respectively
图 10 x方向初始外加
$10^{5} \; \rm{G}$ 磁场时, 不同激光条件下,$10 \ \mathrm{ns}$ 时刻磁压力与磁张力分布图 (a), (b)分别对应入射激光能量为$1000$ 和$100 \ \mathrm{J}$ 时磁压力分布情况; (c), (d)分别对应入射激光能量为$1000$ 和$100 \; \rm{J}$ 时磁张力分布情况Figure 10. Distribution of magnetic pressure and magnetic tension at
$10 \; \rm{ns}$ under different laser conditions when$10^{5} \; \rm{G}$ is initially applied in x-direction: (a), (b) The magnetic pressure distribution corresponding to the incident laser energy of$1000 $ and$100 \ \mathrm{J}$ respectively; (c), (d) the magnetic tension distribution corresponding to the incident laser energy of$1000$ and$100 \; \rm{J}$ respectively
图 11 x方向初始外加
$10^{5} \; \rm{G}$ 磁场时, 不同激光条件下,$10 \ \mathrm{ns}$ 时刻负压力梯度力分布 (a)入射激光能量为$1000 \ \mathrm{J}$ ; (b)入射激光能量为$100 \ \mathrm{J}$ Figure 11. When a
$10^{5} \; \rm{G}$ is initially applied in the x-direction, the negative pressure gradient force distribution at$10 \ \mathrm{ns}$ under different laser conditions: (a) when the incident laser energy is$1000 \ \mathrm{J}$ ; (b) when the incident laser energy is$100 \; \rm{J}$ 表 1 不同初始条件下模拟参数比较.
Table 1. Comparison of simulation parameters under different initial conditions.
算例 靶型 外加磁场/G Biermann
自生磁场激光能量/J 1 调制靶 0 忽略 1000 2 调制靶 0 考虑 1000 3 平面靶 0 考虑 1000 4 调制靶 $10^{4}$ (x方向) 忽略 1000 5 调制靶 $10^{5} $ (x方向) 忽略 1000 6 调制靶 $ 10^{5} $ (y方向) 忽略 1000 7 调制靶 $ 10^{5} $ (x方向) 忽略 100 
DownLoad: CSV
-
[1] Rayleigh L 1882 Proc. R. Math. Soc. s1–14 170
Google Scholar
[2] Taylor G I 1950 Proc. R. Soc. London, Ser. A 201 192
Google Scholar
[3] Zhou Y, Williams R, Ramaprabhu P, Groom M, Attal N 2021 Physica D 423 132838
Google Scholar
[4] 刘昕悦, 李伟民, 刘永江, 戴黎明, 董昊, 李婧, 赵英利 2019 岩石学报 35 1071
Google Scholar
Liu X Y, Li W M, Liu YJ, Dai L M, Dong H, Li J, Zhao Y L 2019 Acta Petrologica Sin. 35 1071
Google Scholar
[5] Uchiyama Y, Aharonian F, Tanaka T, Takahashi T, Maeda Y 2007 Nature 449 576
Google Scholar
[6] Sano T, Tamatani S, Matsuo K, Law K F F, Morita T, Egashira S, Ota M, Kumar R, Shimogawara H, Hara Y 2021 Phys. Rev. E 104 035206
Google Scholar
[7] Kuramitsu Y, Ohnishi N, Sakawa Y, Morita T, Tanji H, Ide T, Nishio K, Gregory C D, Waugh J N, Booth N 2016 Phys. Plasmas 23 032126
Google Scholar
[8] Nymark T K, Fransson C, Kozma C 2006 Astron. Astrophys. 449 171
Google Scholar
[9] Ma X, Delamere P A, Otto A 2016 J. Geophys. Res. 121 5260
Google Scholar
[10] Matsumoto Y, Hoshino M 2004 Geophys. Res. Lett. 31 1
Google Scholar
[11] Wang L F, Ye W H, Chen Z, Li Y S, Ding Y K, Zhao K G, Zhang J, Li Z Y, Yang Y P, Wu J F, et al. 2021 High Power Laser and Particle Beams 33 012001
Google Scholar
[12] Hammel B A, Haan S W, Clark D S, Edwards M J, Langer S H, Marinak M M, Patel M V, Salmonson J D, Scott H A 2010 High Energy Density Phys. 6 171
Google Scholar
[13] Clark D S, Haan S W, Cook A W, Edwards M J, Hammel B A, Koning J M, Marinak M M 2011 Phys. Plasmas 18 082701
Google Scholar
[14] Zhou Y 2017 Phys. Rep. 723–725 1
Google Scholar
[15] Vandenboomgaerde M, Bonnefille M, Gauthier P 2016 Phys. Plasmas 23 020501
Google Scholar
[16] Capelli D, Schmidt D W, Cardenas T Rivera G, Randolph R B, Fierro F, Merritt E C, Flippo K A, Doss F W, Kline J L 2016 Fusion Sci. Technol. 70 316
Google Scholar
[17] 孙伟, 安维明, 仲佳勇 2020 69 244701
Google Scholar
Sun W, An W M, Zhong J Y 2020 Acta Phys. Sin. 69 244701
Google Scholar
[18] Remington B A, Park H S, Casey D T, Cavalloa R M, Clarka D S, Huntingtona C M, Kuranzb C C, Milesa A R, Nagela S R, Ramana K S, Smalyuk V A 2019 Proc. Natl. Acad. Sci. U.S.A. 116 18233
Google Scholar
[19] Chandrasekhar S 1961 Hydrodynamic and Hydromagnetic Stability (Oxford: Clarendon Press) pp481–512
[20] Sun Y B, Wang C 2020 Phys. Rev. E 101 053110
Google Scholar
[21] 赵凯歌, 薛创, 王立锋, 叶文华, 吴俊峰, 丁永坤, 张维岩, 贺贤土 2018 67 094701
Google Scholar
Zhao K G, Xue C, Wang L F, Ye W H, Wu J F, Ding Y K, Zhang W Y, He X T 2018 Acta Phys. Sin. 67 094701
Google Scholar
[22] Wang L F, Ye W H, Li Y J 2010 Phys. Plasmas 17 052305
Google Scholar
[23] Wang L F, Wu J F, Ye W H, Zhang W Y, He X T 2013 Phys. Plasmas 20 042708
Google Scholar
[24] Reckinger S J, Livescu D, Vasilyev O V 2016 J. Comput. Phys. 313 181
Google Scholar
[25] Wieland S, Reckinger S, Hamlington P E, Livescu D 2017 47th AIAA Fluid Dynamics Conference Denver, Colorado, USA, June 5–9, 2017 p3974
[26] 李源, 罗喜胜 2014 63 085203
Google Scholar
Li Y, Luo X S 2014 Acta Phys. Sin. 63 085203
Google Scholar
[27] Rigon G, Casner A, Albertazzi B, Michel Th, Mabey P, Falize E, Ballet J, Van Box Som L, Pikuz S, Sakawa Y, Sano T, Faenov A, Pikuz T, Ozaki N, Kuramitsu Y, Valdivia M P, Tzeferacos P, Lamb D, Koenig M 2019 Phys. Rev. E 100 021201
Google Scholar
[28] Sauppe J P, Palaniyappan S, Tobias B J, Kline J L, Flippo K A, Landen O L, Shvarts D, Batha S H, Bradley P A, Loomis E N, Vazirani N N, Kawaguchi C F, Kot L, Schmidt D W, Day T H, Zylstra A B, Malka E 2020 Phys. Rev. Lett. 124 185003
Google Scholar
[29] Gao L, Nilson P M, Igumenschev I V, Fiksel G, Yan R, Davies J R, Martinez D, Smalyuk V, Haines M G, Blackman E G, Froula D H, Betti R, Meyerhofer D D 2013 Phys. Rev. Lett. 110 185003
Google Scholar
[30] Khiar B, Revet G, Ciardi A, Burdonov K, Filippov E, Béard J, Cerchez M, Chen S N, Gangolf T, Makarov S S, Ouillé M, Safronova M, Skobelev I Y, Soloviev A, Starodubtsev M, Willi O, Pikuz S, Fuchs J 2019 Phys. Rev. Lett. 12320 205001
Google Scholar
[31] Sun Y B, Gou J N, Wang C 2021 Phys. Plasmas 28 092707
Google Scholar
[32] Barbeau Z, Raman K, Manuel M, Nagel S, Shivamoggi B 2022 Phys. Plasmas 29 012306
Google Scholar
[33] Fryxell B, Olson K, Ricker P, Timmes F X, Zingale M, Lamb D Q, Macneice P, Rosner R, Truran J W, Tufo H M 2000 Astrophys. J. Suppl. Ser. 131 273
Google Scholar
[34] Lei Z, Zhao Z H, Yao W P, Xie Y, Jiao J L, Zhou C T, Zhu S P, He X T, Qiao B 2020 Plasma Phys. Controlled Fusion 62 095020
Google Scholar
[35] Macfarlane J J 1989 Comput. Phys. Commun. 56 259
Google Scholar
[36] Sano T, Inoue T, Nishihara K 2013 Phys. Rev. Lett. 111 205001
Google Scholar
[37] Manuel M J E, Khiar B, Rigon G, Albertazzi B, Klein S R, Kroll F, Brack F E, Michel T, Mabey P, Pikuz S, Williams J C, Koenig M, Casner A, Kuranz C C 2021 Matter Radiat. Extrem. 6 026904
Google Scholar
[38] Shivamoggi B K 1982 Z. Angew. Math. Phys. 33 693
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4226
- PDF Downloads: 98
- Cited By: 0
