-
A plasmonic dimer composed of a gold split ring and a gold disk is proposed. The scattering spectra and electromagnetic field distributions on the surface of the dimer are calculated by the finite difference time domain method, and the effects of split gap orientation and structural symmetry breaking of the ring on Fano resonance characteristics are theoretically investigated in detail. The results show the multiple Fano resonances that can be formed due to the destructive interference between the electric dipole mode of the disk and multiple multipolar modes of the split ring, and the red shift when the symmetry breaking of the split ring is broken. When the split gap of the ring is parallel to the interparticle axis of the dimer, multiple Fano resonances based on the odd-order mode and the even-order mode of the split ring can be generated, and more even-order Fano resonances can be formed due to the further symmetry breaking of the split ring. In addition, a more refined scattering spectrum can be obtained as the ring internal surface moves far away from the disk along the interparticle axis. On the other hand, Fano resonances based on the lower order multipolar modes of the ring can be increased as they move away from the split gap. When the split gap of the ring is perpendicular to the interparticle axis of the dimer, only the even-order Fano resonances can be excited, and these resonances increase with the ring internal surface going away from the disk no matter whether the split gap of the ring faces the disk or not. As the structural symmetry of the dimer is further broken due to the ring internal surface moving along the split gap direction, the odd-order Fano resonance can be successfully produced in the dimer with the split gap coming back to the disk, at the same time, the even-order Fano resonances are gradually weaken perhaps due to the complicated competitive behaviors of spectral overlapping between the dipole mode of the disk and multipolar mode of the ring in energy. However, there is no odd-order Fano resonance appearing in the dimer with the split gap facing the disk except for a slightly increased even-order Fano resonance, as the structural symmetry of the dimer is further broken. These results are expected to be useful in designing multiple Fano controllable split ring disk dimers and also developing the application of multiwavelength micro-nano photonics.
-
Keywords:
- Fano resonance /
- surface plasmon /
- dimer /
- finite difference time domain
[1] Maier S A 2007 Plasmonics: Fundamentals and Applications (New York: Springer Science & Business Media) pp107−138
[2] Zheng C, Jia T, Zhao H, Xia Y, Zhang S, Feng D, Sun Z 2018 RSC Adv. 8 3381
Google Scholar
[3] Ling Y, Huang L, Hong W, Liu T, Luan J, Liu W, Lai J, Li H 2018 Nanoscale 10 19517
Google Scholar
[4] Li G, Hu H, Wu L 2019 Phys. Chem. Chem. Phys. 21 252
Google Scholar
[5] Zhang Y, Zhen Y, Neumann O, Day J K, Nordlander P, Halas N J 2014 Nat. Commun. 5 4424
Google Scholar
[6] Zhi L, Liu W, Li Z, Hua C, Chen S, Tian J 2017 Opt. Lett. 42 3117
Google Scholar
[7] Wan W, Zheng W, Chen Y, Liu Z 2014 Nanoscale 6 9093
Google Scholar
[8] Biswas S, Duan J, Nepal D, Park K, Pachter R, Vaia R A 2013 Nano Lett. 13 6287
Google Scholar
[9] Liu S, Yue P, Zhang S, Wang M, Dai H, Chen Y, Nie Z, Cui Y, Han J, Duan H 2020 Adv. Opt. Mater. 8 1901109
Google Scholar
[10] Hu H, Zhang F, Li G, Chen J, Li Q, Wu L 2018 Photon. Res. 6 204
Google Scholar
[11] Dutta A, Alam K, Nuutinen T, Hulkko E, Vartiainen E M 2019 Opt. Express 27 30031
Google Scholar
[12] Yang L, Li P, Liu H, Tang X, Liu J 2015 Chem. Soc. Rev. 44 2837
Google Scholar
[13] Hao F, Larsson E M, Ali T A, Sutherland D S, Nordlander P 2008 Chem. Phys. Lett. 458 262
Google Scholar
[14] Koya A N, Ji B, Hao Z, Lin J 2016 J. Opt. 18 055007
Google Scholar
[15] Dalius S, Gediminas L, Andrius V, Ilvinas K, Gintaras V 2017 Opt. Express 25 25113
Google Scholar
[16] Rockstuhl C, Lederer F, Etrich C, Zentgraf T, Giessen H 2006 Opt. Express 14 8827
Google Scholar
[17] Liu S D, Yue P, Zhu M Q, Wen J, Lei D 2019 Opt. Express 27 26377
Google Scholar
[18] Li Y, Huo Y, Zhang Y and Zhang Z 2017 Plasmonics 12 1613
Google Scholar
[19] Halas N J, Lal S, Chang W S, Nordlander P 2011 Chem. Rev. 111 3913
Google Scholar
[20] Wang W, Li Y, Peng J, Chen Z, Qian J, Chen J, Xu J, Sun Q 2014 J. Opt. 16 035002
Google Scholar
[21] Zhang Q, Wen X, Li G, Ruan Q, Wang J, Xiong Q 2013 ACS Nano 7 11071
Google Scholar
[22] Sun B, Zhao L, Wang C, Yi X, Liu Z, Wang G, Li J 2014 J. Phys. Chem. C 118 25124
Google Scholar
[23] Wang L, Liu Z, Yi X, Zhang Y, Li H, Li J, Wang G 2016 J. Appl. Phys. 119 173106
Google Scholar
[24] Cui J, Ji B, Song X, Lin J 2019 Plasmonics 14 41
Google Scholar
[25] Zhang X, Liu F, Yan X, Liang L, Wei D 2020 J. Opt. 22 115003
Google Scholar
[26] Sheridan A K, Clark A W, Glidle A, Cooper J M, Cumming D R S 2007 Appl. Phys. Lett. 90 143105
Google Scholar
[27] Taflove A, Hagness S 2000 Computational Electrodynamics: The Finite-Difference Time-Domain Method (Vol. 2) (Boston: Artech House) pp75−85
[28] Johnson P B, Christy R W 1972 Phys. Rev. B 6 4370
Google Scholar
[29] Lovera A, Gallinet B, Nordlander P, Martin O 2013 ACS Nano 7 4527
Google Scholar
[30] Zhang Y, Jia T Q, Zhang H M, Xu Z Z 2012 Opt. Lett. 37 4919
Google Scholar
[31] Zhang L, Dong Z, Wang Y, Liu Y, Zhang S, Yang J K W, Qiu C 2015 Nanoscale 7 12018
Google Scholar
[32] Zong X, Li L, Liu Y 2019 Opt. Mater. Express 9 870
Google Scholar
[33] Hu Y, Noelck S J, Drezek R A 2010 ACS Nano 4 1521
Google Scholar
[34] Xiao F, Zhang J, Yu W, Zhu W, Zhao J 2020 Opt. Express 28 3000
Google Scholar
[35] Wang N, Zeisberger M, Huebner U, Giannini V, Schmidt M A 2019 Sci. Rep. 9 2873
Google Scholar
-
图 1 劈裂环-盘二聚体的几何结构示意图, 其中R = 150 nm, R1 = 170 nm, R2 = 200 nm, θ = 30°, g = 10 nm, 二聚体高度为30 nm
Figure 1. Geometrical structure of the split ring-disk dimer, where R = 150 nm, R1 = 170 nm, R2 = 200 nm, θ = 30°, g = 10 nm, the height of dimer is 30 nm.
图 2 当φ = 0°和Δ
x = Δy = 0 nm时二聚体、单独圆盘和单独劈裂环的散射谱. 插图为对应共振波长处激发的二聚体表面电场Ez分布 Figure 2. Scattering spectra of a single dimer, individual disk and individual split ring with φ = 0° and Δx = Δy = 0 nm. Insets show the calculated electric field distributions Ez on the surface of the dimer at the indicated resonances.
图 3 固定φ = 0°和Δy = 0 nm, 二聚体的散射谱随(a) Δx = 0—20 nm, (b) Δx = –20—0 nm的变化. 插图为在指定频率处的二聚体表面电场分布
Figure 3. Scattering spectra of the dimer with φ = 0° and Δy = 0 nm for different values of Δx: (a) Δx = 0–20 nm; (b) Δx = –20–0 nm. Insets show the calculated electric field (a) Ez (Δx = 20 nm), and (b) |E| (Δx = –20 nm) distributions at the indicated resonances.
图 4 固定φ = 0°和Δx = 0 nm, Δy在–20—20 nm变化时二聚体散射谱的变化
Figure 4. Scattering spectra of the dimer with φ = 0° and Δx = 0 nm for different values of Δy = –20–20 nm.
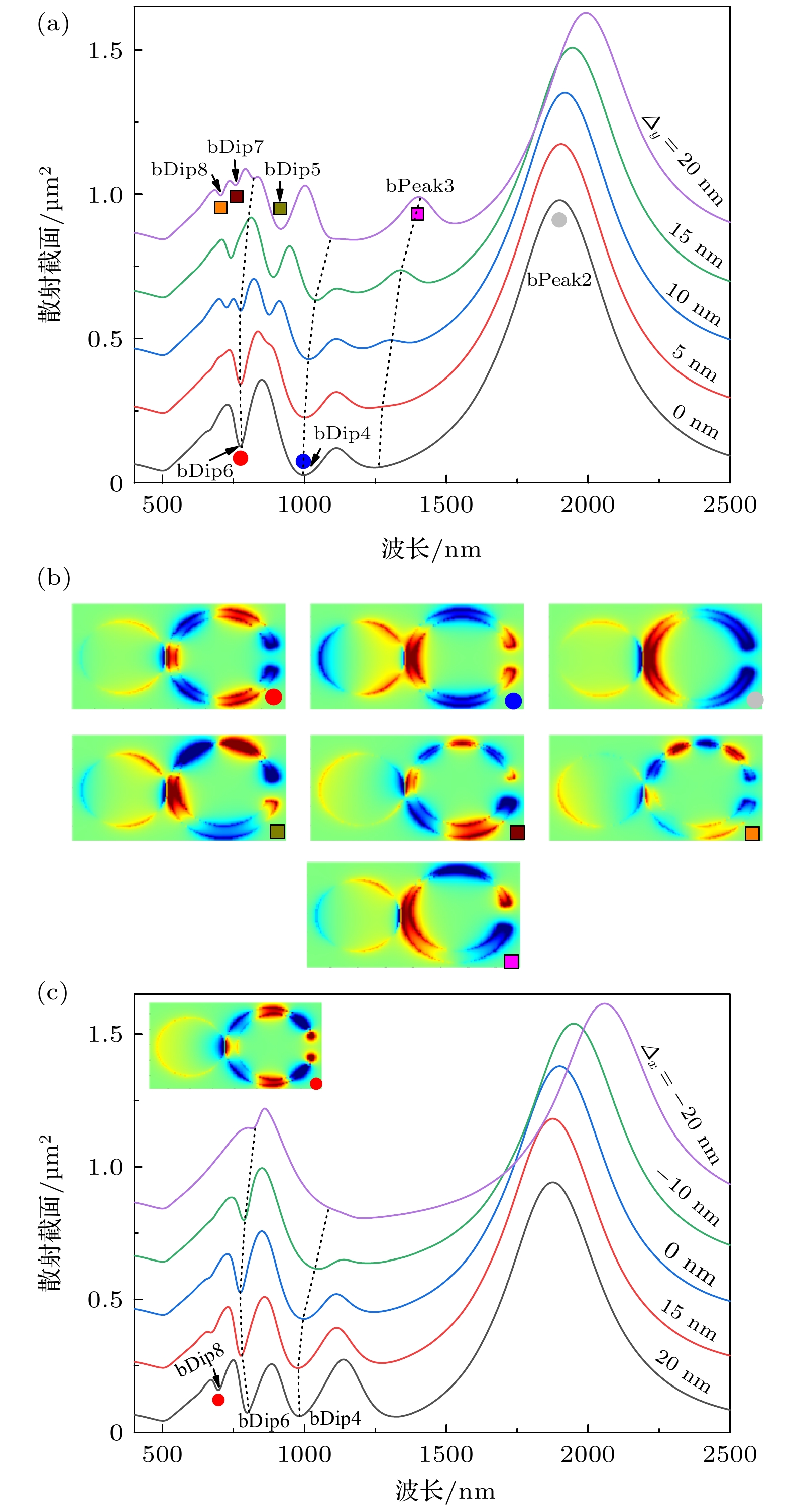
图 5 固定φ = 90°, 二聚体的散射谱随(a) Δx = 0 nm, Δy在0—20 nm, (c) Δy = 0 nm, Δ
x在–20—20 nm改变时的变化; (b)在图(a)散射谱Δy = 0 nm或20 nm中指定频率处的二聚体表面电场分布 Figure 5. Scattering spectra of the dimer with φ = 90° for different values of (a) Δy from 0 nm to 20 nm with Δx = 0 nm, and (c) Δx from –20 nm to 20 nm with Δy = 0 nm; (b) calculated electric field Ez distributions at the indicated resonances shown in Fig.(a) at Δy = 0 nm and 20 nm.
图 6 当φ = –90°时二聚体的散射谱随Δx, Δy的变化 (a) Δx = 0 nm, Δy从0 nm到20 nm; (b) Δy = 0 nm, Δx从–20 nm到20 nm. 插图为Δx = 0 nm, Δy = 20 nm时的散射谱中指定频率处的二聚体表面电场分布
Figure 6. Scattering spectra of the dimer with φ = 90° for different values of (a) Δy from 0 nm to 20 nm with Δx = 0 nm, and (b) Δx from –20 nm to 20 nm with Δy = 0 nm. Inset show the calculated electric field Ez distributions at the indicated resonances with Δx = 0 nm and Δy = 20 nm.
-
[1] Maier S A 2007 Plasmonics: Fundamentals and Applications (New York: Springer Science & Business Media) pp107−138
[2] Zheng C, Jia T, Zhao H, Xia Y, Zhang S, Feng D, Sun Z 2018 RSC Adv. 8 3381
Google Scholar
[3] Ling Y, Huang L, Hong W, Liu T, Luan J, Liu W, Lai J, Li H 2018 Nanoscale 10 19517
Google Scholar
[4] Li G, Hu H, Wu L 2019 Phys. Chem. Chem. Phys. 21 252
Google Scholar
[5] Zhang Y, Zhen Y, Neumann O, Day J K, Nordlander P, Halas N J 2014 Nat. Commun. 5 4424
Google Scholar
[6] Zhi L, Liu W, Li Z, Hua C, Chen S, Tian J 2017 Opt. Lett. 42 3117
Google Scholar
[7] Wan W, Zheng W, Chen Y, Liu Z 2014 Nanoscale 6 9093
Google Scholar
[8] Biswas S, Duan J, Nepal D, Park K, Pachter R, Vaia R A 2013 Nano Lett. 13 6287
Google Scholar
[9] Liu S, Yue P, Zhang S, Wang M, Dai H, Chen Y, Nie Z, Cui Y, Han J, Duan H 2020 Adv. Opt. Mater. 8 1901109
Google Scholar
[10] Hu H, Zhang F, Li G, Chen J, Li Q, Wu L 2018 Photon. Res. 6 204
Google Scholar
[11] Dutta A, Alam K, Nuutinen T, Hulkko E, Vartiainen E M 2019 Opt. Express 27 30031
Google Scholar
[12] Yang L, Li P, Liu H, Tang X, Liu J 2015 Chem. Soc. Rev. 44 2837
Google Scholar
[13] Hao F, Larsson E M, Ali T A, Sutherland D S, Nordlander P 2008 Chem. Phys. Lett. 458 262
Google Scholar
[14] Koya A N, Ji B, Hao Z, Lin J 2016 J. Opt. 18 055007
Google Scholar
[15] Dalius S, Gediminas L, Andrius V, Ilvinas K, Gintaras V 2017 Opt. Express 25 25113
Google Scholar
[16] Rockstuhl C, Lederer F, Etrich C, Zentgraf T, Giessen H 2006 Opt. Express 14 8827
Google Scholar
[17] Liu S D, Yue P, Zhu M Q, Wen J, Lei D 2019 Opt. Express 27 26377
Google Scholar
[18] Li Y, Huo Y, Zhang Y and Zhang Z 2017 Plasmonics 12 1613
Google Scholar
[19] Halas N J, Lal S, Chang W S, Nordlander P 2011 Chem. Rev. 111 3913
Google Scholar
[20] Wang W, Li Y, Peng J, Chen Z, Qian J, Chen J, Xu J, Sun Q 2014 J. Opt. 16 035002
Google Scholar
[21] Zhang Q, Wen X, Li G, Ruan Q, Wang J, Xiong Q 2013 ACS Nano 7 11071
Google Scholar
[22] Sun B, Zhao L, Wang C, Yi X, Liu Z, Wang G, Li J 2014 J. Phys. Chem. C 118 25124
Google Scholar
[23] Wang L, Liu Z, Yi X, Zhang Y, Li H, Li J, Wang G 2016 J. Appl. Phys. 119 173106
Google Scholar
[24] Cui J, Ji B, Song X, Lin J 2019 Plasmonics 14 41
Google Scholar
[25] Zhang X, Liu F, Yan X, Liang L, Wei D 2020 J. Opt. 22 115003
Google Scholar
[26] Sheridan A K, Clark A W, Glidle A, Cooper J M, Cumming D R S 2007 Appl. Phys. Lett. 90 143105
Google Scholar
[27] Taflove A, Hagness S 2000 Computational Electrodynamics: The Finite-Difference Time-Domain Method (Vol. 2) (Boston: Artech House) pp75−85
[28] Johnson P B, Christy R W 1972 Phys. Rev. B 6 4370
Google Scholar
[29] Lovera A, Gallinet B, Nordlander P, Martin O 2013 ACS Nano 7 4527
Google Scholar
[30] Zhang Y, Jia T Q, Zhang H M, Xu Z Z 2012 Opt. Lett. 37 4919
Google Scholar
[31] Zhang L, Dong Z, Wang Y, Liu Y, Zhang S, Yang J K W, Qiu C 2015 Nanoscale 7 12018
Google Scholar
[32] Zong X, Li L, Liu Y 2019 Opt. Mater. Express 9 870
Google Scholar
[33] Hu Y, Noelck S J, Drezek R A 2010 ACS Nano 4 1521
Google Scholar
[34] Xiao F, Zhang J, Yu W, Zhu W, Zhao J 2020 Opt. Express 28 3000
Google Scholar
[35] Wang N, Zeisberger M, Huebner U, Giannini V, Schmidt M A 2019 Sci. Rep. 9 2873
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4432
- PDF Downloads: 76
- Cited By: 0
