-
Based on the thermal blooming effect theory for high-energy laser propagating in atmosphere, the vector model concept of thermal distortion parameter
$ {{\boldsymbol{N}}_{\text{D}}} $ is put forward. Based on the vector model concept of thermal distortion parameter and the laser system simulation software EasyLaser, the scaling law between the centroid offset of laser beam farfield and the vector thermal distortion parameter is simulated and analyzed. The simulation results indicate that the centroid offset quantity is in direct proportion to the modulus of vector thermal distortion parameter$ {{\boldsymbol{N}}_{\text{D}}} $ , and the centroid offset direction is opposite to the direction of vector thermal distortion parameter$ {{\boldsymbol{N}}_{\text{D}}} $ . Based on the scaling law, by real-time measuring the atmosphreic parameters on laser beam propagation path, the beam deviation of laser system can be conveniently estimated in practical application.[1] 苏毅, 万敏 2004 高能激光系统 (北京: 国防工业出版社) 第143页
Su Y, Wan M 2004 High Energy Laser System (Beijing: National Defense Industrial Press) p143 (in Chinese)
[2] 饶瑞中 2012 现代大气光学 (北京: 科学出版社) 第482页
Rao R Z 2012 Modern Atmospheric Optics (Beijing: Science Press) p482 (in Chinese)
[3] Gebhardt F G 1990 Proc. SPIE 1221 2
Google Scholar
[4] Zhang F Z, Li Y K 2004 Proc. SPIE 5832 25
[5] 黄印博, 王英俭, 饶瑞中, 龚知本 2002 光学学报 22 1461
Google Scholar
Huang Y B, Wang Y J, Rao R Z, Gong Z B 2002 Acta Opt. Sin. 22 1461
Google Scholar
[6] 黄印博, 王英俭 2006 55 6715
Google Scholar
Huang Y B, Wang Y J 2006 Acta Phys. Sin. 55 6715
Google Scholar
[7] 乔春红, 范承玉, 王英俭 2008 强激光与粒子束 20 1811
Qiao C H, Fan C Y, Wang Y J 2008 High Power Laser and Particle Beams 20 1811
[8] 张鹏飞, 范承玉, 乔春红 2012 中国激光 39 0213002
Google Scholar
Zhang P F, Fan C Y, Qiao C H 2012 Chin. J. Lasers 39 0213002
Google Scholar
[9] 张鹏飞, 乔春红, 冯晓星, 李南, 黄童, 范承玉, 王英俭 2017 光学学报 37 1001001
Google Scholar
Zhang P F, Qian C H, Feng X X, Li N, Huang T, Fan C Y, Wang Y J 2017 Acta Opt. Sin. 37 1001001
Google Scholar
[10] 吴书云, 李新阳, 罗曦 2018 光电工程 45 170620
Wu S Y, Li X Y, Luo X 2018 Opto-Electronic Eng. 45 170620
[11] 陈小威, 李学斌, 魏合理, 戴聪明, 罗涛, 朱文越, 翁宁泉 2018 光学学报 38 1001001
Google Scholar
Chen X W, Li X B, Wei H L, Dei C M, Luo T, Zhu W Y, Weng N Q 2018 Acta Opt. Sin. 38 1001001
Google Scholar
[12] 李晓庆, 曹建勇, 丁洲林, 季小玲 2019 光学学报 39 0126020
Google Scholar
Li X Q, Cao J Y, Ding Z L, Ji X L 2019 Acta Opt. Sin. 39 0126020
Google Scholar
[13] 闫伟, 陈志华, 杜太焦, 关奇 2016 红外与激光工程 45 1032001
Google Scholar
Yan W, Chen Z H, Du T J, Guan Q 2016 Infrared Laser Eng. 45 1032001
Google Scholar
[14] Bradley L C, Herrmann J 1974 Appl. Opt. 13 331
Google Scholar
[15] 谢晓钢, 张建柱, 岳玉芳 2013 强激光与粒子束 25 2536
Google Scholar
Xie X G, Zhang J Z, Yue Y F 2013 High Power Laser and Particle Beams 25 2536
Google Scholar
[16] 王英俭, 范承玉, 魏合理 2015 激光在大气和海水中传输及应用 (北京: 国防工业出版社) 第157页
Wang Y J, Fan C Y, Wei H L 2015 Laser Beam Propagation and Applications through the Atmosphere and Sea Water (Beijing: National Defense Industrial Press) p157 (in Chinese)
-
图 1 标量叠加与矢量叠加差异示意图
Figure 1. Sketch map of difference between vector add and scalar add.
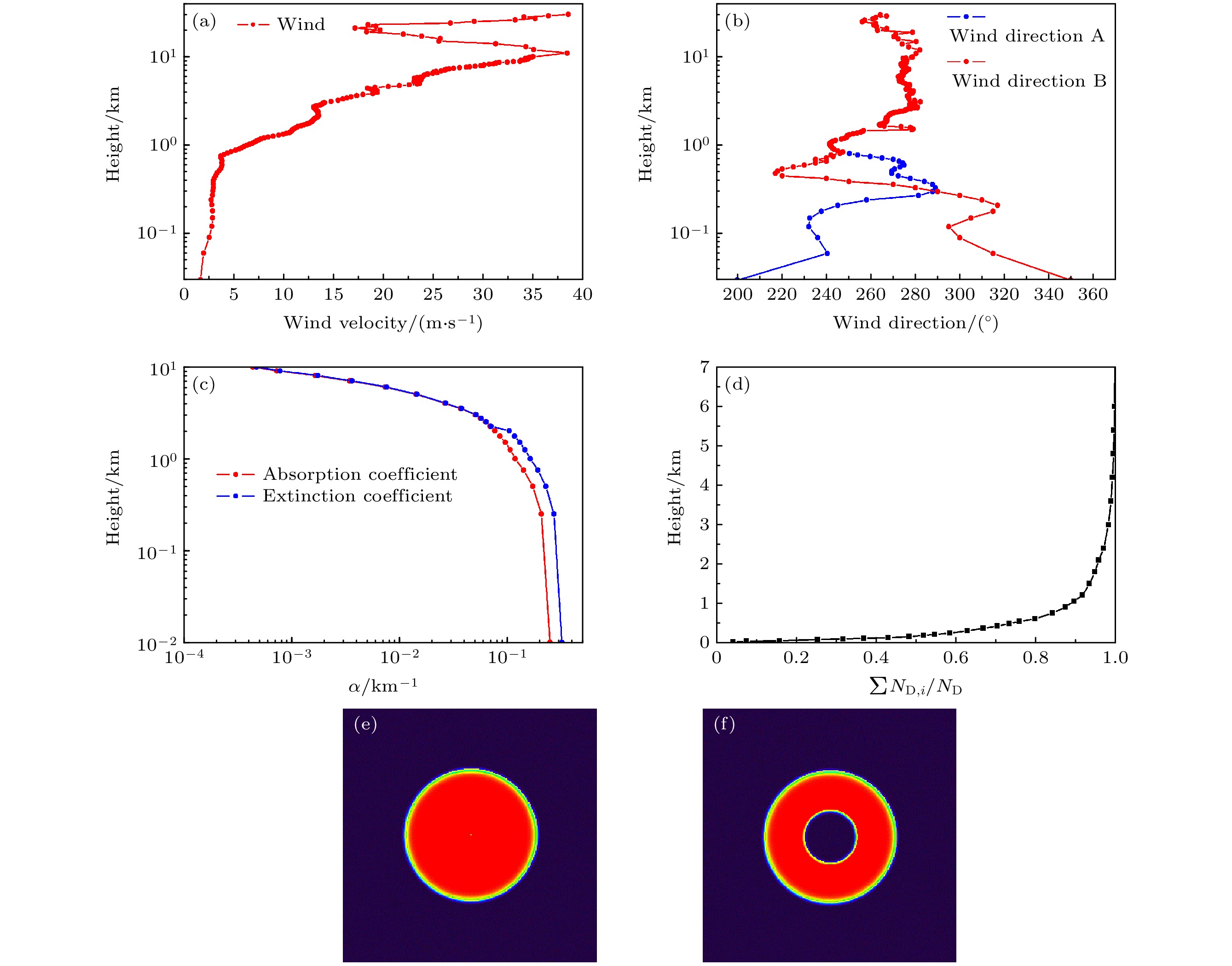
图 2 仿真参数 (a) 自然风速廓线; (b) 自然风向廓线; (c) 大气吸收与消光廓线; (d) 热晕效应分布; (e) 实心平台光束; (f) 遮拦比
$ \varepsilon = 0.4 $ 的环形光束Figure 2. Simulation parameter: (a) Wind velocity outline; (b) wind direction outline; (c) atmospheric absorption and extinction outline; (d) thermal blooming effect distribution; (e) flat circular beam; (f) hollow circle beam of obstructed ratio
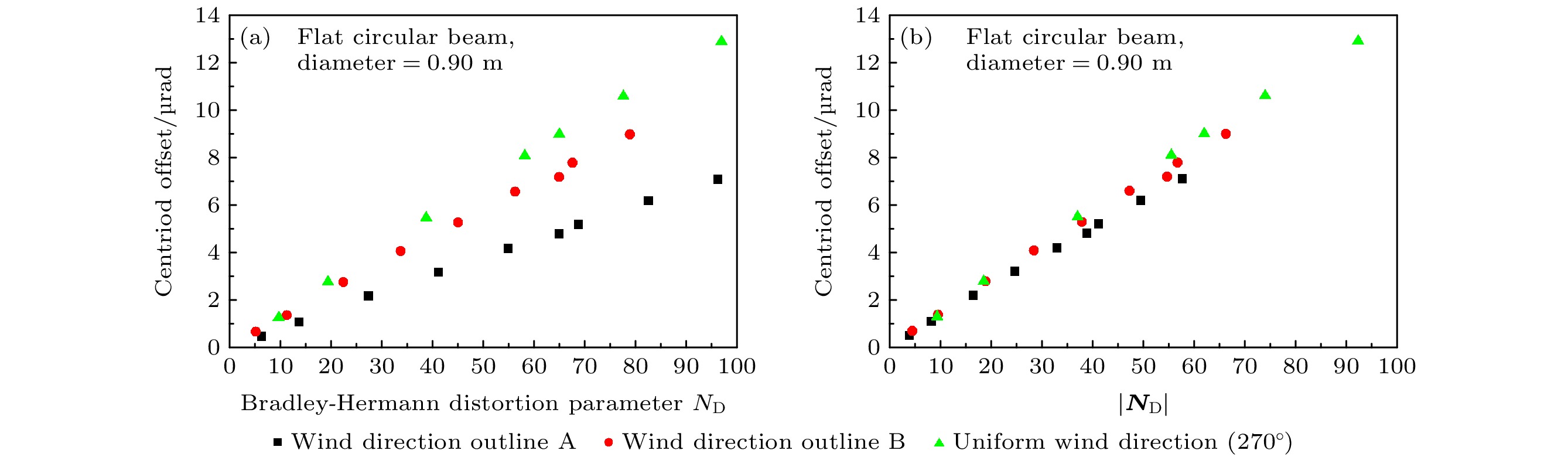
$ \varepsilon = 0.4 $ .
图 3 热畸变参数标量值相同但矢量分布不同时远场光斑典型分布 (a) 风向廓线A; (b) 风向廓线B; (c) 同风向270°
Figure 3. Typical far field distribution of the same thermal blooming distortion parameter
$ {N_{\text{D}}} $ but different$ {{\boldsymbol{N}}_{\text{D}}} $ : (a) Wind direction outline A; (b) wind direction outline B; (c) uniform wind direction (270º).
图 4 远场质心偏移与热畸变参数的关系 (a) 标量热畸变参数模型; (b) 矢量热畸变参数模型
Figure 4. Relation between centriod offset of flat circular beam and thermal blooming distortion parameter: (a) Thermal blooming distortion parameter scalar model; (b) thermal blooming distortion parameter vector model.
图 5 自然风向廓线A条件下平台光束光束偏折与矢量热畸变参数
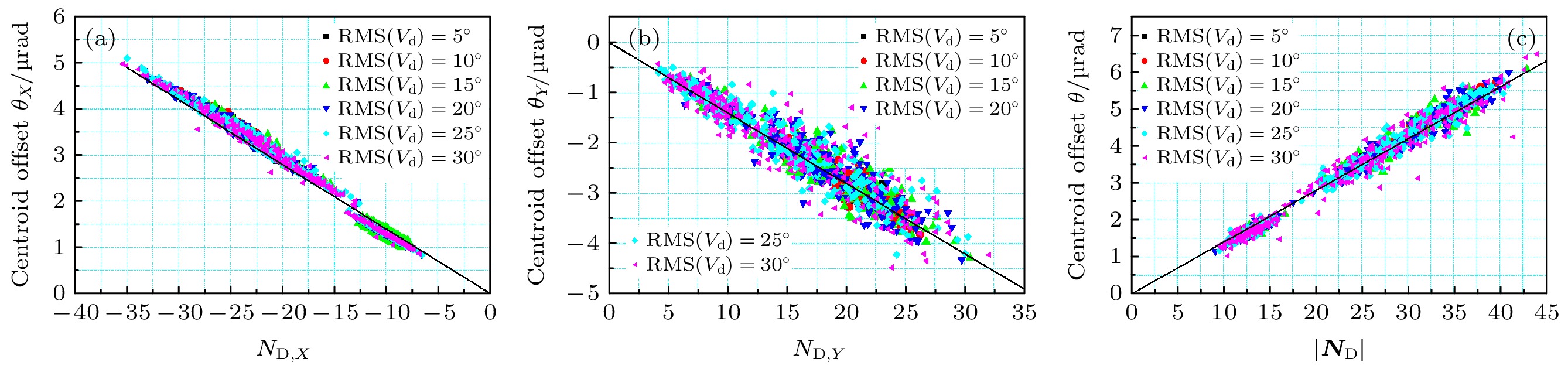
$ {{\boldsymbol{N}}_{\text{D}}} $ 的变化规律 (a) X方向质心偏移; (b) Y方向质心偏移; (c) 质心总偏移Figure 5. Relation between centriod offset of flat circular beam and thermal blooming parameter
$ {{\boldsymbol{N}}_{\text{D}}} $ while wind direction outline A is used: (a) Centriod offset in X axis; (b) centriod offset in Y axis; (c) all centriod offset.
图 6 自然风向廓线B条件下平台光束光束偏折与矢量热畸变参数
$ {{\boldsymbol{N}}_{\text{D}}} $ 的变化规律 (a) X方向质心偏移; (b) Y方向质心偏移; (c) 质心总偏移Figure 6. Relation between centriod offset of flat circular beam and thermal blooming parameter
$ {{\boldsymbol{N}}_{\text{D}}} $ while wind direction outline B is used: (a) Centriod offset in X axis; (b) centriod offset in Y axis; (c) all centriod offset.
图 7 自然风向廓线A条件下环形光束光束偏折与矢量热畸变参数
$ {{\boldsymbol{N}}_{\text{D}}} $ 的变化规律 (a) X方向质心偏移; (b) Y方向质心偏移; (c) 质心总偏移Figure 7. Relation between centriod offset of hollow circle beam and thermal blooming parameter
$ {{\boldsymbol{N}}_{\text{D}}} $ while wind direction outline A is used: (a) Centriod offset in X axis; (b) centriod offset in Y axis; (c) all centriod offset.
图 8 自然风向廓线B条件下环形光束光束偏折与矢量热畸变参数
$ {{\boldsymbol{N}}_{\text{D}}} $ 的变化规律 (a) X方向质心偏移; (b) Y方向质心偏移; (c) 质心总偏移Figure 8. Relation between centriod offset of hollow circle beam and thermal blooming parameter
$ {{\boldsymbol{N}}_{\text{D}}} $ while wind direction outline B is used: (a) Centriod offset in X axis; (b) centriod offset in Y axis; (c) all centriod offset. -
[1] 苏毅, 万敏 2004 高能激光系统 (北京: 国防工业出版社) 第143页
Su Y, Wan M 2004 High Energy Laser System (Beijing: National Defense Industrial Press) p143 (in Chinese)
[2] 饶瑞中 2012 现代大气光学 (北京: 科学出版社) 第482页
Rao R Z 2012 Modern Atmospheric Optics (Beijing: Science Press) p482 (in Chinese)
[3] Gebhardt F G 1990 Proc. SPIE 1221 2
Google Scholar
[4] Zhang F Z, Li Y K 2004 Proc. SPIE 5832 25
[5] 黄印博, 王英俭, 饶瑞中, 龚知本 2002 光学学报 22 1461
Google Scholar
Huang Y B, Wang Y J, Rao R Z, Gong Z B 2002 Acta Opt. Sin. 22 1461
Google Scholar
[6] 黄印博, 王英俭 2006 55 6715
Google Scholar
Huang Y B, Wang Y J 2006 Acta Phys. Sin. 55 6715
Google Scholar
[7] 乔春红, 范承玉, 王英俭 2008 强激光与粒子束 20 1811
Qiao C H, Fan C Y, Wang Y J 2008 High Power Laser and Particle Beams 20 1811
[8] 张鹏飞, 范承玉, 乔春红 2012 中国激光 39 0213002
Google Scholar
Zhang P F, Fan C Y, Qiao C H 2012 Chin. J. Lasers 39 0213002
Google Scholar
[9] 张鹏飞, 乔春红, 冯晓星, 李南, 黄童, 范承玉, 王英俭 2017 光学学报 37 1001001
Google Scholar
Zhang P F, Qian C H, Feng X X, Li N, Huang T, Fan C Y, Wang Y J 2017 Acta Opt. Sin. 37 1001001
Google Scholar
[10] 吴书云, 李新阳, 罗曦 2018 光电工程 45 170620
Wu S Y, Li X Y, Luo X 2018 Opto-Electronic Eng. 45 170620
[11] 陈小威, 李学斌, 魏合理, 戴聪明, 罗涛, 朱文越, 翁宁泉 2018 光学学报 38 1001001
Google Scholar
Chen X W, Li X B, Wei H L, Dei C M, Luo T, Zhu W Y, Weng N Q 2018 Acta Opt. Sin. 38 1001001
Google Scholar
[12] 李晓庆, 曹建勇, 丁洲林, 季小玲 2019 光学学报 39 0126020
Google Scholar
Li X Q, Cao J Y, Ding Z L, Ji X L 2019 Acta Opt. Sin. 39 0126020
Google Scholar
[13] 闫伟, 陈志华, 杜太焦, 关奇 2016 红外与激光工程 45 1032001
Google Scholar
Yan W, Chen Z H, Du T J, Guan Q 2016 Infrared Laser Eng. 45 1032001
Google Scholar
[14] Bradley L C, Herrmann J 1974 Appl. Opt. 13 331
Google Scholar
[15] 谢晓钢, 张建柱, 岳玉芳 2013 强激光与粒子束 25 2536
Google Scholar
Xie X G, Zhang J Z, Yue Y F 2013 High Power Laser and Particle Beams 25 2536
Google Scholar
[16] 王英俭, 范承玉, 魏合理 2015 激光在大气和海水中传输及应用 (北京: 国防工业出版社) 第157页
Wang Y J, Fan C Y, Wei H L 2015 Laser Beam Propagation and Applications through the Atmosphere and Sea Water (Beijing: National Defense Industrial Press) p157 (in Chinese)
DownLoad:
Catalog
Metrics
- Abstract views: 4372
- PDF Downloads: 69
- Cited By: 0
