First-principles study of e interface interaction and photoelectric properties of the solar cell heterojunction CdS/CdMnTe

Luan Li-Jun; He Yi; Wang Tao; Liu Zong-Wen

doi:10.7498/aps.70.20210268

Abstract

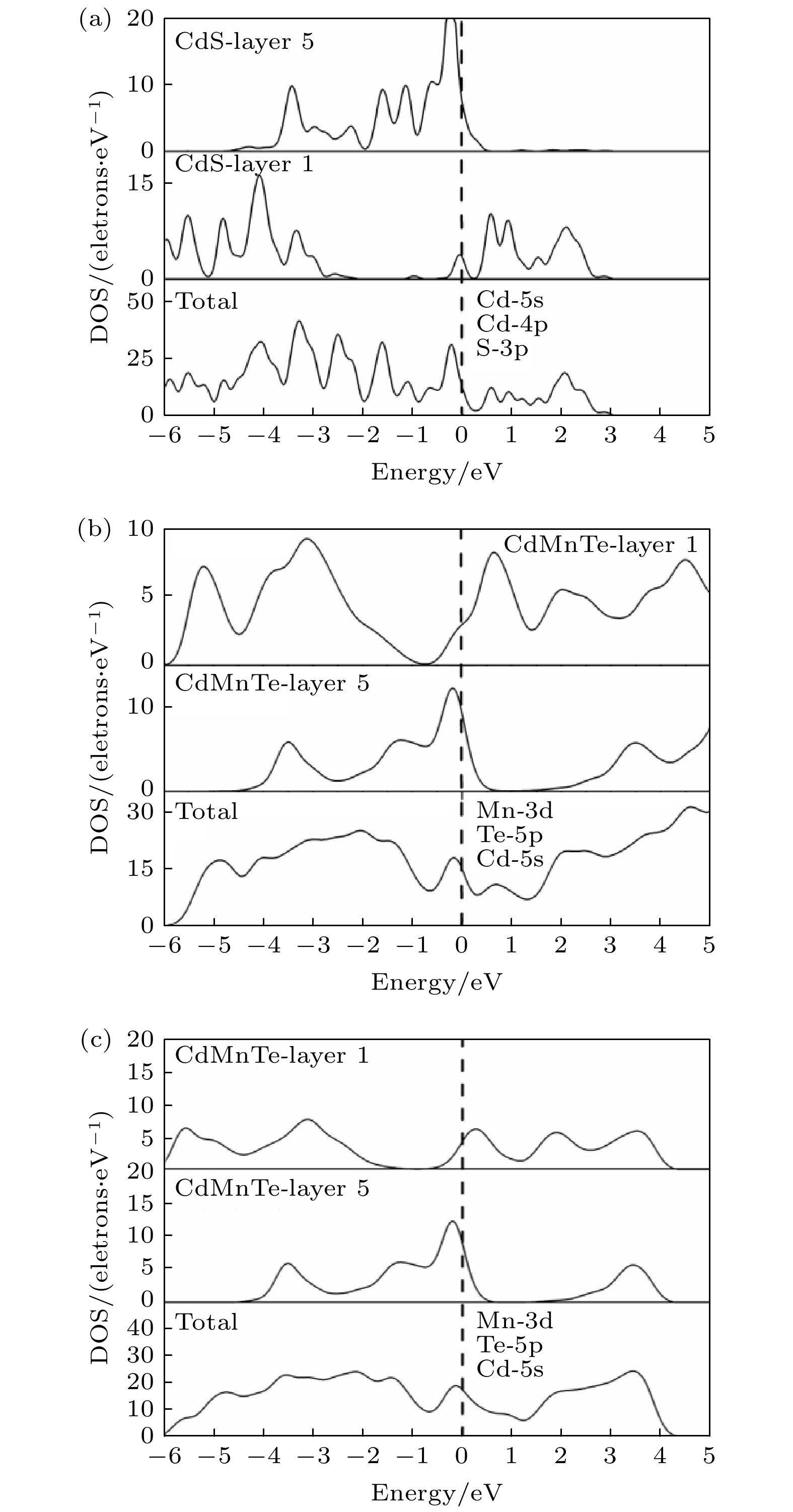
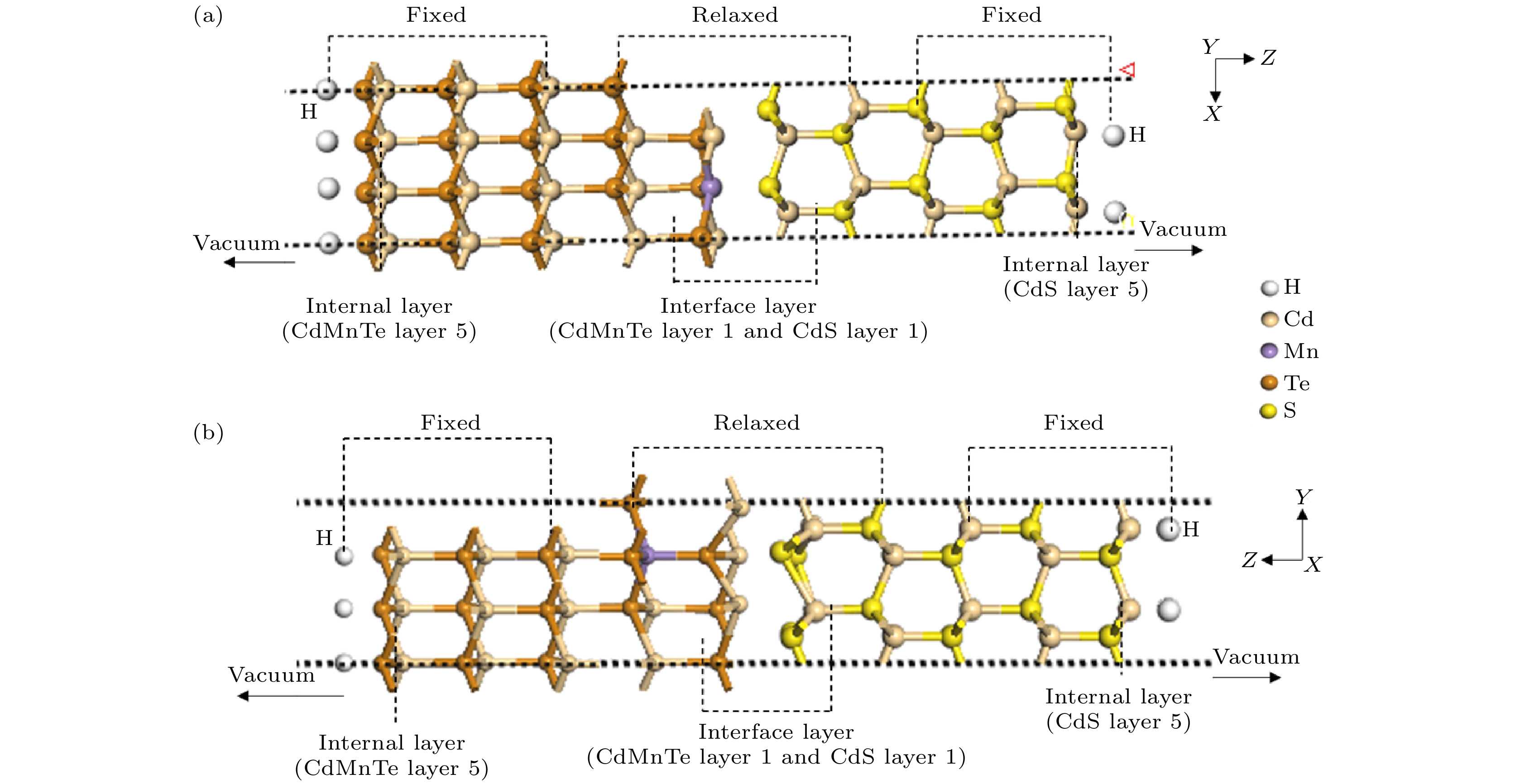
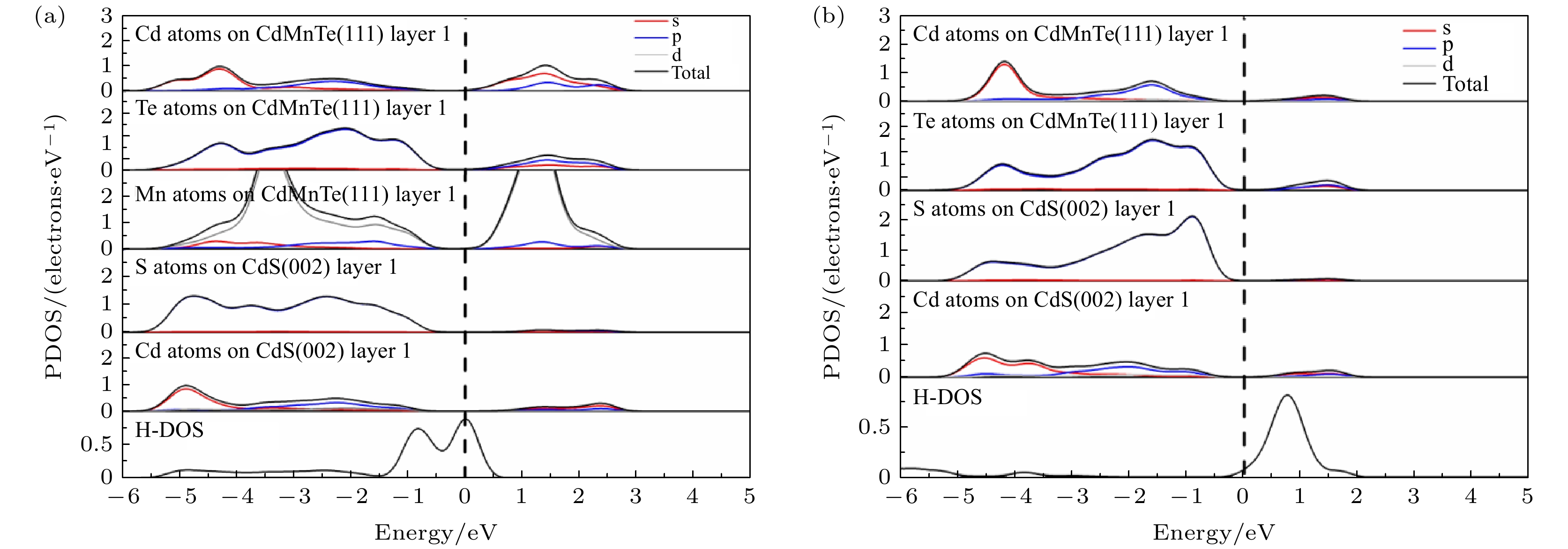
CdS/CdMnTe heterojunction is the core of photoelectric conversion of CdMnTe film solar cells, whose interface properties have an important influence on the cell efficiency. In this study, the first-principles calculation method based on density functional theory is used to build the surface model for each of the CdS (002) and the CdMnTe (111) and the model of CdS/CdMnTe heterojunction with Mn atoms occupying different positions, and to analyze their electronic properties and optical properties. The results show that the lattice mismatch of the CdS/CdMnTe heterojunction is about 3.5%, the atomic positions and bond lengths of the interface change slightly after relaxation. The density of states shows that there is no interface state near the Fermi level in CdS/CdMnTe interface. Besides, the atoms at CdS/CdMnTe interface are hybridized, which can enhance the interface bonding. The differential charge density analyses indicate that the charge transfer mainly occurs at the interface, and electrons transfer from CdMnTe to CdS. The optical analysis shows that CdS/CdMnTe heterojunction mainly absorbs ultraviolet light, and the absorption coefficient can reach 10⁵ cm^–1. However, the optical properties of heterojunctions with different Mn atom positions are slightly different. In a range of 200–250 nm, the absorption coefficient of the heterojunction with Mn atom in the middle layer is larger, but in a range of 250–900 nm, the absorption peak of the heterojunction with Mn atom in the interface layer is higher. The results in this paper can provide some references for improving the photoelectric conversion efficiency of stacked solar cells through the reasonable construction of the heterojunction model and the analysis of the interface photoelectric performance, which is beneficial to the experimental research of multi-band gap heterojunction.

Keywords:

Authors and contacts

1.
School of Materials Science and Engineering, Chang’an University, Xi’an 710064, China
2.
State Key Laboratory of Solidification Technology, Northwestern Polytechnical University, Xi’an 710072, China
3.
Australian Centre for Microscopy & Microanalysis, School of Chemical and Biomolecule Engineering, University of Sydney NSW 2006, Australia

Corresponding author: Luan Li-Jun, nmllj050@chd.edu.cn

Funds: Project supported by the Major Project of International Scientific and Technological Cooperation Plan of Shaanxi Province, China (Grant No. 2020KWZ-008)

FullText HTML : translate this paragraph

References

[1]	朱建国, 孙小松, 李卫 2007 电子与光电子材料 (北京: 国防工业出版社) 第125页 Zhu J G, Sun X S, Li W 2007 Electronic and Optoelectronic Materials (Beijing: Defense Industry Press) p125 (in chinese)
[2]	Miles R W, Hynes K M, Forbes I 2005 Prog. Cryst. Growth Charact. Mater. 51 1 Google Scholar
[3]	Mishima T, Taguchi M, Sakata H, Maruyama E 2011 Sol. Energy Mater. Sol. Cells 95 18 Google Scholar
[4]	Jordan D, Nagle J P 1994 Prog. Photovoltaics 2 171 Google Scholar
[5]	Cheng Y J, Yang S H, Hsu C S 2009 Chem. Rev. 109 5868 Google Scholar
[6]	Mora-Seró I, Bisquert J 2010 J. Phys. Chem. Lett. 1 3046 Google Scholar
[7]	Zhao J 2004 Sol. Energy Mater. Sol. Cells 82 53 Google Scholar
[8]	Cojocaru-Miredin O, Choi P, Wuerz R, Raabe D 2011 Appl. Phys. Lett. 98 73 Google Scholar
[9]	Yang L, Xuan Y, Tan J 2011 Opt. Express 19 A1165 Google Scholar
[10]	Chopra K L, Paulson P D, Dutta V 2004 Prog. Photovoltaics 12 69 Google Scholar
[11]	Zhang K, Guo H 2017 J. Mater. Sci. Mater. Electron. 28 17044 Google Scholar
[12]	Wu X 2004 Sol. Energy 77 803 Google Scholar
[13]	Chander S, De A K, Dhaka M S 2018 Sol. Energy 174 757 Google Scholar
[14]	Chander S, Dhaka M S 2016 Phys. E 84 112 Google Scholar
[15]	Lee S H, Gupta A, Wang S, Compaan A D, McCandless B E 2005 Sol. Energy Mater. Sol. Cells 86 551 Google Scholar
[16]	Chander S, Dhaka M S 2019 Sol. Energy 183 544 Google Scholar
[17]	Rohatgi A, Ringel S A, Welch J, Meeks E, Pollard K, Erbil A, Summers C J, Meyers P V 1988 Sol. Energy 24 185
[18]	Luan L J, Gao L, Lv H H, Yu P F, Wang T, He Y, Zheng D 2020 Sci. Rep. 10 1 Google Scholar
[19]	Wang S L, Lee S H, Gupta A 2004 MRS Proc. 836 L5.39 Google Scholar
[20]	侯泽荣, 万磊, 白治中, 王德亮 2010 中国科学技术大学学报 40 718 Google Scholar Hou Z R, W L, Bai Z Z, Wang D L 2010 J. Univ. Sci. Tech. Chin. 40 718 Google Scholar
[21]	Olusola O I, Madugu M L, Ojo A A, Dharmadasa I M 2020 J. Mater. Sci. Mater. Electron. 31 22151 Google Scholar
[22]	Shafaay B A 2014 J. Chem., Biol. Phys. Sci. 4 1
[23]	Gueddim A, Madjet M E, Zerroug S, Bouarissa N 2016 Opt. Quantum Electron. 48 551 Google Scholar
[24]	Llchuk H, Zmiiovska E, Petrus R, Semkive I, Lopatynskyi I, Kashuba 2020 J. Nano-Electron. Phys. 12 01027 Google Scholar
[25]	Cao A, Tan T T, Zhang H, Du Y, Sun Y, Zha G 2018 Phys. B 545 323 Google Scholar
[26]	Merad A, Kanoun M, Merad G, Cibert J, Aourag H 2005 Mater. Chem. Phys. 92 333 Google Scholar
[27]	Liu H X, Tang F L, Xue H T, Zhang Y, Cheng Y W, Feng Y D 2016 Chin. Phys. B 25 123101 Google Scholar
[28]	Shenoy S, Tarafder K 2020 J. Phys. Condens. Matter 32 275501 Google Scholar
[29]	Kresse G, Joubert D 1999 Phys. Rev. B 59 1758 Google Scholar
[30]	Shi L B, Xu C Y, Yuan H K 2011 Phys. B 406 3187 Google Scholar
[31]	Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865 Google Scholar
[32]	Butler K T, Frost J M, Walsh A 2015 Mater. Horiz. 2 228 Google Scholar
[33]	Wang J S, Tong S C, Tsai Y H, Tsai W J, Yang C S, Chang Y H, Shen J L 2015 J. Alloys Compd. 646 129 Google Scholar
[34]	Kumar S G, Rao K S R K 2014 Energy Environ. Sci. 7 45 Google Scholar
[35]	Cheng Y W, Tang F L, Xue H T, Liu H X, Gao B, Feng Y D 2016 Mater. Sci. Semicond. Process. 45 9 Google Scholar
[36]	Momma K, Izumi F 2008 J. Appl. Crystallogr. 41 653 Google Scholar
[37]	Guo Y, Xue Y, Geng C, Li C, Li X, Niu Y 2019 J. Phys. Chem. C 123 16075 Google Scholar
[38]	Yin W J, Shi T, Yan Y 2015 J. Phys. Chem. C 119 5253 Google Scholar
[39]	Scharber M C, Mühlbacher D, Koppe M, Denk P, Waldauf C, Heeger A J, Brabec C J 2006 Adv. Mater. 18 789 Google Scholar
[40]	Sharma S, Devi N, Verma U P, RajaRam P 2011 Phys. B 406 4547 Google Scholar
[41]	Dadsetani M, Pourghazi A 2006 Phys. Rev. B 73 195102 Google Scholar
[42]	Gajdos M, Hummer K, Kresse G, Furthmueller J, Bechstedt F 2006 Phys. Rev. B 73 045112 Google Scholar

Cited By

图 1 表面模型的建立　(a) CdS (002)表面模型; (b) CdMnTe (111)-A₁表面模型; (c) CdMnTe (111)-B₁表面模型

Figure 1. Building of the surface models: (a) Model of the CdS (002) surface; (b) A₁ surface model of CdMnTe (111); (c) B₁ surface model of CdMnTe (111).

DownLoad: Full-Size Img PowerPoint

图 2 弛豫后的表面原子位置和层间距离的变化　(a) CdS (002)表面模型; (b) CdMnTe (111)-A₁表面模型; (c) CdMnTe (111)-B₁表面模型

Figure 2. Variations of atomic positions and interlayer spacing after relaxation: (a) Model of the CdS (002) surface; (b) A₁ surface model of CdMnTe (111); (c) B₁ surface model of CdMnTe (111).

DownLoad: Full-Size Img PowerPoint

图 3 表面模型总态密度和第1层及第5层局域态密度　(a) CdS (002); (b) CdMnTe (111)-A₁; (c) CdMnTe (111)-B₁

Figure 3. Total density of states and the local density of states of the first layer and the fifth layer: (a) CdS (002); (b) A₁ of CdMnTe (111); (c) B₁ of CdMnTe (111).

DownLoad: Full-Size Img PowerPoint

图 4 CdS/CdMnTe异质结模型　(a) Mn原子位于界面层的异质结模型(模型A₂); (b) Mn原子位于中间层的异质结模型(模型B₂)

Figure 4. CdS/CdMnTe heterojunction models: (a) Mn atom being in the interface layer (model A₂); (b) Mn atom in the internal layer (model B₂).

DownLoad: Full-Size Img PowerPoint

图 5 模型A₂和B₂的总能量随界面间距的变化

Figure 5. Variation of total energy dependent on the interface distance of the models A₂ and B₂, respectively.

DownLoad: Full-Size Img PowerPoint

图 6 弛豫前后界面及其附近原子键长的变化　(a)弛豫前的模型A₂; (b)弛豫后的模型A₂; (c)弛豫前的模型B₂; (d)弛豫后的模型B₂

Figure 6. Bond lengths in/near of the interface before and after relaxation: (a) Before relaxation of A₂; (b) after relaxation of A₂; (c) before relaxation of B₂; (d) after relaxation of B₂.

DownLoad: Full-Size Img PowerPoint

图 7 CdS/CdMnTe异质结总态密度与局域态密度　(a)模型A₂; (b)模型B₂

Figure 7. Total density of states and local density of states of the CdS/CdMnTe heterojunction: (a) Model A₂; (b) model B₂.

DownLoad: Full-Size Img PowerPoint

图 8 CdS/CdMnTe异质结界面处原子的分态密度　(a)模型A₂; (b)模型B₂

Figure 8. Part density of states of different atoms in the CdS/CdMnTe interface: (a) Model A₂; (b) model B₂.

DownLoad: Full-Size Img PowerPoint

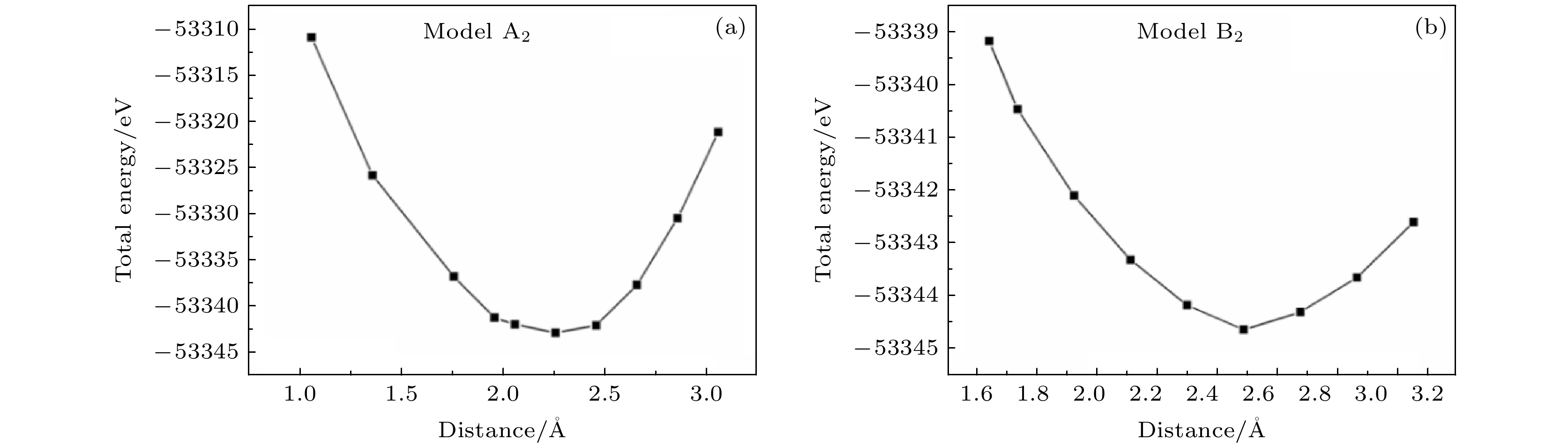
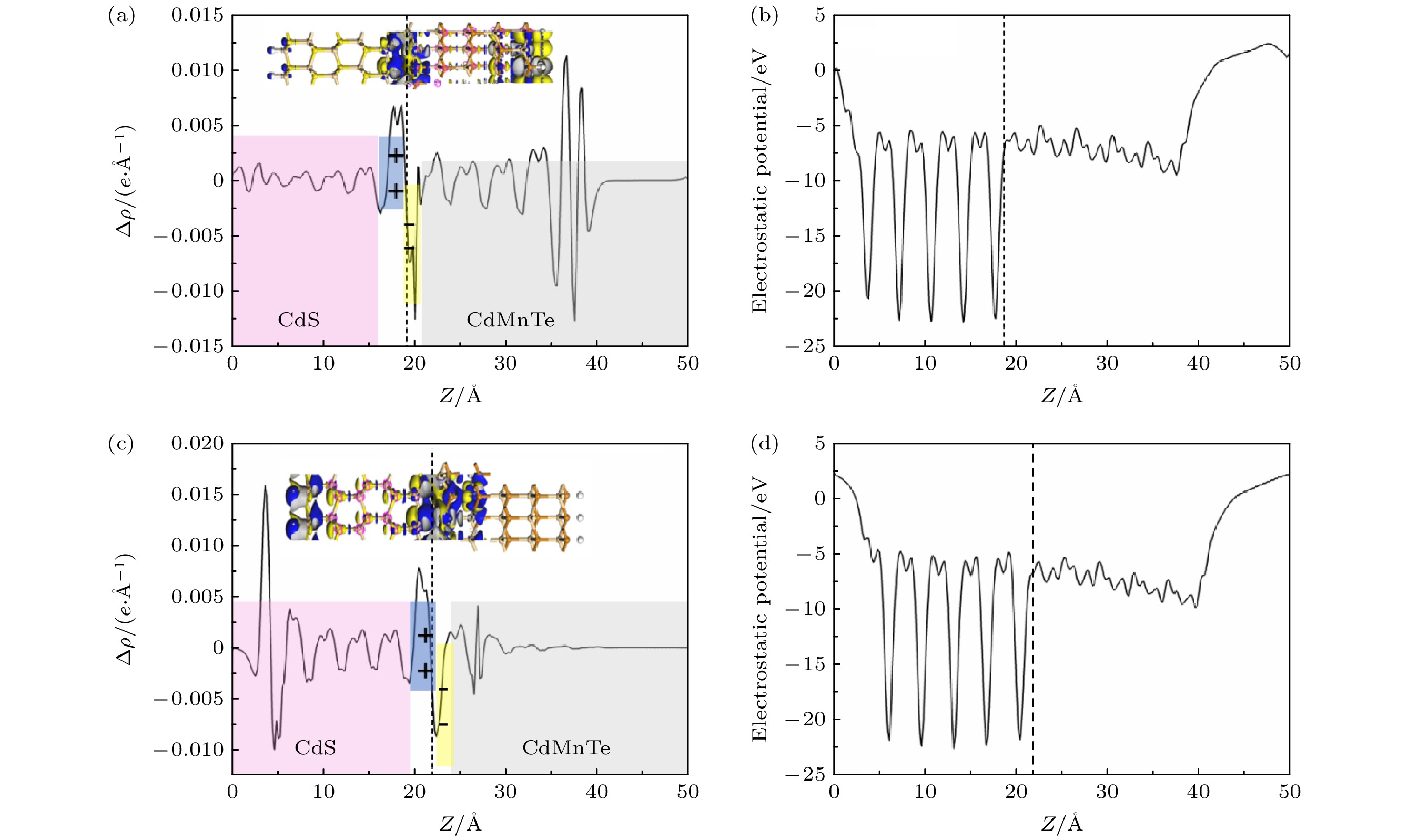
图 9 电荷重新分布图 (a), (c)异质结A₂与B₂的差分电荷示意图和相应平面差分电荷密度曲线, 黄色区域代表电荷消耗, 蓝色区域代表电荷积累, 等值面值为0.00103 e/Å³; (b), (d) A₂与B₂沿z轴方向的平均静电势

Figure 9. Distribution diagram of charges: (a), (c) Charge density difference and the corresponding planar differential charge density curve of the A₂ model and B₂ model, respectively. The yellow region represents charge depletion, the bule region indicates charge accumulation, the isosurface value is 0.00103 e/Å³. (b), (d) the average electrotactic potential difference of the A₂ model and B₂ model, respectively, along the direction of z axis.

DownLoad: Full-Size Img PowerPoint

图 10 表面模型和异质结模型的吸收光谱　(a) CdS (002), CdMnTe (111)-B₁表面和异质结B₂模型; (b)异质结A₂和B₂模型

Figure 10. Absorption spectra of surface models and heterojunction models: (a) Surface CdS (002), surfaces of CdMnTe (111)-B₁, and B₂ model of CdMnTe/CdS heterojunction model; (b) heterojunction A₂ model and B₂ model.

DownLoad: Full-Size Img PowerPoint

表 1 CdTe和CdS晶格参数优化结果

Table 1. Optimal lattice parameters of CdTe and CdS.

	CdTe	CdS	^*CdTe^[25]	^*CdS^[25]	^#CdTe^[26]	^#CdS^[27]
a/Å	6.642	4.214	6.646	4.212	6.481	4.140
b /Å	6.642	4.214	6.646	4.212	6.481	4.140
c /Å	6.642	6.850	6.646	6.858	6.481	6.720
注: ^*为理论值, ^#为实验值.

DownLoad: CSV

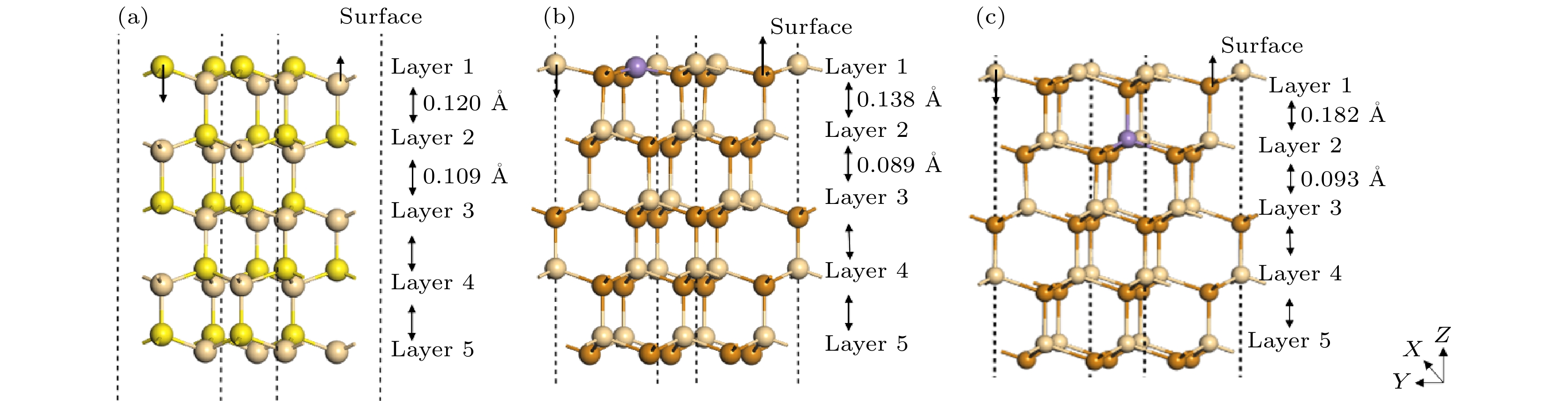
表 2 弛豫后CdMnTe (111)和CdS (002)表面层间距离的变化

Table 2. Variations of interlayer spacing of CdMnTe (111) and CdS (002) surfaces after relaxation.

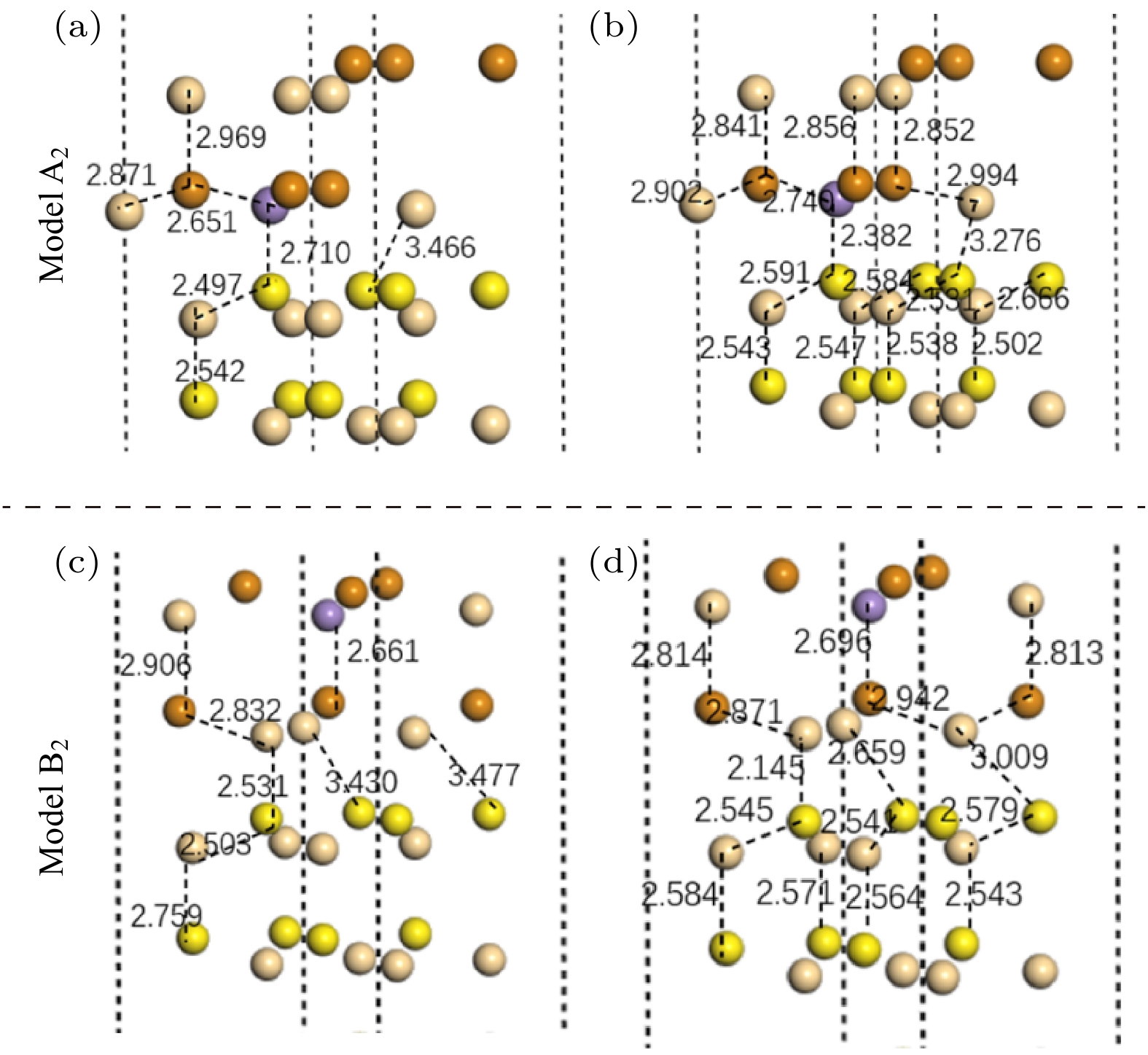
Surface	items	d_1-2/Å	d_2-3/Å	d_3-4/Å	d_4-5/Å
CdMnTe-A₁	d_Cd-Te	2.829	2.837	2.831	2.831
	d_Mn-Te	2.605
	∆d	0.138	0.089	0.000	0.000
CdMnTe-B₁	d_Cd-Te	2.791	2.841	2.831	2.831
	d_Mn-Te		2.697
	∆d	0.182	0.093	0.000	0.000
CdS	d_Cd-S	2.534	2.538	2.536	2.536
	∆d	0.120	0.109	0.000	0.000

DownLoad: CSV

map

[1]	朱建国, 孙小松, 李卫 2007 电子与光电子材料 (北京: 国防工业出版社) 第125页 Zhu J G, Sun X S, Li W 2007 Electronic and Optoelectronic Materials (Beijing: Defense Industry Press) p125 (in chinese)
[2]	Miles R W, Hynes K M, Forbes I 2005 Prog. Cryst. Growth Charact. Mater. 51 1 Google Scholar
[3]	Mishima T, Taguchi M, Sakata H, Maruyama E 2011 Sol. Energy Mater. Sol. Cells 95 18 Google Scholar
[4]	Jordan D, Nagle J P 1994 Prog. Photovoltaics 2 171 Google Scholar
[5]	Cheng Y J, Yang S H, Hsu C S 2009 Chem. Rev. 109 5868 Google Scholar
[6]	Mora-Seró I, Bisquert J 2010 J. Phys. Chem. Lett. 1 3046 Google Scholar
[7]	Zhao J 2004 Sol. Energy Mater. Sol. Cells 82 53 Google Scholar
[8]	Cojocaru-Miredin O, Choi P, Wuerz R, Raabe D 2011 Appl. Phys. Lett. 98 73 Google Scholar
[9]	Yang L, Xuan Y, Tan J 2011 Opt. Express 19 A1165 Google Scholar
[10]	Chopra K L, Paulson P D, Dutta V 2004 Prog. Photovoltaics 12 69 Google Scholar
[11]	Zhang K, Guo H 2017 J. Mater. Sci. Mater. Electron. 28 17044 Google Scholar
[12]	Wu X 2004 Sol. Energy 77 803 Google Scholar
[13]	Chander S, De A K, Dhaka M S 2018 Sol. Energy 174 757 Google Scholar
[14]	Chander S, Dhaka M S 2016 Phys. E 84 112 Google Scholar
[15]	Lee S H, Gupta A, Wang S, Compaan A D, McCandless B E 2005 Sol. Energy Mater. Sol. Cells 86 551 Google Scholar
[16]	Chander S, Dhaka M S 2019 Sol. Energy 183 544 Google Scholar
[17]	Rohatgi A, Ringel S A, Welch J, Meeks E, Pollard K, Erbil A, Summers C J, Meyers P V 1988 Sol. Energy 24 185
[18]	Luan L J, Gao L, Lv H H, Yu P F, Wang T, He Y, Zheng D 2020 Sci. Rep. 10 1 Google Scholar
[19]	Wang S L, Lee S H, Gupta A 2004 MRS Proc. 836 L5.39 Google Scholar
[20]	侯泽荣, 万磊, 白治中, 王德亮 2010 中国科学技术大学学报 40 718 Google Scholar Hou Z R, W L, Bai Z Z, Wang D L 2010 J. Univ. Sci. Tech. Chin. 40 718 Google Scholar
[21]	Olusola O I, Madugu M L, Ojo A A, Dharmadasa I M 2020 J. Mater. Sci. Mater. Electron. 31 22151 Google Scholar
[22]	Shafaay B A 2014 J. Chem., Biol. Phys. Sci. 4 1
[23]	Gueddim A, Madjet M E, Zerroug S, Bouarissa N 2016 Opt. Quantum Electron. 48 551 Google Scholar
[24]	Llchuk H, Zmiiovska E, Petrus R, Semkive I, Lopatynskyi I, Kashuba 2020 J. Nano-Electron. Phys. 12 01027 Google Scholar
[25]	Cao A, Tan T T, Zhang H, Du Y, Sun Y, Zha G 2018 Phys. B 545 323 Google Scholar
[26]	Merad A, Kanoun M, Merad G, Cibert J, Aourag H 2005 Mater. Chem. Phys. 92 333 Google Scholar
[27]	Liu H X, Tang F L, Xue H T, Zhang Y, Cheng Y W, Feng Y D 2016 Chin. Phys. B 25 123101 Google Scholar
[28]	Shenoy S, Tarafder K 2020 J. Phys. Condens. Matter 32 275501 Google Scholar
[29]	Kresse G, Joubert D 1999 Phys. Rev. B 59 1758 Google Scholar
[30]	Shi L B, Xu C Y, Yuan H K 2011 Phys. B 406 3187 Google Scholar
[31]	Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865 Google Scholar
[32]	Butler K T, Frost J M, Walsh A 2015 Mater. Horiz. 2 228 Google Scholar
[33]	Wang J S, Tong S C, Tsai Y H, Tsai W J, Yang C S, Chang Y H, Shen J L 2015 J. Alloys Compd. 646 129 Google Scholar
[34]	Kumar S G, Rao K S R K 2014 Energy Environ. Sci. 7 45 Google Scholar
[35]	Cheng Y W, Tang F L, Xue H T, Liu H X, Gao B, Feng Y D 2016 Mater. Sci. Semicond. Process. 45 9 Google Scholar
[36]	Momma K, Izumi F 2008 J. Appl. Crystallogr. 41 653 Google Scholar
[37]	Guo Y, Xue Y, Geng C, Li C, Li X, Niu Y 2019 J. Phys. Chem. C 123 16075 Google Scholar
[38]	Yin W J, Shi T, Yan Y 2015 J. Phys. Chem. C 119 5253 Google Scholar
[39]	Scharber M C, Mühlbacher D, Koppe M, Denk P, Waldauf C, Heeger A J, Brabec C J 2006 Adv. Mater. 18 789 Google Scholar
[40]	Sharma S, Devi N, Verma U P, RajaRam P 2011 Phys. B 406 4547 Google Scholar
[41]	Dadsetani M, Pourghazi A 2006 Phys. Rev. B 73 195102 Google Scholar
[42]	Gajdos M, Hummer K, Kresse G, Furthmueller J, Bechstedt F 2006 Phys. Rev. B 73 045112 Google Scholar

[1]	Wang Xiu-Yu, Wang Tao, Cui Yu-Ang, Wu Xi-Guang-Run, Wang Yang. First-principles study of effect of impurity compensation on optical properties of Si. Acta Physica Sinica, 2024, 73(11): 116301. doi: 10.7498/aps.73.20231814
[2]	Liu Chen-Xi, Pang Guo-Wang, Pan Duo-Qiao, Shi Lei-Qian, Zhang Li-Li, Lei Bo-Cheng, Zhao Xu-Cai, Huang Yi-Neng. First-principles study of influence of electric field on electronic structure and optical properties of GaN/g-C₃N₄ heterojunction. Acta Physica Sinica, 2022, 71(9): 097301. doi: 10.7498/aps.71.20212261
[3]	Xu Da-Qing, Zhao Zi-Han, Li Pei-Xian, Wang Chao, Zhang Yan, Liu Shu-Lin, Tong Jun. First-principle study on electronic structures, magnetic, and optical properties of different valence Mn ions doped InN. Acta Physica Sinica, 2018, 67(8): 087501. doi: 10.7498/aps.67.20172504
[4]	Tang Shi-Hui, Cao Xiu-Xia, He Lin, Zhu Wen-Jun. Effects of vacancy point defects and phase transitions on optical properties of shocked Al2O3. Acta Physica Sinica, 2016, 65(14): 146201. doi: 10.7498/aps.65.146201
[5]	Gao Min, Shu Wen-Lu, Ye Qiang, He Lin, Zhu Wen-Jun. Optical properties of (Mg0.97, Fe0.03)O ferropericlase under the pressure of the Earth’s lower mantle. Acta Physica Sinica, 2015, 64(11): 119101. doi: 10.7498/aps.64.119101
[6]	Jiao Zhao-Yong, Guo Yong-Liang, Niu Yi-Jun, Zhang Xian-Zhou. The first principle study of electronic and optical properties of defect chalcopyrite XGa2S4 (X=Zn, Cd, Hg). Acta Physica Sinica, 2013, 62(7): 073101. doi: 10.7498/aps.62.073101
[7]	Duan Yong-Hua, Sun Yong. Electronic structure and optical properties of (α, β, γ)-Nb5Si3. Acta Physica Sinica, 2012, 61(21): 217101. doi: 10.7498/aps.61.217101
[8]	Feng Xian-Yang, Lu Yao, Jiang Lei, Zhang Guo-Lian, Zhang Chang-Wen, Wang Pei-Ji. Study of the optical properties of superlattices ZnO doped with indium. Acta Physica Sinica, 2012, 61(5): 057101. doi: 10.7498/aps.61.057101
[9]	Lu Yao, Wang Pei-Ji, Zhang Chang-Wen, Feng Xian-Yang, Jiang Lei, Zhang Guo-Lian. Study of material properties of Fe, S Co-doped SnO2 by first principles. Acta Physica Sinica, 2012, 61(2): 023101. doi: 10.7498/aps.61.023101
[10]	Deng Jiao-Jiao, Liu Bo, Gu Mu, Liu Xiao-Lin, Huang Shi-Ming, Ni Chen. First principles calculation of electronic structures and optical properties for -CuX(X = Cl, Br, I). Acta Physica Sinica, 2012, 61(3): 036105. doi: 10.7498/aps.61.036105
[11]	Jiao Zhao-Yong, Yang Ji-Fei, Zhang Xian-Zhou, Ma Shu-Hong, Guo Yong-Liang. Theoretical investigation of elastic, electronic, and optical properties of zinc-blende structure GaN under high pressure. Acta Physica Sinica, 2011, 60(11): 117103. doi: 10.7498/aps.60.117103
[12]	Yu Feng, Wang Pei-Ji, Zhang Chang-Wen. Electronic structure and optical properties of Al-doped SnO2. Acta Physica Sinica, 2011, 60(2): 023101. doi: 10.7498/aps.60.023101
[13]	Lu Yao, Wang Pei-Ji, Zhang Chang-Wen, Jiang Lei, Zhang Guo-Lian, Song Peng. Material opto-electronic properties of In, N co-doped SnO2 studied by first principles. Acta Physica Sinica, 2011, 60(6): 063103. doi: 10.7498/aps.60.063103
[14]	Lu Yao, Wang Pei-Ji, Zhang Chang-Wen, Feng Xian-Yang, Jiang Lei, Zhang Guo-Lian. First-principles calculation on electronic structure and optical properties of iron-doped SnO2. Acta Physica Sinica, 2011, 60(11): 113101. doi: 10.7498/aps.60.113101
[15]	Gu Mu, Lin Ling, Liu Bo, Liu Xiao-Lin, Huang Shi-Ming, Ni Chen. Fist-principle calculation for electronic structure of M’-GdTaO4. Acta Physica Sinica, 2010, 59(4): 2836-2842. doi: 10.7498/aps.59.2836
[16]	Zhang Xue-Jun, Gao Pan, Liu Qing-Ju. First-principles study on electronic structure and optical properties of anatase TiO2 codoped with nitrogen and iron. Acta Physica Sinica, 2010, 59(7): 4930-4938. doi: 10.7498/aps.59.4930
[17]	Tan Xing-Yi, Jin Ke-Xin, Chen Chang-Le, Zhou Chao-Chao. Electronic structure of YFe2B2by first-principles calculation. Acta Physica Sinica, 2010, 59(5): 3414-3417. doi: 10.7498/aps.59.3414
[18]	Li Pei-Juan, Zhou Wei-Wei, Tang Yuan-Hao, Zhang Hua, Shi Si-Qi. Electronic structure，optical and lattice dynamical properties of CeO2：A first-principles study. Acta Physica Sinica, 2010, 59(5): 3426-3431. doi: 10.7498/aps.59.3426
[19]	Liu Jian-Jun. The effect on electronic density of states and optical properties of ZnO by doping Ga. Acta Physica Sinica, 2010, 59(9): 6466-6472. doi: 10.7498/aps.59.6466
[20]	Yu Feng, Wang Pei-Ji, Zhang Chang-Wen. First-principles study of optical and electronic properties of N-doped SnO2. Acta Physica Sinica, 2010, 59(10): 7285-7290. doi: 10.7498/aps.59.7285

Catalog

Vol. 70, Issue 16 - 2021-08-20

Metrics

Abstract views: 7194
PDF Downloads: 224
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板