-
In this work a new scheme is proposed to accurately calculate the rotational energy level of the rigid symmetric-top molecule subjected to the external electric field, and also to obtain the corresponding analytical wave functions. For this purpose, first we use the different forms of function transformation and variable substitution to transform the differential equation of the polar angle θ into a confluent Heun differential equation, and then we use the characteristics of the confluent Heun differential equation and the confluent Heun function to find two linearly dependent solutions of the same eigenstates, which are used to construct the Wronskian determinant to obtain the exact energy spectrum equation. Finally, with the aid of the Maple software, we calculate the eigenvalues for different quantum states, and then substitute the obtained eigenvalues into the unnormalized eigenfunction to obtain the analytical normalized eigenfunction expressed by the confluent Heun function. These results are conducive to the in-depth study of the Stark effect of symmetric-top molecules.
-
Keywords:
- exactly analytic solutions /
- Stark effect /
- rigid symmetric-top molecules /
- confluent Heun function
[1] Townes C H, Schawlow A L 1975 Microwave Spectroscopy (New York: Dover Publications, Inc.) pp60−62, 248−251
[2] Wollrab J E 1967 Rotational Spectra and Molecular Structure (New York: Academic Press) pp15−18, 247−250
[3] Shirley J H 1963 J. Chem. Phys. 38 2896
Google Scholar
[4] Tomutza L, Mizushima M 1972 J. Quant. Spectrosc. Radiat. Transfer. 12 925
Google Scholar
[5] Roeggen I 1972 Atomic Date 4 289
Google Scholar
[6] Maergoiz A I, Troe J 1993 J. Chem. Phys. 99 3218
Google Scholar
[7] Maergoiz A I, Troe J, Weiss Ch 1994 J. Chem. Phys. 101 1885
Google Scholar
[8] Fernández F M 1995 J. Math. Chem. 18 197
Google Scholar
[9] Burrowst B L, Cohen M, Feldmann T 1995 J. Phys. B 28 4249
Google Scholar
[10] Andreev S N, Makarov V P 2008 Radiophys. Quantum Electron. 51 718
Google Scholar
[11] Sun G H, Chen C Y, Taud H, Yáñez-Márquez C, Dong S H 2020 Phys. Lett. A 384 126480
Google Scholar
[12] Chen C Y, Wang X H, You Y, Sun G H, Dong S H 2020 Int. J. Quantum Chem. 120 e26336
Google Scholar
[13] Chen C Y, You Y, Wang X H, Lu F L, Sun D S, Dong S H 2021 Results Phys. 24 104115
Google Scholar
[14] Chen C Y, Sun D S, Sun G H, Wang X H, You Y, Dong S H 2021 Int. J. Quantum Chem. 121 e26546
Google Scholar
[15] Fiziev P P 2010 J. Phys. A 43 035203
Google Scholar
[16] Downing C A 2013 J. Math. Phys. 54 072101
Google Scholar
[17] Al-Gwaiz M A 2008 Sturm-Liouville Theory and its Applications (London: Springer-Verlag London Limited) pp45−46, 55−59
[18] 程建春 2016 数学物理方程及其近似方法(第2版) (北京: 科学出版社) 第109−111, 133−135页
Cheng J C 2016 The Equations for Mathematical Physics and Their Approximate Methods (2nd Ed.) (Beijing: Science Press) pp109−111, 133−135 (in Chinese)
[19] Wang H Y 2017 Mathematics for Physicists (Beijing: Science Press) pp130−146
-
图 1 对称陀螺分子的
$ f(\lambda ) $ 随λ的变化曲线 (a)$ b = 1, \;K = 0, \;M = 0\, , \;1\, , \;2\, , \;3, \, 4 $ ; (b)$ b = 5, \;K = 1, \;M = 0\, , \;1\, , \;2\, , \;3, \, 4 $ ; (c)$b = $ $ 10, \;K = 2, \;M = 0\, , - 1\, , - 2\, , - 3$ ; (d)$ b = 20, \;K = 3, \;M = 0\, , - 1\, , - 2\, , - 3 $ Figure 1. Plot of
$ f(\lambda ) $ as the function of λ for the symmetric-top molecules: (a)$ b = 1, \;K = 0, \;M = 0\, , \;1\, , \;2\, , \;3, \, 4 $ ; (b)$b = 5, $ $ \;K = 1, \;M = 0\, , \;1\, , \;2\, , \;3, \, 4$ ; (c)$ b = 10, \;K = 2, \;M = 0\, , - 1\, , - 2\, , - 3 $ ; (d)$ b = 20, \;K = 3, \;M = 0\, , - 1\, , - 2\, , - 3 $ .
图 2
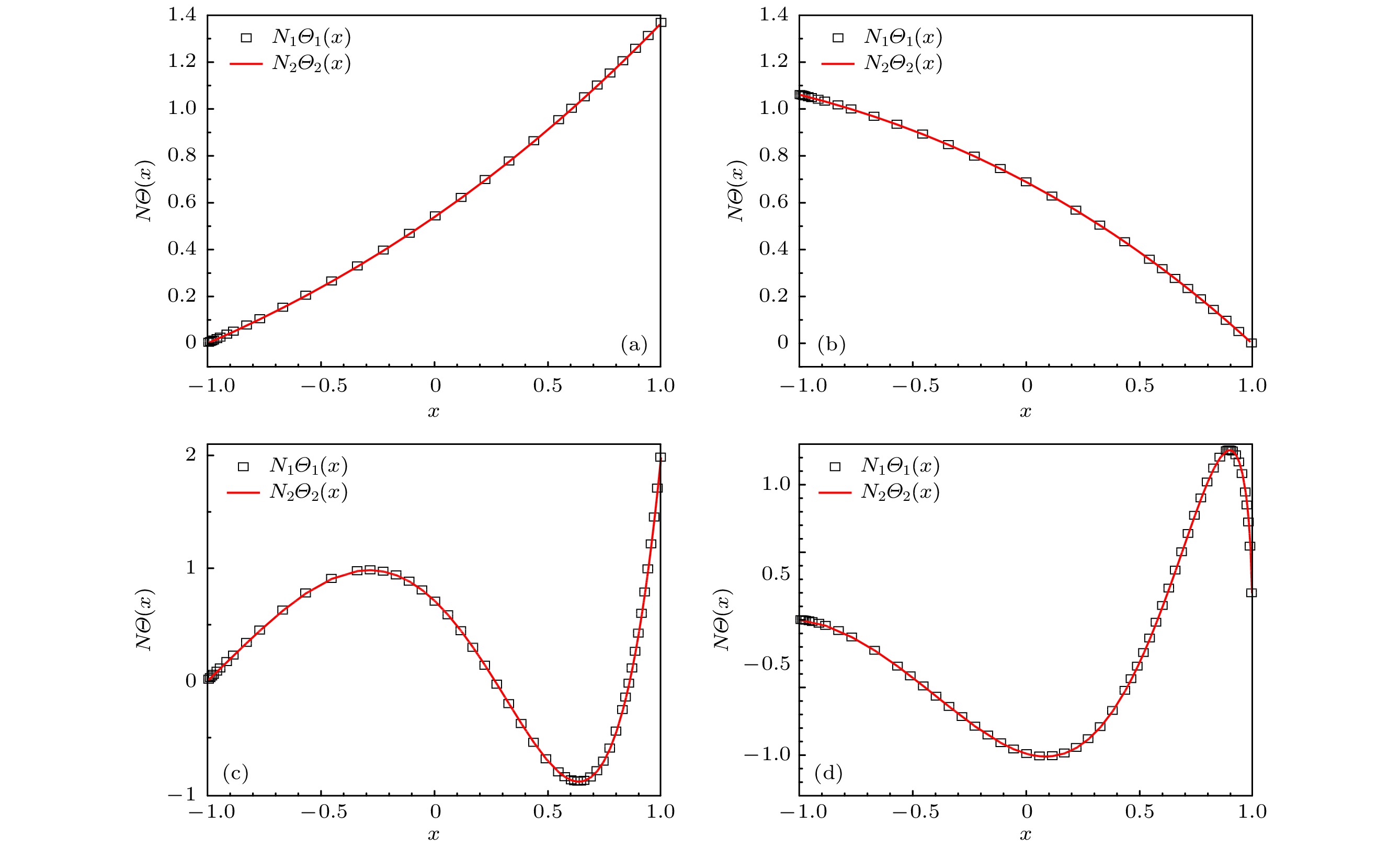
$ {N_1}{\varTheta _1}(x) $ 和$ {( - 1)^n}{N_2}{\varTheta _2}(x) $ 是线性相关的 (a)$ b = 1\, , \;J = 1\, , \;K = 1\, , \;M = 1\, , \;n = 0 $ ; (b)$b = 1\, , \;J = 1\, , \;K = 1\, , \;M = $ $ - 1\, , \;n = 0$ ; (c)$ b = 10\, , \;J = 3\, , \;K = 1\, , \;M = 1\, , \;n = 2 $ ; (d)$ b = 1\, 0, \;J = 3\, , \;K = 1\, , \;M = 2\, , \;n = 1 $ Figure 2. Linear dependence relation between
$ {N_1}{\varTheta _1}(x) $ and$ {( - 1)^n}{N_2}{\varTheta _2}(x) $ : (a)$ b = 1\, , \;J = 1\, , \;K = 1\, , \;M = 1\, , \;n = 0 $ ; (b)$b = $ $ 1\, , \;J = 1\, , \;K = 1\, , \;M = - 1\, , \;n = 0$ ; (c)$ b = 10\, , \;J = 3\, , \;K = 1\, , \;M = 1\, , \;n = 2 $ ; (d)$ b = 1\, 0, \;J = 3\, , \;K = 1\, , \;M = 2\, , \;n = 1 $ .表 1 对称陀螺分子λ的精确值
Table 1. Precise values of λ for the symmetric-top molecules.
J K (K, M) n b = 1 b = 5 b = 10 b = 20 0 0 (0, 0) 0 –0.15766348 –2.36561388 –6.04507511 –14.18676623 1 0 (0, 0) 1 2.09076065 2.63874703 1.72333341 –2.64067155 (0, 1) (0, –1) 0 1.95033391 0.90475071 –1.52336944 –7.83391897 1 (1, 1) (–1, –1) 0 1.46550429 –1.13615048 –4.90088894 –13.09274467 (1, 0) (–1, 0) 0 1.95033391 0.90475071 –1.52336944 –7.83391897 (1, –1) (–1, 1) 0 2.45930791 3.25240982 2.28859798 –2.10241580 2 0 (0, 0) 2 6.02403166 6.64546248 7.87932729 7.59702750 (0, 1) (0, –1) 1 6.01157793 6.14932334 5.86920589 3.21233397 (0, 2) (0, –2) 0 5.97623080 5.42770799 3.91145991 –0.75028417 1 (1, 2) (–1, –2) 0 5.64610143 3.87965848 1.11394605 –5.42557601 (1, 1) (–1, –1) 1 5.83444799 5.02308599 3.42628660 –1.26151418 (1, 0) (–1, 0) 1 6.01157793 6.14932334 5.86920589 3.21233397 (1, –1) (–1, 1) 1 6.17303459 7.17694539 8.52241501 8.17688040 (1, –2) (–1, 2) 0 6.31159733 7.08037678 6.95972394 4.30076661 2 (2, 2) (–2, –2) 0 5.32080726 2.40921111 –1.50915355 –9.82205218 (2, 1) (–2, –1) 0 5.64610143 3.87965848 1.11394605 –5.42557601 (2, 0) (–2, 0) 0 5.97623080 5.42770799 3.91145991 –0.75028417 (2, –1) (–2, 1) 0 6.31159733 7.08037678 6.95972394 4.30076661 (2, –2) (–2, 2) 0 6.65266958 8.89010555 10.43690550 9.90220896 3 0 (0, 0) 3 12.01112256 12.28609326 13.23851702 16.01072933 (0, 1) (0, –1) 2 12.00832614 12.20185604 12.68109763 13.00122422 (0, 2) (0, –2) 1 11.99996510 11.98051777 11.76516026 10.12548434 (0, 3) (0, –3) 0 11.98611962 11.65790586 10.68518379 7.32389600 1 (1, 3) (–1, –3) 0 11.73714675 10.44710964 8.38487920 3.22650134 (1, 2) (–1, –2) 1 11.83176701 11.08791249 9.87421333 6.36151132 (1, 1) (–1, –1) 2 11.92226344 11.68117484 11.32510413 9.60625434 (1, 0) (–1, 0) 2 12.00832614 12.20185604 12.68109763 13.00122422 (1, –1) (–1, 1) 2 12.08964046 12.60886369 13.77998811 16.66271656 (1, –2) (–1, 2) 1 12.16589908 12.84075061 13.66336275 14.14238561 (1, –3) (–1, 3) 0 12.23682184 12.90821524 13.10954794 11.68530530 2 (2, 3) (–2, –3) 0 11.48984080 9.26997167 6.18154889 –0.66884422 (2, 2) (–2, –2) 1 11.66163328 10.18131826 8.01132314 2.77663392 (2, 1) (–2, –1) 1 11.83176701 11.08791249 9.87421333 6.36151132 (2, 0) (–2, 0) 1 11.99996510 11.98051777 11.76516026 10.12548434 (2, –1) (–2, 1) 1 12.16589908 12.84075061 13.66336275 14.14238561 (2, –2) (–2, 2) 1 12.32917589 13.62647723 15.47989534 18.60143861 (2, –3) (–2, 3) 0 12.48932036 14.20615704 15.70403035 16.41950037 3 (3, 3) (–3, –3) 0 11.24414341 8.12208069 4.05742691 –4.40185593 (3, 2) (–3, –2) 0 11.48984080 9.26997167 6.18154889 –0.66884422 (3, 1) (–3, –1) 0 11.73714675 10.44710964 8.38487920 3.22650134 (3, 0) (–3, 0) 0 11.98611962 11.65790586 10.68518379 7.32389600 (3, –1) (–3, 1) 0 12.23682184 12.90821524 13.10954794 11.68530530 (3, –2) (–3, 2) 0 12.48932036 14.20615704 15.70403035 16.41950037 (3, –3) (–3, 3) 0 12.74368721 15.56368561 18.56353300 21.76261674 
DownLoad: CSV
表 2 对称陀螺分子(4, 0, 0)态的λ值
Table 2. Values of λ of the state (4, 0, 0) for the symmetric-top molecules.
b Perturbation Maergoiz[7] This work b Maergoiz[7] This work 0.1 20.00006494 20.00006494 100 15.696 15.69559837 1 20.00649351 20.00649533 200 –31.200 –31.20010397 5 20.16233766 20.164 20.16352072 300 –90.598 –90.59767902 10 20.64935065 20.671 20.67080282 400 –156.41 –156.40991571 20 22.59740260 22.975 22.97522235 500 –226.31 –226.30840320 50 36.23376623 27.681 27.68132358 750 –412.26 –412.26234718
DownLoad: CSV
-
[1] Townes C H, Schawlow A L 1975 Microwave Spectroscopy (New York: Dover Publications, Inc.) pp60−62, 248−251
[2] Wollrab J E 1967 Rotational Spectra and Molecular Structure (New York: Academic Press) pp15−18, 247−250
[3] Shirley J H 1963 J. Chem. Phys. 38 2896
Google Scholar
[4] Tomutza L, Mizushima M 1972 J. Quant. Spectrosc. Radiat. Transfer. 12 925
Google Scholar
[5] Roeggen I 1972 Atomic Date 4 289
Google Scholar
[6] Maergoiz A I, Troe J 1993 J. Chem. Phys. 99 3218
Google Scholar
[7] Maergoiz A I, Troe J, Weiss Ch 1994 J. Chem. Phys. 101 1885
Google Scholar
[8] Fernández F M 1995 J. Math. Chem. 18 197
Google Scholar
[9] Burrowst B L, Cohen M, Feldmann T 1995 J. Phys. B 28 4249
Google Scholar
[10] Andreev S N, Makarov V P 2008 Radiophys. Quantum Electron. 51 718
Google Scholar
[11] Sun G H, Chen C Y, Taud H, Yáñez-Márquez C, Dong S H 2020 Phys. Lett. A 384 126480
Google Scholar
[12] Chen C Y, Wang X H, You Y, Sun G H, Dong S H 2020 Int. J. Quantum Chem. 120 e26336
Google Scholar
[13] Chen C Y, You Y, Wang X H, Lu F L, Sun D S, Dong S H 2021 Results Phys. 24 104115
Google Scholar
[14] Chen C Y, Sun D S, Sun G H, Wang X H, You Y, Dong S H 2021 Int. J. Quantum Chem. 121 e26546
Google Scholar
[15] Fiziev P P 2010 J. Phys. A 43 035203
Google Scholar
[16] Downing C A 2013 J. Math. Phys. 54 072101
Google Scholar
[17] Al-Gwaiz M A 2008 Sturm-Liouville Theory and its Applications (London: Springer-Verlag London Limited) pp45−46, 55−59
[18] 程建春 2016 数学物理方程及其近似方法(第2版) (北京: 科学出版社) 第109−111, 133−135页
Cheng J C 2016 The Equations for Mathematical Physics and Their Approximate Methods (2nd Ed.) (Beijing: Science Press) pp109−111, 133−135 (in Chinese)
[19] Wang H Y 2017 Mathematics for Physicists (Beijing: Science Press) pp130−146
DownLoad:
Catalog
Metrics
- Abstract views: 4343
- PDF Downloads: 92
- Cited By: 0
