-
With the development of intelligent technology, it is essential to develop polarization-conversion devices with adaptable electromagnetic (EM) performance for practical applications. Up to now, most of attempts have relied on PIN diodes and varactor diodes for electrical tuning, typically featuring simplicity and timelineness. However, the shortcomings are also notable, such as less degrees of freedom (DoFs), more complex circuits and more expensive. In view of this, here we propose a kind of spatial-order metasurface for reconfigurable polarization conversion based on kirigami concept. By adjusting the folding angle β, the interaction between neighboring dipoles can be progressively changed and thus the operation frequency of polarization conversion can be shifted. Such a mechanical reconfigurable strategy brings about more DoFs for tuning and is cheaper and extraordinary convenient in practice. To verify the feasibility of our concept, a proof-of-concept spatial-order kirigami metasurface is proposed for the dual-band reconfigurable linear polarization conversion based on asymmetric chiral split ring resonators (SRRs). Experimental results show that the linear polarization operates at 5 and 5.8 GHz when folding angle is β = 10°, these frequencies are shifted to 5.8 and 7.2 GHz when β = 45°: a tuning range is expanded by 18.5%. In addition, the Poisson’s ratio and relative density of proposed kirigami metasurface as a function of β are also theoretically analyzed. The results show that the Poisson’s ratio increases with the value of β increasing. The relative density can be reduced to 1.5% of its unfolded planar counterpart. Our spatial-order kirigami metasurface strategy paves the way for implementing the reconfigurable linear polarization conversion and multifunctional devices.
-
Keywords:
- reconfigurable kirigami metasurfaces /
- polarization conversion /
- split ring resonators /
- spatial-order
[1] 梅中磊, 张黎, 崔铁军 2016 科技导报 34 27
Google Scholar
Mei Z L, Zhang L, Cui T J 2016 Science & Technology Review 34 27
Google Scholar
[2] 王彦朝, 许河秀, 王朝辉, 王明照, 王少杰 2020 69 134101
Google Scholar
Wang Y Z, Xu H X, Wang C H, Wang M Z, Wang S J 2020 Acta Phys. Sin. 69 134101
Google Scholar
[3] Xu H X, Sun S, Tang S, Ma S, He Q, Wang G M, Cai T, Li H P, Zhou L 2016 Sci. Rep. 6 27503
Google Scholar
[4] Xu H X, Ma S, Luo W, Cai T, Sun S, He Q, Zhou L 2016 Appl. Phys. Lett. 109 193506
Google Scholar
[5] Xu H X, Tang S, Ma S, Luo W, Cai T, Sun S, He Q, Zhou L 2016 Sci. Rep. 6 38255
Google Scholar
[6] Zheng G, Mühlenbernd H, Kenney M, Li G, Zentgraf T, Zhang S 2015 Nat. Nanotechnol. 10 308
Google Scholar
[7] Wang C, Xu H X, Wang Y, Zhu S, Wang C, Mao R 2020 J. Phys. D: Appl. Phys. 53 365001
Google Scholar
[8] 李晓楠, 周璐, 赵国忠 2019 68 238101
Google Scholar
Li X N, Zhou L, Zhao G Z 2019 Acta Phys. Sin. 68 238101
Google Scholar
[9] 李勇峰, 张介秋, 屈绍波, 王甲富, 吴翔, 徐卓, 张安学 2015 64 124102
Google Scholar
Li Y F, Zhang J Q, Qu S B, Wang J F, Wu X, Xu Z, Zhang A X 2015 Acta Phys. Sin. 64 124102
Google Scholar
[10] 郭文龙, 王光明, 李海鹏, 侯海生 2016 65 074101
Google Scholar
Guo W L, Wang G M, Li H P, Hou H S 2016 Acta Phys. Sin. 65 074101
Google Scholar
[11] 于惠存, 曹祥玉, 高军, 杨欢欢, 韩江枫, 朱学文, 李桐 2018 67 224101
Google Scholar
Yu H C, Cao X Y, Gao J, Yang H H, Han J F, Zhu X W, Li T 2018 Acta Phys. Sin. 67 224101
Google Scholar
[12] 范亚, 屈绍波, 王甲富, 张介秋, 冯明德, 张安学 2015 64 184101
Google Scholar
Fan Y, Qu S B, Wang J F, Zhang J Q, Feng M D, Zhang A X 2015 Acta Phys. Sin. 64 184101
Google Scholar
[13] Wei Z, Cao Y, Fan Y, Yu X, Li H 2011 Appl. Phys. Lett. 99 221907
Google Scholar
[14] Feng M, Wang J, Ma H, Mo W, Ye H, Qu S 2013 J. Appl. Phys. 114 074508
Google Scholar
[15] Chen H, Wang J, Ma H, Qu S, Xu Z, Zhang A, Yan M, Li Y 2014 J. Appl. Phys. 115 154504
Google Scholar
[16] Shi H, Zhang A, Zheng S, Li J, Jiang Y 2014 Appl. Phys. Lett. 104 034102
Google Scholar
[17] Yin J. Y, Wan X, Zhang Q, Cui T J 2015 Sci. Rep. 5 12476
Google Scholar
[18] Chen M, Chang L, Gao X, Chen H, Wang C, Xiao X, Zhao D 2017 IEEE Photonics J. 9 4601011
[19] Yu Y, Xiao F, He C, Jin R, Zhu W 2020 Opt. Express. 28 11797
Google Scholar
[20] Wang M, Zhai Z 2020 Front. Phys. 8 527394
Google Scholar
[21] Kong X, Wang Q, Jiang S, Kong L, Yuan J, Yan X, Wang X, Zhao X 2020 Sci. Rep. 10 17843
Google Scholar
[22] Chambers B 1999 Smart Mater. Struct. 8 64
Google Scholar
[23] Chen K, Feng Y, Monticone F, Zhao J, Zhu B, Jiang T, Zhang L, Kim Y, Ding X, Zhang S, Alù A, Qiu C W 2017 Adv. Mater. 29 1606422
Google Scholar
[24] Li L, Cui T J, Ji W, Liu S, Ding J, Wan X, Li Y B, Jiang M, Qiu C W, Zhang S 2017 Nat. Commun. 8 197
Google Scholar
[25] Cui T J, Qi M Q, Wan X, Zhao J, Cheng Q 2014 Light-Sci. & Appl. 3 e218
Google Scholar
[26] Tian J, Cao X, Gao J, Yang H, Han J, Yu H, Wang S, Jin R, Li T 2019 J. Appl. Phys. 125 135105
Google Scholar
[27] Zhang X G, Jiang W X, Jiang H L, Wang Q, Tian H W, Bai L, Luo Z J, Sun S, Luo Y, Qiu C W, Cui T J 2020 Nat. Electron. 3 165
Google Scholar
[28] Jiang W, Ma H, Feng M, Yan L, Wang J, Wang J, Qu S 2016 J. Phys. D: Appl. Phys. 49 315302
Google Scholar
[29] Jing L, Wang Z, Zheng B, Wang H, Yang Y, Shen L, Yin W, Li E, Chen H 2018 NPG Asia Mater. 10 888
Google Scholar
[30] Wang Z, Jing L, Yao K, Yang Y, Zheng B, Soukoulis C M, Chen H, Liu Y 2017 Adv. Mater. 29 1700412
Google Scholar
[31] Li M, Shen L, Jing L, Xu S, Zheng B, Lin X, Yang Y, Wang Z, Chen H 2019 Adv. Sci. 6 1901434
Google Scholar
[32] Le D H, Xu Y, Tentzeris M M, Lim S 2020 Extreme Mech. Lett. 36 100670
Google Scholar
-
图 1 本文基于可重构空间序构剪纸超表面的双频线极化转换功能示意图(k代表波矢量方向, fm, fn代表线极化转换的工作频率. 二维超表面按照剪裁线裁成条带结构, 条带结构再按照折叠线折叠可形成本文空间序构超表面, 然后通过改变折叠角度β可调谐fm)
Figure 1. Schematic diagram of the dual-band linear polarization conversion based on reconfigurable spatial-order kirigami metasurfaces. Here, k represents the wave vector, and fm, fn represent the operation frequency of cross-polarization conversion. The spatial-order metasurface is constructed by cutting a two-dimensional metasurface into a sets of strips according to the cutting line, and then by folding these strips according to the folding line to form an assembled structure. The fm of resulting metasurface can be adjusted by changing the folding angle β.
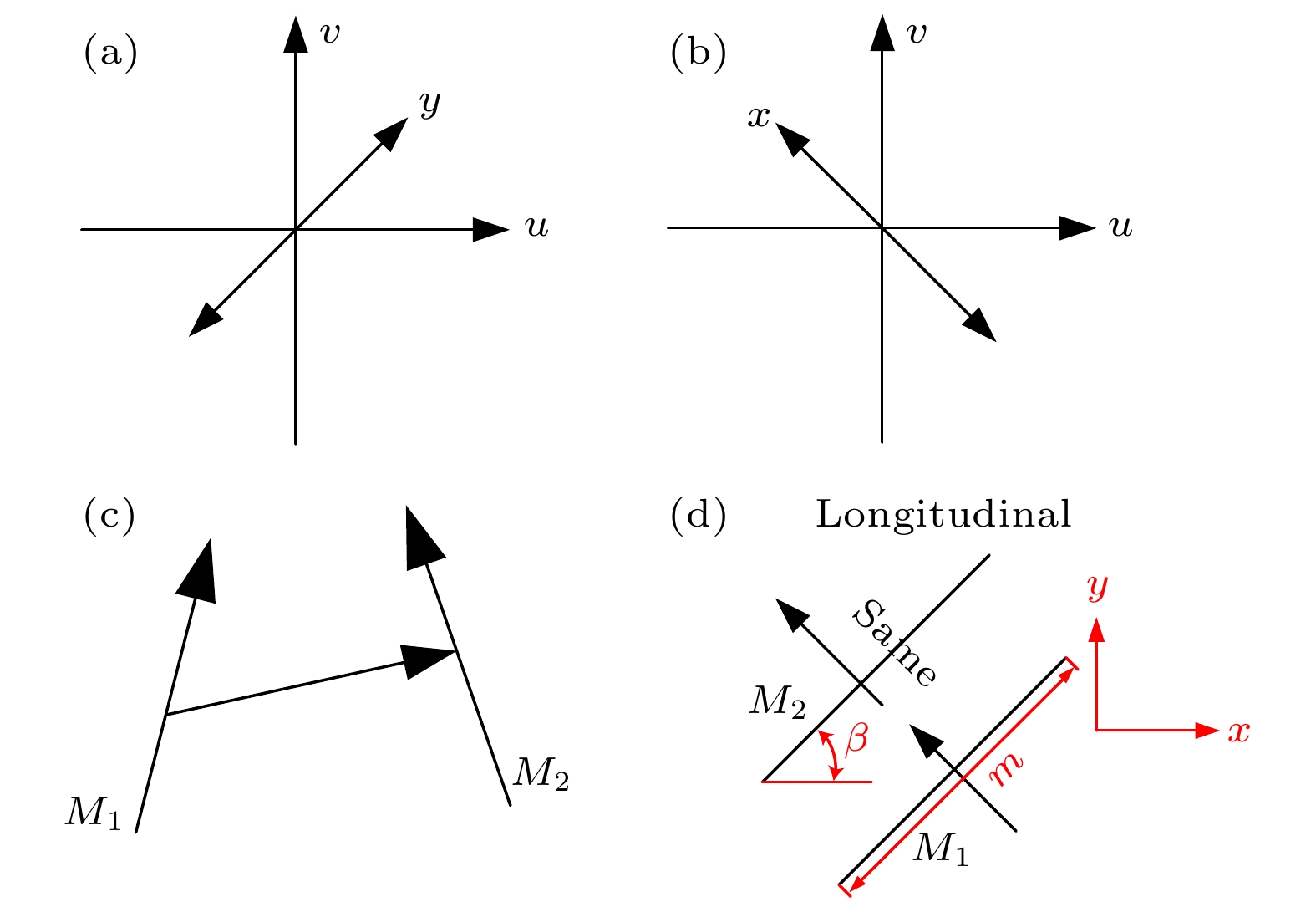
图 2 本文可重构空间序构剪纸超表面的线极化转换与频率调控原理示意图. u-v坐标系下的(a) y极化入射电磁波与(b) x极化反射电磁波; (c)任意两个空间放置磁偶极子的相互作用; (d)两个磁偶极子同向纵向耦合
Figure 2. Schematic principle for linear polarization conversion and operation frequency control of reconfigurable spatial-order kirigami metasurfaces. The (a) incident y-polarized and (b) reflected x-polarized electromagnetic (EM) waves under u-v coordinate. (c) The interaction between two magnetic dipoles placed in free space. (d) The longitudinally coupled magnetic dipoles in identical direction respectively.
图 3 三种不同情形下空间序构超表面单元的结构与数值仿真电磁特性(其中
${\varphi _u}$ (${\varphi _v}$ )和ru(rv)分别表示沿u(v)轴方向电场分量反射相位和反射幅度,${r_{yy}}$ (${r_{xx}}$ )表示y(x)极化波入射时同极化反射电磁波的幅度,${r_{xy}}$ (${r_{yx}}$ )表示y(x)极化波入射时交叉极化反射电磁波的幅度) (a)单元只有开口圆环谐振器; (b)单元只有开口方环谐振器; (c)单元同时包含开口圆环和开口方环谐振器; (d)谐振频率f = 6.8和5.5 GHz处超表面单元SRRs上的表面电流分布; (e)最终空间序构超表面单元的结构, 结构参数依次为m = 18 mm, n = 6.7 mm, d = 0.1 mm, r2 = 3 mm, r1 = r2 – b1 = 2.4 mm, a1 = 6 mm, a2 = a1 – 2b2 = 4.8 mm和g = 0.44 mm, 黄色部分为金属铜, 蓝色部分为介质板, 介质板采为聚酰亚胺板, 介电常数为3.0, 电正切损耗为0.001Figure 3. Layout and numerical characterizations of the spatial-order meta-atoms in three different situations of (a) only circular SRRs along left slope, (b) square SRRs along right slope, and (c) both circular and square SRRs along both slopes. Here,
${\varphi _u}$ (${\varphi _v}$ ) and ru (rv) represent the reflection phase and amplitude for components along u(v) axis,${r_{yy}}$ (${r_{xx}}$ ) represent the reflection amplitude of the incident y(x)-polarized and reflected y(x)-polarized EM waves,${r_{xy}}$ (${r_{yx}}$ ) represent the reflection amplitude of the incident y(x)-polarized and reflected x(y)-polarized EM waves. (d) The Surface current distribution on SRRs at resonant frequencies of f = 6.8 and 5.5 GHz. (e) Layout and geometrical parameters of the finally designed spatial-order meta-atom. They are m = 18 mm, n = 6.7 mm, d = 0.1 mm, r2 = 3 mm, r1 = r2 – b1 = 2.4 mm, a1 = 6 mm, a2 = a1 – 2b2 = 4.8 mm and g = 0.44 mm. The yellow color indicates metallic copper while blue represents dielectric slab, which is a FR4 board with dielectric constant of 3.0 and tangent loss of 0.001.
图 4 最终设计的空间序构超表面单元在不同宽度开口圆环和方环谐振器的电磁波反射幅度仿真结果 (a) y极化和(b) x极化平面电磁波入射时的反射幅度随开口圆环宽度b1变化的关系; (c) y极化和(d) x极化平面电磁波入射时的反射幅度随开口方环宽度b2变化的关系
Figure 4. Finite-difference time-domain (FDTD) calculated reflection amplitude of the finally designed spatial-order meta-atom based on circular SRRs and square SRRs for different widths. Reflection amplitude as a function of (a), (b) b1 and (c), (d) b2 under (a), (c) y-polarized and (b), (d) x-polarized plane wave of normal incidence.
图 5 最终设计空间序构超表面的电磁波反射幅度在不同折叠角度β下的仿真结果 (a), (b) y极化与(c), (d) x极化平面电磁波入射时的反射幅度
Figure 5. FDTD calculated reflection amplitude of EM waves of the finally designed spatial-order metasurfaces at different folding angles β: the reflection amplitude of the incident (a), (b) y-polarized and (c), (d) x-polarized EM waves.
图 6 实验表征及不同极化入射下的测试同极化和交叉极化系数幅度频谱 (a)样品制作过程; (b)实验测试环境; (c) y极化波入射与(d) x极化波入射时电磁波在不同折叠角度下的反射幅度
Figure 6. Experimental characterization and measured co-polarization and cross-polarization reflection amplitude spectrum under different polarizations: (a) The fabrication process of sample; (b) experimental setup; reflection amplitude at different folding angle β under (c) y-polarized and (d) x-polarized EM waves.
图 7 最终设计的空间序构超表面在不同折叠角度下的(a)泊松比和(b)相对密度
Figure 7. (a) Poisson’s ratio and (b) relative density of the finally designed spatial-order metasurfaces as a function of different folding angles.
-
[1] 梅中磊, 张黎, 崔铁军 2016 科技导报 34 27
Google Scholar
Mei Z L, Zhang L, Cui T J 2016 Science & Technology Review 34 27
Google Scholar
[2] 王彦朝, 许河秀, 王朝辉, 王明照, 王少杰 2020 69 134101
Google Scholar
Wang Y Z, Xu H X, Wang C H, Wang M Z, Wang S J 2020 Acta Phys. Sin. 69 134101
Google Scholar
[3] Xu H X, Sun S, Tang S, Ma S, He Q, Wang G M, Cai T, Li H P, Zhou L 2016 Sci. Rep. 6 27503
Google Scholar
[4] Xu H X, Ma S, Luo W, Cai T, Sun S, He Q, Zhou L 2016 Appl. Phys. Lett. 109 193506
Google Scholar
[5] Xu H X, Tang S, Ma S, Luo W, Cai T, Sun S, He Q, Zhou L 2016 Sci. Rep. 6 38255
Google Scholar
[6] Zheng G, Mühlenbernd H, Kenney M, Li G, Zentgraf T, Zhang S 2015 Nat. Nanotechnol. 10 308
Google Scholar
[7] Wang C, Xu H X, Wang Y, Zhu S, Wang C, Mao R 2020 J. Phys. D: Appl. Phys. 53 365001
Google Scholar
[8] 李晓楠, 周璐, 赵国忠 2019 68 238101
Google Scholar
Li X N, Zhou L, Zhao G Z 2019 Acta Phys. Sin. 68 238101
Google Scholar
[9] 李勇峰, 张介秋, 屈绍波, 王甲富, 吴翔, 徐卓, 张安学 2015 64 124102
Google Scholar
Li Y F, Zhang J Q, Qu S B, Wang J F, Wu X, Xu Z, Zhang A X 2015 Acta Phys. Sin. 64 124102
Google Scholar
[10] 郭文龙, 王光明, 李海鹏, 侯海生 2016 65 074101
Google Scholar
Guo W L, Wang G M, Li H P, Hou H S 2016 Acta Phys. Sin. 65 074101
Google Scholar
[11] 于惠存, 曹祥玉, 高军, 杨欢欢, 韩江枫, 朱学文, 李桐 2018 67 224101
Google Scholar
Yu H C, Cao X Y, Gao J, Yang H H, Han J F, Zhu X W, Li T 2018 Acta Phys. Sin. 67 224101
Google Scholar
[12] 范亚, 屈绍波, 王甲富, 张介秋, 冯明德, 张安学 2015 64 184101
Google Scholar
Fan Y, Qu S B, Wang J F, Zhang J Q, Feng M D, Zhang A X 2015 Acta Phys. Sin. 64 184101
Google Scholar
[13] Wei Z, Cao Y, Fan Y, Yu X, Li H 2011 Appl. Phys. Lett. 99 221907
Google Scholar
[14] Feng M, Wang J, Ma H, Mo W, Ye H, Qu S 2013 J. Appl. Phys. 114 074508
Google Scholar
[15] Chen H, Wang J, Ma H, Qu S, Xu Z, Zhang A, Yan M, Li Y 2014 J. Appl. Phys. 115 154504
Google Scholar
[16] Shi H, Zhang A, Zheng S, Li J, Jiang Y 2014 Appl. Phys. Lett. 104 034102
Google Scholar
[17] Yin J. Y, Wan X, Zhang Q, Cui T J 2015 Sci. Rep. 5 12476
Google Scholar
[18] Chen M, Chang L, Gao X, Chen H, Wang C, Xiao X, Zhao D 2017 IEEE Photonics J. 9 4601011
[19] Yu Y, Xiao F, He C, Jin R, Zhu W 2020 Opt. Express. 28 11797
Google Scholar
[20] Wang M, Zhai Z 2020 Front. Phys. 8 527394
Google Scholar
[21] Kong X, Wang Q, Jiang S, Kong L, Yuan J, Yan X, Wang X, Zhao X 2020 Sci. Rep. 10 17843
Google Scholar
[22] Chambers B 1999 Smart Mater. Struct. 8 64
Google Scholar
[23] Chen K, Feng Y, Monticone F, Zhao J, Zhu B, Jiang T, Zhang L, Kim Y, Ding X, Zhang S, Alù A, Qiu C W 2017 Adv. Mater. 29 1606422
Google Scholar
[24] Li L, Cui T J, Ji W, Liu S, Ding J, Wan X, Li Y B, Jiang M, Qiu C W, Zhang S 2017 Nat. Commun. 8 197
Google Scholar
[25] Cui T J, Qi M Q, Wan X, Zhao J, Cheng Q 2014 Light-Sci. & Appl. 3 e218
Google Scholar
[26] Tian J, Cao X, Gao J, Yang H, Han J, Yu H, Wang S, Jin R, Li T 2019 J. Appl. Phys. 125 135105
Google Scholar
[27] Zhang X G, Jiang W X, Jiang H L, Wang Q, Tian H W, Bai L, Luo Z J, Sun S, Luo Y, Qiu C W, Cui T J 2020 Nat. Electron. 3 165
Google Scholar
[28] Jiang W, Ma H, Feng M, Yan L, Wang J, Wang J, Qu S 2016 J. Phys. D: Appl. Phys. 49 315302
Google Scholar
[29] Jing L, Wang Z, Zheng B, Wang H, Yang Y, Shen L, Yin W, Li E, Chen H 2018 NPG Asia Mater. 10 888
Google Scholar
[30] Wang Z, Jing L, Yao K, Yang Y, Zheng B, Soukoulis C M, Chen H, Liu Y 2017 Adv. Mater. 29 1700412
Google Scholar
[31] Li M, Shen L, Jing L, Xu S, Zheng B, Lin X, Yang Y, Wang Z, Chen H 2019 Adv. Sci. 6 1901434
Google Scholar
[32] Le D H, Xu Y, Tentzeris M M, Lim S 2020 Extreme Mech. Lett. 36 100670
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 7007
- PDF Downloads: 270
- Cited By: 0
