-
The luminescence efficiency of conjugated polymers has been a central topic in the study of light emitting. The effect of disorder plays an important role in generating excitons after the conjugated polymers have been excited by photons. In this paper, by using the Su-Schriffer-Heeger model, which has been modified to include intrachain disorder and electron correlation, we investigate the effects of disorder on the photoexcitation, especially on the yield of excitons in a conjugated polymer chain. We adopt the multi-configurational time-dependent Hartree–Fock method to solve the multi-electron time-dependent Schrödinger equation and the Newtonian equation of motion for the lattice vibration. The results show that after the photoexcitation relaxation process, the products of the disordered polymer chain are qualitatively distinct from those of the pure polymer chain. While the pairs of polarons can be generated directly after the photoexcitation in a pure polymer chain, the disorder favors excitons as the products of the photoexcitation, and the yield of excitons depends crucially on the kind and strength of the disorder. Furthermore, the influences of the electron correlation and the conjugation length on the yield of excitons are also discussed. Specifically, we find that in the case of diagonal disorder, when the conjugation length is short and the diagonal disorder is weak, the excitons are mainly generated by the recombination of two lattice defects with a high yield of excitons which will be reduced as the conjugation length increases. The excitons tend to be generated directly with a low yield of excitons which is enlarged as the disorder gets stronger when the conjugation length is long or the diagonal disorder is strong. The on-site Coulomb repulsion favors the generation of excitons as well. The case of off-diagonal disorder is similar to that of diagonal disorder except that the on-site Coulomb potential favors the generation of excitons in the weak disorder regime but depresses the generation of excitons in the strong disorder regime. When both diagonal and off-diagonal disorders are considered, the yield of excitons is dominated by the off-diagonal disorder. We hope that our investigations can provide useful guidance and help for designing organic photoelectric materials and devices.
-
Keywords:
- conjugated polymer /
- exciton /
- polaron /
- disorder
[1] Shirakawa H, Louis E J, MacDiarmid A G, Chiang C K, Heeger A J 1977 J. Chem. Soc. Chem. Commun. 16 578
[2] Heeger A J 2001 Rev. Mod. Phys. 73 681
Google Scholar
[3] Friend R H, Gymer R W, Holmes A B, Burroughes J H, Marks R N, Taliani C, Dos Bradley D C, Santos D A D, Brédas J L, Lögdlund M, Salaneck W R 1999 Nature 397 121
Google Scholar
[4] Dodabalapur A, Katz H E, Torsi L, Haddon R C 1995 Science 269 1560
Google Scholar
[5] Yu G, Gao J, Hummelen J C, Wudl F, Heeger A J 1995 Science 270 1789
Google Scholar
[6] Onsager L 1938 Phys. Rev. 54 554
Google Scholar
[7] Scher H, Rackovsky S 1984 J. Chem. Phys. 81 1994
Google Scholar
[8] Leng J M, Jeglinski S, Wei X, Benner R E, Vardeny Z V, Guo F, Mazumdar S 1994 Phys. Rev. Lett. 72 156
Google Scholar
[9] Kersting R, Lemmer U, Deussen M, Bakker H J, Mahrt R F, Kurz H, Arkhipov V I, Bässler H, Göbel E O 1994 Phys. Rev. Lett. 73 1440
Google Scholar
[10] Barth S, Bässler H 1997 Phys. Rev. Lett. 79 4445
Google Scholar
[11] Graupner W, Cerullo G, Lanzani G, Nisoli M, List E J W, Leising G, De Silvestri S 1998 Phys. Rev. Lett. 81 3259
Google Scholar
[12] Clarke T M, Durrant J R 2010 Chem. Rev. 110 6736
Google Scholar
[13] Sariciftci N S 1998 Primary Photoexcitations in Conjugated Polymers: Molecular Exciton Versus Semiconductor Band Model (Singapore: World Scientific) pp20−50
[14] Moses D, Okumoto H, Lee C H, Heeger A J, Ohnishi T, Noguchi T 1996 Phys. Rev. B 54 4748
[15] Moses D, Dogariu A, Heeger A J 2000 Chem. Phys. Lett. 316 356
Google Scholar
[16] Ruseckas A, Theander M, Andersson M R, Svensson M, Prato M, Inganäs O, Sundström V 2000 Chem. Phys. Lett. 322 136
Google Scholar
[17] Miranda P B, Moses D, Heeger A J 2001 Phys. Rev. B 64 081201(R
Google Scholar
[18] Moses D, Dogariu A, Heeger A J 2000 Phys. Rev. B 61 9373
Google Scholar
[19] De Sio A, Troiani F, Maiuri M, Réhault J, Sommer E, Lim J, Huelga S F, Plenio M B, Rozzi C A, Cerullo G, Molinari E, Lienau C 2016 Nat. Comm. 7 13742
Google Scholar
[20] Wang R, Yao Y, Zhang C F, Zhang Y D, Bin H J, Xue L W, Zhang Z G, Xie X Y, Ma H B, Wang X Y, Li Y F, Xiao M 2019 Nat. Commun. 10 398
Google Scholar
[21] Dong Y F, Nikolis V C, Talnack F, Chin Y C, Benduhn J, Londi G, Kublitski J, Zheng X J, Mannsfeld S C B, Spoltore D, Muccioli L, Li J, Blase X, Beljonne D, Kim J S, Bakulin A A, D′Avino G, Durrant J R, Vandewal K 2020 Nat. Commun. 11 4617
Google Scholar
[22] An Z, Wu C Q, Sun X 2004 Phys. Rev. Lett. 93 216407
Google Scholar
[23] Jaiswal M, Menon R 2006 Polym. Int. 55 1371
Google Scholar
[24] Coropceanu V, Cornil J, da Silva Filho D A, Olivier Y, Silbey R, Brédas J L 2007 Chem. Rev. 107 926
Google Scholar
[25] Shinar J, Shinar R 2008 J. Phys. D: Appl. Phys. 41 133001
Google Scholar
[26] Wang Y D, Zhang X G, Meng Y, Di B, Zhang Y L, An Z 2017 Org. Electron. 49 286
Google Scholar
[27] Sun Z, Li S, Xie S J, An Z 2019 J. Chem. Phys. C 123 21336
Google Scholar
[28] Menon A, Dong H P, Niazimbetova Z I, Rothberg L J, Galvin M E 2002 Chem. Mater. 14 3668
Google Scholar
[29] Konezny S J, Rothberg L J, Galvin M E, Smith D L 2010 Appl. Phys. Lett. 97 143305
Google Scholar
[30] Yuan X J, Dong X F, Li D M, Liu D S 2011 J. Chem. Phys. 134 244901
Google Scholar
[31] Yuan X J, Li D M, Yin S, Gao K, Cui B, Liu D S 2012 Org. Electron. 13 2094
Google Scholar
[32] Sariciftci N S, Heeger A J 1997 Handbook Organic Conductive Molecules Polymers (New York: Wiley) pp413–455
[33] Li X X, Hou D, Chen G 2018 Org. Electron. 54 245
Google Scholar
[34] Wang Y D, Liu J J, Wang X R, Di B, Meng Y 2018 Europhys. Lett. 123 37003
Google Scholar
[35] Miranda R P, Fisher A J, Stella L, Horsfield A P 2011 J. Chem. Phys. 134 244101
Google Scholar
[36] Miranda R P, Fisher A J, Stella L, Horsfield A P 2011 J. Chem. Phys. 134 244102
Google Scholar
[37] Su W P, Schrieffer J R, Heeger A J 1979 Phys. Rev. Lett. 42 1698
Google Scholar
[38] Su W P, Schrieffer J R, Heeger A J 1980 Phys. Rev. B 22 2099
Google Scholar
[39] Brazovskii S A, Kirova N N 1981 Sov. Phys. JETP Lett. 33 4
[40] Heeger A J, Kivelson S, Schrieffer J R, Su W P 1988 Rev. Mod. Phys. 60 781
Google Scholar
[41] Meng Y, Liu X J, Di B, An Z 2009 J. Chem. Phys. 131 244502
Google Scholar
[42] Sun Z, Li Y, Xie S J, An Z, Liu D S 2009 Phys. Rev. B 79 201310(R
Google Scholar
[43] Sun Z, Li Y, Gao K, Liu D S, An Z, Xie S J 2010 Org. Electron. 11 279
Google Scholar
-
图 1 加入对角无序后, 电子
$ \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ 激发后晶格交错序参量$ \delta_i = (-1)^i(u_{i+1}-u_i)/2 $ 随时间的演化 (a)$ L = 64, \; \sigma_\varepsilon = 0.01 $ eV; (b)$ L = 128, \; \sigma_\varepsilon = 0.10 $ eV, 插图显示了$ \varepsilon_2^{\rm v} $ 上一个电子激发到导带$ \varepsilon_2^{\rm c} $ 后, 电子初始占据状态的示意图Figure 1. Dynamical evolution of the staggered bond order parameter
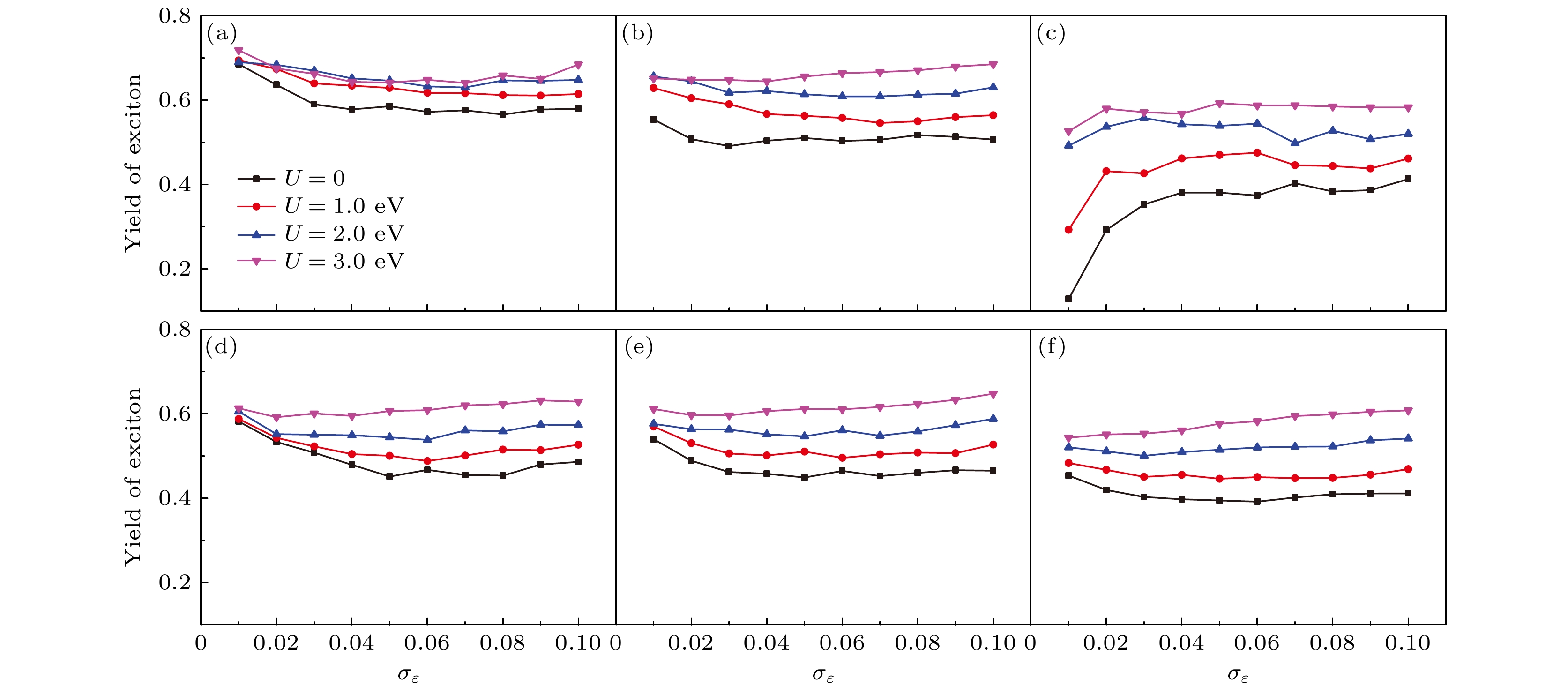
$ \delta_{i} = (-1)^{i}(u_{i+1}-u_{i})/2 $ with time for photoexcitation$ \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ : (a)$ L = 64, \; \sigma_\varepsilon = 0.01 $ eV; (b)$ L = 128, \; \sigma_\varepsilon = 0.10 $ eV. The inset shows the initial electronic levels for a photoexcitation process of$ \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ .
图 2 激子的产率在不同链长和电子关联下随着对角无序强度的变化 (a)
$ L = 64, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ ; (b)$ L = 96, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ ; (c)$L = $ $ 128, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c}$ ; (d)$ L = 64, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ ; (e)$ L = 96, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ ; (f)$ L = 128, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ Figure 2. Dependence of the yield of the exciton on the strength of diagonal disorder: (a)
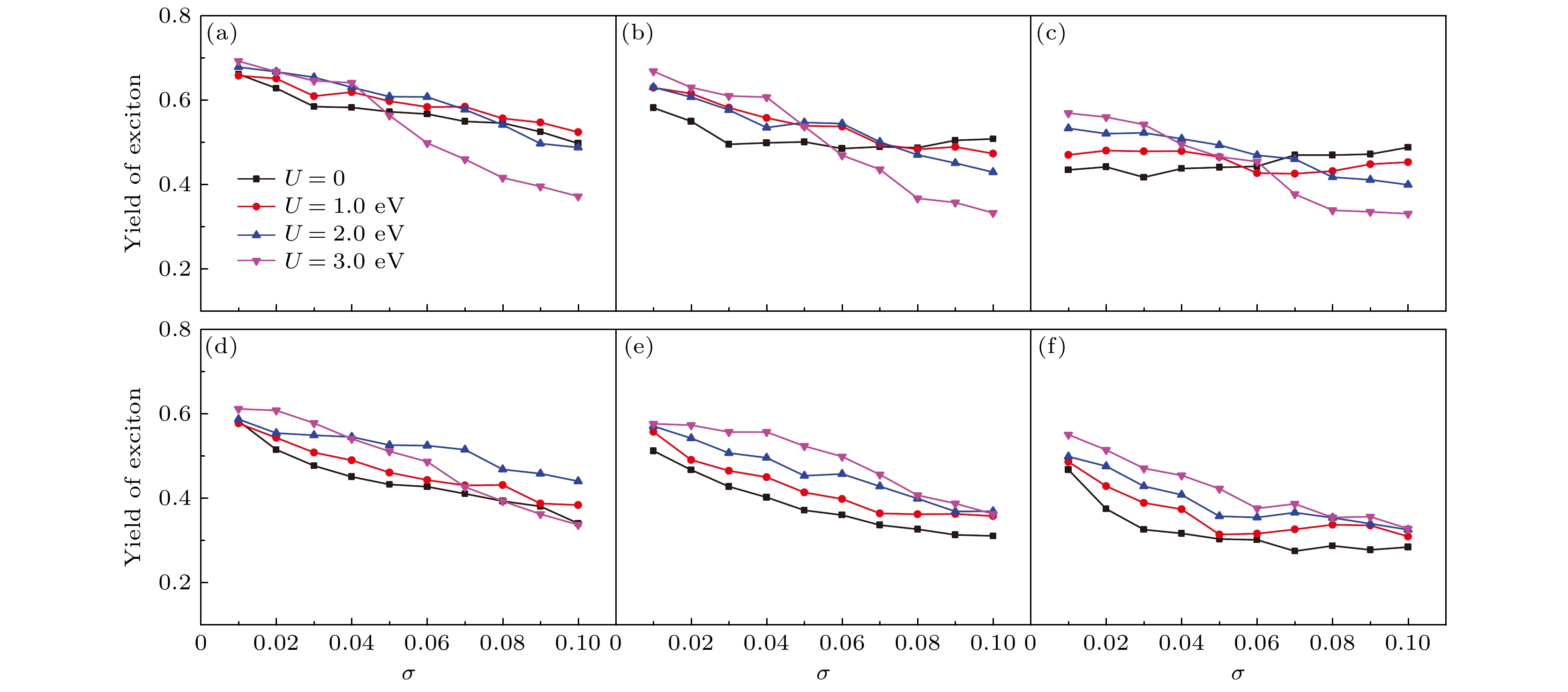
$ L = 64, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ ; (b)$ L = 96, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ ; (c)$ L = 128, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ ; (d)$ L = 64, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ ; (e)$ L = 96, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ ; (f)$ L = 128, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ .
图 3 激子的产率在不同链长和电子关联下随着非对角无序强度的变化 (a)
$ L = 64, \; \varepsilon_2^{\upsilon}\rightarrow\varepsilon_2^c $ ; (b)$ L = 96, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ ; (c)$L = 128, $ $ \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c}$ ; (d)$ L = 64, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ ; (e)$ L = 96, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ ; (f)$ L = 128, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ Figure 3. Dependence of the yield of the exciton on the strength of off-diagonal disorder: (a)
$ L = 64, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ ; (b)$L = $ $ 96, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c}$ ; (c)$ L = 128, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ ; (d)$ L = 64, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ ; (e)$ L = 96, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ ; (f)$ L = 128, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ .
图 4 两种无序同时存在时, 激子的产率在不同链长和电子关联下随着无序强度的变化 (a)
$ L = 64, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ ; (b)$L = 96, $ $ \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c}$ ; (c)$ L = 128, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ ; (d)$ L = 64, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ ; (e)$ L = 96, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ ; (f)$ L = 128, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ Figure 4. Dependence of the yield of the exciton on the strength of both diagonal and off-diagonal disorder: (a)
$ L = 64, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ ; (b)$ L = 96, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ ; (c)$ L = 128, \; \varepsilon_2^{\rm v}\rightarrow\varepsilon_2^{\rm c} $ ; (d)$ L = 64, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ ; (e)$ L = 96, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c} $ ; (f)$L = 128, \; \varepsilon_4^{\rm v}\rightarrow\varepsilon_4^{\rm c}$ . -
[1] Shirakawa H, Louis E J, MacDiarmid A G, Chiang C K, Heeger A J 1977 J. Chem. Soc. Chem. Commun. 16 578
[2] Heeger A J 2001 Rev. Mod. Phys. 73 681
Google Scholar
[3] Friend R H, Gymer R W, Holmes A B, Burroughes J H, Marks R N, Taliani C, Dos Bradley D C, Santos D A D, Brédas J L, Lögdlund M, Salaneck W R 1999 Nature 397 121
Google Scholar
[4] Dodabalapur A, Katz H E, Torsi L, Haddon R C 1995 Science 269 1560
Google Scholar
[5] Yu G, Gao J, Hummelen J C, Wudl F, Heeger A J 1995 Science 270 1789
Google Scholar
[6] Onsager L 1938 Phys. Rev. 54 554
Google Scholar
[7] Scher H, Rackovsky S 1984 J. Chem. Phys. 81 1994
Google Scholar
[8] Leng J M, Jeglinski S, Wei X, Benner R E, Vardeny Z V, Guo F, Mazumdar S 1994 Phys. Rev. Lett. 72 156
Google Scholar
[9] Kersting R, Lemmer U, Deussen M, Bakker H J, Mahrt R F, Kurz H, Arkhipov V I, Bässler H, Göbel E O 1994 Phys. Rev. Lett. 73 1440
Google Scholar
[10] Barth S, Bässler H 1997 Phys. Rev. Lett. 79 4445
Google Scholar
[11] Graupner W, Cerullo G, Lanzani G, Nisoli M, List E J W, Leising G, De Silvestri S 1998 Phys. Rev. Lett. 81 3259
Google Scholar
[12] Clarke T M, Durrant J R 2010 Chem. Rev. 110 6736
Google Scholar
[13] Sariciftci N S 1998 Primary Photoexcitations in Conjugated Polymers: Molecular Exciton Versus Semiconductor Band Model (Singapore: World Scientific) pp20−50
[14] Moses D, Okumoto H, Lee C H, Heeger A J, Ohnishi T, Noguchi T 1996 Phys. Rev. B 54 4748
[15] Moses D, Dogariu A, Heeger A J 2000 Chem. Phys. Lett. 316 356
Google Scholar
[16] Ruseckas A, Theander M, Andersson M R, Svensson M, Prato M, Inganäs O, Sundström V 2000 Chem. Phys. Lett. 322 136
Google Scholar
[17] Miranda P B, Moses D, Heeger A J 2001 Phys. Rev. B 64 081201(R
Google Scholar
[18] Moses D, Dogariu A, Heeger A J 2000 Phys. Rev. B 61 9373
Google Scholar
[19] De Sio A, Troiani F, Maiuri M, Réhault J, Sommer E, Lim J, Huelga S F, Plenio M B, Rozzi C A, Cerullo G, Molinari E, Lienau C 2016 Nat. Comm. 7 13742
Google Scholar
[20] Wang R, Yao Y, Zhang C F, Zhang Y D, Bin H J, Xue L W, Zhang Z G, Xie X Y, Ma H B, Wang X Y, Li Y F, Xiao M 2019 Nat. Commun. 10 398
Google Scholar
[21] Dong Y F, Nikolis V C, Talnack F, Chin Y C, Benduhn J, Londi G, Kublitski J, Zheng X J, Mannsfeld S C B, Spoltore D, Muccioli L, Li J, Blase X, Beljonne D, Kim J S, Bakulin A A, D′Avino G, Durrant J R, Vandewal K 2020 Nat. Commun. 11 4617
Google Scholar
[22] An Z, Wu C Q, Sun X 2004 Phys. Rev. Lett. 93 216407
Google Scholar
[23] Jaiswal M, Menon R 2006 Polym. Int. 55 1371
Google Scholar
[24] Coropceanu V, Cornil J, da Silva Filho D A, Olivier Y, Silbey R, Brédas J L 2007 Chem. Rev. 107 926
Google Scholar
[25] Shinar J, Shinar R 2008 J. Phys. D: Appl. Phys. 41 133001
Google Scholar
[26] Wang Y D, Zhang X G, Meng Y, Di B, Zhang Y L, An Z 2017 Org. Electron. 49 286
Google Scholar
[27] Sun Z, Li S, Xie S J, An Z 2019 J. Chem. Phys. C 123 21336
Google Scholar
[28] Menon A, Dong H P, Niazimbetova Z I, Rothberg L J, Galvin M E 2002 Chem. Mater. 14 3668
Google Scholar
[29] Konezny S J, Rothberg L J, Galvin M E, Smith D L 2010 Appl. Phys. Lett. 97 143305
Google Scholar
[30] Yuan X J, Dong X F, Li D M, Liu D S 2011 J. Chem. Phys. 134 244901
Google Scholar
[31] Yuan X J, Li D M, Yin S, Gao K, Cui B, Liu D S 2012 Org. Electron. 13 2094
Google Scholar
[32] Sariciftci N S, Heeger A J 1997 Handbook Organic Conductive Molecules Polymers (New York: Wiley) pp413–455
[33] Li X X, Hou D, Chen G 2018 Org. Electron. 54 245
Google Scholar
[34] Wang Y D, Liu J J, Wang X R, Di B, Meng Y 2018 Europhys. Lett. 123 37003
Google Scholar
[35] Miranda R P, Fisher A J, Stella L, Horsfield A P 2011 J. Chem. Phys. 134 244101
Google Scholar
[36] Miranda R P, Fisher A J, Stella L, Horsfield A P 2011 J. Chem. Phys. 134 244102
Google Scholar
[37] Su W P, Schrieffer J R, Heeger A J 1979 Phys. Rev. Lett. 42 1698
Google Scholar
[38] Su W P, Schrieffer J R, Heeger A J 1980 Phys. Rev. B 22 2099
Google Scholar
[39] Brazovskii S A, Kirova N N 1981 Sov. Phys. JETP Lett. 33 4
[40] Heeger A J, Kivelson S, Schrieffer J R, Su W P 1988 Rev. Mod. Phys. 60 781
Google Scholar
[41] Meng Y, Liu X J, Di B, An Z 2009 J. Chem. Phys. 131 244502
Google Scholar
[42] Sun Z, Li Y, Xie S J, An Z, Liu D S 2009 Phys. Rev. B 79 201310(R
Google Scholar
[43] Sun Z, Li Y, Gao K, Liu D S, An Z, Xie S J 2010 Org. Electron. 11 279
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4502
- PDF Downloads: 55
- Cited By: 0
