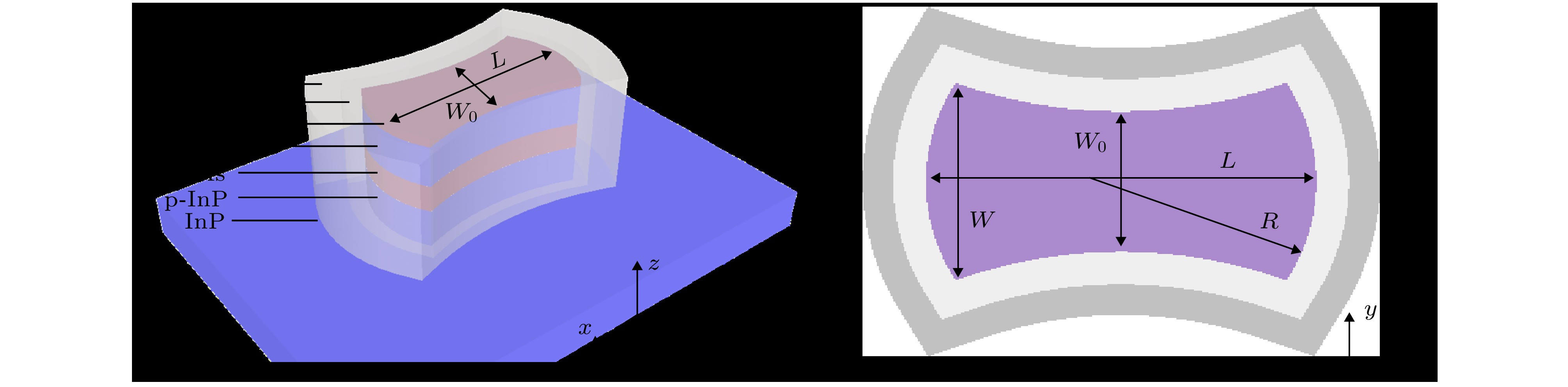
-
Metallic semiconductor nanolaser, as an ultra-small light source, has been increasingly attractive to researchers in last decade. It can have wide potential applications such as in photonic integrated circuits, on-chip interconnect, optical communications,etc. One obstacle to miniaturization of the laser size is that the loss increases rapidly with the cavity volume decreasing. In previous studies, a type of Fabry-Perot cavity with capsule-shaped structure was investigated and demonstrated both numerically and experimentally, showing that its cavity loss is reduced dramatically in contrast to the scenario of conventional rectangular cavities. However, when the cavity size is reduced down to nanoscale, capsule-shaped structure surfers high loss. To overcome this difficulty, in this paper, a novel type of double-concave cavity structure for metallic semiconductor nanolaser in a 1.55 μm wavelength range is proposed and numerically studied. The proposed structure consists of InGaAs/InP waveguide structure encapsulated by metallic clad, and has a cylindrical reflection end face and concave curved sidewalls. The cylindrical reflection end face can push the resonant mode into the cavity center and reduce the optical field overlap with metallic sidewalls, which can reduce the metallic loss. The curved-sidewalls topologically reduce the electric field component perpendicular to the sidewalls, and thus reducing the plasmonic loss. By optimizing the waist width of the double-concave cavity structure, the radiation loss can be effectively reduced, resulting in the improvement of cavity quality factor and the decrease of threshold current. Finite-difference time-domain simulations are conducted to investigate the properties of the proposed cavity structures such as resonant mode distribution, cavity quality factor, confinement factor, threshold gain and threshold current in this paper. The numerical results show that the double-concave cavity laser with cavity volume as small as 0.258 λ3 increases 24.8% of cavity quality factor and reduces 67.5% of threshold current, compared with the conventional capsule-shaped one, demonstrating an effective improvement of metallic nanolaser. With those advantages, the proposed structure can be used for realizing the ultra-small metallic semiconductor nanolasers and relevant applications. -
Keywords:
- semiconductor laser /
- microcavity /
- surface plasmon polaritons
[1] Miller D A B 2017 J. Lightwave Technol. 35 346
Google Scholar
[2] Smit M, Tol J V D, Hill M 2012 Laser Photonics Rev. 6 1
Google Scholar
[3] Roelkens G, Liu L, Liang D, Jones R, Fang A, Koch B, Bowers J 2010 Laser Photonics Rev. 4 751
Google Scholar
[4] Huang K C Y, Seo M K, Sarmiento T, Huo Y, Harris J S, Brongersma M L 2014 Nat. Photonics 8 244
Google Scholar
[5] Hill M T, Gather M C 2014 Nat. Photonics 8 908
Google Scholar
[6] McCall S L, Levi A F J, Slusher R E, Pearton S J, Logan R A 1992 Appl. Phys. Lett. 60 289
Google Scholar
[7] Park H G, Kim S H, Kwon S H, Ju Y G, Yang J K, Baek J H, Kim S B, Lee Y H 2004 Science 305 1444
Google Scholar
[8] Hill M T, Oei Y S, Smalbrugge B, Zhu Y, de Vries T, van Veldhoven P J, van Otten F W M, Eijkemans T J, Turkiewicz J P, de Waardt H, Geluk E J, Kwon S H, Lee Y H, N€otzel R., Smit M K 2007 Nature Photon. 1 589
Google Scholar
[9] Lee J H, Khajavikhan M, Simic A, Gu Q, Bondarenko O, Slutsky B, Nezhad M P, Fainman Y 2011 Opt. Express 19 21524
Google Scholar
[10] Khajavikhan M, Simic A, Katz M, Lee J H, Slutsky B, Mizrahi A, Lomakin V, Fainman Y 2012 Nature 482 204
Google Scholar
[11] Guo C C, Xiao J L, Yang Y D, Huang Y Z 2014 J. Opt. Soc. Am. B 31 865
Google Scholar
[12] Kwon S H, Kang J H, Seassal C, Kim S K, Regreny P, Lee Y H, Lieber C M, Park H G 2010 Nano Lett. 10 3679
Google Scholar
[13] Ding K, Ning C Z 2012 Light: Sci. Appl. 1 e20
Google Scholar
[14] Hill M T, Marell M, Leong E S P, Smalbrugge B, Zhu Y, Sun M, van Veldhoven P J, Geluk E J, Karouta F, Oei Y S, Nötzel1 R, Ning C Z, Smit M K 2009 Opt. Express 17 11107
Google Scholar
[15] Ding K, Liu Z C, Yin L J, Hill M T, Marel M J H, van Veldhoven P J, Nöetzel R, Ning C Z 2012 Phys. Rev. B 85 041301
[16] Ding K, Hill M T, Liu Z C, Yin L J, van Veldhoven P J, Ning C Z 2013 Opt. Express 21 4728
Google Scholar
[17] Zhang B, Okimoto T, Tanemura T, Nakano Y 2014 Jpn. J. Appl. Phys. 53 112703
Google Scholar
[18] Zhang B, Chieda K, Okimoto T, Tanemura T, Nakano Y 2016 Phys. Status Solidi A 213 965
Google Scholar
[19] Xiao Y, Taylor R J E, Yu C, Feng K, Tanemura T, Nakano Y 2017 Appl. Phys. Lett. 111 081107
Google Scholar
[20] Zhang B, Zhu K, Hao J, Wang B, Shen Z, Hu H 2018 IEEE Photon. J. 10 4502110
[21] Taflove A, Hagness S C 2005 Computational Electrodynamics: The Finite-Difference Time-Domain Method (Norwood: Artech House) pp354
[22] Palik E D 1985 Handbook of Optical Constants of Solids (NewYork: Academic) pp350
[23] Long H, Huang Y Z, Zou L X, Yang Y D, Lv X M, Ma X W, Xiao J L 2014 J. Lightwave Technol. 32 3192
Google Scholar
[24] Coldren L A, Corzine S W, Masanovic M L, 2012 Diode Lasers and Photonic Integrated Circuits, ed. K. Chang (New York: Wiley) pp55-70
[25] Coldren L A, Corzine S W, Masanovic M L, 2012 Diode Lasers and Photonic Integrated Circuits, ed. K. Chang (New York: Wiley) pp224
[26] Zielinski E, Schweizer H, Streubel K, Eisele H, Weimann G 1986 J. Appl. Phys. 59 2196
Google Scholar
[27] Zou Y, Osinski J S, Grodzinski P, Dapkus P D, Rideout W C, Shadin W F, Schlafer J, Crawford F D 1993 IEEE J. Quantum Electron. 29 1565
[28] Gu Q, Shane J, Vallini F, Wingad B, Smalley J S T, Frateschi N C, Fainman Y 2014 IEEE J. Quantum Electron. 50 499
Google Scholar
[29] Shane J, Gu Q, Vallini F, Wingad B, Smalley J S T, Frateschi N C, Fainman Y 2014 Proc. SPIE 8980 898027
[30] Ding K, Ning C Z 2013 Semicond. Sci. Technol. 28 124002
Google Scholar
[31] Karouta F 2014 J. Phys. D: Appl. Phys. 47 233501
Google Scholar
[32] Kuttge M, Vesseur E J R, Verhoeven J, Lezec H J, Atwater H A, Polman A 2008 Appl. Phys. Lett. 93 113110
Google Scholar
-
图 1 双凹型金属半导体纳米激光器谐振腔示意图 (a)结构示意图; (b)俯视图
Figure 1. Schematic of double-concave cavity of metallic semiconductor nanolaser: (a) The structure; (b) top view of the double-concave cavity.
图 2 CSC型谐振腔(L = 700 nm, W = 520 nm) Q值与曲率半径R的关系
Figure 2. Q values of the capsule-shaped cavities (L = 700 nm, W = 520 nm) as functions of the radius of curvature R.
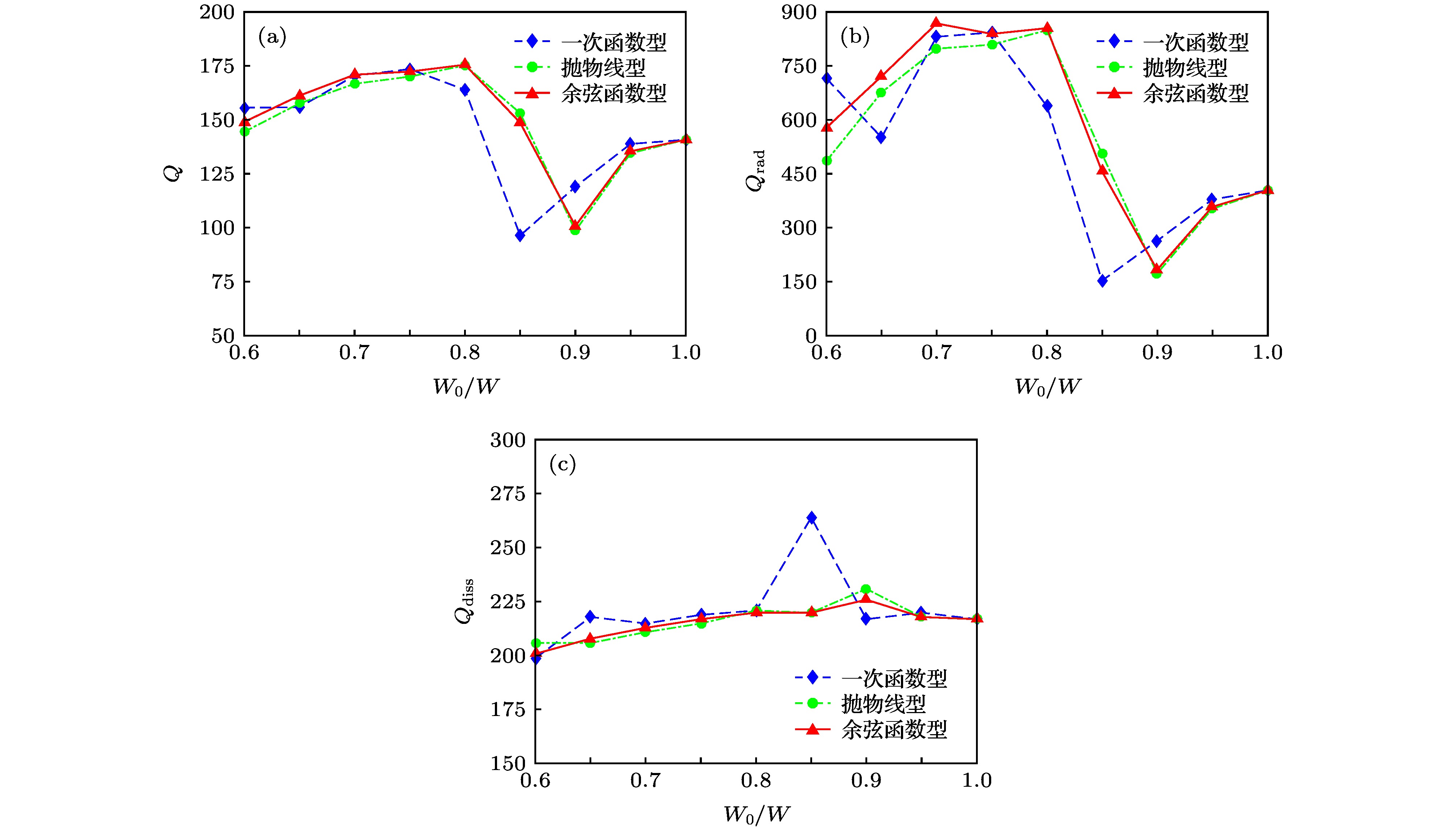
图 3 三种曲线侧壁的双凹型谐振腔(L = 700 nm, W = 520 nm, L/R = 1.43)的品质因子Q、辐射品质因子Qrad和耗散品质因子Qdiss与W0/W的关系 (a) Q; (b) Qrad; (c) Qdiss
Figure 3. Quality factors Q, radiation quality factors Qrad and dissipation quality factors Qdiss of three double-concave cavities with curved sidewalls (L = 700 nm, W = 520 nm, L/R = 1.43) as functions of the W0/W: (a) Q; (b) Qrad; (c) Qdiss.
图 4 不同谐振腔结构的谐振模式(TE模式)的归一化电场强度|E|2在穿过腔中心的xy、yz、xz平面的分布图 (a)— (c)为胶囊型腔; (d)— (f)为一次函数型腔; (g)— (i)为抛物线型腔; (j)—(l)为余弦函数型腔. 所有腔的几何参数详见表2
Figure 4. Normalized electric field intensity distribution |E|2 of the resonant mode (TE mode) in the xy-, yz- and xz-planes crossing the cavity center: (a)–(c) The capsule-shaped cavity; (d)–(f) the linear-function-shaped cavity; (g)–(i) the parabola-shaped cavity; (j)–(l) the cosine-shaped cavity. All the geometric parameters of the cavities are listed in Table 2 in detail.
图 5 三种曲线侧壁双凹型谐振腔(L = 700 nm, W = 520 nm, L/R = 1.43)的金属半导体纳米激光器的限制因子Γ、阈值增益gth和阈值电流Ith与W0/W的关系 (a) Γ; (b) gth; (c) Ith
Figure 5. The confinement factor Γ, threshold gain gth and threshold current Ith of the metallic semiconductor nanolasers with three double-concave cavities with curved sidewalls (L = 700 nm, W = 520 nm, L/R = 1.43) as functions of the W0/W: (a) Γ; (b) gth; (c) Ith.
表 1 双凹型谐振腔的侧壁曲线方程
Table 1. Curve equations of the sidewalls of the double-concave cavities
侧壁曲线类型 侧壁曲线方程 一次函数型 y = |a1x| + W0/2 抛物线型 y = a2x2 + W0/2 余弦函数型 y = a3cos(bx) + W/2 
DownLoad: CSV
表 2 四种谐振腔的金属半导体纳米激光器的几何参数和数值仿真结果
Table 2. Geometric parameters and simulation results of the metallic semiconductor nanolasers with four types of cavities.
参数 胶囊型 一次函数型 抛物线型 余弦函数型 L/nm 700 700 700 700 W/nm 520 520 520 520 W0/W 1.00 0.75 0.8 0.80 L/R 1.43 1.43 1.43 1.43 V/λ3 0.267 0.258 0.257 0.258 λ/nm 1564 1552 1550 1551 Q 141 174 175 176 Г 0.460 0.441 0.440 0.445 gth/cm–1 2190 1870 1850 1830 Ith/μA 800 290 280 260
DownLoad: CSV
-
[1] Miller D A B 2017 J. Lightwave Technol. 35 346
Google Scholar
[2] Smit M, Tol J V D, Hill M 2012 Laser Photonics Rev. 6 1
Google Scholar
[3] Roelkens G, Liu L, Liang D, Jones R, Fang A, Koch B, Bowers J 2010 Laser Photonics Rev. 4 751
Google Scholar
[4] Huang K C Y, Seo M K, Sarmiento T, Huo Y, Harris J S, Brongersma M L 2014 Nat. Photonics 8 244
Google Scholar
[5] Hill M T, Gather M C 2014 Nat. Photonics 8 908
Google Scholar
[6] McCall S L, Levi A F J, Slusher R E, Pearton S J, Logan R A 1992 Appl. Phys. Lett. 60 289
Google Scholar
[7] Park H G, Kim S H, Kwon S H, Ju Y G, Yang J K, Baek J H, Kim S B, Lee Y H 2004 Science 305 1444
Google Scholar
[8] Hill M T, Oei Y S, Smalbrugge B, Zhu Y, de Vries T, van Veldhoven P J, van Otten F W M, Eijkemans T J, Turkiewicz J P, de Waardt H, Geluk E J, Kwon S H, Lee Y H, N€otzel R., Smit M K 2007 Nature Photon. 1 589
Google Scholar
[9] Lee J H, Khajavikhan M, Simic A, Gu Q, Bondarenko O, Slutsky B, Nezhad M P, Fainman Y 2011 Opt. Express 19 21524
Google Scholar
[10] Khajavikhan M, Simic A, Katz M, Lee J H, Slutsky B, Mizrahi A, Lomakin V, Fainman Y 2012 Nature 482 204
Google Scholar
[11] Guo C C, Xiao J L, Yang Y D, Huang Y Z 2014 J. Opt. Soc. Am. B 31 865
Google Scholar
[12] Kwon S H, Kang J H, Seassal C, Kim S K, Regreny P, Lee Y H, Lieber C M, Park H G 2010 Nano Lett. 10 3679
Google Scholar
[13] Ding K, Ning C Z 2012 Light: Sci. Appl. 1 e20
Google Scholar
[14] Hill M T, Marell M, Leong E S P, Smalbrugge B, Zhu Y, Sun M, van Veldhoven P J, Geluk E J, Karouta F, Oei Y S, Nötzel1 R, Ning C Z, Smit M K 2009 Opt. Express 17 11107
Google Scholar
[15] Ding K, Liu Z C, Yin L J, Hill M T, Marel M J H, van Veldhoven P J, Nöetzel R, Ning C Z 2012 Phys. Rev. B 85 041301
[16] Ding K, Hill M T, Liu Z C, Yin L J, van Veldhoven P J, Ning C Z 2013 Opt. Express 21 4728
Google Scholar
[17] Zhang B, Okimoto T, Tanemura T, Nakano Y 2014 Jpn. J. Appl. Phys. 53 112703
Google Scholar
[18] Zhang B, Chieda K, Okimoto T, Tanemura T, Nakano Y 2016 Phys. Status Solidi A 213 965
Google Scholar
[19] Xiao Y, Taylor R J E, Yu C, Feng K, Tanemura T, Nakano Y 2017 Appl. Phys. Lett. 111 081107
Google Scholar
[20] Zhang B, Zhu K, Hao J, Wang B, Shen Z, Hu H 2018 IEEE Photon. J. 10 4502110
[21] Taflove A, Hagness S C 2005 Computational Electrodynamics: The Finite-Difference Time-Domain Method (Norwood: Artech House) pp354
[22] Palik E D 1985 Handbook of Optical Constants of Solids (NewYork: Academic) pp350
[23] Long H, Huang Y Z, Zou L X, Yang Y D, Lv X M, Ma X W, Xiao J L 2014 J. Lightwave Technol. 32 3192
Google Scholar
[24] Coldren L A, Corzine S W, Masanovic M L, 2012 Diode Lasers and Photonic Integrated Circuits, ed. K. Chang (New York: Wiley) pp55-70
[25] Coldren L A, Corzine S W, Masanovic M L, 2012 Diode Lasers and Photonic Integrated Circuits, ed. K. Chang (New York: Wiley) pp224
[26] Zielinski E, Schweizer H, Streubel K, Eisele H, Weimann G 1986 J. Appl. Phys. 59 2196
Google Scholar
[27] Zou Y, Osinski J S, Grodzinski P, Dapkus P D, Rideout W C, Shadin W F, Schlafer J, Crawford F D 1993 IEEE J. Quantum Electron. 29 1565
[28] Gu Q, Shane J, Vallini F, Wingad B, Smalley J S T, Frateschi N C, Fainman Y 2014 IEEE J. Quantum Electron. 50 499
Google Scholar
[29] Shane J, Gu Q, Vallini F, Wingad B, Smalley J S T, Frateschi N C, Fainman Y 2014 Proc. SPIE 8980 898027
[30] Ding K, Ning C Z 2013 Semicond. Sci. Technol. 28 124002
Google Scholar
[31] Karouta F 2014 J. Phys. D: Appl. Phys. 47 233501
Google Scholar
[32] Kuttge M, Vesseur E J R, Verhoeven J, Lezec H J, Atwater H A, Polman A 2008 Appl. Phys. Lett. 93 113110
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 9177
- PDF Downloads: 88
- Cited By: 0
