Numerical investigation on interaction mechanisms between flow field and electromagnetic field for nonequilibrium inductively coupled plasma

Yu Ming-Hao

doi:10.7498/aps.68.20190865

Abstract

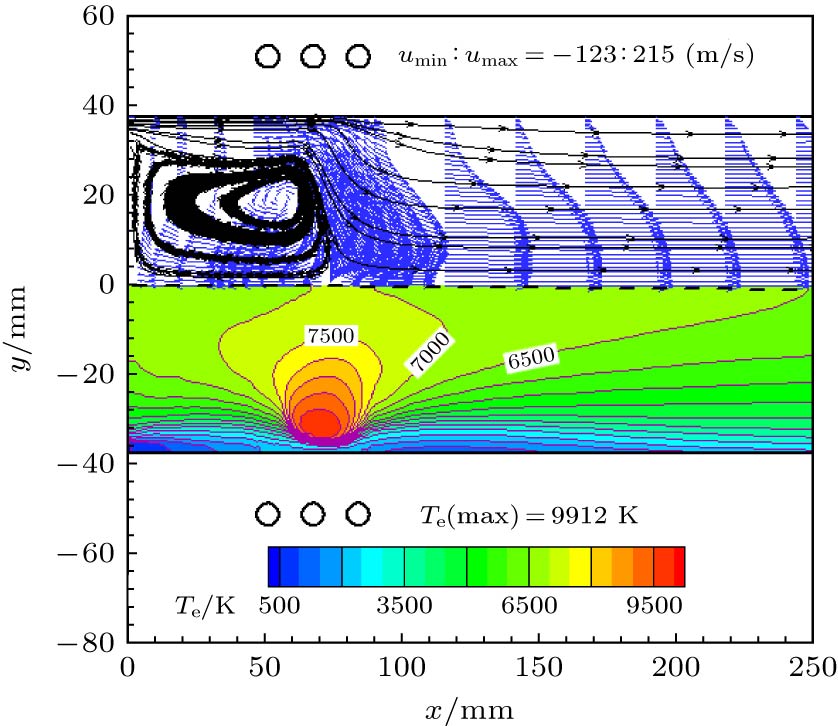
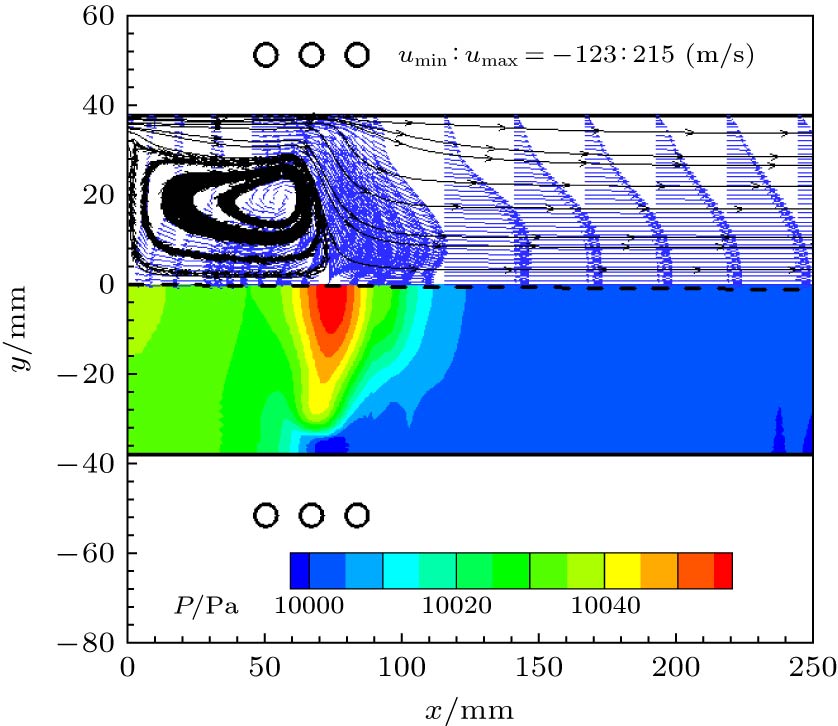
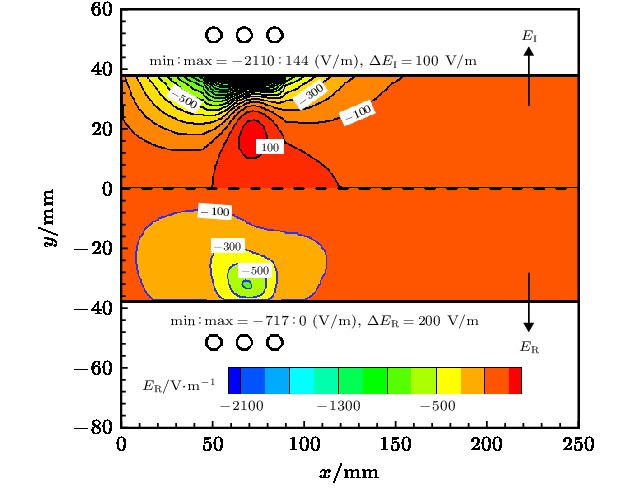
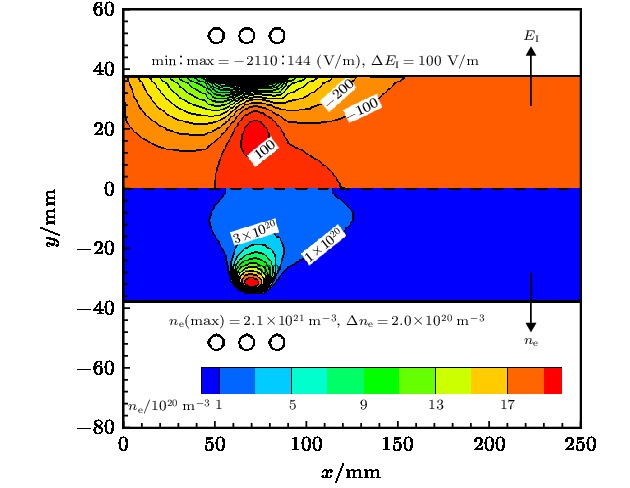
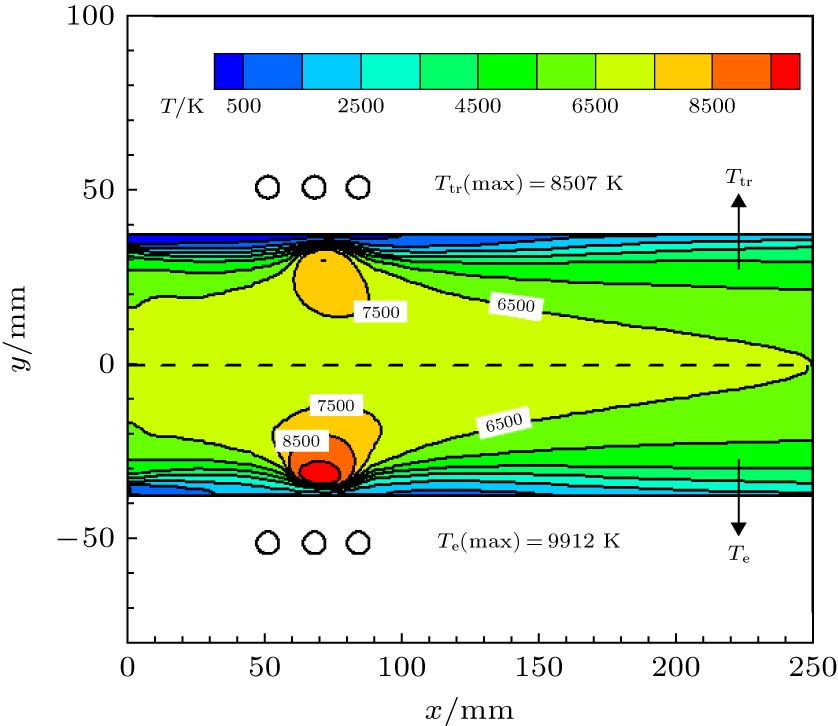
In this paper, the inductively coupled plasma (ICP) wind tunnel, which is widely used in the development of thermal protection system for reentry vehicle in the aerospace field, is studied. The distribution properties and the interaction mechanism of the flow field and electromagnetic field are investigated by numerically solving the multi-physics fields coupling among the flow field, electromagnetic field, thermodynamic field and turbulent field. In the numerical simulation, the thermochemical non-equilibrium plasma magneto-hydrodynamic model is used to accurately simulate the high-frequency discharge, Joule heating, energy conversion, and internal energy exchange of air ICP. Finally, the distribution of electron temperature, particle number density, Lorentz force, Joule heating rate, velocity, pressure and electric field strength of air plasma are obtained through the multi-physics field coupling calculation. The results show that the plasma flow is in a thermodynamic non-equilibrium state near the torch wall in the induction coil region and that the Lorentz force plays an important role in controlling the momentum transfer. A strong eddy flow occurs between the inlet and the second turn of the inductive coil. The eddy flow has a close relationship with the negative pressure gradient and the electromagnetic heating phenomenon in the induction coil region. The radial Lorentz force is always negative. This indicates that the free electrons are generated near the wall due to the fact that the skin effect are always subjected to a force making them move to the central axis of the ICP torch. The maximum value of the radial Lorentz force is 3.95 times higher than that of the axial Lorentz. This indicates that the momentum transfer is predominantly radial. The Joule heating effect of the air particles is also affected by the radial Lorentz force. The maximum value of E_I is 2.9 times larger than the real part of electric field, E_R. The positive E_I is generated by the free electrons inside the plasma. The number density of free electrons reaches a maximum value at a distance of 5.5 mm far from the inner wall surface of the torch below the second induction coil. 91% of N₂ are dissociated into atomic N near the central axis. The maximum electron and translational temperature simulated in this paper are 9921 K and 8507 K, respectively.

Keywords:

Authors and contacts

Yu Ming-Hao

School of Mechanical and Precision Instrument Engineering, Xi’an University of Technology, Xi’an 710048, China

Corresponding author: Yu Ming-Hao, ymh@xaut.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 11705143), the China Postdoctoral Science Foundation (Grant No. 2018M643814XB), and the Natural Science Basic Research Plan in Shaanxi Province of China (Grant No. 2018JQ1016).

FullText HTML : translate this paragraph

References

[1]	Suzuki T, Fujita K, Ando K, Sakai T 2008 J. Thermophys. Heat Tr. 22 382 Google Scholar
[2]	Suzuki T, Fujita K, Sakai T 2010 J. Thermophys. Heat Tr. 24 589 Google Scholar
[3]	Yamada K, Miyatani S, Maeno H, Abe T 2013 Proceedings of 57th Space Sciences and Technology Conference Tottori, Japan, October 9−11, 2013 p2
[4]	熊举坤, 黄海涛, 2011 化工环保 31 119 Google Scholar Xiong J K, Huang H T 2011 Environ. Prot. Chem. Indus. 31 119 Google Scholar
[5]	朱海龙, 叶高英, 程昌明, 杨发展, 童洪辉 2014 稀有金属材料与工程 43 2810 Zhu H L, Ye G Y, Cheng C M, Yang F Z, Tong H H 2014 Rare Metal Mat. Eng. 43 2810
[6]	Watanabe T, Okumiya H 2004 Sci. Technol. Adv. Mater. 5 639 Google Scholar
[7]	Miyatani S, 2014 Proceedings of the 58th Space Science and Technology Union Symposium Nagasaki, Japan, November 11−12, 2014 p2
[8]	Reed T B 1961 J. Appl. Phys. 32 821 Google Scholar
[9]	Mostaghimi J, Proulx P, Boulos M I 1987 J. Appl. Phys. 61 1753 Google Scholar
[10]	Lei F, Li X, Liu Y, Liu D, Yang M, Yu Y 2018 AIP Adv. 8 015003 Google Scholar
[11]	Yu M, Takahashi Y, Kihara H, Abe K, Yamada K, Abe T 2014 Plasma Sci. Technol. 16 930 Google Scholar
[12]	魏小龙, 徐浩军, 李建海, 林敏, 宋慧敏 2015 64 175201 Google Scholar Wei X L, Xu H J, Li J H, Lin M, Song H M 2015 Acta Phys. Sin 64 175201 Google Scholar
[13]	Xin Q, Su X, Alavi S, Wang B, Mostaghimi J 2018 Appl. Therm. Eng. 128 785 Google Scholar
[14]	Morsli M E, Proulx P, Gravelle D 2011 Plasma Sources Sci. Technol. 20 015016 Google Scholar
[15]	Wang Y N, Hou L J, Wang X 2002 Phys. Rev. Lett. 89 155001 Google Scholar
[16]	Gao F, Li X, Zhao S, Wang Y 2012 Chin. Phys. B 21 075203 Google Scholar
[17]	Yu M, Yamada K, Takahashi Y, Liu K, Zhao T 2016 Phys. Plasmas 23 123523 Google Scholar
[18]	朱海龙, 童洪辉, 杨发展, 程昌明, 叶高英 2013 高电压技术 39 1621 Google Scholar Zhu H L, Tong H H, Yang F Z, Cheng C M, Ye G Y 2013 High Volt. Eng. 39 1621 Google Scholar
[19]	Barnes R M, Nikdel S 1976 J. Appl. Phys. 47 3929 Google Scholar
[20]	Mostaghimi J, Boulos M I 1989 Plasma Chem. Plasma Proc. 9 25 Google Scholar
[21]	Punjabi S B, Joshi N K, Mangalvedekar H A, Lande B K, Das A K, Kothari D C 2012 Phys. Plasmas 19 012108 Google Scholar
[22]	Punjabi S, Barve D, Joshi N, Das A, Kothari D, Ganguli A, Sahasrabhude S, Joshi J 2019 Processes 7 133 Google Scholar
[23]	Mostaghimi J, Boulos M I 1990 J. Appl. Phys. 68 2643 Google Scholar
[24]	Stewart R A, Vitello P, Graves D B 1994 J. Vac. Sci. Technol. B 12 478 Google Scholar
[25]	Munafò A, Alfuhaid S A, Cambier J L, Panesi M 2015 J. Appl. Phys. 118 133303 Google Scholar
[26]	Zhang W, Lani A, Panesi M 2016 Phys. Plasmas 23 073512 Google Scholar
[27]	Degrez G, Abeele D V, Barbante P, Bottin B 2004 Int. J. Numer. Method H. 14 538 Google Scholar
[28]	Sumi T, Fujita K, Kurotaki T, Ito T, Mizuno M, Ishida K 2005 Trans. Japan Soc. Aero. Space Sci. 48 40 Google Scholar
[29]	Morsli M E, Proulx P 2007 J. Phys. D: Appl. Phys. 40 4810 Google Scholar
[30]	Yu M, Yamada K, Liu K, Zhao T 2019 AIP Adv. 9 015120 Google Scholar
[31]	Yu M, Liu K, Zhao T, Zhang Y 2016 J. Korean Phys. Soc. 69 1537 Google Scholar
[32]	Yu M, Kihara H, Abe K I, Takahashi Y 2015 J. Korean Phys. Soc. 66 1833 Google Scholar
[33]	Dunn M G, Kang S W 1973 Theoretical and Experimental Studies of Reentry Plasmas (Washionton DC: National Aeronautics and Space Administration) pp55−58
[34]	Morsli M E, Proulx P 2007 J. Phys. D: Appl. Phys. 40 380 Google Scholar
[35]	Park C, Jaffe R L, Partridge H 2001 J. Thermophys. Heat Tr. 15 76 Google Scholar
[36]	Lenzner S, Auweter-Kurtz M, Heiermann J, Sleziona P 2000 J. Thermophys. Heat Tr. 14 388 Google Scholar
[37]	Fujita K, Mizuno M, Ishida K, Ito T 2008 J. Thermophys. Heat Tr. 22 685 Google Scholar
[38]	Yos J M 1963 Transport Properties of Nitrogen, Hydrogen, Oxygen, and Air to 30000 K (Ohio, America: National Aeronautics and Space Administration) pp49−92
[39]	Gupta R N, Yos J M, Thompson R A, Lee K P 1990 A Review of Reaction Rates and Thermodynamic and Transport Properties for an 11-species Air Model for Chemical and Thermal Nonequilibrium Calculations to 30000 K (Verginia, America: National Aeronautics and Space Administration) pp3−69
[40]	Curtiss C F, Hirschfelder J O 1949 J. Chem. Phys. 17 550 Google Scholar
[41]	Devoto R S 1967 Phys. Fluids 10 2105 Google Scholar
[42]	Ghorui S, Das A K 2013 Phys. Plasmas 20 093504 Google Scholar
[43]	Laricchiuta A, Bruno D, Capitelli M, Catalfamo C, Celiberto R, Colonna G, Diomede P, Giordano D, Gorse C, Longo S, Pagano D, Pirani F 2009 Eur. Phys. J. D 54 607 Google Scholar
[44]	Park C 1990 Nonequilibrium Hypersonic Aerothermodynamics (New York: Wiley) p120
[45]	Park C 2004 J. Thermophys. Heat Tr. 18 527 Google Scholar
[46]	Millikan R C, White D R 1963 J. Chem. Phys. 39 3209 Google Scholar
[47]	Lazdinis S, Petrie S L 1974 Phys. Fluids 17 1539 Google Scholar
[48]	Park C 1984 Proceedings of the 19th Thermophysics Conference Snowmass, Colorado, America, June 25−28, 1984 pp3, 4
[49]	Vincenti W G, Kruger C H 1965 Introduction to Physical Gas Dynamics (Malabar: Krieger)
[50]	Kim M, Gülhan A, Boyd I D 2012 J. Thermophys. Heat Tr. 26 244 Google Scholar
[51]	Bourdon A, Vervisch P 1997 Phys. Rev. E 55 4634 Google Scholar
[52]	Appleton J, Bray K 1964 J. Fluid Mech. 20 659 Google Scholar
[53]	Gnoffo P A, Gupta R N, Shinn J L 1989 Conservation Equations and Physical Models for Hypersonic Air Flows in Thermal and Chemical Nonequilibrium (Hampton, America: National Aeronautics and Space Administration) pp10−12
[54]	Abe K, Kondoh T, Nagano Y 1994 Int. J. Heat Mass Trans. 37 139 Google Scholar

Cited By

图 1 ICP风洞系统结构布局

Figure 1. Schematic diagram of the ICP wind tunnel system.

DownLoad: Full-Size Img PowerPoint

图 2 ICP炬计算网格和几何结构　(a) 电磁场与流场计算网格; (b) 等离子体炬几何结构

Figure 2. Computational mesh and geometry of the inductively coupled plasma torch:　(a) Computational mesh of electromagnetic- and flow-field; (b) geometric construction of the ICP torch.

DownLoad: Full-Size Img PowerPoint

图 3 等离子体炬内气体流线和速度矢量(上半部分)以及电子温度云图(下半部分)分布

Figure 3. Distributions of streamlines and velocity vector (upper), and electron temperature (lower) in the torch.

DownLoad: Full-Size Img PowerPoint

图 4 等离子体流线(上半部分)和压力云图(下半部分)分布

Figure 4. Distributions of streamlines (upper) and pressure contour (lower).

DownLoad: Full-Size Img PowerPoint

图 5 轴向洛伦兹力(上半部分)和径向洛伦兹力(下半部分)的分布

Figure 5. Distributions of axial Lorentz force (upper) and radial Lorentz force (lower).

DownLoad: Full-Size Img PowerPoint

图 6 径向洛伦兹力(上半部分)和焦耳加热率(下半部分)的分布

Figure 6. Distributions of Joule heating rate(lower) and radial Lorentz force (upper)

DownLoad: Full-Size Img PowerPoint

图 7 电场强度分布(虚部E_I (上半部分)实部E_R(下半部分))

Figure 7. Distribution of electric-field intensity (imaginary part E_I (upper) and real part E_R (lower)).

DownLoad: Full-Size Img PowerPoint

图 8 电场强度E_i(上)和电子数密度n_e(下)的分布

Figure 8. Distribution of electric field intensity E_I (upper) and electron number density n_e (lower).

DownLoad: Full-Size Img PowerPoint

图 9 感应线圈中心(x = 68 mm)空气粒子径向摩尔分数分布

Figure 9. Mole fraction of air species along the radial direction at the coil center x = 68 mm.

DownLoad: Full-Size Img PowerPoint

图 10 等离子体炬内平动温度(上半部分)和电子温度(下半部分)分布云图

Figure 10. Distributions of translational (upper) and electronic temperatures (lower) in the torch.

DownLoad: Full-Size Img PowerPoint

表 1 空气化学反应模型

Table 1. Chemical reaction model of air.

	r	反应物		生成物	T_f	T_b	C_r	n	θ_r	文献
离解/复合反应 (S₁ = N₂, O₂, NO; S₂ = N, O; S₃ = N₂, O₂; S₄ = NO, N, O)	1—3	N₂ + S₁	$ \rightleftharpoons $	N + N + S₁	$\sqrt {{T_{{\rm{tr}}}}{T_{{\rm{vib}}}}} $	${T_{{\rm{tr}}}}$	7.0 × 10²¹	–1.60	113200	[35]
	4—5	N₂ + S₂	$ \rightleftharpoons $	N + N + S₂	$\sqrt {{T_{{\rm{tr}}}}{T_{{\rm{vib}}}}} $	${T_{{\rm{tr}}}}$	3.0 × 10²²	–1.60	113200	[35]
	6—8	O₂ + S₁	$ \rightleftharpoons $	O + O + S₁	$\sqrt {{T_{{\rm{tr}}}}{T_{{\rm{vib}}}}} $	${T_{{\rm{tr}}}}$	2.0 × 10²¹	–1.50	59500	[35]
	9—10	O₂ + S₂	$ \rightleftharpoons $	O + O + S₂	$\sqrt {{T_{{\rm{tr}}}}{T_{{\rm{vib}}}}} $	${T_{{\rm{tr}}}}$	1.0 × 10²²	–1.50	59500	[35]
	11—12	NO + S₃	$ \rightleftharpoons $	N + O + S₃	$\sqrt {{T_{{\rm{tr}}}}{T_{{\rm{vib}}}}} $	${T_{{\rm{tr}}}}$	5.0 × 10¹⁵	0.00	75500	[35]
	13—15	NO + S₄	$ \rightleftharpoons $	N + O + S₄	${T_{{\rm{tr}}}}$	${T_{{\rm{tr}}}}$	1.1 × 10¹⁷	0.00	75500	[35]
泽尔多维奇反应	16	N₂ + O	$ \rightleftharpoons $	NO + N	${T_{{\rm{tr}}}}$	${T_{{\rm{tr}}}}$	6.4 × 10¹⁷	–1.00	38400	[35]
泽尔多维奇反应	17	NO + O	$ \rightleftharpoons $	N + O₂	${T_{{\rm{tr}}}}$	${T_{{\rm{tr}}}}$	8.4 × 10¹²	0.00	19450	[35]
电量交换反应	18	N2 + N⁺	$ \rightleftharpoons $	N₂⁺ + N	${T_{{\rm{tr}}}}$	${T_{{\rm{tr}}}}$	1.0 × 10¹²	0.50	12200	[35]
	19	O₂⁺ + O	$ \rightleftharpoons $	O⁺ + O₂	${T_{{\rm{tr}}}}$	${T_{{\rm{tr}}}}$	4.0 × 10¹²	–0.09	18000	[35]
	20	NO⁺ + O	$ \rightleftharpoons $	NO + O⁺	${T_{{\rm{tr}}}}$	${T_{{\rm{tr}}}}$	3.63 × 10¹⁵	–0.60	13000	[33]
	21	O⁺ + N₂	$ \rightleftharpoons $	N₂⁺ + O	${T_{{\rm{tr}}}}$	${T_{{\rm{tr}}}}$	9.1 × 10¹¹	0.36	22800	[35]
	22	NO⁺ + O₂	$ \rightleftharpoons $	O₂⁺ + NO	${T_{{\rm{tr}}}}$	${T_{{\rm{tr}}}}$	2.4 × 10¹³	0.41	32600	[35]
	23	NO⁺ + N	$ \rightleftharpoons $	NO + N⁺	${T_{{\rm{tr}}}}$	${T_{{\rm{tr}}}}$	1.0 × 10¹⁹	–0.93	61000	[33]
	24	NO⁺ + O	$ \rightleftharpoons $	N⁺ + O₂	${T_{{\rm{tr}}}}$	${T_{{\rm{tr}}}}$	1.0 × 10¹²	0.50	77200	[35]
副电离反应	25	N + N	$ \rightleftharpoons $	N₂⁺ + e^–	${T_{{\rm{tr}}}}$	${T_{{\rm{tr}}}}$	4.4 × 10⁷	1.50	67500	[35]
	26	O + O	$ \rightleftharpoons $	O₂⁺ + e^–	${T_{{\rm{tr}}}}$	${T_{{\rm{tr}}}}$	7.1 × 10²	2.70	80600	[35]
	27	N + O	$ \rightleftharpoons $	NO⁺ + e^–	${T_{{\rm{tr}}}}$	${T_{{\rm{tr}}}}$	8.8 × 10⁸	1.00	31900	[35]
	28	O₂ + N₂	$ \rightleftharpoons $	NO + NO⁺ + e^–	$\sqrt {{T_{\rm{e}}}{T_{{\rm{vib}}}}} $	${T_{\rm{e}}}$	1.38 × 10²⁰	–1.84	141000	[33]
	29	N₂ + NO	$ \rightleftharpoons $	N₂ + NO⁺ + e^–	$\sqrt {{T_{\rm{e}}}{T_{{\rm{vib}}}}} $	${T_{\rm{e}}}$	2.20 × 10¹⁵	–0.35	108000	[33]
	30	O₂ + NO	$ \rightleftharpoons $	O₂ + NO⁺ + e^–	$\sqrt {{T_{\rm{e}}}{T_{{\rm{vib}}}}} $	${T_{\rm{e}}}$	8.80 × 10¹⁶	–0.35	108000	[33]
电子碰撞电离反应	31	N + e^–	$ \rightleftharpoons $	N⁺ + e^– + e^–	${T_{\rm{e}}}$	${T_{\rm{e}}}$	2.5 × 10³⁴	–3.82	168600	[35]
电子碰撞电离反应	32	O + e^–	$ \rightleftharpoons $	O⁺ + e^– + e^–	${T_{\rm{e}}}$	${T_{\rm{e}}}$	3.9 × 10³³	–3.78	158500	[35]

DownLoad: CSV

map

[1]	Suzuki T, Fujita K, Ando K, Sakai T 2008 J. Thermophys. Heat Tr. 22 382 Google Scholar
[2]	Suzuki T, Fujita K, Sakai T 2010 J. Thermophys. Heat Tr. 24 589 Google Scholar
[3]	Yamada K, Miyatani S, Maeno H, Abe T 2013 Proceedings of 57th Space Sciences and Technology Conference Tottori, Japan, October 9−11, 2013 p2
[4]	熊举坤, 黄海涛, 2011 化工环保 31 119 Google Scholar Xiong J K, Huang H T 2011 Environ. Prot. Chem. Indus. 31 119 Google Scholar
[5]	朱海龙, 叶高英, 程昌明, 杨发展, 童洪辉 2014 稀有金属材料与工程 43 2810 Zhu H L, Ye G Y, Cheng C M, Yang F Z, Tong H H 2014 Rare Metal Mat. Eng. 43 2810
[6]	Watanabe T, Okumiya H 2004 Sci. Technol. Adv. Mater. 5 639 Google Scholar
[7]	Miyatani S, 2014 Proceedings of the 58th Space Science and Technology Union Symposium Nagasaki, Japan, November 11−12, 2014 p2
[8]	Reed T B 1961 J. Appl. Phys. 32 821 Google Scholar
[9]	Mostaghimi J, Proulx P, Boulos M I 1987 J. Appl. Phys. 61 1753 Google Scholar
[10]	Lei F, Li X, Liu Y, Liu D, Yang M, Yu Y 2018 AIP Adv. 8 015003 Google Scholar
[11]	Yu M, Takahashi Y, Kihara H, Abe K, Yamada K, Abe T 2014 Plasma Sci. Technol. 16 930 Google Scholar
[12]	魏小龙, 徐浩军, 李建海, 林敏, 宋慧敏 2015 64 175201 Google Scholar Wei X L, Xu H J, Li J H, Lin M, Song H M 2015 Acta Phys. Sin 64 175201 Google Scholar
[13]	Xin Q, Su X, Alavi S, Wang B, Mostaghimi J 2018 Appl. Therm. Eng. 128 785 Google Scholar
[14]	Morsli M E, Proulx P, Gravelle D 2011 Plasma Sources Sci. Technol. 20 015016 Google Scholar
[15]	Wang Y N, Hou L J, Wang X 2002 Phys. Rev. Lett. 89 155001 Google Scholar
[16]	Gao F, Li X, Zhao S, Wang Y 2012 Chin. Phys. B 21 075203 Google Scholar
[17]	Yu M, Yamada K, Takahashi Y, Liu K, Zhao T 2016 Phys. Plasmas 23 123523 Google Scholar
[18]	朱海龙, 童洪辉, 杨发展, 程昌明, 叶高英 2013 高电压技术 39 1621 Google Scholar Zhu H L, Tong H H, Yang F Z, Cheng C M, Ye G Y 2013 High Volt. Eng. 39 1621 Google Scholar
[19]	Barnes R M, Nikdel S 1976 J. Appl. Phys. 47 3929 Google Scholar
[20]	Mostaghimi J, Boulos M I 1989 Plasma Chem. Plasma Proc. 9 25 Google Scholar
[21]	Punjabi S B, Joshi N K, Mangalvedekar H A, Lande B K, Das A K, Kothari D C 2012 Phys. Plasmas 19 012108 Google Scholar
[22]	Punjabi S, Barve D, Joshi N, Das A, Kothari D, Ganguli A, Sahasrabhude S, Joshi J 2019 Processes 7 133 Google Scholar
[23]	Mostaghimi J, Boulos M I 1990 J. Appl. Phys. 68 2643 Google Scholar
[24]	Stewart R A, Vitello P, Graves D B 1994 J. Vac. Sci. Technol. B 12 478 Google Scholar
[25]	Munafò A, Alfuhaid S A, Cambier J L, Panesi M 2015 J. Appl. Phys. 118 133303 Google Scholar
[26]	Zhang W, Lani A, Panesi M 2016 Phys. Plasmas 23 073512 Google Scholar
[27]	Degrez G, Abeele D V, Barbante P, Bottin B 2004 Int. J. Numer. Method H. 14 538 Google Scholar
[28]	Sumi T, Fujita K, Kurotaki T, Ito T, Mizuno M, Ishida K 2005 Trans. Japan Soc. Aero. Space Sci. 48 40 Google Scholar
[29]	Morsli M E, Proulx P 2007 J. Phys. D: Appl. Phys. 40 4810 Google Scholar
[30]	Yu M, Yamada K, Liu K, Zhao T 2019 AIP Adv. 9 015120 Google Scholar
[31]	Yu M, Liu K, Zhao T, Zhang Y 2016 J. Korean Phys. Soc. 69 1537 Google Scholar
[32]	Yu M, Kihara H, Abe K I, Takahashi Y 2015 J. Korean Phys. Soc. 66 1833 Google Scholar
[33]	Dunn M G, Kang S W 1973 Theoretical and Experimental Studies of Reentry Plasmas (Washionton DC: National Aeronautics and Space Administration) pp55−58
[34]	Morsli M E, Proulx P 2007 J. Phys. D: Appl. Phys. 40 380 Google Scholar
[35]	Park C, Jaffe R L, Partridge H 2001 J. Thermophys. Heat Tr. 15 76 Google Scholar
[36]	Lenzner S, Auweter-Kurtz M, Heiermann J, Sleziona P 2000 J. Thermophys. Heat Tr. 14 388 Google Scholar
[37]	Fujita K, Mizuno M, Ishida K, Ito T 2008 J. Thermophys. Heat Tr. 22 685 Google Scholar
[38]	Yos J M 1963 Transport Properties of Nitrogen, Hydrogen, Oxygen, and Air to 30000 K (Ohio, America: National Aeronautics and Space Administration) pp49−92
[39]	Gupta R N, Yos J M, Thompson R A, Lee K P 1990 A Review of Reaction Rates and Thermodynamic and Transport Properties for an 11-species Air Model for Chemical and Thermal Nonequilibrium Calculations to 30000 K (Verginia, America: National Aeronautics and Space Administration) pp3−69
[40]	Curtiss C F, Hirschfelder J O 1949 J. Chem. Phys. 17 550 Google Scholar
[41]	Devoto R S 1967 Phys. Fluids 10 2105 Google Scholar
[42]	Ghorui S, Das A K 2013 Phys. Plasmas 20 093504 Google Scholar
[43]	Laricchiuta A, Bruno D, Capitelli M, Catalfamo C, Celiberto R, Colonna G, Diomede P, Giordano D, Gorse C, Longo S, Pagano D, Pirani F 2009 Eur. Phys. J. D 54 607 Google Scholar
[44]	Park C 1990 Nonequilibrium Hypersonic Aerothermodynamics (New York: Wiley) p120
[45]	Park C 2004 J. Thermophys. Heat Tr. 18 527 Google Scholar
[46]	Millikan R C, White D R 1963 J. Chem. Phys. 39 3209 Google Scholar
[47]	Lazdinis S, Petrie S L 1974 Phys. Fluids 17 1539 Google Scholar
[48]	Park C 1984 Proceedings of the 19th Thermophysics Conference Snowmass, Colorado, America, June 25−28, 1984 pp3, 4
[49]	Vincenti W G, Kruger C H 1965 Introduction to Physical Gas Dynamics (Malabar: Krieger)
[50]	Kim M, Gülhan A, Boyd I D 2012 J. Thermophys. Heat Tr. 26 244 Google Scholar
[51]	Bourdon A, Vervisch P 1997 Phys. Rev. E 55 4634 Google Scholar
[52]	Appleton J, Bray K 1964 J. Fluid Mech. 20 659 Google Scholar
[53]	Gnoffo P A, Gupta R N, Shinn J L 1989 Conservation Equations and Physical Models for Hypersonic Air Flows in Thermal and Chemical Nonequilibrium (Hampton, America: National Aeronautics and Space Administration) pp10−12
[54]	Abe K, Kondoh T, Nagano Y 1994 Int. J. Heat Mass Trans. 37 139 Google Scholar

[1]	Ye Xin, Shan Yan-Guang. Numerical simulation of modal evolution and flow field structure of vibrating droplets on hydrophobic surface. Acta Physica Sinica, 2021, 70(14): 144701. doi: 10.7498/aps.70.20210161
[2]	Chen Guo-Hua, Shi Ke-Jun, Chu Jin-Ke, Wu Hao, Zhou Chi-Lou, Xiao Shu. Numerical simulation and optimization of cooling flow field of cylindrical cathode with annular magnetic field. Acta Physica Sinica, 2021, 70(7): 075203. doi: 10.7498/aps.70.20201368
[3]	He Xin, Jiang Tao, Gao Cheng, Zhang Zhen-Fu, Yang Jun-Bo. A simplified method of calculating electronic energy level populations in nonequilibrium plasmas. Acta Physica Sinica, 2021, 70(14): 145202. doi: 10.7498/aps.70.20202119
[4]	Niu Yue, Bao Wei-Min, Li Xiao-Ping, Liu Yan-Ming, Liu Dong-Lin. Numerical simulation and experimental study of high-power thermal equilibrium inductively coupled plasma. Acta Physica Sinica, 2021, 70(9): 095204. doi: 10.7498/aps.70.20201610
[5]	Jiang Chun-Hua, Zhao Zheng-Yu. Numerical simulation of recombination rate effect on development of equatorial plasma bubbles. Acta Physica Sinica, 2019, 68(19): 199401. doi: 10.7498/aps.68.20190173
[6]	Cheng Yu-Guo, Cheng Mou-Sen, Wang Mo-Ge, Li Xiao-Kang. Numerical study on the effects of magnetic field on helicon plasma waves and energy absorption. Acta Physica Sinica, 2014, 63(3): 035203. doi: 10.7498/aps.63.035203
[7]	Wang Xin-Xin, Fan Ding, Huang Jian-Kang, Huang Yong. Numerical simulation of coupled arc in double electrode tungsten inert gas welding. Acta Physica Sinica, 2013, 62(22): 228101. doi: 10.7498/aps.62.228101
[8]	Chen Shi, Wang Hui, Shen Sheng-Qiang, Liang Gang-Tao. The drop oscillation model and the comparison with the numerical simulations. Acta Physica Sinica, 2013, 62(20): 204702. doi: 10.7498/aps.62.204702
[9]	Pang Xue-Xia, Deng Ze-Chao, Jia Peng-Ying, Liang Wei-Hua. Numerical simulation of NOx species behaviour in atmosphere plasma. Acta Physica Sinica, 2011, 60(12): 125201. doi: 10.7498/aps.60.125201
[10]	Qian Xian-Mei, Zhu Wen-Yue, Rao Rui-Zhong. Phase screen distribution for simulating laser propagation along an inhomogeneous atmospheric path. Acta Physica Sinica, 2009, 58(9): 6633-6639. doi: 10.7498/aps.58.6633
[11]	Deng Feng, Zhao Zheng-Yu, Shi Run, Zhang Yuan-Nong. Two-dimensional simulation of high-frequency-induced large-scale irregularities in F region. Acta Physica Sinica, 2009, 58(10): 7382-7391. doi: 10.7498/aps.58.7382
[12]	Gao Fei, Mao Ming, Ding Zhen-Feng, Wang You-Nian. Langmuir probe measurement and theoretical studies on inductively coupled plasma in Ar-N2 discharge. Acta Physica Sinica, 2008, 57(8): 5123-5129. doi: 10.7498/aps.57.5123
[13]	Liu Feng, Meng Yue-Dong, Ren Zhao-Xing, Shu Xing-Sheng. Characterization of ZrN films deposited by ICP enhanced RF magnetron sputtering. Acta Physica Sinica, 2008, 57(3): 1796-1801. doi: 10.7498/aps.57.1796
[14]	Ma Xiao-Tao, Zheng Wan-Hua, Ren Gang, Fan Zhong-Chao, Chen Liang-Hui. Inductively coupled plasma etching of two-dimensional InP/InGaAsP-based photonic crystal. Acta Physica Sinica, 2007, 56(2): 977-981. doi: 10.7498/aps.56.977
[15]	Guo Wen-Qiong, Zhou Xiao-Jun, Zhang Xiong-Jun, Sui Zhan, Wu Deng-Sheng. Simulation electro-optic switch of plasma-electrode Pockels cells driven by one-pulse process. Acta Physica Sinica, 2006, 55(7): 3519-3523. doi: 10.7498/aps.55.3519
[16]	Di Xiao-Lian, Xin Yu, Ning Zhao-Yuan. Effect of antenna configuration on power transfer efficiency for planar inductively coupled plasmas. Acta Physica Sinica, 2006, 55(10): 5311-5317. doi: 10.7498/aps.55.5311
[17]	Huang Song, Xin Yu, NingZhao-Yuan. Studies on C22 radical by optical emission spectroscopy in an induc tively-coupled CF44/CH44 plasma. Acta Physica Sinica, 2005, 54(4): 1653-1658. doi: 10.7498/aps.54.1653
[18]	Yuan Xing-Qiu, Li Hui, Zhao Tai-Zhe, Wang Fei, Guo Wen-Kang, Xu Ping. Numerical study of supersonic plasma torch. Acta Physica Sinica, 2004, 53(3): 788-792. doi: 10.7498/aps.53.788
[19]	Zi Bing-Tao, Yao Ke-Fu, Xu Guang-Ming, Cui Jian-Zhong. Numerical simulation of liguid alloy flow field during solidification under applied pulsed magnetic fields. Acta Physica Sinica, 2003, 52(1): 115-119. doi: 10.7498/aps.52.115
[20]	YU YAN-MEI, YANG GEN-CANG, ZHAO DA-WEN, Lü YI-LI, A. KARMA, C. BECKERMANN. NUMERICAL SIMULATION OF DENDRITIC GROWTH IN UNDERCOOLED MELT USING PHASE-FIELD APPROACH. Acta Physica Sinica, 2001, 50(12): 2423-2428. doi: 10.7498/aps.50.2423

Catalog

Vol. 68, Issue 18 - 2019-09-20

Metrics

Abstract views: 11768
PDF Downloads: 131
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板