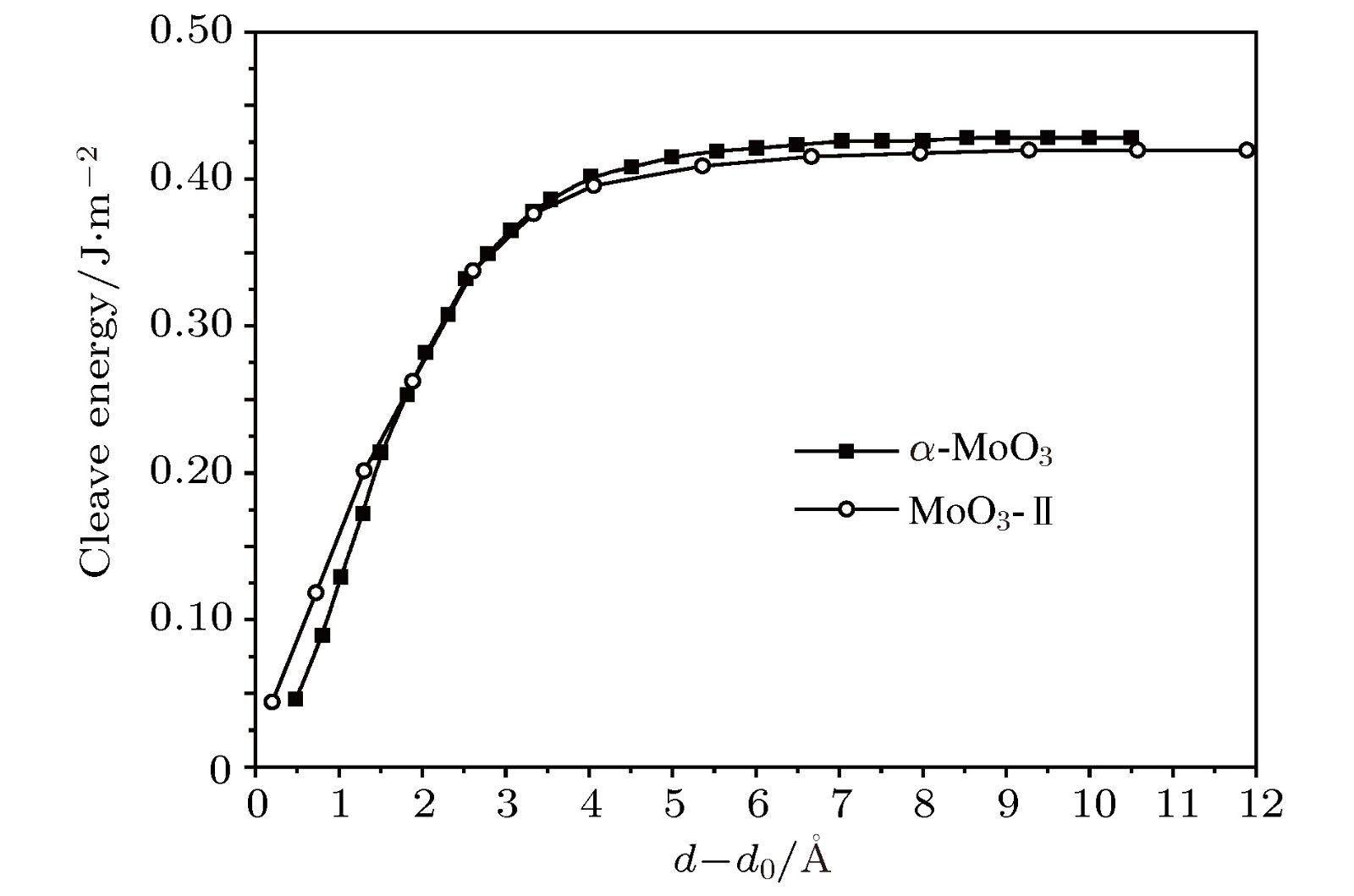
-
According to the pseudopotential plane-wave method of first-principles calculation based on the spin density functional theory, the electronic structure, magnetic and optical properties of laminated molybdenum oxides (orthonormal and monoclinic MoO3) are studied theoretically. The interlaminar dissociation energy, band-structure, spin polarization, dielectric function, and the optical absorption/reflectivity in a charged state are systematically calculated to explore the potential technology applications of laminated MoO3 as electrochromic or electromagnetic materials in optoelectronic devices. The semilocal GGA-PW91 and nonlocal HSE06 exchange-correlation functional are employed to obtain the more accurate crystal structure and band gap respectively. The cleavage energy results indicate that the single layers can easily flake off from the bulk material of these molybdenum oxides. The band structure and atomic-projected density of states prove that the conduction band minimum and valence band maximum are mainly derived from the atom-orbitals bonding oriented in layer-plane, representing characteristic two-dimensional electronic structure. The spin polarized calculations imply that the evident magnetic-moment will engender in MoO6 octahedron layers of the perfect MoO3 due to the substantial spin polarization of Mo and vertex O atoms which are ferromagnetic-coupling to produce significant net magnetic moments, essentially accounting for the magnetic source of bulk MoO3. The Mo vacancy reduces the electronic density of states derived from the spin polarized d-orbitals, leading the net magnetic moment to decrease, while the OI vacancy can reduce the density of spin-down states in the MoO3, resulting in the significant improvement of net magnetic moment. The existence of OII vacancy leads to the energetic spin-splitting of O-2p and Mo-4d orbital states, and thus increasing net magnetic moment by raising the electronic density of polarized spin-up states. The electron spin polarization of Mo-4d orbital component dominantly contributes to the bulk magnetism. The laminated MoO3 presents a significant optical response in the visible region with obvious anisotropy of optical absorption spectra, which will represent a considerable blue shift or new low-frequency absorption peaks for visible light when loading charges. The calculation results demonstrate that the laminated molybdenum oxides have evident electrochromic property with controllable magnetic moment, which provides theoretical basis and technical data for developing novel functional materials with high performance to be used in electromagnetic or optoelectronic devices.
-
Keywords:
- laminated molybdenum oxide /
- first-principles calculation /
- electronic structure /
- electrochromic material
[1] Novoselov K S, Mishchenko A, Carvalho A, Castro Neto A H 2016 Science 353 aac9439
Google Scholar
[2] Lin S Y, Wang C M, Kao K S, Chen Y C, Liu C C 2010 J. Sol-Gel Sci. Techn. 53 51
Google Scholar
[3] Rahmani M, Keshmiri S, Yu J, Sadek A, Al-Mashat L, Moafi A, Latham K, Li Y, Wlodarski W, Kalantar-zadeh K 2010 Actuat. B: Chem. 145 13
Google Scholar
[4] Chen Y, Lu C, Xu L, Ma Y, Hou W, Zhu J J 2010 Cryst. Eng. Commun. 12 3740
Google Scholar
[5] Huang L, Xu H, Zhang R, Cheng X, Xia J, Xu Y, Li H 2013 Appl. Surf. Sci. 283 25
Google Scholar
[6] Kumar V, Sumboja A, Wang J, Bhavanasi V, Nguyen V C, Lee P S 2014 Chem. Mater. 26 5533
Google Scholar
[7] Sreedhara M, Matte H, Govindaraj A, Rao C 2013 Chem. Asian J. 8 2430
Google Scholar
[8] Balendhran S, Deng J, Ou J Z, Walia S, Scott J, Tang J, Wang K L, Field M R, Russo S, Zhuiykov S, Strano M S, Medhekar N, Sriram S, Bhaskaran M, Kalantar-zadeh K 2013 Adv. Mater. 25 109
Google Scholar
[9] Zhou G, Xu X, Yu J, Feng B, Zhang Y, Hu J, Zhou Y 2014 Cryst. Eng. Commun. 16 9025
Google Scholar
[10] Liu D, Lei W W, Hao J, Liu D D, Liu B B, Wang X, Chen X H, Cui Q L, Zou G T, Liu J, Jiang S 2009 J. Appl. Phys. 105 023513
Google Scholar
[11] Baker B, Feist T P, Mccarron E M 1995 J. Solid State Chem. 119 199
Google Scholar
[12] Wang F, Pang Z, Lin L, Fang S, Dai Y, Han S 2009 J. Magn. Magn. Mater. 321 3067
Google Scholar
[13] Peng H, Li J, Li S S, Xia J B 2009 Phys. Rev. B 79 092411
Google Scholar
[14] Han X, Lee J, Yoo H I 2009 Phys. Rev. B 79 100403(R)
Google Scholar
[15] Venkatesan M, Fitzgerald C B, Coey J M D 2004 Nature 430 630
Google Scholar
[16] Gacic M, Jakob G, Herbort C, Adrian H, Tietze T, Brück S, Goering E 2007 Phys. Rev. B 75 205206
Google Scholar
[17] Hu J, Zhang Z, Zhao M, Qin H, Jiang M 2008 Appl. Phys. Lett. 93 192503
Google Scholar
[18] Sundaresan A, Bhargavi R, Rangarajan N, Siddesh U, Rao C N R 2006 Phys. Rev. B 74 161306(R)
Google Scholar
[19] Zuo X, Yoon S D, Yang A, Duan W H, Vittoria C, Harris V G 2009 J. Appl. Phys. 105 07C508
Google Scholar
[20] Rahman G, García-Suárez V M, Hong S C 2008 Phys. Rev. B 78 184404
Google Scholar
[21] Wang F, Pang Z, Lin L, Fang S, Dai Y, Han S 2009 Phys. Rev. B 80 144424
Google Scholar
[22] Peng H, Xiang H J, Wei S H, Li S S, Xia J B, Li J 2009 Phys. Rev. Lett. 102 017201
Google Scholar
[23] Thakur P, Cezar J C, Brookes N B, Choudhary R J, Prakash R, Phase D M, Chae K H, Kumar R 2009 Appl. Phys. Lett. 94 062501
Google Scholar
[24] Okumu J, Koerfer F, Salinga C, Wutting M 2004 J. Appl. Phys. 95 7632
Google Scholar
[25] Wang F, Pang Z, Lin L, Fang S, Dai Y, Han S 2010 Phys. Rev. B 81 134407
Google Scholar
[26] Ding H, Ray K G, Ozolins V, Asta M 2012 Phys. Rev. B 85 012104
[27] Peelaers H, Van de Walle C G 2014 J. Phys.: Condens. Matter 26 305502
Google Scholar
[28] Ding H, Lin H, Sadigh B, Zhou F, Ozolinš V, Asta M 2014 J. Phys. Chem. C 118 15565
Google Scholar
[29] Kröger M, Hamwi S, Meyer J, Riedl T, Kowalsky W, Kahn A 2009 Appl. Phys. Lett. 95 123301
Google Scholar
[30] Becke A D 1993 J. Chem. Phys. 98 5648
Google Scholar
[31] Perdew J P, Chevary J A, Vosko S H, Jackson K A, Pederson M R, Singh D J, Fiolhais C 1992 Phys. Rev. B 46 6671
Google Scholar
[32] Tkatchenko A, Scheffler M 2009 Phys. Rev. Lett. 102 073005
Google Scholar
[33] Krukau A V, Vydrov O A, Izmaylov A F, Scuseria G E 2006 J. Chem. Phys. 125 224106
Google Scholar
[34] Cococcioni M, Gironcoli S 2005 Phys. Rev. B 71 035105
Google Scholar
[35] Kress G, Joubert D 1999 Phys. Rev. B 59 1758
[36] Milman V, Lee M H, Payne M C 1994 Phys. Rev. B 49 16300
Google Scholar
[37] Payne M C, Teter M P, Allan D C, Arias T A, Joannopoulos J D 1992 Rev. Mod. Phys. 64 1045
Google Scholar
[38] Marzari N, Vanderbilt D, Payne M C 1997 Phys. Rev. Lett. 79 1337
Google Scholar
[39] Chantis A N, Christensen N E, Svane A, Cardona M 2010 Phys. Rev. B 81 205205
Google Scholar
[40] Pfrommer B G, Cote M, Louie S G, Cohen M L 1997 J. Comput. Phys. 131 233
Google Scholar
[41] Segall M D, Shah R, Pickard C J, Payne M C 1996 Phys. Rev. B 54 16317
Google Scholar
[42] Negishi H, Negishi S, Kuroiwa Y, Sato N, Aoyagi S 2004 Phys. Rev. B 69 064111
Google Scholar
[43] Kalantar-zadeh K, Tang J, Wang M, Wang K L, Shailos A, Galatsis K, Kojima R, Strong V, Lech A, Wlodarski W, Kaner R B 2010 Nanoscale 2 429
Google Scholar
[44] Dandogbessi B S, Akin-Ojo O 2016 J. Appl. Phys. 120 055105
Google Scholar
[45] Zhong M Z, Zhou K, Wei Z M, Li Y, Li T, Dong H L, Jiang L, Li J B, Hu W P 2018 2D Mater. 5 035033
Google Scholar
-
图 1 (a)
$\alpha $ -MoO3和(b) MoO3-Ⅱ晶体结构, 顶点、共角和共棱氧原子分别标识为OⅠ, OⅡ和OⅢFigure 1. The crystal structures of (a)
$\alpha $ -MoO3 and (b) MoO3-Ⅱ. The apical, corner-sharing and edge-sharing oxygen atoms are denoted by OⅠ, OⅡ and OⅢ respectively.
图 2
$\alpha $ -MoO3和MoO3-Ⅱ在解离过程中的解离能随层间距离d的变化Figure 2. The cleavage energies of
$\alpha $ -MoO3 and MoO3-Ⅱ, varying with inter-layer distance d.
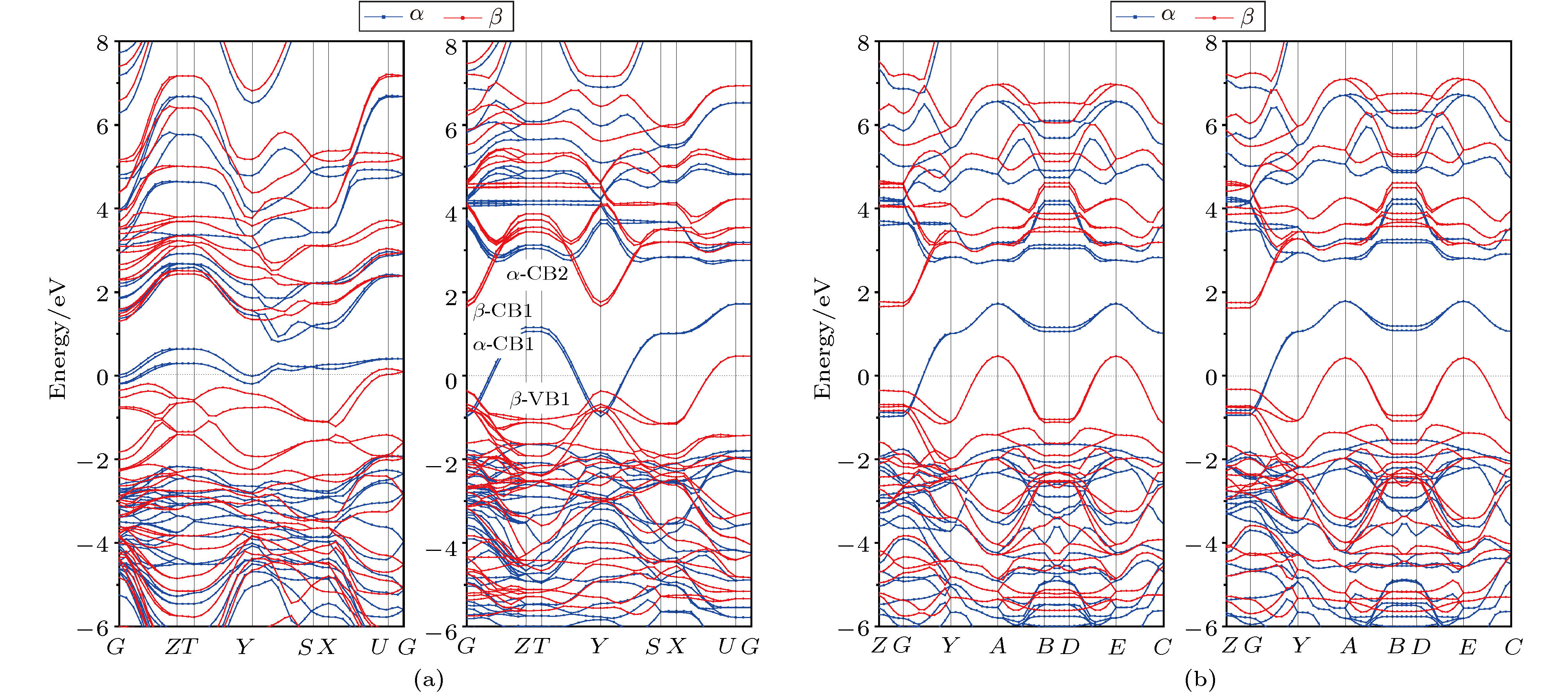
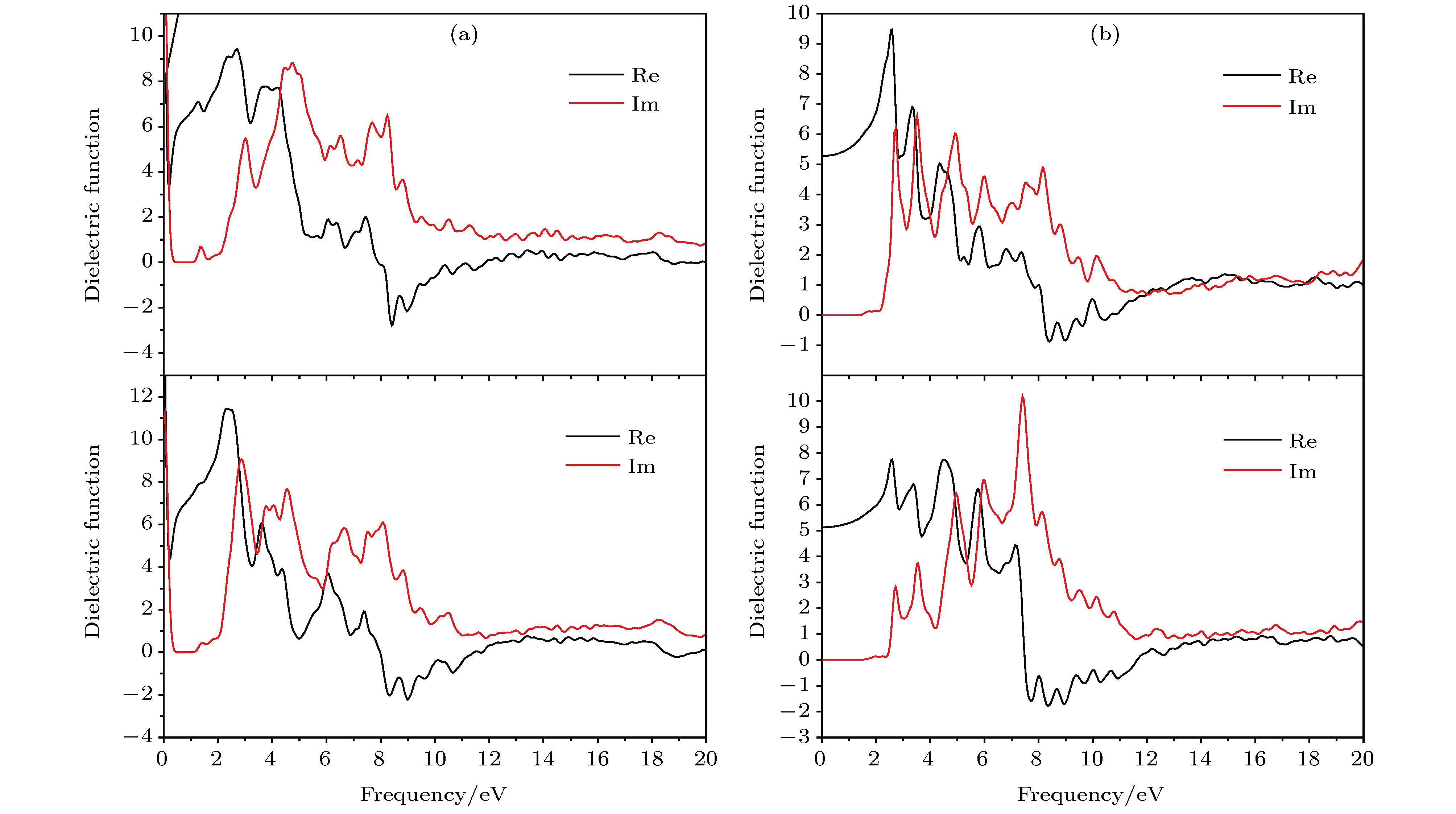
图 3 用PW91泛函(左图)和HSE06泛函(右图)计算的(a)
$\alpha $ -MoO3和(b) MoO3-Ⅱ能带结构, 费米能级作为能量参考零点(水平虚线)Figure 3. Band structures of (a)
$\alpha $ -MoO3 and (b) MoO3-Ⅱ calculated by PW91 functional (left panels) and HSE06 functional (right panels), with the Fermi energy level being set as reference energy zero (horizontal dot line).
图 4 (a)
$\alpha $ -MoO3和(b) MoO3-Ⅱ的DOS,Mo和O的PDOSFigure 4. The total density of states, Mo and O atomic projected density of states for (a)
$\alpha $ -MoO3 and (b) MoO3-Ⅱ.
图 5 (a)
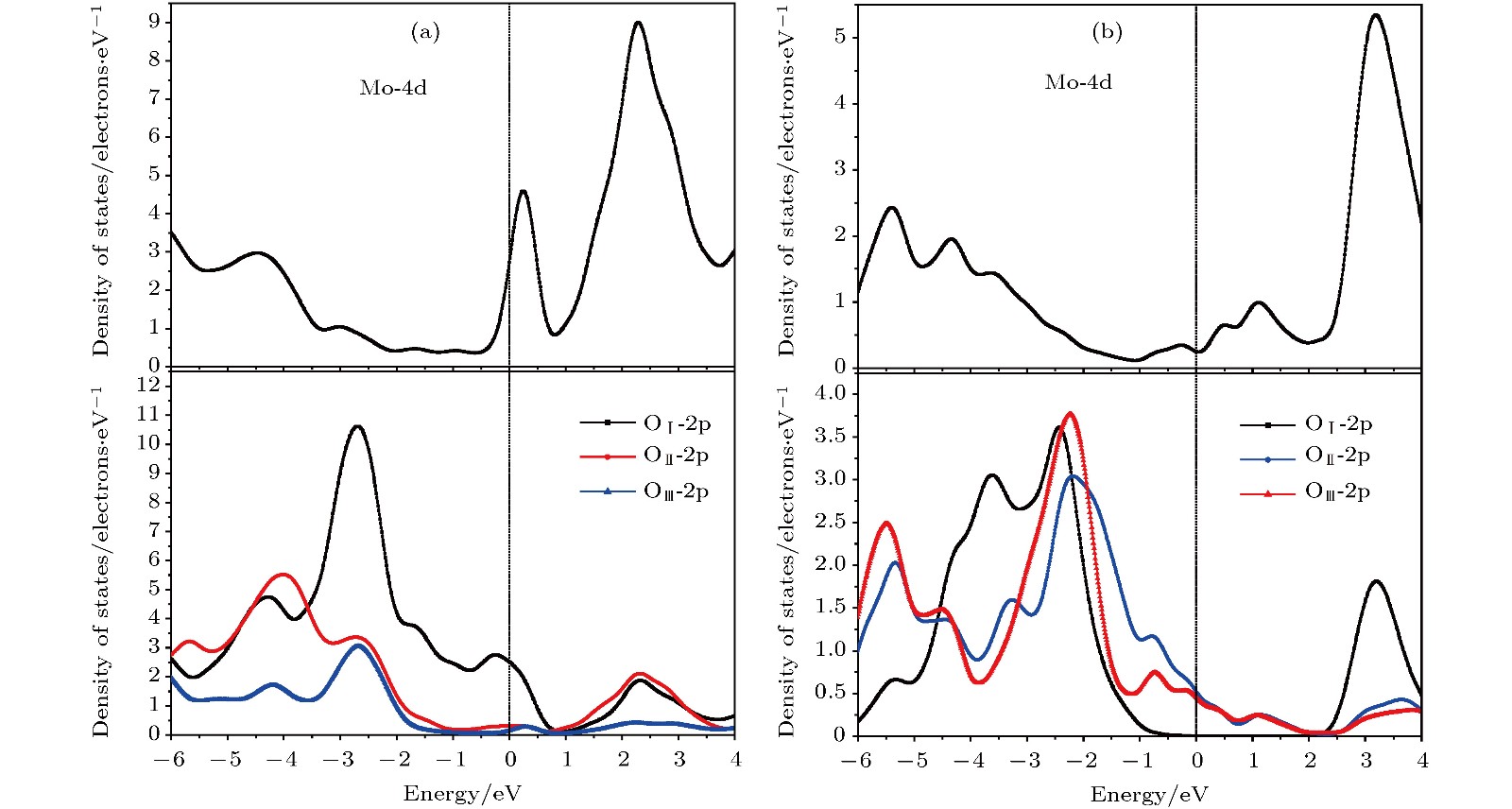
$\alpha $ -MoO3和(b) MoO3 -Ⅱ PDOS的轨道成分: Mo原子的4d轨道成分(上图)和三种等价氧原子OⅠ, OⅡ和OⅢ的2p轨道成分(下图)Figure 5. Partial orbital components of atomic projected density of states for (a)
$\alpha $ -MoO3 and (b) MoO3 -Ⅱ: Mo-4d orbital (above panels) and O-2p orbital (below panels) of three equivalent oxygens OⅠ, OⅡ and OⅢ.
图 6
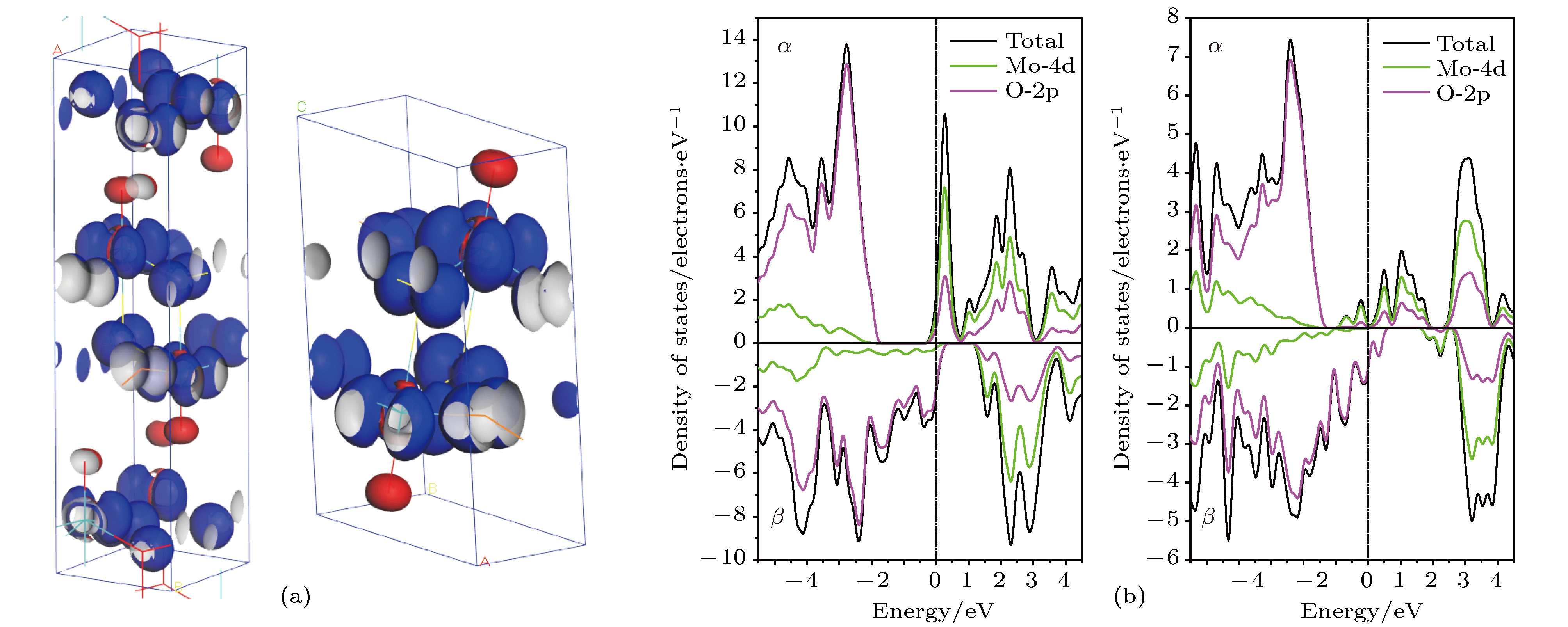
$\alpha $ -MoO3(左图)和MoO3-Ⅱ(右图)的(a)自旋分布的等密度面空间分布, 自旋密度等值面密度为0.05 electrons/Å3; (b) Mo-4d和O-2p的部分DOS(上旋态$\alpha $ 和下旋态$\beta $ ), 费米能量为能量参考零点(垂直虚线)Figure 6. (a) The spin distribution representing as spin densityisosurface contoured at 0.05 electrons/Å3 and (b) the spin-resolved partial DOS of Mo-4d and O-2p with Fermi energy being referenced as energy zero indicated by vertical dashed line, for
$\alpha $ -MoO3 (left panels) and MoO3-Ⅱ (right panels).
图 7 含有6% Mo空位、OⅠ空位、OⅡ空位和OⅢ空位的 (a)
$\alpha $ -MoO3和 (b) MoO3 -II缺陷晶体的Mo-4d和O-2p的部分DOS (上旋态$\alpha $ 和下旋态$\beta $ ), 费米能量为能量参考零点(垂直虚线)Figure 7. The spin-resolved partial DOS of Mo-4d and O-2p for (a)
$\alpha $ -MoO3 and (b) MoO3 -Ⅱ crystals with 6% Mo, OⅠ, OⅡ and OⅢ vacancies respectively. The Fermi energy is referenced as energy zero indicated by vertical dashed line.
图 8
$\alpha $ -MoO3(左图)和MoO3-Ⅱ(右图)含有6% OⅠ(上图)和OⅡ(下图)空位的自旋密度等值面, 等值面的密度为0.05 electrons /Å3Figure 8. The isosurface of spin density of
$\alpha $ -MoO3 (left images) and MoO3-Ⅱ (right images) with 6% OⅠ (above images) and OⅡ (below images) vacancies respectively. The isosurfaces are contoured at 0.05 electrons/Å3.
图 9 (a)
$\alpha $ -MoO3和(b) MoO3-Ⅱ介电函数谱线, 入射非偏振光分别沿着原子层平面的垂直(上图)和平行(下图)方向入射Figure 9. The calculateddielectric functions of (a)
$\alpha $ -MoO3 and (b) MoO3-Ⅱ with the light incident along the directions perpendicular (above panels) and parallel (below panels) to atomic-layer plane respectively.
图 10 (a)
$\alpha $ -MoO3和(b) MoO3-Ⅱ在不同带电(–2e—+2e/单胞)状态下的光吸收谱, 入射非偏振光分别沿着原子层平面的垂直(左图)和平行(右图)方向入射Figure 10. The calculatedabsorption spectra of (a)
$\alpha $ -MoO3 and (b) MoO3-Ⅱ with the light incident along the directions perpendicular (left panels) and parallel (right panels) to atomic-layer plane respectively, under different charge loading (–2e—+2e per unit cell).表 1
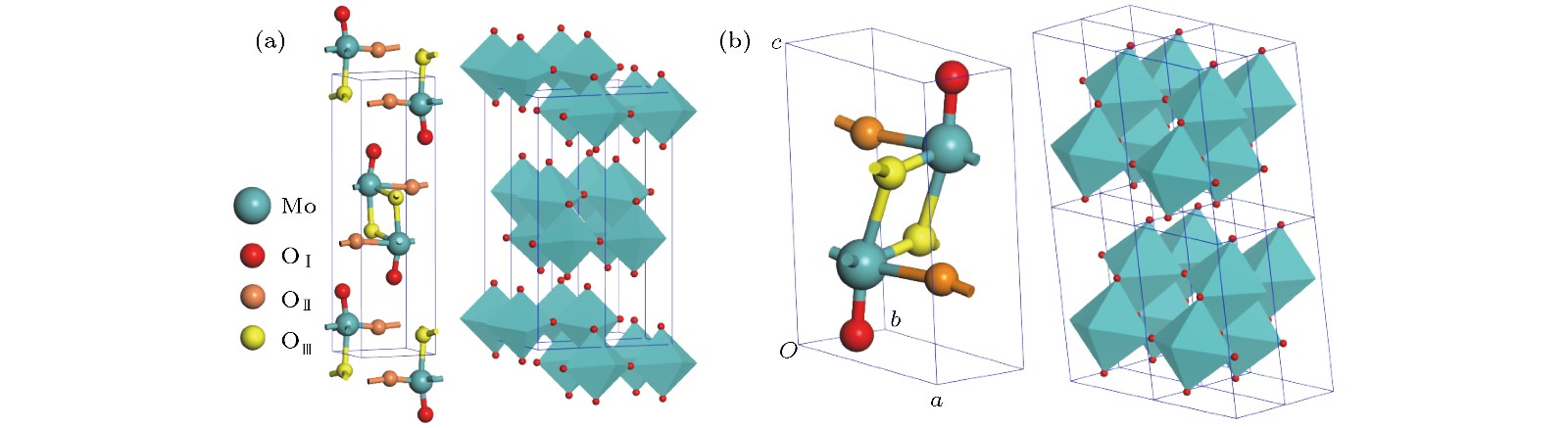
$\alpha $ -MoO3和MoO3-Ⅱ的晶格参数Table 1. Crystal parameters of
$\alpha $ -MoO3 and MoO3-Ⅱ.晶体结构 $\alpha $-MoO3 MoO3-Ⅱ 对称空间群 Pnma ($\alpha $ = $\beta $ = $\gamma $ = 90°) P21/m ($\alpha $ = $\gamma $ = 90°) 晶格
参数PBE a = 14.668 Å, b = 3.858 Å, c = 3.965 Å a = 3.783 Å, b = 3.739 Å, c = 8.067 Å, $\beta $ = 100.979° PBE + DFT-D/TS a = 13.012 Å, b = 3.812 Å, c = 3.876 Å a = 3.882 Å, b = 3.812 Å, c = 7.544 Å, $\beta $ = 104.879° PW91 a = 13.213 Å, b = 3.856 Å, c = 3.990 Å a = 3.809 Å, b = 3.743 Å, c = 7.863 Å, $\beta $ = 101.098° PW91 + DFT-D/OBS a = 13.484 Å, b = 3.866 Å, c = 3.964 Å a = 3.909 Å, b = 3.820 Å, c = 7.293 Å, $\beta $ = 104.263° 实验[41,42] a = 13.350 Å, b = 3.703 Å, c = 3.918 Å a = 3.954 Å, b = 3.687 Å, c = 7.095 Å, $\beta $ = 103.745° 
DownLoad: CSV
表 2
$\alpha $ -MoO3和MoO3 -Ⅱ光学能带带隙的计算值和文献报道实验数据Table 2. The calculated optical band-gaps of
$\alpha $ -MoO3 and MoO3 -Ⅱ in comparison with reported experimental data.带隙/eV $\alpha $-MoO3 MoO3 -Ⅱ PW91/LDA + U 上旋态 1.38 2.99 下旋态 1.64 2.01 HSE06 上旋态 2.85 2.92 下旋态 2.14 2.12 实验值[29] 3.0 —
DownLoad: CSV
表 3 用PW91梯度校正泛函和HSE06杂化泛函计算的两种层堆叠钼氧化物的EA和电IP, 最后一行中列出文献[29]中
$\alpha $ -MoO3的实验结果Table 3. The calculated electron affinity (EA) and ionization potential (IP) of two laminated molybdenum oxides employing PW91 and HSE06 functionals respectively. The experimental results of
$\alpha $ -MoO3 from Ref. [29] are also listed for comparison.$\alpha $-MoO3 MoO3 -Ⅱ EA/eV IP/eV EA/eV IP/eV 计算 PW91 6.555 7.94 6.11 8.12 HSE06 6.615 8.76 6.17 8.29 实验 6.700 9.68 — —
DownLoad: CSV
表 4 不同点空位类型MoO3晶体的总自旋值(每个单胞), 点空位浓度为6%
Table 4. The total spin values (per unit cell) of MoO3 configurations with different vacancies of 6% concentration.
总自旋h·bar/2/单胞 完整晶体 VMo VO-Ⅰ VO-Ⅱ VO-Ⅲ $\alpha $-MoO3 8.206 7.7855 8.235 8.244 8.214 MoO3 -Ⅱ 4.395 3.995 4.770 4.800 4.378
DownLoad: CSV
-
[1] Novoselov K S, Mishchenko A, Carvalho A, Castro Neto A H 2016 Science 353 aac9439
Google Scholar
[2] Lin S Y, Wang C M, Kao K S, Chen Y C, Liu C C 2010 J. Sol-Gel Sci. Techn. 53 51
Google Scholar
[3] Rahmani M, Keshmiri S, Yu J, Sadek A, Al-Mashat L, Moafi A, Latham K, Li Y, Wlodarski W, Kalantar-zadeh K 2010 Actuat. B: Chem. 145 13
Google Scholar
[4] Chen Y, Lu C, Xu L, Ma Y, Hou W, Zhu J J 2010 Cryst. Eng. Commun. 12 3740
Google Scholar
[5] Huang L, Xu H, Zhang R, Cheng X, Xia J, Xu Y, Li H 2013 Appl. Surf. Sci. 283 25
Google Scholar
[6] Kumar V, Sumboja A, Wang J, Bhavanasi V, Nguyen V C, Lee P S 2014 Chem. Mater. 26 5533
Google Scholar
[7] Sreedhara M, Matte H, Govindaraj A, Rao C 2013 Chem. Asian J. 8 2430
Google Scholar
[8] Balendhran S, Deng J, Ou J Z, Walia S, Scott J, Tang J, Wang K L, Field M R, Russo S, Zhuiykov S, Strano M S, Medhekar N, Sriram S, Bhaskaran M, Kalantar-zadeh K 2013 Adv. Mater. 25 109
Google Scholar
[9] Zhou G, Xu X, Yu J, Feng B, Zhang Y, Hu J, Zhou Y 2014 Cryst. Eng. Commun. 16 9025
Google Scholar
[10] Liu D, Lei W W, Hao J, Liu D D, Liu B B, Wang X, Chen X H, Cui Q L, Zou G T, Liu J, Jiang S 2009 J. Appl. Phys. 105 023513
Google Scholar
[11] Baker B, Feist T P, Mccarron E M 1995 J. Solid State Chem. 119 199
Google Scholar
[12] Wang F, Pang Z, Lin L, Fang S, Dai Y, Han S 2009 J. Magn. Magn. Mater. 321 3067
Google Scholar
[13] Peng H, Li J, Li S S, Xia J B 2009 Phys. Rev. B 79 092411
Google Scholar
[14] Han X, Lee J, Yoo H I 2009 Phys. Rev. B 79 100403(R)
Google Scholar
[15] Venkatesan M, Fitzgerald C B, Coey J M D 2004 Nature 430 630
Google Scholar
[16] Gacic M, Jakob G, Herbort C, Adrian H, Tietze T, Brück S, Goering E 2007 Phys. Rev. B 75 205206
Google Scholar
[17] Hu J, Zhang Z, Zhao M, Qin H, Jiang M 2008 Appl. Phys. Lett. 93 192503
Google Scholar
[18] Sundaresan A, Bhargavi R, Rangarajan N, Siddesh U, Rao C N R 2006 Phys. Rev. B 74 161306(R)
Google Scholar
[19] Zuo X, Yoon S D, Yang A, Duan W H, Vittoria C, Harris V G 2009 J. Appl. Phys. 105 07C508
Google Scholar
[20] Rahman G, García-Suárez V M, Hong S C 2008 Phys. Rev. B 78 184404
Google Scholar
[21] Wang F, Pang Z, Lin L, Fang S, Dai Y, Han S 2009 Phys. Rev. B 80 144424
Google Scholar
[22] Peng H, Xiang H J, Wei S H, Li S S, Xia J B, Li J 2009 Phys. Rev. Lett. 102 017201
Google Scholar
[23] Thakur P, Cezar J C, Brookes N B, Choudhary R J, Prakash R, Phase D M, Chae K H, Kumar R 2009 Appl. Phys. Lett. 94 062501
Google Scholar
[24] Okumu J, Koerfer F, Salinga C, Wutting M 2004 J. Appl. Phys. 95 7632
Google Scholar
[25] Wang F, Pang Z, Lin L, Fang S, Dai Y, Han S 2010 Phys. Rev. B 81 134407
Google Scholar
[26] Ding H, Ray K G, Ozolins V, Asta M 2012 Phys. Rev. B 85 012104
[27] Peelaers H, Van de Walle C G 2014 J. Phys.: Condens. Matter 26 305502
Google Scholar
[28] Ding H, Lin H, Sadigh B, Zhou F, Ozolinš V, Asta M 2014 J. Phys. Chem. C 118 15565
Google Scholar
[29] Kröger M, Hamwi S, Meyer J, Riedl T, Kowalsky W, Kahn A 2009 Appl. Phys. Lett. 95 123301
Google Scholar
[30] Becke A D 1993 J. Chem. Phys. 98 5648
Google Scholar
[31] Perdew J P, Chevary J A, Vosko S H, Jackson K A, Pederson M R, Singh D J, Fiolhais C 1992 Phys. Rev. B 46 6671
Google Scholar
[32] Tkatchenko A, Scheffler M 2009 Phys. Rev. Lett. 102 073005
Google Scholar
[33] Krukau A V, Vydrov O A, Izmaylov A F, Scuseria G E 2006 J. Chem. Phys. 125 224106
Google Scholar
[34] Cococcioni M, Gironcoli S 2005 Phys. Rev. B 71 035105
Google Scholar
[35] Kress G, Joubert D 1999 Phys. Rev. B 59 1758
[36] Milman V, Lee M H, Payne M C 1994 Phys. Rev. B 49 16300
Google Scholar
[37] Payne M C, Teter M P, Allan D C, Arias T A, Joannopoulos J D 1992 Rev. Mod. Phys. 64 1045
Google Scholar
[38] Marzari N, Vanderbilt D, Payne M C 1997 Phys. Rev. Lett. 79 1337
Google Scholar
[39] Chantis A N, Christensen N E, Svane A, Cardona M 2010 Phys. Rev. B 81 205205
Google Scholar
[40] Pfrommer B G, Cote M, Louie S G, Cohen M L 1997 J. Comput. Phys. 131 233
Google Scholar
[41] Segall M D, Shah R, Pickard C J, Payne M C 1996 Phys. Rev. B 54 16317
Google Scholar
[42] Negishi H, Negishi S, Kuroiwa Y, Sato N, Aoyagi S 2004 Phys. Rev. B 69 064111
Google Scholar
[43] Kalantar-zadeh K, Tang J, Wang M, Wang K L, Shailos A, Galatsis K, Kojima R, Strong V, Lech A, Wlodarski W, Kaner R B 2010 Nanoscale 2 429
Google Scholar
[44] Dandogbessi B S, Akin-Ojo O 2016 J. Appl. Phys. 120 055105
Google Scholar
[45] Zhong M Z, Zhou K, Wei Z M, Li Y, Li T, Dong H L, Jiang L, Li J B, Hu W P 2018 2D Mater. 5 035033
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 18334
- PDF Downloads: 272
- Cited By: 0
