冷却速率对La基非晶合金<i>β</i>弛豫行为和应力弛豫的影响

孟绍怡; 郝奇; 王兵; 段亚娟; 乔吉超

doi:10.7498/aps.73.20231417

摘要

β弛豫行为及应力弛豫是探索非晶合金微观结构非均匀性的重要切入口. β弛豫行为及应力弛豫与非晶合金扩散、玻璃转变行为以及塑性变形等密切相关, 探究它们之间关联有重要科学意义. 本文以典型的La基非晶合金作为研究载体, 系统地探究了通过不同冷却速率所得块体样品与条带样品的β弛豫特征及应力弛豫行为. 结果表明, 冷却速率是控制玻璃体系能量状态, 进而影响其物理力学性能的重要参数. 冷却速率越大, 低温内耗更大, 对应β弛豫激活能更小, β弛豫行为在温度谱中展宽更大, 表明高冷却速率导致原子移动性更大, 微观尺度结构非均匀程度更高. 基于应力弛豫测量与分析, 发现高冷却速率变形特征时间减小, 归一化应力衰减幅度更大, 在外加力场作用下更易变形, 变形单元更易激活以容纳结构形变. 本研究为进一步厘清非晶合金β弛豫、变形及微观结构非均匀性之间关联提供了思路.

关键词:

Abstract

The dynamic relaxation process and stress relaxation process are two important processes which can reflect the microstructures of materials, for they are closely related to the diffusions, the glass transition phenomena and the microstructural heterogeneities. It is of great significance to explore the relationship among them. In the current research, the β-relaxation characteristics and stress relaxation behaviors of bulk and ribbon samples obtained by different cooling rates are systematically investigated by taking the typical La-based amorphous alloys as model systems. The experimental results demonstrate that the cooling rate is an important parameter for controlling the energy state of the glass system, which further affects its physical and mechanical properties. Based on the dynamical mechanical spectra, the larger the cooling rate, the greater the low-temperature internal friction is and the smaller the beta relaxation activation energy according to Arrhenius calculations, and the greater the broadening of the beta relaxation behavior in the temperature spectra, suggesting that the higher cooling rate leads to greater atomic mobility and a high degree of heterogeneity in the microstructure. Thermodynamic analysis is conducted to study the slow process of thermal activation and the fast process driven by stress. At low temperature, the activation volume of the strip sample is larger than that of the bulk sample, and the activation volume values of the two samples are almost the same, as the cooling rate only affect the β relaxation stage, but exert little effect on the α relaxation, which is consistent with the conclusion that the stress relaxation behavior and β relaxation behavior are related to the structural non-uniformity of the amorphous alloy. The stress relaxation tests show that the characteristic time of deformation decreases at higher cooling rate, the normalized stress decay is larger, it is easier to deform under an applied force field, and the deformation unit is more likely to activate to accommodate structural deformation. The correlation between stress relaxation and β relaxation of amorphous alloy is further confirmed, and the proportion of liquid-like region is proportional to the relaxation mode spectrum, which also shows that β relaxation and stress relaxation are consistent. Finally, by calculating relaxation enthalpy $ \Delta {H}_{{\mathrm{r}}{\mathrm{e}}{\mathrm{l}}} $, the variation of microstructure heterogeneity with cooling rate is experimentally verified. The research sheds new light on further clarifying the relationship among β relaxation, deformation and microstructural heterogeneity of the amorphous alloy.

Keywords:

作者及机构信息

1.
西北工业大学力学与土木建筑学院, 西安　710072

2.
西北工业大学物理科学与技术学院, 西安　710072

3.
西北工业大学重庆科创中心, 重庆　401135

通信作者: 王兵, wangbing0614@nwpu.edu.cn ; 乔吉超, qjczy@nwpu.edu.cn

基金项目: 国家自然科学基金(批准号: 51971178, 52271153, 52101201)、陕西省杰出青年基金(批准号: 2021JC-12)、重庆市自然科学基金(批准号: cstc2021jcyj-msxmX0369)、西北工业大学硕士研究生实践创新能力培育基金(批准号: PF2023088)和陕西省科技创新团队科学基金(批准号: 2021TD-14)资助的课题.

Authors and contacts

1.
School of Mechanics, Civil Engineering and Architecture, Northwestern Polytechnical University, Xi’an 710072, China

2.
School of Physical Science and Technology, Northwestern Polytechnical University, Xi’an 710072, China

3.
Chongqing Innovation Center, Northwestern Polytechnical University, Chongqing 401135, China

Corresponding author: Wang Bing, wangbing0614@nwpu.edu.cn ; Qiao Ji-Chao, qjczy@nwpu.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 51971178, 52271153, 52101201), the Outstanding Youth Foundation of Shaanxi Province, China (Grant No. 2021JC-12), the Natural Science Foundation of Chongqing, China (Grant No. cstc2021jcyj-msxmX0369), the Practical Innovation Ability Cultivation Fund for Postgraduate Students of Northwestern Polytechnical University, China (Grant No. PF2023088), and the Science and Technology Innovation Team Science Fund of Shaanxi Province, China (Grant No. 2021TD-14).

文章全文 : translate this paragraph

参考文献

[1]	Angell C A 1996 Curr. Opin. Solid State Mater. Sci. 1 578 Google Scholar
[2]	Greer A L 1995 Science 267 1947 Google Scholar
[3]	Chang C, Zhang H P, Zhao R, Li F C, Luo P, Li M Z, Bai H Y 2022 Nat. Mater. 21 1240 Google Scholar
[4]	Qiao J C, Wang Q, Pelletier J M, Kato H, Casalini R, Crespo D, Pineda E, Yao Y, Yang Y 2019 Prog. Mater. Sci. 104 250
[5]	Wang Q, Liu J J, Ye Y F, Liu T T, Wang S, Liu C T, Lu J, Yang Y 2017 Mater. Today 20 293 Google Scholar
[6]	Wang Q, Shang Y H, Yang Y 2023 Mater. Futures 2 017501 Google Scholar
[7]	Wang W H 2019 Prog. Mater. Sci. 106 100561 Google Scholar
[8]	乔吉超, 张浪渟, 童钰, 吕国建, 郝奇, 陶凯 2022 力学进展 52 117 Google Scholar Qiao J C, Zhang L T, Tong Y, Lyu G J, Hao Q, Tao K 2022 Adv. Mech. 52 117 Google Scholar
[9]	Wang X D, Ruta B, Xiong L H, Zhang D W, Chushkin Y, Sheng H W, Lou H B, Cao Q P, Jiang J Z 2015 Acta Mater. 99 290 Google Scholar
[10]	胡丽娜, 张春芝, 岳远征, 边秀房 2010 科学通报 55 115 Hu L N, Zhang C Z, Yue Y Z, Bian X F 2010 Sci. Bull. 55 115
[11]	Capaccioli S, Paluch M, Prevosto D, Wang L M, Ngai K L 2012 J. Phys. Chem. Lett. 3 735 Google Scholar
[12]	Ngai K L, Wang Z, Gao X Q, Yu H B, Wang W H 2013 J. Chem. Phys. 139 014502 Google Scholar
[13]	Wang W H 2011 J. Appl. Phys. 110 053521 Google Scholar
[14]	Yu H B, Wang W H, Bai H Y, Wu Y, Chen M V 2010 Phys. Rev. B 81 220201 Google Scholar
[15]	Yu H B, Wang W H, Bai H Y, Samwer K 2014 Nat. Sci. Rev. 1 429 Google Scholar
[16]	Li N, Xu X N, Zheng Z Z, Liu L 2014 Acta Mater. 65 400 Google Scholar
[17]	Yu H B, Samwer K, Wu Y, Wang W H 2012 Phys. Rev. Lett. 109 095508 Google Scholar
[18]	Yu H B, Wang W H, Samwer K 2013 Mater. Today 16 183 Google Scholar
[19]	Ashby M F, Greer A L 2006 Scr. Mater. 54 321 Google Scholar
[20]	Dyre J C 2006 Rev. Mod. Phys. 78 953 Google Scholar
[21]	Ediger M D, Harrowell P 2012 J. Chem. Phys. 137 080901 Google Scholar
[22]	Goldstein M 2011 J. Non-Cryst. Solids 357 249 Google Scholar
[23]	Hu L, Yue Y 2009 J. Phys. Chem. C 113 15001 Google Scholar
[24]	Wang W H, Dong C, Shek C H 2004 Mater. Sci. Eng. , R 44 45 Google Scholar
[25]	汪卫华 2013 物理学进展 33 177 Wang W H 2013 Prog. Phys. 33 177
[26]	Zhao L Z, Wang W H, Bai H Y 2014 J. Non-Cryst. Solids 405 207 Google Scholar
[27]	Ma Z J, Guo Y C, Li Y, Lugee T, Gao P H, Yang Z, Zeng X R 2015 J. Alloys Compd. 648 18 Google Scholar
[28]	Wang B, Shang B S, Gao X Q, Wang W H, Bai H Y, Pan M X, Guan P F 2016 J. Phys. Chem. Lett. 7 4945 Google Scholar
[29]	Zhang L T, Duan Y J, Wang Y J, Yang Y, Qiao J C 2023 Sci. China-Technol. Sci. 66 173 Google Scholar
[30]	Jiao W, Sun B A, Wen P, Bai H Y, Kong Q P, Wang W H 2013 Appl. Phys. Lett. 103 101903 Google Scholar
[31]	Ke H B, Zhang P, Sun B A, Zhang P G, Liu T W, Chen P H, Wu M, Huang H G 2019 J. Alloys Compd. 788 391 Google Scholar
[32]	Milkus R, Zaccone A 2017 Phys. Rev. E 95 023001 Google Scholar
[33]	Qiao J C, Wang Y J, Pelletier J M, Keer L M, Fine M E, Yao Y 2015 Acta Mater. 98 43 Google Scholar
[34]	Qiao J C, Wang Y J, Zhao L Z, Dai L H, Crespo D, Pelletier J M, Keer L M, Yao Y 2016 Phys. Rev. B 94 104203 Google Scholar
[35]	Liang S Y, Zhang L T, Wang B, Wang Y J, Pineda E, Qiao J C 2024 Intermetallics 164 108115 Google Scholar
[36]	Luo P, Li M X, Jiang H Y, Wen P, Bai H Y, Wang W H 2017 J. Appl. Phys. 121 135104 Google Scholar
[37]	Luo P, Wen P, Bai H Y, Ruta B, Wang W H 2017 Phys. Rev. Lett. 118 225901 Google Scholar
[38]	Jiao W, Wen P, Peng H L, Bai H Y, Sun B A, Wang W H 2013 Appl. Phys. Lett. 102 101903 Google Scholar
[39]	Wang Z, Sun B A, Bai H Y, Wang W H 2014 Nat. Commun. 5 5823 Google Scholar
[40]	汪卫华 2014 中国科学: 物理学力学天文学 44 396 Google Scholar Wang W H, 2014 Sci. Sin. Phys. Mech. Astr. 44 396 Google Scholar
[41]	Li R, Pang S, Ma C L, Zhang T 2007 Acta Mater. 55 3719 Google Scholar
[42]	Lin X H, Johnson W L 1995 J. Appl. Phys. 78 6514 Google Scholar
[43]	Perez J 1990 Solid State Ionics 39 69 Google Scholar
[44]	Perez J, Etienne S, Tatibouät J 1990 Phys. Status Solidi A 121 129 Google Scholar
[45]	Qiao J C, Pelletier J M 2014 J. Mater. Sci. Technol. 30 523 Google Scholar
[46]	Wang Q, Pelletier J M, Blandin J J, Suery M 2005 J. Non-Cryst. Solids 351 2224 Google Scholar
[47]	Perez J 2018 Physics and Mechanics of Amorphous Polymers (London: Routledge) pp117–119
[48]	Rinaldi R, Gaertner R, Chazeau L, Gauthier C 2011 Int. J. Non-Linear Mech. 46 496 Google Scholar
[49]	Evenson Z, Naleway S E, Wei S, Gross O, Kruzic J J, Gallino I, Possart W, Stommel M, Busch R 2014 Phys. Rev. B 89 174204 Google Scholar
[50]	Wang B, Gao X Q, Qiao J C 2024 Rare Met. Mater. Eng. 53 70 Google Scholar
[51]	孟绍怡, 郝奇, 吕国建, 乔吉超 2023 72 076101 Google Scholar Meng S Y, Hao Q, Lyu G J, Qiao J C 2023 Acta Phys. Sin. 72 076101 Google Scholar
[52]	Kawamura Y, Shibata T, Inoue A, Masumoto T 1999 Mater. Trans., JIM 40 335 Google Scholar
[53]	Rösner P, Samwer K, Lunkenheimer P 2004 Europhys. Lett. 68 226 Google Scholar
[54]	Zhang P, Maldonis J J, Liu Z, Schroers J, Voyles P M 2018 Nat. Commun. 9 1129 Google Scholar
[55]	Polk D E, Turnbull D 1972 Acta Metall. 20 493 Google Scholar
[56]	Spaepen F 1977 Acta Metall. 25 407 Google Scholar
[57]	Heggen M, Spaepen F, Feuerbacher M 2004 J. Appl. Phys. 97 033506
[58]	Fan Y, Iwashita T, Egami T 2014 Nat. Commun. 5 5083 Google Scholar
[59]	Fan Y, Iwashita T, Egami T 2015 Phys. Rev. Lett. 115 045501 Google Scholar
[60]	Duan Y J, Zhang L T, Qiao J C, Wang Y J, Yang Y, Wada T, Kato H, Pelletier J M, Pineda E, Crespo D 2022 Phys. Rev. Lett. 129 175501 Google Scholar
[61]	Mayr S G 2006 Phys. Rev. Lett. 97 195501 Google Scholar
[62]	Wagner H, Bedorf D, Küchemann S, Schwabe M, Zhang B, Arnold W, Samwer K 2011 Nat. Mater. 10 439 Google Scholar
[63]	Chen H S 1980 Rep. Prog. Phys. 43 353 Google Scholar
[64]	Zhang L T, Wang Y J, Yang Y, Qiao J C 2023 Sci. China Phys. Mech. 66 286111 Google Scholar
[65]	Afonin G V, Mitrofanov Y P, Kobelev N P, da Silva Pinto M W, Wilde G, Khonik V A 2019 Scr. Mater. 166 6 Google Scholar

施引文献

图 1 (a) (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅非晶合金内耗随温度演化(升温速率为2 K/min, 加载频率为1 Hz); (b) (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅非晶合金损耗模量随温度演化, 实线为准点缺陷公式拟合β弛豫结果

Fig. 1. (a) Temperature dependence of the internal friction for (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅ amorphous alloy (heating rate: 2 K/min, driving frequency: 1 Hz); (b) temperature dependence of the loss modulus $ {E}'' $ for (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅ amorphous alloy. The solid line is the β relaxation result fitted by QPD theory.

下载: 全尺寸图片幻灯片

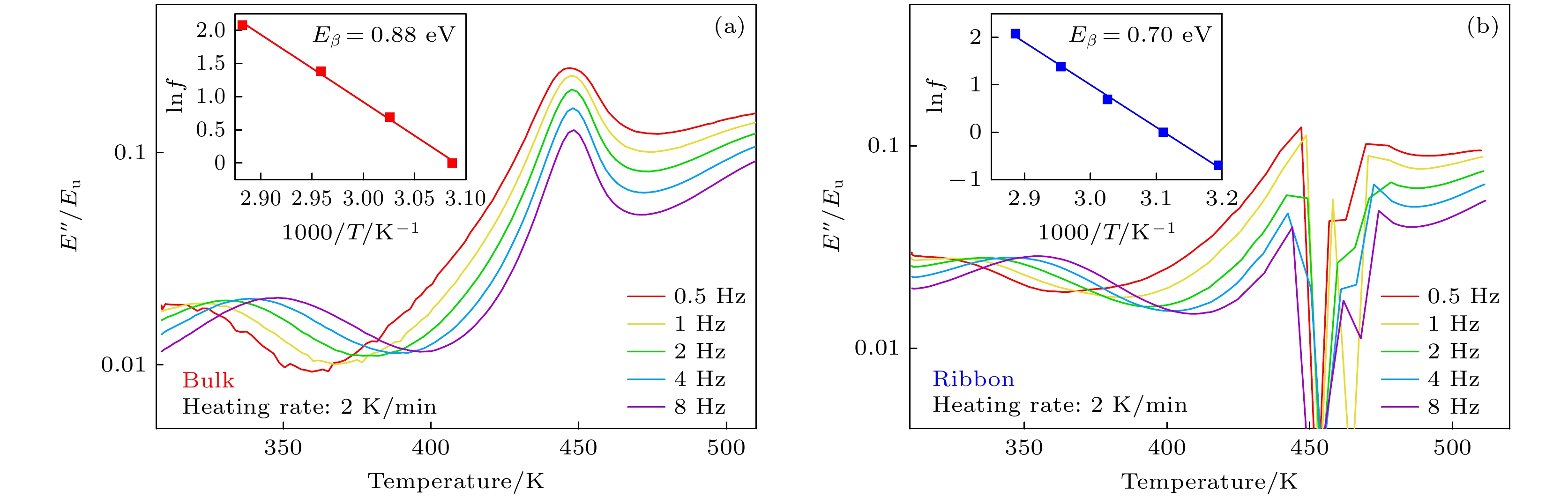
图 2 (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅非晶合金不同加载频率归一化损耗模量$ {E''}/{E}_{{\mathrm{u}}} $随温度演化　(a)块体样品; (b) 条带样品; 升温速率为2 K/min; 插图为β弛豫峰温与加载频率关系, 实线为Arrhenius方程拟合

Fig. 2. Temperature dependence of the normalized loss modulus ${E''}/{E}_{{\mathrm{u}}} $ for (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅ amorphous alloy with different frequency: (a) Bulk; (b) ribbon. Inset shows the frequency versus peak temperature of the β relaxation, the solid line is the Arrhenius equation fit.

下载: 全尺寸图片幻灯片

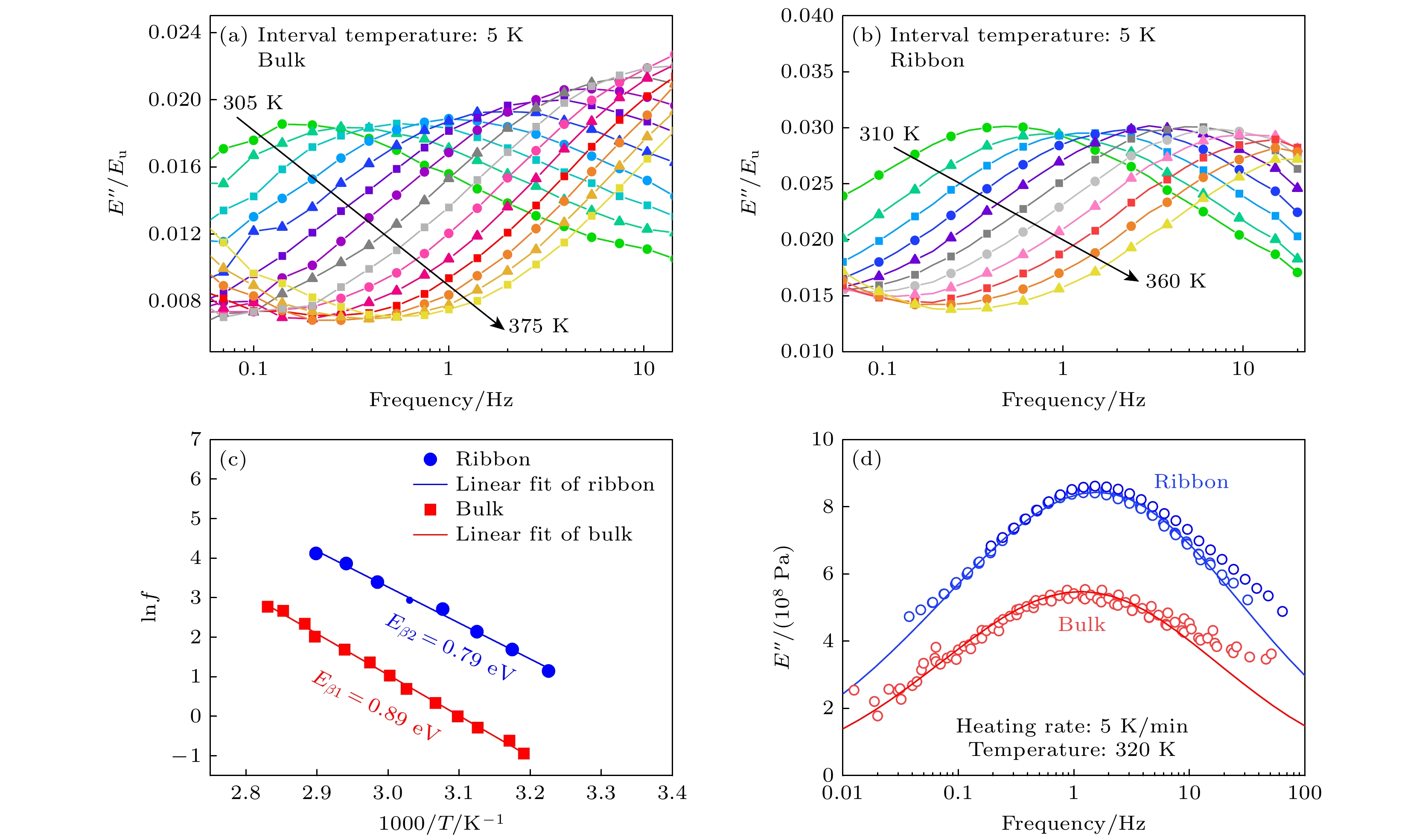
图 3 (a) 不同温度下(La_0.6Ce_0.4)₆₅Al₁₀Co₂₅非晶合金块体样品归一化损耗模量$ {E''}/{E}_{{\mathrm{u}}} $随加载频率演化^[51]; (b) 不同温度下(La_0.6Ce_0.4)₆₅Al₁₀Co₂₅非晶合金条带样品归一化损耗模量$ {E''}/{E}_{{\mathrm{u}}} $随加载频率演化; (c) (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅非晶合金块体样品和条带样品等温扫频条件β弛豫峰温和频率的关系, 实线为Arrhenius方程拟合; (d) (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅非晶合金块体样品和条带样品的损耗模量在320 K参考温度下主曲线(实线为(1)式拟合结果)

Fig. 3. (a) Frequency dependence of the normalized loss modulus ${E''}/{E}_{{\mathrm{u}}} $ for (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅ amorphous alloy with different temperature (bulk); (b) frequency dependence of the normalized loss modulus ${E''}/{E}_{{\mathrm{u}}} $ for (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅ amorphous alloy with different temperature (ribbon); (c) the frequency versus peak temperature of the β relaxation with different states (bulk and ribbon), the solid line is the Arrhenius equation fit; (d) main curve of loss modulus for amorphous alloy with different states (bulk sample and ribbon sample) at 320 K (solid line is fitting result of Eq. (1)).

下载: 全尺寸图片幻灯片

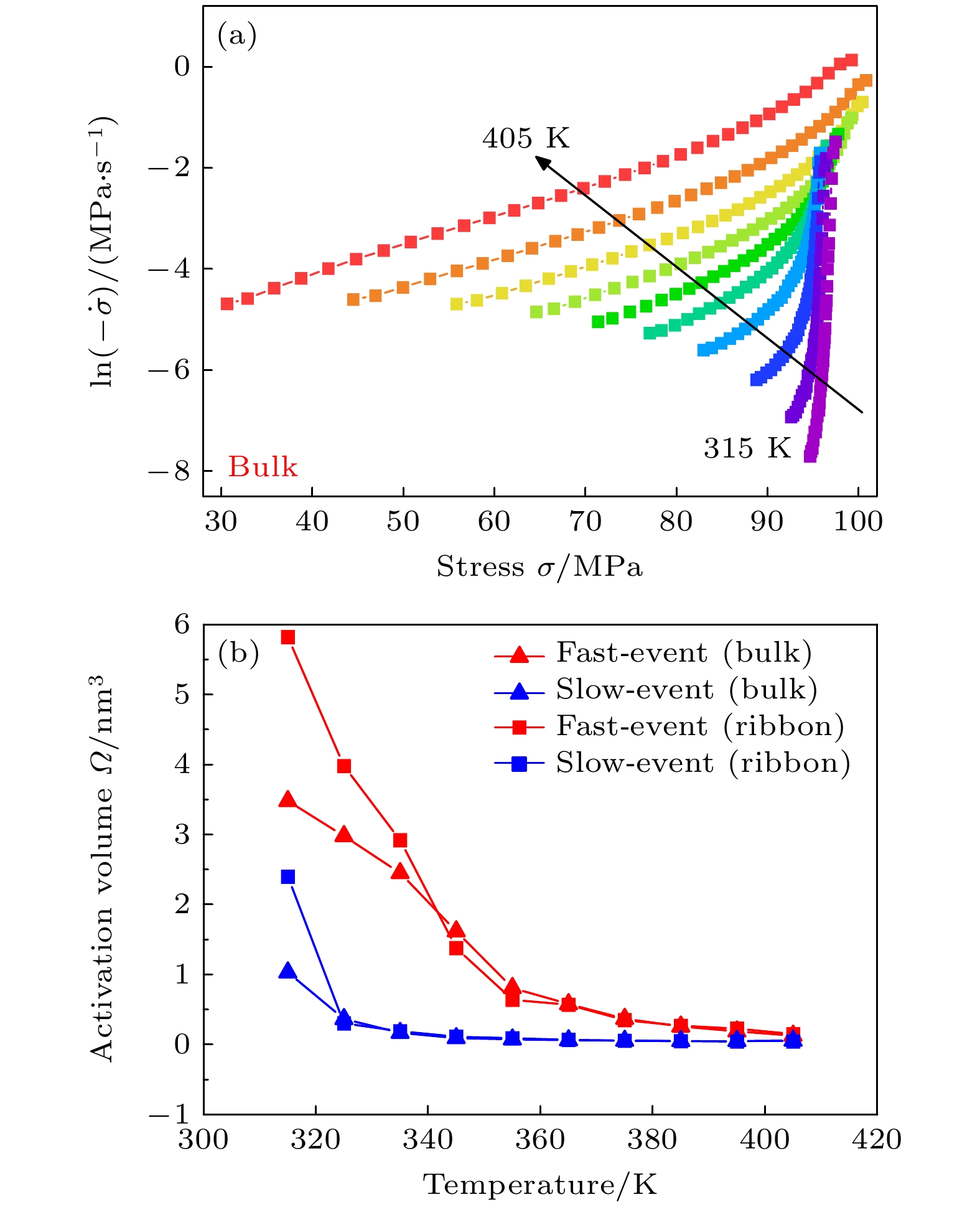
图 4 (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅非晶合金在310—425 K 的应力弛豫曲线(实线为KWW 方程拟合结果)　(a) 块体样品; (b) 条带样品; (c) 370 K下块体样品及条带样品应力弛豫曲线; (d) (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅非晶合金应力弛豫过程中特征弛豫时间$ {\tau }_{{\mathrm{c}}} $对数随温度演化

Fig. 4. Stress relaxation curve of (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅ amorphous alloy from 310 K to 425 K (solid line is the fitting results based on KWW equation): (a) Bulk; (b) ribbon; (c) stress relaxation curves of 370 K for bulk and ribbon; (d) temperature dependence of the logarithm of characteristic relaxation time $ {\tau }_{{\mathrm{c}}} $ in stress relaxation process for (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅ amorphous alloy.

下载: 全尺寸图片幻灯片

图 5 (a) (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅非晶合金块体样品$\ln(-\dot{\sigma }) $与应力关系; (b) 快过程和慢过程的激活体积随温度演化

Fig. 5. (a) Dependence of $ \ln(-\dot{\sigma }) $ on stress for (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅ amorphous alloy bulk sample; (b) temperature dependence of the derived activation volume for the fast and slow events.

下载: 全尺寸图片幻灯片

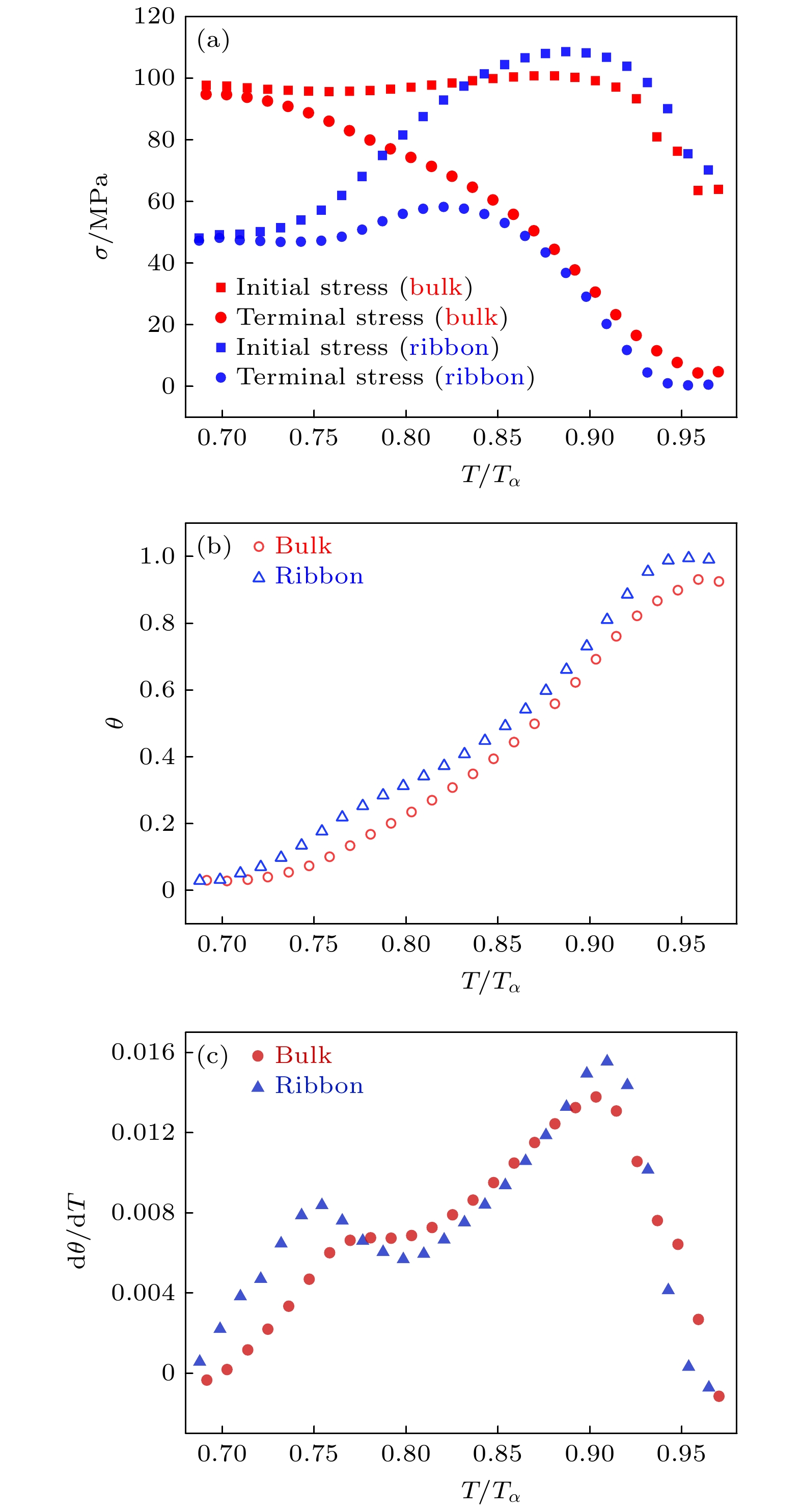
图 6 (a) (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅非晶合金不同温度下应力弛豫曲线初始应力和最终应力演化; (b) $ \theta $随温度演化; (c) $ {\mathrm{d}}\theta /{\mathrm{d}}T $随温度演化

Fig. 6. (a) Initial stress and terminal stress evolution of stress relaxation curves for (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅ amorphous alloy at different temperatures; (b) temperature dependence of the $ \theta $ for bulk and ribbon samples; (c) temperature dependence of the $ {\mathrm{d}}\theta /{\mathrm{d}}T $ for bulk and ribbon samples.

下载: 全尺寸图片幻灯片

图 7 块体样品和条带样品(La_0.6Ce_0.4)₆₅Al₁₀Co₂₅非晶合金DSC曲线

Fig. 7. DSC curves of (La_0.6Ce_0.4)₆₅Al₁₀Co₂₅ amorphous alloy for bulk and ribbon samples.

下载: 全尺寸图片幻灯片

map

[1]	Angell C A 1996 Curr. Opin. Solid State Mater. Sci. 1 578 Google Scholar
[2]	Greer A L 1995 Science 267 1947 Google Scholar
[3]	Chang C, Zhang H P, Zhao R, Li F C, Luo P, Li M Z, Bai H Y 2022 Nat. Mater. 21 1240 Google Scholar
[4]	Qiao J C, Wang Q, Pelletier J M, Kato H, Casalini R, Crespo D, Pineda E, Yao Y, Yang Y 2019 Prog. Mater. Sci. 104 250
[5]	Wang Q, Liu J J, Ye Y F, Liu T T, Wang S, Liu C T, Lu J, Yang Y 2017 Mater. Today 20 293 Google Scholar
[6]	Wang Q, Shang Y H, Yang Y 2023 Mater. Futures 2 017501 Google Scholar
[7]	Wang W H 2019 Prog. Mater. Sci. 106 100561 Google Scholar
[8]	乔吉超, 张浪渟, 童钰, 吕国建, 郝奇, 陶凯 2022 力学进展 52 117 Google Scholar Qiao J C, Zhang L T, Tong Y, Lyu G J, Hao Q, Tao K 2022 Adv. Mech. 52 117 Google Scholar
[9]	Wang X D, Ruta B, Xiong L H, Zhang D W, Chushkin Y, Sheng H W, Lou H B, Cao Q P, Jiang J Z 2015 Acta Mater. 99 290 Google Scholar
[10]	胡丽娜, 张春芝, 岳远征, 边秀房 2010 科学通报 55 115 Hu L N, Zhang C Z, Yue Y Z, Bian X F 2010 Sci. Bull. 55 115
[11]	Capaccioli S, Paluch M, Prevosto D, Wang L M, Ngai K L 2012 J. Phys. Chem. Lett. 3 735 Google Scholar
[12]	Ngai K L, Wang Z, Gao X Q, Yu H B, Wang W H 2013 J. Chem. Phys. 139 014502 Google Scholar
[13]	Wang W H 2011 J. Appl. Phys. 110 053521 Google Scholar
[14]	Yu H B, Wang W H, Bai H Y, Wu Y, Chen M V 2010 Phys. Rev. B 81 220201 Google Scholar
[15]	Yu H B, Wang W H, Bai H Y, Samwer K 2014 Nat. Sci. Rev. 1 429 Google Scholar
[16]	Li N, Xu X N, Zheng Z Z, Liu L 2014 Acta Mater. 65 400 Google Scholar
[17]	Yu H B, Samwer K, Wu Y, Wang W H 2012 Phys. Rev. Lett. 109 095508 Google Scholar
[18]	Yu H B, Wang W H, Samwer K 2013 Mater. Today 16 183 Google Scholar
[19]	Ashby M F, Greer A L 2006 Scr. Mater. 54 321 Google Scholar
[20]	Dyre J C 2006 Rev. Mod. Phys. 78 953 Google Scholar
[21]	Ediger M D, Harrowell P 2012 J. Chem. Phys. 137 080901 Google Scholar
[22]	Goldstein M 2011 J. Non-Cryst. Solids 357 249 Google Scholar
[23]	Hu L, Yue Y 2009 J. Phys. Chem. C 113 15001 Google Scholar
[24]	Wang W H, Dong C, Shek C H 2004 Mater. Sci. Eng. , R 44 45 Google Scholar
[25]	汪卫华 2013 物理学进展 33 177 Wang W H 2013 Prog. Phys. 33 177
[26]	Zhao L Z, Wang W H, Bai H Y 2014 J. Non-Cryst. Solids 405 207 Google Scholar
[27]	Ma Z J, Guo Y C, Li Y, Lugee T, Gao P H, Yang Z, Zeng X R 2015 J. Alloys Compd. 648 18 Google Scholar
[28]	Wang B, Shang B S, Gao X Q, Wang W H, Bai H Y, Pan M X, Guan P F 2016 J. Phys. Chem. Lett. 7 4945 Google Scholar
[29]	Zhang L T, Duan Y J, Wang Y J, Yang Y, Qiao J C 2023 Sci. China-Technol. Sci. 66 173 Google Scholar
[30]	Jiao W, Sun B A, Wen P, Bai H Y, Kong Q P, Wang W H 2013 Appl. Phys. Lett. 103 101903 Google Scholar
[31]	Ke H B, Zhang P, Sun B A, Zhang P G, Liu T W, Chen P H, Wu M, Huang H G 2019 J. Alloys Compd. 788 391 Google Scholar
[32]	Milkus R, Zaccone A 2017 Phys. Rev. E 95 023001 Google Scholar
[33]	Qiao J C, Wang Y J, Pelletier J M, Keer L M, Fine M E, Yao Y 2015 Acta Mater. 98 43 Google Scholar
[34]	Qiao J C, Wang Y J, Zhao L Z, Dai L H, Crespo D, Pelletier J M, Keer L M, Yao Y 2016 Phys. Rev. B 94 104203 Google Scholar
[35]	Liang S Y, Zhang L T, Wang B, Wang Y J, Pineda E, Qiao J C 2024 Intermetallics 164 108115 Google Scholar
[36]	Luo P, Li M X, Jiang H Y, Wen P, Bai H Y, Wang W H 2017 J. Appl. Phys. 121 135104 Google Scholar
[37]	Luo P, Wen P, Bai H Y, Ruta B, Wang W H 2017 Phys. Rev. Lett. 118 225901 Google Scholar
[38]	Jiao W, Wen P, Peng H L, Bai H Y, Sun B A, Wang W H 2013 Appl. Phys. Lett. 102 101903 Google Scholar
[39]	Wang Z, Sun B A, Bai H Y, Wang W H 2014 Nat. Commun. 5 5823 Google Scholar
[40]	汪卫华 2014 中国科学: 物理学力学天文学 44 396 Google Scholar Wang W H, 2014 Sci. Sin. Phys. Mech. Astr. 44 396 Google Scholar
[41]	Li R, Pang S, Ma C L, Zhang T 2007 Acta Mater. 55 3719 Google Scholar
[42]	Lin X H, Johnson W L 1995 J. Appl. Phys. 78 6514 Google Scholar
[43]	Perez J 1990 Solid State Ionics 39 69 Google Scholar
[44]	Perez J, Etienne S, Tatibouät J 1990 Phys. Status Solidi A 121 129 Google Scholar
[45]	Qiao J C, Pelletier J M 2014 J. Mater. Sci. Technol. 30 523 Google Scholar
[46]	Wang Q, Pelletier J M, Blandin J J, Suery M 2005 J. Non-Cryst. Solids 351 2224 Google Scholar
[47]	Perez J 2018 Physics and Mechanics of Amorphous Polymers (London: Routledge) pp117–119
[48]	Rinaldi R, Gaertner R, Chazeau L, Gauthier C 2011 Int. J. Non-Linear Mech. 46 496 Google Scholar
[49]	Evenson Z, Naleway S E, Wei S, Gross O, Kruzic J J, Gallino I, Possart W, Stommel M, Busch R 2014 Phys. Rev. B 89 174204 Google Scholar
[50]	Wang B, Gao X Q, Qiao J C 2024 Rare Met. Mater. Eng. 53 70 Google Scholar
[51]	孟绍怡, 郝奇, 吕国建, 乔吉超 2023 72 076101 Google Scholar Meng S Y, Hao Q, Lyu G J, Qiao J C 2023 Acta Phys. Sin. 72 076101 Google Scholar
[52]	Kawamura Y, Shibata T, Inoue A, Masumoto T 1999 Mater. Trans., JIM 40 335 Google Scholar
[53]	Rösner P, Samwer K, Lunkenheimer P 2004 Europhys. Lett. 68 226 Google Scholar
[54]	Zhang P, Maldonis J J, Liu Z, Schroers J, Voyles P M 2018 Nat. Commun. 9 1129 Google Scholar
[55]	Polk D E, Turnbull D 1972 Acta Metall. 20 493 Google Scholar
[56]	Spaepen F 1977 Acta Metall. 25 407 Google Scholar
[57]	Heggen M, Spaepen F, Feuerbacher M 2004 J. Appl. Phys. 97 033506
[58]	Fan Y, Iwashita T, Egami T 2014 Nat. Commun. 5 5083 Google Scholar
[59]	Fan Y, Iwashita T, Egami T 2015 Phys. Rev. Lett. 115 045501 Google Scholar
[60]	Duan Y J, Zhang L T, Qiao J C, Wang Y J, Yang Y, Wada T, Kato H, Pelletier J M, Pineda E, Crespo D 2022 Phys. Rev. Lett. 129 175501 Google Scholar
[61]	Mayr S G 2006 Phys. Rev. Lett. 97 195501 Google Scholar
[62]	Wagner H, Bedorf D, Küchemann S, Schwabe M, Zhang B, Arnold W, Samwer K 2011 Nat. Mater. 10 439 Google Scholar
[63]	Chen H S 1980 Rep. Prog. Phys. 43 353 Google Scholar
[64]	Zhang L T, Wang Y J, Yang Y, Qiao J C 2023 Sci. China Phys. Mech. 66 286111 Google Scholar
[65]	Afonin G V, Mitrofanov Y P, Kobelev N P, da Silva Pinto M W, Wilde G, Khonik V A 2019 Scr. Mater. 166 6 Google Scholar

[1]	张婧祺, 郝奇, 吕国建, 熊必金, 乔吉超. 基于微观结构非均匀性理解非晶态聚苯乙烯的应力松弛行为. , 2024, 73(3): 037601. doi: 10.7498/aps.73.20231240
[2]	张剑, 郝奇, 张浪渟, 乔吉超. 不同力学激励形式探索La基非晶合金微观结构非均匀性. , 2024, 73(4): 046101. doi: 10.7498/aps.73.20231421
[3]	孟绍怡, 郝奇, 吕国建, 乔吉超. La基非晶合金β弛豫行为: 退火和加载应变的影响. , 2023, 72(7): 076101. doi: 10.7498/aps.72.20222389
[4]	黄蓓蓓, 郝奇, 吕国建, 乔吉超. 锆基非晶合金的动态弛豫和应力松弛. , 2023, 72(13): 136101. doi: 10.7498/aps.72.20230181
[5]	武振伟, 汪卫华. 非晶态物质原子局域连接度与弛豫动力学. , 2020, 69(6): 066101. doi: 10.7498/aps.69.20191870
[6]	周边, 杨亮. 分子动力学模拟冷却速率对非晶合金结构与变形行为的影响. , 2020, 69(11): 116101. doi: 10.7498/aps.69.20191781
[7]	柳延辉. 非晶合金的高通量制备与表征. , 2017, 66(17): 176106. doi: 10.7498/aps.66.176106
[8]	陈娜, 张盈祺, 姚可夫. 源于非晶合金的透明磁性半导体. , 2017, 66(17): 176113. doi: 10.7498/aps.66.176113
[9]	柯海波, 蒲朕, 张培, 张鹏国, 徐宏扬, 黄火根, 刘天伟, 王英敏. 铀基非晶合金的发展现状. , 2017, 66(17): 176104. doi: 10.7498/aps.66.176104
[10]	冯涛, Horst Hahn, Herbert Gleiter. 纳米结构非晶合金材料研究进展. , 2017, 66(17): 176110. doi: 10.7498/aps.66.176110
[11]	孙星, 默广, 赵林志, 戴兰宏, 吴忠华, 蒋敏强. 小角X射线散射表征非晶合金纳米尺度结构非均匀. , 2017, 66(17): 176109. doi: 10.7498/aps.66.176109
[12]	王峥, 汪卫华. 非晶合金中的流变单元. , 2017, 66(17): 176103. doi: 10.7498/aps.66.176103
[13]	卞西磊, 王刚. 非晶合金的离子辐照效应. , 2017, 66(17): 178101. doi: 10.7498/aps.66.178101
[14]	管鹏飞, 王兵, 吴义成, 张珊, 尚宝双, 胡远超, 苏锐, 刘琪. 不均匀性：非晶合金的灵魂. , 2017, 66(17): 176112. doi: 10.7498/aps.66.176112
[15]	高鹏飞, 刘铁, 柴少伟, 董蒙, 王强. 磁感应强度和冷却速率对Tb0.27Dy0.73Fe1.95合金凝固过程中取向行为的影响. , 2016, 65(3): 038104. doi: 10.7498/aps.65.038104
[16]	郑乃超, 刘海蓉, 刘让苏, 梁永超, 莫云飞, 周群益, 田泽安. 冷速对液态合金Ca50Zn50快速凝固过程中微观结构演变的影响. , 2012, 61(24): 246102. doi: 10.7498/aps.61.246102
[17]	闫志杰, 李金富, 周尧和, 仵彦卿. 压痕塑性变形诱导非晶合金的晶化. , 2007, 56(2): 999-1003. doi: 10.7498/aps.56.999
[18]	范隆, 郝跃. 辐射感生应力弛豫对AlmGa1－mN/GaN HEMT电学特性的影响. , 2007, 56(6): 3393-3399. doi: 10.7498/aps.56.3393
[19]	程伟东, 孙民华, 李佳云, 王爱屏, 孙永丽, 刘芳, 刘雄军. Cu60Zr30Ti10非晶合金弛豫和晶化过程的小角X射线散射研究. , 2006, 55(12): 6673-6676. doi: 10.7498/aps.55.6673
[20]	周效锋, 陶淑芬, 刘佐权, 阚家德, 李德修. Fe73.5Cu1Nb3Si13.5B9非晶合金的激波纳米晶化速率和晶化度的对比研究. , 2002, 51(2): 322-325. doi: 10.7498/aps.51.322

计量

文章访问数: 2363
PDF下载量: 73
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

冷却速率对La基非晶合金β弛豫行为和应力弛豫的影响