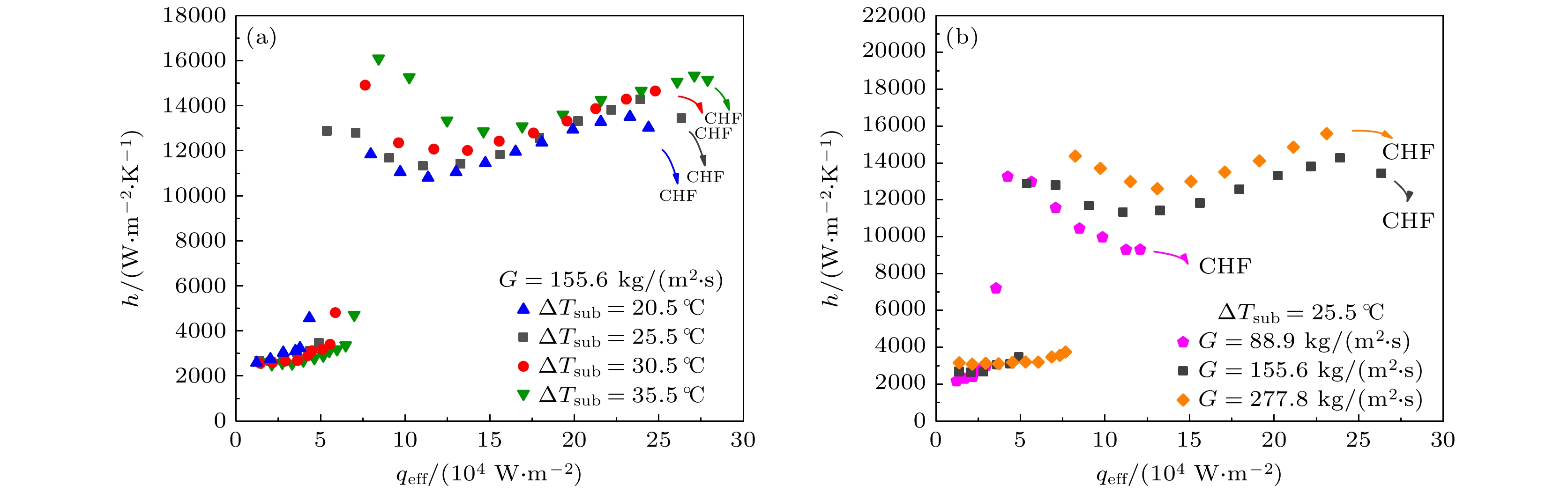
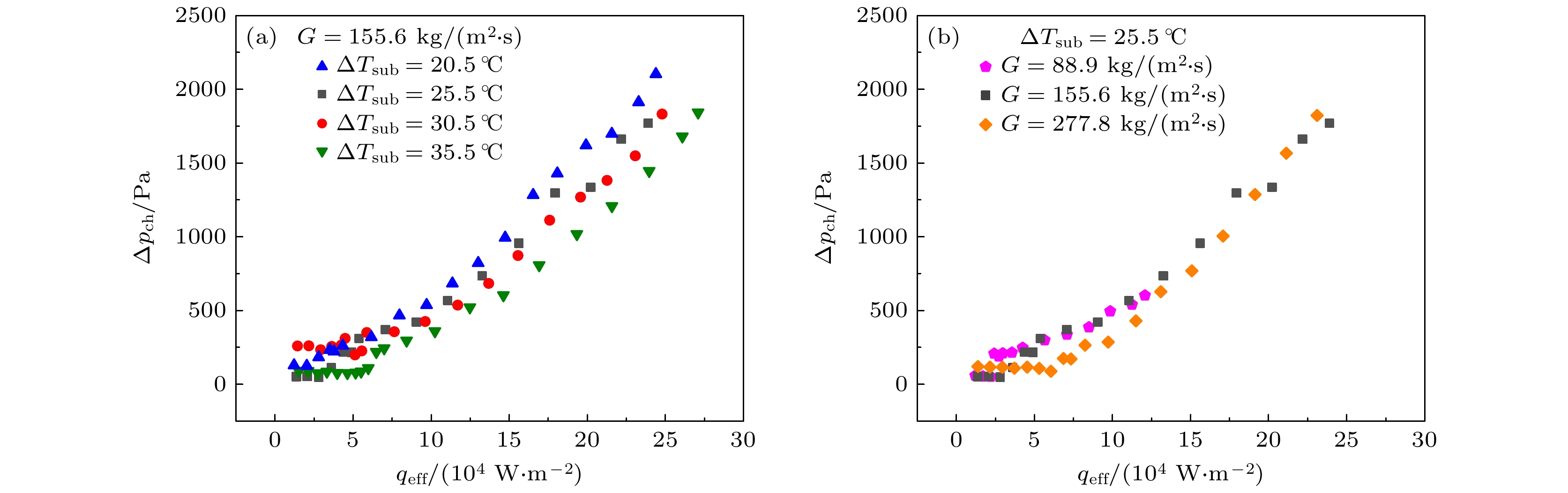
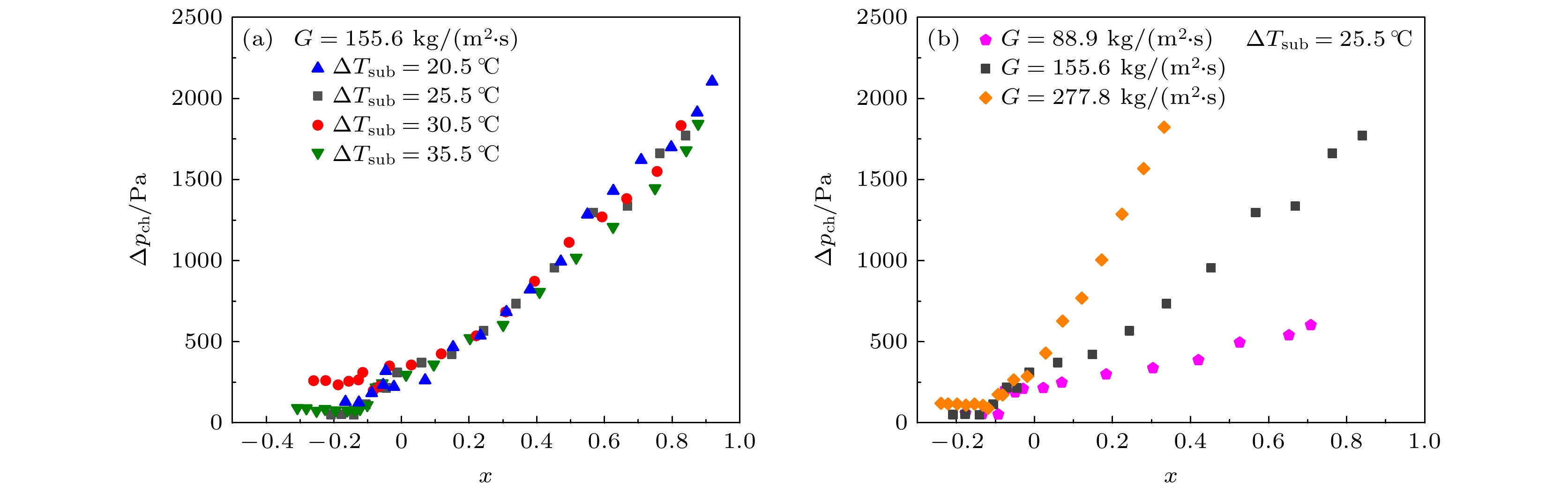
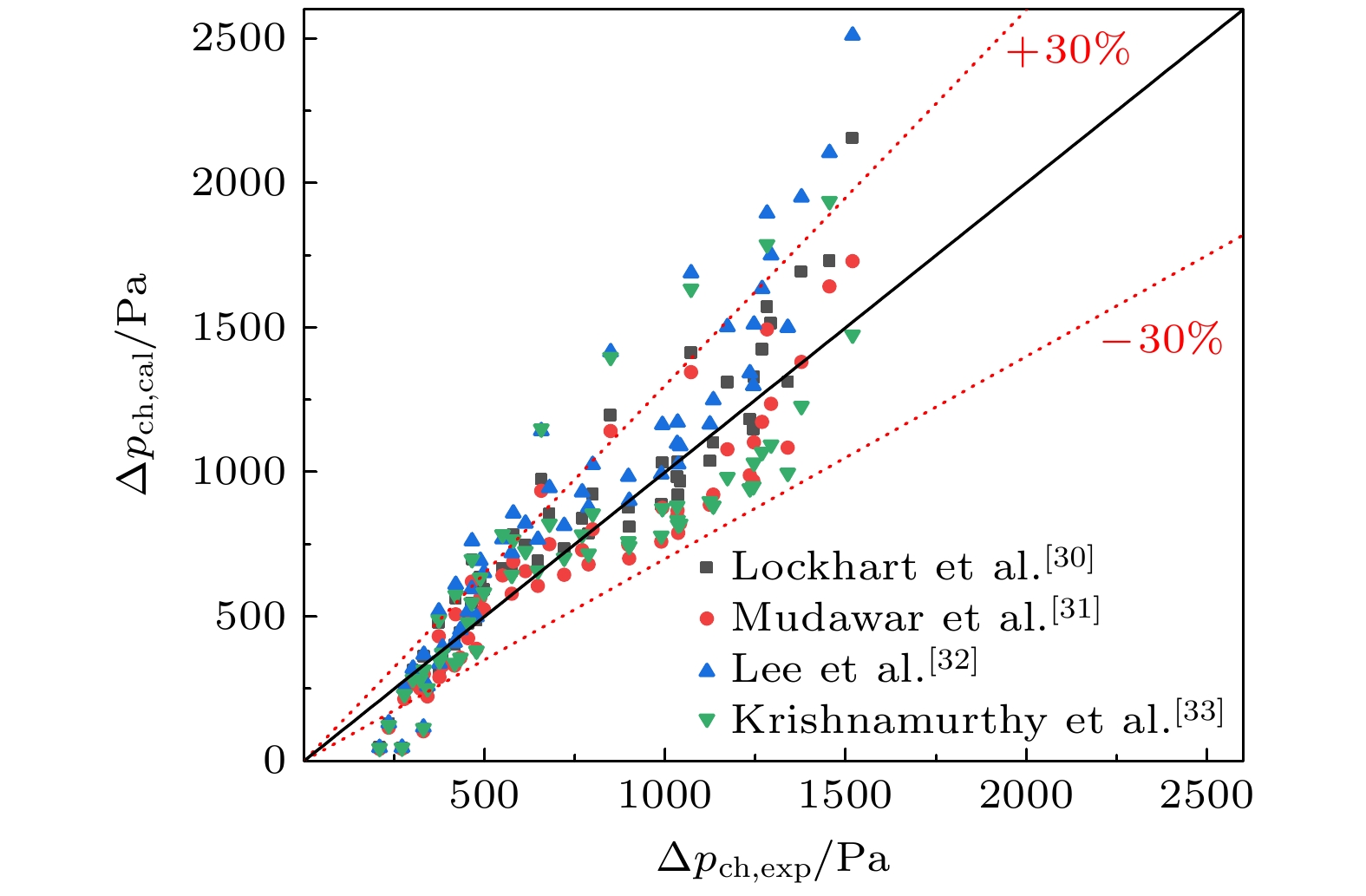
-
微通道流动沸腾冷却技术兼具相变潜热和微尺度效应的诸多优点, 是解决微电子器件热致失效问题的重要方法之一. HFE-7100是一种安全环保的电子氟化液, 特别适用于微电子器件的冷却. 本文在水力直径为0.5 mm的矩形平行微通道内, 对HFE-7100的流动沸腾传热和两相流动特性进行了实验研究， 测量范围为常压下质量流率88.9—277.8 kg·m–2·s–1、入口过冷度20.5—35.5 ℃和有效热流密度12—279 kW·m–2. 本文分析了质量流率、入口过冷度、有效热流密度和干度对传热系数和压降的影响, 发现在较低的入口过冷度下HFE-7100出现了沸腾迟滞现象, 且增大入口过冷度和质量流率会延缓沸腾起始点的发生, 且会提高传热系数和临界热流密度. 两相压降受有效热流密度影响较大, 且在定干度下不同质量流率的两相压降在塞状流和环状流阶段有明显差异. 同时, 通过观测两相流型, 对流动沸腾传热现象进行了分析. 本文还将两相压降实验数据与文献关联式预测值进行了对比, 与Lockhart提出的关联式预测值偏差为19.6%. 本文研究结果可为微电子器件散热设备的优化设计提供理论指导: 以HFE-7100作为传热工质并将微通道流动沸腾冷却技术应用于微电子器件散热设备, 可以提高设备的稳定性和可靠性; 在不同热流密度的设备中通过控制入口过冷度和质量流率可有效地提升其散热性能; 采用Lockhart提出的两相压降关联式可预测散热设备所需的泵功.With the rapid development of microelectronic technology, the integration and power of chip are increasing. Heat dissipation with high heat flux in limited space has become a bottleneck restricting the efficient and stable operation of the microelectronic devices. Flow boiling in microchannel heat sink is one of the most essential candidates for solving this problem. It has been shown that remarkable high heat transfer performance can be achieved through the liquid-to-vapor change process, which can dissipate a large amount of heat from a small area. In addition, dielectric fluorinated fluids, such as HFE-7100, HFE-7200, and FC-72, are especially suitable for cooling microelectronic devices, because of their excellent safety and environmental characteristics. However, dielectric fluorinated fluids have poorer thermophysical properties than water. Thus, the flow boiling heat transfer characteristics of dielectric fluorinated fluids can be different from those of water. In this work, flow boiling heat transfer and flow characteristics of HFE-7100 in a rectangular parallel microchannel are investigated. The tests are conducted at mass fluxes from 88.9 to 277.8 kg·m–2·s–1, inlet subcooling temperature from 20.5 to 35.5 ℃ and effective heat flux from 12 to 279 kW·m–2 at nearly atmospheric pressure. The effects of mass flux, inlet subcooling temperature, effective heat flux and vapor quality are examined and analyzed. Additionally, flow visualization is also obtained to explain the heat transfer mechanism during the experiments. The results show that the boiling hysteresis is observed for HFE-7100 at low inlet subcooling temperature, and the increasing inlet subcooling temperature and mass flux can delay the onset of nucleate boiling. The increases of inlet subcooling temperature and mass flux can enhance the two-phase heat transfer coefficient. And the two-phase heat transfer coefficient is significantly dependent on the inlet subcooling temperature in the slug flow, while it is significantly dependent on the mass flux in the annular flow. The two-phase pressure drop increases drastically as the effective heat flux increases. And the two-phase pressure drops with different mass fluxes at constant vapor quality are obviously different between the slug flow and the annular flow. Furthermore, the experimental data are compared with four predicted values of the literature. It is found that the correlation of Lockhart has the best statistical agreement with an MAE of 19.6% and over 85% of points in the deviation bandwidth of ±30%. The results in this paper give valuable theoretical guidance for designing and optimizing heat dissipation equipment for microelectronic devices. By utilizing HFE-7100 as the coolant and microchannel heat sinks in flow boiling, it is possible to enhance the stability and reliability of the electronic devices. Additionally, the heat transfer performance associated with different heat fluxes can be improved by regulating the inlet subcooling and mass flow rate. Finally, the two-phase pressure drop correlation proposed by Lockhart can be employed to predict the pump power for heat dissipation equipment.
-
Keywords:
- flow boiling /
- microchannel /
- HFE-7100 /
- heat transfer /
- electronic devices
[1] Karayiannis T G, Mahmoud M M 2017 Appl. Therm. Eng. 115 1372
Google Scholar
[2] Manetti L L, Ribatski G, de Souza R R, Cardoso E M 2020 Exp. Therm. Fluid Sci. 113 110025
Google Scholar
[3] Harirchian T, Garimella S V 2009 Int. J. Multiphase Flow 35 349
Google Scholar
[4] Asrar P, Ghiaasiaan S M, Joshi Y K. 2021 J. Heat Transfer 143 023001
Google Scholar
[5] 杨晓强, 秋穗正, 贾晓鸿, 尹海峰, 贾斗南, 卢冬华 2007 核动力工程 28 38
Google Scholar
Yang X Q, Qiu S Z, Jia X H, Yin H F, Jia D N, Lu D H 2007 Nucl. Power Eng. 28 38
Google Scholar
[6] Wang Y, Sefiane K 2012 Int. J. Heat Mass Transfer 55 2235
Google Scholar
[7] Al-Zaidi A H, Mahmoud M M, Karayiannis T G 2019 Int. J. Heat Mass Transfer 140 100
Google Scholar
[8] Al-Zaidi A H, Mahmoud M M, Karayiannis T G 2021 Int. J. Heat Mass Transfer 164 120587
Google Scholar
[9] Cheng X, Wu H 2023 Exp. Therm. Fluid Sci. 142 110805
Google Scholar
[10] Yang K S, Jeng Y R, Huang C M, Wang C C 2011 Heat Transfer Eng. 32 697
Google Scholar
[11] Tuo H, Hrnjak P 2013 Int. J. Refrig. 36 1263
Google Scholar
[12] Rausch M H, Kretschmer L, Will S, Leipertz A, Froba A P 2015 J. Chem. Eng. Data 60 3759
Google Scholar
[13] Liang G, Mudawar I 2020 Int. J. Heat Mass Transfer 146 118864
Google Scholar
[14] Cui P, Liu Z 2021 Int. J. Heat Mass Transfer 175 121387
Google Scholar
[15] Feng S, Yan Y, Lai C 2022 Int. J. Heat Mass Transfer 186 122468
Google Scholar
[16] Zhuang X R, Yu P, Li X, Deng Q Y 2020 PRC Patent CN202122664314 [2021-11-02
[17] Abu-Eishah S I 2001 Int. J. Thermophys. 22 1855
Google Scholar
[18] Machrafi H, Sadoun N, Rednikov A, Dehaeck S, Dauby P C, Colinet P 2013 Microgravity Sci. Technol. 25 251
Google Scholar
[19] Nassar M, Vazquez P, Chauris N, Daaboul M B, Louste C 2020 IEEE Trans. Ind. Appl. 56 4193
Google Scholar
[20] Qu W, Siu-Ho A 2008 J. Heat Transfer 130 122402
Google Scholar
[21] Blevins R D 1984 Applied Fluid Dynamics Handbook (New York: Van Nostrand Reinhold) pp12–20
[22] Taylor B N, Kuyatt C E 1994 Guidelines for Evaluating and Expressing the Uncertainty of NIST Measurement Results (Gaithersburg: Department of Commerce, Technology Administration, National Institute of Standards and Technology) pp11–20
[23] Žukauskas A 1972 Adv. Heat Transfer 8 93
[24] Chang J Y, Prasher R, Chau D, Myers A, He D 2005 International Electronic Packaging Technical Conference and Exhibition San Francisco, California, USA, July 17–22, 2005 p183
[25] Brunschwiler T, Michel B, Rothuizen H, Kloter U, Wunderle B, Oppermann H, Reichl H 2009 Microsyst. Technol. 15 57
Google Scholar
[26] Xu F, Wu H 2018 J. Heat Transfer 140 122401
Google Scholar
[27] Kong L J, Liu Z G, Jia L, Lv M M, Liu Y 2020 Exp. Therm. Fluid Sci. 115 109946
Google Scholar
[28] Ming H S, Ma J, Bu X W 1993 Int. J. Heat Mass Transfer 36 4461
Google Scholar
[29] Zhuang X R, Xie Y C, Li X, Yue S J, Wang H T, Wang H D, Yu P 2023 Appl. Therm. Eng. 225 120180
Google Scholar
[30] Lockhart W R 1949 Chem. Eng. Prog. 45 39
[31] Mudawar I, Qu W 2003 Int. J. Heat Mass Transfer 46 2737
Google Scholar
[32] Lee H J, Lee S Y 2001 Int. J. Multiphase Flow 27 2043
Google Scholar
[33] Krishnamurthy S, Peles Y 2007 Phys. Fluids 19 043302
Google Scholar
-
图 2 微通道测试段结构图 (a) 紫铜热沉热电偶排布; (b) 测试段组装示意图; (c) 平行微通道结构尺寸
Fig. 2. Schematic view of the test section: (a) Thermocouple arrangement on the copper heat sink; (b) assembly drawing; (c) dimensional drawing of the microchannels.
图 3 单相 (a) Nu-Re和 (b) f-Re 的验证结果
Fig. 3. Verifications of single-phase (a) Nu-Re and (b) f-Re
图 4 不同(a)入口过冷度和(b)质量流率下HFE-7100的沸腾传热曲线
Fig. 4. Boiling heat transfer curve of HFE-7100 under different operating conditions: (a) Inlet subcooling temperature; (b) mass flux
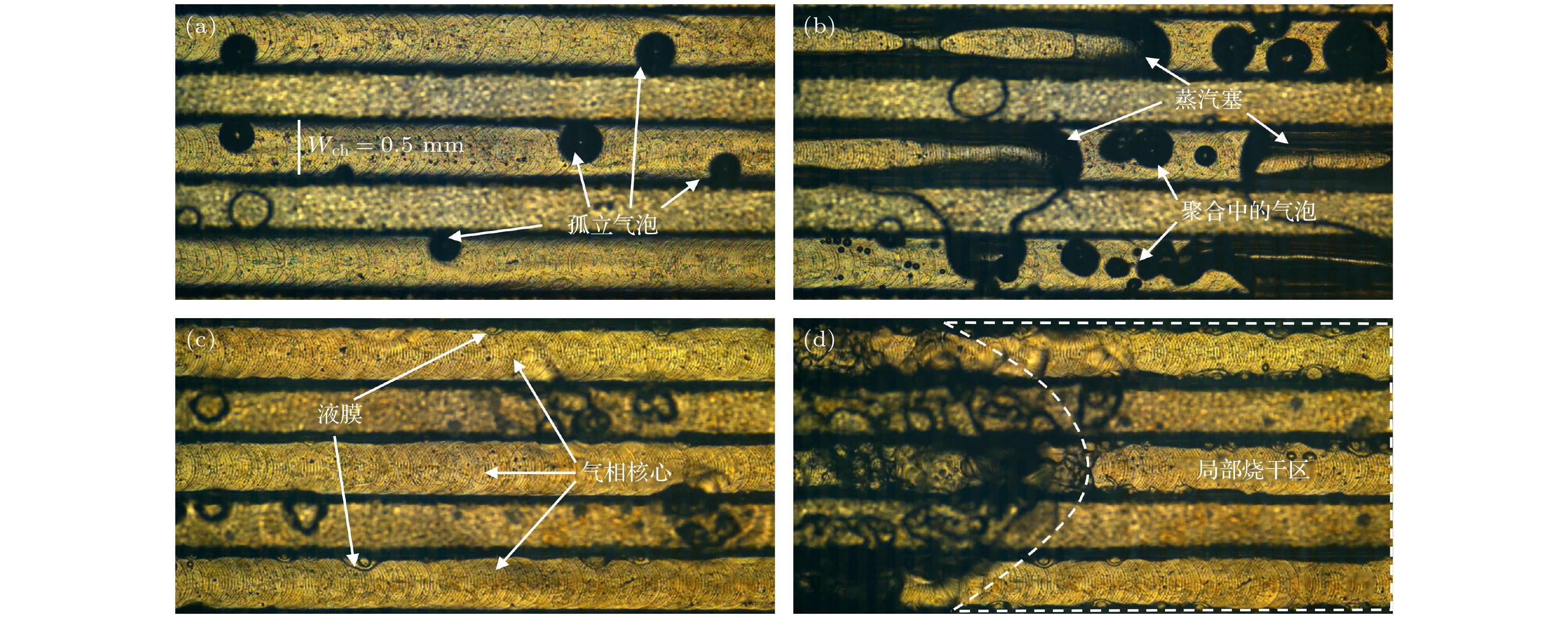
图 5 G = 155.6 kg·m–2·s–1和∆Tsub = 25.5 ℃下, HFE-7100的沸腾两相流动形态 (a) 泡状流 (qeff = 90.6 kW·m–2); (b) 塞状流 (qeff = 110.6 kW·m–2); (c) 环状流 (qeff = 202.3 kW·m–2); (d) 局部烧干状态 (qeff = 263.4 kW·m–2)
Fig. 5. Boiling two-phase flow patterns of HFE-7100 at G = 155.6 kg·m–2·s–1 and ∆Tsub = 25.5℃: (a) Bubbly flow (qeff = 90.6 kW·m–2); (b) slug flow (qeff = 110.6 kW·m–2); (c) annular flow (qeff = 202.3 kW·m–2); (d) local drying state (qeff = 263.4 kW·m–2).
图 6 不同(a)入口过冷度和(b)质量流率下HFE-7100流动沸腾传热系数随有效热流密度的变化
Fig. 6. The variation of flow boiling heat transfer coefficient of HFE-7100 with respect to the effective heat flux density under different operating conditions: (a) Inlet subcooling temperature; (b) mass flux.
图 7 不同(a)入口过冷度和(b) 质量流率下HFE-7100流动沸腾传热系数随干度的变化
Fig. 7. The variation of flow boiling heat transfer coefficient of HFE-7100 with dryness under different operating conditions: (a) Inlet subcooling temperature; (b) mass flux.
图 8 不同(a)入口过冷度和(b)质量流率下HFE-7100流动沸腾沿程压降随有效热流密度的变化
Fig. 8. The variation of flow boiling heat transfer pressure drop of HFE-7100 with effective heat flux density under different operating conditions: (a) Inlet subcooling temperature; (b) mass flux.
图 9 不同(a)入口过冷度和(b)质量流率下HFE-7100流动沸腾沿程压降随干度的变化
Fig. 9. The variation of flow boiling heat transfer pressure drop of HFE-7100 with dryness under different operating conditions: (a) Inlet subcooling temperature; (b) mass flux.
图 10 平行微通道两相压降实验结果与文献关联式预测值对比
Fig. 10. Comparison of experimental results of two-phase pressure drop in the microchannel with predicted values from literature correlations.
表 1 测量参数的不确定度
Table 1. Uncertainty of measurement parameters.
测量参数 测试设备 测量范围 不确定度 温度 Omega T/K 0—300 ℃ ±0.2 ℃ 压力 Star CYYZ11 0—0.4 MPa ±0.1% 压差 Star CCY15 0—20 kPa ±0.25% 质量流量 Bronkhorst MINI CORI-FLOW M14 0.3—15 kg·h–1 ±0.2% 长度 LINKS游标卡尺 0—150 mm ±0.01 mm 两相热流密度 — — 2.2%—9.1% 两相传热系数 — — 2.5%—9.7% 干度 — — 1.7%—6.3% 
下载: 导出CSV
表 2 两相压降关联式计算结果与实验结果的对比
Table 2. Comparison between the calculated results of the two-phase pressure drop correlation and the experimental results
文献 两相压降关联式 MAE/% δ/% [30] $ {X_{vv}} = {\left( {\dfrac{{{\mu _{{\text{l, out, t}}}}}}{{{\mu _{{\text{g, out, t}}}}}}} \right)^{0.274}}{\left( {\dfrac{{1 - {x_{{\text{out }}}}}}{{{x_{{\text{out }}}}}}} \right)^{0.727}}{\left( {\dfrac{{{\text{ }}R{e_{{\text{g, out, t}}}}}}{{R{e_{{\text{l, out, t}}}}}}} \right)^{0.5}} $, ${f_{\text{l}}} = \dfrac{{20.09}}{{Re_{{\text{l, out}}\;}^{0.547}}}$ 19.6 85.5 $\phi _{\text{f}}^2 = 1 + \dfrac{5}{{{X_{vv}}}} + \dfrac{1}{{X_{vv}^2}}$, $\Delta {p_{{\text{ch, cal}}}} = \dfrac{{2{f_{\text{l}}}{G^2}\phi _{\text{f}}^2\left( {L - {S_{\text{L}}}} \right)}}{{3{\rho _{\text{l}}}{D_{{\text{ch}}}}}}\left( {x_{{\text{out}}\;}^2 - 3{x_{{\text{out}}\;}} + 3} \right)$ [31] $ {X_{vv}} = {\left( {\dfrac{{{\mu _{{\text{l, out, t}}}}}}{{{\mu _{{\text{g, out, t}}}}}}} \right)^{0.274}}{\left( {\dfrac{{1 - {x_{{\text{out }}}}}}{{{x_{{\text{out }}}}}}} \right)^{0.727}}{\left( {\dfrac{{{\text{ }}R{e_{{\text{g, out, t}}}}}}{{R{e_{{\text{l, out, t}}}}}}} \right)^{0.5}} $, ${f_{\text{l}}} = \dfrac{{20.09}}{{Re_{{\text{l, out, t}}}^{{0}{.547}}}}$ 20.6 85.5 ${C_{\text{M}}} = 21\left( {1 - {{\text{e}}^{ - 319{D_{{\text{fin}}}}}}} \right)\left( {0.00418 G + 0.0613} \right)$, $\phi _{\text{f}}^2 = 1 + \dfrac{{{C_{\text{M}}}}}{{{X_{vv}}}} + \dfrac{1}{{X_{vv}^2}}$ $\Delta {p_{{\text{ch, cal}}}} = \dfrac{{2{f_{\text{l}}}{G^2}\phi _{\text{f}}^2\left( {L - {S_{\text{L}}}} \right)}}{{3{\rho _{\text{l}}}{D_{{\text{ch}}}}}}\left( {x_{{\text{out}}\;}^2 - 3{x_{{\text{out}}\;}} + 3} \right)$ [32] $ {X_{vv}} = {\left( {\dfrac{{{\mu _{{\text{l, out, t}}}}}}{{{\mu _{{\text{g, out, t}}}}}}} \right)^{0.274}}{\left( {\dfrac{{1 - {x_{{\text{out }}}}}}{{{x_{{\text{out }}}}}}} \right)^{0.727}}{\left( {\dfrac{{{\text{ }}R{e_{{\text{g, out, t}}}}}}{{R{e_{{\text{l, out, t}}}}}}} \right)^{0.5}} $, ${f_{\text{l}}} = \dfrac{{20.09}}{{Re_{{\text{l, out, t}}}^{{0}{.547}}}}$ 27.8 63.6 ${C_{\text{L}}} = 2566{G^{0.5466}}D_{{\text{ch}}}^{0.8819}\left( {1 - {{\rm e} ^{ - 319{D_{{\text{ch}}}}}}} \right)$, $\phi _{\text{f}}^2 = 1 + \dfrac{{{C_{\text{L}}}}}{{{X_{vv}}}} + \dfrac{1}{{X_{vv}^2}}$ $\Delta {p_{{\text{ch, cal}}}} = \dfrac{{2{f_{\text{l}}}{G^2}\phi _{\text{f}}^2\left( {L - {S_{\text{L}}}} \right)}}{{3{\rho _{\text{l}}}{D_{{\text{ch}}}}}}\left( {x_{{\text{out}}\;}^2 - 3{x_{{\text{out}}\;}} + 3} \right)$ [33] $ {X_{vv}} = {\left( {\dfrac{{{\mu _{{\text{l, out, t}}}}}}{{{\mu _{{\text{g, out, t}}}}}}} \right)^{0.274}}{\left( {\dfrac{{1 - {x_{{\text{out }}}}}}{{{x_{{\text{out }}}}}}} \right)^{0.727}}{\left( {\dfrac{{{\text{ }}R{e_{{\text{g, out, t}}}}}}{{R{e_{{\text{l, out, t}}}}}}} \right)^{0.5}} $, ${f_{\text{l}}} = \dfrac{{20.09}}{{Re_{{\text{l, out, t}}}^{0.547}}}$ 25.5 74.5 ${C_{\text{K}}} = \dfrac{{0.0358}}{{Re_{{\text{l, out, t}}}^{{0}{.547}}}}$, $\phi _{\text{f}}^2 = 1 + \dfrac{{{C_{\text{K}}}}}{{{X_{vv}}}} + \dfrac{1}{{X_{vv}^2}}$ $\Delta {p_{{\text{ch, cal}}}} = \dfrac{{2{f_{\text{l}}}{G^2}\phi _{\text{f}}^2\left( {L - {S_{\text{L}}}} \right)}}{{3{\rho _{\text{l}}}{D_{{\text{ch}}}}}}\left( {x_{{\text{out}}\;}^2 - 3{x_{{\text{out}}\;}} + 3} \right)$
下载: 导出CSV
-
[1] Karayiannis T G, Mahmoud M M 2017 Appl. Therm. Eng. 115 1372
Google Scholar
[2] Manetti L L, Ribatski G, de Souza R R, Cardoso E M 2020 Exp. Therm. Fluid Sci. 113 110025
Google Scholar
[3] Harirchian T, Garimella S V 2009 Int. J. Multiphase Flow 35 349
Google Scholar
[4] Asrar P, Ghiaasiaan S M, Joshi Y K. 2021 J. Heat Transfer 143 023001
Google Scholar
[5] 杨晓强, 秋穗正, 贾晓鸿, 尹海峰, 贾斗南, 卢冬华 2007 核动力工程 28 38
Google Scholar
Yang X Q, Qiu S Z, Jia X H, Yin H F, Jia D N, Lu D H 2007 Nucl. Power Eng. 28 38
Google Scholar
[6] Wang Y, Sefiane K 2012 Int. J. Heat Mass Transfer 55 2235
Google Scholar
[7] Al-Zaidi A H, Mahmoud M M, Karayiannis T G 2019 Int. J. Heat Mass Transfer 140 100
Google Scholar
[8] Al-Zaidi A H, Mahmoud M M, Karayiannis T G 2021 Int. J. Heat Mass Transfer 164 120587
Google Scholar
[9] Cheng X, Wu H 2023 Exp. Therm. Fluid Sci. 142 110805
Google Scholar
[10] Yang K S, Jeng Y R, Huang C M, Wang C C 2011 Heat Transfer Eng. 32 697
Google Scholar
[11] Tuo H, Hrnjak P 2013 Int. J. Refrig. 36 1263
Google Scholar
[12] Rausch M H, Kretschmer L, Will S, Leipertz A, Froba A P 2015 J. Chem. Eng. Data 60 3759
Google Scholar
[13] Liang G, Mudawar I 2020 Int. J. Heat Mass Transfer 146 118864
Google Scholar
[14] Cui P, Liu Z 2021 Int. J. Heat Mass Transfer 175 121387
Google Scholar
[15] Feng S, Yan Y, Lai C 2022 Int. J. Heat Mass Transfer 186 122468
Google Scholar
[16] Zhuang X R, Yu P, Li X, Deng Q Y 2020 PRC Patent CN202122664314 [2021-11-02
[17] Abu-Eishah S I 2001 Int. J. Thermophys. 22 1855
Google Scholar
[18] Machrafi H, Sadoun N, Rednikov A, Dehaeck S, Dauby P C, Colinet P 2013 Microgravity Sci. Technol. 25 251
Google Scholar
[19] Nassar M, Vazquez P, Chauris N, Daaboul M B, Louste C 2020 IEEE Trans. Ind. Appl. 56 4193
Google Scholar
[20] Qu W, Siu-Ho A 2008 J. Heat Transfer 130 122402
Google Scholar
[21] Blevins R D 1984 Applied Fluid Dynamics Handbook (New York: Van Nostrand Reinhold) pp12–20
[22] Taylor B N, Kuyatt C E 1994 Guidelines for Evaluating and Expressing the Uncertainty of NIST Measurement Results (Gaithersburg: Department of Commerce, Technology Administration, National Institute of Standards and Technology) pp11–20
[23] Žukauskas A 1972 Adv. Heat Transfer 8 93
[24] Chang J Y, Prasher R, Chau D, Myers A, He D 2005 International Electronic Packaging Technical Conference and Exhibition San Francisco, California, USA, July 17–22, 2005 p183
[25] Brunschwiler T, Michel B, Rothuizen H, Kloter U, Wunderle B, Oppermann H, Reichl H 2009 Microsyst. Technol. 15 57
Google Scholar
[26] Xu F, Wu H 2018 J. Heat Transfer 140 122401
Google Scholar
[27] Kong L J, Liu Z G, Jia L, Lv M M, Liu Y 2020 Exp. Therm. Fluid Sci. 115 109946
Google Scholar
[28] Ming H S, Ma J, Bu X W 1993 Int. J. Heat Mass Transfer 36 4461
Google Scholar
[29] Zhuang X R, Xie Y C, Li X, Yue S J, Wang H T, Wang H D, Yu P 2023 Appl. Therm. Eng. 225 120180
Google Scholar
[30] Lockhart W R 1949 Chem. Eng. Prog. 45 39
[31] Mudawar I, Qu W 2003 Int. J. Heat Mass Transfer 46 2737
Google Scholar
[32] Lee H J, Lee S Y 2001 Int. J. Multiphase Flow 27 2043
Google Scholar
[33] Krishnamurthy S, Peles Y 2007 Phys. Fluids 19 043302
Google Scholar
下载:
计量
- 文章访问数: 3172
- PDF下载量: 108
- 被引次数: 0
