-
在聚变辐照环境下, 面向等离子体材料钨的近表层中会产生位错环缺陷, 影响材料的力学性能和氢/氦滞留. 本文通过分子动力学方法, 从原子层次研究了半径1 nm的
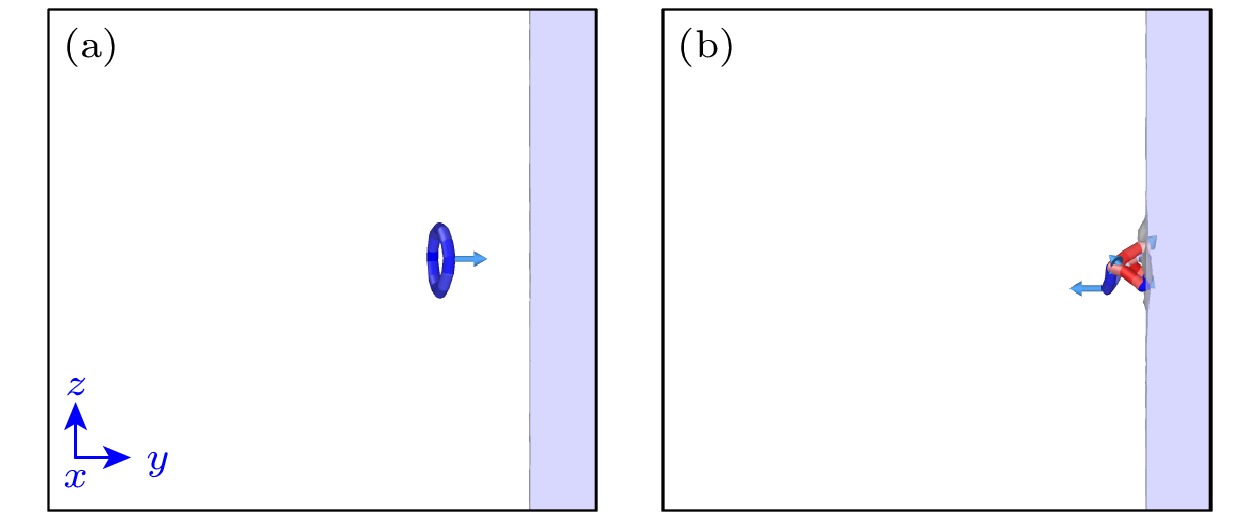
$\langle 100\rangle $ 间隙位错环在W-$(010)$ 近表面的迁移行为, 分析取向、温度、深度因素和间隙原子对的存在对位错环运动的影响. 结果表明:$ {\boldsymbol{b}}/ / {\boldsymbol{n}} $ ( b 代表柏氏矢量, n 代表表面法线方向)位错环易向表面移动,$ {\boldsymbol{b}} \bot {\boldsymbol{n}} $ 位错环倾向滞留在材料内, 移动过程中伴随着惯习面翻转和内应力释放;$ {\boldsymbol{b}}/ / {\boldsymbol{n}} $ 位错环在温度高于800 K且深度小于5 nm时逸出表面的概率超过90%, 其他模拟情况下基本全滞留;$ {\boldsymbol{b}} \bot {\boldsymbol{n}} $ 位错环可在温度800 K且深度小于2 nm时逸出表面, 而在更深处位错环不易移动, 但会在温度升高时发生$\langle 100\rangle $ 环分解为${1 \mathord{\left/ {\vphantom {1 2}} \right. } 2}\langle 111\rangle $ 位错的现象; 氦原子对的存在阻碍位错环迁移, 延长其在材料内的滞留时间, 同时内应力场的变化导致氦在近表层分布不均匀.In the fusion irradiation environment, dislocation loop defects occurring under plasma-facing tungsten surface affect its mechanical properties and hydrogen/helium retention. This paper studies the dynamic behaviors of a$\langle 100\rangle $ loop with a radius of 1 nm under the W$(010)$ surface by using molecular dynamics simulation at the atomic level. It is found that the dislocation loop direction, bulk temperature, depth, and helium atoms can greatly affect the motion of dislocation loops, showing that the$ {\boldsymbol{b}}/ / {\boldsymbol{n}} $ dislocation loop, where$ {\boldsymbol{b}} $ is the Burgers vector and$ {\boldsymbol{n}} $ denotes the surface normal direction, tends to move towards the surface and the$ {\boldsymbol{b}} \bot {\boldsymbol{n}} $ dislocation loop tends to stay in the material. In the course of its migration, the habit plane of dislocation loop may change and the internal stress decreases gradually. The probability of a$ {\boldsymbol{b}}/ / {\boldsymbol{n}} $ dislocation loops escaping from the surface is over 90% when the temperature is higher than 800 K and their initial depth is less than 5 nm. The$ {\boldsymbol{b}} \bot {\boldsymbol{n}} $ dislocation loop can escape from the surface when the temperature is 800 K and the initial depth is less than 2 nm. It is found that$\langle 100\rangle $ dislocation loops decompose into${1 \mathord{\left/ {\vphantom {1 2}} \right. } 2}\langle 111\rangle $ dislocations at elevated temperatures. Helium atoms impede the migration of dislocation loops and increase their retention time. The existence of dislocation loops results in the uneven distribution of helium atoms under the W surface, and will potentially affect the surface morphology of tungsten.-
Keywords:
- molecular dynamics simulation /
- dislocation loop /
- image force /
- helium
[1] Krasheninnikov S I 2022 Plasma Phys. Control. Fusion 64 124005
Google Scholar
[2] Janeschitz G 2001 J. Nucl. Mater. 290–293 1
Google Scholar
[3] Philipps V 2011 J. Nucl. Mater. 415 S2
Google Scholar
[4] El-Atwani O, El-Atwani E, Esquivel E, Efe M, Wang Y Q, Maloy S A 2018 Acta Mater. 147 277
Google Scholar
[5] 马玉田, 刘俊标, 韩立, 田利丰, 王雪聪, 孟祥敏, 肖善曲, 王波 2019 68 040702
Google Scholar
Ma Y T, Liu J B, Han L, Tian L F, Wang X C, Meng X M, Xiao S Q, Wang B 2019 Acta Phys. Sin. 68 040702
Google Scholar
[6] Cui M H, Gao N, Wang D, Gao X, Wang Z G 2019 Nucl. Instrum. Meth. B 460 60
Google Scholar
[7] Bernard E, Sakamoto R, Yoshida N, Yamada H 2015 J. Nucl. Mater. 463 316
Google Scholar
[8] Grigorev P, Bakaev A, Terentyev D, Van Oost G, Noterdaeme J M, Zhurkin E E 2017 Nucl. Instrum. Methods Phys. Res. Sect. B-Beam Interact. Mater. Atoms 393 164
Google Scholar
[9] Matsui T, Muto S, Tanabe T 2000 J. Nucl. Mater. 283 1139
Google Scholar
[10] Guo W G, Wang S W, Ge L, Fu E G, Yuan Y, Cheng L, Lu G H 2020 Nucl. Fusion 60 034002
Google Scholar
[11] El-Atwani O, Cunningham W S, Trelewicz J R, Li M, Wirth B D, Maloy S A 2020 J. Nucl. Mater. 538 152150
Google Scholar
[12] Harrison R W, Greaves G, Hinks J A, Donnelly S E 2017 J. Nucl. Mater. 495 492
Google Scholar
[13] Yi X O, Jenkins M L, Kirk M A, Zhou Z F, Roberts S G 2016 Acta Mater. 112 105
Google Scholar
[14] 贺冉 2020 硕士学位论文 (兰州: 兰州理工大学)
He R 2020 M. S. Thesis ( Lanzhou: Lanzhou University of Technology
[15] Yi X O, Jenkins M L, Hattar K, Edmondson P D, Roberts S G 2015 Acta Mater. 92 163
Google Scholar
[16] Groves P P, Bacon D J 1970 Philos. Mag. 22 83
Google Scholar
[17] Fikar J, Gröger R, Schäublin R 2017 Nucl. Instrum. Meth. B 393 186
Google Scholar
[18] Juslin N, Wirth B D 2013 J. Nucl. Mater. 432 61
Google Scholar
[19] Marinica M C, Ventelon L, Gilbert M R, Proville L, Dudarev S L, Marian J, Bencteux G, Willaime F 2013 J. Phys. Condens. 25 395502
Google Scholar
[20] Harder J M, Bacon D J 1988 Phil. Mag. A 58 165
Google Scholar
[21] Chen L, Liu Y L, Zhou H B, Jin S, Zhang Y, Lu G H 2012 Sci. China-Phys. Mech. Astron. 55 614
Google Scholar
[22] Gao N, Shen T L, Kurtz R, Wang Z G, Gao F 2017 Scr. Mater. 136 64
Google Scholar
[23] Sharafat S, Takahashi A, Hu Q, Ghoniem N M 2009 J. Nucl. Mater. 386–388 900
Google Scholar
[24] Zhang H Y, Sun J Z, Wang Y M, Stirner T, Hamid A Y, Sang C F 2020 Fusion Eng. Des. 161 112004
Google Scholar
-
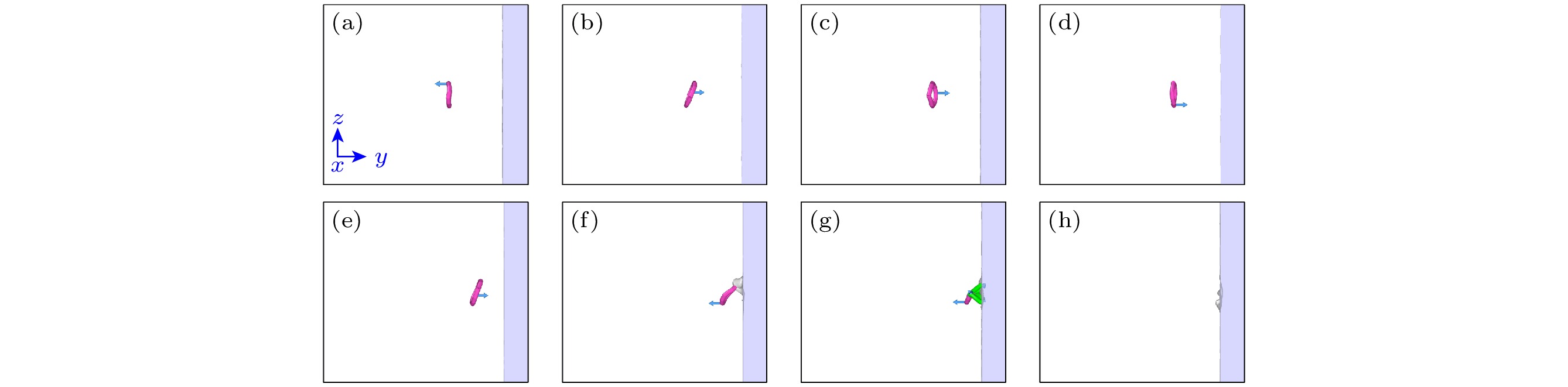
图 1 $ {\boldsymbol{b}}/ / {\boldsymbol{n}} $位错环的取向变化和分解($T = 800$ K, 初始深度$h = 5$ nm) (a) $t = 0.001$; (b) $t = 0.018$; (c) $t = 0.115$; (d) $t = $$ 0.170$; (e) $t = 0.239$; (f) $t = 0.245$; (g) $t = 0.246$; (h) $t = 0.247$; 图中粉红颜色代表$\langle 100\rangle $位错, 绿颜色代表${1 \mathord{\left/ {\vphantom {1 2}} \right. } 2}\langle 111\rangle $位错, 蓝色箭头表示柏氏矢量方向
Fig. 1. Orientational change and decomposition of a $ {\boldsymbol{b}}/ / {\boldsymbol{n}} $ dislocation loop at $T = 800$ K and the initial depth of $h = 5$ nm: (a) $t = 0.001$; (b) $t = 0.018$; (c) $t = 0.115$; (d) $t = 0.170$; (e) $t = 0.239$; (f) $t = 0.245$; (g) $t = 0.246$; (h) $t = 0.247$. The pink loop stands for the $\langle 100\rangle $ type dislocation, the green one for ${1 \mathord{\left/ {\vphantom {1 2}} \right. } 2}\langle 111\rangle $. The blue arrows denote the direction of Burgers vector.
图 2 $ {\boldsymbol{b}}/ / {\boldsymbol{n}} $位错环移动过程中的应力变化图($T = 800$ K, 初始深度$h = 5$ nm) (a)—(e) 位错环向表面移动过程中的应力图, 其中(a) t = 0.014, (b) t = 0.018, (c) t = 0.066, (d) t = 0.115, (e) t = 0.239; (f) 位错环完全移出表面后的应力图, t = 0.247
Fig. 2. Stress variation of a $ {\boldsymbol{b}}/ / {\boldsymbol{n}} $ dislocation loop at $T = 800$ K and initial depth of $h = 5$ nm in motion: (a)–(e) The stress of the dislocation loop as it moves toward the surface ((a) t = 0.014, (b) t = 0.018, (c) t = 0.066, (d) t = 0.115, (e) t = 0.239); (f) the stress after the dislocation loop has been completely removed from the surface, t = 0.247.
图 3 $ {\boldsymbol{b}}/ / {\boldsymbol{n}} $位错环与表面相互作用后的表面形貌
Fig. 3. Surface morphology after the interaction between $ {\boldsymbol{b}}/ / {\boldsymbol{n}} $ dislocation loop and surface has ceased to occur.
图 4 $ {\boldsymbol{b}}/ / {\boldsymbol{n}} $位错环演化过程中位错类型变化图 (a) 位错环未与表面发生作用时的状态; (b) 位错环与表面发生强烈作用时的状态. 图中蓝色为刃位错, 红色为螺位错
Fig. 4. Variation of the dislocation geometry of a $ {\boldsymbol{b}}/ / {\boldsymbol{n}} $ dislocation loop in motion: (a) The geometry of the dislocation loop away from the surface; (b) the geometry change of the dislocation loop while interacting with the surface. Blue represents the edge dislocation, red represents a screw dislocation.
图 5 $ {\boldsymbol{b}} \bot {\boldsymbol{n}} $ 型位错环的取向变化和分解($T = 800$ K) (a) $t = 0.018$; (b) $t = 0.115$; (c) $t = 0.170$; (d) $t = 0.245$; (e) $t = $$ 0.246$; (f) $t = 0.247$; 图中粉红颜色的为$\langle 100\rangle $型位错, 绿颜色的为${1 \mathord{\left/ {\vphantom {1 2}} \right. } 2}\langle 111\rangle $型位错, 底部物理量代表位错环初始深度
Fig. 5. Orientational change and decomposition of a $ {\boldsymbol{b}} \bot {\boldsymbol{n}} $ dislocation loop at $T = 800$ K: (a) $t = 0.018$; (b) $t = 0.115$; (c) $t = 0.170$; (d) $t = 0.245$; (e) $t = 0.246$; (f) $t = 0.247$. The pink loop stands for the $\langle 100\rangle $ dislocation, the green part for ${1 \mathord{\left/ {\vphantom {1 2}} \right. } 2}\langle 111\rangle $, the physical quantity at the bottom represents the initial depth of the dislocation loop.
图 6 位错环($ {\boldsymbol{b}}/ / {\boldsymbol{n}} $和$ {\boldsymbol{b}} \bot {\boldsymbol{n}} $)形成能随距离表面深度的变化
Fig. 6. Variation of the formation energies of the dislocation loops ($ {\boldsymbol{b}}/ / {\boldsymbol{n}} $ and $ {\boldsymbol{b}} \bot {\boldsymbol{n}} $) with increasing depth.
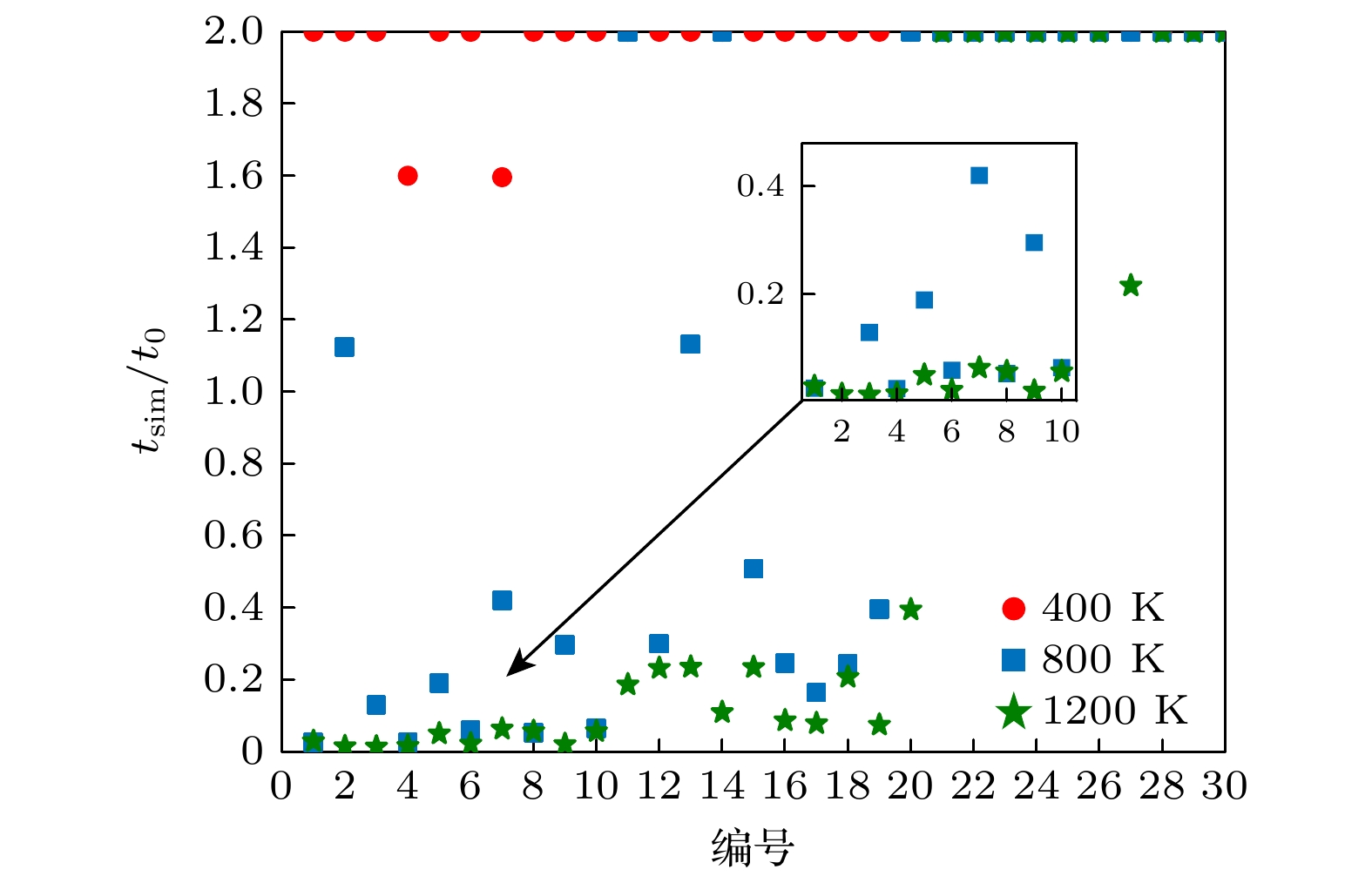
图 8 不同温度和深度下$ {\boldsymbol{b}}/ / {\boldsymbol{n}} $位错环的滞留时间变化, 图中编号1—10, 11—20 和21—30分别是初始深度$h = $$ 3, 5, 7$nm的位错环
Fig. 8. Retention time variation of the $ {\boldsymbol{b}}/ / {\boldsymbol{n}} $ dislocation loops with temperature and depth. The numbers 1−10, 11−20, and 21−30 are used to specify the dislocation loops located at the initial depth of $h = 3, 5, 7$nm, respectively.
图 7 位错环($ {\boldsymbol{b}}/ / {\boldsymbol{n}} $和$ {\boldsymbol{b}} \bot {\boldsymbol{n}} $)映像力随距离表面深度的变化
Fig. 7. Image forces of the dislocation loops ($ {\boldsymbol{b}}/ / {\boldsymbol{n}} $ and $ {\boldsymbol{b}} \bot {\boldsymbol{n}} $) with increasing depth.
图 9 位错环与氦间隙原子对的结合能 (a), (b) $ {\boldsymbol{b}}/ / {\boldsymbol{n}} $位错环分别在方向Ⅰ和方向Ⅱ上的结和能; (c), (d) $ {\boldsymbol{b}} \bot {\boldsymbol{n}} $位错环分别在方向Ⅰ和方向Ⅱ上的结和能, $ {\text{loo}}{{\text{p}}_{1}}—{\text{loo}}{{\text{p}}_{5}} $分别对应$h = 1.5, {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} 2, {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} 2.5, {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} 3, {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} 3.5$ nm的位错环. (a), (c) 横坐标是氦原子对距离位错环的距离; (b), (d) 横坐标是氦原子对距表面的深度
Fig. 9. Binding energy of the dislocation loop with a pair of helium atoms: (a), (b) The binding energies in direction Ⅰ and Ⅱ of $ {\boldsymbol{b}}/ / {\boldsymbol{n}} $ dislocation loops; (c), (d) the binding energies for the $ {\boldsymbol{b}} \bot {\boldsymbol{n}} $ dislocation loop. $ {\text{loo}}{{\text{p}}_{1}}-{\text{loo}}{{\text{p}}_{5}} $ correspond to the dislocation loops located at $h = 1.5, {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} 2, {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} 2.5, {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} 3, {\kern 1 pt} {\kern 1 pt} {\kern 1 pt} 3.5$ nm. The abscissa of (a) and (c) represents the distances between the pair of helium atoms and the dislocation loop, and the ordinate of (b) and (d) is for the depth.
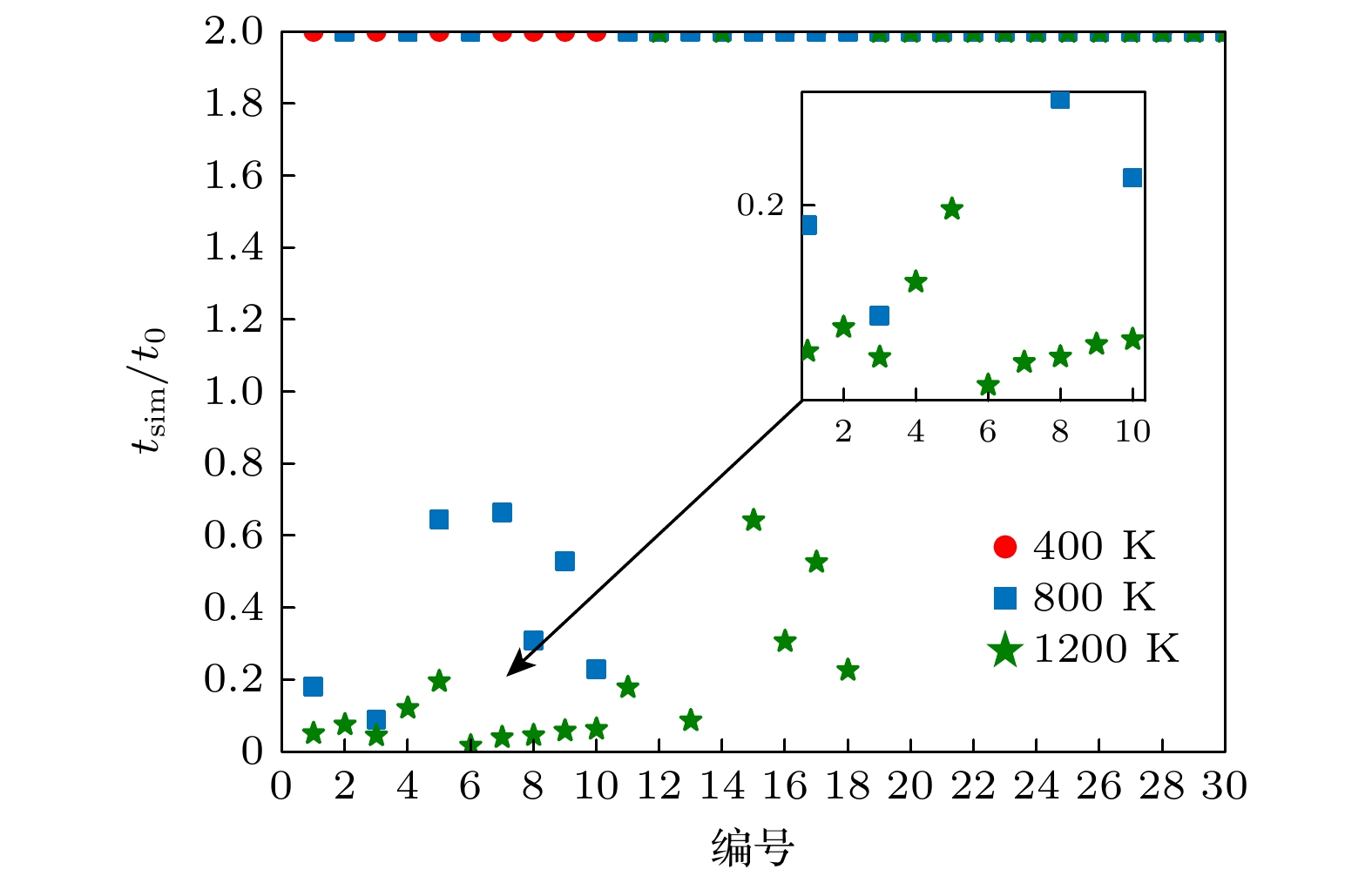
图 10 不同温度下$ {\boldsymbol{b}}/ / {\boldsymbol{n}} $位错环的滞留时间图(体系中含氦原子对) 图中编号1 — 10, 11—20和21—30分别是初始深度h = 3 , 5 , 7 nm的位错环
Fig. 10. Variation of the retention time with temperature and depth for the $ {\boldsymbol{b}}/ / {\boldsymbol{n}} $ dislocation loops existing in the W lattice cell containing a pair of helium atoms. The numbers 1–10, 11–20, and 21–30 are used to specify the dislocation loops located at the initial depth of $h = 3, 5, 7$nm, respectively.
图 A1 不同温度$ {\boldsymbol{b}} \bot {\boldsymbol{n}} $位错环的运动演化过程(初始深度h = 5 nm) (a) $t = 0.980$; (b) $t = 2.000$; (c) $t = 0.980$; (d) $t = 2.000$; (e) $t = 0.540$; (f) $t = 0.960$; (g) $t = 1.600$; (h) $t = 2.000$; 图中粉红颜色的为$\langle 100\rangle $型位错, 绿颜色的为${1 \mathord{\left/ {\vphantom {1 2}} \right. } 2}\langle 111\rangle $型位错
Fig. A1. Orientational change and decomposition of a $ {\boldsymbol{b}} \bot {\boldsymbol{n}} $ dislocation loop at the initial depth of $h = 5$ nm: (a) $t = 0.980$; (b) $t = 2.000$; (c) $t = 0.980$; (d) $t = 2.000$; (e) $t = 0.540$; (f) $t = 0.960$; (g) $t = 1.600$; (h) $t = 2.000$. The pink loop stands for the $\langle 100\rangle $ type dislocation, the green one for ${1 \mathord{\left/ {\vphantom {1 2}} \right. } 2}\langle 111\rangle $.
-
[1] Krasheninnikov S I 2022 Plasma Phys. Control. Fusion 64 124005
Google Scholar
[2] Janeschitz G 2001 J. Nucl. Mater. 290–293 1
Google Scholar
[3] Philipps V 2011 J. Nucl. Mater. 415 S2
Google Scholar
[4] El-Atwani O, El-Atwani E, Esquivel E, Efe M, Wang Y Q, Maloy S A 2018 Acta Mater. 147 277
Google Scholar
[5] 马玉田, 刘俊标, 韩立, 田利丰, 王雪聪, 孟祥敏, 肖善曲, 王波 2019 68 040702
Google Scholar
Ma Y T, Liu J B, Han L, Tian L F, Wang X C, Meng X M, Xiao S Q, Wang B 2019 Acta Phys. Sin. 68 040702
Google Scholar
[6] Cui M H, Gao N, Wang D, Gao X, Wang Z G 2019 Nucl. Instrum. Meth. B 460 60
Google Scholar
[7] Bernard E, Sakamoto R, Yoshida N, Yamada H 2015 J. Nucl. Mater. 463 316
Google Scholar
[8] Grigorev P, Bakaev A, Terentyev D, Van Oost G, Noterdaeme J M, Zhurkin E E 2017 Nucl. Instrum. Methods Phys. Res. Sect. B-Beam Interact. Mater. Atoms 393 164
Google Scholar
[9] Matsui T, Muto S, Tanabe T 2000 J. Nucl. Mater. 283 1139
Google Scholar
[10] Guo W G, Wang S W, Ge L, Fu E G, Yuan Y, Cheng L, Lu G H 2020 Nucl. Fusion 60 034002
Google Scholar
[11] El-Atwani O, Cunningham W S, Trelewicz J R, Li M, Wirth B D, Maloy S A 2020 J. Nucl. Mater. 538 152150
Google Scholar
[12] Harrison R W, Greaves G, Hinks J A, Donnelly S E 2017 J. Nucl. Mater. 495 492
Google Scholar
[13] Yi X O, Jenkins M L, Kirk M A, Zhou Z F, Roberts S G 2016 Acta Mater. 112 105
Google Scholar
[14] 贺冉 2020 硕士学位论文 (兰州: 兰州理工大学)
He R 2020 M. S. Thesis ( Lanzhou: Lanzhou University of Technology
[15] Yi X O, Jenkins M L, Hattar K, Edmondson P D, Roberts S G 2015 Acta Mater. 92 163
Google Scholar
[16] Groves P P, Bacon D J 1970 Philos. Mag. 22 83
Google Scholar
[17] Fikar J, Gröger R, Schäublin R 2017 Nucl. Instrum. Meth. B 393 186
Google Scholar
[18] Juslin N, Wirth B D 2013 J. Nucl. Mater. 432 61
Google Scholar
[19] Marinica M C, Ventelon L, Gilbert M R, Proville L, Dudarev S L, Marian J, Bencteux G, Willaime F 2013 J. Phys. Condens. 25 395502
Google Scholar
[20] Harder J M, Bacon D J 1988 Phil. Mag. A 58 165
Google Scholar
[21] Chen L, Liu Y L, Zhou H B, Jin S, Zhang Y, Lu G H 2012 Sci. China-Phys. Mech. Astron. 55 614
Google Scholar
[22] Gao N, Shen T L, Kurtz R, Wang Z G, Gao F 2017 Scr. Mater. 136 64
Google Scholar
[23] Sharafat S, Takahashi A, Hu Q, Ghoniem N M 2009 J. Nucl. Mater. 386–388 900
Google Scholar
[24] Zhang H Y, Sun J Z, Wang Y M, Stirner T, Hamid A Y, Sang C F 2020 Fusion Eng. Des. 161 112004
Google Scholar
-
[1] 徐驰, 万发荣. 聚变材料钨辐照后退火形成的位错环特性及inside-outside衬度分析. , 2023, 72(5): 056801. doi: 10.7498/aps.72.20222124 [2] 李然然, 张一帆, 殷玉鹏, 渡边英雄, 韩文妥, 易晓鸥, 刘平平, 张高伟, 詹倩, 万发荣. 注氢纯铝中间隙型位错环一维迁移现象的原位观察. , 2022, 71(1): 016102. doi: 10.7498/aps.71.20211229 [3] 张博佳, 安敏荣, 胡腾, 韩腊. 镁中位错和非晶作用机制的分子动力学模拟. , 2022, 71(14): 143101. doi: 10.7498/aps.71.20212318 [4] 王瑾, 贺新福, 曹晗, 贾丽霞, 豆艳坤, 杨文. 不同温度下bcc-Fe中螺位错滑移及其与½[ ${{11}}\bar {{1}}$ ]位错环相互作用行为. , 2021, 70(6): 068701. doi: 10.7498/aps.70.20201659[5] 李然然, 张一帆, 殷玉鹏, 渡边英雄, 韩文妥, 易晓鸥, 刘平平, 张高伟, 詹倩, 万发荣. 注氢纯铝中间隙型位错环一维迁移现象的原位观察. , 2021, (): . doi: 10.7498/aps.70.20211229 [6] 梁晋洁, 高宁, 李玉红. 表面效应对铁 ${\left\langle 100 \right\rangle} $ 间隙型位错环的影响. , 2020, 69(3): 036101. doi: 10.7498/aps.69.20191379[7] 梁晋洁, 高宁, 李玉红. 体心立方Fe中 ${ \langle 100 \rangle}$ 位错环对微裂纹扩展影响的分子动力学研究. , 2020, 69(11): 116102. doi: 10.7498/aps.69.20200317[8] 崔丽娟, 高进, 杜玉峰, 张高伟, 张磊, 龙毅, 杨善武, 詹倩, 万发荣. 氢离子辐照纯钒中形成的位错环. , 2016, 65(6): 066102. doi: 10.7498/aps.65.066102 [9] 张其黎, 张弓木, 赵艳红, 刘海风. 氘、氦及其混合物状态方程第一原理研究. , 2015, 64(9): 094702. doi: 10.7498/aps.64.094702 [10] 张宝玲, 宋小勇, 侯氢, 汪俊. 高密度氦相变的分子动力学研究. , 2015, 64(1): 016202. doi: 10.7498/aps.64.016202 [11] 王欣欣, 张颖, 周洪波, 王金龙. 铌对钨中氦行为影响的第一性原理研究. , 2014, 63(4): 046103. doi: 10.7498/aps.63.046103 [12] 姜少宁, 万发荣, 龙毅, 刘传歆, 詹倩, 大貫惣明. 氦、氘对纯铁辐照缺陷的影响. , 2013, 62(16): 166801. doi: 10.7498/aps.62.166801 [13] 谢红献, 于涛, 刘波. 温度对镍基单晶高温合金γ/γ'相界面上错配位错运动影响的分子动力学研究. , 2011, 60(4): 046104. doi: 10.7498/aps.60.046104 [14] 陈敏. 分子动力学方法研究金属Ti中He小团簇的迁移. , 2011, 60(12): 126602. doi: 10.7498/aps.60.126602 [15] 汪俊, 张宝玲, 周宇璐, 侯氢. 金属钨中氦行为的分子动力学模拟. , 2011, 60(10): 106601. doi: 10.7498/aps.60.106601 [16] 卢果, 方步青, 张广财, 许爱国. 有限温度下位错环的脱体现象. , 2009, 58(11): 7934-7946. doi: 10.7498/aps.58.7934 [17] 汪俊, 侯氢. 金属钛中氦团簇生长行为的分子动力学研究. , 2009, 58(9): 6408-6412. doi: 10.7498/aps.58.6408 [18] 谢 朝, 侯 氢, 汪 俊, 孙铁英, 龙兴贵, 罗顺忠. 金属钛中氦团簇融合的分子动力学模拟. , 2008, 57(8): 5159-5164. doi: 10.7498/aps.57.5159 [19] 张 颖, 陈其峰, 顾云军, 蔡灵仓, 卢铁城. 部分电离稠密氦等离子体物态方程的自洽变分计算. , 2007, 56(3): 1318-1324. doi: 10.7498/aps.56.1318 [20] 郑思孝, 罗顺忠, 刘仲阳, 龙兴贵, 王培禄, 彭述明, 廖小东, 刘 宁. 纳米晶钛膜中氦注入的保持剂量. , 2004, 53(2): 555-560. doi: 10.7498/aps.53.555
下载:
计量
- 文章访问数: 1967
- PDF下载量: 71
- 被引次数: 0
