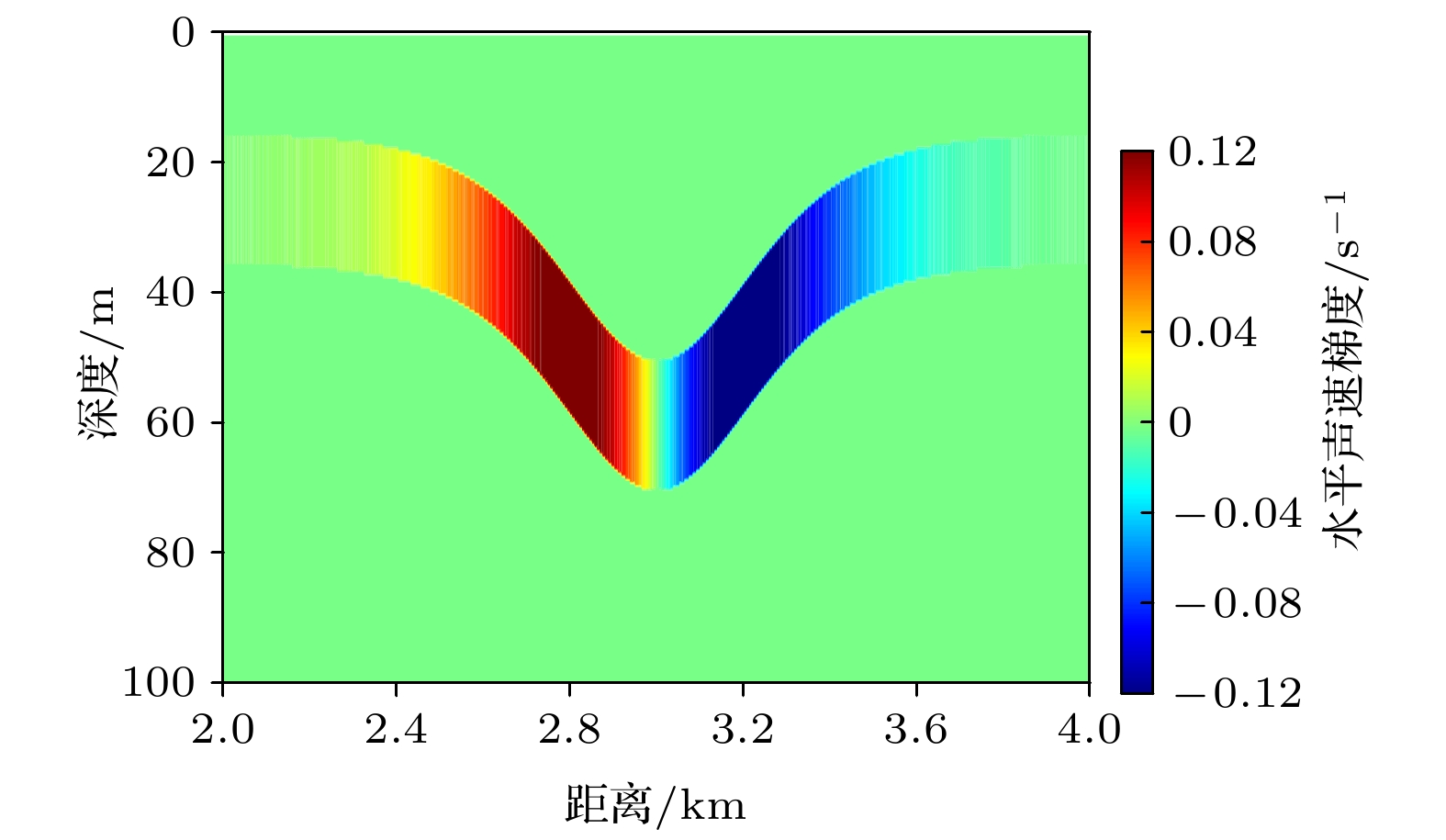
-
大陆坡海域内波普遍存在, 其陆坡地形和内波过程都会引起显著的声场起伏. 已有研究工作主要关注内波或大陆坡单扰动因子对模态耦合和强度起伏的影响, 少见将内波和海底地形起伏同时作为影响因子进行研究. 文章考虑孤立子内波和海底地形对声传播的双重影响, 首先构建海洋波导模型, 然后基于简正波理论数值对比分析各波导模型条件下模态的耦合规律, 进而研究声场强度起伏特性及其物理机理. 研究结果表明, 当声波朝向或远离内波中心传播时, 模态耦合在内波与大陆坡的共同作用下出现耦合增强或衰减, 高号模态耦合系数振荡; 内波扰动的作用使得能量由低号模态耦合至高号模态, 提高了声场强度衰减; 斜坡的作用使得声波下坡传播时, 波导模态数增加、模态强度衰减降低; 大陆坡内波环境中的模态强度总和大于内波环境、小于大陆坡环境, 且模态组间的能量转移比只有内波或者大陆坡时更强, 高号模态从耦合中获得更多能量, 使得跃层以上水层能量增强.The topographic variation underwater of the continental slope is one of the main causes for triggering off the formation of internal waves, and the continental slope internal waves are ubiquitous in the ocean. The horizontal variation of waveguide environment, caused by the internal wave and the continental slope, can lead to acoustic normal mode coupling, and then generate sound field fluctuation. Most of the existing research work focused on studying the effect of single perturbation factor of either the internal waves or the continental slope on acoustic mode coupling and intensity fluctuation, while it is hard to find some research work that takes into account both the internal waves and the topographic variations as influencing factors. In this work, numerical simulations for the sound waves to propagate through the internal waves in the downhill direction are performed by using the acoustic coupled normal-mode model in four waveguide environments: thermocline, internal wave, continental slope and continental slope internal wave. And the mode coupling and intensity fluctuation characteristics and their physical mechanisms are studied by comparing and analyzing the simulation results of the four different waveguide environment constructed. Some conclusions are obtained as follows. The intra-mode conduction coefficients are symmetric with respect to the center of the internal wave, while the inter-mode coupling coefficients are antisymmetric around it. As the sound waves propagate toward or away from the center of the internal wave, the acoustic mode coupling becomes enhanced or weakened, and the coupling coefficients curves for large mode oscillate. The influence of internal wave perturbation makes the energy transfer from the smaller modes to the larger modes, which increases the attenuation of sound field intensity. The number of the waveguide modes increases and the mode intensity attenuation decreases, when the sound waves propagate downhill. The total intensity of all modes for the continental slope internal wave environment is greater than for the internal wave environment and less than for the continental environment, and the energy transfer between mode groups is stronger than for individual effect of internal wave or continental slope, which leads more energy to transfer from the smaller to larger mode groups and the energy of the sound field above the thermocline to increase.
-
Keywords:
- continental slope /
- internal solitary waves /
- sound field fluctuation /
- mode coupling
[1] Whalen C B, Lavergne C D, Garabato N A C, Klymak J M, Mackinnon J A, Sheen 2020 Nature 1 606
[2] Alford M H, Mackinnon J A, Simmons H L Nash J D 2016 Annu. Rev. Mar. Sci. 8 95
Google Scholar
[3] Zhao Z, Alford M H, Girton J B, Rainville L, Simmons H L 2016 J. Phys. Oceanogr. 46 1657
Google Scholar
[4] Grisouard N, Staquet C 2010 Nonlinear Processes Geophys. 17 575
Google Scholar
[5] 张泽众, 骆文于, 庞哲, 周益清 2019 68 204302
Google Scholar
Zhang Z Z, Luo W Y, Pan Z, Zhou Y Q 2019 Acta Phys. Sin. 68 204302
Google Scholar
[6] Preisig J C, Duda T F 1997 IEEE J. Oceanic Eng. 22 256
Google Scholar
[7] Zhou J X, Zhang X Z, Rogers P H 1991 J. Acoust. Soc. Am. 90 2042
Google Scholar
[8] Rouseff D, Turgut A, Wolf S N, Finette S, Orr M H, Pasewark B H, Apel J R, Badiey M, Chiu C S, Headrick R H, Lynch J F, Kemp J N, Newhall A E, von der Heydt K, Tielbuerger D 2002 J. Acoust. Soc. Am. 111 1655
Google Scholar
[9] Katsnelson B G, Pereselkov S A 2000 Acoust. Phys. 46 684
Google Scholar
[10] Lin Y T, Duda T F, Lynch J F 2009 J. Acoust. Soc. Am. 126 1752
Google Scholar
[11] Milone M A, DeCourcy B J, Lin Y T, Siegmann 2019 J. Acoust. Soc. Am. 146 1934
Google Scholar
[12] 秦继兴, Katsnelson Boris, 彭朝晖, 李整林, 张仁和, 骆文于 2016 65 034301
Google Scholar
Qin J X, Katsnelson B G, Peng Z H, Li Z L, Zhang R H, Luo W Y 2016 Acta Phys. Sin. 65 034301
Google Scholar
[13] Chiu C S, Ramp S R, Miller C W, Lynch J F, Duda T F, Tang T Y 2004 IEEE J. Oceanic Eng. 29 1249
Google Scholar
[14] 秦继兴, Katsnelson Boris, 李整林, 张仁和, 骆文于 2016 声学学报 41 145
Qin J X, Katsnelson B G, Li Z L, Zhang R H, Luo W Y 2016 Acta Acustia 41 145
[15] Badiey M, Katsnelson B G, Lynch J F, Pereselkov S, Siegmann W L 2005 J. Acoust. Soc. Am. 117 613
Google Scholar
[16] 李沁然, 孙超, 谢磊 2022 71 024302
Google Scholar
Li Q R, Sun C, Xie L 2022 Acta Phys. Sin. 71 024302
Google Scholar
[17] Chiu L Y S, Chang A Y Y, Reeder D B 2015 J. Acoust. Soc. Am. 138 515
Google Scholar
[18] 刘代, 李整林, 刘若芸 2021 70 034302
Google Scholar
Liu D, Li Z L, Liu R Y 2021 Acta Phys. Sin. 70 034302
Google Scholar
[19] 莫亚枭, 朴胜春, 张海刚, 李丽 2014 63 214302
Google Scholar
Mo Y X, Piao S C, Zhang H G Li L 2014 Acta Phys. Sin. 63 214302
Google Scholar
[20] Sagers J D, Ballard M S, Knobles D P 2014 J. Acoust. Soc. Am. 136 2453
Google Scholar
[21] Chiu L Y S, Reeder D B, Chang Y Y, Chen C F, Chiu C S, Lynch J F 2013 J. Acoust. Soc. Am. 133 1306
Google Scholar
[22] Porter M B 1991 The KRAKEN Normal Mode Program (La Spezia: SACLANT Undersea Research Centre) Technical Report SM-2
[23] Jensen F B, Kuperman W A, Porter M B, Schmidt H 2011 Computational Ocean Acoustics (New York: Springer) pp403–408
[24] Apel J R, Ostrovsky L A, Stepanyants Y A, Lynch J F 2007 J. Acoust. Soc. Am. 121 695
Google Scholar
[25] Yang T C 2014 J. Acoust. Soc. Am. 135 610
Google Scholar
[26] Dozier L B, Tappert F D 1978 J. Acoust. Soc. Am. 63 353
Google Scholar
[27] Yang T C 2017 IEEE J. Oceanic Eng. 42 663
Google Scholar
-
图 1 大陆坡内波波导环境参数示意图
Fig. 1. Diagram of parameters for continental slope internal wave waveguide environment.
图 2 仿真用四种典型海洋环境 (a) 环境1(跃层环境); (b) 环境2(内波环境); (c)环境3(大陆坡环境); (d) 环境4(大陆坡内波环境)
Fig. 2. Four typical environments for simulation: (a) Environment 1 (thermocline); (b) environment 2 (internal wave); (c) environment 3 (continental slope); (d) environment 4 (continental slope internal wave).
图 3 内波环境水平声速梯度分布
Fig. 3. Distributions of horizontal sound speed gradient in internal wave environment.
图 4 第1—4号简正波在不同波导环境中水平距离2—4 km处的模内传导系数
$ C_{m, m}^{j + 1} $ (a) 1号模态; (b) 2号模态; (c) 3号模态; (d) 4号模态Fig. 4. The intra-mode conduction coefficients
$ C_{m, m}^{j + 1} $ of mode 1, 2, 3 and 4 at range 2–4 km in different waveguide environments: (a) Mode 1; (b) mode 2; (c) mode 3; (d) mode 4.
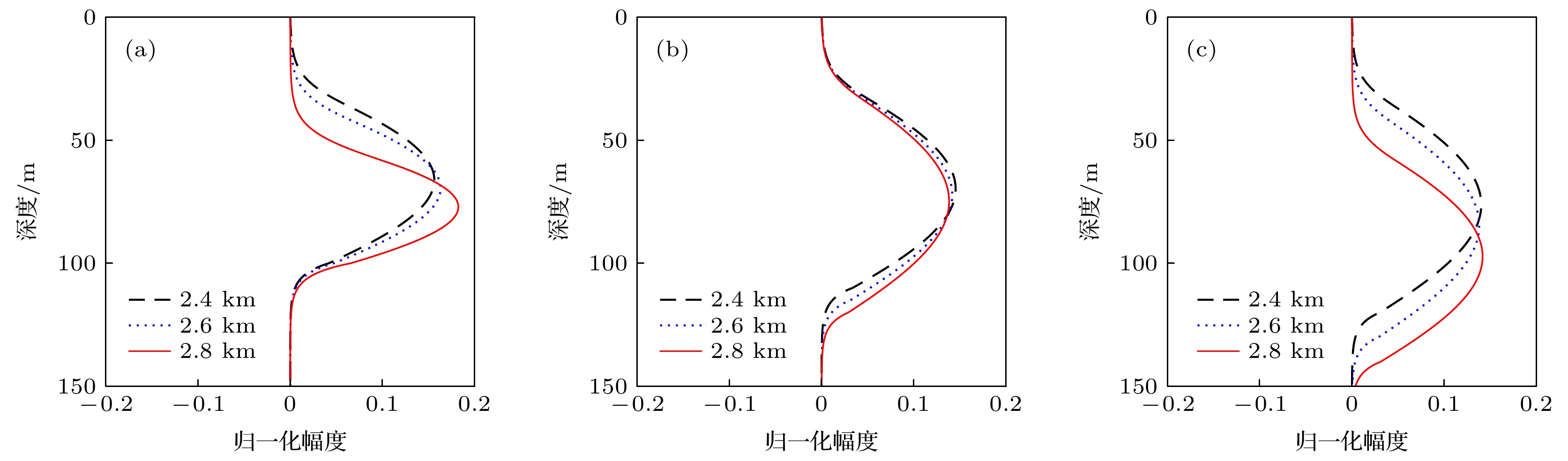
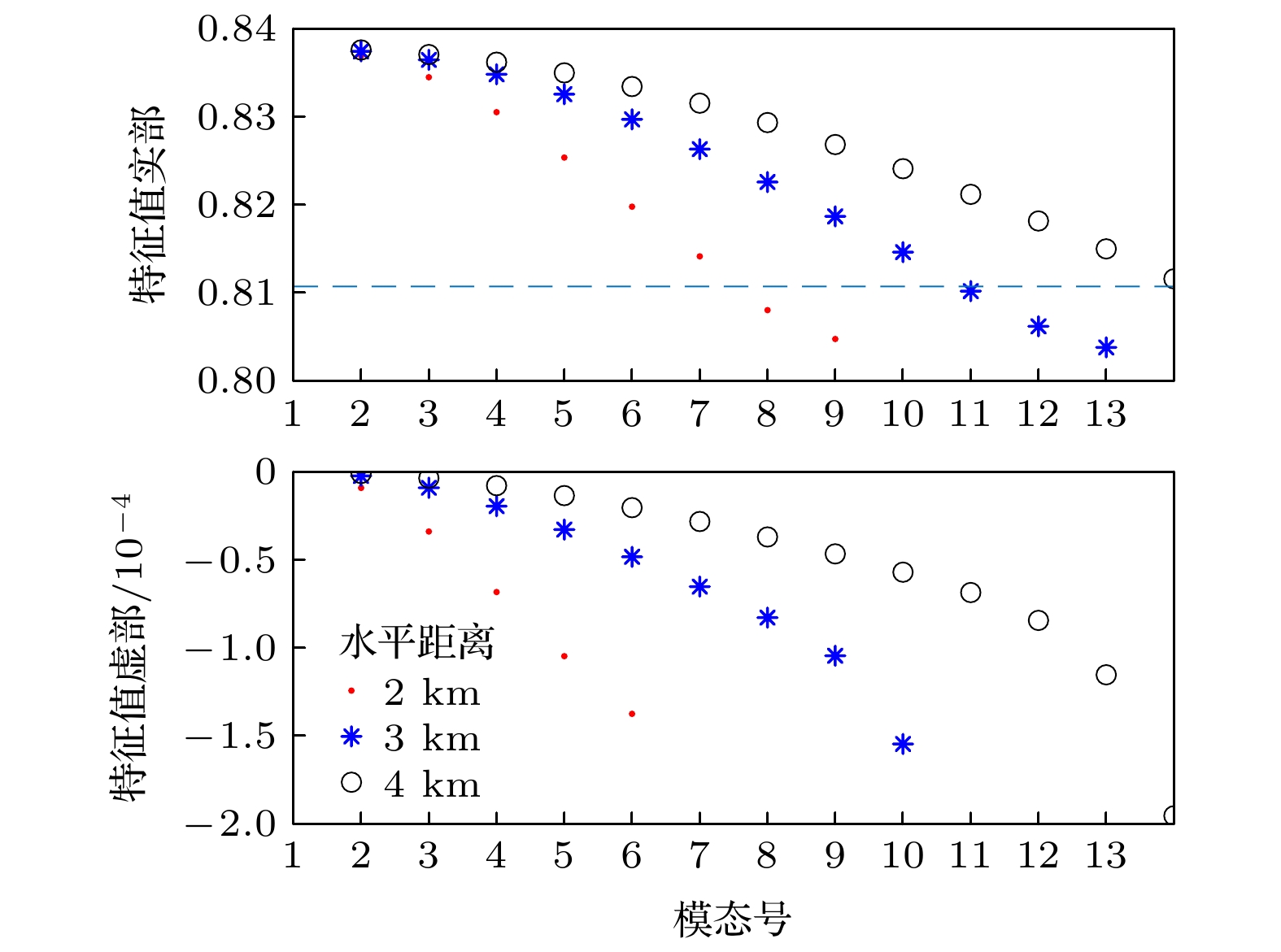
图 5 不同波导环境中水平距离2—4 km处的模间耦合系数
$ C_{1, n}^{j{\text{ + }}1} $ (a) 1号与2号模态; (b) 1号与3号模态; (c) 1号与5号模态; (d) 1号与13号模态Fig. 5. The inter-mode coupling coefficients
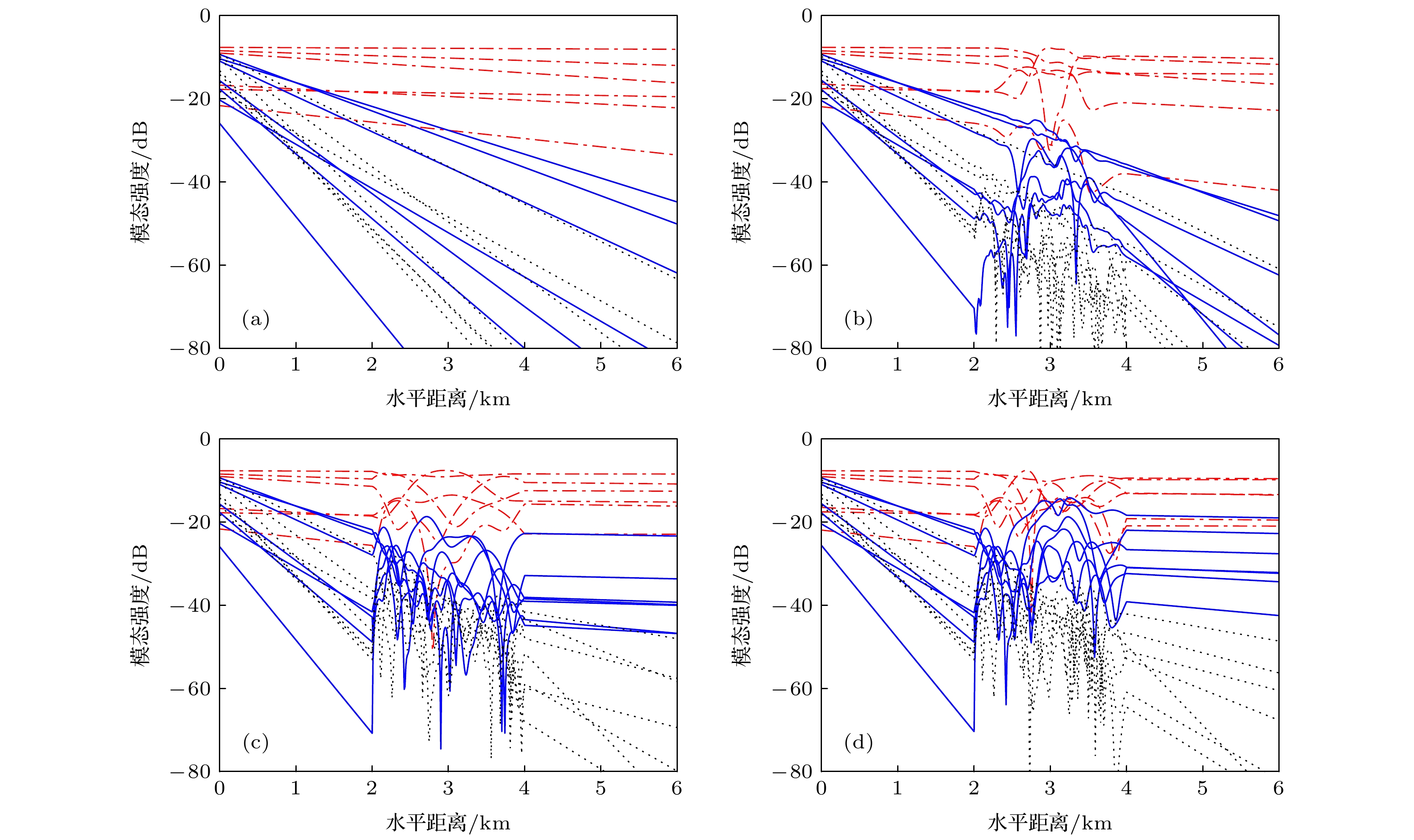
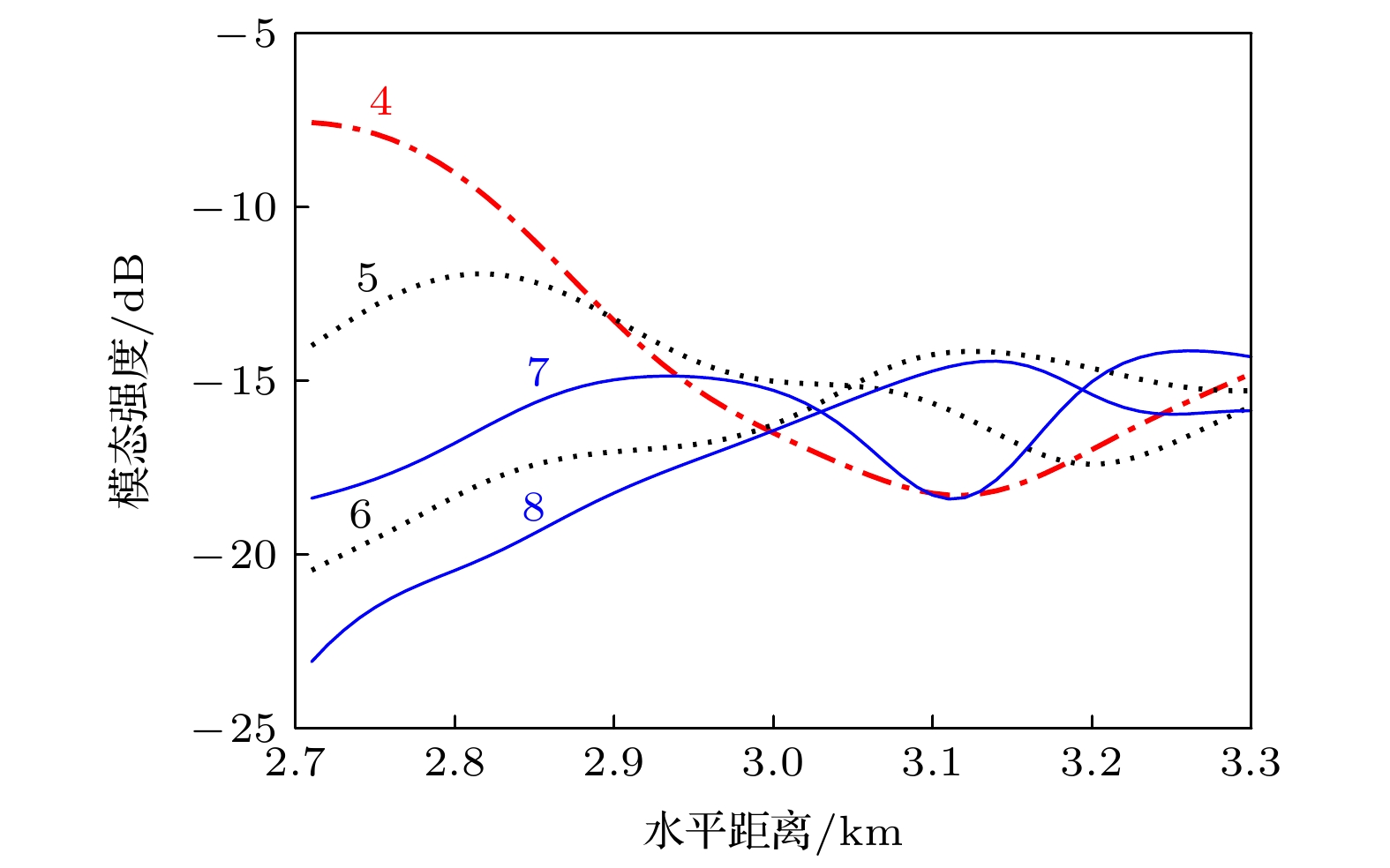
$ C_{1, n}^{j{\text{ + }}1} $ at range of 2–4 km in different waveguide environments: (a) Mode 1 with 2; (b) mode 1 with 3; (c) mode 1 with 5; (d) mode 1 with 13.
图 6 不同波导环境中1号局地模态函数 (a) 内波环境; (b) 大陆坡环境; (c) 大陆坡内波环境
Fig. 6. The local function of mode 1 in different waveguide environments: (a) Internal wave environment; (b) continental slope environment; (c) continental slope internal wave environment.
图 7 不同波导环境中1—20号简正波模态强度随距离变化 (a) 跃层环境; (b) 内波环境; (c) 大陆坡环境; (d) 大陆坡内波环境, 红色点划线为1—6号模态, 蓝色实线为7—13号模态, 黑色点线为14—20号模态
Fig. 7. Modes 1–20 intensity variation with range in different waveguide environments: (a) Thermocline environment; (b) internal wave environment; (c) continental slope environment; (d) continental slope internal wave environment, the red dotted lines, blue solid lines and black dotted lines represent mode groups of 1–6, 7–13 and 14–20, respectively.
图 8 内波波导环境中8号(a), 11号(b)简正波模态声场强度分布
Fig. 8. The mode 8 (a) and mode 11 (b) intensity versus range and depth in the internal wave environment.
图 9 大陆坡波导环境中不同水平距离前13号简正波模态特征值分布, 特征值实部大于横虚线为波导模态
Fig. 9. Eigenvalues of the first 13 modes of different ranges in the continental slope environment.
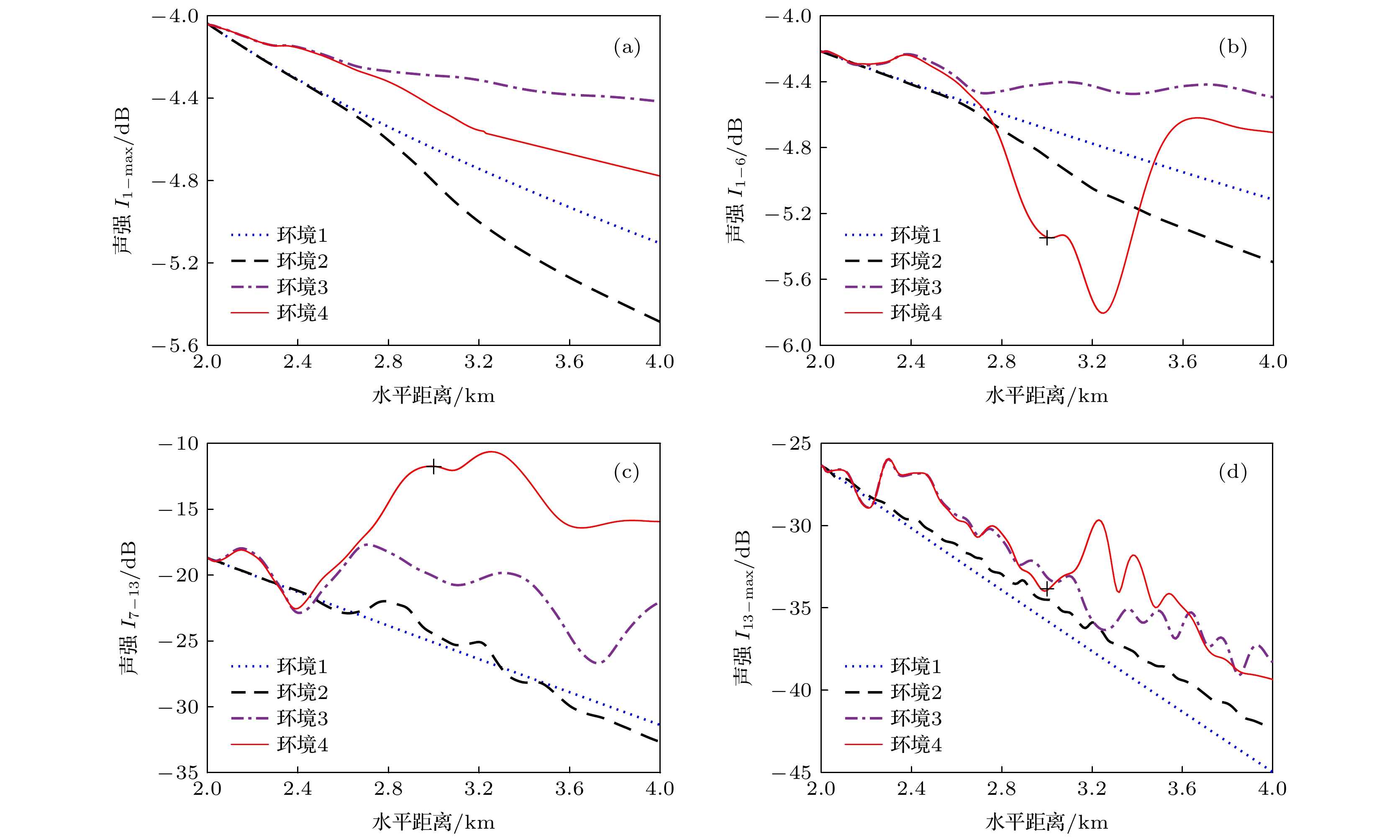
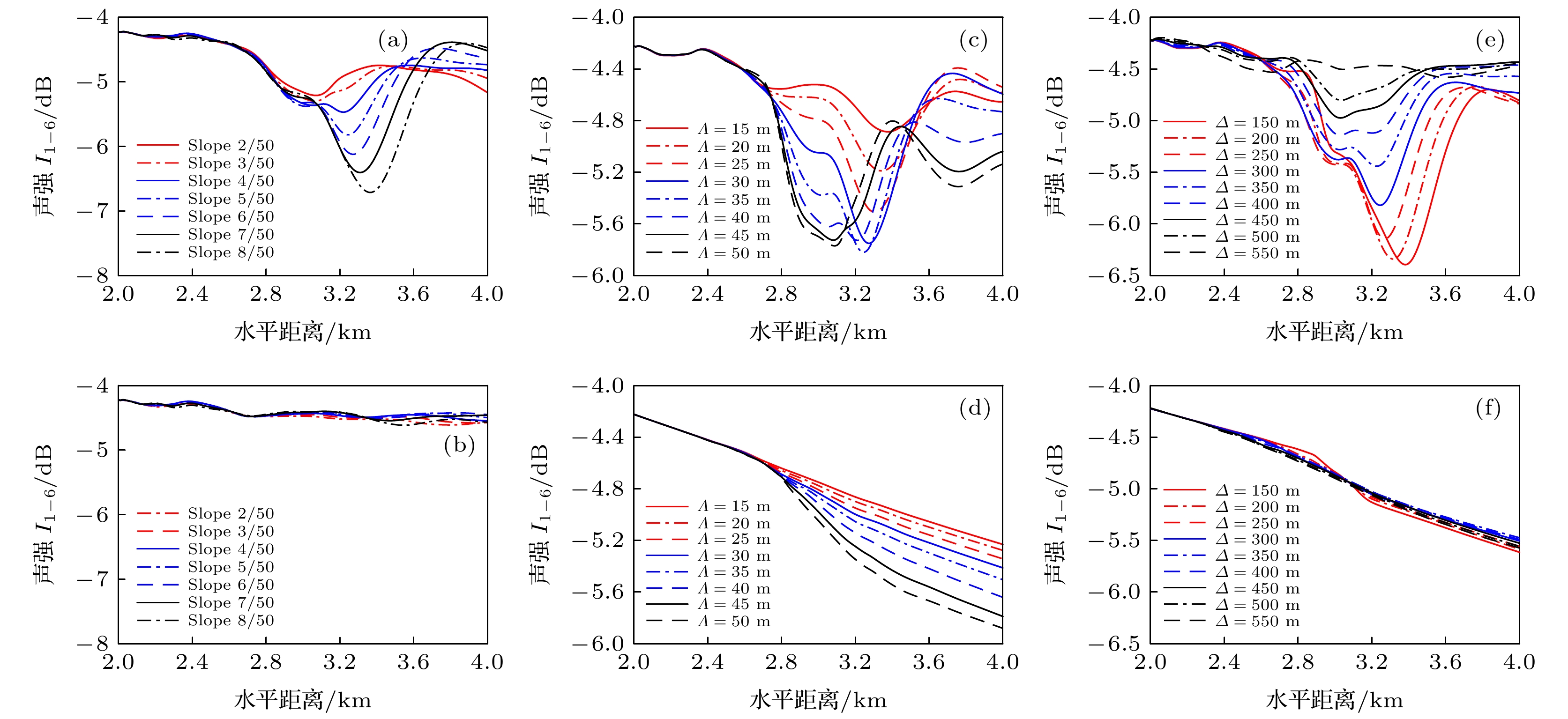
图 10 不同波导环境中各组模态强度之和随距离变化 (a) 所有模态
$ {I_{{\text{1}}—{\max}}} $ ; (b) 1—6号模态$ {I_{{\text{1}}—{\text{6}}}} $ ; (c) 7—13号模态$ {I_{{\text{7}}—{\text{13}}}} $ ; (d) 14号以上模态$ {I_{{\text{14}}—{\max}}} $ Fig. 10. The sum of intensity of each mode groups versus range in different environments: (a)
$ {I_{{\text{1}}—{\max}}} $ ; (b)$ {I_{{\text{1}}—{\text{6}}}} $ ; (c)${I_{{\text{7}}—{\text{13}}}}$ ; (d)$ {I_{{\text{14}}—{\max}}} $ .
图 11 大陆坡内波环境中2.7—3.3 km范围内第4—8号简正波模态强度
Fig. 11. Intensity of modes 4–8 for at range 2.7–3.3 km in the continental slope internal wave environment.
图 12 不同环境中参数变数时的模态强度之和
$ {I_{{\text{1}}—{\text{6}}}} $ 随距离的变化 (a), (c), (e) 大陆坡内波环境; (b) 大陆坡环境; (d), (f) 内波环境Fig. 12. The sum of intensity
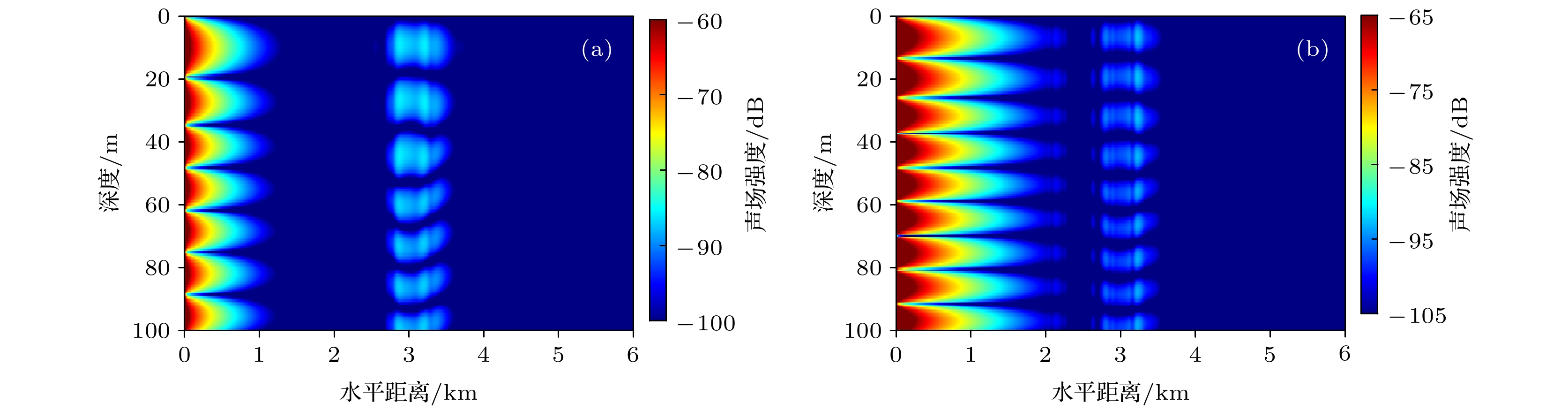
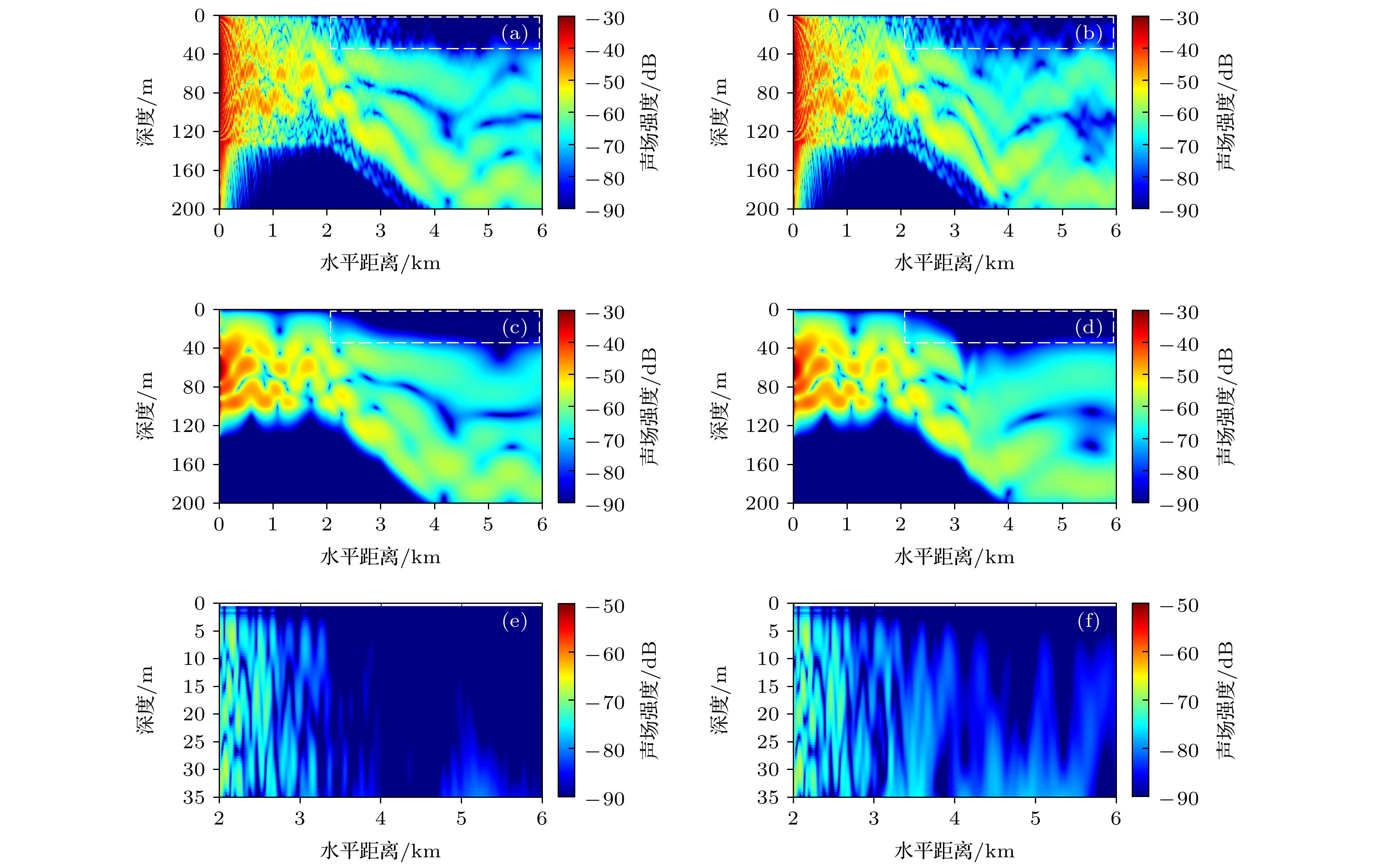
$ {I_{{\text{1}}—{\text{6}}}} $ versus range in different environments of various parameters: (a), (c), (e) Continental slope internal wave environment; (b) continental slope environment; (d), (f) internal wave environment.
图 13 不同环境中的声场分布 (a), (c), (e) 大陆坡环境3中的所有模态、1—6号模态、7—max号模态声场; (b), (d), (f) 大陆坡内波环境4中的所有模态、1—6号模态、7—max号模态声场. 其中, 图中虚线方框标记了0—35 m深度、2—6 km水平距离的区域
Fig. 13. The sound field in different environments: (a), (c), (e) The sound fields of the whole modes, models 1–6 and modes 7–max in continental slope environment, respectively; (b), (d), (f) the sound fields of the whole modes, models 1–6 and modes 7–max in continental slope internal wave environment, respectively. The white dashed boxes mark the area of 0–35 m and 2–6 km horizontal distance.
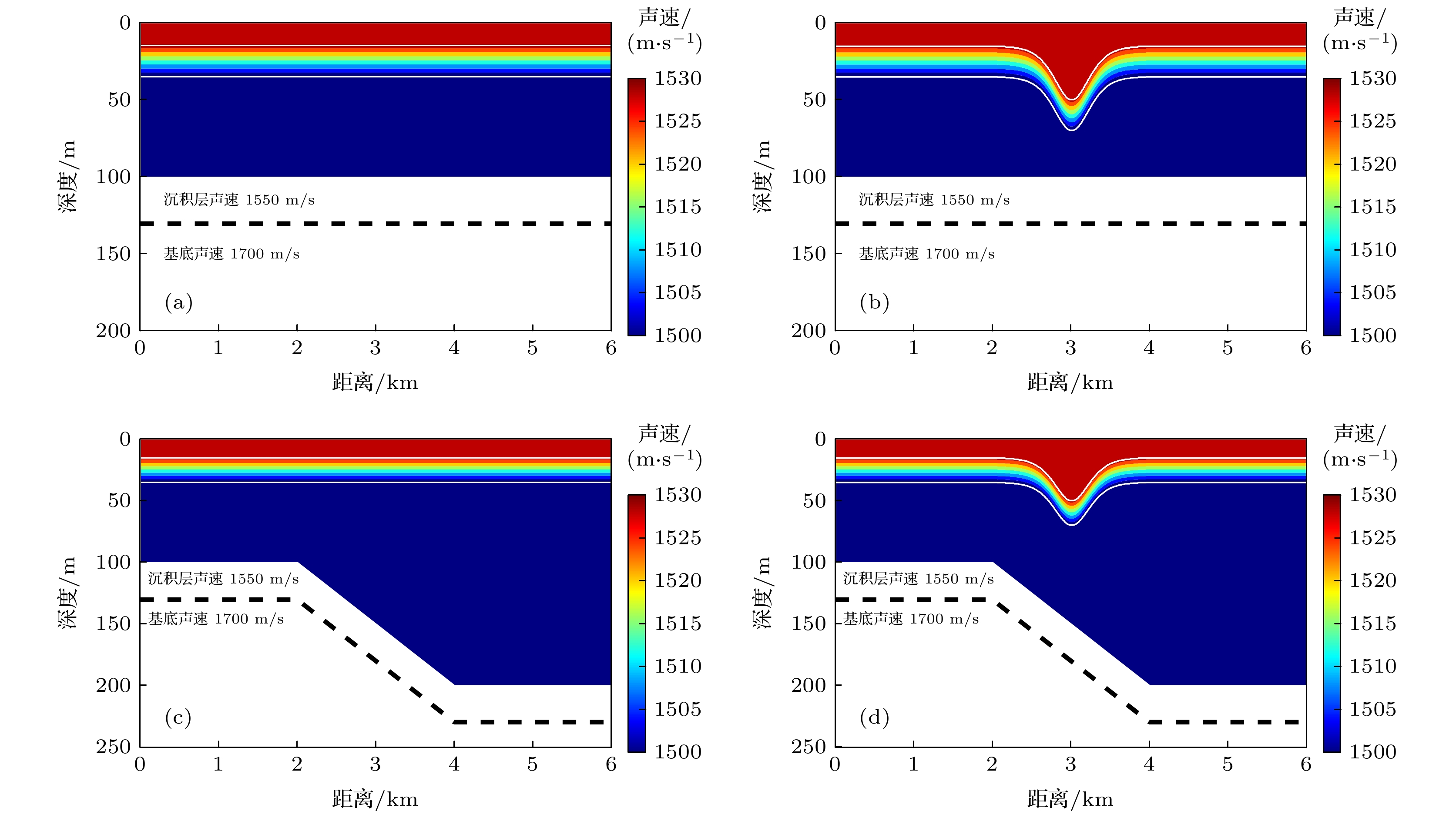
表 1 仿真环境参数配置
Table 1. Configuration of environment parameters for simulations.
参数类型 参数值 跃层上边界深度$ {z_{\text{u}}}/{\text{m}} $ 15 跃层下边界深度$ {z_{\text{l}}} $/m 35 水体声速$ {c_{\text{u}}} $, $ {c_{\text{l}}} $/(m·s–1) 1530, 1500 内波的幅度$\varLambda$/m 35 内波的中心距离$ {r_0} $/km 3 内波的波宽$\varDelta$/m 300 大陆坡起点距离$ {r_{\text{s}}}/{\text{km}} $、水深$ {H_{\text{s}}}/{\text{m}} $ 2, 100 大陆坡终点距离$ {r_{\text{e}}}/{\text{km}} $、水深$ {H_{\text{e}}}/{\text{m}} $ 4, 200 大陆坡坡度/(°) 2.86 (1/10) 
下载: 导出CSV
-
[1] Whalen C B, Lavergne C D, Garabato N A C, Klymak J M, Mackinnon J A, Sheen 2020 Nature 1 606
[2] Alford M H, Mackinnon J A, Simmons H L Nash J D 2016 Annu. Rev. Mar. Sci. 8 95
Google Scholar
[3] Zhao Z, Alford M H, Girton J B, Rainville L, Simmons H L 2016 J. Phys. Oceanogr. 46 1657
Google Scholar
[4] Grisouard N, Staquet C 2010 Nonlinear Processes Geophys. 17 575
Google Scholar
[5] 张泽众, 骆文于, 庞哲, 周益清 2019 68 204302
Google Scholar
Zhang Z Z, Luo W Y, Pan Z, Zhou Y Q 2019 Acta Phys. Sin. 68 204302
Google Scholar
[6] Preisig J C, Duda T F 1997 IEEE J. Oceanic Eng. 22 256
Google Scholar
[7] Zhou J X, Zhang X Z, Rogers P H 1991 J. Acoust. Soc. Am. 90 2042
Google Scholar
[8] Rouseff D, Turgut A, Wolf S N, Finette S, Orr M H, Pasewark B H, Apel J R, Badiey M, Chiu C S, Headrick R H, Lynch J F, Kemp J N, Newhall A E, von der Heydt K, Tielbuerger D 2002 J. Acoust. Soc. Am. 111 1655
Google Scholar
[9] Katsnelson B G, Pereselkov S A 2000 Acoust. Phys. 46 684
Google Scholar
[10] Lin Y T, Duda T F, Lynch J F 2009 J. Acoust. Soc. Am. 126 1752
Google Scholar
[11] Milone M A, DeCourcy B J, Lin Y T, Siegmann 2019 J. Acoust. Soc. Am. 146 1934
Google Scholar
[12] 秦继兴, Katsnelson Boris, 彭朝晖, 李整林, 张仁和, 骆文于 2016 65 034301
Google Scholar
Qin J X, Katsnelson B G, Peng Z H, Li Z L, Zhang R H, Luo W Y 2016 Acta Phys. Sin. 65 034301
Google Scholar
[13] Chiu C S, Ramp S R, Miller C W, Lynch J F, Duda T F, Tang T Y 2004 IEEE J. Oceanic Eng. 29 1249
Google Scholar
[14] 秦继兴, Katsnelson Boris, 李整林, 张仁和, 骆文于 2016 声学学报 41 145
Qin J X, Katsnelson B G, Li Z L, Zhang R H, Luo W Y 2016 Acta Acustia 41 145
[15] Badiey M, Katsnelson B G, Lynch J F, Pereselkov S, Siegmann W L 2005 J. Acoust. Soc. Am. 117 613
Google Scholar
[16] 李沁然, 孙超, 谢磊 2022 71 024302
Google Scholar
Li Q R, Sun C, Xie L 2022 Acta Phys. Sin. 71 024302
Google Scholar
[17] Chiu L Y S, Chang A Y Y, Reeder D B 2015 J. Acoust. Soc. Am. 138 515
Google Scholar
[18] 刘代, 李整林, 刘若芸 2021 70 034302
Google Scholar
Liu D, Li Z L, Liu R Y 2021 Acta Phys. Sin. 70 034302
Google Scholar
[19] 莫亚枭, 朴胜春, 张海刚, 李丽 2014 63 214302
Google Scholar
Mo Y X, Piao S C, Zhang H G Li L 2014 Acta Phys. Sin. 63 214302
Google Scholar
[20] Sagers J D, Ballard M S, Knobles D P 2014 J. Acoust. Soc. Am. 136 2453
Google Scholar
[21] Chiu L Y S, Reeder D B, Chang Y Y, Chen C F, Chiu C S, Lynch J F 2013 J. Acoust. Soc. Am. 133 1306
Google Scholar
[22] Porter M B 1991 The KRAKEN Normal Mode Program (La Spezia: SACLANT Undersea Research Centre) Technical Report SM-2
[23] Jensen F B, Kuperman W A, Porter M B, Schmidt H 2011 Computational Ocean Acoustics (New York: Springer) pp403–408
[24] Apel J R, Ostrovsky L A, Stepanyants Y A, Lynch J F 2007 J. Acoust. Soc. Am. 121 695
Google Scholar
[25] Yang T C 2014 J. Acoust. Soc. Am. 135 610
Google Scholar
[26] Dozier L B, Tappert F D 1978 J. Acoust. Soc. Am. 63 353
Google Scholar
[27] Yang T C 2017 IEEE J. Oceanic Eng. 42 663
Google Scholar
下载:
计量
- 文章访问数: 3800
- PDF下载量: 89
- 被引次数: 0
