基于压电声子晶体板波声场的微粒操控

王俊; 蔡飞燕; 张汝钧; 李永川; 周伟; 李飞; 邓科; 郑海荣

doi:10.7498/aps.73.20231886

摘要

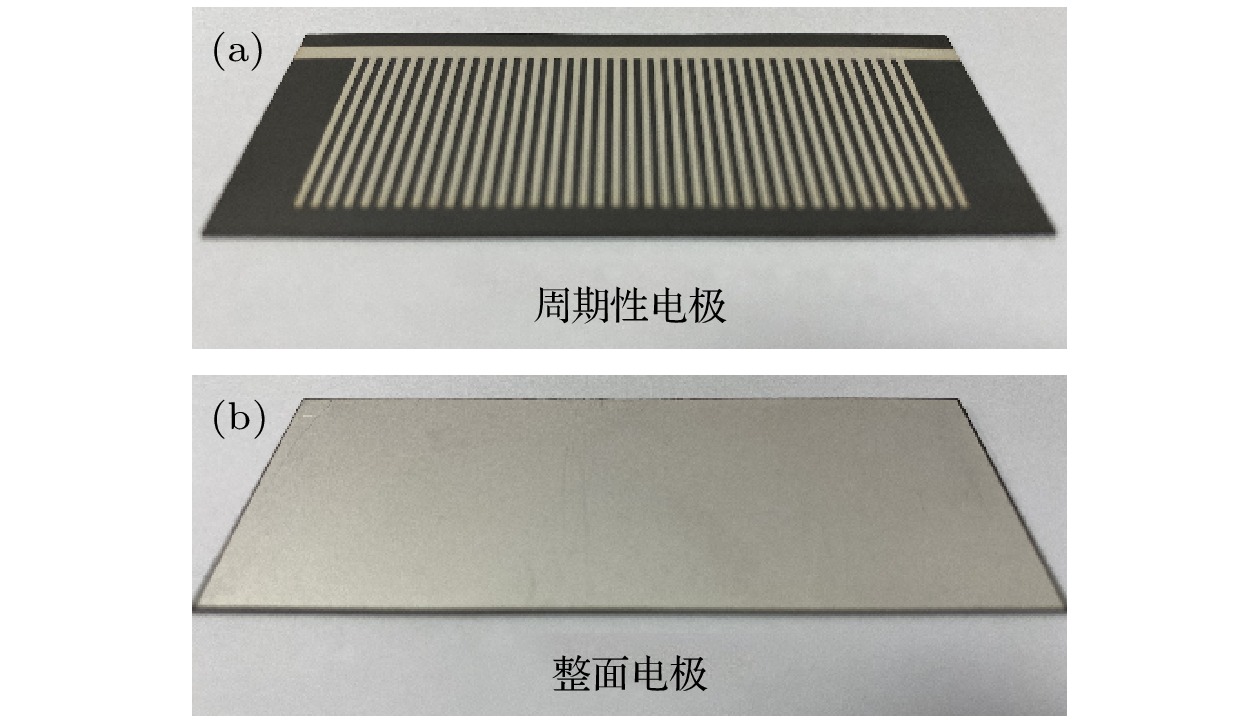
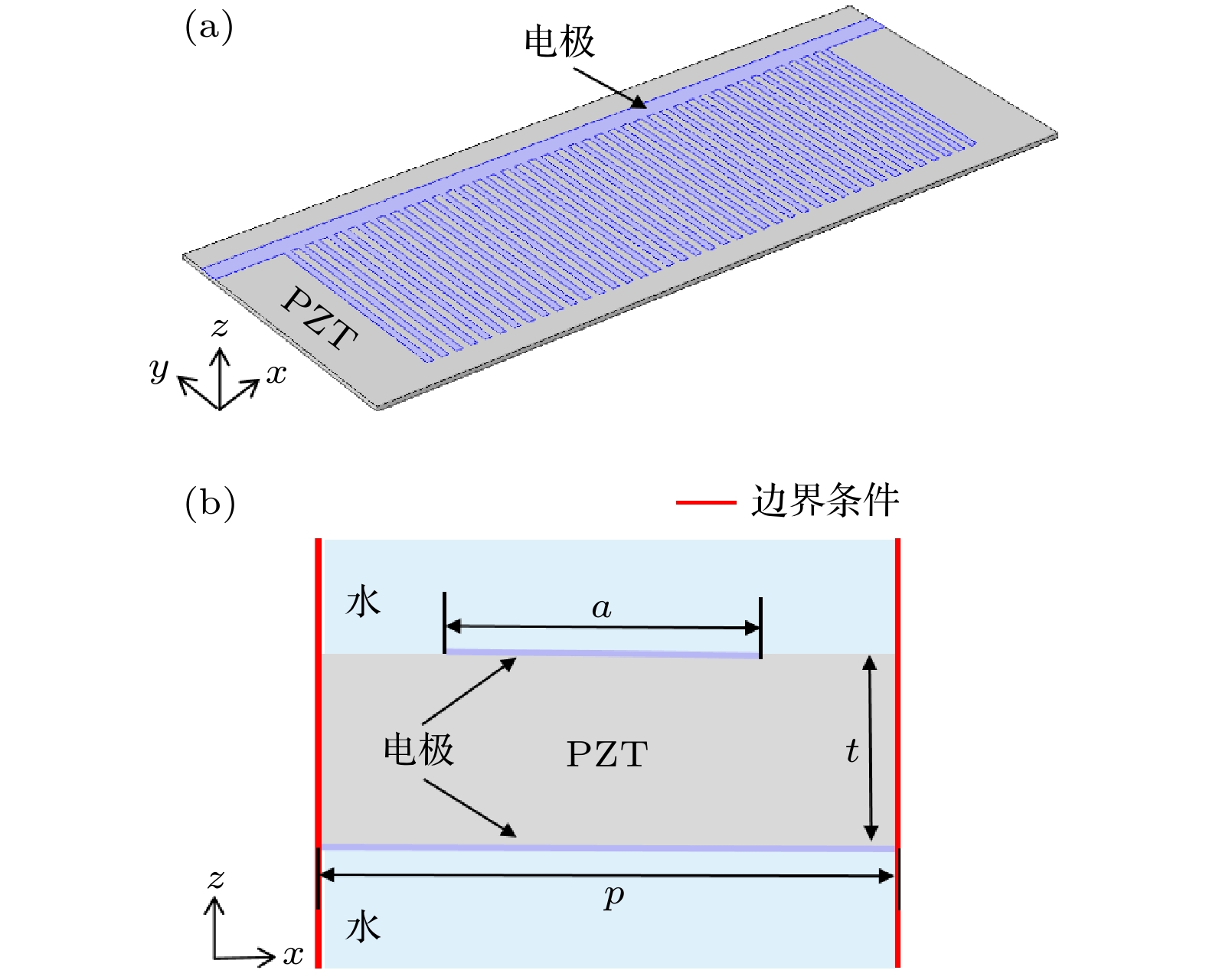
声镊可以非接触、无损伤地操控微粒, 在细胞分离、组织工程、材料组装等领域具有广阔的应用前景. 近期有研究利用声人工结构调控声场提升声镊性能, 然而, 与换能器分离的人工结构导致声镊装置复杂且操控现象不太稳定. 本文基于压电声子晶体板调制声场实现对微粒的灵活操控, 其主要机制是由压电陶瓷片构成的压电声子晶体板可激发A₀模式Lamb波模式和共振周期声场模式, 板上微粒在这两个模式中分别受到平行于板面的声停驻力和垂直于板面的声捕获力或声悬浮力, 从而实现排列、捕获、悬浮等多种模式的灵活操控. 由于压电声子晶体板整合了换能器与声人工结构, 该器件为研究高精度、低能耗、紧凑型声镊技术提供了物理基础和实验验证.

关键词:

Abstract

Acoustic tweezer is a promising device for manipulating particles, which does not need contact does not cause damage, or requires transparent materials. They have diverse applications in cell separation, tissue engineering, and material assembly. To control particle movement, this technology relies on the exchange of momentum between the particle and the acoustic field, generating an acoustic radiation force. Achieving high-performance acoustic tweezers necessitates the precise shaping of the acoustic fields. Traditionally, there are mainly two types of acoustic tweezers: bulk acoustic wave (BAW) and surface acoustic wave (SAW). The SAW-based acoustic tweezer operates at high frequencies, realizing precise manipulation. The BAW-based acoustic tweezer operates at lower frequencies and requires artificial structure on the transducer surface to shape the field. However, the separation of the artificial structure from the transducer brings complexity and instability into the manipulation process. In this study, we propose a novel approach to overcoming these challenges, that is, using piezoelectric phononic crystal plates to integrate the transducer and acoustic artificial structure. By designing the thickness, periodicity, and electrode width of the piezoelectric phononic crystal plate, we can excite the A₀ Lamb wave mode and the periodic resonant mode, resulting in a periodic gradient field and a periodic weak gradient field, respectively. These fields enable particle to be trapped or levitated on the surface. To validate this approach, an experimental device is constructed, and successful particle manipulation is achieved by using Lamb wave mode or periodic resonant mode through using the piezoelectric phononic crystal plate. This technological breakthrough serves as a crucial foundation and experimental validation for developing the compact, low-energy and high-precision acoustic tweezers.

Keywords:

作者及机构信息

1.
吉首大学物理系, 吉首　416000

2.
中国科学院深圳先进技术研究院, 劳特伯生物医学成像研究中心, 深圳　518055

3.
医学成像科学与技术系统中国科学院重点实验室, 深圳　518055

通信作者: 蔡飞燕, fy.cai@siat.ac.cn ; 邓科, dengke@jsu.edu.cn ;

基金项目: 国家自然科学基金(批准号: 11974372, 12004409, 11964011, 12074402)和深圳市科技计划(批准号: RCJC20221008092808013, JCYJ20200109105823170, JCYJ20200109110006136)资助的课题.

Authors and contacts

1.
Department of Physics, Jishou University, Jishou 416000, China

2.
Paul C. Lauterbur Research Center for Biomedical Imaging, Shenzhen Institutes of Advanced Technology, Chinese Academy of Sciences, Shenzhen 518055, China

3.
Key Laboratory of Biomedical Imaging Science and System, Chinese Academy of Sciences, Shenzhen 518055, China

Corresponding author: Cai Fei-Yan, fy.cai@siat.ac.cn ; Deng Ke, dengke@jsu.edu.cn ;

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 11974372, 12004409, 11964011, 12074402) and the Science and Technology Program of Shenzhen, China (Grant Nos. RCJC20221008092808013, JCYJ20200109105823170, JCYJ20200109110006136).

文章全文 : translate this paragraph

补充材料

7-20231886 实验视频.mp4

参考文献

[1]	Borgnis F E 1953 Rev. Mod. Phys. 25 653 Google Scholar
[2]	Takahi H, Yasutaka H, Akio A, Hideki N, Masahiko K, Naoki I 1993 J. Acoust. Soc. Am. 93 154 Google Scholar
[3]	Tatsuki F, Asier M, Bruce W, Thomas L H 2019 Appl. Phys. Lett. 115 064101 Google Scholar
[4]	Hirayama R, Martinez P D, Masuda N, Subramanian S 2019 Nature 575 320 Google Scholar
[5]	Smalley D E, Nygaard E, Squire K, Van W J, Rasmussen J, Gneiting S, Qaderi K, Goodsell J, Roger W, Lindsey M 2018 Nature 553 486 Google Scholar
[6]	Wiklund M, Radel S, Hawkes J J 2013 Lab. Chip. 13 25 Google Scholar
[7]	Gao Y, Harder R, Southworth S H, Guest J R, Huang X J, Yan Z J, Ocola L E, Yifat Y, Sule N, Ho P J 2019 Proc. Natl. Acad. Sci. U. S. A. 116 4018 Google Scholar
[8]	Ozcelik A, Rufo J, Guo F, Gu Y Y, Li P, Lata J, Huang T J 2018 Nat. Methods 15 1021 Google Scholar
[9]	Wixforth A 2003 Superlattice Microst. 33 389 Google Scholar
[10]	Strobl C J, Von G Z, Wixforth A 2004 IEEE T. Ultrason. Ferr. 51 1432 Google Scholar
[11]	Alzuaga S, Manceau J F, Bastien F 2005 J. Sound Vib. 282 151 Google Scholar
[12]	Wixforth A 2005 Methods Mol. Med. 114 121
[13]	Alvarez M, Friend J R, Yeo L Y 2008 Langmuir 24 10629 Google Scholar
[14]	Shi J, Ahmed D, Mao X, Lin S C S, Lawit A, Huang T J 2009 Lab. Chip. 9 2890 Google Scholar
[15]	Li P Q, Zhou W, Peng B X, Zhang C Q, Zhu X F, Meng L, Wu J R, Zheng H R 2023 Phys. Rev. A 20 064003 Google Scholar
[16]	Huang Y Q, Das P K, Bhethanabotla V R 2021 Sens. Actuators Rep. 3 100041 Google Scholar
[17]	Wu J R 1991 J. Acoust. Soc. Am. 89 2140 Google Scholar
[18]	Lee J, Teh S Y, Lee A, Kim H H, Lee C, Shung K K 2009 Appl. Phys. Lett. 95 73701 Google Scholar
[19]	Marzo A, Seah S A, Drinkwater B W, Sahoo D R, Long B, Subramanian S 2015 Nat. Commum. 6 8661 Google Scholar
[20]	Melde K, Mark A G, Qiu T, Fischer P 2016 Nature 537 518 Google Scholar
[21]	Memoli G, Caleap M, Asakawa M, Sahoo D R, Drinkwater B W, Subramanian S 2017 Nat. Commun. 8 14608 Google Scholar
[22]	Li F, Cai F Y, Zhang L K, Liu Z Y, Li F, Meng L, Wu J R, Li J Y, Zhang X F, Zheng H R 2020 Phys. Rev. A 13 044077 Google Scholar
[23]	Li F, Cai F Y, Liu Z Y, Meng L, Qian M, Wang C, Cheng Q, Qian M L, Liu X, Wu J R, Li J Y, Zheng H R 2014 Phys. Rev. A 1 051001 Google Scholar
[24]	COMSOL, Sweden S https://cn.comsol.com/ [2023-11-21]
[25]	He Z J, Jia H, Qiu C Y, Peng S S, Mei X F, Cai F Y, Peng P, Ke M Z, Liu Z Y 2010 Phys. Rev. Lett. 105 074301 Google Scholar
[26]	Gor’kov L P 1962 Sov. Phys. Dokl. 6 773 Google Scholar
[27]	Bruus H 2012 Lab Chip 12 1014 Google Scholar

施引文献

图 1 (a)压电声子晶体板示意图; (b)压电声子晶体板单包示意图

Fig. 1. (a) Schematic of the piezoelectric phononic crystal plate; (b) schematic of the piezoelectric phononic crystal plate in one unit cell.

下载: 全尺寸图片幻灯片

图 2 (a) 压电声子晶板色散曲线 (蓝色点线是A₀模式, 红色点线是S₀模式, 黑色实线是水线); (b) 不同厚度的压电声子晶板共振与频率的关系; (c) 压电声子晶体板共振声场分布, f₁ = 0.998 MHz (左)和f₂ = 1.072 MHz (右)

Fig. 2. (a) Dispersion curve of the piezoelectric phononic crystal plate (Blue dotted line is A₀ mode, red dotted line is S₀ mode, and the solid black line is the water line); (b) resonance spectrum at normal incidence versus frequency for the piezoelectric phononic crystal plate with different heights; (c) resonant sound field distribution of the piezoelectric phononic crystal plate, f₁ = 0.998 MHz (left) and f₂ = 1.072 MHz (right).

下载: 全尺寸图片幻灯片

图 3 共振频率不同时, PS微球在压电声子晶体周围受到的声辐射力分布 (颜色深浅表示声辐射力的大小, 箭头方向表示声辐射力的方向)　(a) 共振频率f₁; (b) 共振频率f₂

Fig. 3. Distribution of the acoustic radiation force of PS microspheres around the piezoelectric phononic crystal at the different frequency (The color represents the magnitude of the acoustic radiation force, and the direction of the arrow represents the direction of the acoustic radiation force): (a) At the first frequency f₁; (b) at the second resonant frequency f₂.

下载: 全尺寸图片幻灯片

图 4 压电声子晶体板实验样品图　(a) 样品的上表面; (b) 样品的下表面

Fig. 4. Experimental sample diagram of the piezoelectric phononic crystal plate: (a) Upper surface of the sample; (b) lower surface of the sample.

下载: 全尺寸图片幻灯片

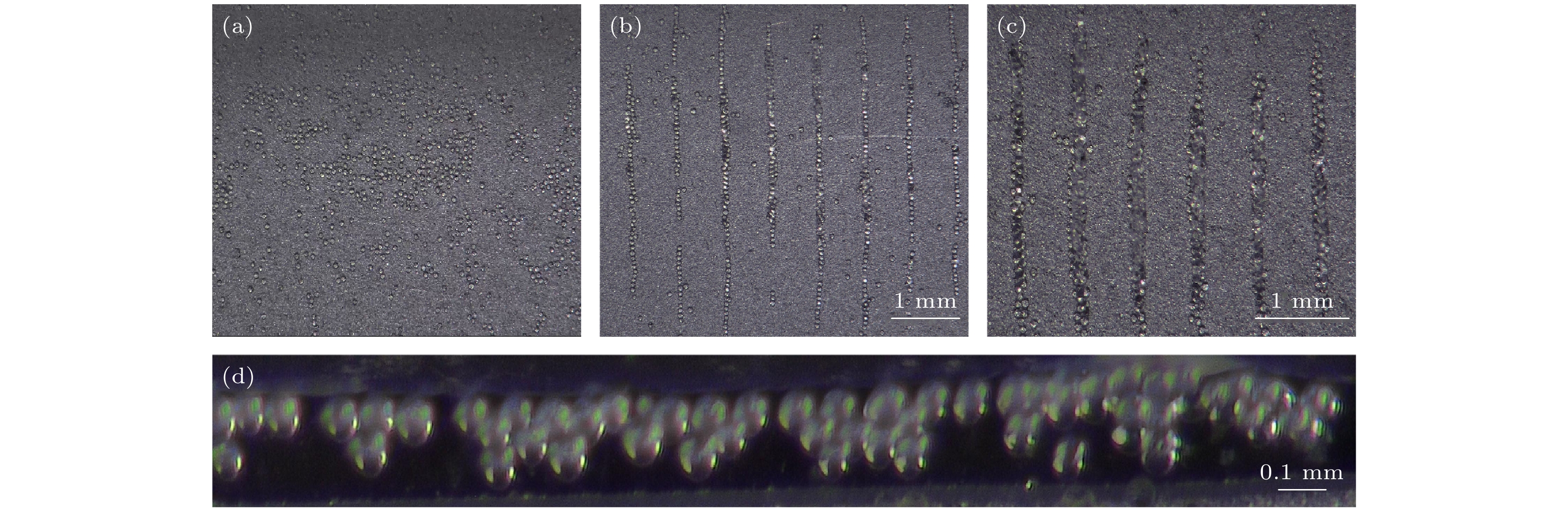
图 5 PS微球操控实验效果图　(a) 当压电声子晶体板未加载电信号时PS微球的初始状态; (b) 当压电声子晶体板加载共振频率为0.998 MHz的电信号时PS微球的状态; (c) 加载共振频率为1.072 MHz的电信号时PS微球的状态; (d) 图(c)侧视图

Fig. 5. (a) State of the PS particles when the piezoelectric phononic crystal plate is not loaded with an electrical signal; (b) state of the PS particles when the piezoelectric phononic crystal plate is loaded with an electrical signal at a resonant frequency of 0.998 MHz; (c) the same as panel (b) but at a resonant frequency of 1.072 MHz; (d) side view of panel (c).

下载: 全尺寸图片幻灯片

表 1 材料声学参数

Table 1. Material acoustic parameters.

	材料特性	符号	数值
		C₁₁	15.6
		C₁₂	8.9
	弹性系数C_pq/GPa	C₁₃	8.8
		C₃₃	13.2
		C₄₄	3.1
TJ-47		C₆₆	3.4
		e₁₅	14.3
	压电系数e_ip/(C·m^–2)	e₃₁	4.9
		e₃₃	18.5
	相对渗透系数$\varepsilon_{pq} $	$\varepsilon_{11} $	960
		$\varepsilon_{33} $	870
	密度/(kg·m^–3)	ρ	7800
	纵波速度/(m·s^–1)	c_l	2500
PS微球	横波速度/(m·s^–1)	c_t	1300
	密度/(kg·m^–3)	ρ	1080
水	纵波速度/(m·s^–1)	c_l	1500
水	密度/(kg·m^–3)	ρ	1000

下载: 导出CSV

map

[1]	Borgnis F E 1953 Rev. Mod. Phys. 25 653 Google Scholar
[2]	Takahi H, Yasutaka H, Akio A, Hideki N, Masahiko K, Naoki I 1993 J. Acoust. Soc. Am. 93 154 Google Scholar
[3]	Tatsuki F, Asier M, Bruce W, Thomas L H 2019 Appl. Phys. Lett. 115 064101 Google Scholar
[4]	Hirayama R, Martinez P D, Masuda N, Subramanian S 2019 Nature 575 320 Google Scholar
[5]	Smalley D E, Nygaard E, Squire K, Van W J, Rasmussen J, Gneiting S, Qaderi K, Goodsell J, Roger W, Lindsey M 2018 Nature 553 486 Google Scholar
[6]	Wiklund M, Radel S, Hawkes J J 2013 Lab. Chip. 13 25 Google Scholar
[7]	Gao Y, Harder R, Southworth S H, Guest J R, Huang X J, Yan Z J, Ocola L E, Yifat Y, Sule N, Ho P J 2019 Proc. Natl. Acad. Sci. U. S. A. 116 4018 Google Scholar
[8]	Ozcelik A, Rufo J, Guo F, Gu Y Y, Li P, Lata J, Huang T J 2018 Nat. Methods 15 1021 Google Scholar
[9]	Wixforth A 2003 Superlattice Microst. 33 389 Google Scholar
[10]	Strobl C J, Von G Z, Wixforth A 2004 IEEE T. Ultrason. Ferr. 51 1432 Google Scholar
[11]	Alzuaga S, Manceau J F, Bastien F 2005 J. Sound Vib. 282 151 Google Scholar
[12]	Wixforth A 2005 Methods Mol. Med. 114 121
[13]	Alvarez M, Friend J R, Yeo L Y 2008 Langmuir 24 10629 Google Scholar
[14]	Shi J, Ahmed D, Mao X, Lin S C S, Lawit A, Huang T J 2009 Lab. Chip. 9 2890 Google Scholar
[15]	Li P Q, Zhou W, Peng B X, Zhang C Q, Zhu X F, Meng L, Wu J R, Zheng H R 2023 Phys. Rev. A 20 064003 Google Scholar
[16]	Huang Y Q, Das P K, Bhethanabotla V R 2021 Sens. Actuators Rep. 3 100041 Google Scholar
[17]	Wu J R 1991 J. Acoust. Soc. Am. 89 2140 Google Scholar
[18]	Lee J, Teh S Y, Lee A, Kim H H, Lee C, Shung K K 2009 Appl. Phys. Lett. 95 73701 Google Scholar
[19]	Marzo A, Seah S A, Drinkwater B W, Sahoo D R, Long B, Subramanian S 2015 Nat. Commum. 6 8661 Google Scholar
[20]	Melde K, Mark A G, Qiu T, Fischer P 2016 Nature 537 518 Google Scholar
[21]	Memoli G, Caleap M, Asakawa M, Sahoo D R, Drinkwater B W, Subramanian S 2017 Nat. Commun. 8 14608 Google Scholar
[22]	Li F, Cai F Y, Zhang L K, Liu Z Y, Li F, Meng L, Wu J R, Li J Y, Zhang X F, Zheng H R 2020 Phys. Rev. A 13 044077 Google Scholar
[23]	Li F, Cai F Y, Liu Z Y, Meng L, Qian M, Wang C, Cheng Q, Qian M L, Liu X, Wu J R, Li J Y, Zheng H R 2014 Phys. Rev. A 1 051001 Google Scholar
[24]	COMSOL, Sweden S https://cn.comsol.com/ [2023-11-21]
[25]	He Z J, Jia H, Qiu C Y, Peng S S, Mei X F, Cai F Y, Peng P, Ke M Z, Liu Z Y 2010 Phys. Rev. Lett. 105 074301 Google Scholar
[26]	Gor’kov L P 1962 Sov. Phys. Dokl. 6 773 Google Scholar
[27]	Bruus H 2012 Lab Chip 12 1014 Google Scholar

[1]	林基艳, 林书玉. 管柱型近周期声子晶体点缺陷结构的大尺寸压电超声换能器. , 2023, 72(9): 094301. doi: 10.7498/aps.72.20230195
[2]	陈聪, 张若钦, 李锋, 李志远. 基于亚波长管道增强的漩涡声场悬浮操控微粒和液滴的实验研究. , 2023, 72(12): 124302. doi: 10.7498/aps.72.20230383
[3]	潘瑞琪, 李凡, 杜芷玮, 胡静, 莫润阳, 王成会. 平面波声场中内置偏心液滴的弹性球壳声辐射力. , 2023, 72(5): 054302. doi: 10.7498/aps.72.20222155
[4]	齐绍富, 蔡飞燕, 田振, 黄先玉, 周娟, 王金萍, 李文成, 郑海荣, 邓科. 基于一维声栅共振场的大规模微粒并行排列的实验研究. , 2023, 72(2): 024301. doi: 10.7498/aps.72.20221793
[5]	王燕萍, 蔡飞燕, 李飞, 张汝钧, 李永川, 王金萍, 张欣, 郑海荣. 基于二维声子晶体板共振声场的微粒操控. , 2023, 72(14): 144207. doi: 10.7498/aps.72.20230099
[6]	刘浪, 王一平. 基于可调频光力晶格中声子-光子拓扑性质的模拟和探测. , 2022, 71(22): 224202. doi: 10.7498/aps.71.20221286
[7]	臧雨宸, 苏畅, 吴鹏飞, 林伟军. 零阶Bessel驻波场中任意粒子声辐射力和力矩的Born近似. , 2022, 71(10): 104302. doi: 10.7498/aps.71.20212251
[8]	臧雨宸, 林伟军, 苏畅, 吴鹏飞. Gauss声束对离轴椭圆柱的声辐射力矩. , 2021, 70(8): 084301. doi: 10.7498/aps.70.20201635
[9]	朱纪霖, 高东宝, 曾新吾. 基于相位变换声镊的单个微粒平面移动操控. , 2021, 70(21): 214302. doi: 10.7498/aps.70.20210981
[10]	耿治国, 彭玉桂, 沈亚西, 赵德刚, 祝雪丰. 手性声子晶体中拓扑声传输. , 2019, 68(22): 227802. doi: 10.7498/aps.68.20191007
[11]	孙炜海, 张超群, 鞠桂玲, 潘晶雯. 含磁电弹夹层的压电/压磁声子晶体带隙特性研究. , 2018, 67(19): 194303. doi: 10.7498/aps.67.20180925
[12]	陈泽国, 吴莹. 声子晶体中的多重拓扑相. , 2017, 66(22): 227804. doi: 10.7498/aps.66.227804
[13]	黄先玉, 蔡飞燕, 李文成, 郑海荣, 何兆剑, 邓科, 赵鹤平. 空气中一维声栅对微粒的声操控. , 2017, 66(4): 044301. doi: 10.7498/aps.66.044301
[14]	梁彬, 袁樱, 程建春. 声单向操控研究进展. , 2015, 64(9): 094305. doi: 10.7498/aps.64.094305
[15]	任洪亮, 丁攀峰, 李小燕. 光镊轴向阱位操控及器件安装误差对径向阱位操控的影响. , 2012, 61(21): 210701. doi: 10.7498/aps.61.210701
[16]	杨立峰, 王亚非, 周鹰. 一维压电Fibonacci类准周期声子晶体传输特性. , 2012, 61(10): 107702. doi: 10.7498/aps.61.107702
[17]	魏琦, 程营, 刘晓峻. 点缺陷阵列对声子晶体波导定向辐射性能的影响. , 2011, 60(12): 124301. doi: 10.7498/aps.60.124301
[18]	陈圣兵, 韩小云, 郁殿龙, 温激鸿. 不同压电分流电路对声子晶体梁带隙的影响. , 2010, 59(1): 387-392. doi: 10.7498/aps.59.387
[19]	李晓春, 易秀英, 肖清武, 梁宏宇. 三组元声子晶体中的缺陷态. , 2006, 55(5): 2300-2305. doi: 10.7498/aps.55.2300
[20]	张碧星, 汪承灏, Anders Bostr?m. 压电条SH声辐射场研究. , 2005, 54(5): 2111-2117. doi: 10.7498/aps.54.2111

7-20231886 实验视频.mp4

计量

文章访问数: 1824
PDF下载量: 77
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板