-
研究了在环形势阱中自旋-轨道耦合旋转玻色-爱因斯坦凝聚体的基态结构. 探索了自旋-轨道耦合作用和旋转效应对基态的影响. 结果发现, 在环形势阱下, 基态结构呈现环形分布的half-skyrmion链. 调节自旋-轨道耦合强度, 不仅可以改变体系内half-skyrmion数量, 而且能够调控half-skyrmion环形排列的对称性. 随着旋转频率增大, 体系从平面波相转化为环形对称排列的half-skyrmion链相, 最后过渡到三角格子的half-skyrmion相. 讨论了自旋相互作用和势阱形状对基态的影响. 自旋-轨道耦合强度和旋转频率作为体系的调控参数, 可用于控制不同基态相间的转化.
-
关键词:
- 玻色-爱因斯坦凝聚体 /
- 环形势阱 /
- 自旋-轨道耦合 /
- 旋转
Realization of spinor Bose-Einstein condensate in an optical trap has made it possible to create a variety of topological nontrivial structures, due to the vector character of the order parameter. Recently, artificial spin-orbit coupling in the spinor Bose-Einstein condensate, owing to coupling between the spin and the center-of-mass motion of the atom, provides an unprecedented opportunity to search for novel quantum states. As is well known, the potential well in the Bose-Einstein condensate is adjustable. The toroidal trap is an important model potential because of its simplicity and richness in physics. In particular, the spinor Bose-Einstein condensate under the toroidal trap has brought an ideal platform for studying fascinating properties of a superfluid, such as persistent flow and symmetry-breaking localization. For the case of the spin-orbit-coupled Bose-Einstein condensate, the previous studies of the toroidal trap mainly focused on the two-component or antiferromagnetic case. However, in the presence of a toroidal trap, there remains an open question whether the combined effects of the spin-orbit coupling and rotation can produce previously unknown types of topological excitations in the ferromagnetic Bose-Einstein condensate. In this work, by using quasi two-dimensional Gross-Pitaevskii equations, we study the ground state structure of spin-orbit coupled rotating ferromagnetic Bose-Einstein condensate in the toroidal trap. We concentrate on the effects of the spin-orbit coupling and the rotation on the ground states. The numerical results show that in the presence of a toroidal trap, the ground state structure is displayed as half-skyrmion chain with circular distribution. Adjusting the strength of spin-orbit coupling not only changes the number of half-skyrmion in the system, but also controls the symmetry of half-skyrmion with circular distribution. As the rotation frequency increases, the system undergoes the transitions from the plane wave to the half-skyrmion chain with circular distribution, and eventually developing the half-skyrmion phase of triangular lattice. Next, we examine the effect of spin-independent interaction on spin-orbit coupled rotating spinor Bose-Einstein condensate. As the spin-independent interaction increases, the topological defects in the condensate increase due to the variation of the local magnetic order. We also discuss the influence of well shape on the ground state structure. These topological structures can be detected via the time-of-flight absorption imaging technique. The spin-orbit coupled spinor Bose-Einstein condensate in the toroidal trap is an important quantum platform, which not only opens up a new avenue for exploring the exotic topological structures, but also is crucial for realizing the transitions among different ground states. This work paves the way for futureexploring the topological defects and the corresponding dynamical stability in quantum system subjected to the toroidal trap.-
Keywords:
- Bose-Einstein condensate /
- toroidal trap /
- spin-orbit coupling /
- rotation
[1] Ho T L 1998 Phys. Rev. Lett. 81 742
Google Scholar
[2] Görlitz A, Gustavson T L, Leanhardt A E, Löw R, Chikkatur A P, Gupta S, Inouye S, Pritchard D E, Ketterle W 2003 Phys. Rev. Lett. 90 090401
Google Scholar
[3] Bloch I, Dalibard J, Zwerger W 2008 Rev. Mod. Phys. 80 885
Google Scholar
[4] Stamper-Kurn D M, Ueda M 2013 Rev. Mod. Phys. 85 1191
Google Scholar
[5] Li L, Li Z D, Malomed B A, Mihalache D, Liu W M 2005 Phys. Rev. A 72 033611
Google Scholar
[6] Li Z D, Li Q Y, He P B, Liang J Q, Liu W M, Fu G S 2010 Phys. Rev. A 81 015602
Google Scholar
[7] Zhao D, Song S W, Wen L, Li Z D, Luo H G, Liu W M 2015 Phys. Rev. A 91 013619
Google Scholar
[8] Isoshima T, Machida K, Ohmi T 2001 J. Phys. Soc. Jpn. 70 1604
Google Scholar
[9] Klausen N N, Bohn J L, Greene C H 2001 Phys. Rev. A 64 053602
Google Scholar
[10] Tuchiya S J, Kurihara S 2001 J. Phys. Soc. Jpn. 70 1182
Google Scholar
[11] Kasamatsu K, Tsubota M, Ueda M 2005 Int. J. Mod. Phys. B 19 1835
Google Scholar
[12] Martikainen J P, Collin A, Suominen K A 2002 Phys. Rev. A 66 053604
Google Scholar
[13] Mizushima T, Machida K, Kita T 2002 Phys. Rev. A 66 053610
Google Scholar
[14] Mizushima T, Kobayashi N, Machida K 2004 Phys. Rev. A 70 043613
Google Scholar
[15] Schweikhard V, Coddington I, Engels P, Tung S, Cornell E A 2004 Phys. Rev. Lett. 93 210403
Google Scholar
[16] Ji A C, Liu W M, Song J L, Zhou F 2008 Phys. Rev. Lett. 101 010402
Google Scholar
[17] Stenger J, Inouye S, Stamper-Kurn D M, Miesner H J, Chikkatur A P, Ketterle W 1998 Nature 396 345
Google Scholar
[18] Seo S W, Kang S J, Kwon W J, Shin Y 2015 Phys. Rev. Lett. 115 015301
Google Scholar
[19] Seo S W, Kwon W J, Kang S J, Y. Shin Y 2016 Phys. Rev. Lett. 116 185301
Google Scholar
[20] Mizushima T, Machida K, Kita T 2002 Phys. Rev. Lett. 89 030401
Google Scholar
[21] Orlova N V, Kuopanportti P, Milošević M V 2016 Phys. Rev. A 94 023617
Google Scholar
[22] Lin Y J, Jiménez-García K, Spielman I B 2011 Nature 471 83
Google Scholar
[23] Ji S C, Zhang J Y, Zhang L, Du Z D, Zheng W, Deng Y J, Zhai H, Chen S, Pan J W 2014 Nat. Phys. 10 314
Google Scholar
[24] Wu Z, Zhang L, Sun W, 1 Xu X T, Wang B Z, Ji S C, Deng Y J, Chen S, Liu X J, Pan J W 2016 Science 354 83
Google Scholar
[25] Huang L H, Meng Z M, Wang P J, Peng P, Zhang S L, Chen L C, Li D H, Zhou Q, Zhang J 2016 Nat. Phys. 12 540
Google Scholar
[26] Wang P J, Yu Z Q, Fu Z K, Miao J, Huang L H, Chai S J, Zhai H, Zhang J 2012 Phys. Rev. Lett. 109 095301
Google Scholar
[27] Campbell D L, Juzeliúnas G, Spielman I B 2011 Phys. Rev. A 84 025602
Google Scholar
[28] Zhang J Y, Ji S C, Chen Z, Zhang L, Du Z D, Yan B, Pan J S, Zhao B, Deng Y J, Zhai H, Chen S, Pan J W 2012 Phys. Rev. Lett. 109 115301
Google Scholar
[29] Liu X J, Borunda M F, Liu X, Sinova J 2009 Phys. Rev. Lett. 102 046402
Google Scholar
[30] Anderson B M, Spielman I B, Juzeliúnas G 2013 Phys. Rev. Lett. 111 125301
Google Scholar
[31] Anderson B M, Juzeliúnas G, Galitski V M, Spielman I B 2012 Phys. Rev. Lett. 108 235301
Google Scholar
[32] Cheuk L W, Sommer A T, Hadzibabic Z, Yefsah T, Bakr W S, Zwierlein M W 2012 Phys. Rev. Lett. 109 095302
Google Scholar
[33] Ruseckas J, Juzeliúnas G, Öhberg P, Fleischhauer M 2005 Phys. Rev. Lett. 95 010404
Google Scholar
[34] Lan Z H, Öhberg P 2014 Phys. Rev. A 89 023630
Google Scholar
[35] Wang C J, Gao C, Jian C M, Zhai H 2010 Phys. Rev. Lett. 105 160403
Google Scholar
[36] Su S W, Liu I K, Tsai Y C, Liu W M, Gou S C 2012 Phys. Rev. A 86 023601
Google Scholar
[37] Sinha S, Nath R, Santos L 2011 Phys. Rev. Lett. 107 270401
Google Scholar
[38] Hu H, Ramachandhran B, Pu H, Liu X J 2012 Phys. Rev. Lett. 108 010402
Google Scholar
[39] Gopalakrishnan S, Martin I, Demler E A 2013 Phys. Rev. Lett. 111 185304
Google Scholar
[40] Liu C F, Liu W M 2012 Phys. Rev. A 86 033602
Google Scholar
[41] 刘超飞, 万文娟, 张赣源 2013 62 200306
Google Scholar
Liu C F, Wan W J, Zhang G Y 2013 Acta Phys. Sin. 62 200306
Google Scholar
[42] Capuzzi P, Jezek D M 2009 J. Phys. B: At. Mol. Opt. Phys. 42 145301
Google Scholar
[43] Benakli M, Raghavan S, Smerzi A, Fantoni S, Shenoy S R 1999 Europhys. Lett. 46 275
Google Scholar
[44] Mason P, Berloff N G 2009 Phys. Rev. A 79 043620
Google Scholar
[45] Aftalion A, Mason P 2010 Phys. Rev. A 81 023607
Google Scholar
[46] Ryu C, Andersen M F, Cladé P, Natarajan V, Helmerson K, Phillips W D 2007 Phys. Rev. Lett. 99 260401
Google Scholar
[47] Abad M, Guilleumas M, Mayol R, Pi M 2010 Phys. Rev. A 81 043619
Google Scholar
[48] Zhang X F, Kato M, Han W, Zhang S G, Saito H 2017 Phys. Rev. A 95 033620
Google Scholar
[49] Wang H, Wen L H, Yang H, Shi C X, Li J H 2017 J. Phys. B: At. Mol. Opt. Phys. 50 155301
Google Scholar
[50] Wang J G, Xu L L, Yang S J 2017 Phys. Rev. A 96 033629
Google Scholar
[51] Li J, Yu Y M, Zhuang L, Liu W M 2017 Phys. Rev. A 95 043633
Google Scholar
[52] Liu C F, Yu Y M, Gou S C, Liu W M 2013 Phys. Rev. A 87 063630
Google Scholar
[53] Han W, Zhang X F, Song S W, Saito H, Zhang W, Liu W M, Zhang S G 2016 Phys. Rev. A 94 033629
Google Scholar
[54] Li J, Zhang X F, Liu W M 2018 Ann. Phys.-New York 396 87
Google Scholar
[55] 刘静思, 李吉, 刘伍明 2017 66 130305
Google Scholar
Liu J S, Li J, Liu W M 2017 Acta Phys. Sin. 66 130305
Google Scholar
[56] 李吉, 刘伍明 2018 67 110302
Google Scholar
Li J, Liu W M 2018 Acta Phys. Sin. 67 110302
Google Scholar
[57] Urban M 2005 Phys. Rev. A 71 033611
Google Scholar
[58] Wang H Y, Zheng Z, Zhuang L, Tai Y H, Shi J S, Liu W M 2020 J. Phys.: Condens. Matter 32 235701
Google Scholar
-
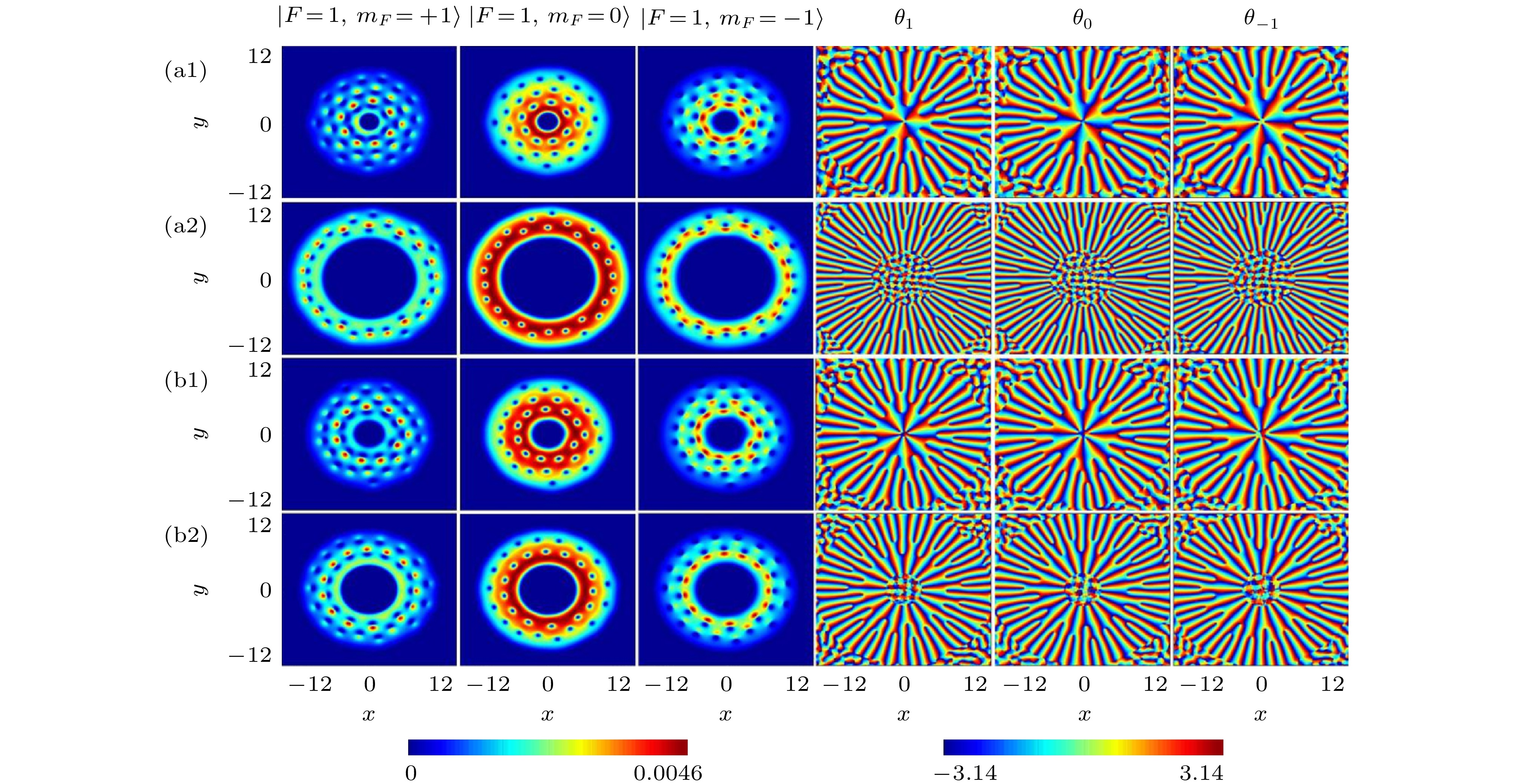
图 1 环形势阱中不同自旋-轨道耦合强度下铁磁87Rb BEC基态粒子数密度分布(第1, 2, 3列)和相位分布(第4, 5, 6列). 改变自旋-轨道耦合强度, 可以调控体系中half-skyrmion数量以及环形排列的对称性 (a)
$\kappa = 0.4$ ; (b)$\kappa = 0.8$ ; (c)$\kappa = 1.2$ ; (d)$\kappa = 3$ . 该图其余模拟参数选为${\lambda _0} = 3200$ ,${\lambda _2} = - 32$ ,$\varOmega = 0.2$ ,${V_0} = 300$ ,$\sigma = 2$ 和ω = 2π × 250 HzFig. 1. Ground state of the rotating ferromagnetic BEC of 87Rb for the different spin-orbit coupling strengths under the toroidal trap. The first, second and third columns show the particle number densities. The fourth, fifth and sixth columns show phase distributions. Changing the strength of spin-orbit coupling can control the number of half-skyrmion in the system and the symmetry of half-skyrmion with circular distribution. The parameters are set as follows: (a)
$\kappa = 0.4$ ; (b)$\kappa = 0.8$ ; (c)$\kappa = 1.2$ ; (d)$\kappa = 3$ . And the rest of parameters are${\lambda _0} = 3200$ ,${\lambda _2} = - 32$ ,$\varOmega = 0.2$ ,${V_0} = 300$ ,$\sigma = 2$ and ω = 2π × 250 Hz.
图 2 不同旋转频率对基态的影响. 随着旋转频率增大, 体系从平面波相转化为环形对称排列的half-skyrmion链相. 第1, 2, 3列描述粒子数密度分布; 第4, 5, 6列表示对应的相位分布 (a)
$\varOmega = 0.1$ ; (b)$\varOmega = 0.2$ ; (c)$\varOmega = 0.6$ ; (d)$\varOmega = 0.9$ . 该图其余模拟参数选为${\lambda _0} = 3200$ ,${\lambda _2} = - 32$ ,${V_0} = 300$ ,$\sigma = 2$ ,$\kappa = 0.4$ 和ω = 2π × 250 HzFig. 2. Effects of the different rotation frequency on ground state. With the increase of rotation frequency, the system transforms from plane wave phase to half-skyrmion chain phase with circular symmetry arrangement. The first, second and third columns are the particle number densities. The fourth, the fifth and the sixth columns are the corresponding phase distributions. The parameters are set as follows: (a)
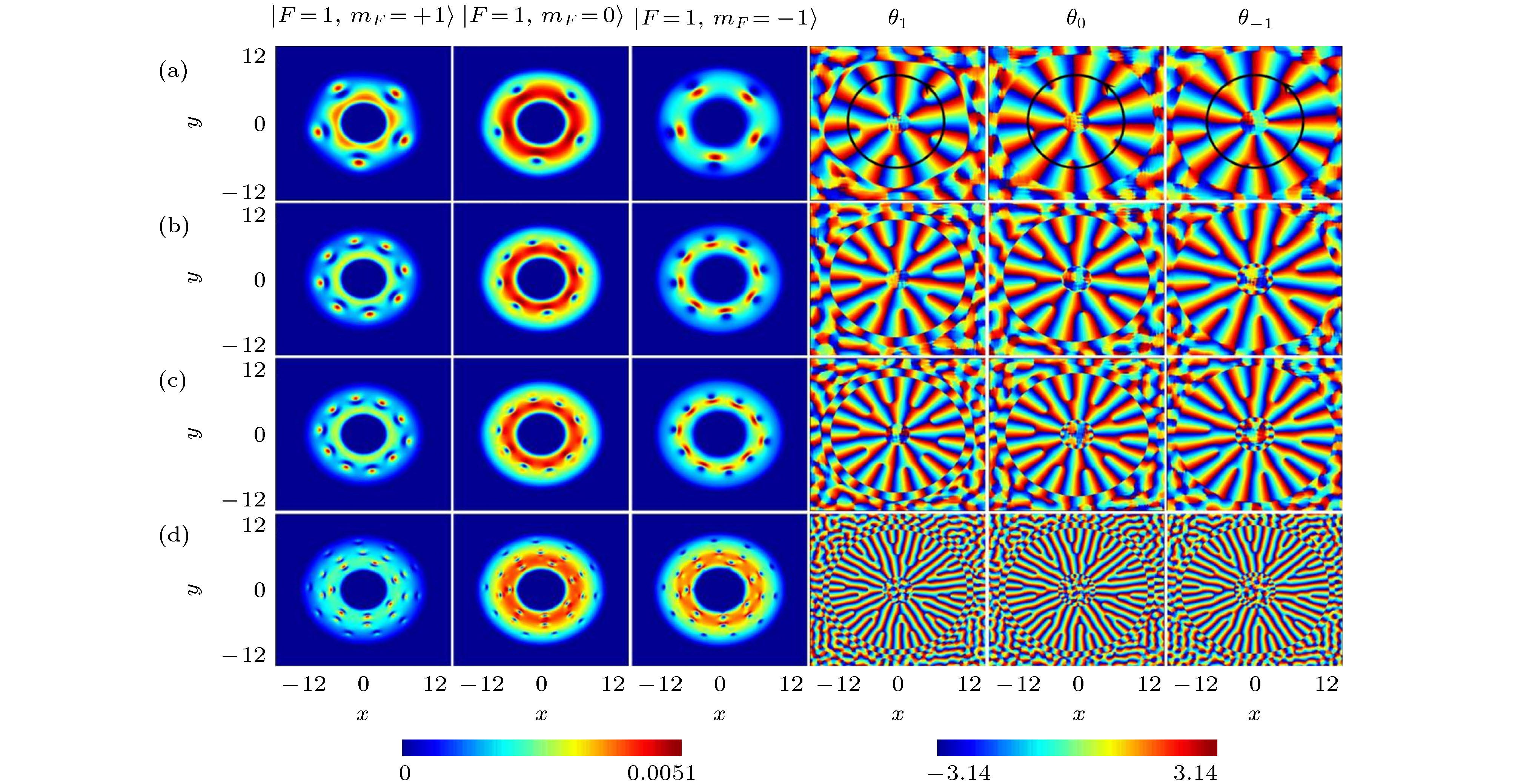
$\varOmega = 0.1$ ; (b)$\varOmega = 0.2$ ; (c)$\varOmega = 0.6$ ; (d)$\varOmega = 0.9$ . And the other parameters are${\lambda _0} = 3200$ ,${\lambda _2} = - 32$ ,${V_0} = 300$ ,$\sigma = 2$ ,$\kappa = 0.4$ and ω = 2π × 250 Hz.
图 3 不同自旋非相关作用和自旋相关作用对基态的影响. 随着自旋非相关作用强度增加, half-skyrmion分布从环形单层排列转化为环形双层排列. 不同自旋相关相互作用对half-skyrmion的数目影响微弱, 仅仅使half-skyrmion环形排列变得更规则 (a1)
${\lambda _0} = $ 1200,${\lambda _2} = - 32$ ; (a2)${\lambda _0} = 4600$ ,${\lambda _2} = - 32$ ; (b1)${\lambda _0} = 3200$ ,${\lambda _2} = - 12$ ; (b2)${\lambda _0} = 3200$ ,${\lambda _2} = - 9{\rm{6}}$ . 该图其余模拟参数选为$\varOmega = 0.6$ ,${V_0} = 300$ ,$\sigma = 2$ ,$\kappa = 0.4$ 和ω = 2π × 250 HzFig. 3. Effects of the different spin-independent and spin-dependent interactions on ground state. Increasing the strength of spin-independent interaction can induce the transition of half-skyrmion distribution from a circular monolayer arrangement to a circular bilayer arrangement. The influence of different spin-dependent interaction on the number of half-skyrmion is weak, which only makes the ring arrangement of half-skyrmion more regular. The parameters are set as follows: (a1)
${\lambda _0} = 1200$ ,${\lambda _2} = - 32$ ; (a2)${\lambda _0} = $ 4600,${\lambda _2} = - 32$ ; (b1)${\lambda _0} = 3200$ ,${\lambda _2} = - 12$ ; (b2)${\lambda _0} = 3200$ ,${\lambda _2} = - 9{\rm{6}}$ . And the other parameters are$\varOmega = 0.6$ ,${V_0} = 300$ ,$\sigma = 2$ ,$\kappa = 0.4$ and ω = 2π × 250 Hz.
图 4 不同势阱宽度和势阱高度对基态的影响. 改变势阱宽度和高度, 可以调控half-skyrmion链的环形分布. 基态粒子数密度分布如1, 2, 3列所示; 对应相位分布如4, 5, 6列所示 (a1)
${V_0} = 300$ ,$\sigma = 0.8$ ; (a2)${V_0} = 300$ ,$\sigma = 4$ ; (b1)${V_0} = 60$ ,$\sigma = 2$ ; (b2)${V_0} = {\rm{6}}00$ ,$\sigma = 2$ . 该图其余模拟参数选为${\lambda _0} = 3200$ ,${\lambda _2} = - 32$ ,$\varOmega = 0.6$ ,$\kappa = 0.4$ 和ω = 2π × 250 HzFig. 4. Effects of the width and the central height of the toroidal potential on ground state. The ring distribution of half-skyrmion chain can be controlled by changing the width and height of potential well. The particle number densities of ground state are shown in the first, second and third columns. The corresponding phase distributions are shown in the fourth, fifth and sixth columns. The parameters are set as follows: (a1)
${V_0} = 300$ ,$\sigma = 0.8$ ; (a2)${V_0} = 300$ ,$\sigma = 4$ ; (b1)${V_0} = 60$ ,$\sigma = 2$ ; (b2)${V_0} = {\rm{6}}00$ ,$\sigma = 2$ . And the other parameters are${\lambda _0} = 3200$ ,${\lambda _2} = - 32$ ,$\varOmega = 0.6$ ,$\kappa = 0.4$ and ω = 2π × 250 Hz.
图 5 不同基态的自旋结构 (a) 对应图2(a)的自旋构型; (b) 对应图1(b)的自旋构型; (c) 对应图2(c)的自旋构型; (d) 对应图2(d)的自旋构型. 图中圆圈区域表示一个half-skyrmion结构. 自旋密度平均值变化范围从–1(蓝色) 到 1(红色)
Fig. 5. The spin texture of the ground state: (a) Spin texture corresponding to the Fig. 2(a); (b) spin texture corresponding to the Fig. 1(b); (c) spin texture corresponding to the Fig. 2(c); (d) spin texture corresponding to the Fig. 2(d). The circle region in the graph represents a half-skyrmion structure. Values of the spin density are from –1 (blue) to 1 (red).
-
[1] Ho T L 1998 Phys. Rev. Lett. 81 742
Google Scholar
[2] Görlitz A, Gustavson T L, Leanhardt A E, Löw R, Chikkatur A P, Gupta S, Inouye S, Pritchard D E, Ketterle W 2003 Phys. Rev. Lett. 90 090401
Google Scholar
[3] Bloch I, Dalibard J, Zwerger W 2008 Rev. Mod. Phys. 80 885
Google Scholar
[4] Stamper-Kurn D M, Ueda M 2013 Rev. Mod. Phys. 85 1191
Google Scholar
[5] Li L, Li Z D, Malomed B A, Mihalache D, Liu W M 2005 Phys. Rev. A 72 033611
Google Scholar
[6] Li Z D, Li Q Y, He P B, Liang J Q, Liu W M, Fu G S 2010 Phys. Rev. A 81 015602
Google Scholar
[7] Zhao D, Song S W, Wen L, Li Z D, Luo H G, Liu W M 2015 Phys. Rev. A 91 013619
Google Scholar
[8] Isoshima T, Machida K, Ohmi T 2001 J. Phys. Soc. Jpn. 70 1604
Google Scholar
[9] Klausen N N, Bohn J L, Greene C H 2001 Phys. Rev. A 64 053602
Google Scholar
[10] Tuchiya S J, Kurihara S 2001 J. Phys. Soc. Jpn. 70 1182
Google Scholar
[11] Kasamatsu K, Tsubota M, Ueda M 2005 Int. J. Mod. Phys. B 19 1835
Google Scholar
[12] Martikainen J P, Collin A, Suominen K A 2002 Phys. Rev. A 66 053604
Google Scholar
[13] Mizushima T, Machida K, Kita T 2002 Phys. Rev. A 66 053610
Google Scholar
[14] Mizushima T, Kobayashi N, Machida K 2004 Phys. Rev. A 70 043613
Google Scholar
[15] Schweikhard V, Coddington I, Engels P, Tung S, Cornell E A 2004 Phys. Rev. Lett. 93 210403
Google Scholar
[16] Ji A C, Liu W M, Song J L, Zhou F 2008 Phys. Rev. Lett. 101 010402
Google Scholar
[17] Stenger J, Inouye S, Stamper-Kurn D M, Miesner H J, Chikkatur A P, Ketterle W 1998 Nature 396 345
Google Scholar
[18] Seo S W, Kang S J, Kwon W J, Shin Y 2015 Phys. Rev. Lett. 115 015301
Google Scholar
[19] Seo S W, Kwon W J, Kang S J, Y. Shin Y 2016 Phys. Rev. Lett. 116 185301
Google Scholar
[20] Mizushima T, Machida K, Kita T 2002 Phys. Rev. Lett. 89 030401
Google Scholar
[21] Orlova N V, Kuopanportti P, Milošević M V 2016 Phys. Rev. A 94 023617
Google Scholar
[22] Lin Y J, Jiménez-García K, Spielman I B 2011 Nature 471 83
Google Scholar
[23] Ji S C, Zhang J Y, Zhang L, Du Z D, Zheng W, Deng Y J, Zhai H, Chen S, Pan J W 2014 Nat. Phys. 10 314
Google Scholar
[24] Wu Z, Zhang L, Sun W, 1 Xu X T, Wang B Z, Ji S C, Deng Y J, Chen S, Liu X J, Pan J W 2016 Science 354 83
Google Scholar
[25] Huang L H, Meng Z M, Wang P J, Peng P, Zhang S L, Chen L C, Li D H, Zhou Q, Zhang J 2016 Nat. Phys. 12 540
Google Scholar
[26] Wang P J, Yu Z Q, Fu Z K, Miao J, Huang L H, Chai S J, Zhai H, Zhang J 2012 Phys. Rev. Lett. 109 095301
Google Scholar
[27] Campbell D L, Juzeliúnas G, Spielman I B 2011 Phys. Rev. A 84 025602
Google Scholar
[28] Zhang J Y, Ji S C, Chen Z, Zhang L, Du Z D, Yan B, Pan J S, Zhao B, Deng Y J, Zhai H, Chen S, Pan J W 2012 Phys. Rev. Lett. 109 115301
Google Scholar
[29] Liu X J, Borunda M F, Liu X, Sinova J 2009 Phys. Rev. Lett. 102 046402
Google Scholar
[30] Anderson B M, Spielman I B, Juzeliúnas G 2013 Phys. Rev. Lett. 111 125301
Google Scholar
[31] Anderson B M, Juzeliúnas G, Galitski V M, Spielman I B 2012 Phys. Rev. Lett. 108 235301
Google Scholar
[32] Cheuk L W, Sommer A T, Hadzibabic Z, Yefsah T, Bakr W S, Zwierlein M W 2012 Phys. Rev. Lett. 109 095302
Google Scholar
[33] Ruseckas J, Juzeliúnas G, Öhberg P, Fleischhauer M 2005 Phys. Rev. Lett. 95 010404
Google Scholar
[34] Lan Z H, Öhberg P 2014 Phys. Rev. A 89 023630
Google Scholar
[35] Wang C J, Gao C, Jian C M, Zhai H 2010 Phys. Rev. Lett. 105 160403
Google Scholar
[36] Su S W, Liu I K, Tsai Y C, Liu W M, Gou S C 2012 Phys. Rev. A 86 023601
Google Scholar
[37] Sinha S, Nath R, Santos L 2011 Phys. Rev. Lett. 107 270401
Google Scholar
[38] Hu H, Ramachandhran B, Pu H, Liu X J 2012 Phys. Rev. Lett. 108 010402
Google Scholar
[39] Gopalakrishnan S, Martin I, Demler E A 2013 Phys. Rev. Lett. 111 185304
Google Scholar
[40] Liu C F, Liu W M 2012 Phys. Rev. A 86 033602
Google Scholar
[41] 刘超飞, 万文娟, 张赣源 2013 62 200306
Google Scholar
Liu C F, Wan W J, Zhang G Y 2013 Acta Phys. Sin. 62 200306
Google Scholar
[42] Capuzzi P, Jezek D M 2009 J. Phys. B: At. Mol. Opt. Phys. 42 145301
Google Scholar
[43] Benakli M, Raghavan S, Smerzi A, Fantoni S, Shenoy S R 1999 Europhys. Lett. 46 275
Google Scholar
[44] Mason P, Berloff N G 2009 Phys. Rev. A 79 043620
Google Scholar
[45] Aftalion A, Mason P 2010 Phys. Rev. A 81 023607
Google Scholar
[46] Ryu C, Andersen M F, Cladé P, Natarajan V, Helmerson K, Phillips W D 2007 Phys. Rev. Lett. 99 260401
Google Scholar
[47] Abad M, Guilleumas M, Mayol R, Pi M 2010 Phys. Rev. A 81 043619
Google Scholar
[48] Zhang X F, Kato M, Han W, Zhang S G, Saito H 2017 Phys. Rev. A 95 033620
Google Scholar
[49] Wang H, Wen L H, Yang H, Shi C X, Li J H 2017 J. Phys. B: At. Mol. Opt. Phys. 50 155301
Google Scholar
[50] Wang J G, Xu L L, Yang S J 2017 Phys. Rev. A 96 033629
Google Scholar
[51] Li J, Yu Y M, Zhuang L, Liu W M 2017 Phys. Rev. A 95 043633
Google Scholar
[52] Liu C F, Yu Y M, Gou S C, Liu W M 2013 Phys. Rev. A 87 063630
Google Scholar
[53] Han W, Zhang X F, Song S W, Saito H, Zhang W, Liu W M, Zhang S G 2016 Phys. Rev. A 94 033629
Google Scholar
[54] Li J, Zhang X F, Liu W M 2018 Ann. Phys.-New York 396 87
Google Scholar
[55] 刘静思, 李吉, 刘伍明 2017 66 130305
Google Scholar
Liu J S, Li J, Liu W M 2017 Acta Phys. Sin. 66 130305
Google Scholar
[56] 李吉, 刘伍明 2018 67 110302
Google Scholar
Li J, Liu W M 2018 Acta Phys. Sin. 67 110302
Google Scholar
[57] Urban M 2005 Phys. Rev. A 71 033611
Google Scholar
[58] Wang H Y, Zheng Z, Zhuang L, Tai Y H, Shi J S, Liu W M 2020 J. Phys.: Condens. Matter 32 235701
Google Scholar
下载:
计量
- 文章访问数: 9457
- PDF下载量: 345
- 被引次数: 0
