-
仿星器线圈的构形形变在制造和装配过程中是不可避免的, 这些形变会导致误差场的产生, 仿星器的磁场位形对误差场非常敏感, 严重制约等离子体的约束性能. 因此, 评估线圈形变对仿星器磁拓扑结构的影响是非常重要的研究课题. 本文研究了中国首台准环对称仿星器(CFQS)上非平面模块化线圈(MC)形变对真空场下磁拓扑结构的影响. 利用磁岛宽度变化来衡量线圈形变造成的误差场, 采用3种旋转变换(ι = 2/4, 2/5和2/6)的磁岛位形, 分别考虑了每个模块化线圈的面内扰动和面外扰动. 结果表明, 同一线圈的形变会产生不同的共振误差场, 且这些误差场的幅度各不相同; 共振误差场对每个线圈形变的敏感度不同, 最复杂线圈的面内扰动可能对磁拓扑结构的影响并不明显; 共振误差场对线圈面外扰动的灵敏度高于面内扰动的灵敏度. 这些结果表明放宽特定线圈的构形误差不会显著影响仿星器的磁场位形, 有望缓解对MC线圈设计和制造的工程限制. 此外, 这项工作还有助于为即将进行的CFQS磁位形示踪实验提供精确的理论模型.The configuration deformation of stellarator coil is inevitable during fabrication and assembly, resulting in error fields. The magnetic field configuration in stellarator is sensitive to the error field, which seriously restricts the confinement performance of the plasma. Therefore, it is essential to estimate the influence of coil deformations on a stellarator magnetic topology. This work is dedicated to studying the influence of deformations of nonplanar modular coils (MC) on the magnetic topology in the Chinese First Quasi-toroidally symmetric Stellarator (CFQS). In this work, by changing the Fourier coefficients that represent the current-carrying surface(CCS) and the coil, two types of deformation coils, i.e. "in-surface" and "out-of-surface" disturbance on each MC can be obtained. Subsequently, three kinds of magnetic islands with rotational transformsι = 2/4, 2/5, and 2/6 are used to identify coil deviations that have a significant influence on the CFQS magnetic configuration. Several important results are obtained as follows. i) The same deformation of a coil gives rise to various resonant error fields with different amplitudes. ii) The sensitivity of a resonant error field to the deformation of each coil is different. The in-surface disturbance of the most complex coil may not have a significant influence on the magnetic topology structure. iii) The sensitivity of the resonant error field to out-of-surface disturbance in the coil is higher than that to in-surface disturbance. These results indicate that relaxing the configuration error of specific coil will not significantly affect the magnetic field configuration of the stellarator, which is expected to alleviate engineering limitations on MC coil design and fabrication. In addition, this work will also contribute to providing an accurate computational model for the upcoming CFQS magnetic configuration tracing experiment.
-
Keywords:
- quasi-axisymmetric stellarator /
- coil deformations /
- error fields /
- magnetic configuration
-
图 1 CFQS的线圈系统, 线圈系统包含16个非平面模块化线圈, 12个环向场线圈和4个极向场线圈
Fig. 1. Main components of the CFQS coil system, the coil system includes 16 non-planar modular coils, 12 planar toroidal field coils, and 4 poloidal field coils.
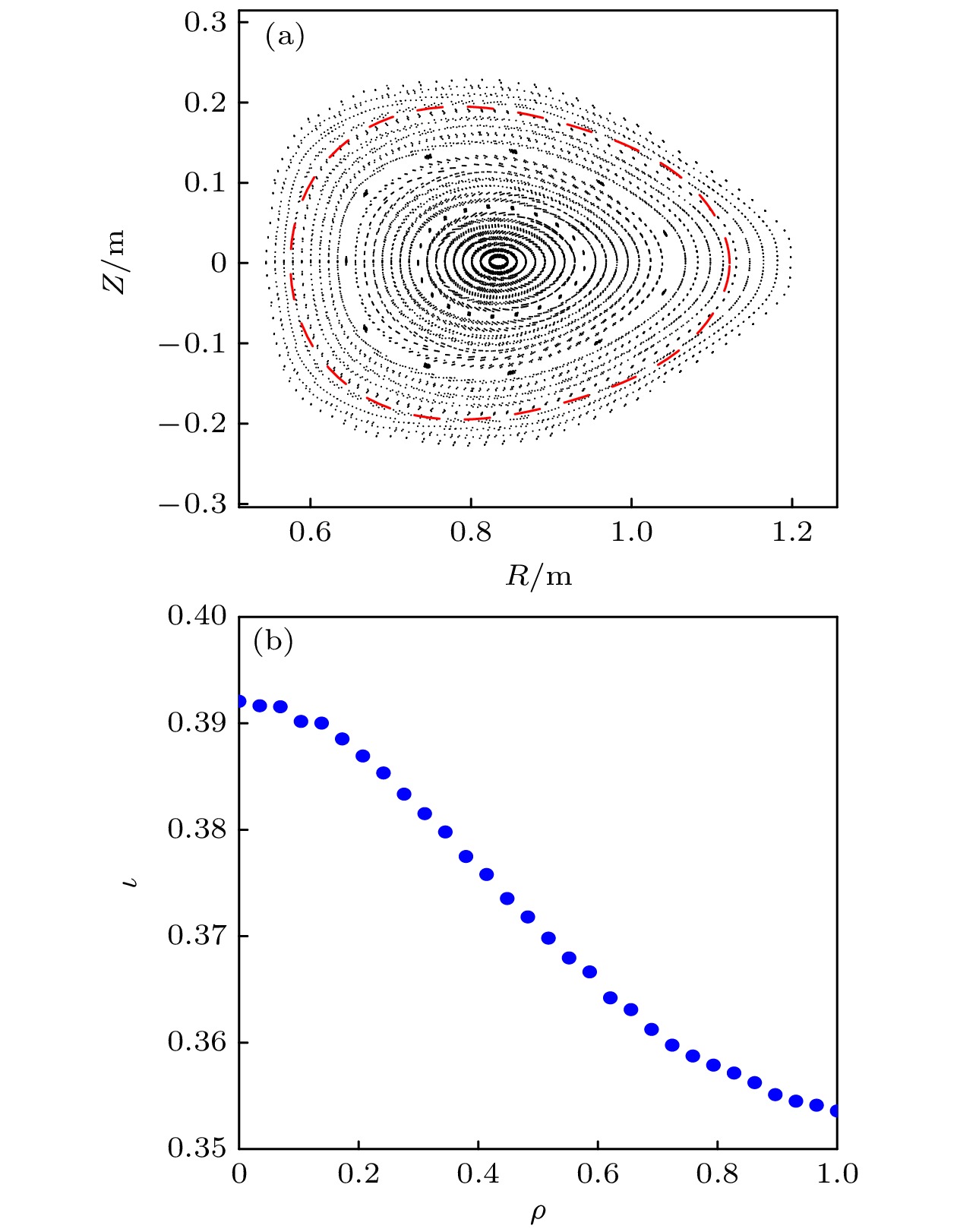
图 2 (a) 由磁力线追踪计算得到的理想MC线圈产生的磁场在环向角ξ = 90°横截面处的庞加莱图(黑色虚线)和目标等离子体边界(红色虚线), 追踪的初始位置在Z = 0, R∈[0.5446, 0.8359]处, 追踪周期为270; (b) 与该磁场截面对应的旋转变换剖面, 横坐标为归一化半径
Fig. 2. (a) Poincaré plots (black dots) based on tracing field lines in the magnetic configuration produced by the designed MCs and the target plasma boundary (red dashed) at the triangular-shaped cross-section, field lines with initial positions R∈ [0.5446, 0.8359] and Z = 0 are traced 270 periods; (b) the corresponding rotational transform profile with the normalized radius as its abscissa.
图 3 由理想线圈产生的n/m = 2/4 (a), 2/5(b)和2/6(c) 的3种磁岛位形的庞加莱截面图及旋转变换剖面, 横坐标表示从主磁轴到磁场外侧的半径, 每个磁面的追踪周期为540, 追踪的初始位置为 (a) Z = 0, R∈[0.54, 0.81]; (b) Z = 0, R∈[0.56, 0.83]; (c) Z = 0, R∈[0.44, 0.76]
Fig. 3. Poincaré plots of three island configurations with n/m = 2/4 (a), 2/5(b) and 2/6(c) and their rotational transform profiles produced by undeformed coils, the abscissa denotes radius from the main magnetic axis to the outboard side, field lines with initial positions R∈[0.54, 0.81] and Z = 0 (a), R∈[0.56, 0.83] and Z = 0 (b), R∈[0.44, 0.76] and Z = 0 (c) are traced 540 periods.
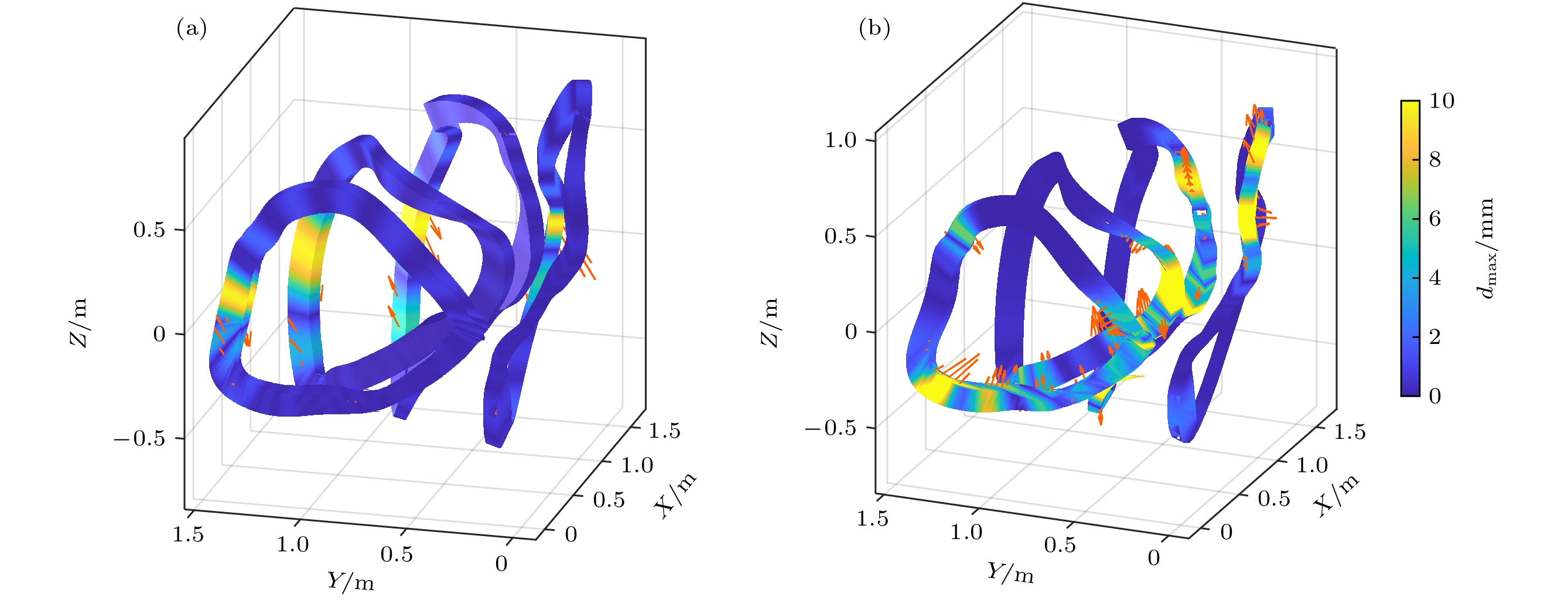
图 4 四种不同类型MC线圈的面内(a)和面外(b)形变分布, 在MC1, MC2, MC3和MC4上设置($ {\delta }_{1} $, $ {\delta }_{2} $) = (0.00003, 0.0001), (0.00002, 0.00009), (0.00002, 0.00009)和(0.00004, 0.000095)以产生线圈的面内扰动, 在CCS上设置$ {\delta }_{3} $ = 0.0113, 0.086, 0.094, 0.074以产生面外线圈扰动, 在这两种情况下, 每个MC的最大形变量均为10 mm, $ {\delta }_{1} $, $ {\delta }_{2} $, $ {\delta }_{3} $的数值均为随机选取
Fig. 4. Local (a) and broad (b) deformation distributions on four different types of MCs, ($ {\delta }_{1} $, $ {\delta }_{2} $) = (0.00003, 0.0001), (0.00002, 0.00009), (0.00002, 0.00009), (0.00004, 0.000095) are set on MC1, MC2, MC3 and MC4 to produce local perturbations of coils and $ {\delta }_{3} $ = 0.0113, 0.086, 0.094, 0.074 are set on MC1, MC2, MC3, MC4 to produce broad perturbations of coils. For these two cases the maximum deformation of each MC is 10 mm.
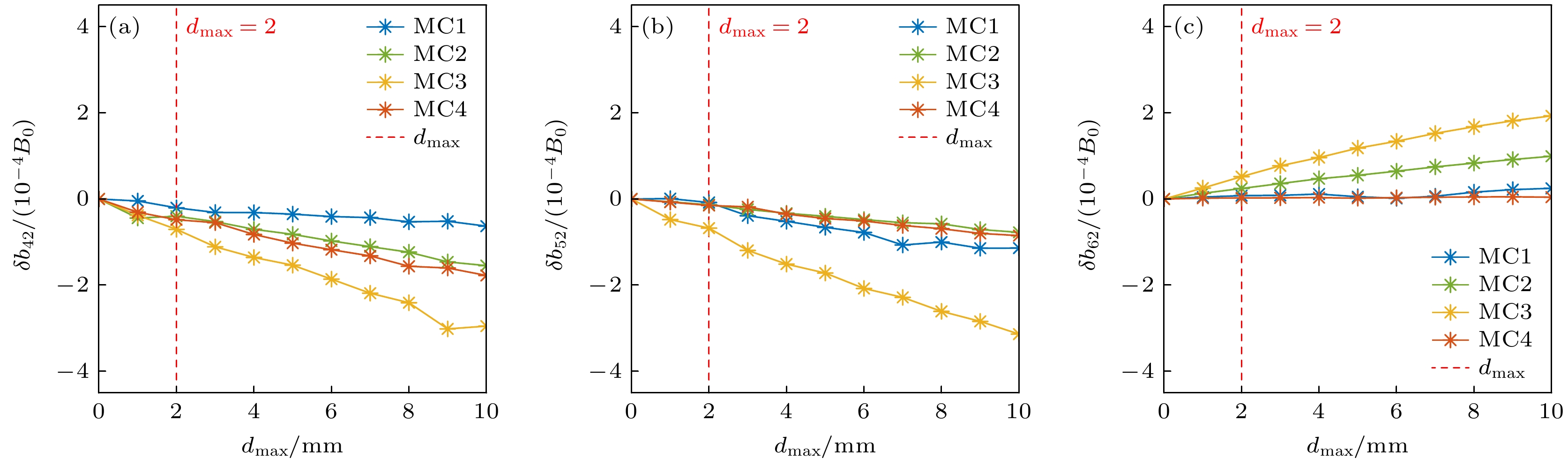
图 5 扰动MC1线圈使其产生最大形变量为10 mm时的3种磁岛位形的庞加莱截图, 红色虚线和蓝色虚线分别表示由理想线圈产生的磁岛边界和MC1线圈发生形变时的磁岛边界. 场线数值与图4相同
Fig. 5. Poincaré plots of three island configurations with n/m = 2/4 (a), 2/5(b) and 2/6(c) produced by perturbed MC1 with the maximum deviations of 10 mm (other coils sustain undeformed). Red and blue dots denote boundaries of the island chains induced by designed coils and the deformed MCs. Numerical details for field line tracing are the same as shown in Fig. 4.
图 6 在n/m = 2/4(a), 2/5(b), 2/6(c)岛链中, 磁岛宽度变化量的绝对值与线圈最大形变量的关系, 每个MC线圈的形变扰动均受到载流面的限制
Fig. 6. The absolute value of the magnetic island width as a function of dmax of each MC in n/m = 2/4 (a), 2/5(b) and 2/6(c) island chains, the deformations of each MC are without perturbations of CCS.
图 7 在n/m = 2/4(a), 2/5(b), 2/6(c)岛链中, 误差场振幅与MC线圈最大形变量的关系, 每个MC线圈的形变扰动均受到载流面的限制
Fig. 7. The amplitudes of error fields as a function of dmax of each MC in n/m = 2/4 (a), 2/5(b) and 2/6(c) island chains, the deformations of each MC are without perturbations of CCS.
图 9 在n/m = 2/4(a), 2/5(b)和2/6(c)岛链中, 误差场振幅与MC线圈最大形变量的关系. 每个MC线圈的形变扰动均未受到载流面的限制
Fig. 9. The amplitudes of error fields as a function of dmax of each MC in n/m = 2/4 (a), 2/5(b) and 2/6(c) island chains, the deformations of each MC are with perturbations of CCS.
图 8 在n/m = 2/4(a), 2/5(b), 2/6(c)的岛链中, 磁岛宽度变化量的绝对值与MC线圈最大形变量之间的关系, 每个MC线圈的形变扰动均未受到载流面的限制
Fig. 8. The absolute value of the magnetic island width as a function of dmax of each MC in n/m = 2/4 (a), 2/5(b) and 2/6(c) island chains. The deformations of each MC are with perturbations of CCS.
表 1 CFQS中3种磁岛位形下, 模块化线圈、环向场线圈和极向场线圈的电流设置
Table 1. Coil currents in MCs, TFCs and PFCs for n/m = 2/4, 2/5, and 2/6 magnetic island configurations of CFQS.
磁岛位形(n/m) 2/4 2/5 2/6 线圈电流 IMC/kA MC1 312.5 312.5 406.3 MC2 281.6 MC3 MC4 ITFC/kA TFC_10 –60 –24 0 TFC_32 –90 –36 TFC_70 IPFC/kA PFC_OV 0 0 –82 PFC_IV 41 
下载: 导出CSV
-
[1] Rummel T, Risse K, Viebke H, Braeuer T, Kisslinger J 2004 IEEE Trans. Appl. Supercond. 14 1394
Google Scholar
[2] Xiong G Z, Xu Y H, Isobe M, Shimizu A, Ogawa K, Kinoshita S, Liu H F, Wang X Q, Cheng J, Liu H, Huang J, Zhang X, Zhang Y C, Yin D P, Wang A Z, Okamura S, Tang C J 2023 Plasma Phys. Control. Fusion 65 035020
Google Scholar
[3] Shoji M, Shimizu A, Kinoshita S, Okamura S, Xu Y H, Liu H F 2023 Plasma Fusion Res. 18 2405026
Google Scholar
[4] Boozer A H 2005 Rev. Mod. Phys. 76 1071
Google Scholar
[5] Waelbroeck F L 2009 Nucl. Fusion 49 104025
Google Scholar
[6] Lazerson S A, Bozhenkov S, Israeli B, Otte M, Niemann H, Bykov V, Endler M, Andreeva T, Ali A, Drewelow P, Jakubowski M, Sitjes A P, Pisano F, Cannas B, W7-X Team 2018 Plasma Phys. Control. Fusion 60 124002
Google Scholar
[7] Kißlinger J, Andreevab T 2005 Fusion Eng. Des. 74 623
Google Scholar
[8] Andreeva T, Bräuer T, Endler M, Kißlinger J, Toussaint U V 2009 Fusion Eng. Des. 84 408
Google Scholar
[9] Yamazaki K, Yanagi N, Ji H, Kaneko H, Ohyabu N, Satow T, Morimoto S, Yamamoto J, Motojima O, the LHD Design Group 1993 Fusion Eng. Des. 20 79
Google Scholar
[10] Strykowsky R L, Brown T, Chrzanowski J, Cole M, Heitzenroeder P, Neilson G H, Rej D, Viol M 2009 23rd IEEE/NPSS Symposium on Fusion Engineering San Diego, CA, USA, June 01-05, 2009 p1
[11] Brooks A, Reiersen W 2003 20th IEEE/NPSS Symposium on Fusion Engineering San Diego, CA, USA, 14-17 October, 2003 p553
[12] Nührenberg J, Sindoni E, Lotz W, Troyon F, Gori S, Vaclavik J 1994 Proceedings of the Joint Varenna Lausanne International Workshop on Theory of Fusion Plasmas Varenna, Italy, August 22-26, 1994 p3
[13] Garabedian P 1996 Phys. Plasmas 3 2483
Google Scholar
[14] Huang J, Nakata M, Xu Y H, Shimizu A, Isobe M, Okamura S, Liu H F, Wang X Q, Zhang X, Liu H, Cheng J, Tang C J 2022 Phys. Plasmas 29 052505
Google Scholar
[15] Xu Y H, Liu H F, Xiong G Z, Shimizu A, Kinoshita S, Isobe M, Okamura S, Nakata M, Yin D, Wan Y, Wilfred, Cooper A, Zhu C X, Liu H, Zhang X, Huang J, Wang X Q, Tang C J 2018 27th IAEA Fusion Energy Conference Ahmedabad, India, October 22-27, 2018 p5
[16] Isobe M, Shimizu A, Liu H F, Liu H, Xiong G Z, Yin D P, Ogawa K, Yoshimura Y, Nakata M, Kinoshita S, Okamura S, Tang C J, Xu Y H, the CFQS Team 2019 Plasma Fusion Res. 14 3402074
Google Scholar
[17] Wang X Q, Xu Y H, Shimizu A, Isobe M, Okamura S, Todo Y, Wang H, Liu H F, Huang J, Zhang X 2021 Nucl. Fusion 61 036021
Google Scholar
[18] 黄捷, 李沫杉, 覃程, 王先驱 2022 71 185202
Google Scholar
Huang J, Li M S, Qin C, Wang X Q 2022 Acta Phys. Sin. 71 185202
Google Scholar
[19] 苏祥, 王先驱, 符添, 许宇鸿 2023 72 215205
Google Scholar
Su X, Wang X Q, Fu T, Xu Y H 2023 Acta Phys. Sin. 72 215205
Google Scholar
[20] Zhu C X, Gates D A, Hudson S R, Liu H F, Xu Y H, Shimizu A, Okamura S 2019 Nucl. Fusion 59 126007
Google Scholar
[21] Shimizu A, Liu H F, Kinoshita S, Isobe M, Okamura S, Ogawa K, Nakata M, Satake S, Suzuki C, Xiong G Z, Xu Y H, Liu H, Zhang X, Huang J, Wang X Q, Tang C J, Yin D P, Wan Y, the CFQS Team 2019 Plasma Fusion Res. 14 3403151
Google Scholar
[22] Zhu C X, Hudson S R, Song Y T, Wan Y X 2018 Plasma Phys. Control. Fusion 60 065008
Google Scholar
[23] Zhu C X, Hudson S R, Lazerson S A, Song Y T, Wan Y X 2018 Plasma Phys. Control. Fusion 60 054016
Google Scholar
[24] Okamura S, Liu H F, Shimizu A, Kinoshita S, Isobe M, Xiong G Z, Xu Y H 2020 J. Plasma Phys. 86 815860402
Google Scholar
[25] Shimizu A, Kinoshita S, Isobe M, Okamura S, Ogawa K, Nakata M, Yoshimura Y, Suzuki C, Osakabe M, Murase T, Nakagawa S, Tanoue H, Xu Y H, Liu H F, Liu H, Huang J, Wang X Q, Cheng J, Xiong G Z, Tang C J, Yin D P, Wan Y 2022 Nucl. Fusion 62 016010
Google Scholar
[26] Liu H F, Shimizu A, Xu Y H, Okamura S, Kinoshita S, Isobe M, Li Y B, Xiong G Z, Wang X Q, Huang J, Cheng J, Liu H, Zhang X, Yin D P, Wang Y, Murase T, Nakagawa S, Tang C J 2021 Nucl. Fusion 61 016014
Google Scholar
[27] Li Y B, Liu H F, Xu Y H, Shimizu A, Kinoshita S, Okamura S, Isobe X, Xiong G Z, Luo Y, Cheng J, Liu H, Wang X Q, Huang J, Zhang X, Yin D P, Wan Y, Tang C J 2020 Plasma Phys. Control. Fusion 62 125004
Google Scholar
[28] Liu H F, Zhang J, Xu Y H, Shimizu A, Cooper W A, Okamura S, Isobe M, Wang X Q, Huang J, Cheng J, Liu H, Zhang X, Tang C J 2023 Nucl. Fusion 63 026018
Google Scholar
[29] Kinoshita S, Shimizu A, Okamura S, Isobe M, Xiong G Z, Liu H F, Xu Y H, the CQFS Team 2019 Plasma Fusion Res. 14 3405097
Google Scholar
[30] Liu H F, Shimizu A, Isobe m, Okamura S, Nishimura s, Suzuki C, Xu Y H, Zhang X, Liu B , Huang J, Wang X Q, Liu H, Tang C J, Yin D P, Wan Y, the CFQS Team 2018 Plasma Fusion Res. 13 3405067
[31] Boozer A H 2015 Nucl. Fusion 55 025001
Google Scholar
[32] Pedersen T S, Otte M, Lazerson S, Helander P, Bozhenkov S, Biedermann C, Klinger T, Wolf R C, Bosch F S, the Wendelstein 7-X Team 2016 Nat. Commun. 7 13493
Google Scholar
[33] Merkel P 1987 Nucl. Fusion 27 867
Google Scholar
[34] Zhu C X, Hudson S R, Song Y T, Wan Y X 2018 Nucl. Fusion 58 016008
Google Scholar
[35] Drevlak M 1998 Fusion Technol. 33 106
Google Scholar
下载:
计量
- 文章访问数: 414
- PDF下载量: 3
- 被引次数: 0
