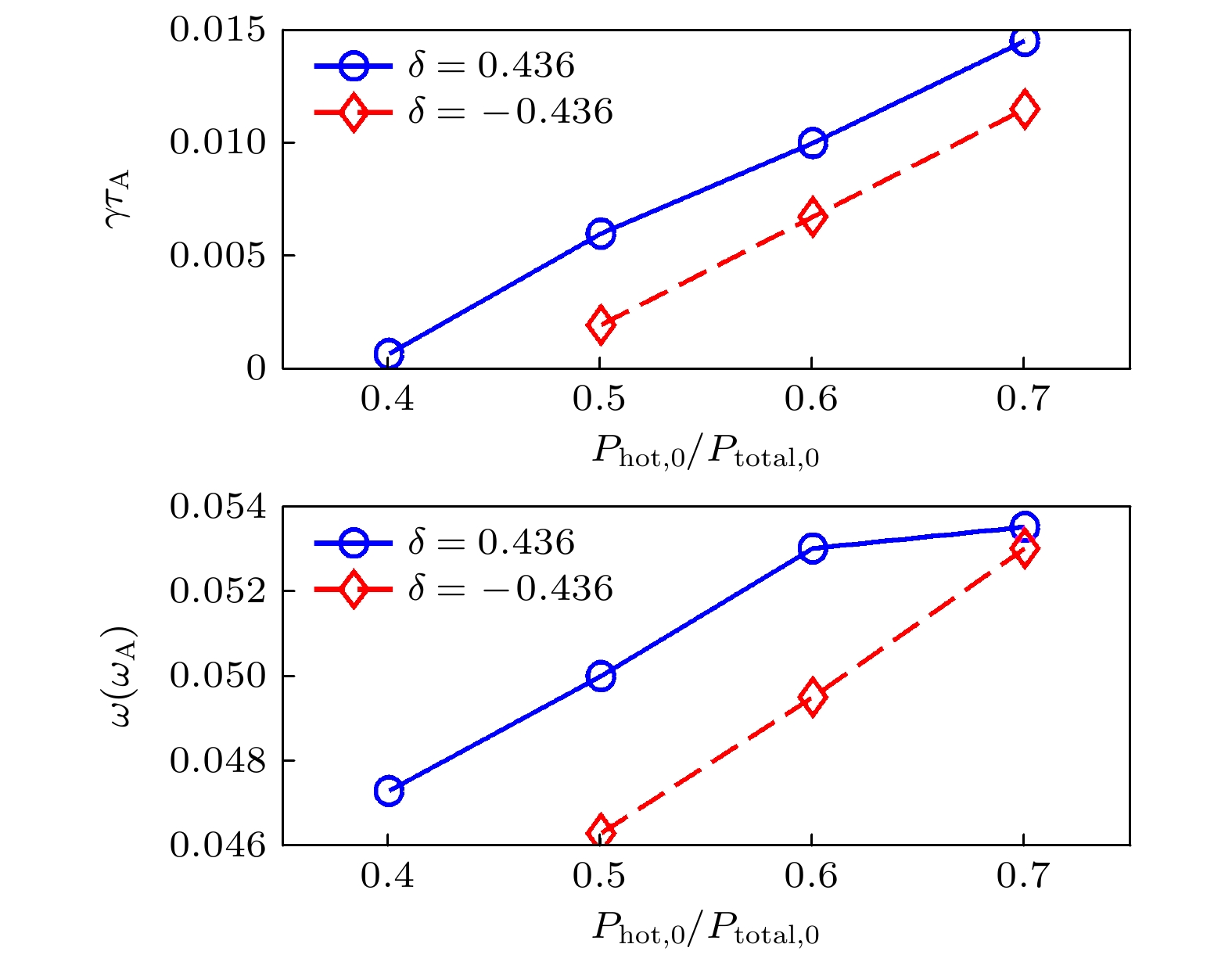
-
负三角形变位形下的托卡马克具有更低的湍流输运和更好的能量约束, 被认为是未来聚变堆一个更好的选择. 为了探索负三角形变位形下高能量粒子激发不稳定性的特征, 使用动理学-磁流体混合模型程序M3D-K开展了此位形下高能量离子激发鱼骨模的线性不稳定性和非线性演化的模拟研究. 基于类EAST参数条件, 模拟发现负三角形变解稳理想内扭曲模不稳定性, 但会致稳鱼骨模不稳定性. 非线性模拟发现在没有磁流体非线性效应时, 负三角形变位形下的鱼骨模更不容易饱和, 可能的解释是相比于正三角形变位形, 在负三角形变位形下的高能量离子轨道更接近与芯部, 因而更容易驱动鱼骨模不稳定性. 这些结果表明考虑高能量粒子激发的鱼骨模不稳定性后, 负三角形变位形相比于正三角形变位形并没有明显优势.The discharges with negative triangularity have lower turbulence induced transport and better energy confinement, so the tokamak with negative triangularity is recognized to be a better choice for future fusion device. In order to explore the features of the energetic particle driven instabilities with negative triangularity, the kinetic-magnetohydrodynamic hybrid code M3D-K is used to investigate the linear instability and nonlinear evolution of the fishbone driven by energetic ions with different triangularity. Based on EAST-like parameters, it is found that the negative triangularity destabilizes the ideal internal kink mode, but stabilizes the fishbone instability. Nonlinear simulations show that the fishbone instability with negative triangularity is hard to saturate without fluid nonlinearity. The possible explanation is that the orbits of fast ions are located more centrally with negative triagularity, so the energy exchange between energetic ions and the fishbone is more efficient than that with positive triangularity. These simulation results demonstrate that the negative triangularity does not have an obvious advantage over the positive triangularity, with the fishbone driven by energetic particles considered.
-
Keywords:
- negative triangularity /
- fast ions /
- fishbone instability
[1] Hofmann F, Sauter O, Reimerdes H, et al. 1998 Phys. Rev. Lett. 81 2918
Google Scholar
[2] Camenen Y, Pochelon A, Behn R, et al. 2007 Nucl. Fusion 47 510
Google Scholar
[3] Solomon W M, Snyder P B, Burrell K H, et al. 2014 Phys. Rev. Lett. 113 135001
Google Scholar
[4] Snyder P B, Solomon W M, Burrell K H, et al. 2015 Nucl. Fusion 55 083026
Google Scholar
[5] Reimerdes H, Pochelon A, Sauter O, et al. 2000 Plasma Phys. Control. Fusion 42 629
Google Scholar
[6] Marinoni A, Brunner S, Camenen Y, et al. 2009 Plasma Phys. Control. Fusion 51 055016
Google Scholar
[7] Austin M E, Marinoni A, Walker M L, et al. 2019 Phys. Rev. Lett. 122 115001
Google Scholar
[8] Medvedev S, Kikuchi M, Villard L, et al. 2015 Nucl. Fusion 55 063013
Google Scholar
[9] Chen W, Wang Z X 2020 Chin. Phys. Lett. 37 125001
Google Scholar
[10] McGuire K, Goldston R, Bell M, et al. 1983 Phys. Rev. Lett. 50 891
Google Scholar
[11] Chen L, White R B, Rosenbluth M N 1984 Phys. Rev. Lett. 52 1122
Google Scholar
[12] Coppi B, Porcelli F 1986 Phys. Rev. Lett. 57 2272
Google Scholar
[13] Heidbrink W W, Bol K, Buchenauer D, et al. 1986 Phys. Rev. Lett. 57 835
Google Scholar
[14] Heidbrink W W, Sager G 1990 Nucl. Fusion 30 1015
Google Scholar
[15] Nave M F F, Campbell D J, Joffrin E, et al. 1991 Nucl. Fusion 31 697
Google Scholar
[16] von Goeler S, Roquemore A L, Johnson L C, et al. 1996 Rev. Sci. Instrum 67 473
Google Scholar
[17] Kass T, Bosch H S, Hoenen F, et al. 1998 Nucl. Fusion 38 807
Google Scholar
[18] Chen W, Ding X T, Liu Y, et al. 2010 Nucl. Fusion 50 084008
Google Scholar
[19] Xu L Q, Zhang J Z, Chen K Y, et al. 2015 Phys. Plasmas 22 122510
[20] Shi P W, Chen W, Duan X R 2021 Chin. Phys. Lett. 38 035202
Google Scholar
[21] Van Zeeland M A, Collins C S, Heidbrink W W, et al. 2019 Nucl. Fusion 59 086028
Google Scholar
[22] Park W, Belova E V, Fu G Y, et al. 1999 Phys. Plasmas 6 1796
Google Scholar
[23] Fu G Y, Park W, Strauss H R, et al. 2006 Phys. Plasmas 13 052517
Google Scholar
[24] Lang J Y, Fu G Y, Chen Y 2010 Phys. Plasmas 17 042309
Google Scholar
[25] Cai H S, Fu G Y 2012 Phys. Plasmas 19 072506
Google Scholar
[26] Shen W, Fu G Y, Sheng Z M, et al. 2014 Phys. Plasmas 21 092514
Google Scholar
[27] Shen W, Wang F, Fu G Y, et al. 2017 Nucl. Fusion 57 116035
Google Scholar
[28] Wang F, Fu G Y, Shen W 2017 Nucl. Fusion 57 016034
Google Scholar
[29] Wang F, Yu L M, Fu G Y, et al. 2017 Nucl. Fusion 57 056013
Google Scholar
[30] Ren Z Z, Wang F, Fu G Y, et al. 2017 Phys. Plasmas 24 052501
Google Scholar
[31] Ren Z Z, Fu G Y, Van Zeeland M A, et al. 2018 Phys. Plasmas 25 122504
Google Scholar
[32] Shen W, Porcelli F 2018 Nucl. Fusion 58 106035
Google Scholar
[33] Chen W, Zhu X L, Wang F, et al. 2019 Nucl. Fusion 59 096037
Google Scholar
[34] Shen W, Wang F, Fu G Y, et al. 2020 Nucl. Fusion 60 106016
Google Scholar
[35] Xu L Q, Shen W, Ren Z Z, et al. 2021 Nucl. Fusion 61 076005
Google Scholar
[36] Ren Z Z, Shen W, Li G Q, et al. 2022 AIP Advances 12 075318
Google Scholar
[37] Porcelli F 1991 Plasma Phys. Control. Fusion 33 1601
Google Scholar
[38] Wang X Q, Wang X G 2016 Nucl. Fusion 56 036024
Google Scholar
[39] Wang X Q, Wang X G 2017 Nucl. Fusion 57 016039
Google Scholar
[40] Eriksson H G, Wahlberg C 2002 Phys. Plasmas 9 1606
Google Scholar
[41] Martynov A, Graves J P, Sauter O 2005 Plasma Phys. Control. Fusion 47 1743
Google Scholar
[42] Bussac M N, Pellat R, Edery D, et al. 1975 Phys. Rev. Lett. 35 1638
Google Scholar
-
图 2 不同三角形变下模频率和线性增长率与快离子压强比值
$ P_{{\rm{hot}}, 0}/P_{{\rm{total}}, 0} $ 的关系Fig. 2. Mode frequency and linear growth rate as a function of the fast ion pressure fraction
$ P_{{\rm{hot}}, 0}/P_{{\rm{total}}, 0} $ .
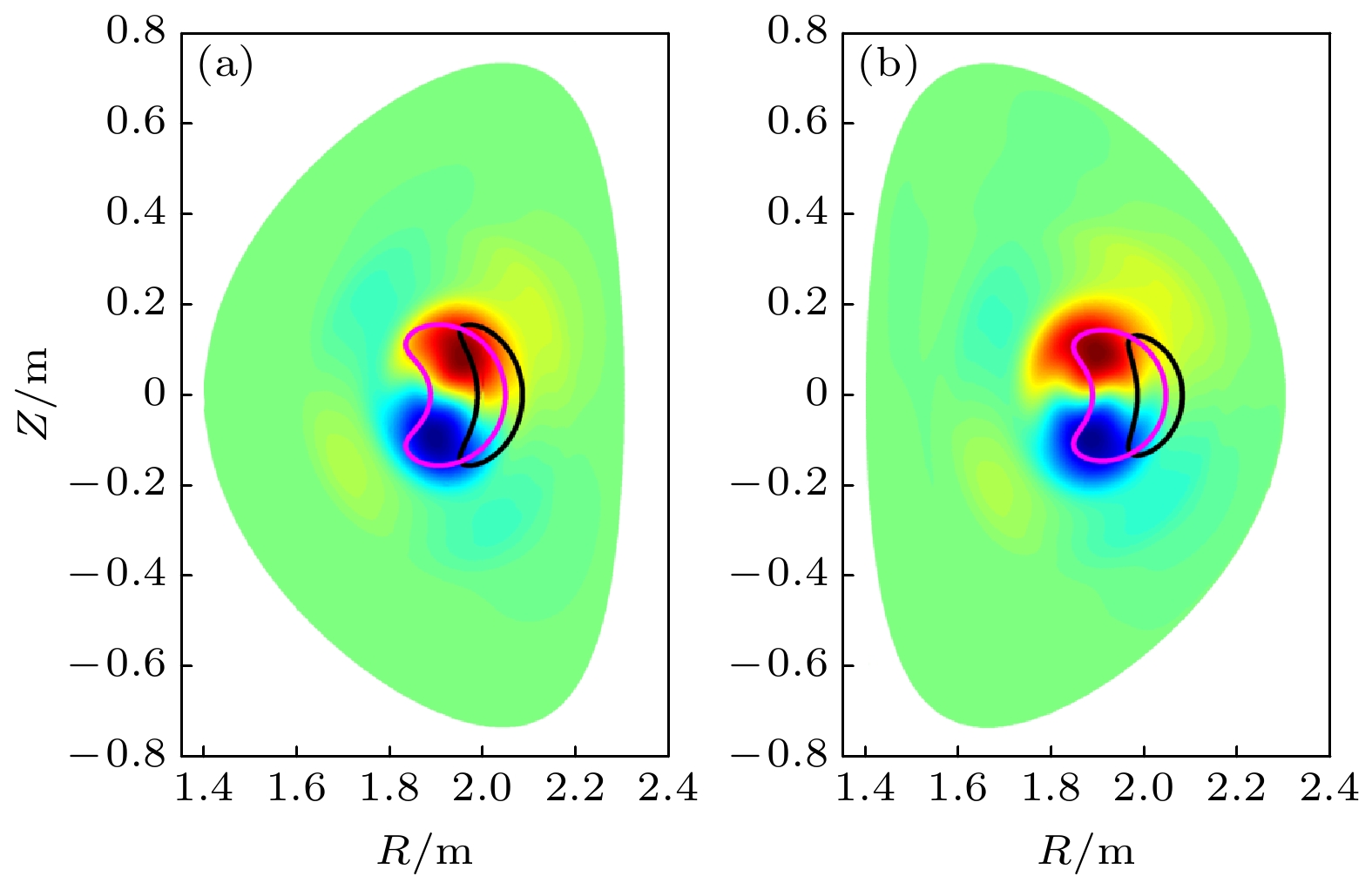
图 3 不同高能量离子压强比值
$ P_{{\rm{hot}}, 0}/P_{{\rm{total}}, 0} $ 下的流函数$ U $ (a)$ \delta = - 0.436 $ ,$ P_{{\rm{hot}}, 0}/P_{{\rm{total}}, 0} = 0 $ ; (b)$ \delta = - 0.436 $ ,$ P_{{\rm{hot}}, 0}/P_{{\rm{total}}, 0} = 0.15 $ ; (c)$ \delta = - 0.436 $ ,$ P_{{\rm{hot}}, 0}/P_{{\rm{total}}, 0} = $ $ 0.4 $ ; (d)$ \delta=0.436 $ ,$ P_{{\rm{hot}}, 0}/P_{{\rm{total}}, 0} = 0.4 $ Fig. 3. Velocity stream function
$ U $ at different fast ion pressure fraction$ P_{{\rm{hot}}, 0}/P_{{\rm{total}}, 0} $ : (a)$ \delta = - 0.436 $ ,$ P_{{\rm{hot}}, 0}/ P_{{\rm{total}}, 0} = $ $ 0 $ ; (b)$ \delta = - 0.436 $ ,$ P_{{\rm{hot}}, 0}/P_{{\rm{total}}, 0} = 0.15 $ ; (c)$ \delta = - 0.436 $ ,$ P_{{\rm{hot}}, 0}/P_{{\rm{total}}, 0} = 0.4 $ ; (d)$ \delta = 0.436 $ ,$ P_{{\rm{hot}}, 0}/ P_{{\rm{total}}, 0} $ $ = 0.4 $ .
图 4 芯部压强剖面平坦下模频率和线性增长率与快离子压强比值
$ P_{{\rm{hot}}, 0}/P_{{\rm{total}}, 0} $ 的关系Fig. 4. Mode frequency and linear growth rate as a function of the fast ion pressure fraction
$ P_{{\rm{hot}}, 0}/P_{{\rm{total}}, 0} $ with flat pressure profile.
图 5 没有磁流体非线性效应的鱼骨模非线性演化 (a) 动能的
$ n = 1 $ 分量的演化; (b) 模频率演化Fig. 5. Time evolution of the fishbone without fluid nonlinearity: (a)
$ n = 1 $ kinetic energy; (b) mode frequency.
图 6 动能和磁能的
$ n = 1 $ 分量随时间演化Fig. 6. Time evolutions of
$ n = 1 $ kinetic energy and magnetic energy.
图 7 不同三角形变参数下的鱼骨模动能的
$ n = 1 $ 分量的演化 (a)$ \beta_{{\rm{total}}, 0} = $ $ 4.61 {\text{%}}$ ; (b)$ \beta_{{\rm{total}}, 0} = 3.91{\text{%}} $ Fig. 7. The
$ n = 1 $ kinetic energy evolution of the fishbone with different triangularity: (a)$ \beta_{{\rm{total}}, 0} = 4.61{\text{%}} $ ; (b)$ \beta_{{\rm{total}}, 0} = $ $ 3.91{\text{%}} $ .
图 8 不同三角形变位形下的捕获高能量离子轨道 (a)
$ \delta = $ $ - 0.436 $ ,$ \beta_{{\rm{total}}, 0} = 3.91{\text{%}}$ ; (b)$ \delta = 0.436 $ ,$ \beta_{{\rm{total}}, 0} = 4.61{\text{%}} $ Fig. 8. Orbits of trapped fast ions with different triangularity: (a)
$ \delta = - 0.436 $ ,$ \beta_{{\rm{total}}, 0} = 3.91{\text{%}} $ ; (b)$ \delta = 0.436 $ ,$ \beta_{{\rm{total}}, 0} = $ $ 4.61{\text{%}} $ . -
[1] Hofmann F, Sauter O, Reimerdes H, et al. 1998 Phys. Rev. Lett. 81 2918
Google Scholar
[2] Camenen Y, Pochelon A, Behn R, et al. 2007 Nucl. Fusion 47 510
Google Scholar
[3] Solomon W M, Snyder P B, Burrell K H, et al. 2014 Phys. Rev. Lett. 113 135001
Google Scholar
[4] Snyder P B, Solomon W M, Burrell K H, et al. 2015 Nucl. Fusion 55 083026
Google Scholar
[5] Reimerdes H, Pochelon A, Sauter O, et al. 2000 Plasma Phys. Control. Fusion 42 629
Google Scholar
[6] Marinoni A, Brunner S, Camenen Y, et al. 2009 Plasma Phys. Control. Fusion 51 055016
Google Scholar
[7] Austin M E, Marinoni A, Walker M L, et al. 2019 Phys. Rev. Lett. 122 115001
Google Scholar
[8] Medvedev S, Kikuchi M, Villard L, et al. 2015 Nucl. Fusion 55 063013
Google Scholar
[9] Chen W, Wang Z X 2020 Chin. Phys. Lett. 37 125001
Google Scholar
[10] McGuire K, Goldston R, Bell M, et al. 1983 Phys. Rev. Lett. 50 891
Google Scholar
[11] Chen L, White R B, Rosenbluth M N 1984 Phys. Rev. Lett. 52 1122
Google Scholar
[12] Coppi B, Porcelli F 1986 Phys. Rev. Lett. 57 2272
Google Scholar
[13] Heidbrink W W, Bol K, Buchenauer D, et al. 1986 Phys. Rev. Lett. 57 835
Google Scholar
[14] Heidbrink W W, Sager G 1990 Nucl. Fusion 30 1015
Google Scholar
[15] Nave M F F, Campbell D J, Joffrin E, et al. 1991 Nucl. Fusion 31 697
Google Scholar
[16] von Goeler S, Roquemore A L, Johnson L C, et al. 1996 Rev. Sci. Instrum 67 473
Google Scholar
[17] Kass T, Bosch H S, Hoenen F, et al. 1998 Nucl. Fusion 38 807
Google Scholar
[18] Chen W, Ding X T, Liu Y, et al. 2010 Nucl. Fusion 50 084008
Google Scholar
[19] Xu L Q, Zhang J Z, Chen K Y, et al. 2015 Phys. Plasmas 22 122510
[20] Shi P W, Chen W, Duan X R 2021 Chin. Phys. Lett. 38 035202
Google Scholar
[21] Van Zeeland M A, Collins C S, Heidbrink W W, et al. 2019 Nucl. Fusion 59 086028
Google Scholar
[22] Park W, Belova E V, Fu G Y, et al. 1999 Phys. Plasmas 6 1796
Google Scholar
[23] Fu G Y, Park W, Strauss H R, et al. 2006 Phys. Plasmas 13 052517
Google Scholar
[24] Lang J Y, Fu G Y, Chen Y 2010 Phys. Plasmas 17 042309
Google Scholar
[25] Cai H S, Fu G Y 2012 Phys. Plasmas 19 072506
Google Scholar
[26] Shen W, Fu G Y, Sheng Z M, et al. 2014 Phys. Plasmas 21 092514
Google Scholar
[27] Shen W, Wang F, Fu G Y, et al. 2017 Nucl. Fusion 57 116035
Google Scholar
[28] Wang F, Fu G Y, Shen W 2017 Nucl. Fusion 57 016034
Google Scholar
[29] Wang F, Yu L M, Fu G Y, et al. 2017 Nucl. Fusion 57 056013
Google Scholar
[30] Ren Z Z, Wang F, Fu G Y, et al. 2017 Phys. Plasmas 24 052501
Google Scholar
[31] Ren Z Z, Fu G Y, Van Zeeland M A, et al. 2018 Phys. Plasmas 25 122504
Google Scholar
[32] Shen W, Porcelli F 2018 Nucl. Fusion 58 106035
Google Scholar
[33] Chen W, Zhu X L, Wang F, et al. 2019 Nucl. Fusion 59 096037
Google Scholar
[34] Shen W, Wang F, Fu G Y, et al. 2020 Nucl. Fusion 60 106016
Google Scholar
[35] Xu L Q, Shen W, Ren Z Z, et al. 2021 Nucl. Fusion 61 076005
Google Scholar
[36] Ren Z Z, Shen W, Li G Q, et al. 2022 AIP Advances 12 075318
Google Scholar
[37] Porcelli F 1991 Plasma Phys. Control. Fusion 33 1601
Google Scholar
[38] Wang X Q, Wang X G 2016 Nucl. Fusion 56 036024
Google Scholar
[39] Wang X Q, Wang X G 2017 Nucl. Fusion 57 016039
Google Scholar
[40] Eriksson H G, Wahlberg C 2002 Phys. Plasmas 9 1606
Google Scholar
[41] Martynov A, Graves J P, Sauter O 2005 Plasma Phys. Control. Fusion 47 1743
Google Scholar
[42] Bussac M N, Pellat R, Edery D, et al. 1975 Phys. Rev. Lett. 35 1638
Google Scholar
下载:
计量
- 文章访问数: 2296
- PDF下载量: 90
- 被引次数: 0
