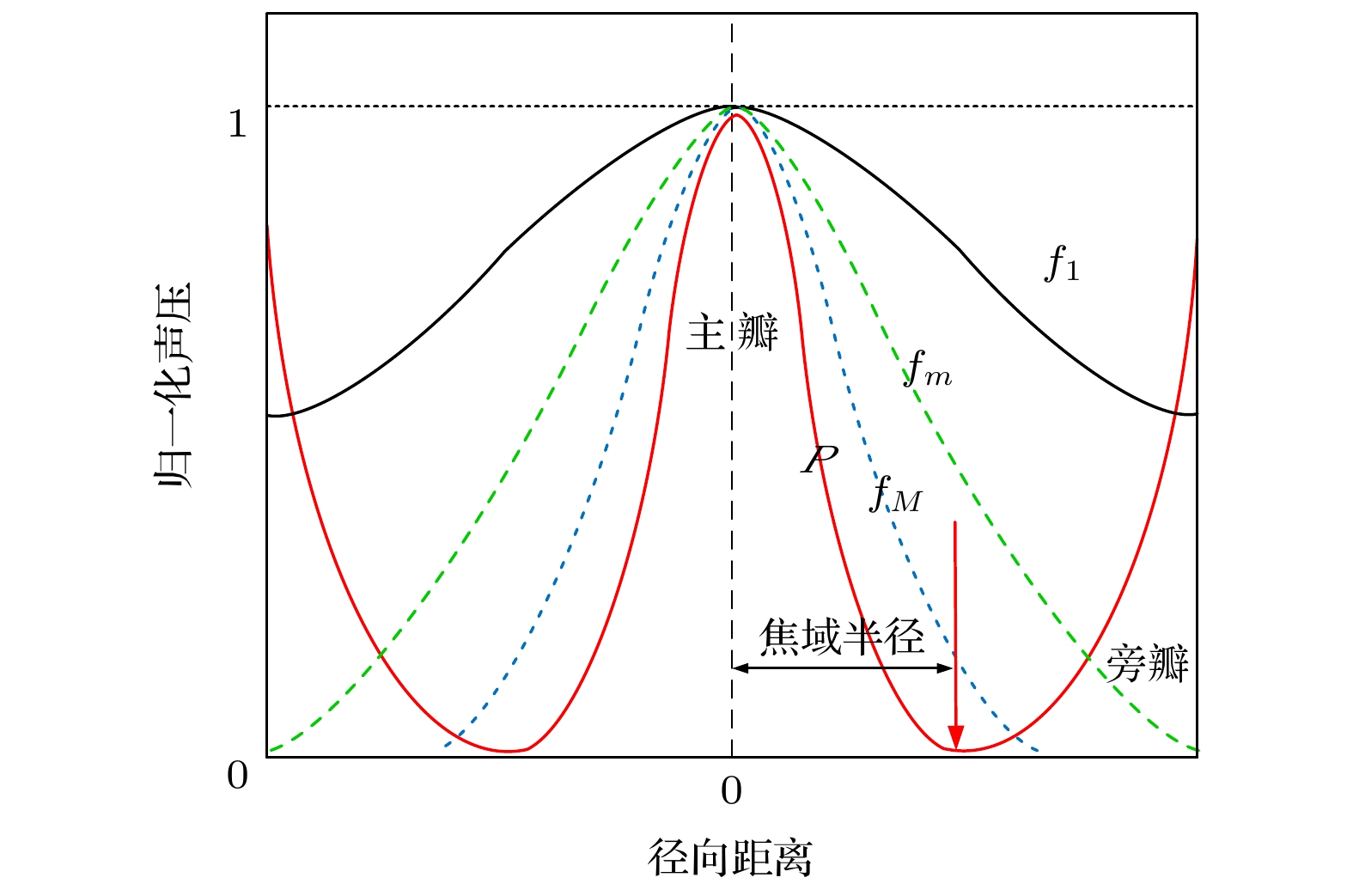
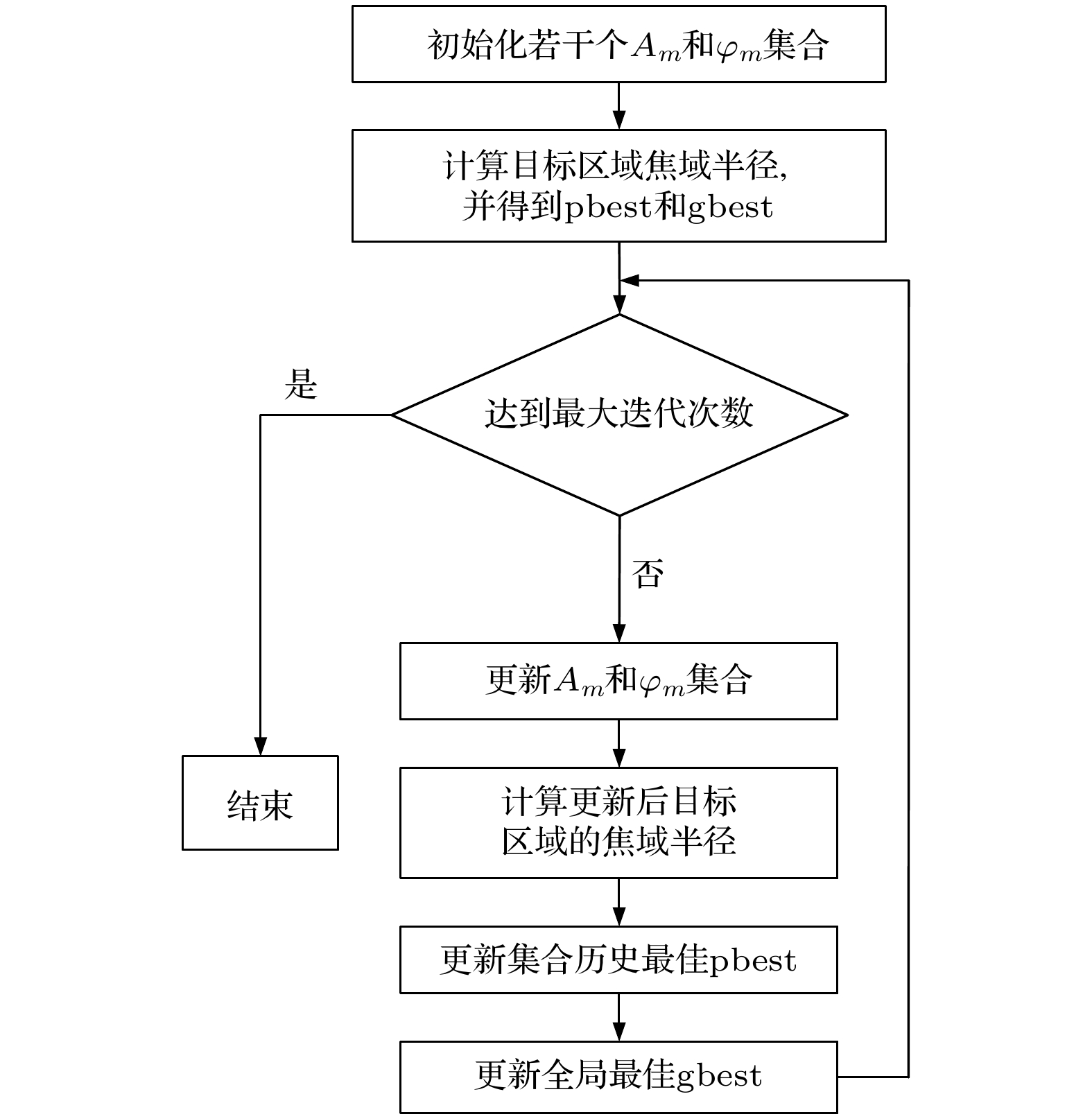
-
针对传统声束的衍射极限问题, 如何构建具有更高分辨率的聚焦声场, 是实现超分辨声成像和声操控领域的重大挑战之一. 本文在考虑成像分辨率同时兼顾声场可控制性, 提出了一种基于粒子群优化算法的多频超振荡超分辨聚焦声场设计方法. 基于常规换能器声场的衍射效应, 利用半波带法设计中心频率菲涅耳透镜, 并以中心频率为基准在换能器带宽范围内设置多频信号来构建超振荡声场, 进一步通过粒子群算法对多频声束的振幅和相位进行优化, 在远场构建了焦域半径能够小于中心频率半波长的超振荡声场, 还发现其尺寸小于最高频率声场的所形成焦域半径, 进一步证明其焦域半径随着中心频率和超振荡频率数的增大而减小. 研究结果为可控超分辨声聚焦提供了一种简便易行的方法.The spatial resolution of conventional waves is restricted by the diffraction limit of half wavelength. Hence, how to construct super-resolution acoustic beams with a smaller focal radius is one of the major challenges in recent studies. In the present paper, the super-resolution acoustic focusing method is proposed based on the superposition of multi-frequency super-oscillation beams and the Particle Swarm Optimization (PSO), which can improve the spatial resolution concurrently with good controllability. Based on the diffraction effect of traditional ultrasound fields, the acoustic lens of Fresnel zone plane (FZP) at the center frequency is designed by the half-wave zone method. Multiple acoustic beams at several preset frequencies within the transducer bandwidth are sent out to build the super-oscillation focal area by the pressure superposition. The radius of the super-resolution focal spot constructed by the PSO algorithm with optimized amplitudes and phases is less than the half wavelength at the center frequency, which is even smaller than the focal radius at the highest frequency. Furthermore, the focal radius is also proved to decrease with the increase of the number of multiple frequencies and the center frequency. The favorable results demonstrate the feasibility of super-resolution acoustic focusing based on the PSO of super-oscillation, and provide an applicable strategy for the high-resolution acoustic imaging and manipulation.
-
Keywords:
- super-resolution acoustic focusing /
- super-oscillation /
- particle swarm optimization /
- Fresnel zone plane
[1] Gettle L M, Revzin M V 2020 Radiol. Clin. North. Am. 58 653
Google Scholar
[2] Chen Q Y, Song H J, Yu J, Kim K 2021 Sensors (Basel). 21 2417
Google Scholar
[3] Liu Y L, L Liu J H, Ai K, Yuan Q H, Lu L H 2014 Contrast Media Mol. Imaging 9 26
Google Scholar
[4] Tzourio-Mazoyer N, Landeau B, Papathanassiou D, Crivello F, Etard O, Delcroix N, Mazoyer B, Joliot M 2002 Neuroimage. 15 273
Google Scholar
[5] Boellaard R, O’Doherty M J, Chiti A 2010 Eur. J. Nucl. Med. Mol. Imaging 37 181
Google Scholar
[6] Kim K, Chen Q Y, Yu J 2019 J. Acoust. Soc. Am. 145 1703
Google Scholar
[7] Lin F L, Tsuruta J K, Rojas J D, Dayton P A 2017 Ultrasound Med. Biol. 43 2488
Google Scholar
[8] Soulioti D E, Espindola D, Dayton P A, Pinton G F 2020 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 67 25
Google Scholar
[9] 丁昌林, 董仪宝, 赵晓鹏 2018 67 194301
Google Scholar
Ding C L, Dong Y B, Zhao X P 2018 Acta. Phys. Sin. 67 194301
Google Scholar
[10] Liu Z, Zhang X, Mao Y, Zhu Y Y, Yang Z, Chan C T, Sheng P 2000 Science 289 1734
Google Scholar
[11] Zhu J, Christensen J, Jung J, Martin-Moreno L, Yin X, Fok L, Zhang X, Garcia-Vidal F J 2011 Nat. Phys. 7 52
Google Scholar
[12] Deng K, Ding Y Q, He Z J, Zhao H P, Shi J, Liu Z Y 2009 J. Appl. Phys. 105 124909
Google Scholar
[13] Errico C, Pierre J, Pezet S, Desailly Y, Lenkei Z, Couture O, Tanter M 2015 Nature 527 499
Google Scholar
[14] Yuan B G, Liu J Y, Liu C, Cheng Y, Liu X J 2021 Appl. Acoust. 178 107993
Google Scholar
[15] 董永康, 王培峰, 郁高坤 2018 声学技术 37 146
Dong Y K, Wang P F, Yu G K 2018 Tech. Acoust. 37 146
[16] Berry M V, Popescu S 2006 J. Phys. A: Math. Gen. 39 6965
Google Scholar
[17] Shen Y X, Peng Y G, Cai F Y, Huang K, Zhao D G, Qiu C W, Zheng H R, Zhu X F 2019 Nat. Commun. 10 3411
Google Scholar
[18] Hashimoto H, Tanaka S, Sato K 1991 TRANSDUCERS '91 International Conference on Solid-State Sensors and Actuators San Francisco CA, USA, June 24–27, 1991 p853
[19] Ellens N P K, Lucht B B C, Gunaseelan S T, Hudson J M, Hynynen K H 2015 Phys. Med. Biol. 60 2195
Google Scholar
[20] Yamada K, Shimizu H 1985 IEEE 1985 Ultrasonics Symposium San Francisco, USA, Oct 16–18, 1985 p745
[21] Zhao J J, Ye H P, Huang K, Chen Z N, Li B, Qiu C W 2014 Sci. Rep. 4 6257
Google Scholar
[22] Huang F M, Chen Y F, de Abajo F J G, Zheludev N I 2007 J. Opt. A:Pure. Appl. Opt. 9 S285
Google Scholar
[23] Dennis M R, Hamilton A C, Courtial J 2008 Opt. Lett. 33 2976
Google Scholar
[24] Venkatesh S S, Mishra D 2021 Int. J. Intell. Syst. 30 142
Google Scholar
[25] Sabat S L, Ali L, Udgata S K 2011 Appl. Soft. Comput. 11 574
Google Scholar
[26] Xu G, Liu B B, Song J, Xiao S J, Wu A J 2019 Nat. Comput. 18 313
Google Scholar
[27] Li C X, Wang J J, Ma Z H, Li B, Kang K, Wei L, Zhang W 2020 World J. Surg. Oncol. 18 103
Google Scholar
[28] Zhang J B, Li N, Dong F H, Liang S Y, Wang D, An J, Long Y F, Wang Y X, Luo Y K, Zhang J 2020 J. Ultrasound Med. 39 1507
Google Scholar
-
图 1 基于菲涅耳声透镜的声场聚焦示意图
Fig. 1. Sketch map of the acoustic focusing based on the acoustic lens of Fresnel zone plane.
图 2 基于多频超振荡的超分辨声场焦域的径向声压分布示意图
Fig. 2. Schematic diagram of the radial pressure distributions in the focal plane for the super-resolution acoustic focusing based on the multi-frequency super-oscillation.
图 4 中心频率1.0 MHz的菲涅耳透镜所形成聚焦声场的归一化焦域半径(
$ r/\lambda $ )和焦距(F )的关系Fig. 4. Relationship between the normalized focal radius (
$ r/\lambda $ ) and the focal length (F ) for the Fresnel lens at the center frequency of 1.0 MHz.
图 5 频率0.6—1.4 MHz多频声束经过F = 50 mm的菲涅耳透镜形成聚焦声场的轴向声压剖面分布
Fig. 5. Axial pressure profiles focused by the Fresnel lens (F = 50 mm) for acoustic beams at the frequencies of 0.6–1.4 MHz.
图 6 频率0.6—1.4 MHz多频声束经过F = 40 mm的菲涅耳透镜所形成聚焦声场的轴向声压剖面分布
Fig. 6. Axial pressure profiles focused by the Fresnel lens (F = 40 mm) for acoustic beams at the frequencies of 0.6–1.4 MHz.
图 7 频率0.6—1.4 MHz多频声束经过F = 30 mm的菲涅耳透镜所形成聚焦声场的轴向声压剖面分布
Fig. 7. Axial pressure profiles focused by the Fresnel lens (F = 30 mm) for acoustic beams at the frequencies of 0.6–1.4 MHz.
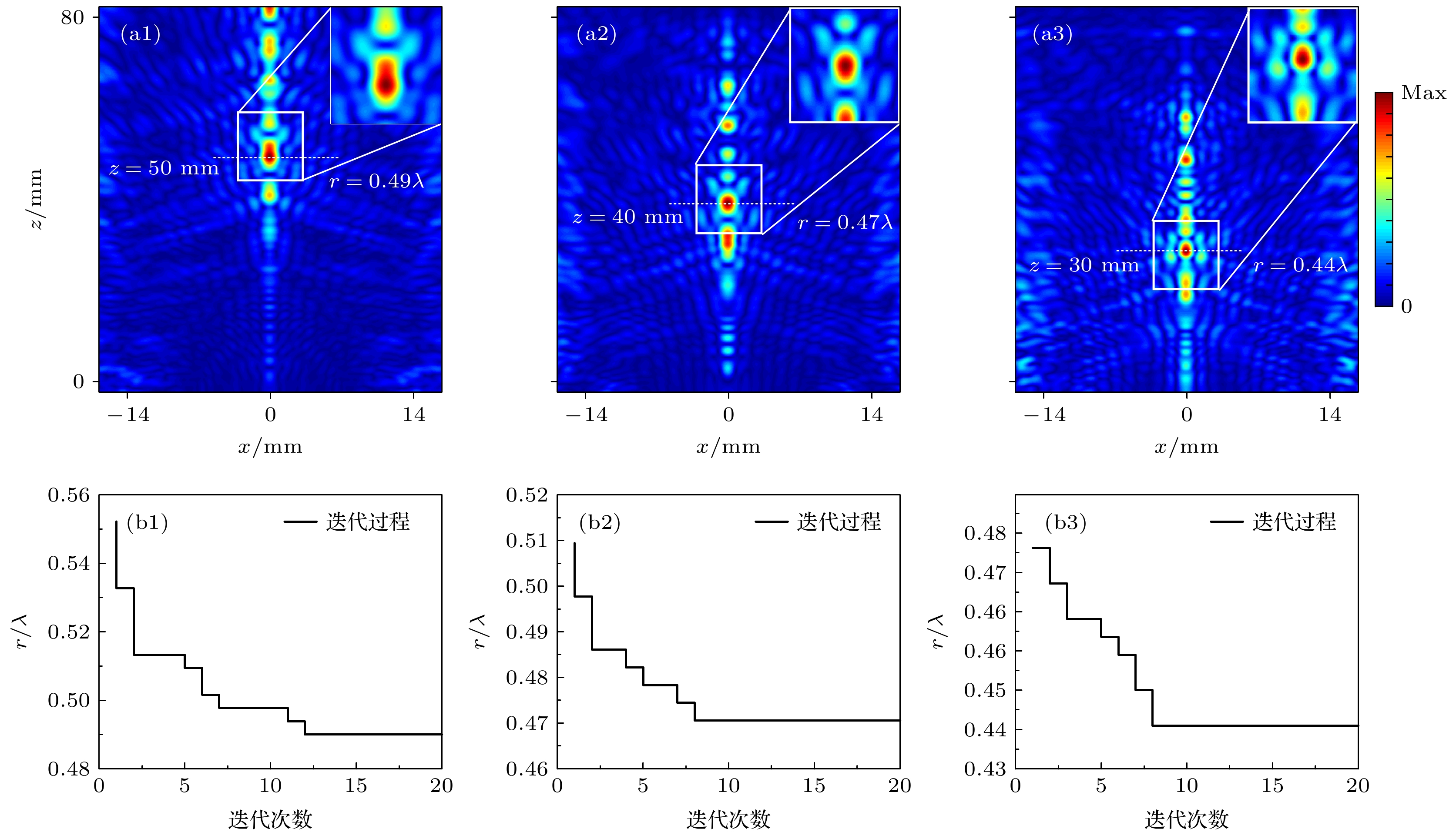
图 8 (a1)—(a3) 多频声束经过F = 50, 40, 30 mm的三种菲涅耳透镜所构建超振荡聚焦声场的轴向剖面声压分布(b1)—(b3)相应的粒子群算法的迭代优化过程
Fig. 8. (a1)–(a3) Axial pressure profiles of super-oscillation super-resolution acoustic fields; (b1)–(b3) the corresponding iteration processes of the PSO for three kinds of Fresnel lenses with F = 50, 40 and 30 mm.
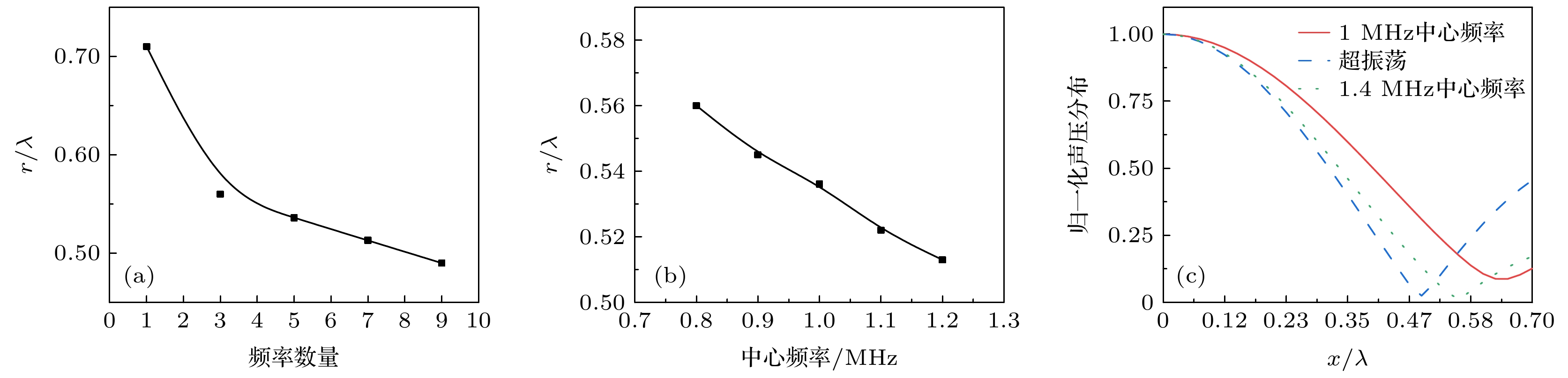
图 9 超振荡声场的焦域半径与(a)多频声束频率数和(b)中心频率的关系, 以及(c)三种聚焦声场焦平面内的径向声压分布
Fig. 9. Distributions of the focal radius of the super-oscillation acoustic field with respect to (a) the number of multiple frequencies and (b) the center frequency, and (c) the radial pressure distributions in the focal plane for three acoustic fields.
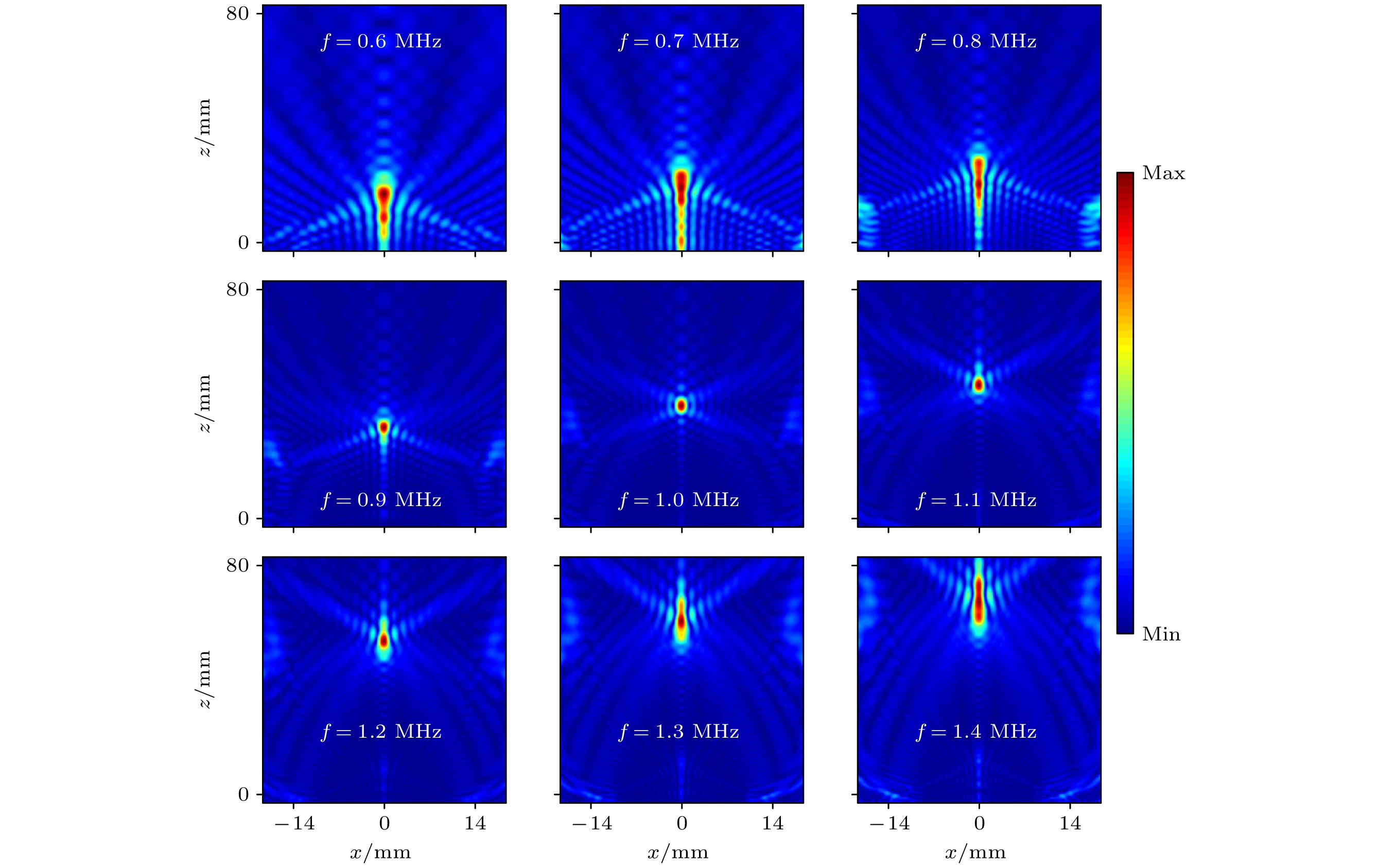
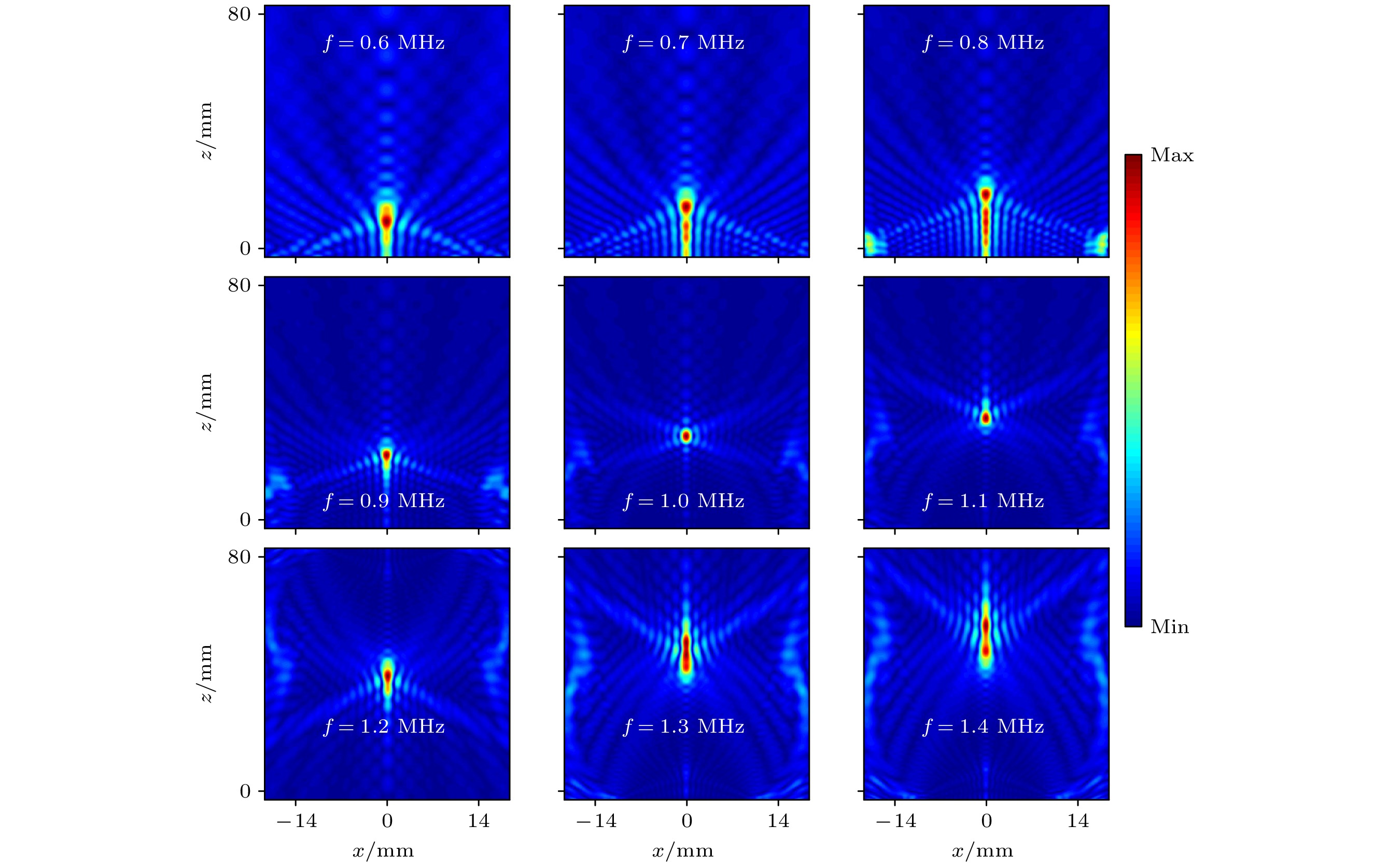
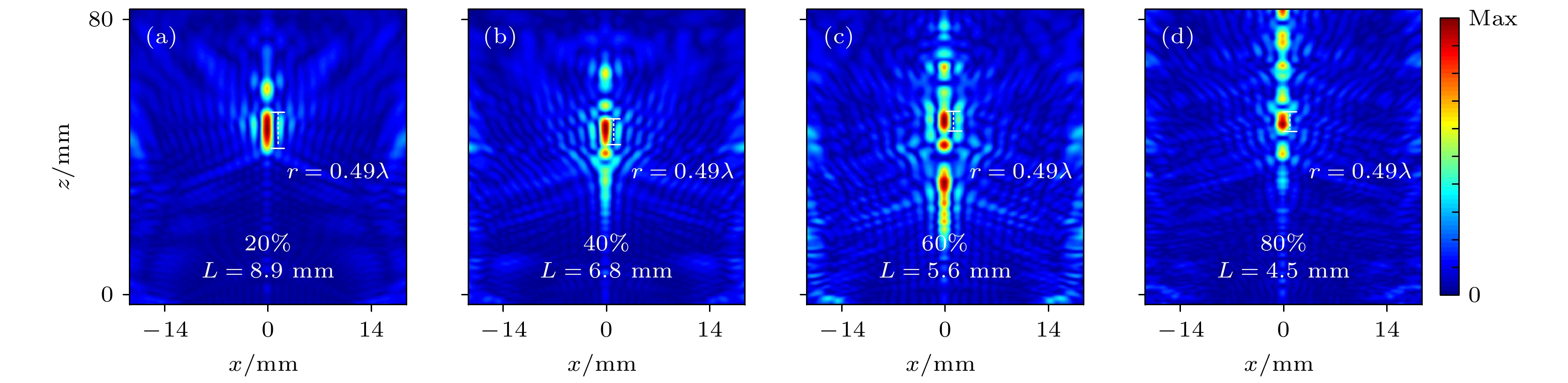
图 10 中心频率1.0 MHz, 相对带宽分别为 (a) 20%, (b) 40%, (c) 60% 和 (d) 80%的多频声束经F = 50 mm的菲涅耳透镜所构建超振荡声场的轴向声压分布
Fig. 10. Axial pressure profiles of super-oscillation acoustic fields formed by the Fresnel lens with F = 50 mm for multi-frequency beams with the relative bandwidths of (a) 20%, (b) 40%, (c) 60%, and (d) 80% at the center frequency of 1.0 MHz.
表 1 粒子群算法优化后所得的参数
Table 1. Parameters optimized by the PSO algorithm.
频率 菲涅耳透镜的焦距 f/MHz 50 mm 40 mm 30 mm 0.6 $\rm 2.68{e^{ - j0.958}} $ $\rm 2.86{e^{j0.834}} $ $\rm 9.38{e^{j{\text{0.396}}}} $ 0.7 $\rm 2.48{e^{ - j2.122}} $ $\rm 1.25{e^{ - j1.45}} $ $\rm 9.13{e^{j{\text{0.706}}}} $ 0.8 $\rm 5.26{e^{ - j2.646}} $ $\rm 1.93{e^{j1.470}} $ $\rm 4.91{e^{j{\text{0.274}}}} $ 0.9 $\rm 6.34{e^{j1.448}} $ $\rm3.54{e^{j0.020}} $ $\rm7.49{e^{j1.366}} $ 1.0 $\rm 5.25{e^{ - j2.652}} $ $\rm 3.27{e^{ - j0.685}} $ $\rm 8.52{e^{ - j{\text{0.465}}}} $ 1.1 $\rm 1.95{e^{ - j0.082}} $ $\rm2.10{e^{ - j1.221}} $ $\rm5.89{e^{j{\text{0.879}}}} $ 1.2 $\rm 4.11{e^{j0.725}} $ $\rm 1.59{e^{ - j1.905}} $ $\rm 0.57{e^{j{\text{0.493}}}} $ 1.3 $\rm 4.82{e^{j2.031}} $ $\rm 2.43{e^{j1.160}} $ $\rm 4.16{e^{j{\text{0.684}}}} $ 1.4 $\rm 5.34{e^{ - j2.808}} $ $\rm 1.67{e^{j1.022}} $ $\rm 6.56{e^{ - j{\text{0.299}}}} $ 
下载: 导出CSV
表 2 不同频率声束和超振荡声场的焦域半径和实际焦距
Table 2. Focal radii and focal lengths for the focused beams at different frequencies and the super-oscillation field.
频率 菲涅耳透镜焦距 f/MHz 50 mm 40 mm 30 mm r/$ \lambda $ F/mm r/$ \lambda $ F/mm r/$ \lambda $ F/mm 0.6 0.91 22.6 0.87 19.5 0.82 12.2 0.7 0.82 28.7 0.79 21.4 0.75 16.9 0.8 0.73 33.9 0.56 22.5 0.69 20.8 0.9 0.72 41.3 0.67 33.2 0.58 24.1 1.0 0.71 50.0 0.65 40.0 0.58 30.0 1.1 0.68 56.9 0.63 46.8 0.56 35.8 1.2 0.67 65.2 0.63 53.3 0.55 41.3 1.3 0.65 72.6 0.60 59.6 0.51 50.5 1.4 0.65 78.9 0.58 65.9 0.50 55.9 超振荡 0.49 49.5 0.47 40.0 0.44 30.0
下载: 导出CSV
表 3 多频声束经不同焦距的菲涅耳透镜后所形成的声场的焦域半径
Table 3. Focal radii for Fresnel lenses with different focal lengths.
菲涅耳透镜焦距 焦平面内的最小焦域半径 F /mm r / λ 20 0.40 30 0.44 40 0.47 50 0.49 60 0.51 70 0.54
下载: 导出CSV
-
[1] Gettle L M, Revzin M V 2020 Radiol. Clin. North. Am. 58 653
Google Scholar
[2] Chen Q Y, Song H J, Yu J, Kim K 2021 Sensors (Basel). 21 2417
Google Scholar
[3] Liu Y L, L Liu J H, Ai K, Yuan Q H, Lu L H 2014 Contrast Media Mol. Imaging 9 26
Google Scholar
[4] Tzourio-Mazoyer N, Landeau B, Papathanassiou D, Crivello F, Etard O, Delcroix N, Mazoyer B, Joliot M 2002 Neuroimage. 15 273
Google Scholar
[5] Boellaard R, O’Doherty M J, Chiti A 2010 Eur. J. Nucl. Med. Mol. Imaging 37 181
Google Scholar
[6] Kim K, Chen Q Y, Yu J 2019 J. Acoust. Soc. Am. 145 1703
Google Scholar
[7] Lin F L, Tsuruta J K, Rojas J D, Dayton P A 2017 Ultrasound Med. Biol. 43 2488
Google Scholar
[8] Soulioti D E, Espindola D, Dayton P A, Pinton G F 2020 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 67 25
Google Scholar
[9] 丁昌林, 董仪宝, 赵晓鹏 2018 67 194301
Google Scholar
Ding C L, Dong Y B, Zhao X P 2018 Acta. Phys. Sin. 67 194301
Google Scholar
[10] Liu Z, Zhang X, Mao Y, Zhu Y Y, Yang Z, Chan C T, Sheng P 2000 Science 289 1734
Google Scholar
[11] Zhu J, Christensen J, Jung J, Martin-Moreno L, Yin X, Fok L, Zhang X, Garcia-Vidal F J 2011 Nat. Phys. 7 52
Google Scholar
[12] Deng K, Ding Y Q, He Z J, Zhao H P, Shi J, Liu Z Y 2009 J. Appl. Phys. 105 124909
Google Scholar
[13] Errico C, Pierre J, Pezet S, Desailly Y, Lenkei Z, Couture O, Tanter M 2015 Nature 527 499
Google Scholar
[14] Yuan B G, Liu J Y, Liu C, Cheng Y, Liu X J 2021 Appl. Acoust. 178 107993
Google Scholar
[15] 董永康, 王培峰, 郁高坤 2018 声学技术 37 146
Dong Y K, Wang P F, Yu G K 2018 Tech. Acoust. 37 146
[16] Berry M V, Popescu S 2006 J. Phys. A: Math. Gen. 39 6965
Google Scholar
[17] Shen Y X, Peng Y G, Cai F Y, Huang K, Zhao D G, Qiu C W, Zheng H R, Zhu X F 2019 Nat. Commun. 10 3411
Google Scholar
[18] Hashimoto H, Tanaka S, Sato K 1991 TRANSDUCERS '91 International Conference on Solid-State Sensors and Actuators San Francisco CA, USA, June 24–27, 1991 p853
[19] Ellens N P K, Lucht B B C, Gunaseelan S T, Hudson J M, Hynynen K H 2015 Phys. Med. Biol. 60 2195
Google Scholar
[20] Yamada K, Shimizu H 1985 IEEE 1985 Ultrasonics Symposium San Francisco, USA, Oct 16–18, 1985 p745
[21] Zhao J J, Ye H P, Huang K, Chen Z N, Li B, Qiu C W 2014 Sci. Rep. 4 6257
Google Scholar
[22] Huang F M, Chen Y F, de Abajo F J G, Zheludev N I 2007 J. Opt. A:Pure. Appl. Opt. 9 S285
Google Scholar
[23] Dennis M R, Hamilton A C, Courtial J 2008 Opt. Lett. 33 2976
Google Scholar
[24] Venkatesh S S, Mishra D 2021 Int. J. Intell. Syst. 30 142
Google Scholar
[25] Sabat S L, Ali L, Udgata S K 2011 Appl. Soft. Comput. 11 574
Google Scholar
[26] Xu G, Liu B B, Song J, Xiao S J, Wu A J 2019 Nat. Comput. 18 313
Google Scholar
[27] Li C X, Wang J J, Ma Z H, Li B, Kang K, Wei L, Zhang W 2020 World J. Surg. Oncol. 18 103
Google Scholar
[28] Zhang J B, Li N, Dong F H, Liang S Y, Wang D, An J, Long Y F, Wang Y X, Luo Y K, Zhang J 2020 J. Ultrasound Med. 39 1507
Google Scholar
下载:
计量
- 文章访问数: 4153
- PDF下载量: 72
- 被引次数: 0
