难熔金属钒熔化行为的局域原子结构模拟与分析

蒋元祺

doi:10.7498/aps.69.20200185

摘要

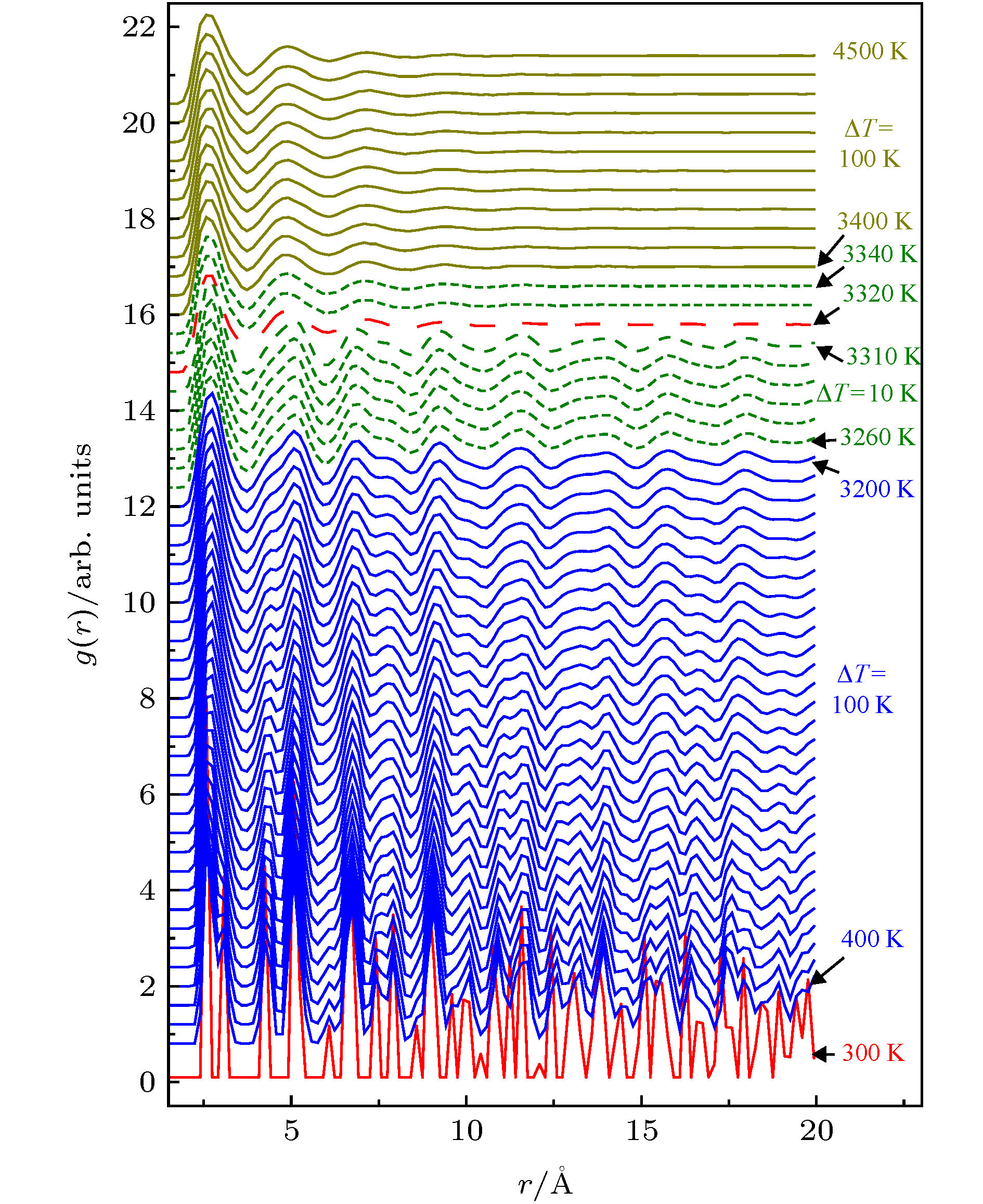
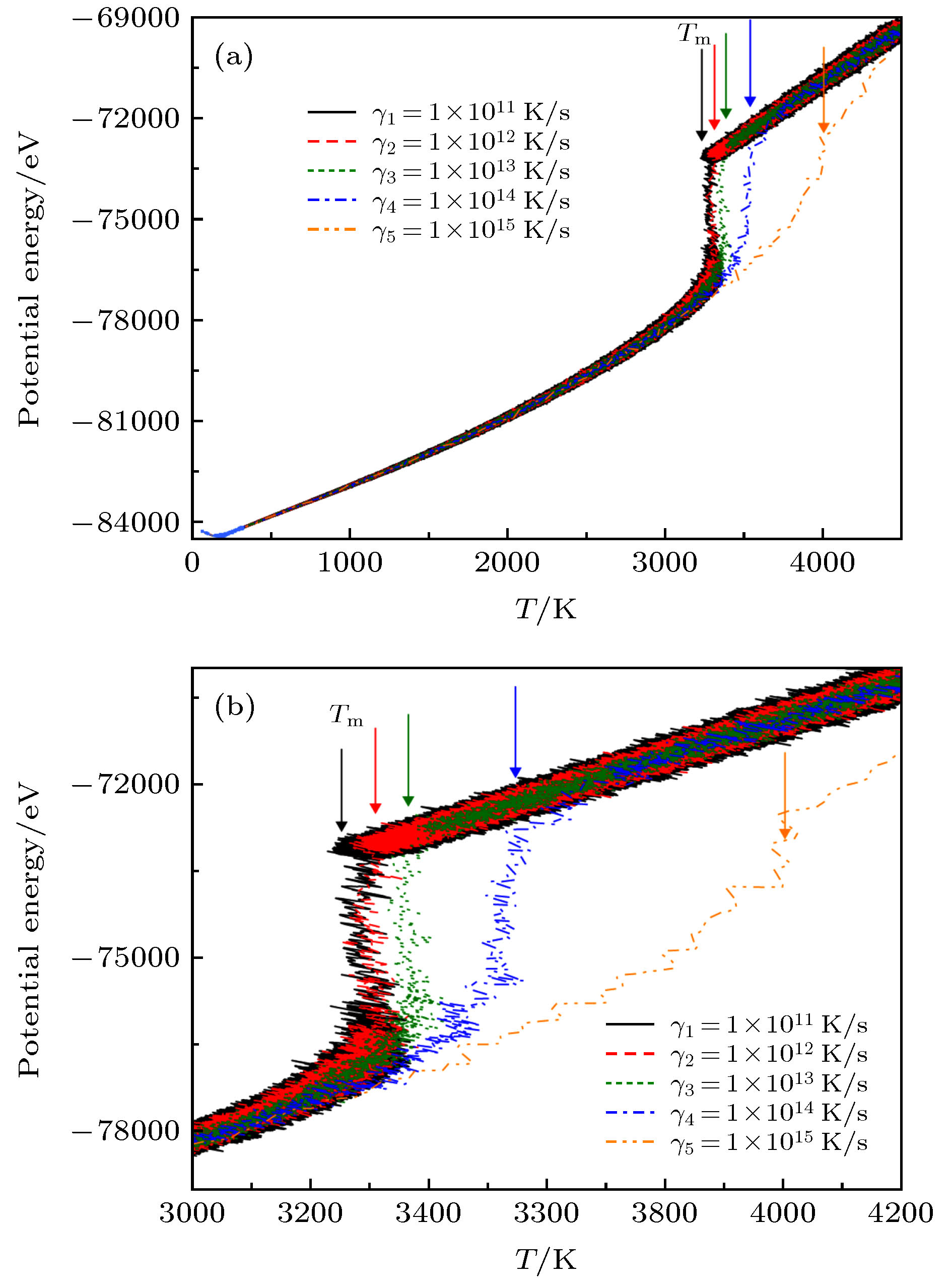
采用经典分子动力学(molecular dynamics, MD)方法, 模拟了16000个钒原子在5种不同熔化速率(γ₁ = 1 × 10¹¹ K/s, γ₂ = 1 × 10¹² K/s, γ₃ = 1 × 10¹³ K/s, γ₄ = 1 × 10¹⁴ K/s 与γ₅ = 1 × 10¹⁵ K/s)下原子结构的熔化行为. 结果表明: 不同熔化速率对难熔金属钒的熔点影响明显, 不过随着温度升高, 体系特征原子结构诸如体心立方(BCC)、六角密堆(HCP)、面心立方(FCC)、简单立方(SC)以及二十面体(ICO)的相对分布次序并不随熔化速率的改变而改变, 温度仍然是影响原子结构分布的主要因素. 通过从头算分子动力学(ab initio MD)与热力学分析发现, ICO能够在液态金属区域稳定存在, 一方面是因为其孤立团簇的相对稳定性和团簇寿命要优于晶体型原子团簇, 另一方面是因为其拥有相对较高的团簇熵与相对较低的自由能.

关键词:

分子动力学 /
钒 /
熔化 /
团簇

Abstract

By using large-scale atomic/molecular massively parallel simulator (LAMMPS) code, a molecular dynamics simulation is performed in the NPT ensemble at zero pressure to investigate the influence of melting rates γ on the evolutional characteristics of vanadium atomic structure such as body-centered cubic (BCC), hexagonal close-packed structure (HCP), face centered cubic (FCC), simple cubic (SC) and icosahedra (ICO) during the rapid melting of solid vanadium crystal at five different melting rates (γ₁ = 1 × 10¹¹ K/s, γ₂ = 1 × 10¹² K/s, γ₃ = 1 × 10¹³ K/s, γ₄ = 1 × 10¹⁴ K/s , γ₅ = 1 × 10¹⁵ K/s), in which 16000 atoms in a cubic box under the periodic boundary condition are considered, and their motion equations are solved by Verlet’s algorithm in the velocity form in time steps of 1 fs. Constant pressure P and temperature T are imposed by a modified Nose-Hoover method for both P and T variables, and an embedded-atom model (EAM) potential is utilized. For identifying the local atomic structures of liquid and solid vanadium at different temperatures, a polyhedral template matching method (PTMM) is used by measuring the root-mean square deviation (RMSD), in which clusters are classified as the topology of the local atomic environment without any ambiguity in the classification. Subsequently, the variation of the potential energy, entropy and Gibbs free energy of FCC, HCP, BCC and ICO vanadium clusters are calculated through ab initio MD simulation in the canonical ensemble (NVT) at selected temperatures, and the lowest-energy dynamic structure and its corresponding static heating structure are also shown in this paper. Based on the above calculated results, it is found that the melting point of refractory metal vanadium increases obviously with the increase of heating rate, but the heating rate only presents a limited effect on the population of atomic structure for each of BCC, HCP, FCC, SC and ICO. Namely, the temperature still plays a dominant role in the rapid melting process of V rather than heating rate. Moreover, the ab initio MD simulation and thermodynamics analysis further reveal that lots of ICO clusters of vanadium can exist stably in the liquid region rather than in solid crystal, which is not only due to its higher stability and longer lifetime than those of crystalline atomic clusters, but also because ICO possesses higher entropy and lower Gibbs free energy in high temperature liquid region.

Keywords:

作者及机构信息

蒋元祺^1,2

1.
南昌师范学院物理系, 南昌　330032

2.
吉林大学物理学院, 长春　130012

通信作者: 蒋元祺, yuanqi325@163.com

基金项目: 江西省自然科学基金(批准号: 20202BAB204004, 20171BAB216001)、江西省教育厅科学技术研究项目(批准号: GJJ191114, GJJ161242)、南昌师范学院首批“青蓝学者”人才计划和国家自然科学基金(批准号: 51871096, 11664028)资助的课题

Authors and contacts

Jiang Yuan-Qi^1,2

1.
Department of Physics, Nanchang Normal University, Nanchang 330032, China

2.
College of Physics, Jilin University, Changchun 130012, China

Corresponding author: Jiang Yuan-Qi, yuanqi325@163.com

Funds: Project supported by the Jiangxi Provincial Natural Science Foundation, China (Grant Nos. 20202BAB204004, 20171BAB216001), the Scientific Research Project of Jiangxi Provincial Education Department, China (Grant Nos. GJJ191114, GJJ161242), the Qinglan Scholars Program of Nanchang Normal University, China, and the National Natural Science Foundation of China (Grant Nos. 51871096, 11664028)

文章全文 : translate this paragraph

参考文献

[1]	Cheng Y Q, Ma E 2011 Prog. Mater. Sci. 56 379 Google Scholar
[2]	Baletto F 2005 Rev. Mod. Phys. 77 371 Google Scholar
[3]	Turci F, Tarjus G, Royall C P 2017 Phys. Rev. Lett. 118 215501 Google Scholar
[4]	Shen Y T, Kim T H, Gangopadhyay A K, Kelton K F 2009 Phys. Rev. Lett. 102 057801 Google Scholar
[5]	Sprakel J, Zaccone A, Spaepen F, Schall P, Weitz D 2017 Phys. Rev. Lett. 118 088003 Google Scholar
[6]	Şopu D, Stukowski A, Stoica M, Scudino S 2017 Phys. Rev. Lett. 119 195503 Google Scholar
[7]	Jiang Y Q, Wen D D, Peng P 2017 J. Mole. Liquids 230 271 Google Scholar
[8]	Jiang Y Q, Wen D D, Peng P, Han S C, Hou Z Y 2015 Comput. Mater. Sci. 99 156 Google Scholar
[9]	Zhong L, Wang J, Sheng H, Zhang Z, Mao S X 2014 Nature 512 177 Google Scholar
[10]	蒋元祺, 彭平 2018 67 132101 Google Scholar Jiang Y Q, Peng P 2018 Acta Phys. Sin. 67 132101 Google Scholar
[11]	Jiang Y Q, Peng P 2020 Chin. Phys. B. 29 046105 Google Scholar
[12]	Jiang Y Q, Wen D D, He W X, Peng P 2018 Mol. Simulat. 44 1183 Google Scholar
[13]	武振伟, 李茂枝, 徐莉梅, 汪卫华 2017 66 176405 Google Scholar Wu Z W, Li M Z, Xu L M, Wang W H 2017 Acta Phys. Sin. 66 176405 Google Scholar
[14]	邓永和, 文大东, 彭超, 韦彦丁, 赵瑞, 彭平 2016 65 066401 Google Scholar Deng Y H, Wen D D, Peng C, Wei Y D, Zhao R, Peng P 2016 Acta Phys. Sin. 65 066401 Google Scholar
[15]	孙保安, 王利峰, 邵建华 2017 66 178103 Google Scholar Sun B A, Wang L F, Shao J H 2017 Acta Phys. Sin. 66 178103 Google Scholar
[16]	Yang M H, Cai B, Sun Y, Zhang F, Wang Y F, Wang C Z, Ho K M 2019 Phys. Rev. Mater. 3 125602 Google Scholar
[17]	孙奕韬, 王超, 吕玉苗, 胡远超, 罗鹏, 刘明, 咸海杰, 赵德乾, 丁大伟, 孙保安, 潘明祥, 闻平, 白海洋, 柳延辉, 汪卫华 2018 67 126101 Google Scholar Sun Y T, Wang C, Lü Y M, Hu Y C, Luo P, Liu M, Xian H J, Zhao D Q, Ding D W, Sun B A, Pan M X, Wen P, Bai H Y, Liu Y H, Wang W H 2018 Acta Phys. Sin. 67 126101 Google Scholar
[18]	Li M X, Zhao S F, Lu Z, Hirata A, Wen P, Bai H Y, Chen M W, Schroers J, Liu Y H, Wang W H 2019 Nature 569 99 Google Scholar
[19]	Sheng H W, Luo W K, Alamgir F M, Bai J M, Ma E 2006 Nature 439 419 Google Scholar
[20]	Hirata A, Kang L J, Fujita T, Klumov B, Matsue K, Kotani M, Yavari A R, Chen M W 2013 Science 341 376 Google Scholar
[21]	Wang H Q, Li H F 2015 RSC Adv. 5 94685 Google Scholar
[22]	Sosso C C, Chen J, Stephen J, Fitzner M, Pedevilla P, Zen A, Michaelides A 2016 Chem. Rev. 116 7078 Google Scholar
[23]	Taherkhani F, Akbarzadeh H, Rezania H 2014 J. Alloys. Compd. 617 746 Google Scholar
[24]	Chen L, Wang Q, Xiong L 2017 J. Nanopart. Res. 19 300 Google Scholar
[25]	Zhang Z, Hu W, Xiao S 2006 Phys. Rev. B 73 125443 Google Scholar
[26]	Nunez S, Lopez J M, Aguado A 2012 Nanoscale 4 6481 Google Scholar
[27]	Steenbergen K G, Gaston N 2013 Phys. Chem. Chem. Phys. 15 15325 Google Scholar
[28]	Taran S, Garip A K, Arslan H 2020 J. Clus. Sci. 10876 Google Scholar
[29]	Taran S, Garip A K, Arslan H 2020 Chin. Phys. B 29 077801 Google Scholar
[30]	Elatresh S F, Bonev S A, Gregoryanz E, Ashcroft N W 2016 Phys. Rev. B 94 104107 Google Scholar
[31]	Zhao C Y, Tao Y, Yu Y S 2020 Int. J. Mass. Tran. 150 119382 Google Scholar
[32]	Feng D, Feng Y, Yuan S, Zhang X, Wang G 2017 Appl. Therm. Eng. 111 1457 Google Scholar
[33]	Fang X W, Wang C Z, Yao Y X, Ding Z J, Ho K M 2011 Phys. Rev. B 83 224203 Google Scholar
[34]	Aguado A, Jarrold M F 2011 Annu. Rev. Phys. Chem. 62 151 Google Scholar
[35]	Lekka C E, Papaconstantopoulos D A 2007 Surf. Sci. 601 3937 Google Scholar
[36]	Pyfer K L, Kafader J O, Yalamanchali A, Jarrold M F 2014 J. Phys. Chem. A 118 4900 Google Scholar
[37]	Haberland H, Hippler T, Donges J, Kostko O, Schmidt M, Issendorff B 2005 Phys. Rev. Lett. 94 035701 Google Scholar
[38]	Zhang L, Sun H 2010 Phys. Status Solidi 207 1178 Google Scholar
[39]	Jena P, Sun Q 2018 Chem. Rev. 118 5755 Google Scholar
[40]	Chacko S, Joshi K, Kanhere D G 2004 Phys. Rev. Lett. 92 135506 Google Scholar
[41]	Rapacioli M, Tarrat N, Spiegelman F 2018 J. Phys. Chem. A 122 4092 Google Scholar
[42]	Breaux G.A, Benirschke R C, Sugai T, Kinnear B S, Jarrold M F 2003 Phys. Rev. Lett. 91 215508 Google Scholar
[43]	Nelli D, Ferrando R 2019 Nanoscale 11 13040 Google Scholar
[44]	Settem M, Kanjarla A K 2020 Sci. Rep. 10 3296 Google Scholar
[45]	Wu J, Qi L, You H, Gross A, Li J, Yang H 2012 J. Am. Chem. Soc. 134 11880 Google Scholar
[46]	Zhang J, Chen J, Hu P, Wang H 2020 Chin. Chem. Lett. 31 890 Google Scholar
[47]	Mao H K, Chen X J, Ding Y, Li B, Wang B 2018 Rev. Mod. Phys. 90 015007 Google Scholar
[48]	Plimpton S 1995 J. Comput. Phys. 117 1 Google Scholar
[49]	Cai J, Ye Y 1996 Phys. Rev. B 54 8398 Google Scholar
[50]	Stukowski A 2010 Model. Simul. Mater. Sc. 18 015012 Google Scholar
[51]	Larsen P M, Schmidt S, Schiøtz J 2016 Model. Simul. Mater. Sc. 24 055007 Google Scholar
[52]	蒋元祺 2015 博士学位论文(长沙: 湖南大学) Jiang Y Q 2015 Ph. D. Dissertation (Changsha: Hunan University) (in Chinese)

施引文献

图 1 5种不同熔化速率下金属钒体系的势能变化与温度之间的演变关系, (b)是(a)的局部放大图

Fig. 1. The potential energy of the vanadium metal as a function of temperature at various melting rates γ, (b) is a partial enlarged view of (a).

下载: 全尺寸图片幻灯片

图 2 5种不同熔化速率下金属钒体系的体积变化与温度之间的演变关系, (b)是(a)的局部放大图

Fig. 2. The volume of the vanadium metal as a function of temperature at various melting rates γ, (b) is a partial enlarged view of (a).

下载: 全尺寸图片幻灯片

图 3 不同熔化速率下体系压强随温度的演变关系

Fig. 3. The pressure of the vanadium metal as a function of temperature at various melting rates γ.

下载: 全尺寸图片幻灯片

图 4 熔化速率为γ₂ = 1 × 10¹² K/s时RDF在不同温度区间的演变关系

Fig. 4. The RDF for vanadium metal at different temperature obtained by rapid heating rate γ₂ = 1 × 10¹² K/s.

下载: 全尺寸图片幻灯片

图 5 晶体钒熔化过程不同类型团簇的演变示意图(γ₂ = 1 × 10¹² K/s)

Fig. 5. Different type atomic schematic diagraph in vanadium system at heating rate γ₂ = 1 × 10¹² K/s.

下载: 全尺寸图片幻灯片

图 6 利用多面体模板匹配法分析得到的5种不同熔化速率下FCC, HCP, BCC, ICO以及SC类型团簇原子随温度的演变关系

Fig. 6. The population of the FCC, HCP, BCC, ICO and SC types atoms in V system as a function of temperature obtained from the polyhedral template matching at various rates, respectively.

下载: 全尺寸图片幻灯片

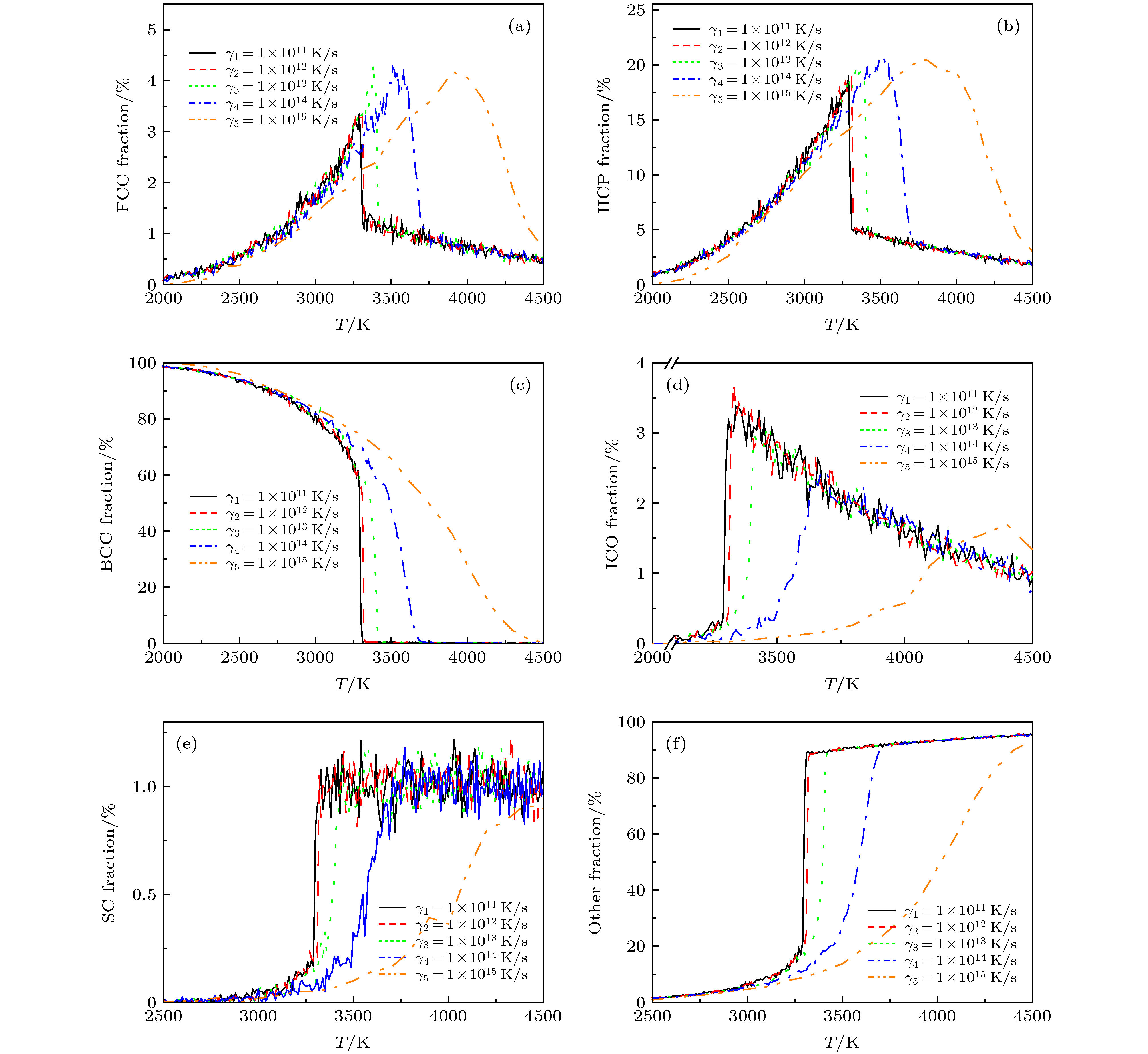
图 7 利用多面体模板匹配法分析得到的5种不同熔化速率下各种类型团簇原子分布随温度的演变关系　(a) FCC; (b) HCP; (c) BCC; (d) ICO; (e) SC; (f)其他类型团簇

Fig. 7. The fraction of the various types atoms in V system as a function of temperature obtained from the polyhedral template matching at five various rates: (a) FCC; (b) HCP; (c) BCC; (d) ICO; (e) SC; (f) other types atoms counts.

下载: 全尺寸图片幻灯片

图 8 FCC, HCP, BCC以及ICO 团簇平均每原子势能随模拟步长的演变趋势　(a) 300 K; (b) 500 K; (c) 1100 K

Fig. 8. The variation of the potential energy of per atom of FCC, HCP, BCC and ICO cluster as a function of time step, respectively: (a) 300 K; (b) 500 K; (c) 1100 K

下载: 全尺寸图片幻灯片

图 9 FCC, HCP, BCC及ICO 原子团簇的熵与吉布斯自由能随温度的演变关系　(a) 熵; (b) 吉布斯自由能

Fig. 9. The variation of the entropy and Gibbs free energy of FCC, HCP, BCC and ICO cluster as a function of temperature, respectively: (a) Entropy; (b) Gibbs free energy.

下载: 全尺寸图片幻灯片

map

[1]	Cheng Y Q, Ma E 2011 Prog. Mater. Sci. 56 379 Google Scholar
[2]	Baletto F 2005 Rev. Mod. Phys. 77 371 Google Scholar
[3]	Turci F, Tarjus G, Royall C P 2017 Phys. Rev. Lett. 118 215501 Google Scholar
[4]	Shen Y T, Kim T H, Gangopadhyay A K, Kelton K F 2009 Phys. Rev. Lett. 102 057801 Google Scholar
[5]	Sprakel J, Zaccone A, Spaepen F, Schall P, Weitz D 2017 Phys. Rev. Lett. 118 088003 Google Scholar
[6]	Şopu D, Stukowski A, Stoica M, Scudino S 2017 Phys. Rev. Lett. 119 195503 Google Scholar
[7]	Jiang Y Q, Wen D D, Peng P 2017 J. Mole. Liquids 230 271 Google Scholar
[8]	Jiang Y Q, Wen D D, Peng P, Han S C, Hou Z Y 2015 Comput. Mater. Sci. 99 156 Google Scholar
[9]	Zhong L, Wang J, Sheng H, Zhang Z, Mao S X 2014 Nature 512 177 Google Scholar
[10]	蒋元祺, 彭平 2018 67 132101 Google Scholar Jiang Y Q, Peng P 2018 Acta Phys. Sin. 67 132101 Google Scholar
[11]	Jiang Y Q, Peng P 2020 Chin. Phys. B. 29 046105 Google Scholar
[12]	Jiang Y Q, Wen D D, He W X, Peng P 2018 Mol. Simulat. 44 1183 Google Scholar
[13]	武振伟, 李茂枝, 徐莉梅, 汪卫华 2017 66 176405 Google Scholar Wu Z W, Li M Z, Xu L M, Wang W H 2017 Acta Phys. Sin. 66 176405 Google Scholar
[14]	邓永和, 文大东, 彭超, 韦彦丁, 赵瑞, 彭平 2016 65 066401 Google Scholar Deng Y H, Wen D D, Peng C, Wei Y D, Zhao R, Peng P 2016 Acta Phys. Sin. 65 066401 Google Scholar
[15]	孙保安, 王利峰, 邵建华 2017 66 178103 Google Scholar Sun B A, Wang L F, Shao J H 2017 Acta Phys. Sin. 66 178103 Google Scholar
[16]	Yang M H, Cai B, Sun Y, Zhang F, Wang Y F, Wang C Z, Ho K M 2019 Phys. Rev. Mater. 3 125602 Google Scholar
[17]	孙奕韬, 王超, 吕玉苗, 胡远超, 罗鹏, 刘明, 咸海杰, 赵德乾, 丁大伟, 孙保安, 潘明祥, 闻平, 白海洋, 柳延辉, 汪卫华 2018 67 126101 Google Scholar Sun Y T, Wang C, Lü Y M, Hu Y C, Luo P, Liu M, Xian H J, Zhao D Q, Ding D W, Sun B A, Pan M X, Wen P, Bai H Y, Liu Y H, Wang W H 2018 Acta Phys. Sin. 67 126101 Google Scholar
[18]	Li M X, Zhao S F, Lu Z, Hirata A, Wen P, Bai H Y, Chen M W, Schroers J, Liu Y H, Wang W H 2019 Nature 569 99 Google Scholar
[19]	Sheng H W, Luo W K, Alamgir F M, Bai J M, Ma E 2006 Nature 439 419 Google Scholar
[20]	Hirata A, Kang L J, Fujita T, Klumov B, Matsue K, Kotani M, Yavari A R, Chen M W 2013 Science 341 376 Google Scholar
[21]	Wang H Q, Li H F 2015 RSC Adv. 5 94685 Google Scholar
[22]	Sosso C C, Chen J, Stephen J, Fitzner M, Pedevilla P, Zen A, Michaelides A 2016 Chem. Rev. 116 7078 Google Scholar
[23]	Taherkhani F, Akbarzadeh H, Rezania H 2014 J. Alloys. Compd. 617 746 Google Scholar
[24]	Chen L, Wang Q, Xiong L 2017 J. Nanopart. Res. 19 300 Google Scholar
[25]	Zhang Z, Hu W, Xiao S 2006 Phys. Rev. B 73 125443 Google Scholar
[26]	Nunez S, Lopez J M, Aguado A 2012 Nanoscale 4 6481 Google Scholar
[27]	Steenbergen K G, Gaston N 2013 Phys. Chem. Chem. Phys. 15 15325 Google Scholar
[28]	Taran S, Garip A K, Arslan H 2020 J. Clus. Sci. 10876 Google Scholar
[29]	Taran S, Garip A K, Arslan H 2020 Chin. Phys. B 29 077801 Google Scholar
[30]	Elatresh S F, Bonev S A, Gregoryanz E, Ashcroft N W 2016 Phys. Rev. B 94 104107 Google Scholar
[31]	Zhao C Y, Tao Y, Yu Y S 2020 Int. J. Mass. Tran. 150 119382 Google Scholar
[32]	Feng D, Feng Y, Yuan S, Zhang X, Wang G 2017 Appl. Therm. Eng. 111 1457 Google Scholar
[33]	Fang X W, Wang C Z, Yao Y X, Ding Z J, Ho K M 2011 Phys. Rev. B 83 224203 Google Scholar
[34]	Aguado A, Jarrold M F 2011 Annu. Rev. Phys. Chem. 62 151 Google Scholar
[35]	Lekka C E, Papaconstantopoulos D A 2007 Surf. Sci. 601 3937 Google Scholar
[36]	Pyfer K L, Kafader J O, Yalamanchali A, Jarrold M F 2014 J. Phys. Chem. A 118 4900 Google Scholar
[37]	Haberland H, Hippler T, Donges J, Kostko O, Schmidt M, Issendorff B 2005 Phys. Rev. Lett. 94 035701 Google Scholar
[38]	Zhang L, Sun H 2010 Phys. Status Solidi 207 1178 Google Scholar
[39]	Jena P, Sun Q 2018 Chem. Rev. 118 5755 Google Scholar
[40]	Chacko S, Joshi K, Kanhere D G 2004 Phys. Rev. Lett. 92 135506 Google Scholar
[41]	Rapacioli M, Tarrat N, Spiegelman F 2018 J. Phys. Chem. A 122 4092 Google Scholar
[42]	Breaux G.A, Benirschke R C, Sugai T, Kinnear B S, Jarrold M F 2003 Phys. Rev. Lett. 91 215508 Google Scholar
[43]	Nelli D, Ferrando R 2019 Nanoscale 11 13040 Google Scholar
[44]	Settem M, Kanjarla A K 2020 Sci. Rep. 10 3296 Google Scholar
[45]	Wu J, Qi L, You H, Gross A, Li J, Yang H 2012 J. Am. Chem. Soc. 134 11880 Google Scholar
[46]	Zhang J, Chen J, Hu P, Wang H 2020 Chin. Chem. Lett. 31 890 Google Scholar
[47]	Mao H K, Chen X J, Ding Y, Li B, Wang B 2018 Rev. Mod. Phys. 90 015007 Google Scholar
[48]	Plimpton S 1995 J. Comput. Phys. 117 1 Google Scholar
[49]	Cai J, Ye Y 1996 Phys. Rev. B 54 8398 Google Scholar
[50]	Stukowski A 2010 Model. Simul. Mater. Sc. 18 015012 Google Scholar
[51]	Larsen P M, Schmidt S, Schiøtz J 2016 Model. Simul. Mater. Sc. 24 055007 Google Scholar
[52]	蒋元祺 2015 博士学位论文(长沙: 湖南大学) Jiang Y Q 2015 Ph. D. Dissertation (Changsha: Hunan University) (in Chinese)

[1]	邢赫威, 陈占秀, 杨历, 苏瑶, 李源华, 呼和仓. 不凝性气体对纳米通道内水分子流动传热影响的分子动力学模拟. , 2024, 73(9): 094701. doi: 10.7498/aps.73.20240192
[2]	文大东, 祁青华, 黄欣欣, 易洲, 邓永和, 田泽安, 彭平. 液态Ta快凝过程中团簇的遗传及其与局域对称性的关联. , 2023, 72(24): 246101. doi: 10.7498/aps.72.20231153
[3]	华颖鑫, 陈小辉, 李俊, 郝龙, 孙毅, 王玉峰, 耿华运. 钒的冲击熔化原位X射线衍射测量研究. , 2022, 71(7): 076201. doi: 10.7498/aps.71.20212065
[4]	郑治秀, 张林. Fe基体中包含Cu团簇的Fe-Cu二元体系在升温过程中结构变化的原子尺度计算. , 2017, 66(8): 086301. doi: 10.7498/aps.66.086301
[5]	汪志刚, 黄娆, 文玉华. Pt-Au核-壳结构纳米粒子热稳定性的分子动力学研究. , 2013, 62(12): 126101. doi: 10.7498/aps.62.126101
[6]	周耐根, 胡秋发, 许文祥, 李克, 周浪. 硅熔化特性的分子动力学模拟–-不同势函数的对比研究. , 2013, 62(14): 146401. doi: 10.7498/aps.62.146401
[7]	李春丽, 段海明, 买力坦, 开来木. Aln(n=13–32)团簇熔化行为的分子动力学模拟研究. , 2013, 62(19): 193104. doi: 10.7498/aps.62.193104
[8]	汪志刚, 黄娆, 文玉华. Au-Pd共晶纳米粒子熔化行为的分子动力学研究. , 2012, 61(16): 166102. doi: 10.7498/aps.61.166102
[9]	张英杰, 肖绪洋, 李永强, 颜云辉. 分子动力学模拟Cu(010)基体对负载Co-Cu双金属团簇熔化过程的影响. , 2012, 61(9): 093602. doi: 10.7498/aps.61.093602
[10]	韩小静, 王音, 林正喆, 张文献, 庄军, 宁西京. 团簇异构体生长概率的理论预测. , 2010, 59(5): 3445-3449. doi: 10.7498/aps.59.3445
[11]	卢敏, 许卫兵, 刘维清, 侯春菊, 刘志勇. 银纳米杆高温熔化断裂弛豫性能的原子级模拟研究. , 2010, 59(9): 6377-6383. doi: 10.7498/aps.59.6377
[12]	樊沁娜, 李蔚, 张林. 熔融Cu57团簇在急冷过程中弛豫和局域结构转变的分子动力学研究. , 2010, 59(4): 2428-2433. doi: 10.7498/aps.59.2428
[13]	张林, 徐送宁, 李蔚, 孙海霞, 张彩碚. 小尺寸铜团簇冷却与并合过程中结构变化的原子尺度研究. , 2009, 58(13): 58-S66. doi: 10.7498/aps.58.58
[14]	文玉华, 孙世刚, 张杨, 朱梓忠. 铂纳米晶在升温过程中结构演化与熔化特征的原子级模拟研究. , 2009, 58(4): 2585-2589. doi: 10.7498/aps.58.2585
[15]	赵骞, 张林, 祁阳, 张宗宁. 低温下Cu13团簇负载于Cu(001)表面上结构变化的分子动力学研究. , 2009, 58(13): 47-S52. doi: 10.7498/aps.58.47
[16]	张宗宁, 刘美林, 李蔚, 耿长建, 赵骞, 张林. 熔融Cu55团簇在Cu(010)表面上凝固过程的分子动力学模拟. , 2009, 58(13): 67-S71. doi: 10.7498/aps.58.67
[17]	张林, 张彩碚, 祁阳. 低温下Au959团簇负载于MgO(100)表面后结构变化的分子动力学研究. , 2009, 58(13): 53-S57. doi: 10.7498/aps.58.53
[18]	徐送宁, 张林, 张彩碚, 祁阳. 熔融Cu55团簇在铜块体中凝固过程的分子动力学模拟. , 2009, 58(13): 40-S46. doi: 10.7498/aps.58.40
[19]	刘建廷, 段海明. 不同势下铱团簇结构和熔化行为的分子动力学模拟. , 2009, 58(7): 4826-4834. doi: 10.7498/aps.58.4826
[20]	张林, 王绍青, 叶恒强. 大角度Cu晶界在升温、急冷条件下晶界结构的分子动力学研究. , 2004, 53(8): 2497-2502. doi: 10.7498/aps.53.2497

计量

文章访问数: 7830
PDF下载量: 134
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

难熔金属钒熔化行为的局域原子结构模拟与分析