-
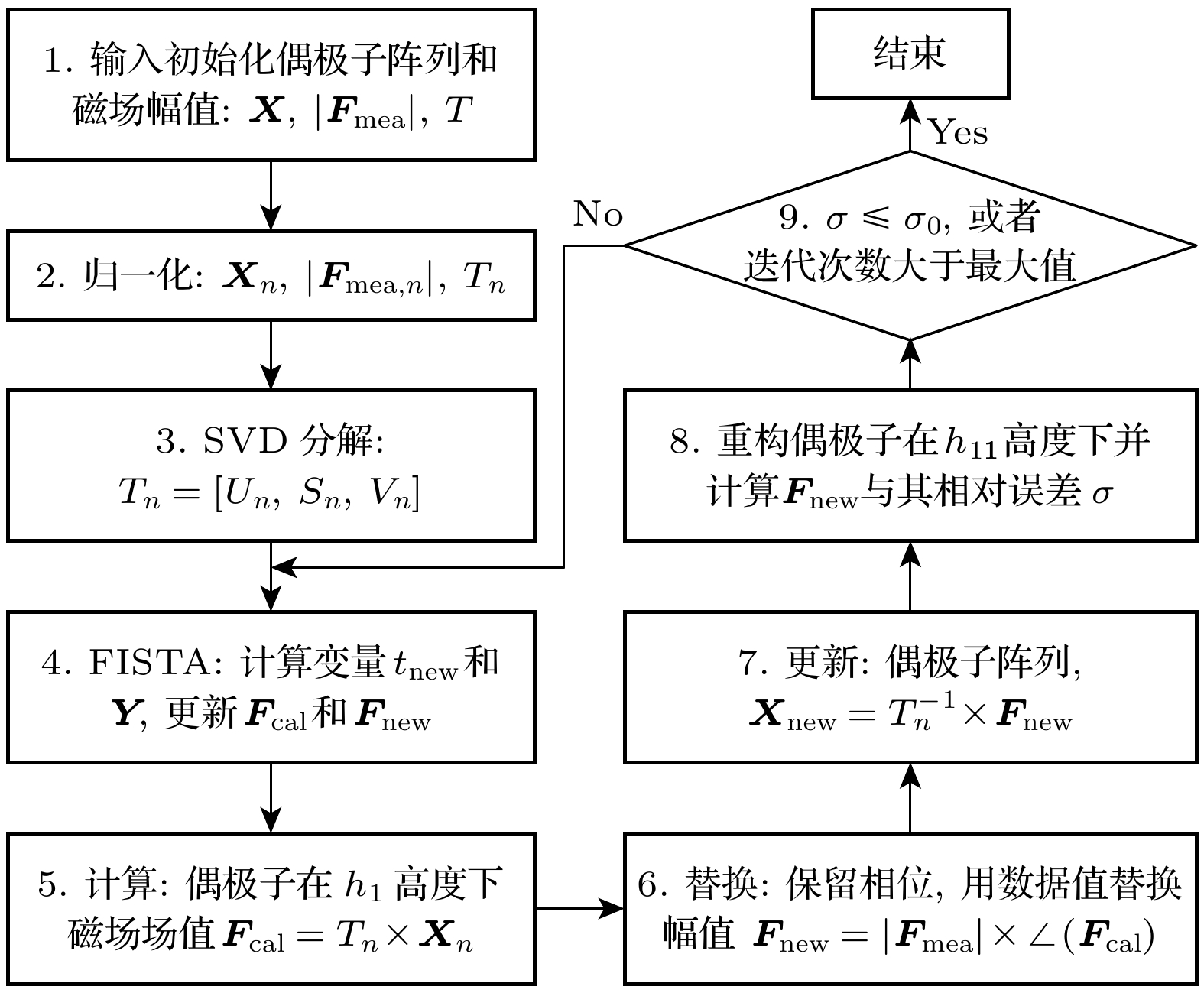
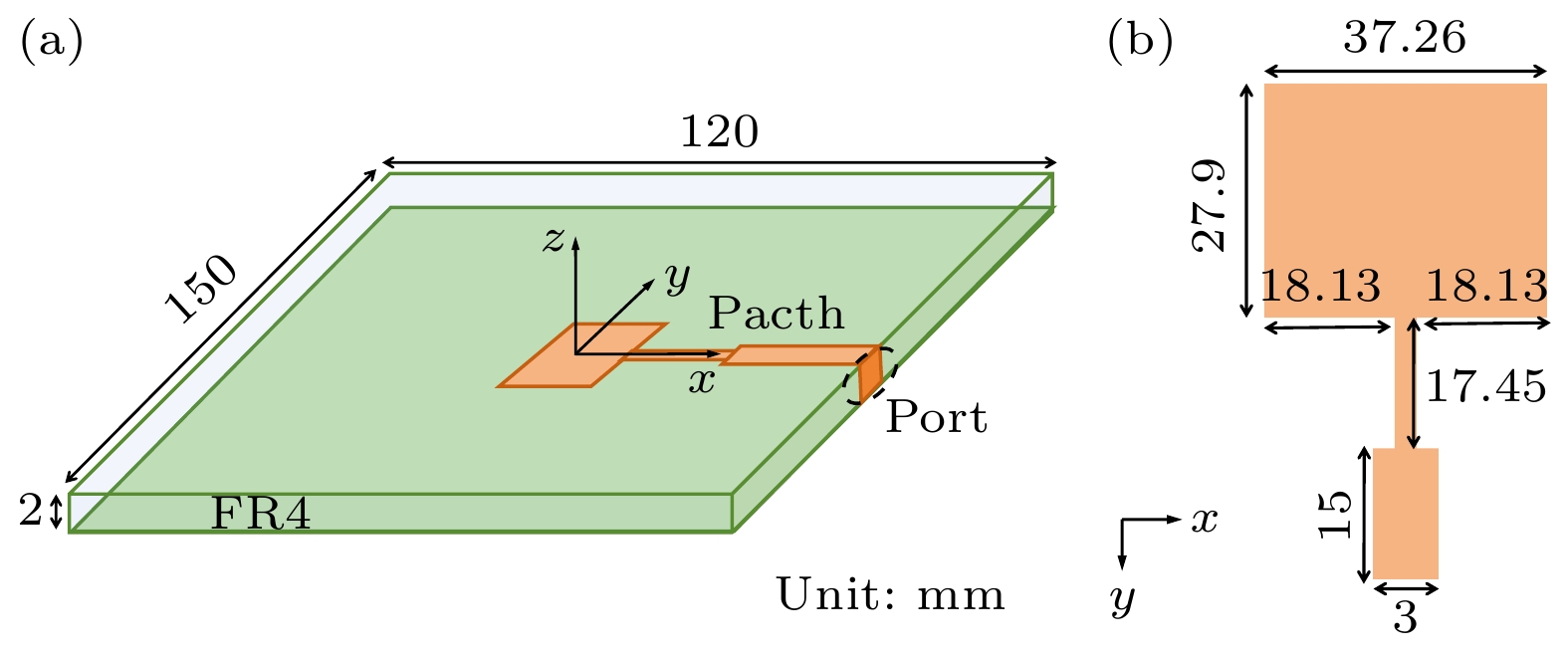
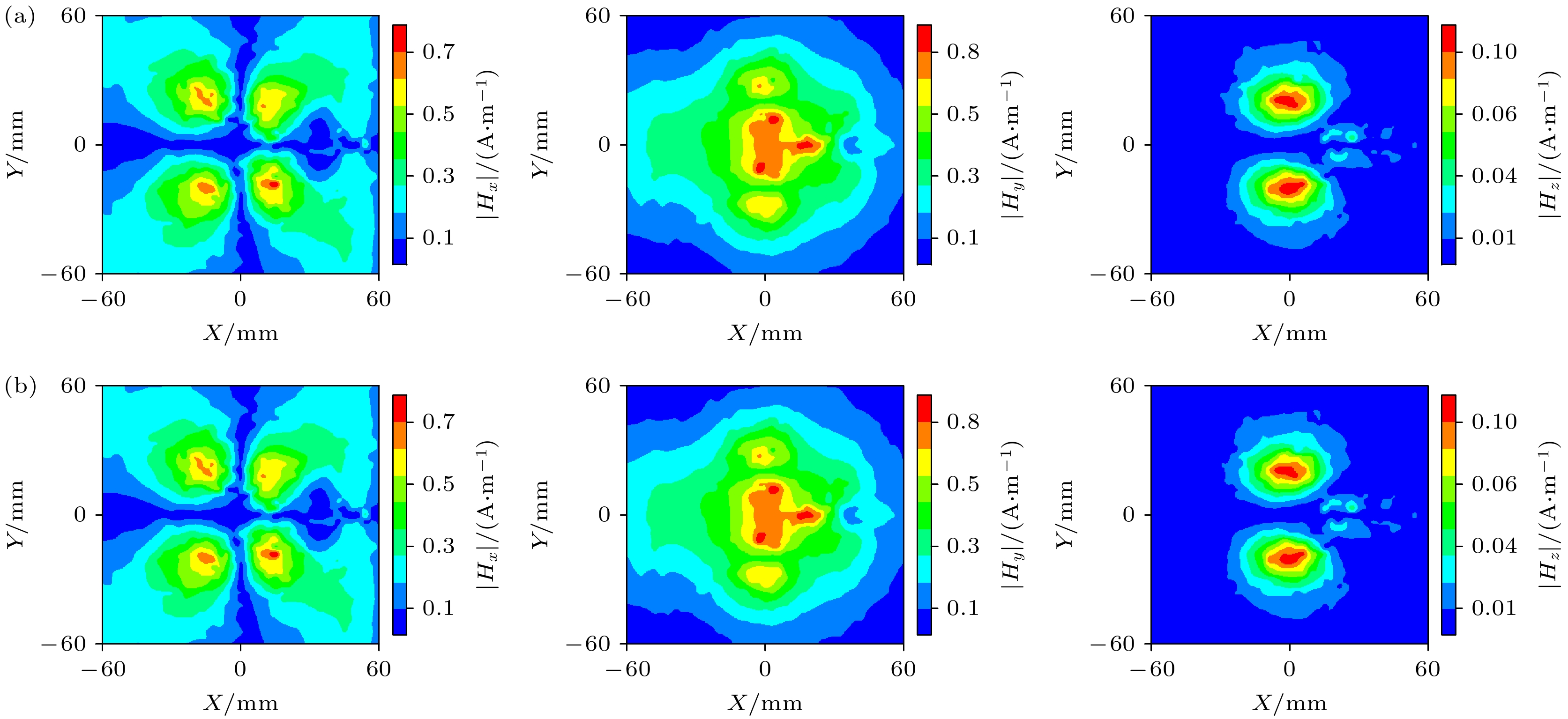
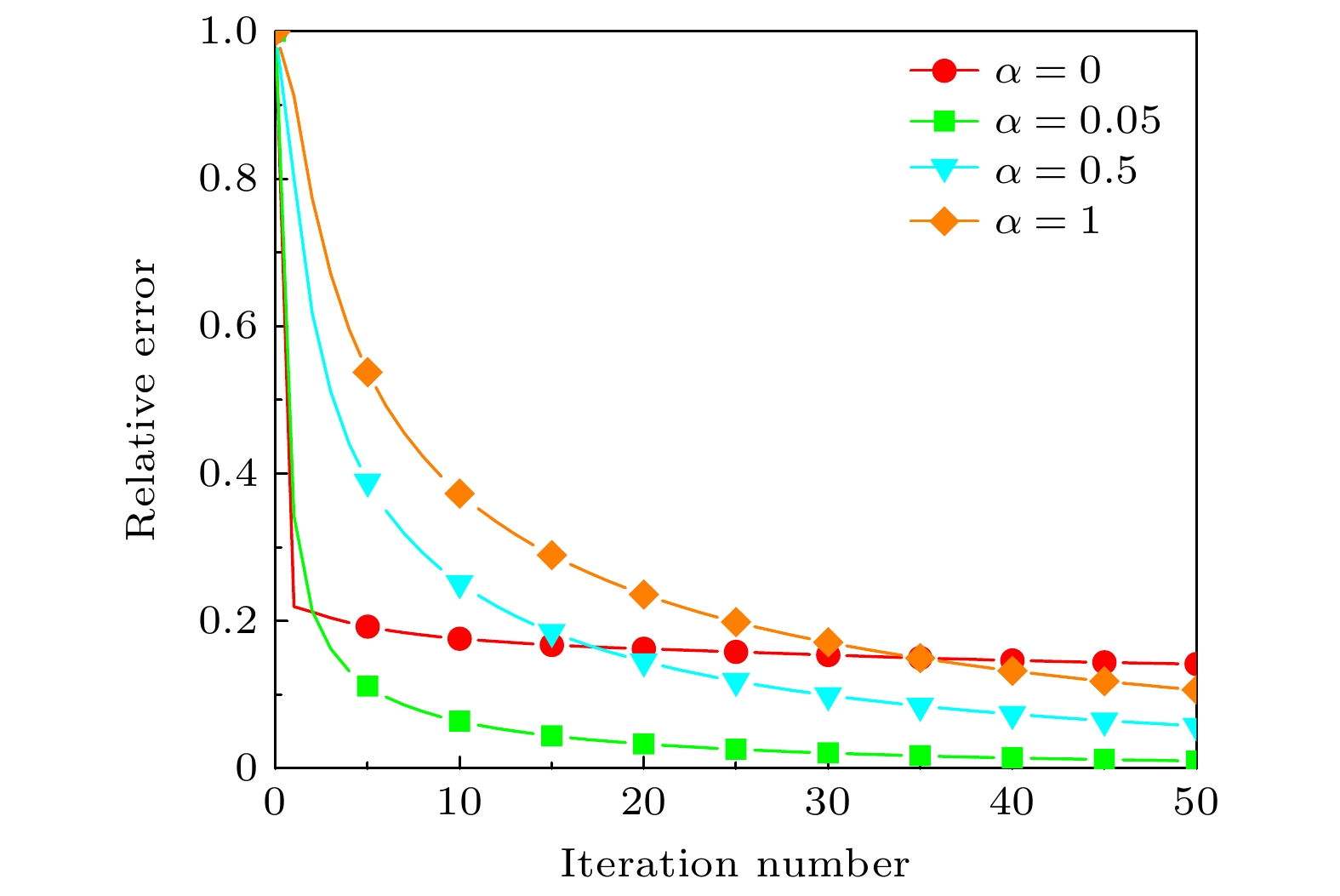
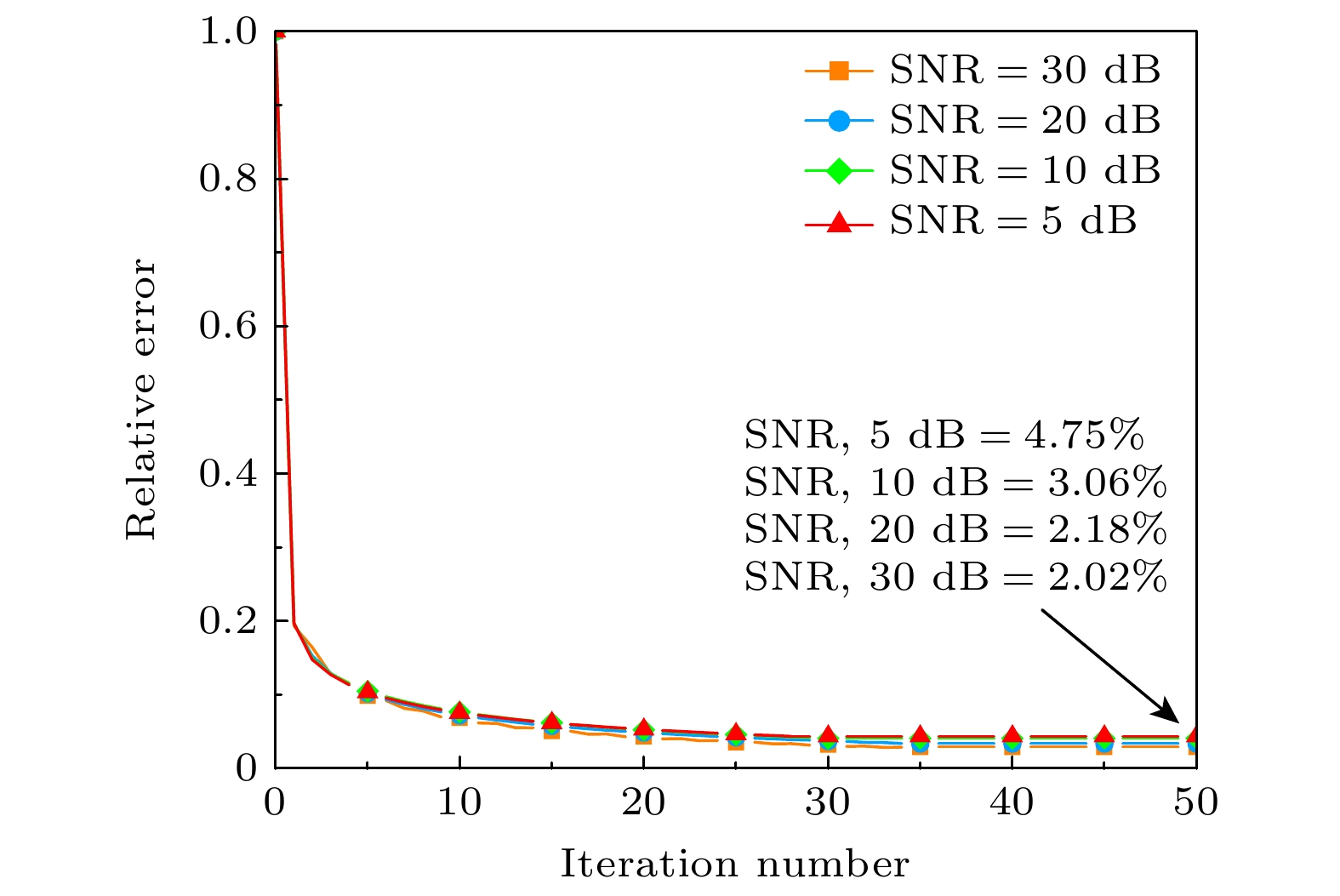
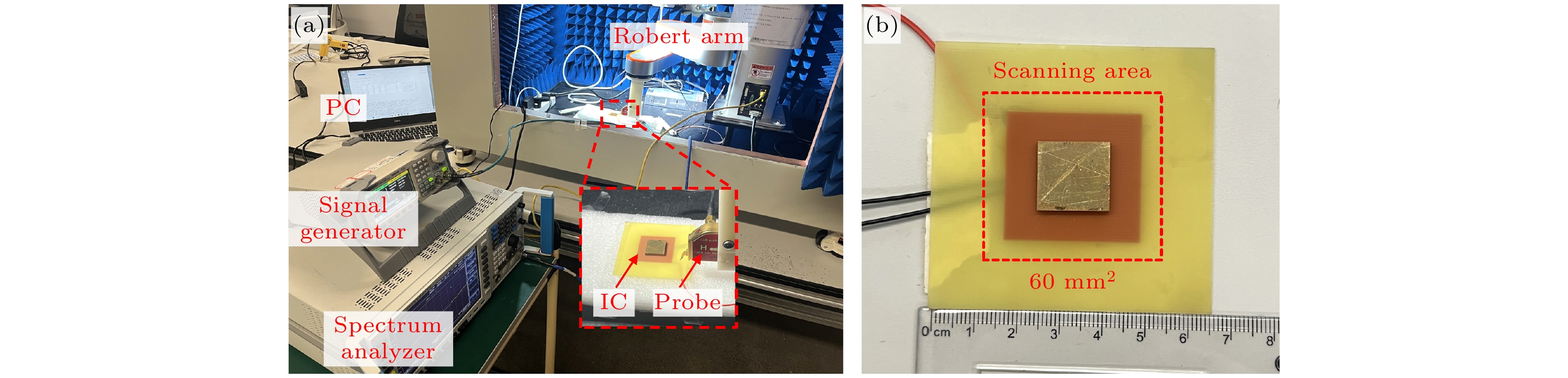
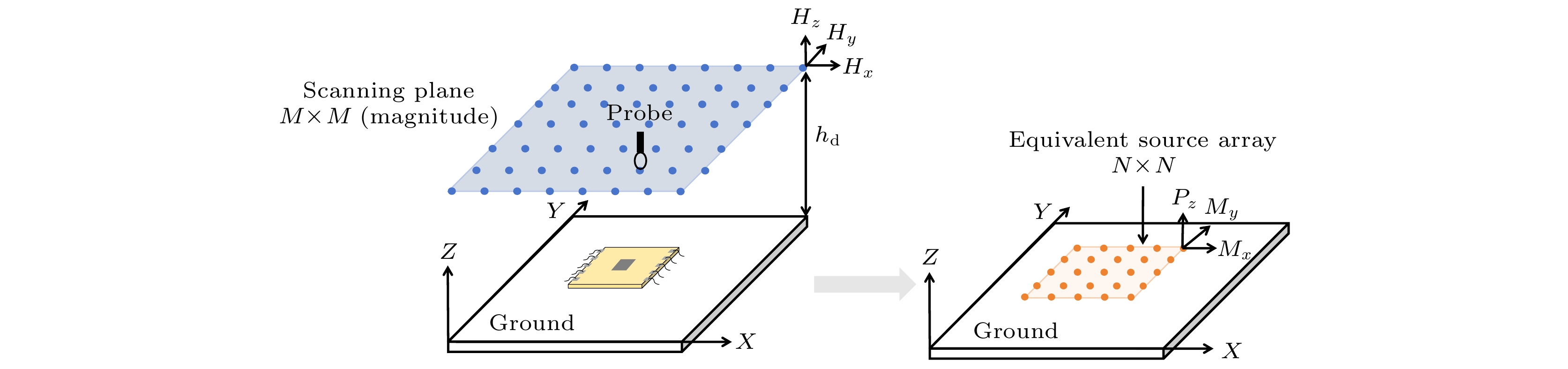
This paper proposes a phaseless radiation source reconstruction method based on singular value decomposition (SVD) regularization and fast iterative shrinkage-thresholding algorithm (FISTA), aimed at efficiently identifying electromagnetic interference (EMI) sources in integrated circuits (ICs). The method acquires electromagnetic field data through near-field scanning and reconstructs an equivalent dipole array on the surface of the radiation source using the source reconstruction method (SRM). During the reconstruction process, the SVD regularization term enhances the algorithm's stability and noise resistance, while FISTA accelerates the convergence speed.
To validate the effectiveness of the proposed method, dipole array reconstruction was first performed using near-field data at a height of 5mm for a patch antenna simulation model, followed by an analysis of magnetic field data at a 10mm validation plane. At the 35th iteration, the total relative error of the reconstruction was 1.21%. The influence of the regularization parameter α on the results was then investigated, and it was found that α = 0.05 yielded the smallest error. The method was also tested under different Gaussian white noise conditions, with relative errors remaining below 5%, demonstrating strong robustness.
Finally, chip experiments were conducted to verify the method. The proposed method converged stably within 35 iterations, with a relative error of 2.3% in the reconstruction results. The total iteration time is 61.7% of the single-layer phaseless interpolation algorithm, and the relative error is reduced by 52% compared to the double-layer phasless iteration algorithm.The experimental results demonstrate that the proposed method can efficiently and accurately reconstruct phaseless radiation sources, with good noise robustness, making it suitable for EMI analysis in integrated circuits.-
Keywords:
- Singular Value Decomposition (SVD) /
- Fast Iterative Shrinkage-Thresholding Algorithm (FISTA) /
- near-field scanning (NFS) /
- source reconstruction method (SRM)
-
[1] Schuman C D, Kulkarni S R, Parsa M, Mitchell J P, Date P, Kay B 2022 Nature Comput 2 10
[2] S. Serpaud, A. Boyer, S. B. Dhia and F. Coccetti 2022 IEEE Trans. Electromagn. Compat 64 816
[3] A. Boyer, N. Nolhier, F. Caignet and S. B. Dhia 2022 IEEE Trans. Electromagn. Compat 64 1230
[4] Cao Zhong, Du Ping-An, Nie Bao-Lin, Ren Dan, Zhang Qi-Dao 2014 Acta. Phys. Sin. 63 165 (in Chinese)[曹钟, 杜平安, 聂宝林, 任丹, 张其道 2014 63 165]
[5] Yang R, Wei X C, Shu Y F, Yi D, Yang Y B 2019 IEEE Trans. Antennas Propag 67 6821
[6] Zhang J, Kam K W, Min J, Khilkevich V V, Pommerenke D, Fan J 2013 IEEE Trans Instrum. Meas 62 648
[7] Wang L, Zhang Y, Han F, Zhou J, Liu QH 2020 IEEE Trans. Microw. Theory Techn 68 4151
[8] Weng H, Beetner D G, DuBroff R E 2011 IEEE Trans. Electromagn. Compat 53 891
[9] Zuo P, Li Y, Xu Y, Zheng H, Li E P 2019 IEEE Trans. Compon., Packag., Manuf. Technol 9 329
[10] Yu Z, Mix JA, Sajuyigbe S, Slattery KP, Fan J 2012 IEEE Trans. Electromagn. Compat 55 97
[11] Kornprobst J, Mauermayer R A, Neitz O, Knapp J, Eibert TF 2019 Progr. Electromagn. Res 165 47
[12] Yi Z, Zou J, Tian X, Huang Q, Fang W, Shao W, En Y, Gao Y, Han P 2023 IEEE Trans. Electromagn. Compat 65 879
[13] Regue J R, Ribó M, Garrell J M, Martín A 2001 IEEE Trans. Electromagn. Compat 43 520
[14] Han D H, Wei X C, Wang D, Liang W T, Song TH, Gao RX 2024 IEEE Trans. Electromagn. Compat 66 566
[15] Xiang F P, Li E P, Wei X C, Jin J M 2015 IEEE Trans. Electromagn. Compat 57 1197
[16] Shu Y F, Wei X C, Fan J, Yang R, Yang Y B. 2019 IEEE Trans. Microw. Theory Tech 67 1790
[17] Zhang J, Fan J 2017 IEEE Trans. Electromagn. Compat 59 557
[18] Shu Y F, Wei X C, Yang R, Liu E X. 2017 IEEE Trans. Electromagn. Compat 60 937
[19] Yi Z, Zou J, Tian X, Huang Q, Fang W 2023 IEEE Trans. Electromagn. Compat 65 879
[20] Beck A, Teboulle M 2009 SIAM J. Imaging Sci 2 183
Metrics
- Abstract views: 67
- PDF Downloads: 4
- Cited By: 0
下载:
