Capture of Rayleigh particles by focused double-ring perfect vortex beams

SHI Pengbo; YANG Chen; YIN Xiaojin; WANG Jie

doi:10.7498/aps.74.20241768

Abstract

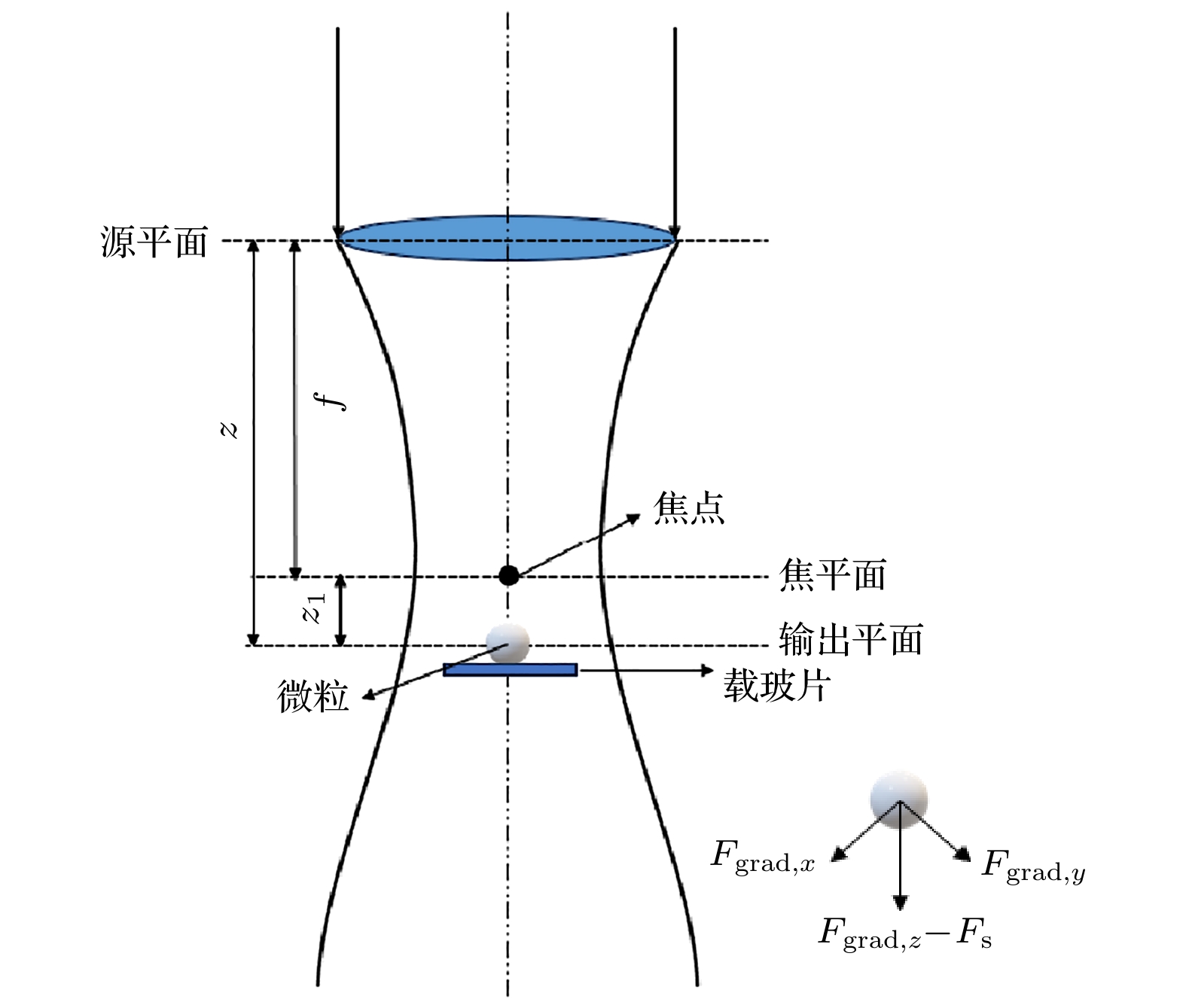
The double-ring perfect vortex beam (DR-PVB) is generated through the superposition of two concentric perfect vortex beams (PVBs). In this work, firstly, the intensity and phase distribution of the DR-PVB in the source plane are studied. Secondly, based on the Huygens-Fresnel principle and the Collins formula, the intensity distribution of the DR-PVB after being focused by an ABCD optical system that includes a focusing lens is obtained. The results indicate that the intensity distribution of the focused beam is consistent with the interference pattern of two Bessel Gaussian beams. Furthermore, the number of spots in the focused intensity distribution is a multiple of the absolute value of the difference in topological charges between two PVBs. On the other hand, the overall size of the light beam can be adjusted by changing the focal length of the lens. Thirdly, the optical radiation force, exerted by the focused DR-PVB, on Rayleigh particles with different refractive indices, silica and bubbles, are analyzed, respectively. The results show that the focused DR-PVB can capture both high and low refractive index particles in water. In addition, by comparing the focused DR-PVBs under different radius combinations, it found that the light intensity distribution can be changed with the beam radius, which leads the position and quantity of the captured particles to change. This result provides a new idea for adjusting the capture of particles in future experiments. Finally, the gradient forces, scattering, and Brownian forces acting on the particles in the x, y, and z directions are analyzed, respectively. Based on our analysis, the condition for stable particle capture, where the gradient force must overcome the effects of Brownian motion and scattering forces, is established. Therefore, the theoretical size range of particles that can be captured by the focused DR-PVB is determined. Compared with other beams, such as Airy beams and Bessel beams, the focused DR-PVB can be modulated by changing the topological charges of the two PVBs, making it possible to capture multiple particles. These results have potential applications in optical manipulation.

Keywords:

Authors and contacts

Shanxi Center of Technology Innovation for Light Manipulations and Applications, School of Applied Science, Taiyuan University of Science and Technology, Taiyuan 030024, China

Corresponding author: YANG Chen, 2020012@tyust.edu.cn

Funds: Project supported by the Young Scientists Found of the National Natural Science Foundation of China (Grant No. 62305238), the Fundamental Research Program of Shanxi Province, China (Grant No. 202103021223299), the Scientific Research Initial Funding of Taiyuan University of Science and Technology, China (Grant Nos. 20202014, 20202064, 20222009, 20222054), and the Postgraduate Education Innovation Program of Taiyuan University of Science and Technology, China (Grant No. SY2023056).

FullText HTML

References

[1]	Ashkin A, Dziedzic J M, Bjorkholm J E, Chu S 1986 Opt. Lett. 11 288 Google Scholar
[2]	Ciarlo A, Pastore R, Greco F, Sasso A, Pesce G 2023 Sci. Rep. 13 7408 Google Scholar
[3]	Chen H C, Cheng C J 2022 Appl. Sci. 12 10244 Google Scholar
[4]	Bustamante C J, Chemla Y R, Liu S, Wang M D 2021 Nat. Rev. Methods Primers 1 25 Google Scholar
[5]	Anderegg L, Cheuk L W, Bao Y, Burchesky S, Ketterle W, Ni K K, Doyle J M 2019 Science 365 1156 Google Scholar
[6]	Tian Y H, Wang L L, Duan G Y, Yu L 2021 Opt. Commun. 485 126712 Google Scholar
[7]	Munoz-Perez F M, Ferrando V, Furlan W D, Castro-Palacio J C, Arias-Gonzalez J R, Monsoriu J A 2023 iScience 26 107987 Google Scholar
[8]	闫玮植, 范青, 杨鹏飞, 李刚, 张鹏飞, 张天才 2023 72 114202 Google Scholar Yan W Z, Fan Q, Yang P F, Li G, Zhang P F, Zhang T C 2023 Acta Phys. Sin. 72 114202 Google Scholar
[9]	Santamato E, Daino B, Romagnoli M, Settembre M, Shen Y 1991 J. Opt. 22 158
[10]	Bonin K D, Kourmanov B, Walker T G 2002 Opt. Express 10 984 Google Scholar
[11]	Pedaci F, Huang Z, Van Oene M, Barland S, Dekker N H 2011 Biophys. J. 100 10a
[12]	Grier D G 2003 Nature 424 810 Google Scholar
[13]	Huang S J, Miao Z, He C, Pang F F, Li Y C, Wang T Y 2016 Opts. Lasers. Eng. 78 132 Google Scholar
[14]	Andrade U M S, Garcia A M, Rocha M S 2021 Appl. Opt. 60 3422 Google Scholar
[15]	Ostrovsky A S, Rickenstorff-Parrao C, Arrizon V 2013 Opt. Lett. 38 534 Google Scholar
[16]	Vaity P, Rusch L 2015 Opt. Lett. 40 597 Google Scholar
[17]	Chen Y, Fang Z X, Ren Y X, Gong L, Lu R D 2015 Appl. Opt. 54 8030 Google Scholar
[18]	Liu X J, Li Y L, Yao G P, Li C X, Fang B, Tang Y, Hong Z, Jing X F 2024 Chin. J. Phys. 91 828 Google Scholar
[19]	Ma H X, Li X Z, Tai Y P, Li H H, Wang J G, Tang M M, Tang J, Wang Y S, Nie Z G 2017 Ann. Phys. 529 1700285 Google Scholar
[20]	Das B K, Granados C, Krüger M, Ciappina M F 2024 Opt. Commun. 570 130918 Google Scholar
[21]	Chen M Z, Mazilu M, Arita Y 2015 Opt. Rev. 22 162 Google Scholar
[22]	Tkachenko G, Chen M Z, Dholakia K, Mazilu M 2017 Optica 4 330 Google Scholar
[23]	Liang Y S, Lei M, Yan S H, Li M M, Cai Y A, Wang Z J, Yu X H, Yao B L 2018 Appl. Opt. 57 79 Google Scholar
[24]	Wang S L, Xu J P, Yang Y P, Cheng M J 2024 Opt. Commun. 556 130258 Google Scholar
[25]	Chen M, Mazilu M, Arita Y, Wright E M, Dholakia K 2013 Opt. Lett. 38 4919 Google Scholar
[26]	Arita Y, Chen M, Wright E M, Dholakia K 2017 J. Opt. Soc. Am. B 34 C14 Google Scholar
[27]	Garcés-Chávez V, McGloin D, Melville H, Sibbett W, Dholakia K 2002 Nature 419 145 Google Scholar
[28]	Christodoulides D N 2008 Nat. Photonics 2 652 Google Scholar
[29]	Baumgartl J, Mazilu M, Dholakia K 2008 Nat. Photonics 2 675 Google Scholar
[30]	Zhu F Q, Huang S J, Shao W, Zhang J, Chen M S, Zhang W B, Zeng J Z 2017 Opt. Commun. 396 50 Google Scholar
[31]	Collins S A 1970 J. Opt. Soc. Am. 60 156 Google Scholar
[32]	Gradshteyn I S, Ryzhik I M 2014 Table of Integrals, Series, and Products (San Diego: Academic Press
[33]	Chen C H, Tai P T, Hsieh W F 2004 Appl. Opt. 43 6001 Google Scholar
[34]	Harada Y, Asakura T 1996 Opt. Commun. 124 529 Google Scholar

Cited By

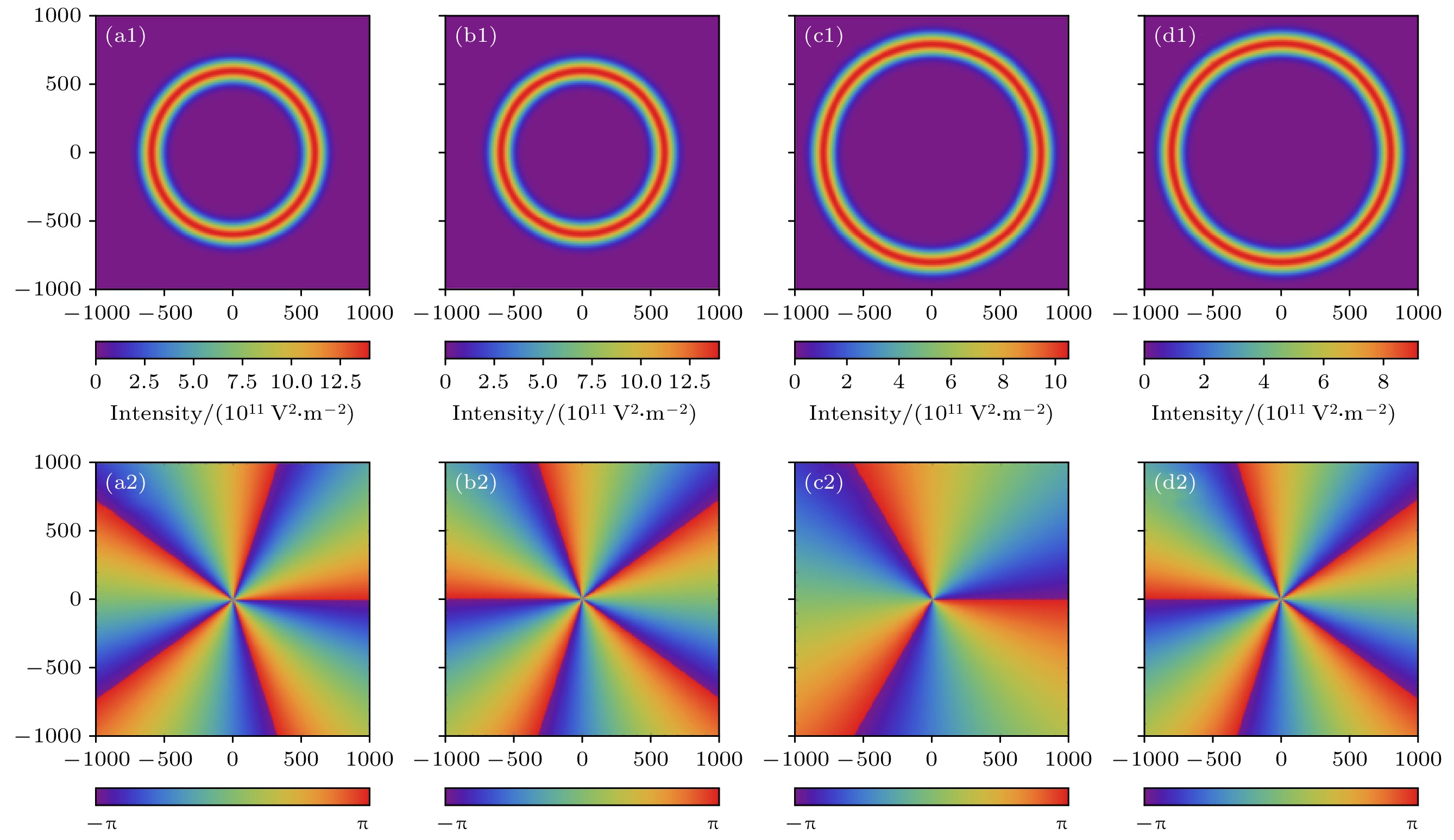
图 1 PVB在源平面处的光强分布图和相位分布图　(a1), (a2) R = 600 μm, m = –5; (b1), (b2) R = 600 μm, m = 5; (c1), (c2) R = 800 μm, m = 3; (d1), (d2) R = 800 μm, m = 5. 其余参数为A₀ = 30 V/μm, w₀= 100 μm

Figure 1. Intensity distribution and phase distribution of PVB at the source plane: (a1), (a2) R = 600 μm, m = –5; (b1), (b2) R = 600 μm, m = 5; (c1), (c2 ) R = 800 μm, m = 3; (d1), (d2) R = 800 μm, m = 5. The rest of the parameters are A₀ = 30 V/μm, w₀= 100 μm.

DownLoad: Full-Size Img PowerPoint

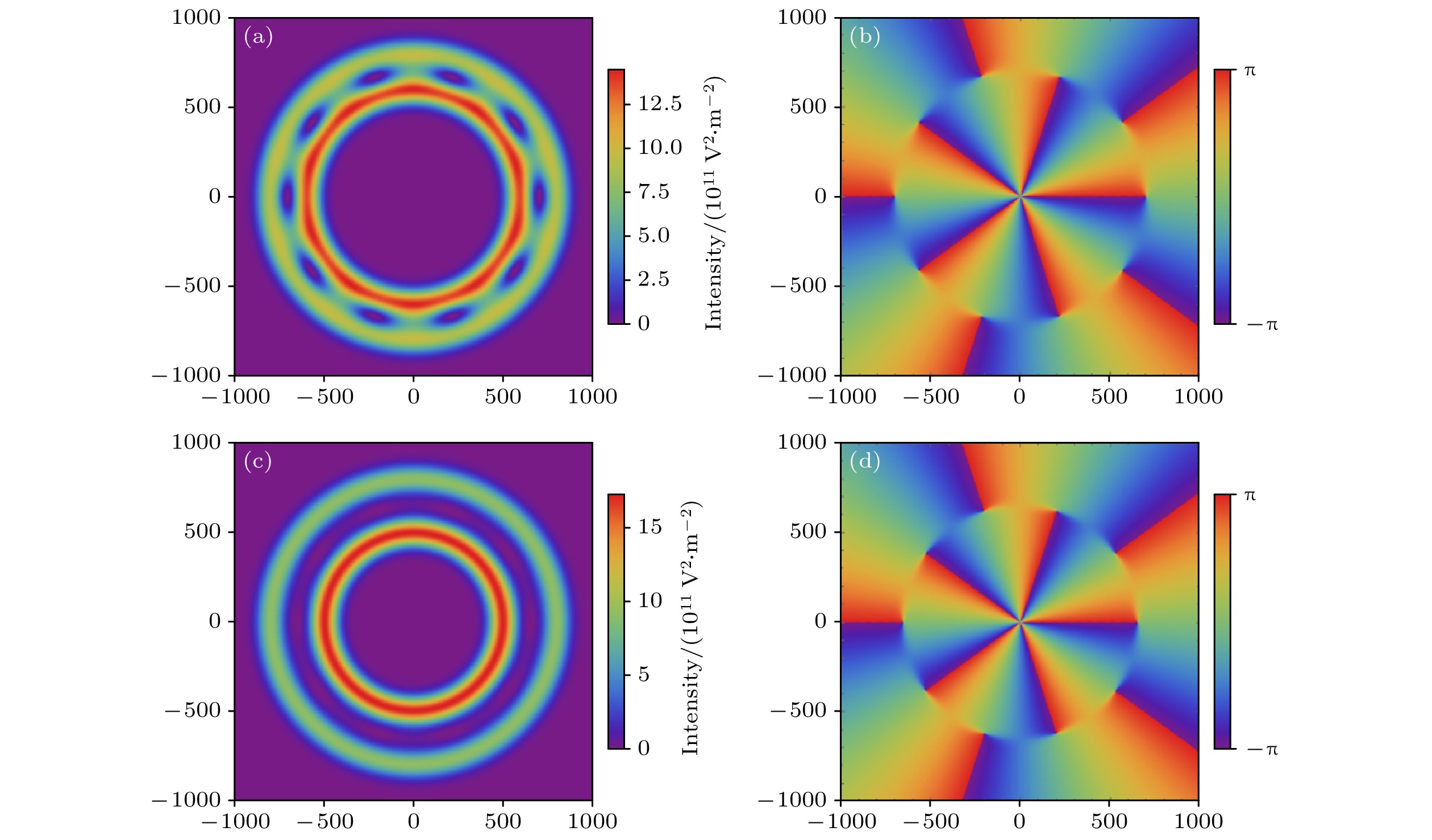
图 2 DR-PVB在源平面处(z = 0)的光强分布图和相位分布图　(a), (b) R₁ = 600 μm, m₁ = –5; R₂ = 800 μm, m₂ = 5; (c), (d) R₁ = 500 μm, m₁ = –5; R₂ = 800 μm, m₂ = 5. 其余参数与图1相同

Figure 2. Intensity distribution and phase distribution of DR-PVB at the source plane (z = 0): (a), (b) R₁ = 600 μm, m₁ = –5; R₂ = 800 μm, m₂ = 5; (c), (d) R₁ = 500 μm, m₁ = –5; R₂ = 800 μm, m₂ = 5. The rest of the parameters are the same as in Fig. 1

DownLoad: Full-Size Img PowerPoint

图 3 系统装置图及微粒受力分析图(右下)

Figure 3. System device diagram and particle force analysis diagram (lower right).

DownLoad: Full-Size Img PowerPoint

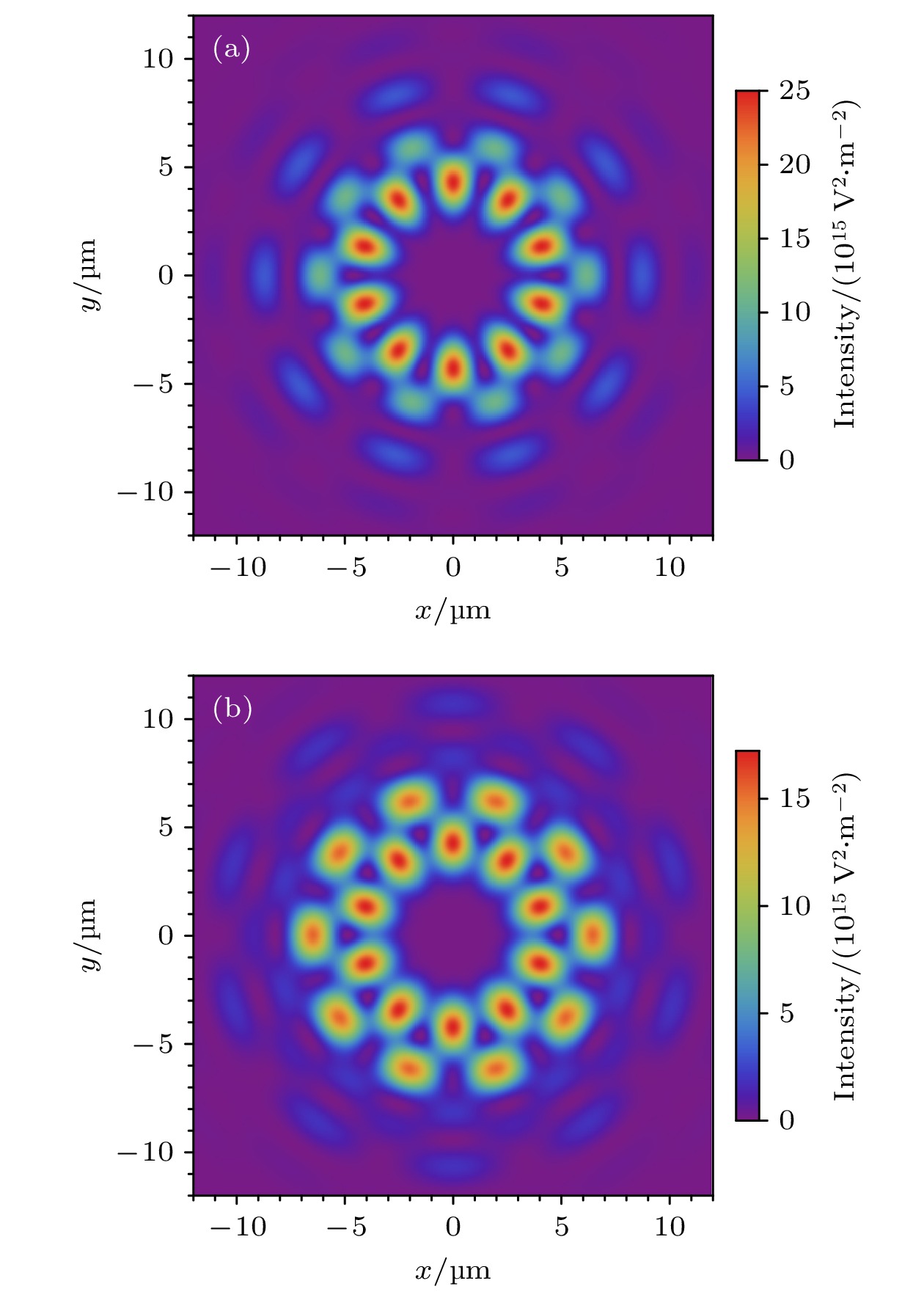
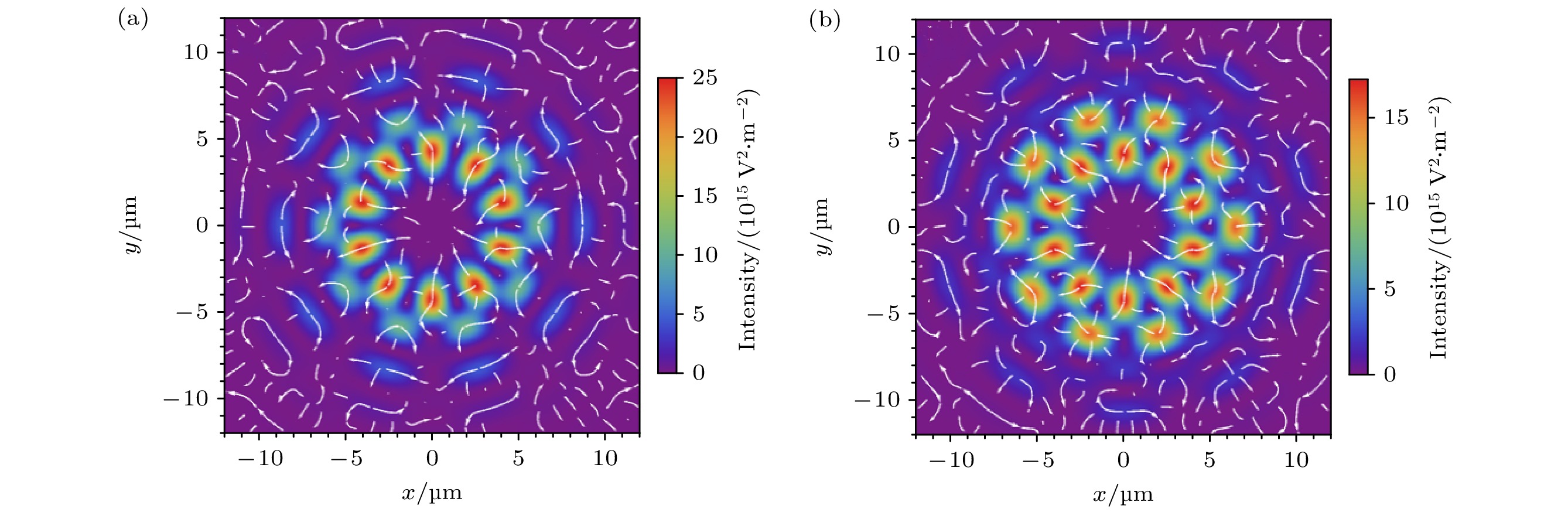
图 4 DR-PVB在焦平面处的光强分布, f = 5 mm, λ₀ = 0.6328 μm　(a) R₁ = 600 μm, m₁ = –5; R₂ = 800 μm, m₂ = 5; (b) R₁ = 500 μm, m₁ = –5; R₂ = 800 μm, m₂ = 5

Figure 4. Intensity distribution of DR-PVB at the focal plane, f = 5 mm, λ₀ = 0.6328 μm: (a) R₁ = 600 μm, m₁ = –5; R₂ = 800 μm, m₂ = 5; (b) R₁ = 500 μm, m₁ = –5; R₂ = 800 μm, m₂ = 5.

DownLoad: Full-Size Img PowerPoint

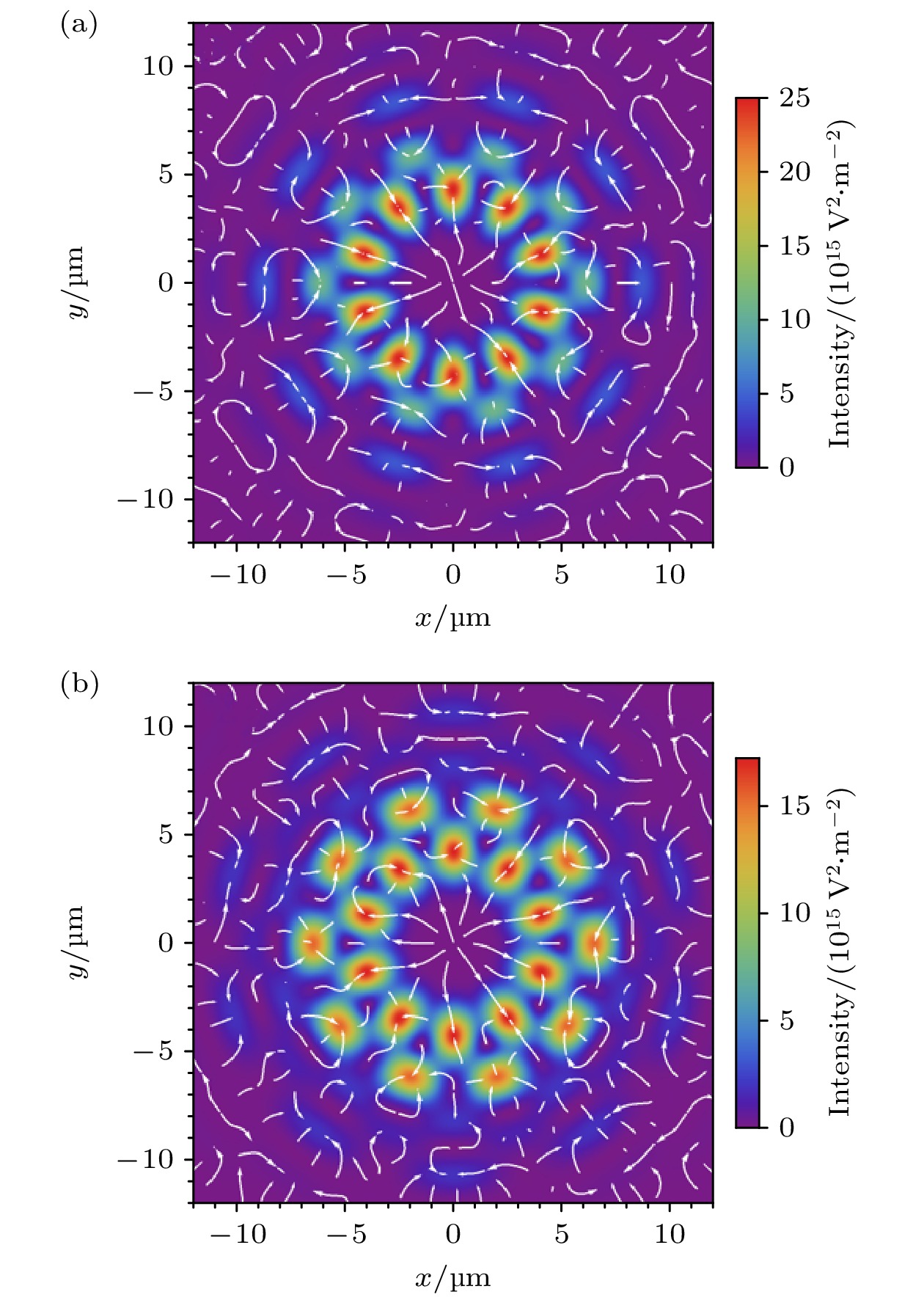
图 5 聚焦DR-PVB对高折射率微粒在x-y平面的光辐射力分布, 箭头表示F_Grad,x和F_Grad,y合力的方向, 背景为焦平面处的光强分布, n_m = 1.332, n_p = 1.592, a = 5 nm　(a) R₁ = 600 μm, R₂ = 800 μm; (b) R₁ = 500 μm, R₂ = 800 μm; 其余参数与图4相同

Figure 5. Distribution of optical radiation force in the x-y plane for high refractive index particles by focused DR-PVB, the arrows indicate the direction of the combined F_Grad,x and F_Grad,y, and the background is the intensity distribution at the focal plane, n_m = 1.332, n_p = 1.592, a = 5 nm: (a) R₁ = 600 μm, R₂ = 800 μm; (b) R₁ = 500 μm, R₂ = 800 μm; the rest of the parameters are the same as in Fig. 4.

DownLoad: Full-Size Img PowerPoint

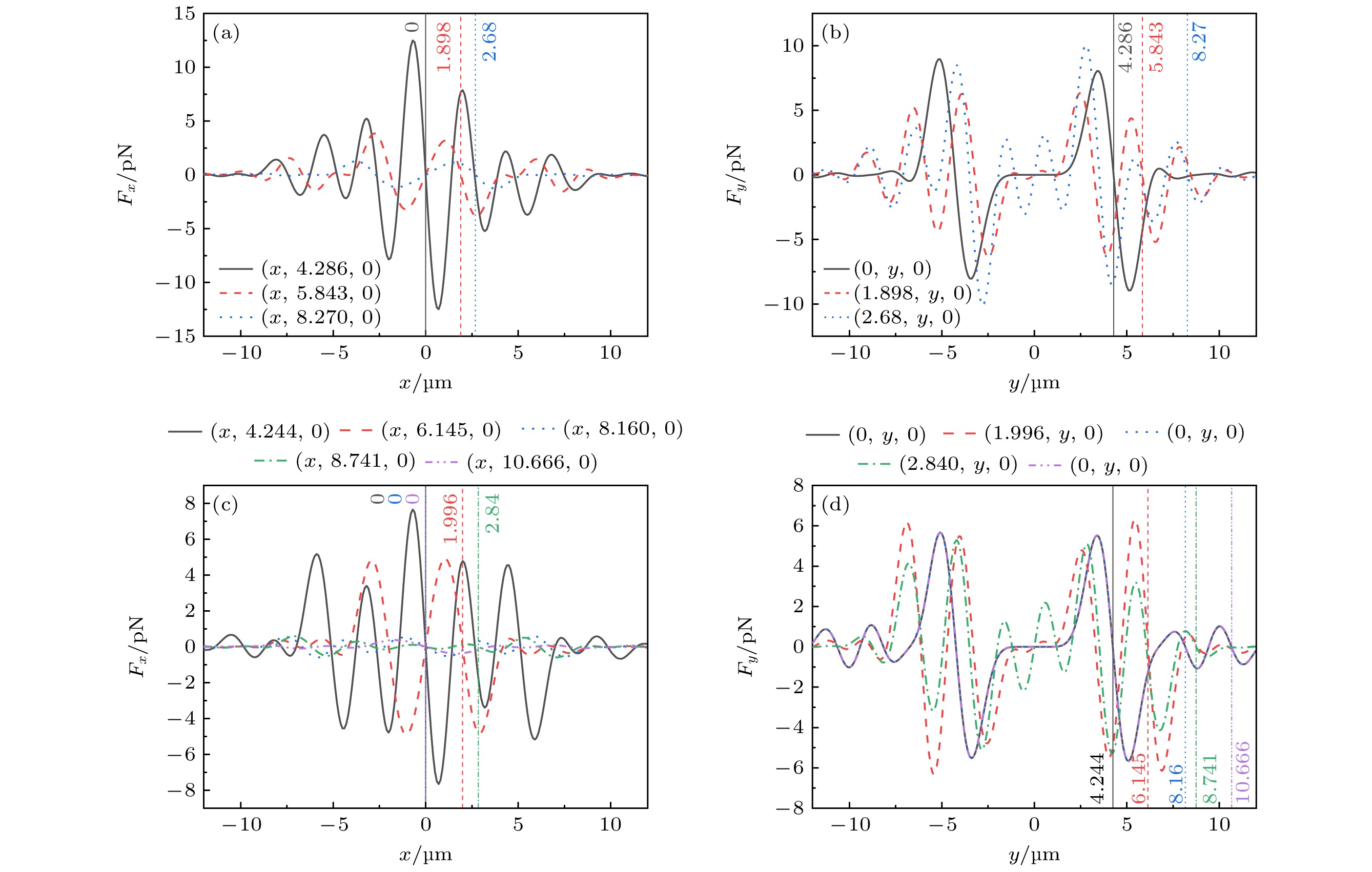
图 6 微粒在不同光强极大值位置处受到的光辐射力随x轴或y轴的变化图　(a), (b) R₁ = 600 μm, R₂ = 800 μm; (c),(d) R₁ = 500 μm, R₂ = 800 μm, 其余参数与图5相同

Figure 6. Plots of the variation of the optical radiative force received by the particles at the positions of different light intensity maxima with the x-axis or y-axis: (a), (b) R₁ = 600 μm, R₂ = 800 μm; (c), (d) R₁ = 500 μm, R₂ = 800 μm, and the rest of the parameters are the same as in Fig. 5.

DownLoad: Full-Size Img PowerPoint

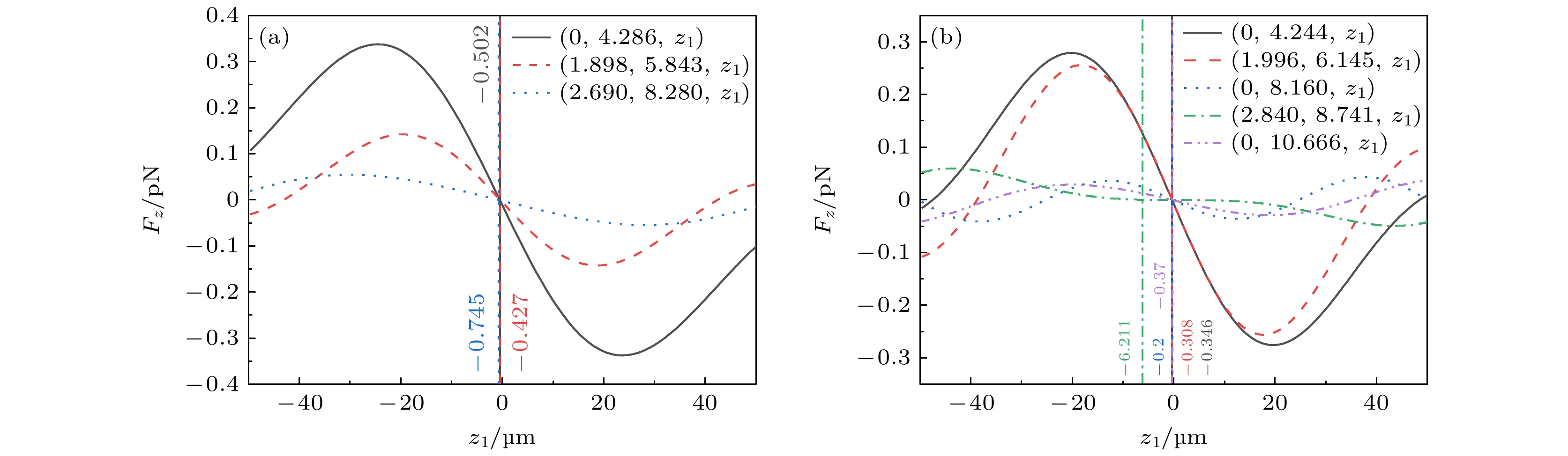
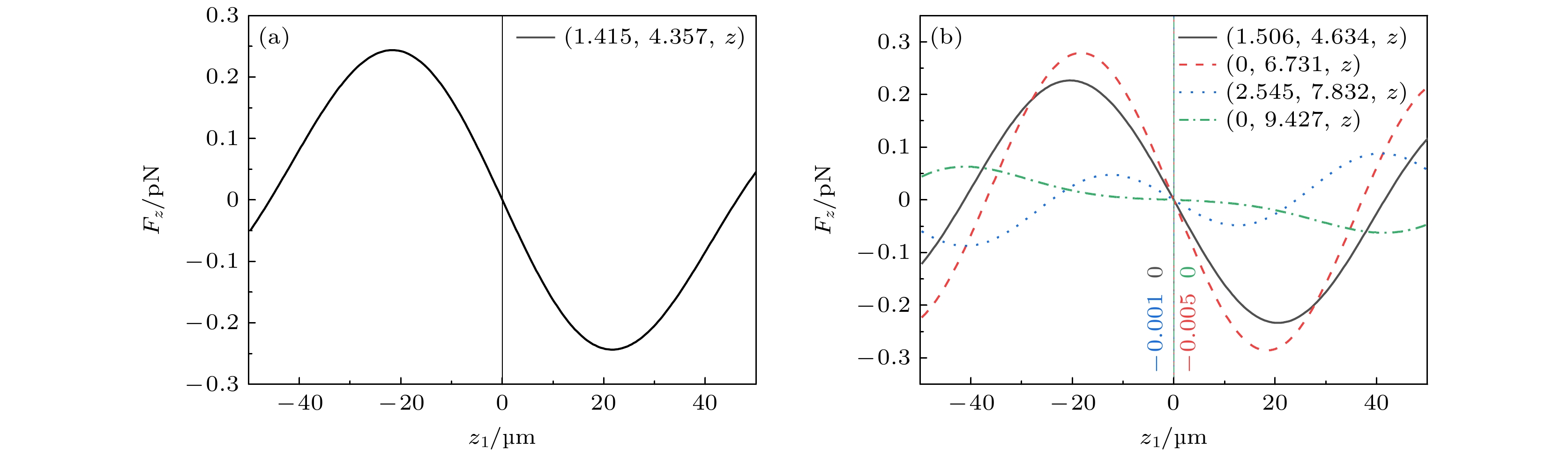
图 7 微粒在点附近受到的z轴方向光辐射力F_z (F_Grad,z -F_scat)随z轴坐标的变化　(a) R₁ = 600 μm, R₂ = 800 μm; (b) R₁ = 500 μm, R₂ = 800 μm, 其余参数与图5相同

Figure 7. Variation of z-axis oriented optical radiation force F_z (F_Grad,z -F_scat) with z-axis coordinates for particles near the point: (a) R₁ = 600 μm, R₂ = 800 μm; (b) R₁ = 500 μm, R₂ = 800 μm, and the rest of the parameters are the same as in Fig. 5.

DownLoad: Full-Size Img PowerPoint

图 8 聚焦DR-PVB对低折射率微粒在x-y平面的光辐射力分布, n_p = 1, n_m = 1.332, a = 5 nm　(a) R₁ = 600 μm, R₂ = 800 μm; (b) R₁ = 500 μm, R₂ = 800 μm; 其余参数与图4相同

Figure 8. Distribution of optical radiation force in the x-y plane for low refractive index particles by focused DR-PVB, n_p = 1, n_m = 1.332, a = 5 nm: (a) R₁ = 600 μm, R₂ = 800 μm; (b) R₁ = 500 μm, R₂ = 800 μm; the rest of the parameters are the same as in Fig. 4

DownLoad: Full-Size Img PowerPoint

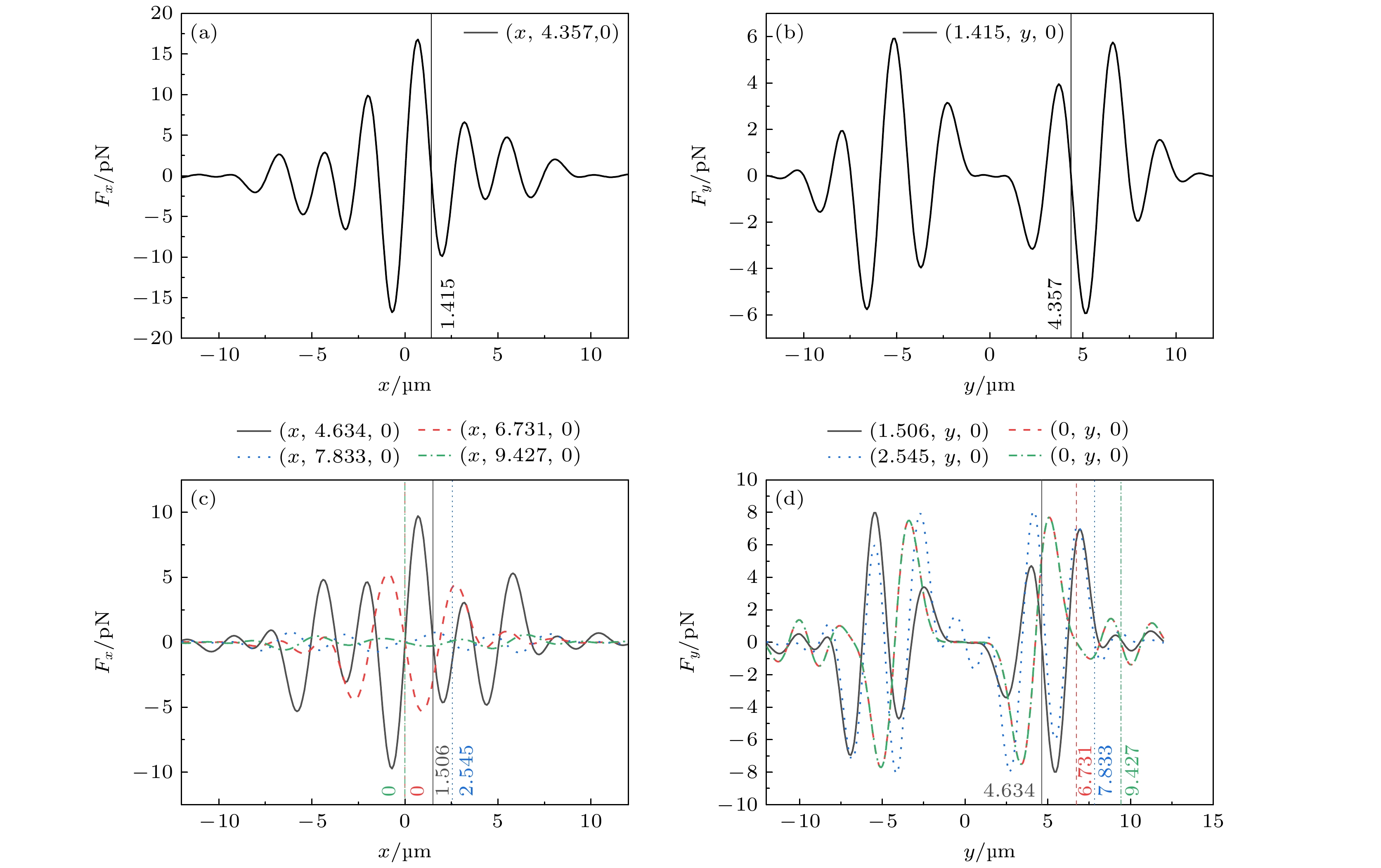
图 9 微粒在光强极小值位置处受到的光辐射力随x轴或y轴的变化图　(a), (b) R₁ = 600 μm, R₂ = 800 μm; (c), (d) R₁ = 500 μm, R₂ = 800 μm, 其余参数与图8相同

Figure 9. Plots of the optical radiation force on particles at the position of the light intensity minima versus the x-axis or y-axis: (a), (b) R₁ = 600 μm, R₂ = 800 μm; (c), (d) R₁ = 500 μm, R₂ = 800 μm, and the rest of the parameters are the same as in Fig. 8.

DownLoad: Full-Size Img PowerPoint

图 10 微粒在光强极小值点附近受到的光辐射力F_z (F_Grad,z –F_scat)随z轴坐标的变化　(a) R₁ = 600 μm, R₂ = 800 μm; (b) R₁ = 500 μm, R₂ = 800 μm, 其余参数与图8相同

Figure 10. Variation of z-axis-directed optical radiation F_z (F_Grad,z –F_scat) received by particles near the point of light intensity minima with z-axis coordinates: (a) R₁ = 600 μm, R₂ = 800 μm; (b) R₁ = 500 μm, R₂ = 800 μm, and the rest of the parameters are the same as in Fig. 8.

DownLoad: Full-Size Img PowerPoint

图 11 在不同R组合情况下, 高(n_p = 1.592)、低(n_p = 1)折射率粒子所受到的最大轴向梯度力F_{Grad,z max}与其他力(F_B, F_Scat)的比值随粒子半径的变化图, n_m = 1.332　(a) R₁ = 600 μm, R₂ = 800 μm; (b) R₁ = 500 μm, R₂ = 800 μm, 其余参数与图4相同

Figure 11. Plots of the ratio of the maximum axial gradient force F_{Grad,z max} to the other forces (F_B, F_Scat) with respect to the particle radius for high (n_p = 1.592) and low (n_p = 1) refractive index particles for different R combinations, n_m = 1.332: (a) R₁ = 600 μm, R₂ = 800 μm; (b) R₁ = 500 μm, R₂ = 800 μm, and the rest of the parameters are the same as in Fig. 4.

DownLoad: Full-Size Img PowerPoint

表 1 图5中光强极大值位置

Table 1. Location of light intensity maxima in Fig. 5.

不同R组合	焦平面处光强极大值位置
R₁ = 600 μm, R₂ = 800 μm	第1组	(0, ±4.286, 0), (±2.519, ±3.467, 0), (±4.076, ±1.324, 0)
	第2组	(±1.898, ±5.843, 0), (±4.97, ±3.611, 0), (±6.144, 0, 0)
	第3组	(±2.69, ±8.27, 0), (±7.043, ±5.117, 0), (±8.706, 0, 0)
R₁ = 500 μm, R₂ = 800 μm	第1组	(0, ±4.244, 0), (±2.494, ±3.433, 0), (±4.036, ±1.311, 0)
	第2组	(±1.996, ±6.145, 0), (±5.227, ±3.798, 0), (±6.461, 0, 0)
	第3组	(0, ±8.160, 0), (±4.796, ±6.601, 0), (±7.760, ±2.521, 0)
	第4组	(±2.839, ±8.740, 0), (±7.435, ±5.401, 0), (±9.190, 0, 0)
	第5组	(0, ±10.658, 0), (±10.136, ±3.293, 0), (±6.264, ±8.622, 0)

DownLoad: CSV

表 2 不同R组合情况下聚焦DR-PVB对高折射率粒子的俘获位置

Table 2. Capture positions of high refractive index particles by focused DR-PVB for different R combinations.

不同R组合		稳定俘获位置
R₁ = 600 μm, R₂ = 800 μm	第1组	(0, ±4.286, –0.502) (±2.519, ±3.467, –0.502) (±4.076, ±1.324, –0.502)
	第2组	(±1.898, ±5.843, –0.427) (±4.97, ±3.611, –0.427) (±6.144, 0, –0.427)
	第3组	(±2.69, ±8.27, –0.745) (±7.043, ±5.117, –0.745) (±8.706, 0, –0.745)
R₁ = 500 μm, R₂ = 800 μm	第1组	(0, ±4.244, –0.346) (±2.49, ±3.43, –0.346) (±4.03, ±1.31, –0.346)
	第2组	(±1.99, ±6.14, –0.308) (±5.22, ±3.79, –0.308) (±6.46, 0, –0.308)
	第3组	(0, ±8.160, –0.2) (±4.796, ±6.601, –0.2) (±7.760, ±2.521, –0.2)
	第4组	(0, ±10.658, –0.37) (±10.136, ±3.293, –0.37) (±6.264, ±8.622, –0.37)

DownLoad: CSV

表 3 图8中光强极小值位置

Table 3. Location of light intensity minima in Fig. 8.

不同R组合	焦平面处光强极小值位置
R₁ = 600 μm, R₂ = 800 μm	第1组	(±1.415, ±4.357, 0), (±3.706, ±2.693, 0), (±4.581, 0, 0)
R₁ = 500 μm, R₂ = 800 μm	第1组	(±1.505, ±4.634, 0) (±3.942, ±2.864, 0) (±4.872, 0, 0)
	第2组	(0, ±6.731, 0) (±3.956, ±5.445, 0) (±6.401, ±2.08, 0)
	第3组	(±2.545, ±7.833, 0) (±6.663, ±4.840, 0) (±8.236, 0, 0)
	第4组	(0, ±9.427, 0) (±5.541, ±7.627, 0) (±8.966, ±2.913, 0)

DownLoad: CSV

表 4 不同R组合情况下聚焦DR-PVB对低折射率粒子的俘获情况

Table 4. Capture positons of low refractive index particles by focused DR-PVB for different R combinations.

不同R组合		稳定俘获位置
R₁ = 600 μm, R₂ = 800 μm	第1组	(±1.415, ±4.357, 0) (±3.706, ±2.693, 0) (±4.581, 0, 0)
R₁ = 500 μm, R₂ = 800 μm	第1组	(±1.505, ±4.634, 0) (±3.942, ±2.864, 0) (±4.872, 0, 0)
	第2组	(0, ±6.731, –0.005) (±3.956, ±5.445, –0.005) (±6.401, ±2.08, –0.005)
	第3组	(±2.545, ±7.833, –0.001) (±6.663, ±4.840, –0.001) (±8.236, 0, –0.001)
	第4组	(0, ±9.427, 0) (±5.541, ±7.627, 0) (±8.966, ±2.913, 0)

DownLoad: CSV

表 5 不同R组合情况下聚焦DR-PVB所能俘获微粒的尺寸范围

Table 5. Size range of particles captured by focused DR-PVB with different radius combinations.

不同半径组合对不同粒子的俘获半径范围	高折射率粒子	低折射率粒子
R₁ = 600 μm, R₂ = 800 μm	0.44 nm < a < 22.07 nm	0.50 nm < a < 23.35 nm
R₁ = 500 μm, R₂ = 800 μm	0.47 nm < a < 23.53 nm	0.47 nm < a < 22.68 nm

DownLoad: CSV

map

[1]	Ashkin A, Dziedzic J M, Bjorkholm J E, Chu S 1986 Opt. Lett. 11 288 Google Scholar
[2]	Ciarlo A, Pastore R, Greco F, Sasso A, Pesce G 2023 Sci. Rep. 13 7408 Google Scholar
[3]	Chen H C, Cheng C J 2022 Appl. Sci. 12 10244 Google Scholar
[4]	Bustamante C J, Chemla Y R, Liu S, Wang M D 2021 Nat. Rev. Methods Primers 1 25 Google Scholar
[5]	Anderegg L, Cheuk L W, Bao Y, Burchesky S, Ketterle W, Ni K K, Doyle J M 2019 Science 365 1156 Google Scholar
[6]	Tian Y H, Wang L L, Duan G Y, Yu L 2021 Opt. Commun. 485 126712 Google Scholar
[7]	Munoz-Perez F M, Ferrando V, Furlan W D, Castro-Palacio J C, Arias-Gonzalez J R, Monsoriu J A 2023 iScience 26 107987 Google Scholar
[8]	闫玮植, 范青, 杨鹏飞, 李刚, 张鹏飞, 张天才 2023 72 114202 Google Scholar Yan W Z, Fan Q, Yang P F, Li G, Zhang P F, Zhang T C 2023 Acta Phys. Sin. 72 114202 Google Scholar
[9]	Santamato E, Daino B, Romagnoli M, Settembre M, Shen Y 1991 J. Opt. 22 158
[10]	Bonin K D, Kourmanov B, Walker T G 2002 Opt. Express 10 984 Google Scholar
[11]	Pedaci F, Huang Z, Van Oene M, Barland S, Dekker N H 2011 Biophys. J. 100 10a
[12]	Grier D G 2003 Nature 424 810 Google Scholar
[13]	Huang S J, Miao Z, He C, Pang F F, Li Y C, Wang T Y 2016 Opts. Lasers. Eng. 78 132 Google Scholar
[14]	Andrade U M S, Garcia A M, Rocha M S 2021 Appl. Opt. 60 3422 Google Scholar
[15]	Ostrovsky A S, Rickenstorff-Parrao C, Arrizon V 2013 Opt. Lett. 38 534 Google Scholar
[16]	Vaity P, Rusch L 2015 Opt. Lett. 40 597 Google Scholar
[17]	Chen Y, Fang Z X, Ren Y X, Gong L, Lu R D 2015 Appl. Opt. 54 8030 Google Scholar
[18]	Liu X J, Li Y L, Yao G P, Li C X, Fang B, Tang Y, Hong Z, Jing X F 2024 Chin. J. Phys. 91 828 Google Scholar
[19]	Ma H X, Li X Z, Tai Y P, Li H H, Wang J G, Tang M M, Tang J, Wang Y S, Nie Z G 2017 Ann. Phys. 529 1700285 Google Scholar
[20]	Das B K, Granados C, Krüger M, Ciappina M F 2024 Opt. Commun. 570 130918 Google Scholar
[21]	Chen M Z, Mazilu M, Arita Y 2015 Opt. Rev. 22 162 Google Scholar
[22]	Tkachenko G, Chen M Z, Dholakia K, Mazilu M 2017 Optica 4 330 Google Scholar
[23]	Liang Y S, Lei M, Yan S H, Li M M, Cai Y A, Wang Z J, Yu X H, Yao B L 2018 Appl. Opt. 57 79 Google Scholar
[24]	Wang S L, Xu J P, Yang Y P, Cheng M J 2024 Opt. Commun. 556 130258 Google Scholar
[25]	Chen M, Mazilu M, Arita Y, Wright E M, Dholakia K 2013 Opt. Lett. 38 4919 Google Scholar
[26]	Arita Y, Chen M, Wright E M, Dholakia K 2017 J. Opt. Soc. Am. B 34 C14 Google Scholar
[27]	Garcés-Chávez V, McGloin D, Melville H, Sibbett W, Dholakia K 2002 Nature 419 145 Google Scholar
[28]	Christodoulides D N 2008 Nat. Photonics 2 652 Google Scholar
[29]	Baumgartl J, Mazilu M, Dholakia K 2008 Nat. Photonics 2 675 Google Scholar
[30]	Zhu F Q, Huang S J, Shao W, Zhang J, Chen M S, Zhang W B, Zeng J Z 2017 Opt. Commun. 396 50 Google Scholar
[31]	Collins S A 1970 J. Opt. Soc. Am. 60 156 Google Scholar
[32]	Gradshteyn I S, Ryzhik I M 2014 Table of Integrals, Series, and Products (San Diego: Academic Press
[33]	Chen C H, Tai P T, Hsieh W F 2004 Appl. Opt. 43 6001 Google Scholar
[34]	Harada Y, Asakura T 1996 Opt. Commun. 124 529 Google Scholar

[1]	ZHANG Shenglan, TIAN Ximin, XU Junwei, XU Yaning, LI Liang, LIU Jielong. Generation and independent-manipulation of multi-channel high-capacity perfect vector vortex beams based on geometric metasurfaces. Acta Physica Sinica, 2025, 74(6): 064201. doi: 10.7498/aps.74.20241725
[2]	Hadiqa⋅ Abdugopur, Tan Le-Tao, Yu Tao, Xie Wen-Ke, Liu Jing, Shao Zheng-Zheng. Study of off-axis incident rotational speed measurement based on coherent synthetic vortex beams. Acta Physica Sinica, 2024, 73(16): 168701. doi: 10.7498/aps.73.20240655
[3]	Liu Zhe, Wang Lei-Lei, Shi Peng-Peng, Cui Hai-Hang. Experiments and analytical solutions of light driven flow in nanofluid droplets. Acta Physica Sinica, 2020, 69(6): 064701. doi: 10.7498/aps.69.20191508
[4]	Zhong Zhe-Qiang, Mu Jie, Wang Xiao, Zhang Bin. Analysis of coherent combination characteristics of beam array via tight focusing. Acta Physica Sinica, 2020, 69(9): 094204. doi: 10.7498/aps.69.20200034
[5]	Zhou Ning, Zhang Lan-Zhi, Li Dong-Wei, Chang Jun-Wei, Wang Bi-Yi, Tang Lei, Lin Jing-Quan, Hao Zuo-Qiang. Filamentation and supercontinuum emission with flattened femtosecond laser beam by use of microlens array in fused silica. Acta Physica Sinica, 2018, 67(17): 174205. doi: 10.7498/aps.67.20180306
[6]	Shi Jian-Zhen, Yang Shen, Zou Ya-Qi, Ji Xian-Ming, Yin Jian-Ping. Generation of vortex beams by the four-step phase plates. Acta Physica Sinica, 2015, 64(18): 184202. doi: 10.7498/aps.64.184202
[7]	Wang Lin, Yuan Cao-Jin, Nie Shou-Ping, Li Chong-Guang, Zhang Hui-Li, Zhao Ying-Chun, Zhang Xiu-Ying, Feng Shao-Tong. Measuring topology charge of vortex beam using digital holography. Acta Physica Sinica, 2014, 63(24): 244202. doi: 10.7498/aps.63.244202
[8]	Wang Hui, Ding Pan-Feng, Pu Ji-Xiong. Tight focusing properties of off-center Gaussian vortex beams. Acta Physica Sinica, 2014, 63(21): 214202. doi: 10.7498/aps.63.214202
[9]	Huang Su-Juan, Gu Ting-Ting, Miao Zhuang, He Chao, Wang Ting-Yun. Experimental study on multiple-ring vortex beams. Acta Physica Sinica, 2014, 63(24): 244103. doi: 10.7498/aps.63.244103
[10]	Zhang Jin, Zhou Xin-Xing, Luo Hai-Lu, Wen Shuang-Chun. Cross polarization effects of vortex beam in reflection. Acta Physica Sinica, 2013, 62(17): 174202. doi: 10.7498/aps.62.174202
[11]	Tang Bi-Hua, Luo Ya-Mei, Jiang Yun-Hai, Chen Shu-Qiong. Study on the characteristics of cosh-Gaussian vortex beams in the far field. Acta Physica Sinica, 2013, 62(13): 134202. doi: 10.7498/aps.62.134202
[12]	Ding Pan-Feng, Pu Ji-Xiong. Analysis of the facula of partially coherent vortex beam in propagation. Acta Physica Sinica, 2012, 61(17): 174201. doi: 10.7498/aps.61.174201
[13]	Fang Gui-Juan, Sun Shun-Hong, Pu Ji-Xiong. Experimental study on fractional double-vortex beams. Acta Physica Sinica, 2012, 61(6): 064210. doi: 10.7498/aps.61.064210
[14]	Zhou Guo-Quan. The beam propagation factor and the kurtosis parameter of a Gaussian vortex beam. Acta Physica Sinica, 2012, 61(17): 174102. doi: 10.7498/aps.61.174102
[15]	Ding Pan-Feng, Pu Ji-Xiong. Propagation of Laguerre-Gaussian vortex beam. Acta Physica Sinica, 2011, 60(9): 094204. doi: 10.7498/aps.60.094204
[16]	Cheng Ke, Zhang Hong-Run, Lü Bai-Da. Coherence vortex properties of partially coherent vortex beams. Acta Physica Sinica, 2010, 59(1): 246-255. doi: 10.7498/aps.59.246
[17]	Chen Xiao-Yi, Li Hai-Xia, Song Hong-Sheng, Teng Shu-Yun, Cheng Chuan-Fu, Liu Man. Measurement of orbital angular momentum of Laguerre-Gaussian beam by using phase vortices of interference fields. Acta Physica Sinica, 2010, 59(12): 8490-8498. doi: 10.7498/aps.59.8490
[18]	Li Yang-Yue, Chen Zi-Yang, Liu Hui, Pu Ji-Xiong. Generation and interference of vortex beams. Acta Physica Sinica, 2010, 59(3): 1740-1748. doi: 10.7498/aps.59.1740
[19]	Jiang Yun-Feng, Lu Xuan-Hui, Zhao Cheng-Liang. Radiation force of highly focused cosine-Gaussian beam on a particle in the Rayleigh scattering regime. Acta Physica Sinica, 2010, 59(6): 3959-3964. doi: 10.7498/aps.59.3959
[20]	Yan Hong-Wei, Cheng Ke, Lü Bai-Da. Composite optical vortices formed by two flattened Gaussian vortex beams and their propagation in free space. Acta Physica Sinica, 2008, 57(9): 5542-5549. doi: 10.7498/aps.57.5542

Catalog

Vol. 74, Issue 8 - 2025-04-20

Metrics

Abstract views: 432
PDF Downloads: 9
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板