-
In this work, a one-dimensional interacting anyon model with a Stark potential in the finite size is studied. Using the fractional Jordan Wigner transformation, the anyons in the one-dimensional system are mapped onto bosons, which are described by the following Hamiltonian: $ \begin{aligned} \hat{H}^{\text{boson}}=-J\sum_{j=1}^{L-1}\left( \hat{b}_{j}^{\dagger}\hat{b}_{j+1}{\mathrm{e}}^{{\mathrm{i}}\theta \hat{n}_{j}}+{\mathrm{h.c.}}\right)+\frac{U}{2}\sum_{j=1}^{L}\hat{n}_{j}\left( \hat{n}_{j}-1\right)+\sum_{j=1}^{L}{h}_{j}\hat{n}_{j},\;\;\;\;\;\;\;\;\;\end{aligned}$where θ is the statistical angle, and the on-site potential is $h_{j}=-\gamma\left(j-1\right) +\alpha\Big( \dfrac{j-1}{L-1}\Big)^{2}$ with γ representing the strength of the Stark linear potential and α denoting the strength of the nonlinear part. Using the exact diagonalization method, the spectral statistics, half-chain entanglement entropy and particle imbalance are numerically analyzed to investigate the onset of many-body localization (MBL) in this interacting anyon system, induced by increasing the linear potential strength. As the Stark linear potential strength increases, the spectral statistics transforms from a Gaussian ensemble into a Poisson ensemble. In the ergodic phase, except for θ = 0 and π, where the average value of the gap-ratio parameter $\left\langle r\right\rangle\approx 0.53$, due to the destruction of time reversal symmetry, the Hamiltonian matrix becomes a complex Hermit matrix and $\left\langle r\right\rangle\approx 0.6$. In the MBL phase, $\left\langle r\right\rangle\approx 0.39$, which is independent of θ. However, in the intermediate γ regime, the value of $\left\langle r\right\rangle$ strongly depends on the choice of θ. The average of the half-chain entanglement entropy transforms from a volume law into an area law, which allows us to construct a θ-dependent MBL phase diagram. In the ergodic phase, the entanglement entropy S(t) of the half chain increases linearly with time. In the MBL phase, S(t) grows logarithmically with time, reaching a stable value that depends on the anyon statistical angle. The localization of particles in a quench dynamics can provide the evidence for the breakdown of ergodicity and is experimentally observable. It is observed that with the increase of γ, the even-odd particle imbalance changes from zero to non-zero values in the long-time limit. In the MBL phase, the long-time average value of the imbalance is dependent on the anyon statistical angle θ. From the Hamiltonian $\hat{H}^{\text{boson}}$, it can be inferred that the statistical behavior of anyon system equally changes the hopping interactions in boson system, which is a many-body effect. By changing the statistical angle θ, the many-body interactions in the system are correspondingly changed. And the change of the many-body interaction strength affects the occurrence of the MBL transition, which is also the reason for MBL transition changing with the anyon statistical angle θ. Our results provide new insights into the study of MBL in anyon systems and whether such phenomena persist in the thermodynamic limit needs further discussing in the future. -
Keywords:
- anyon /
- Stark potential /
- many-body localization
[1] Schreiber M, Hodgman S S, Bordia P, Lüschen H P, Fischer M H, Vosk R, Altman E, Schneider U, Bloch I 2015 Science 349 842
Google Scholar
[2] Bordia P, Lüschen H P, Hodgman S S, Schreiber M, Bloch I, Schneider U 2016 Phys. Rev. Lett. 116 140401
Google Scholar
[3] Smith J, Lee A, Richerme P, Neyenhuis B, Hess P W, Hauke P, Heyl M, Huse D A, Monroe C 2016 Nat. Phys. 12 907
Google Scholar
[4] Xu K, Chen J J, Zeng Y, Zhang Y R, Song C, Liu W X, Guo Q J, Zhang P F, Xu D, Deng H, Huang K Q, Wang H, Zhu X B, Zheng D N, Fan H 2018 Phys. Rev. Lett. 120 050507
Google Scholar
[5] Guo Q, Cheng C, Sun Z H, Song Z, Li H, Wang Z, Ren W, Dong H, Zheng D, Zhang Y R, Mondaini R, Fan H, Wang H 2021 Nat. Phys. 17 234
Google Scholar
[6] Guo Q J, Cheng C, Li H K, Xu S B, Zhang P F, Wang Z, Song C, Liu W X, Ren W H, Dong H, Mondaini R, Wang H 2021 Phys. Rev. Lett. 127 240502
Google Scholar
[7] Morong W, Liu F, Becker P, Collins K S, Feng L, Kyprianidis A, Pagano G, You T, Gorshkov A V, Monroe C 2021 Nature 599 393
Google Scholar
[8] Scherg S, Kohlert T, Sala P, Pollmann F, Hebbe Madhusudhana B, Bloch I, Aidelsburger M 2021 Nat. Commun. 12 4490
Google Scholar
[9] Liu J H, Xu Z H 2023 Phys. Rev. B 108 184205
Google Scholar
[10] Leinaas J M, Myrheim J 1977 Nuovo Cimento Soc. Ital. Fis. B 37 1
Google Scholar
[11] Tsui D C, Stormer H L, Gossard A C 1982 Phys. Rev. Lett. 48 1559
Google Scholar
[12] Laughlin R B 1983 Phys. Rev. Lett. 50 1395
Google Scholar
[13] Nayak C, Simon S H, Stern A, Freedman M, Das Sarma S 2008 Rev. Mod. Phys. 80 1083
Google Scholar
[14] Kitaev A 2006 Ann. Phys. 321 2
Google Scholar
[15] Stern A 2008 Ann. Phys. 323 204
Google Scholar
[16] Haldane F D M 1991 Phys. Rev. Lett. 67 937
Google Scholar
[17] Vitoriano C, Coutinho-Filho M D 2009 Phys. Rev. Lett. 102 146404
Google Scholar
[18] Keilmann T, Lanzmich S, McCulloch I, Roncaglia M 2011 Nat. Commun. 2 361
Google Scholar
[19] Greschner S, Santos L 2015 Phys. Rev. Lett. 115 053002
Google Scholar
[20] Sträter C, Srivastava S C L, Eckardt A 2016 Phys. Rev. Lett. 117 205303
Google Scholar
[21] Zuo Z W, Li G L, Li L 2018 Phys. Rev. B 97 115126
Google Scholar
[22] Liu F, Garrison J R, Deng D L, Gong Z X, Gorshkov A V 2018 Phys. Rev. Lett. 121 250404
Google Scholar
[23] Zhang G Q, Zhang D W, Li Z, Wang Z D, Zhu S L 2020 Phys. Rev. B 102 054204
Google Scholar
[24] Wang Y Y, Sun Z H, Fan H 2021 Phys. Rev. B 104 205122
Google Scholar
[25] 王利, 贾丽芳, 张云波 2022 71 130501
Google Scholar
Wang L, Jia L F, Zhang Y B 2022 Acta. Rhys. Sin. 71 130501
Google Scholar
[26] 刘敬鹄, 徐志浩 2024 73 077202
Google Scholar
Liu J H, Xu Z H 2024 Acta. Rhys. Sin. 73 077202
Google Scholar
[27] Wu H, Vallières M, Feng D H, Sprung D W L 1990 Phys. Rev. A 42 1027
Google Scholar
[28] Hamazaki R, Kawabata K, Kura N, Ueda M 2020 Phys. Rev. Res. 2 023286
Google Scholar
[29] Oganesyan V, Huse D A 2007 Phys. Rev. B 75 155111
Google Scholar
[30] Serbyn M, Papić Z, Abanin D A 2013 Phys. Rev. Lett. 111 127201
Google Scholar
[31] Van Nieuwenburg E, Baum Y, Refael G 2019 Proc. Natl. Acad. Sci. U.S.A. 116 9269
Google Scholar
-
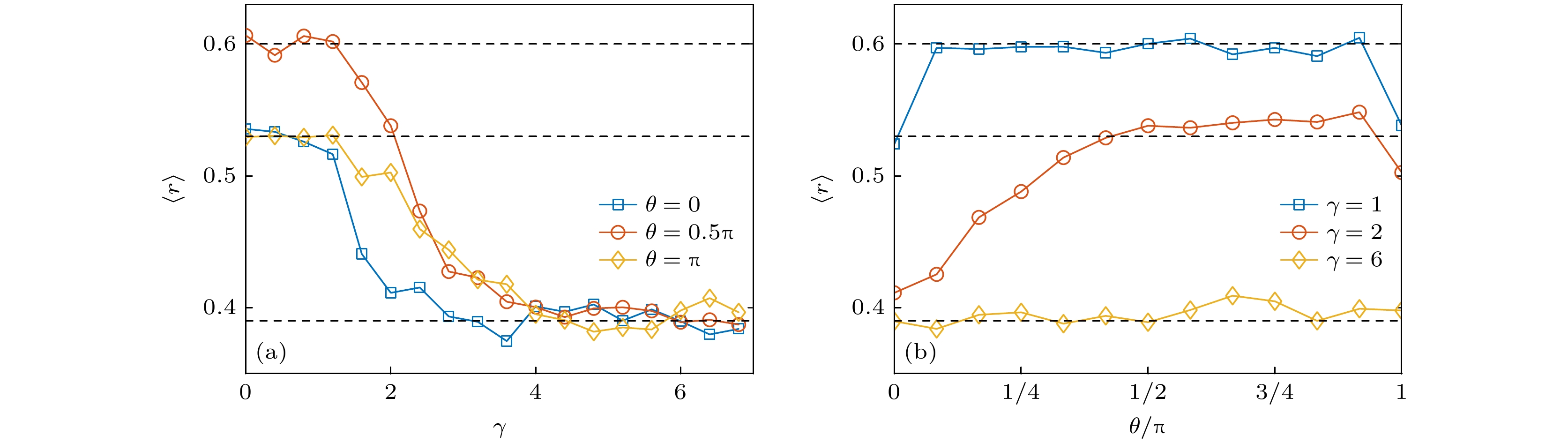
图 1 (a) 不同统计角θ下平均能级差比率$ \langle r\rangle $与Stark线性势强度γ的关系; (b) 平均能级差比率$ \langle r\rangle $在不同线性势强度γ下与统计角θ的关系, 其中L = 12
Figure 1. (a) The mean value of the gap-ratio parameter $ \langle r\rangle $ as a function of the Stark linear potential strength γ for different statistical angles θ; (b) $ \langle r\rangle $ as a function of the statistical angle θ for different γ. Here, L = 12.
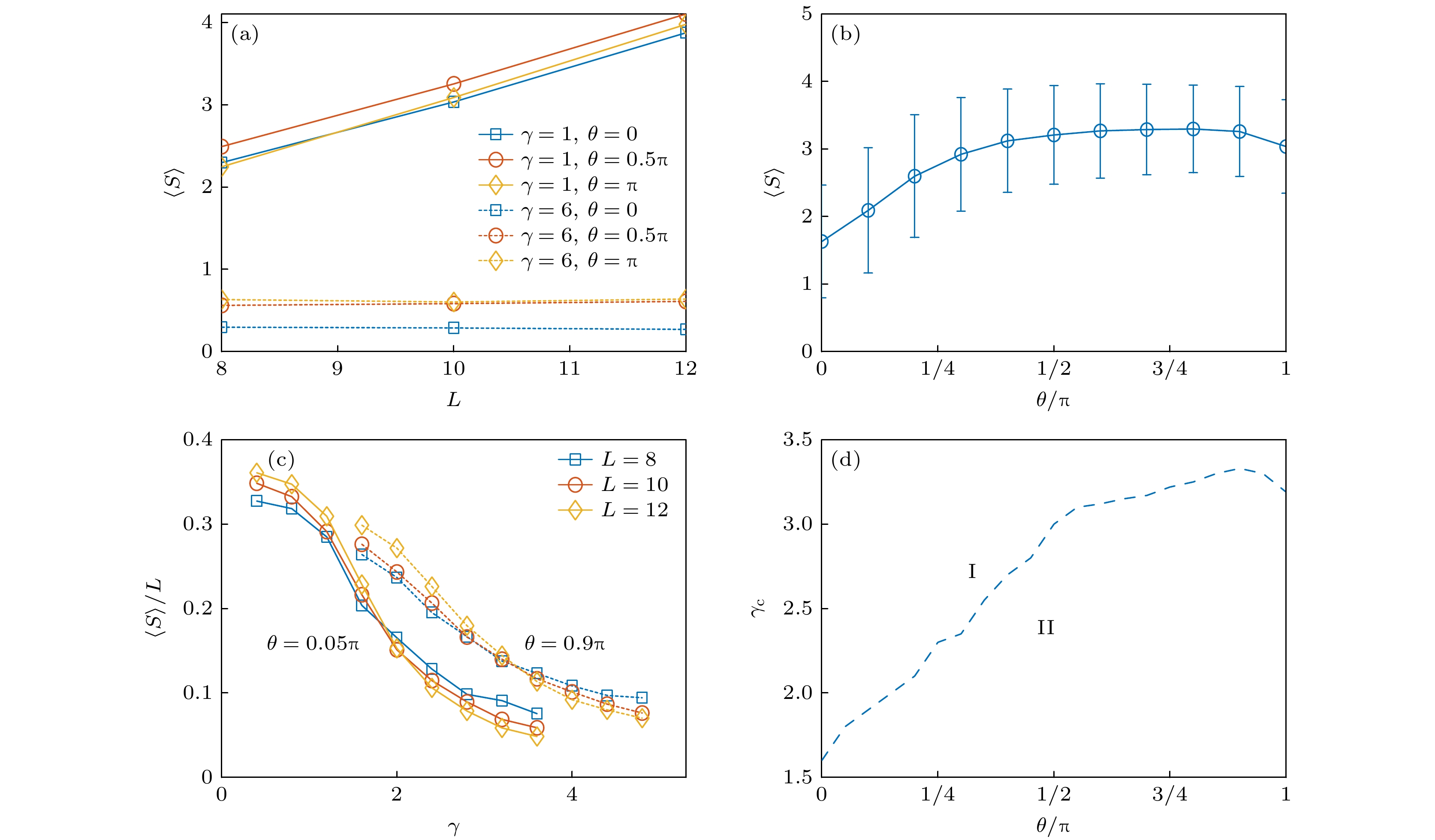
图 2 (a) 线性势强度分别为$ \gamma=1 $和6时, 不同统计角的平均半链纠缠熵与系统尺寸L的关系图; (b)当$ L=12 $, $ \gamma=2 $时, 平均半链纠缠熵随统计角θ的变化趋势; (c) 任意子统计角分别为$ \theta=0.05{\mathrm{\pi}} $和$ 0.9\pi $时, 不同尺寸的平均半链纠缠熵$ \langle S\rangle /L $随线性势强度γ的变化; (d) 多体局域化转变点$ \gamma_{\mathrm{c}} $随着统计角θ变化的情况. I和II区域分别为多体局域相和遍历相
Figure 2. (a) The average half-chain entanglement entropy $ \langle S\rangle $ as a function of the system size L for different θ with $ \gamma=1 $ and $ 6 $; (b) $ \langle S\rangle $ as a function of θ with $ \gamma=2 $ and $ L=12 $; (c) $ \langle S\rangle /L $ as a function of γ for $ \theta=0.05\pi $ and $ 0.9\pi $; (d) the many-body transition points $ \gamma_{\mathrm{c}} $ as a function of θ, where region I and region II correspond to many-body localization and ergodic phases, respectively.
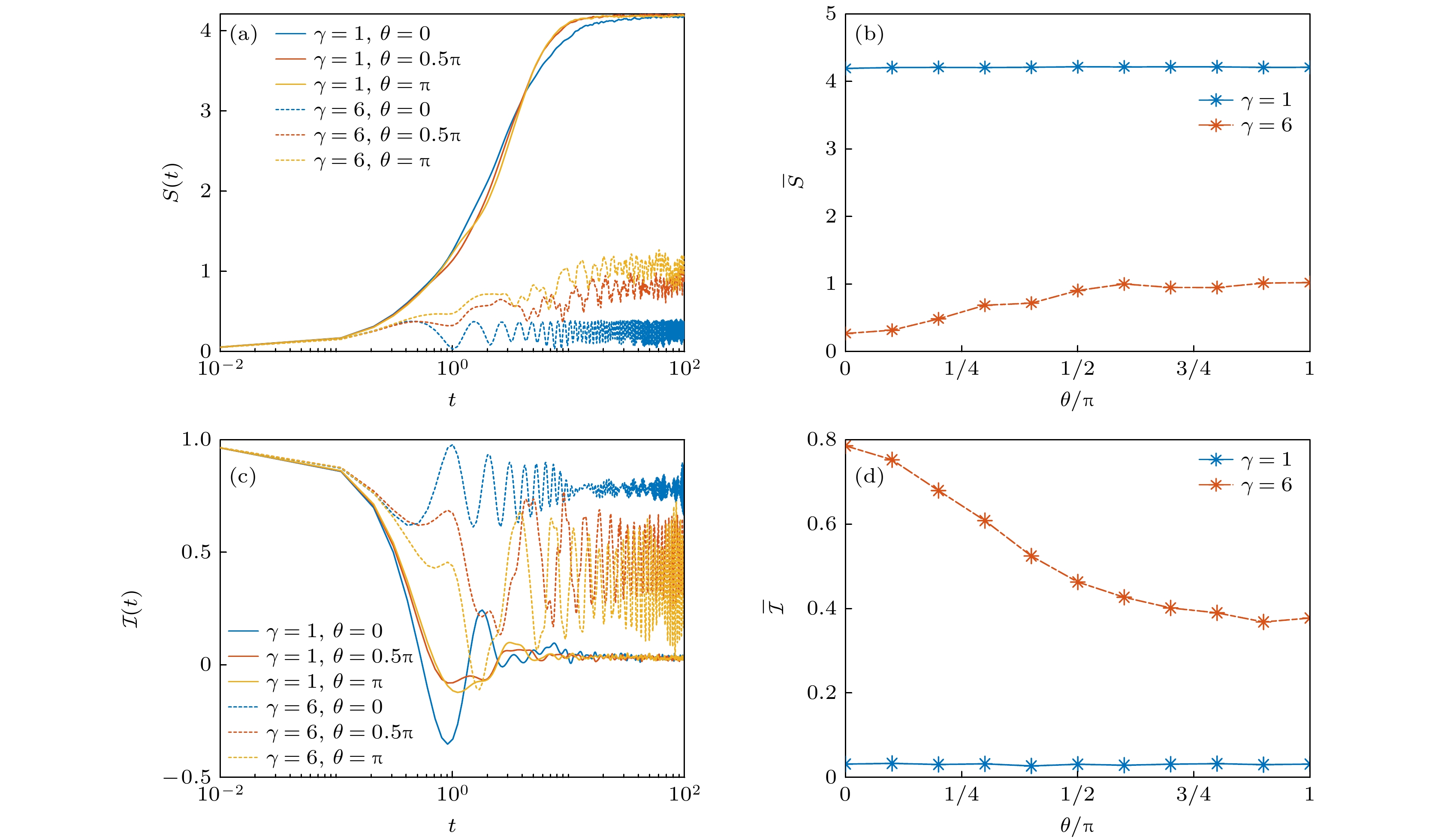
图 3 (a) 不同θ和γ时半链纠缠熵$ S(t) $随时间t的演化行为; (b) 对于不同的γ, 半链纠缠熵平均值$ \overline{S} $随统计角θ的变化情况; (c) 不同θ和γ时, 粒子非平衡态占据数$ \mathcal{I}\left( t\right) $随时间演化的情况; (d) 对于不同的γ, 非平衡态占据数平均值$ \overline{\mathcal{I}} $随统计角θ的变化情况, 其中$ L=12 $
Figure 3. (a) Evolution of the half-chain entanglement entropy $ S(t) $ vs. t for different θ and γ; (b) $ \overline{S} $ as a function of θ for different γ; (c) evolution of the particle imbalance $ \mathcal{I}\left( t\right) $ for different θ and γ; (d) $ \overline{\mathcal{I}} $ as a function of θ for different γ. Here, $ L=12 $.
-
[1] Schreiber M, Hodgman S S, Bordia P, Lüschen H P, Fischer M H, Vosk R, Altman E, Schneider U, Bloch I 2015 Science 349 842
Google Scholar
[2] Bordia P, Lüschen H P, Hodgman S S, Schreiber M, Bloch I, Schneider U 2016 Phys. Rev. Lett. 116 140401
Google Scholar
[3] Smith J, Lee A, Richerme P, Neyenhuis B, Hess P W, Hauke P, Heyl M, Huse D A, Monroe C 2016 Nat. Phys. 12 907
Google Scholar
[4] Xu K, Chen J J, Zeng Y, Zhang Y R, Song C, Liu W X, Guo Q J, Zhang P F, Xu D, Deng H, Huang K Q, Wang H, Zhu X B, Zheng D N, Fan H 2018 Phys. Rev. Lett. 120 050507
Google Scholar
[5] Guo Q, Cheng C, Sun Z H, Song Z, Li H, Wang Z, Ren W, Dong H, Zheng D, Zhang Y R, Mondaini R, Fan H, Wang H 2021 Nat. Phys. 17 234
Google Scholar
[6] Guo Q J, Cheng C, Li H K, Xu S B, Zhang P F, Wang Z, Song C, Liu W X, Ren W H, Dong H, Mondaini R, Wang H 2021 Phys. Rev. Lett. 127 240502
Google Scholar
[7] Morong W, Liu F, Becker P, Collins K S, Feng L, Kyprianidis A, Pagano G, You T, Gorshkov A V, Monroe C 2021 Nature 599 393
Google Scholar
[8] Scherg S, Kohlert T, Sala P, Pollmann F, Hebbe Madhusudhana B, Bloch I, Aidelsburger M 2021 Nat. Commun. 12 4490
Google Scholar
[9] Liu J H, Xu Z H 2023 Phys. Rev. B 108 184205
Google Scholar
[10] Leinaas J M, Myrheim J 1977 Nuovo Cimento Soc. Ital. Fis. B 37 1
Google Scholar
[11] Tsui D C, Stormer H L, Gossard A C 1982 Phys. Rev. Lett. 48 1559
Google Scholar
[12] Laughlin R B 1983 Phys. Rev. Lett. 50 1395
Google Scholar
[13] Nayak C, Simon S H, Stern A, Freedman M, Das Sarma S 2008 Rev. Mod. Phys. 80 1083
Google Scholar
[14] Kitaev A 2006 Ann. Phys. 321 2
Google Scholar
[15] Stern A 2008 Ann. Phys. 323 204
Google Scholar
[16] Haldane F D M 1991 Phys. Rev. Lett. 67 937
Google Scholar
[17] Vitoriano C, Coutinho-Filho M D 2009 Phys. Rev. Lett. 102 146404
Google Scholar
[18] Keilmann T, Lanzmich S, McCulloch I, Roncaglia M 2011 Nat. Commun. 2 361
Google Scholar
[19] Greschner S, Santos L 2015 Phys. Rev. Lett. 115 053002
Google Scholar
[20] Sträter C, Srivastava S C L, Eckardt A 2016 Phys. Rev. Lett. 117 205303
Google Scholar
[21] Zuo Z W, Li G L, Li L 2018 Phys. Rev. B 97 115126
Google Scholar
[22] Liu F, Garrison J R, Deng D L, Gong Z X, Gorshkov A V 2018 Phys. Rev. Lett. 121 250404
Google Scholar
[23] Zhang G Q, Zhang D W, Li Z, Wang Z D, Zhu S L 2020 Phys. Rev. B 102 054204
Google Scholar
[24] Wang Y Y, Sun Z H, Fan H 2021 Phys. Rev. B 104 205122
Google Scholar
[25] 王利, 贾丽芳, 张云波 2022 71 130501
Google Scholar
Wang L, Jia L F, Zhang Y B 2022 Acta. Rhys. Sin. 71 130501
Google Scholar
[26] 刘敬鹄, 徐志浩 2024 73 077202
Google Scholar
Liu J H, Xu Z H 2024 Acta. Rhys. Sin. 73 077202
Google Scholar
[27] Wu H, Vallières M, Feng D H, Sprung D W L 1990 Phys. Rev. A 42 1027
Google Scholar
[28] Hamazaki R, Kawabata K, Kura N, Ueda M 2020 Phys. Rev. Res. 2 023286
Google Scholar
[29] Oganesyan V, Huse D A 2007 Phys. Rev. B 75 155111
Google Scholar
[30] Serbyn M, Papić Z, Abanin D A 2013 Phys. Rev. Lett. 111 127201
Google Scholar
[31] Van Nieuwenburg E, Baum Y, Refael G 2019 Proc. Natl. Acad. Sci. U.S.A. 116 9269
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 614
- PDF Downloads: 27
- Cited By: 0
