Polarization properties of partially coherent mixed dislocation beams transmitting in biological tissues

Feng Jiao-Jiao; Duan Mei-Ling; Shan Jing; Wang Ling-Hui; Xue Ting

doi:10.7498/aps.73.20240985

Abstract

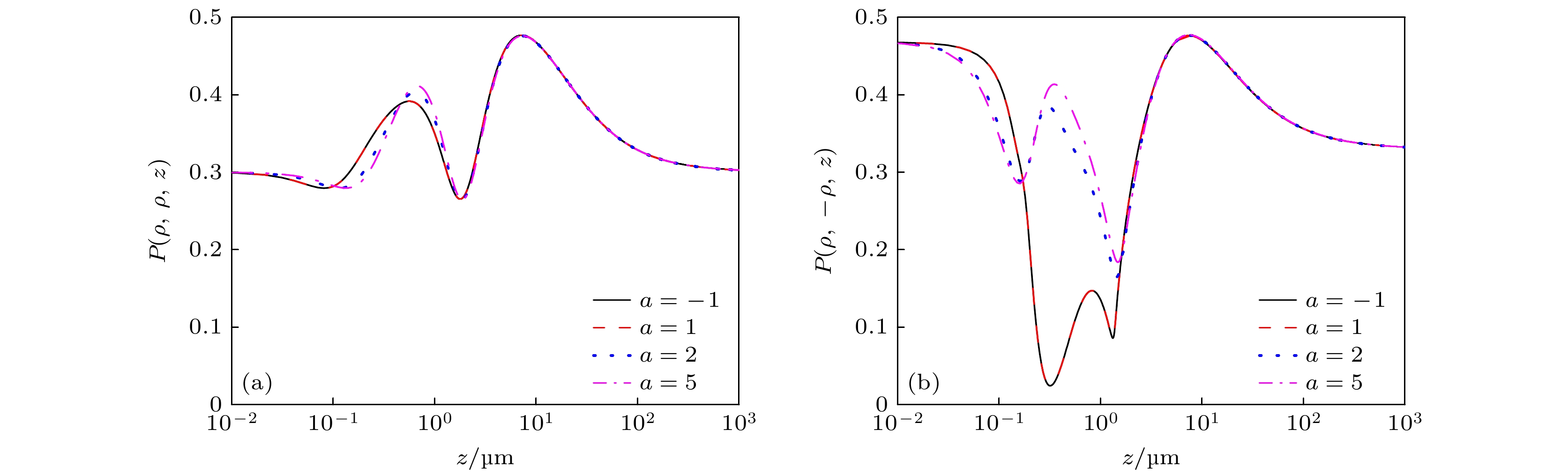
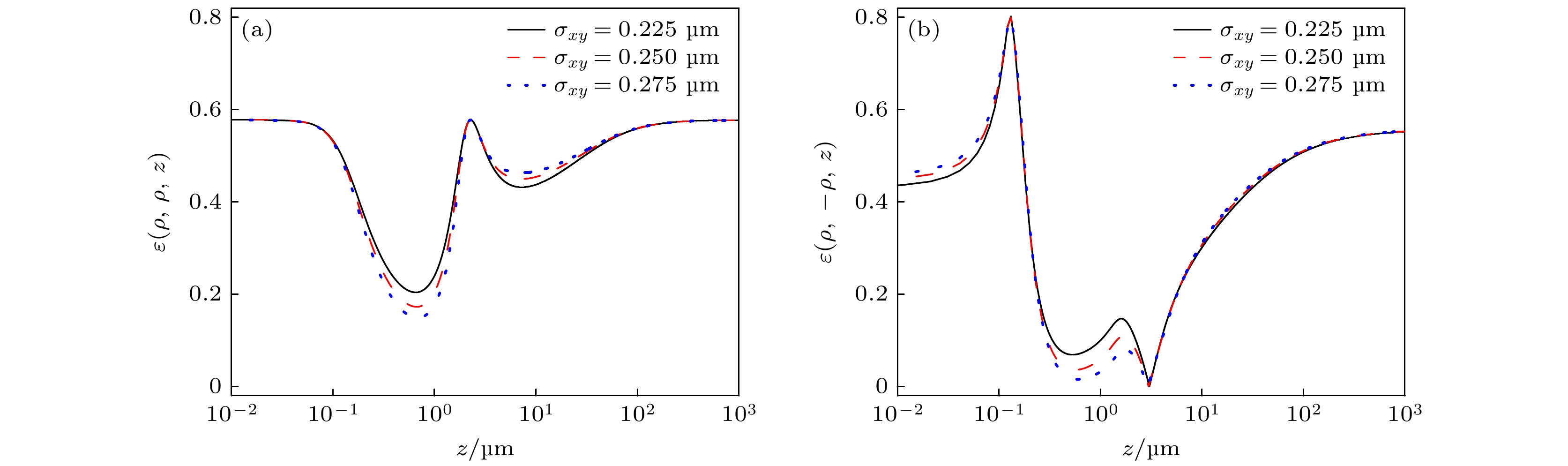
Objective The optical information change of beams acting on biological tissue can get an insight into the new optical effects of tissue, and even can provide a theoretical basis for developing biphotonic medical diagnosis and therapy technologies. Polarization technology is also widely used in the field of biological detection due to its advantages of non-contact, rich information and without staining markers. In this work, the polarization behaviors of partially coherent screw-linear edge mixed dislocation beam transmitting in biological tissue are analyzed and explored. Simultaneously, in order to more clearly and more intuitively understand a mixed dislocation beam, both the normalized intensities and phase distributions at source plane for different parameters a and b are also discussed. We hope that the obtained results will provide theoretical and experimental foundation for expanding the application of singularity beams in biological tissue imaging technology. Method By combining the Schell term with the field distribution of the screw-linear edge mixed dislocation beam at the source plane, and based on the generalized Huygens-Fresnel principle, the analytical expressions of the cross-spectral density matrix elements of partially coherent screw-linear edge dislocation beam propagating in biological tissues are derived. Adopting the unified theory of coherence and polarization, the polarization behaviors of the beams can be investigated in detail. Results At the source plane, the intensity has a non axisymmetric distribution, and there exists a coherent vortex with a topological charge size of 1 and a linear edge dislocation. The sign of a is related to the rotation direction of the phase singularity. The larger the value of b, the farther the linear edge dislocation is from the origin. At the source plane, the degree of polarization and ellipticity between the two identical points are independent of the four parameters: dimensionless parameter a, off-axis distance of edge dislocation b, spatial self-correlation length σ_yy, and spatial mutual-correlation length σ_xy, the orientation angle is only independent of a and σ_xy; the polarization of two different points is independent of a and b, but is related to σ_yy and σ_xy. In transmission, the polarization degrees and ellipticity of two different points fluctuate greatly and the orientation angle displays less fluctuation. Finally, all the polarization state parameters tend to be their corresponding values, respectively. Conclusions The results show that when b is smaller, the linear edge dislocation is paraxial and plays an important role in the polarization state change; when b is larger, the polarization state changes of the screw-linear edge mixed dislocation beam will tend to be the pattern of spiral beams. The absolute value of the difference between σ_yy and σ_xy is also one of main factors influencing the polarization state. The sign of a does not affect the change in polarization state, but its magnitude can influe the change of speed. Due to more complex factors determining the correlation fluctuations between different points in the light field, the changes of two different points are more sensitive than those of the two identical points in shallow biological tissue. Beams with different parameters can be selected for different application requirements.

Keywords:

mixed screw-linear edge dislocation beam /
biological tissue /
cross-spectral density /
polarization state

Authors and contacts

School of Semiconductors and Physics, North University, Taiyuan 030051, China

Corresponding author: Duan Mei-Ling, meilingduan@nuc.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 12204439), the Fundamental Research Program of Shanxi Province, China (Grant No. 202203021211192), and the Applied Basic Research Foundation of Shanxi Province, China (Grant No. 201701D121011).

FullText HTML

References

[1]	Zhou Y, Cheng K, Sun X, Zhao M R, Chen G 2022 J. Mod. Opt. 69 233 Google Scholar
[2]	杨宁, 赵亮, 许颖, 徐勇根 2022 激光与红外 52 1167 Google Scholar Yang N, Zhao L, Xu Y, Xu Y G 2022 Laser Infrared 52 1167 Google Scholar
[3]	乔文龙, 周亮, 刘朝晖, 龚勇辉, 姜乐, 吕媛媛, 赵鹤童 2022 光谱学与光谱分析 42 1070 Google Scholar Qiao W L, Zhou L, Liu Z H, Gong Y H, Jiang L, Lu Y Y, Zhao H T 2022 Spectrosc. Spect. Anal. 42 1070 Google Scholar
[4]	Zhao C G, Yin X J, Yang C, Wang J, Li J H 2023 Microw. Opt. Techn. Let. 65 1054 Google Scholar
[5]	王亚伟, 刘莹, 卜敏, 王立峰 2008 激光与红外 38 7 Google Scholar Wang Y W, Liu Y, Bu M, Wang L F 2008 Laser Infrared 38 7 Google Scholar
[6]	杜玲艳, 詹旭, 雷跃荣, 宋弘, 文宇桥 2009 红外与激光工程 38 466 Google Scholar Du L Y, Zhan X, Lei Y R, Song H, Wen Y Q 2009 Infrared Laser Eng. 38 466 Google Scholar
[7]	Sdobnov A, Ushenko V A, Trifonyuk L, Dubolazov O V, Ushenko Y A, Ushenko A G, Soltys I V, Gantyuk V K, Bykov A, Meglinski I 2023 Opt. Laser. Eng. 171 107806 Google Scholar
[8]	张钰新, 樊志鹏, 翟好宇, 何宏辉, 王毅, 何超, 马辉 2023 中国激光 50 111 Google Scholar Zhang Y X, Fan Z P, Zhai H Y, He H H, Wang Y, He C, Ma H 2023 Chin. J. Lasers 50 111 Google Scholar
[9]	Zhang W H, Wang L, Wang W N, Zhao S M 2019 OSA Continuum 2 3281 Google Scholar
[10]	Liang Q Y, Yang D Y, Zhang Y X, Zheng Y, Hu L F 2020 OSA Continuum 3 2429 Google Scholar
[11]	黄慧, 寿倩, 陈志超 2020 激光与光电子学进展 57 244 Google Scholar Huang H, Shou Q, Chen Z C 2020 Laser Optoelectron. Prog. 57 244 Google Scholar
[12]	叶东, 李俊瑶, 李宗辰, 张颐 2024 激光技术 48 261 Google Scholar Ye D, Li J Y, Li Z C, Zhang Y 2024 Laser Technol. 48 261 Google Scholar
[13]	Biton N, Kupferman J, Arnon S 2021 Sci. Rep. 11 2047 Google Scholar
[14]	段美玲, 杜娇, 赵志国, 黄小东, 高燕琴, 丁超亮 2021 光子学报 50 0929001 Google Scholar Duan M L, Du J, Zhao Z G, Huang X D, Gao Y Q, Ding C L 2021 Acta Photonica Sin. 50 0929001 Google Scholar
[15]	Chen K, Ma Z Y, Hu Y Y 2023 Chin. Phys. B 32 024208 Google Scholar
[16]	Zhou Y Q, Cui Z W, Han Y P 2022 Opt. Express 30 23448 Google Scholar
[17]	闫皙玉, 杨艳芳, 何英, 李路路, 王俊杰 2022 光学学报 42 184 Google Scholar Yan X Y, Yang Y F, He Y, Li L L, Wang J J 2022 Acta Opt. Sin. 42 184 Google Scholar
[18]	Gao P H, Lu M H, Li J Y 2023 Opt. Continuum 2 2374 Google Scholar
[19]	Cao J, Tong R F, Huang K, Li Y Q, Xu Y G 2024 J. Opt. Soc. Am. A 41 371 Google Scholar
[20]	殷子昂, 段美玲 2024 光学技术 50 99 Google Scholar Yin Z A, Duan M L 2024 Opt. Tech. 50 99 Google Scholar
[21]	Gao P H, Bai L, Li J L 2020 OSA Continuum 3 2997 Google Scholar
[22]	Gao P H, Lie J H, Cheng K, Duan M L 2017 Opt. Appl. 47 471 Google Scholar
[23]	Wang Y K, Bai L, Gao P H 2019 Cross Strait Quad-Regional Radio Science and Wireless Technology Conference Taiyuan, China, July 18–21, 2019 pp1–3
[24]	Wolf E 2007 Introduction to the Theory of Coherence and Polarization of Light (Cambridge: Cambridge University Press) pp59–60
[25]	Wolf E, 蒲继雄 2014 光的相干与偏振理论导论 (北京: 北京大学出版社) 第210页 Wolf E, Pu J X 2014 Introduction to the Theory of Coherence and Polarization of Light (Beijing: Peking University Press) p210
[26]	Kotlyar V, Kovalev A, Porfirev A 2017 Phys. Rev. A 95 053805 Google Scholar
[27]	Ishimaru A 1977 Appl. Opt. 16 3190 Google Scholar
[28]	Roychowdhury H, Korotkova O 2005 Opt. Commun. A 249 379 Google Scholar
[29]	Andrews L C, Phillips R L 2005 Laser Beam Propagation Through Random Media (Washington: SPIE Press) p820
[30]	Shirron J J 1997 Siam. Rev. 39 803
[31]	Mandel L, Wolf E 1995 Optical Coherence and Quantum Optics (Cambridge: Cambridge University Press) p170
[32]	Freund I, Shvartsman N 1994 Phys. Rev. A 50 5164 Google Scholar
[33]	贺改梅, 段美玲, 殷子昂, 单晶, 冯姣姣 2024 光学学报 44 0217002 Google Scholar He G M, Duan M L, Yin Z A, Shan J, Feng J J 2024 Acta Opt. Sin. 44 0217002 Google Scholar
[34]	Deng Y, Zeng S Q, Luo Q M, Zhang Z H, Fu L 2008 Opt. Lett. 33 77 Google Scholar

Cited By

图 1 a不同时归一化光强分布　(a) a = –1; (b) a = 1; (c) a = 2; (d) a = 5

Figure 1. Normalized light intensity distribution for different a values: (a) a = –1; (b) a = 1; (c) a = 2; (d) a = 5.

DownLoad: Full-Size Img PowerPoint

图 2 b不同时归一化光强分布　 (a) b = 0.2 μm; (b) b = 0.3 μm; (c) b = 1 μm; (d) b = 3 μm

Figure 2. Normalized light intensity distribution for different b values: (a) b = 0.2 μm; (b) b = 0.3 μm; (c) b = 1 μm; (d) b = 3 μm.

DownLoad: Full-Size Img PowerPoint

图 3 a不同时相位分布　 (a) a = –1; (b) a = 1; (c) a = 2; (d) a = 5

Figure 3. Phase distribution for different a values: (a) a = –1; (b) a = 1; (c) a = 2; (d) a = 5.

DownLoad: Full-Size Img PowerPoint

图 4 b不同时相位分布　(a) b = 0.2 μm; (b) b = 0.3 μm; (c) b = 1 μm; (d) b = 3 μm

Figure 4. Phase distribution for different b values: (a) b = 0.2 μm; (b) b = 0.3 μm; (c) b = 1 μm; (d) b = 3 μm.

DownLoad: Full-Size Img PowerPoint

图 5 a不同时偏振度随z的变化　 (a) P(ρ, ρ, z); (b) P(ρ, –ρ, z)

Figure 5. Variation of polarization degree with z for different a: (a) P(ρ, ρ, z); (b) P(ρ, –ρ, z).

DownLoad: Full-Size Img PowerPoint

图 6 a不同时方位角随z的变化　 (a) θ(ρ, ρ, z); (b) θ(ρ, –ρ, z)

Figure 6. Variation of orientation angle with z for different a: (a) θ(ρ, ρ, z); (b) θ(ρ, –ρ, z).

DownLoad: Full-Size Img PowerPoint

图 7 a不同时椭圆率随z的变化　(a) ε(ρ, ρ, z); (b) ε(ρ, –ρ, z)

Figure 7. Variation of ellipticity with z when a is different: (a) ε(ρ, ρ, z); (b) ε(ρ, –ρ, z).

DownLoad: Full-Size Img PowerPoint

图 8 b不同时偏振度随z的变化　 (a) P(ρ, ρ, z); (b) P(ρ, –ρ, z)

Figure 8. Variation of polarization degree with z for different b: (a) P(ρ, ρ, z); (b) P(ρ, –ρ, z).

DownLoad: Full-Size Img PowerPoint

图 9 b不同时方位角随z的变化　 (a) θ(ρ, ρ, z); (b) θ(ρ, –ρ, z)

Figure 9. Variation of orientation angle with z for different b: (a) θ(ρ, ρ, z); (b) θ(ρ, –ρ, z).

DownLoad: Full-Size Img PowerPoint

图 10 b不同时椭圆率ε随z的变化　 (a) ε(ρ, ρ, z); (b) ε(ρ, –ρ, z)

Figure 10. Variation of ellipticity with z for different b: (a) ε(ρ, ρ, z); (b) ε(ρ, –ρ, z).

DownLoad: Full-Size Img PowerPoint

图 11 σ_xy不同时偏振度随z的变化　(a) P(ρ, ρ, z); (b) P(ρ, –ρ, z)

Figure 11. Polarization degree vs. z for different σ_xy: (a) P(ρ, ρ, z); (b) P(ρ, –ρ, z).

DownLoad: Full-Size Img PowerPoint

图 12 σ_xy不同时方位角随z的变化　 (a) θ(ρ, ρ, z); (b) θ(ρ, –ρ, z)

Figure 12. Orientation angle vs. z for different σ_xy: (a) θ(ρ, ρ, z); (b) θ(ρ, –ρ, z).

DownLoad: Full-Size Img PowerPoint

图 13 σ_xy不同时椭圆率随z的变化　(a) ε(ρ, ρ, z); (b) ε(ρ, –ρ, z)

Figure 13. Ellipticity vs. z for different σ_xy: (a) ε(ρ, ρ, z); (b) ε(ρ, –ρ, z).

DownLoad: Full-Size Img PowerPoint

图 14 σ_yy不同时偏振度随z的变化　(a) P(ρ, ρ, z); (b) P(ρ, –ρ, z)

Figure 14. Polarization degree vs. z for different σ_yy: (a) P(ρ, ρ, z); (b) P(ρ, –ρ, z).

DownLoad: Full-Size Img PowerPoint

图 16 σ_yy不同时椭圆率随z的变化　 (a) ε(ρ, ρ, z); (b) ε(ρ, –ρ, z)

Figure 16. Ellipticity vs. z for different σ_yy: (a) ε(ρ, ρ, z); (b) ε(ρ, –ρ, z).

DownLoad: Full-Size Img PowerPoint

图 15 σ_yy不同时方位角随z的变化　 (a) θ(ρ, ρ, z); (b) θ(ρ, –ρ, z)

Figure 15. Orientation angle vs. z for different σ_yy: (a) θ(ρ, ρ, z); (b) θ(ρ, –ρ, z).

DownLoad: Full-Size Img PowerPoint

map

[1]	Zhou Y, Cheng K, Sun X, Zhao M R, Chen G 2022 J. Mod. Opt. 69 233 Google Scholar
[2]	杨宁, 赵亮, 许颖, 徐勇根 2022 激光与红外 52 1167 Google Scholar Yang N, Zhao L, Xu Y, Xu Y G 2022 Laser Infrared 52 1167 Google Scholar
[3]	乔文龙, 周亮, 刘朝晖, 龚勇辉, 姜乐, 吕媛媛, 赵鹤童 2022 光谱学与光谱分析 42 1070 Google Scholar Qiao W L, Zhou L, Liu Z H, Gong Y H, Jiang L, Lu Y Y, Zhao H T 2022 Spectrosc. Spect. Anal. 42 1070 Google Scholar
[4]	Zhao C G, Yin X J, Yang C, Wang J, Li J H 2023 Microw. Opt. Techn. Let. 65 1054 Google Scholar
[5]	王亚伟, 刘莹, 卜敏, 王立峰 2008 激光与红外 38 7 Google Scholar Wang Y W, Liu Y, Bu M, Wang L F 2008 Laser Infrared 38 7 Google Scholar
[6]	杜玲艳, 詹旭, 雷跃荣, 宋弘, 文宇桥 2009 红外与激光工程 38 466 Google Scholar Du L Y, Zhan X, Lei Y R, Song H, Wen Y Q 2009 Infrared Laser Eng. 38 466 Google Scholar
[7]	Sdobnov A, Ushenko V A, Trifonyuk L, Dubolazov O V, Ushenko Y A, Ushenko A G, Soltys I V, Gantyuk V K, Bykov A, Meglinski I 2023 Opt. Laser. Eng. 171 107806 Google Scholar
[8]	张钰新, 樊志鹏, 翟好宇, 何宏辉, 王毅, 何超, 马辉 2023 中国激光 50 111 Google Scholar Zhang Y X, Fan Z P, Zhai H Y, He H H, Wang Y, He C, Ma H 2023 Chin. J. Lasers 50 111 Google Scholar
[9]	Zhang W H, Wang L, Wang W N, Zhao S M 2019 OSA Continuum 2 3281 Google Scholar
[10]	Liang Q Y, Yang D Y, Zhang Y X, Zheng Y, Hu L F 2020 OSA Continuum 3 2429 Google Scholar
[11]	黄慧, 寿倩, 陈志超 2020 激光与光电子学进展 57 244 Google Scholar Huang H, Shou Q, Chen Z C 2020 Laser Optoelectron. Prog. 57 244 Google Scholar
[12]	叶东, 李俊瑶, 李宗辰, 张颐 2024 激光技术 48 261 Google Scholar Ye D, Li J Y, Li Z C, Zhang Y 2024 Laser Technol. 48 261 Google Scholar
[13]	Biton N, Kupferman J, Arnon S 2021 Sci. Rep. 11 2047 Google Scholar
[14]	段美玲, 杜娇, 赵志国, 黄小东, 高燕琴, 丁超亮 2021 光子学报 50 0929001 Google Scholar Duan M L, Du J, Zhao Z G, Huang X D, Gao Y Q, Ding C L 2021 Acta Photonica Sin. 50 0929001 Google Scholar
[15]	Chen K, Ma Z Y, Hu Y Y 2023 Chin. Phys. B 32 024208 Google Scholar
[16]	Zhou Y Q, Cui Z W, Han Y P 2022 Opt. Express 30 23448 Google Scholar
[17]	闫皙玉, 杨艳芳, 何英, 李路路, 王俊杰 2022 光学学报 42 184 Google Scholar Yan X Y, Yang Y F, He Y, Li L L, Wang J J 2022 Acta Opt. Sin. 42 184 Google Scholar
[18]	Gao P H, Lu M H, Li J Y 2023 Opt. Continuum 2 2374 Google Scholar
[19]	Cao J, Tong R F, Huang K, Li Y Q, Xu Y G 2024 J. Opt. Soc. Am. A 41 371 Google Scholar
[20]	殷子昂, 段美玲 2024 光学技术 50 99 Google Scholar Yin Z A, Duan M L 2024 Opt. Tech. 50 99 Google Scholar
[21]	Gao P H, Bai L, Li J L 2020 OSA Continuum 3 2997 Google Scholar
[22]	Gao P H, Lie J H, Cheng K, Duan M L 2017 Opt. Appl. 47 471 Google Scholar
[23]	Wang Y K, Bai L, Gao P H 2019 Cross Strait Quad-Regional Radio Science and Wireless Technology Conference Taiyuan, China, July 18–21, 2019 pp1–3
[24]	Wolf E 2007 Introduction to the Theory of Coherence and Polarization of Light (Cambridge: Cambridge University Press) pp59–60
[25]	Wolf E, 蒲继雄 2014 光的相干与偏振理论导论 (北京: 北京大学出版社) 第210页 Wolf E, Pu J X 2014 Introduction to the Theory of Coherence and Polarization of Light (Beijing: Peking University Press) p210
[26]	Kotlyar V, Kovalev A, Porfirev A 2017 Phys. Rev. A 95 053805 Google Scholar
[27]	Ishimaru A 1977 Appl. Opt. 16 3190 Google Scholar
[28]	Roychowdhury H, Korotkova O 2005 Opt. Commun. A 249 379 Google Scholar
[29]	Andrews L C, Phillips R L 2005 Laser Beam Propagation Through Random Media (Washington: SPIE Press) p820
[30]	Shirron J J 1997 Siam. Rev. 39 803
[31]	Mandel L, Wolf E 1995 Optical Coherence and Quantum Optics (Cambridge: Cambridge University Press) p170
[32]	Freund I, Shvartsman N 1994 Phys. Rev. A 50 5164 Google Scholar
[33]	贺改梅, 段美玲, 殷子昂, 单晶, 冯姣姣 2024 光学学报 44 0217002 Google Scholar He G M, Duan M L, Yin Z A, Shan J, Feng J J 2024 Acta Opt. Sin. 44 0217002 Google Scholar
[34]	Deng Y, Zeng S Q, Luo Q M, Zhang Z H, Fu L 2008 Opt. Lett. 33 77 Google Scholar

[1]	Wang Zhi-Quan, Shi Wei. Holographic detection of pulsed terahertz waves in terahertz time-domain spectroscopy. Acta Physica Sinica, 2022, 71(18): 188704. doi: 10.7498/aps.71.20220983
[2]	. Polarization free of plasmonic heterodimer based on capped nanostructure. Acta Physica Sinica, 2021, (): . doi: 10.7498/aps.70.20211381
[3]	Zhao Gu-Hao, Mao Shao-Jie, Zhao Shang-Hong, Meng Wen, Zhu Jie, Zhang Xiao-Qiang, Wang Guo-Dong, Gu Wen-Yuan. Principle and experimental study of self-stability of reflector based on two magneto-optical crystals and two mirrors under effect of temperature and radiation. Acta Physica Sinica, 2019, 68(16): 164202. doi: 10.7498/aps.68.20190429
[4]	Hong Xin, Wang Chen-Chen, Liu Jiang-Tao, Wang Xiao-Qiang, Yin Xue-Jie. Photothermal properties of core-capped gold nanoparticles. Acta Physica Sinica, 2018, 67(19): 195202. doi: 10.7498/aps.67.20180909
[5]	Wang Mei-Jie, Jia Wei-Guo, Zhang Si-Yuan, Menke Nei-Mu-Le, Yang Jun, Zhang Jun-Ping. Effect of Raman gain on the state of polarization evolution in a low-birefringence fiber. Acta Physica Sinica, 2015, 64(3): 034212. doi: 10.7498/aps.64.034212
[6]	Liu Ji-Lin, Chen Zi-Yang, Zhang Lei, Pu Ji-Xiong. Polarization and propagation characteristics of the azimuthally polarized non-diffracting beam. Acta Physica Sinica, 2015, 64(6): 064201. doi: 10.7498/aps.64.064201
[7]	Wang Mei-Jie, Jia Wei-Guo, Zhang Si-Yuan, Qiao Hai-Long, Yang Jun, Zhang Jun-Ping, Menke Nei-Mu-Le. Influence of Raman effect on the state of polarization evolution in a low-birefringence fiber. Acta Physica Sinica, 2014, 63(10): 104204. doi: 10.7498/aps.63.104204
[8]	Wang Qiang, Guan Bao-Lu, Liu Ke, Shi Guo-Zhu, Liu Xin, Cui Bi-Feng, Han Jun, Li Jian-Jun, Xu Chen. Temperature characteristics of VCSEL with liquid crystal overlay. Acta Physica Sinica, 2013, 62(23): 234206. doi: 10.7498/aps.62.234206
[9]	Zhao Gu-Hao, Zhao Shang-Hong, Yao Zhou-Shi, Hao Chen-Lu, Meng Wen, Wang Xiang, Zhu Zhi-Hang, Liu Feng. Experimental study on polarization-independent reflector structure based on magneto-optical crystal and two mirrors. Acta Physica Sinica, 2013, 62(13): 134201. doi: 10.7498/aps.62.134201
[10]	Ma Jun, Yuan Cao-Jin, Feng Shao-Tong, Nie Shou-Ping. Full-field detection of polarization state based on multiplexing digital holography. Acta Physica Sinica, 2013, 62(22): 224204. doi: 10.7498/aps.62.224204
[11]	Chen Yuan-Yuan, Zou Ren-Hua, Song Gang, Zhang Kai, Yu Li, Zhao Yu-Fang, Xiao Jing-Hua. The polarization characteristics of the excitation and emission of surface plasmon polarization in the Ag nanowires. Acta Physica Sinica, 2012, 61(24): 247301. doi: 10.7498/aps.61.247301
[12]	Zhang Xuan-Ni, Zhang Chun-Min. The optical transmission and improvement of flux for the static polarization wind imaging interferometer. Acta Physica Sinica, 2012, 61(10): 104210. doi: 10.7498/aps.61.104210
[13]	Xavier Mateos, Valentin Petrov, Zhang Huai-Jin, Wang Ji-Yang, Liu Jun-Hai, Han Wen-Juan. Comparative study on the spectroscopic and laser propertiesof mixed vanadates Ybt:Y x Gd1-t-x VO4with different compositions. Acta Physica Sinica, 2011, 60(1): 014211. doi: 10.7498/aps.60.014211
[14]	Liu Hong-Yao, Lü Qiang, Luo Hai-Lu, Wen Shuang-Chun. Focusing properties of the uniaxially anisotropic metamaterial slab lens. Acta Physica Sinica, 2010, 59(1): 256-263. doi: 10.7498/aps.59.256
[15]	Wang Qing-Hua, Zhang Ying-Ying, Lai Jian-Cheng, Li Zhen-Hua, He An-Zhi. Application of Mie theory in biological tissue scattering characteristics analysis. Acta Physica Sinica, 2007, 56(2): 1203-1207. doi: 10.7498/aps.56.1203
[16]	Zhang Hang. The optical tomography of tissues by a δ sound field. Acta Physica Sinica, 2004, 53(8): 2515-2519. doi: 10.7498/aps.53.2515
[17]	Wang Chen, Yuan Jing-He, Wang Gui-Ying, Xu Zhi-Zhan. The influence of polarized light on fluorescence emission in total internal refl ection microscopy. Acta Physica Sinica, 2003, 52(12): 3014-3019. doi: 10.7498/aps.52.3014
[18]	Guo Hong-Lian, Cheng Bing-Ying, Zhang Dao-Zhong. Photic intensity distribution simulations of biological tissues with polystyrene spheres. Acta Physica Sinica, 2003, 52(2): 324-327. doi: 10.7498/aps.52.324
[19]	Su Hui-Min, Zheng Xi Guang, Wang Xia, Xu Jian-Feng, Wang He-Zhou. . Acta Physica Sinica, 2002, 51(5): 1044-1048. doi: 10.7498/aps.51.1044
[20]	QIAN SHENG-YOU, WANG HONG-ZHANG. THEORETICAL STUDY OF THE THERMAL EFFECT IN BIOLOGICAL MEDIUM GENERATED BY FOCUSED ULTRASOUND SOURCE. Acta Physica Sinica, 2001, 50(3): 501-506. doi: 10.7498/aps.50.501

Catalog

Vol. 73, Issue 18 - 2024-09-20

Metrics

Abstract views: 1469
PDF Downloads: 33
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板