Directional emission properties of thin film microdisk

Xu Yu-Xuan; Yao Tai-Yu; Deng Li; Chen Shi-Mei; Xu Chen-Yao; Tang Wen-Xuan

doi:10.7498/aps.73.20231754

Abstract

Based on the directional emission effect of semiconductor deformed microcavities, the fabrication of deformed microcavities in isotropic thin films will provide a new solution for multifunctional and highly integrated photonic active chips. Because the Limacon shaped microcavity has become one of the important configurations of single-mode, low threshold on-chip lasers, the directional emission properties of microdisks fabricated in thin film are investigated. 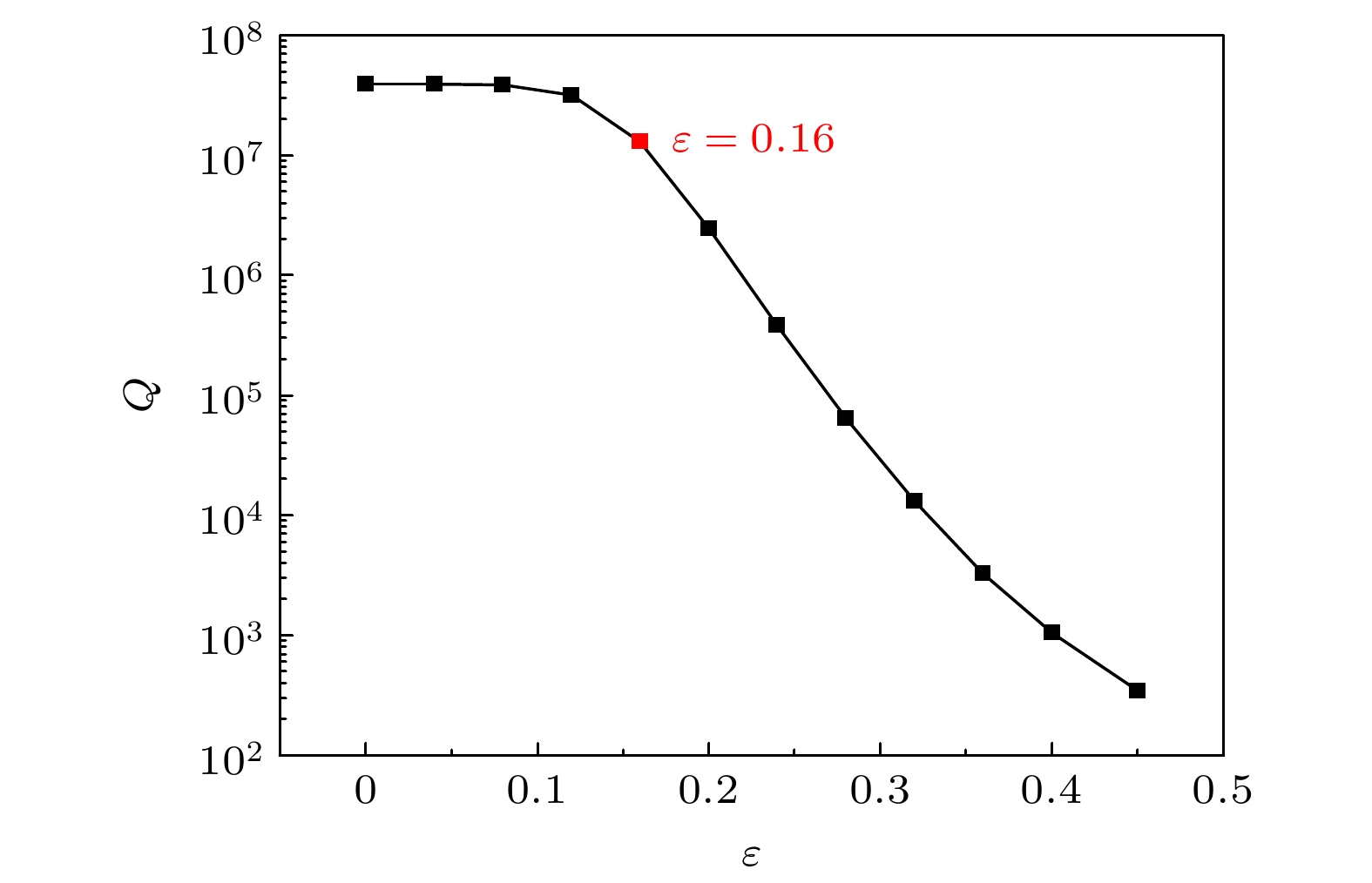Taking the TE_20,1 mode existing in the Z-cut lithium niobate thin film microdisk for example, according to two-dimensional wave optics theory, the mode distribution, quality factor Q, and directional emission factor D of microdisk variations with deformation factor $\varepsilon $ are respectively analyzed through using the wave optics module of COMSOL. Adopting classical scattering theory, Poincaré surfaces of sections under different deformation factors are simulated by optimizing the Dynamical Billards.jl library in Julia. In the simulation realized by Julia, 200 particle collisions are used 200 times to simulate 200 reflections of rays and finally PSOS images are obtained. Simulation results reveal that when the azimuthal quantum number of the light wave mode remains unchanged, although the shape of the microdisk varies, the ratio of the resonant wavelength inside the microdisk to the circumference of the microdisk is approximately a constant, which can predict the microdisk size and resonant wavelength estimation of microcavities. The corresponding PSOS shows that when $\varepsilon > 0.45$, the entire region is covered by chaotic sea area, therefore $\varepsilon $ values of 0, 0.16, 0.24, 0.28, 0.45 are selected to simulate the TE_20,1 mode distribution, far-field radiation flux angle distribution, and PSOS. Theoretical simulation results show that when the deformation factor is greater than 0.24, the microdisk has good unidirectional lasing property, with a Q factor greater than 10⁵. When the deformation factor is greater than 0.4, the PSOS is almost occupied by the chaotic sea area, with a Q factor below 10³. Therefore, when the deformation factor of the limacon microdisk in the thin film can be chosen between 0.24 and 0.4, under which circumstance the microdisk not only carries high quality factor (about 10³–10⁵), but also forms high laser directionality (about 6.45–8.32). The theoretical simulation results will provide a certain theoretical reference for conducting the experimental research of thin film deformation microcavities.

Keywords:

Authors and contacts

School of Physics and Electronic Science, East China Normal University, Shanghai 200241, China

Corresponding author: Deng Li, ldeng@phy.ecnu.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 12174113).

FullText HTML : translate this paragraph

References

[1]	Baaske M D, Foreman M R, Vollmer F 2014 Nat. Nanotechnol. 9 933 Google Scholar
[2]	Kippenberg T J, Holzwarth R, Diddams S A 2011 Science 332 555 Google Scholar
[3]	Kippenberg T J, Vahala K J 2008 Science 321 1172 Google Scholar
[4]	Michael C P, Srinivasan K, Johnson T J, Painter O, Lee K H, Hennessy K, Kim H, Hu E 2007 Appl. Phys. Lett. 90 051108 Google Scholar
[5]	Redding B, Ge L, Song Q H, Wiersig J, Solomon G S, Cao H 2012 Phys. Rev. Lett. 108 253902 Google Scholar
[6]	Fang W, Cao H, Solomon G S 2007 Appl. Phys. Lett. 90 081108 Google Scholar
[7]	Unterhinninghofen J, Wiersig J, Hentschel M 2008 Phys. Rev. E 78 016201 Google Scholar
[8]	Michler P, Kiraz A, Becher C, Schoenfeld W V, Petroff P M, Zhang L, Hu E, Imamoglu A 2000 Science 290 2282 Google Scholar
[9]	Jiang X F, Zou C L, Wang L, Gong Q H, Xiao Y F 2016 Laser Photonics Rev. 10 40 Google Scholar
[10]	Chern G D, Tureci H E, Stone A D, Chang R K, Kneissl M, Johnson N M 2003 Appl. Phys. Lett. 83 1710 Google Scholar
[11]	Kurdoglyan M S, Lee S Y, Rim S, Kim C M 2004 Opt. Lett. 29 2758 Google Scholar
[12]	Baryshnikov Y, Heider P, Parz W, Zharnitsky V 2004 Phys. Rev. Lett. 93 133902 Google Scholar
[13]	Gao J, Heider P, Chen C J, Yang X D, Husko C A, Wong C W 2007 Appl. Phys. Lett. 91 181101 Google Scholar
[14]	Wiersig J, Hentschel M 2006 Phys. Rev. A 73 031802 Google Scholar
[15]	Fang W, Yamilov A, Cao H 2005 Phys. Rev. A 72 023815 Google Scholar
[16]	Lee S Y, Kurdoglyan M S, Rim S, Kim C M 2004 Phys. Rev. A 70 023809 Google Scholar
[17]	Lebental M, Lauret J S, Hierle R, Zyss J 2006 Appl. Phys. Lett. 88 031108 Google Scholar
[18]	Wiersig J, Hentschel M 2008 Phys. Rev. Lett. 100 033901 Google Scholar
[19]	Yi C H, Kim M W, Kim C M 2009 Appl. Phys. Lett. 95 141107 Google Scholar
[20]	Song Q H, Fang W, Liu B Y, Ho S T, Solomon G S, Cao H 2009 Phys. Rev. A 80 041807 Google Scholar
[21]	Kim K, Bittner S, Jin Y H, Zeng Y Q, Wang Q J, Cao H 2023 Opt. Lett. 48 574 Google Scholar
[22]	Yan C L, Shi J W, Li P, Li H, Zhang J J 2014 Opt. Laser Technol. 56 285 Google Scholar
[23]	Fang Z W, Haque S, Farajollahi S, Luo H P, Lin J, Wu R B, Zhang J H, Wang Z, Wang M, Cheng Y, Lu T 2020 Phys. Rev. Lett. 125 173901 Google Scholar
[24]	Lin J T, Farajollahi S, Fang Z W, Yao N, Gao R H, Guan J L, Deng L, Lu T, Wang M, Zhang H S, Fang W, Qiao L L, Cheng Y 2022 Adv. Photonics 4 036001 Google Scholar
[25]	Foreman M R, Vollmer F 2013 New J. Phys. 15 083006 Google Scholar
[26]	Swaim J D, Knittel J, Bowen W P 2011 Appl. Phys. Lett. 99 243109 Google Scholar
[27]	Lee J, Rim S, Cho J, Kim C M 2008 Phys. Rev. Lett. 101 064101 Google Scholar
[28]	Farajollahi S, Fang Z W, Lin J T, Honari S, Cheng Y, Lu T 2023 Phys. Rev. A 108 033520 Google Scholar
[29]	Cao H, Wiersig J 2015 Rev. Mod. Phys. 87 61 Google Scholar
[30]	Ryu J W, Rim S, Park Y J, Kim C M, Lee S Y 2008 Phys. Lett. A 372 3531 Google Scholar
[31]	邹长铃, 董春华, 崔金明, 孙方稳, 杨勇, 吴晓伟, 韩正甫,郭光灿 2012 中国科学: 物理学力学天文学 42 1155 Zou C L, Dong C H, Cui J M, Sun F W, Yang Y, Wu X W, Han Z F, Guo G C 2012 Sci. Sin. Phys. Mech. Astron. 42 1155
[32]	Qi J W, Yan C L, Diehl L, Hentschel M, Wiersig J, Yu N F, Pflügl C, Belkin M A, Edamura T, Yamanishi M, Kan H, Capasso F 2009 New J. Phys. 11 125018 Google Scholar
[33]	Li J C, Huang Y T, Hao Y Z, Yang Y D, Xiao J L 2022 Single-mode Lasing Deformed Square Microcavity Lasers (USA: SPIE) p125011I
[34]	Wu R, Zhang J, Yao N, Fang W, Qiao L, Chai Z, Lin J, Cheng Y 2018 Opt. Lett. 43 4116 Google Scholar
[35]	Ilchenko V S, Savchenkov A A, Matsko A B, Maleki L 2004 Phys. Rev. Lett. 92 043903 Google Scholar
[36]	Pan Y, Lin G, Diallo S, Zhang X, Chembo Y K 2017 IEEE Photonics J. 9 1 Google Scholar
[37]	Wang L, Wang C, Wang J, Bo F, Zhang M, Gong Q, Loncar M, Xiao Y F 2018 Opt. Lett. 43 2917 Google Scholar
[38]	Gao A, Yang C, Chen L K, Zhang R, Luo Q, Wang W, Cao Q T, Hao Z Z, Bo F, Zhang G Q, Xu J J 2022 Photonics Res. 10 401 Google Scholar
[39]	Zhu D, Shao L B, Yu M J, Cheng R, Desiatov B, Xin C J, Hu Y W, Holzgrafe J, Ghosh S, Shams-Ansari A, Puma E, Sinclair N, Reimer C, Zhang M, Lončar M 2021 Adv. Opt. Photonics 13 242 Google Scholar
[40]	Gopalan V, Dierolf V, Scrymgeour D A 2007 Annu. Rev. Mater. Res. 37 449 Google Scholar
[41]	Sanna S, Schmidt W G 2010 Phys. Rev. B 81 214116 Google Scholar
[42]	Xiao Y F, Zou C L, Li Y, Dong C H, Han Z F, Gong Q H 2010 Front. Optoelectron. 3 109 Google Scholar
[43]	Yang Q F, Jiang X F, Cui Y L, Shao L B, Xiao Y F 2013 Phys. Rev. A 88 023810 Google Scholar
[44]	Jiang X F, Shao L B, Zhang S X, Yi X, Wiersig J, Wang L, Gong Q H, Loncar M, Yang L, Xiao Y F 2017 Science 358 344 Google Scholar
[45]	Xiao Y F, Jiang X F, Yang Q F, Wang L, Shi K B, Li Y, Gong Q H 2013 Laser Photonics Rev. 7 L51 Google Scholar
[46]	Boriskina S V, Benson T M, Sewell P, Nosich A I 2006 IEEE J. Sel. Top. Quantum Electron. 12 52 Google Scholar
[47]	Boriskina S V, Sewell P, Benson T M, Nosich A I 2004 J. Opt. Soc. Am. A 21 393 Google Scholar
[48]	Zelmon D E, Small D L, Jundt D 1997 J. Opt. Soc. Am. B 14 3319 Google Scholar

Cited By

图 1 Z切向铌酸锂XOY面结构的示意图

Figure 1. Structure diagram of Z-cut LN XOY plane.

DownLoad: Full-Size Img PowerPoint

图 2 PSOS图像, 图中散点区域为混沌海区域, 椭圆形区域为“岛屿”, 虚线为KAM曲线

Figure 2. PSOS image. The scattered area in the figure is a chaotic area; the elliptical area is called an “island”, the dashed line represents the KAM curve.

DownLoad: Full-Size Img PowerPoint

图 3 (4)式的实部与虚部在$y = 0$附近的图像

Figure 3. Illustration of the real part and imaginary part of Eq. (4) near $y = 0$.

DownLoad: Full-Size Img PowerPoint

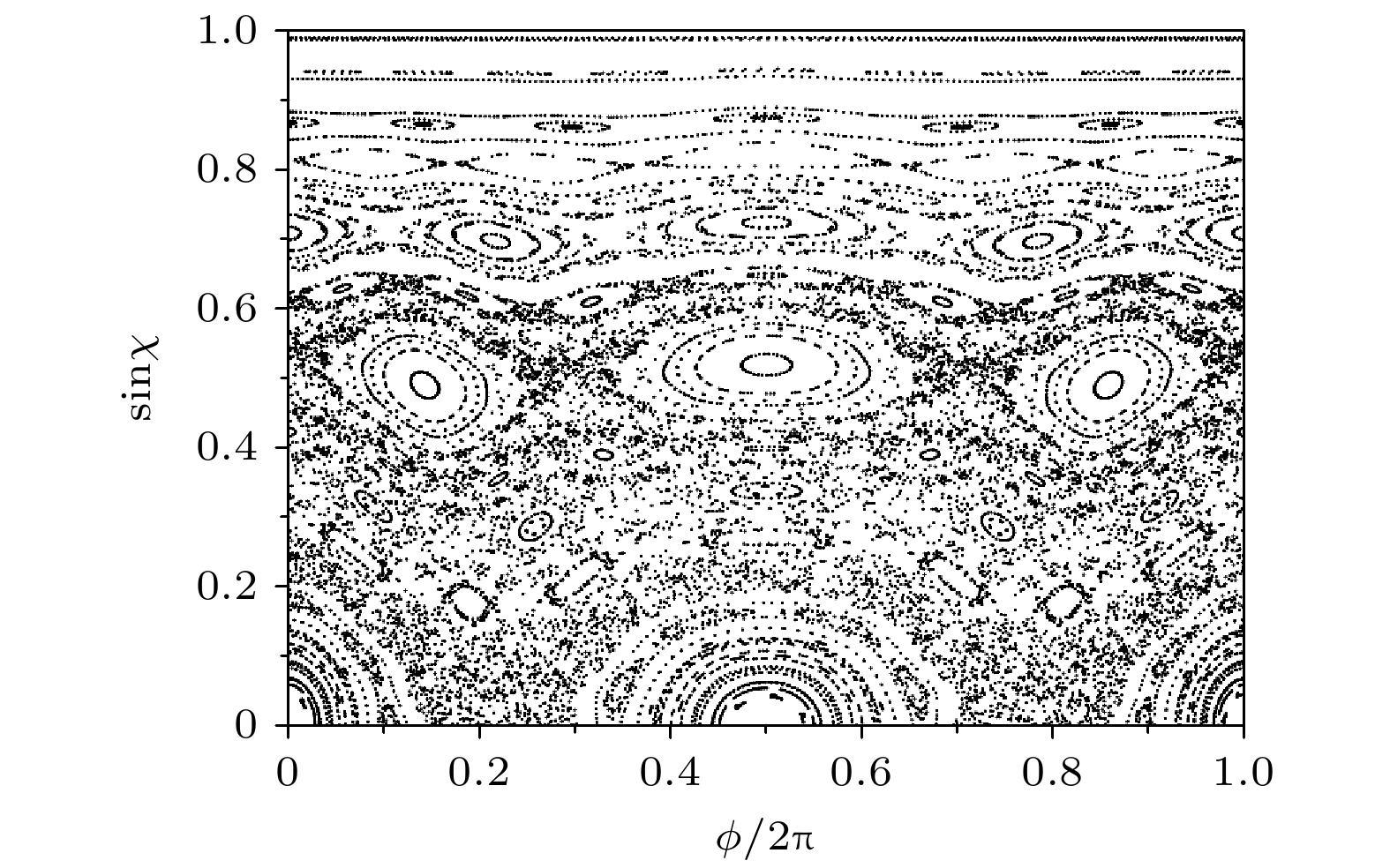
图 4 变形因子$\varepsilon $取　(a) 0, (b) 0.16, (c) 0.24, (d) 0.28, (e) 0.45时的TE_{20, 1}模式分布图, 远场辐射通量$S\left( \varphi \right)$角分布图以及PSOS

Figure 4. The distribution diagram of TE_{20, 1} mode, far-field radiation flux angle and PSOS with deformations $\varepsilon $ of (a) 0, (b) 0.16, (c) 0.24, (d) 0.28, (e) 0.45.

DownLoad: Full-Size Img PowerPoint

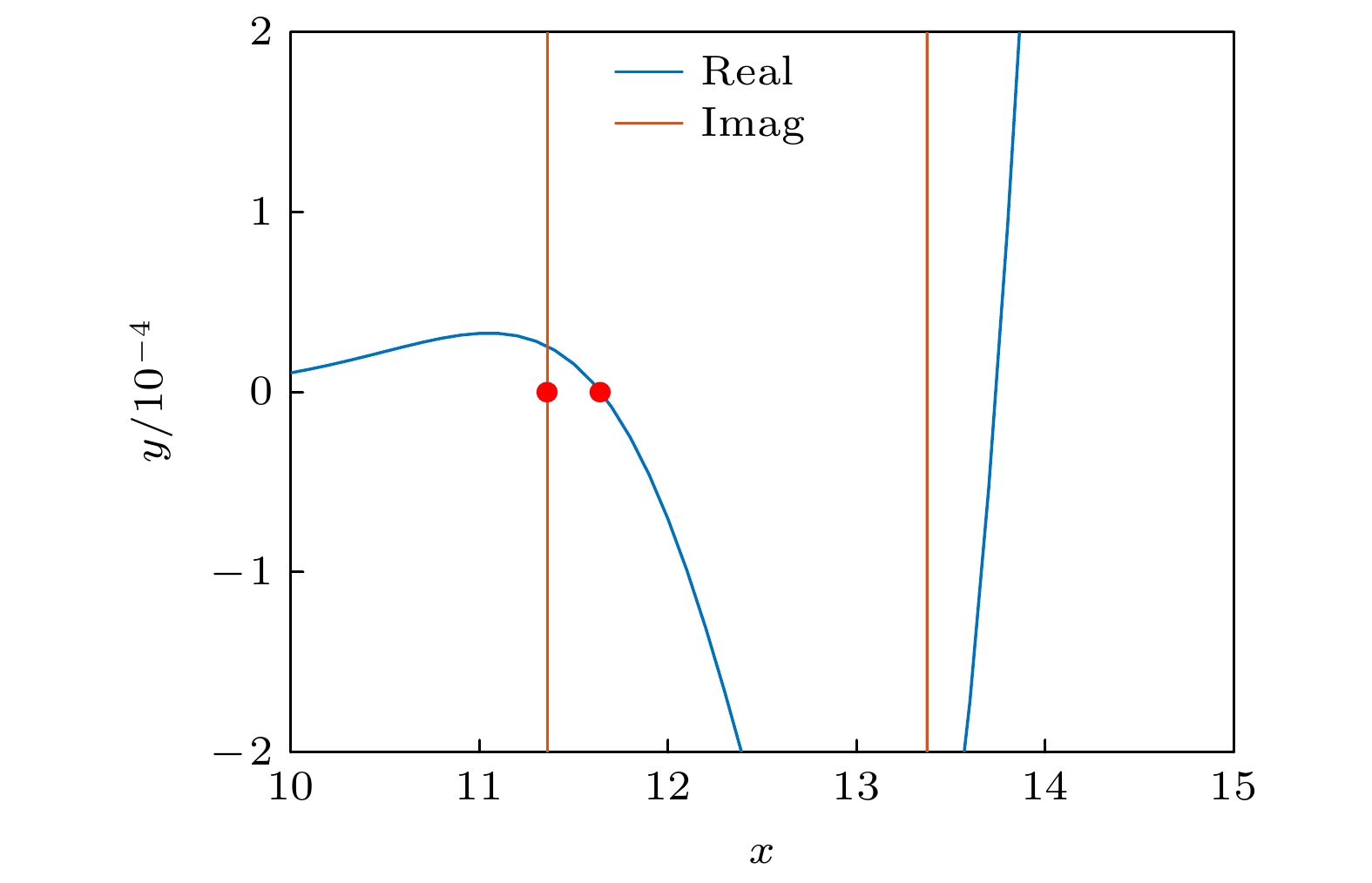
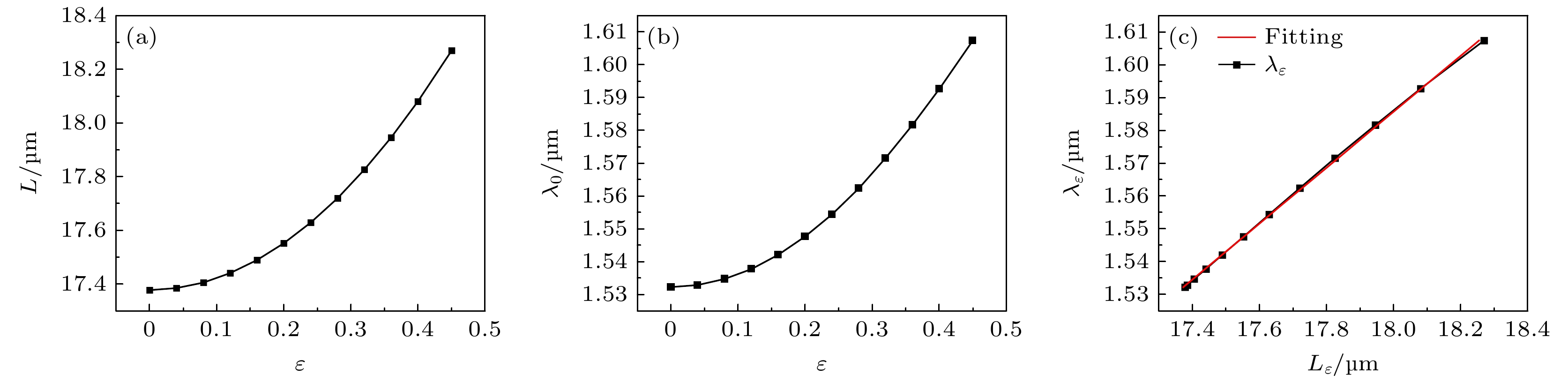
图 5 TE_{20, 1}模式分布　(a) 微盘周长${L_\varepsilon }$与变形因子$\varepsilon $间的关系; (b) 谐振波长${\lambda _\varepsilon }$与变形因子$\varepsilon $间的关系; (c) 谐振波长${\lambda _\varepsilon }$与微盘周长${L_\varepsilon }$间存在线性变化关系

Figure 5. Under the TE_{20, 1} mode: (a) resonant wavelength $ {\lambda }_{\varepsilon } $ variation with $\varepsilon $; (b) microdisk’s perimeter ${L_\varepsilon }$ variation with $\varepsilon $; (c) resonant wavelength $ {\lambda }_{\varepsilon } $ variation with perimeter ${L_\varepsilon }$ and linear fitting.

DownLoad: Full-Size Img PowerPoint

图 6 $Q$值与变形因子$\varepsilon $间的变化关系

Figure 6. $Q$ variation with $\varepsilon $.

DownLoad: Full-Size Img PowerPoint

图 7 方向性$D$与$\varepsilon $间的关系, $\varepsilon $间隔取为0.01

Figure 7. Directivity $D$ variation with $\varepsilon $, $\varepsilon $ increasing at intervals of 0.01.

DownLoad: Full-Size Img PowerPoint

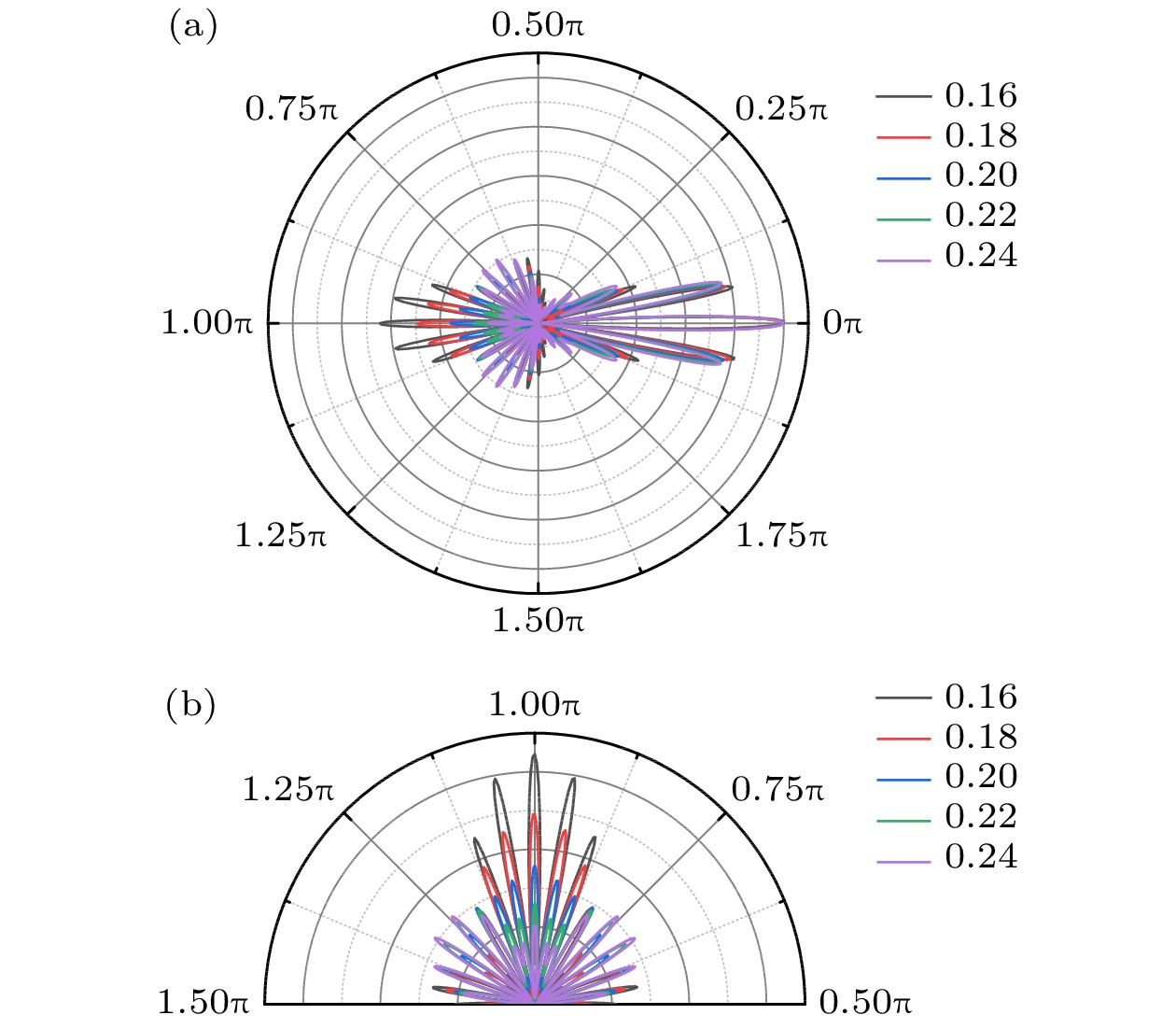
图 8 $\varepsilon $分别为0.16, 0.18, 0.20, 0.22, 0.24时, 以$S{\left( \varphi \right)_{{\text{max}}}}$归一化的 (a)全局远场辐射通量$S\left( \varphi \right)$角分布图, (b)角度在$90^\circ $—$270^\circ $之间的远场辐射通量$S\left( \varphi \right)$的角分布图

Figure 8. (a) Global far-field radiation flux angular distribution diagram; (b) the angular distribution of far-field radiation flux at angles between $90^\circ $ and $270^\circ $, with the deformation factor $\varepsilon $ taken as 0.16, 0.18, 0.20, 0.22, 0.24, according to the normalization of $S{\left( \varphi \right)_{{\text{max}}}}$.

DownLoad: Full-Size Img PowerPoint

表 1 不同$\varepsilon $的最大远场辐射通量密度$S{\left( \varphi \right)_{{\text{max}}}}$对应的定向角$\varphi $

Table 1. $S{\left( \varphi \right)_{{\text{max}}}}$ and $\varphi $ with different $\varepsilon $.

变形因子$\varepsilon $	最大远场辐射通量密度$S{\left( \varphi \right)_{{\text{max}}}}/({\text{W}}{\cdot} {{\text{m}}^{ - 2}})$	定向角$\varphi /(^\circ )$
0	$6.06 \times {10^1}$	17.98
0.04	$6.18 \times {10^1}$	179.75
0.08	$8.20 \times {10^1}$	180.25
0.12	$1.71 \times {10^2}$	0
0.16	$6.06 \times {10^2}$	0
0.20	$3.13 \times {10^3}$	0
0.24	$1.93 \times {10^4}$	0
0.28	$1.17 \times {10^5}$	0.50
0.32	$6.24 \times {10^5}$	0
0.36	$2.63 \times {10^6}$	359.00
0.40	$8.30 \times {10^6}$	0
0.45	$2.27 \times {10^7}$	0

DownLoad: CSV

map

[1]	Baaske M D, Foreman M R, Vollmer F 2014 Nat. Nanotechnol. 9 933 Google Scholar
[2]	Kippenberg T J, Holzwarth R, Diddams S A 2011 Science 332 555 Google Scholar
[3]	Kippenberg T J, Vahala K J 2008 Science 321 1172 Google Scholar
[4]	Michael C P, Srinivasan K, Johnson T J, Painter O, Lee K H, Hennessy K, Kim H, Hu E 2007 Appl. Phys. Lett. 90 051108 Google Scholar
[5]	Redding B, Ge L, Song Q H, Wiersig J, Solomon G S, Cao H 2012 Phys. Rev. Lett. 108 253902 Google Scholar
[6]	Fang W, Cao H, Solomon G S 2007 Appl. Phys. Lett. 90 081108 Google Scholar
[7]	Unterhinninghofen J, Wiersig J, Hentschel M 2008 Phys. Rev. E 78 016201 Google Scholar
[8]	Michler P, Kiraz A, Becher C, Schoenfeld W V, Petroff P M, Zhang L, Hu E, Imamoglu A 2000 Science 290 2282 Google Scholar
[9]	Jiang X F, Zou C L, Wang L, Gong Q H, Xiao Y F 2016 Laser Photonics Rev. 10 40 Google Scholar
[10]	Chern G D, Tureci H E, Stone A D, Chang R K, Kneissl M, Johnson N M 2003 Appl. Phys. Lett. 83 1710 Google Scholar
[11]	Kurdoglyan M S, Lee S Y, Rim S, Kim C M 2004 Opt. Lett. 29 2758 Google Scholar
[12]	Baryshnikov Y, Heider P, Parz W, Zharnitsky V 2004 Phys. Rev. Lett. 93 133902 Google Scholar
[13]	Gao J, Heider P, Chen C J, Yang X D, Husko C A, Wong C W 2007 Appl. Phys. Lett. 91 181101 Google Scholar
[14]	Wiersig J, Hentschel M 2006 Phys. Rev. A 73 031802 Google Scholar
[15]	Fang W, Yamilov A, Cao H 2005 Phys. Rev. A 72 023815 Google Scholar
[16]	Lee S Y, Kurdoglyan M S, Rim S, Kim C M 2004 Phys. Rev. A 70 023809 Google Scholar
[17]	Lebental M, Lauret J S, Hierle R, Zyss J 2006 Appl. Phys. Lett. 88 031108 Google Scholar
[18]	Wiersig J, Hentschel M 2008 Phys. Rev. Lett. 100 033901 Google Scholar
[19]	Yi C H, Kim M W, Kim C M 2009 Appl. Phys. Lett. 95 141107 Google Scholar
[20]	Song Q H, Fang W, Liu B Y, Ho S T, Solomon G S, Cao H 2009 Phys. Rev. A 80 041807 Google Scholar
[21]	Kim K, Bittner S, Jin Y H, Zeng Y Q, Wang Q J, Cao H 2023 Opt. Lett. 48 574 Google Scholar
[22]	Yan C L, Shi J W, Li P, Li H, Zhang J J 2014 Opt. Laser Technol. 56 285 Google Scholar
[23]	Fang Z W, Haque S, Farajollahi S, Luo H P, Lin J, Wu R B, Zhang J H, Wang Z, Wang M, Cheng Y, Lu T 2020 Phys. Rev. Lett. 125 173901 Google Scholar
[24]	Lin J T, Farajollahi S, Fang Z W, Yao N, Gao R H, Guan J L, Deng L, Lu T, Wang M, Zhang H S, Fang W, Qiao L L, Cheng Y 2022 Adv. Photonics 4 036001 Google Scholar
[25]	Foreman M R, Vollmer F 2013 New J. Phys. 15 083006 Google Scholar
[26]	Swaim J D, Knittel J, Bowen W P 2011 Appl. Phys. Lett. 99 243109 Google Scholar
[27]	Lee J, Rim S, Cho J, Kim C M 2008 Phys. Rev. Lett. 101 064101 Google Scholar
[28]	Farajollahi S, Fang Z W, Lin J T, Honari S, Cheng Y, Lu T 2023 Phys. Rev. A 108 033520 Google Scholar
[29]	Cao H, Wiersig J 2015 Rev. Mod. Phys. 87 61 Google Scholar
[30]	Ryu J W, Rim S, Park Y J, Kim C M, Lee S Y 2008 Phys. Lett. A 372 3531 Google Scholar
[31]	邹长铃, 董春华, 崔金明, 孙方稳, 杨勇, 吴晓伟, 韩正甫,郭光灿 2012 中国科学: 物理学力学天文学 42 1155 Zou C L, Dong C H, Cui J M, Sun F W, Yang Y, Wu X W, Han Z F, Guo G C 2012 Sci. Sin. Phys. Mech. Astron. 42 1155
[32]	Qi J W, Yan C L, Diehl L, Hentschel M, Wiersig J, Yu N F, Pflügl C, Belkin M A, Edamura T, Yamanishi M, Kan H, Capasso F 2009 New J. Phys. 11 125018 Google Scholar
[33]	Li J C, Huang Y T, Hao Y Z, Yang Y D, Xiao J L 2022 Single-mode Lasing Deformed Square Microcavity Lasers (USA: SPIE) p125011I
[34]	Wu R, Zhang J, Yao N, Fang W, Qiao L, Chai Z, Lin J, Cheng Y 2018 Opt. Lett. 43 4116 Google Scholar
[35]	Ilchenko V S, Savchenkov A A, Matsko A B, Maleki L 2004 Phys. Rev. Lett. 92 043903 Google Scholar
[36]	Pan Y, Lin G, Diallo S, Zhang X, Chembo Y K 2017 IEEE Photonics J. 9 1 Google Scholar
[37]	Wang L, Wang C, Wang J, Bo F, Zhang M, Gong Q, Loncar M, Xiao Y F 2018 Opt. Lett. 43 2917 Google Scholar
[38]	Gao A, Yang C, Chen L K, Zhang R, Luo Q, Wang W, Cao Q T, Hao Z Z, Bo F, Zhang G Q, Xu J J 2022 Photonics Res. 10 401 Google Scholar
[39]	Zhu D, Shao L B, Yu M J, Cheng R, Desiatov B, Xin C J, Hu Y W, Holzgrafe J, Ghosh S, Shams-Ansari A, Puma E, Sinclair N, Reimer C, Zhang M, Lončar M 2021 Adv. Opt. Photonics 13 242 Google Scholar
[40]	Gopalan V, Dierolf V, Scrymgeour D A 2007 Annu. Rev. Mater. Res. 37 449 Google Scholar
[41]	Sanna S, Schmidt W G 2010 Phys. Rev. B 81 214116 Google Scholar
[42]	Xiao Y F, Zou C L, Li Y, Dong C H, Han Z F, Gong Q H 2010 Front. Optoelectron. 3 109 Google Scholar
[43]	Yang Q F, Jiang X F, Cui Y L, Shao L B, Xiao Y F 2013 Phys. Rev. A 88 023810 Google Scholar
[44]	Jiang X F, Shao L B, Zhang S X, Yi X, Wiersig J, Wang L, Gong Q H, Loncar M, Yang L, Xiao Y F 2017 Science 358 344 Google Scholar
[45]	Xiao Y F, Jiang X F, Yang Q F, Wang L, Shi K B, Li Y, Gong Q H 2013 Laser Photonics Rev. 7 L51 Google Scholar
[46]	Boriskina S V, Benson T M, Sewell P, Nosich A I 2006 IEEE J. Sel. Top. Quantum Electron. 12 52 Google Scholar
[47]	Boriskina S V, Sewell P, Benson T M, Nosich A I 2004 J. Opt. Soc. Am. A 21 393 Google Scholar
[48]	Zelmon D E, Small D L, Jundt D 1997 J. Opt. Soc. Am. B 14 3319 Google Scholar

[1]	Li Yu-Qing, Wang Hong-Guang, Zhai Yong-Gui, Yang Wen-Jin, Wang Yue, Li Yun, Li Yong-Dong. Influence of quality factor on operating mode of TM₀₂ mode relativistic backwave oscillator. Acta Physica Sinica, 2024, 73(3): 035202. doi: 10.7498/aps.73.20231577
[2]	Xia Zhao-Sheng, Liu Yu-Hang, Bao Zheng, Wang Li-Hua, Wu Bo, Wang Gang, Wang Hui, Ren Xin-Gang, Huang Zhi-Xiang. Strong circular dichroism chiral metasurfaces generated by quasi bound state in continuum domain. Acta Physica Sinica, 2024, 73(17): 178102. doi: 10.7498/aps.73.20240834
[3]	Xiong Lei, Ding Hong-Wei, Li Guang-Yuan. Quadrupolar lattice plasmon modes induced by diffraction of high-quality factors in silver nanoparticle arrays. Acta Physica Sinica, 2022, 71(4): 047802. doi: 10.7498/aps.71.20211629
[4]	Jiang Li-Ying, Yi Ying-Ting, Yi Zao, Yang Hua, Li Zhi-You, Su Ju, Zhou Zi-Gang, Chen Xi-Fang, Yi You-Gen. A four-band perfect absorber based on high quality factor and high figure of merit of monolayer molybdenum disulfide. Acta Physica Sinica, 2021, 70(12): 128101. doi: 10.7498/aps.70.20202163
[5]	. Diffraction-induced quadrupolar lattice plasmon modes of high-quality factors for silver nanoparticle arrays. Acta Physica Sinica, 2021, (): . doi: 10.7498/aps.70.20211629
[6]	Chen Heng-Jie, Xue Hang, Li Shao-Xiong, Wang Zhen. A method of determining microwave dissipation of Josephson junctions with non-linear frequency response. Acta Physica Sinica, 2019, 68(11): 118501. doi: 10.7498/aps.68.20190167
[7]	Liu Hong, Zhu Jing-Ping, Wang Kai. Modification of geometrical attenuation factor of bidirectional reflection distribution function based on random surface microfacet theory. Acta Physica Sinica, 2015, 64(18): 184213. doi: 10.7498/aps.64.184213
[8]	Guo Ze-Bin, Tang Jun, Liu Jun, Wang Ming-Huan, Shang Cheng-Long, Lei Long-Hai, Xue Chen-Yang, Zhang Wen-Dong, Yan Shu-Bin. Optical model raciprocity of disk resonator excitated by tapered fiber. Acta Physica Sinica, 2014, 63(22): 227802. doi: 10.7498/aps.63.227802
[9]	Chen Xiang, Mi Xian-Wu. Characteristics of spontaneous emission from a two-level atom in a very high Q cavity. Acta Physica Sinica, 2011, 60(10): 104204. doi: 10.7498/aps.60.104204
[10]	Zhang Jing, Pan Wei, Yan Lian-Shan, Luo Bin. Dispersion management optimization of multi-wavelength all-optical regeneration based on self-phase modulation. Acta Physica Sinica, 2010, 59(10): 7002-7007. doi: 10.7498/aps.59.7002
[11]	Jiang Bin, Liu An-Jin, Chen Wei, Xing Ming-Xin, Zhou Wen-Jun, Zheng Wan-Hua. The characteristic of the stero-coupling high-Q photonic crystal slab cavity. Acta Physica Sinica, 2010, 59(12): 8548-8553. doi: 10.7498/aps.59.8548
[12]	Chen Wei, Xing Ming-Xin, Ren Gang, Wang Ke, Du Xiao-Yu, Zhang Ye-Jin, Zheng Wan-Hua. Design of high polarization and single-mode photonic crystal laser. Acta Physica Sinica, 2009, 58(6): 3955-3960. doi: 10.7498/aps.58.3955
[13]	Chen Yan-Ping, Wang Chuan-Bing, Zhou Guo-Cheng. Maser instability driven by an electron beam with losscone-beam distribution. Acta Physica Sinica, 2005, 54(7): 3221-3227. doi: 10.7498/aps.54.3221
[14]	Cheng Yuan-Ying, Wang You-Qing, Hu Jin, Li Jia-Rong. A novel eigenvector method for calculation of optical resonator modes and beam propagation. Acta Physica Sinica, 2004, 53(8): 2576-2582. doi: 10.7498/aps.53.2576
[15]	Du Qi-Zhen, Yang Hui-Zhu. . Acta Physica Sinica, 2002, 51(9): 2101-2108. doi: 10.7498/aps.51.2101
[16]	LI FU-LI. INFLUENCE OF CAVITY LOSS ON GENERATION OF STEADY STATE FOURTH-ORDER AND SQUARED AMPLITUDE SQUEEZING IN MICROMASER. Acta Physica Sinica, 1996, 45(4): 563-572. doi: 10.7498/aps.45.563
[17]	LI FU-LI. A THEORETICAL STUDY OF GENERATION OF A STRONG SQUEEZED CAVITY FIELD BY USE OF ONE PHOTON MICROMASER. Acta Physica Sinica, 1990, 39(11): 1721-1729. doi: 10.7498/aps.39.1721
[18]	LI TIE-CHENG, HO YU-PING. ON THE THEORY OF RAMAN MASERS. Acta Physica Sinica, 1965, 21(12): 1933-1950. doi: 10.7498/aps.21.1933
[19]	FANG LI-ZHI, LUO YI-ZU. ON THE LINEWIDTH OF LASERS. Acta Physica Sinica, 1964, 20(11): 1079-1089. doi: 10.7498/aps.20.1079
[20]	. ВЛИЯНЕ РАСПРЕДЕЛЕНИЯ ФОТОЭЛЕКТРОНОВ ПО СКОРОСТЯМ НА КАЧЕСТВО ИЗОБРАЖЕНИЯ В ЭМИССИОННЫХ ЭЛЕКТРОННО-ОПТИЧЕСКИХ СИСТЕМАХ (Ⅱ). Acta Physica Sinica, 1957, 13(1): 78-89. doi: 10.7498/aps.13.78

Catalog

Vol. 73, Issue 8 - 2024-04-20

Metrics

Abstract views: 1547
PDF Downloads: 35
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板