Theoretical study on macroscopic thermodynamic properties of NO<sup>+</sup> ion system

Hu Min-Li; Fang Fan; Fan Qun-Chao; Fan Zhi-Xiang; Li Hui-Dong; Fu Jia; Xie Feng

doi:10.7498/aps.72.20230541

Abstract

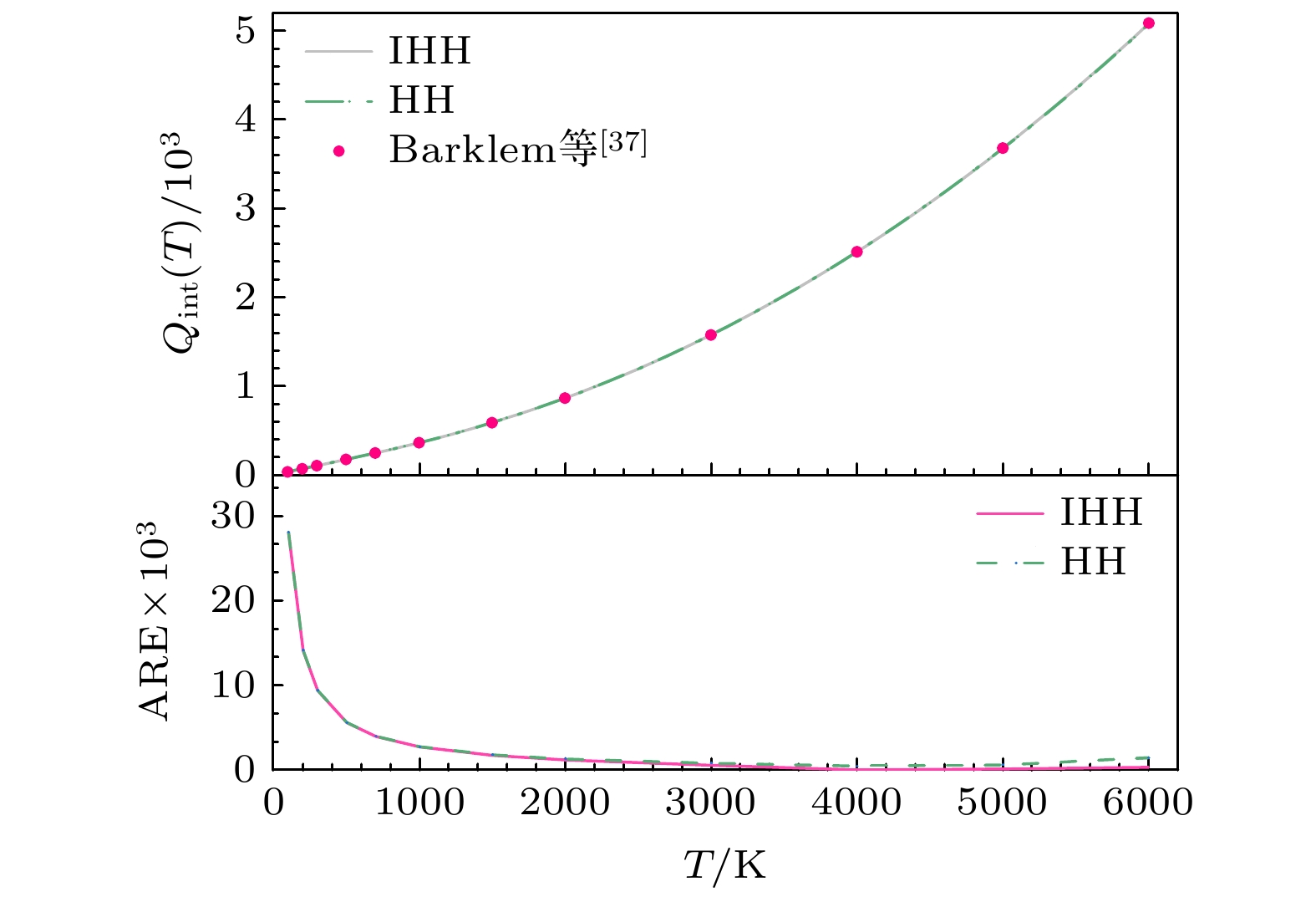
NO⁺ is one of the most important ions in the atmospheric ionosphere and ionospheric phenomena such as auroras, and is one of the most stable diatomic cations existing in interstellar clouds. It is crucial to understand the thermodynamic properties of NO⁺ ion for exploring the composition of interstellar gas. To obtain macroscopic thermodynamic properties of diatomic molecules and ions, a practical theoretical method is to determine the partition function associated with a potential model. This approach can be used to calculate various thermodynamic properties of the system based on the microscopic information.In this work, the improved Hulbert-Hirschfelder (IHH) based potential energy model is used to simulate the potential energy curve of NO⁺ in the ground electronic state. Then, the rovibrational energy levels for the ground electronic state of the NO⁺ are obtained by numerically solving the radial Schrödinger equation through using the LEVEL program for the IHH potential function. Finally, the total partition function and the thermodynamic properties such as the molar heat capacity, entropy, enthalpy and reduced molar Gibbs free energy of NO⁺ in a temperature range of 100–6000 K are calculated in the frame of the quantum statistical ensemble theory. The comparison indicates that the potential energy curve calculated based on IHH potential energy function is in better agreement with the experimental data. The root mean square error of IHH potential and experimental Rydberg-Klein-Rees (RKR) potential is 96.9 cm^–1, the root mean square error of Hulbert-Hirschfelder (HH) potential is 112.7 cm^–1, and the root mean square error of MRCI/aug-cc-pV6Z potential is 133 cm^–1. And the macroscopic thermodynamic properties of NO⁺ predicted by IHH are closer to the experimental values, which shows that the IHH potential model is also applicable to the ion system.A feasible method is presented to obtain the thermodynamic properties of gaseous diatomic ions based on microscopic information by constructing reliable analytical potential energy function associated with quantum statistical ensemble theory.

Keywords:

NO⁺ ion /
improved Hulburt-Hirschfelder potential /
partition function /
thermodynamic properties

Authors and contacts

1.
Key Laboratory of High Performance Scientific Computation, School of Science, Xihua University, Chengdu 610039, China
2.
Key Laboratory of Advanced Reactor Engineering and Safety of Ministry of Education, Collaborative Innovation Center of Advanced Nuclear Energy Technology, Institute of Nuclear and New Energy Technology, Tsinghua University, Beijing 100084, China

Corresponding author: Fan Qun-Chao, fanqunchao@mail.xhu.edu.cn ; Fan Zhi-Xiang, fanzhixiang@mail.xhu.edu.cn ;

Funds: Project supported by the Central Government Funds of Guiding Local Scientific and Technological Development for Sichuan Province (Grant No. 2021ZYD0050), the Natural Science Foundation of Sichuan Province, China (Grant No. 2022NSFSC1857), the Open Research Fund of Computational Physics Key Laboratory of Sichuan Province, Yibin University (Grant No. ybxyjswl-zd-2020-006), and the National Undergraduate Innovation and Entrepreneurship Training Program of China (Grant No. S202210623057).

FullText HTML : translate this paragraph

References

[1]	Petrou A L 2012 Chem. Geo. 308 50 Google Scholar
[2]	Ding Q C, Jia C S, Liu J Z, Li J, Du R F, Liu J Y, Peng X L, Wang C W, Tang H X 2022 Chem. Phys. Lett. 803 139844 Google Scholar
[3]	Peduzzi E, Boissonnet G, Maréchal F 2016 Fuel 181 207 Google Scholar
[4]	汪志诚 2013 热力学·统计物理 (第五版) (北京: 高等教育出版社) 第 1 页 Wang Z C 2013 Thermodynamic Statistical Physics (Vol. 5) (Beijing: Higher Education Press) p1 (in Chinese)
[5]	Kjelstrup S, Magnanelli E 2020 Trends Food Sci. Technol. 104 84 Google Scholar
[6]	Horton T E 1970 The Computation of Partition Functions and Thermo-chemistry Data for Atomic, Ionic, Diatomic, and Polyatomic Species Technical Report 32-1425, National Aeronautics and Space Administration (NASA), Jet Propulsion Laboratory, California Institute of Technology, Pasadena, CA (N-70 20647, NASA CR108970)
[7]	Bartl J, Bartlova M 1989 Contrib. Plasma Phys. 29 285 Google Scholar
[8]	Peterson K A, Flowers B A, Francisco J S 2001 J. Chem. Phys. 115 7513 Google Scholar
[9]	Capitelli M, Colonna G, Giordano D, Maraffa L, Casavola A, Minelli P, Pagano D, Pietanza L D, Taccogna F 2005 Tables of Internal Partition Functions and Thermodynamic Properties of High-Temperature Mars-Atmosphere Species from 50 K to 50000 K (Netherlands: European Space Agency Publications Division) p3
[10]	Maltsev M A, Kulikov A N, Morozov IV 2016 J. Phys. Conf. Ser. 774 012023 Google Scholar
[11]	Qin Z, Zhao J M, Liu L H 2018 J. Quant. Spectrosc. Radiat. Transfer. 210 1 Google Scholar
[12]	Liang R H, Liu Y M, Li F Y 2021 Contrib. Plasma Phys. 61 e202100036 Google Scholar
[13]	Eyube E S, Notani P P, Dikko A B 2022 Eur. Phys. J. Plus. 137 329 Google Scholar
[14]	Maltsev M A, Morozov I V, Osina E L 2019 High. Temp. 57 335 Google Scholar
[15]	Maltsev M A, Morozov I V, Osina E L 2019 High Temp. 57 37 Google Scholar
[16]	Maltsev M A, Morozov I V, Osina E L 2020 High Temp. 58 184 Google Scholar
[17]	Maltsev M A, Aksenova S A, Morozov I V, Minenkov Y, Osina E L 2023 J. Comput. Chem. 44 1189 Google Scholar
[18]	Tian H R, Fan Q C, Fan Z X, Fu J, Li H D, Ma J, Xie F 2022 Int. J. Quantum Chem. 122 e26983 Google Scholar
[19]	Partridge H, Langhoff S R, Bauschlicher C W 1990 J. Chem. Phys. 93 7179 Google Scholar
[20]	Cosby P C, Helm H 1981 J. Chem. Phys. 75 3882 Google Scholar
[21]	Werner H J, Rosmus P 1982 J. Mol. Spectrosc. 96 362 Google Scholar
[22]	Sheehan C H, Maurice J P S 2004 J. Geophys. Res. 109 A03302 Google Scholar
[23]	Liu H, Liu L H, Wei L Q, Chu B X, Qin Z Z, Jin G Z, Tong Z F, Dong L H, Li B 2020 Fuel 272 117738 Google Scholar
[24]	Smith D, Chippendale T W E, Španěl P 2014 Rapid Commun. Mass Spectrom. 28 1917 Google Scholar
[25]	Dias M, Gurusinghe R M, Suits A G 2022 J. Phys. Chem. A. 126 5354 Google Scholar
[26]	Chase M W 1998 Journal of Physical and Chemical Reference Data Monograph (Vol. 9) (New York: National Institute of Standards and Technology Gaithersburg) p1604
[27]	Dunham J L 1932 Phys. Rev. 41 721 Google Scholar
[28]	Ilieva T, Iliev I 2016 J. Mol. Spectrosc. 330 28 Google Scholar
[29]	Le Roy R J 2017 J. Quant. Spectrosc. Radiat. Transfer. 186 167 Google Scholar
[30]	Schwabl F 2006 Statistical Mechanics (2nd Ed.) (Berlin Heidelberg: Springer-Verlag) p409
[31]	Huber K P, Herzberg G 1950 Molecular Spectra and Molecular Structure: Spectra of Diatomic Molecules (New York: Van Nostrand Reinhold Company) p9
[32]	Gordillo-Vázquez F J, Kunc J A 1998 J. Appl. Phys. 84 4693 Google Scholar
[33]	Holbrook R, Kaledin L, Kunc J A 1993 Phys. Rev. E 47 1285 Google Scholar
[34]	Holbrook R, Kunc J A 1994 Phys. Plasmas. 1 1075 Google Scholar
[35]	Zhang J P, Cheng X L, Zhang H, Yang X D 2011 Chinese Phys. B. 20 060401 Google Scholar
[36]	Reddy R R, Nazeer Ahammed Y, Baba Basha D, Narasimhulu K, Siva Sankar Reddy L, Rama Gopal K 2006 J. Quant. Spectrosc. Radiat. Transfer. 97 344 Google Scholar
[37]	Barklem P S, Collet R 2016 Astron. Astrophys. 588 A96 Google Scholar

Cited By

图 1 NO⁺离子的势能曲线对比

Figure 1. Comparison of potential energy curves of NO⁺.

DownLoad: Full-Size Img PowerPoint

图 2 NO⁺离子的内部配分函数与其他数据^[37]结果对比(ARE为相对误差的绝对值)

Figure 2. Comparison of internal partition function of NO⁺ and other data^[37] (ARE means the absolute relative errors).

DownLoad: Full-Size Img PowerPoint

图 3 NO⁺离子摩尔热容与NIST实验数据^[26]对比

Figure 3. Comparison of molar heat capacity of NO⁺ and NIST experimental data^[26].

DownLoad: Full-Size Img PowerPoint

图 4 NO⁺离子相对摩尔焓与NIST实验数据^[26]对比

Figure 4. Comparison of the molar enthalpy increment of NO⁺ and NIST experimental data^[26].

DownLoad: Full-Size Img PowerPoint

图 5 NO⁺离子摩尔熵与NIST实验数据^[26]对比

Figure 5. Comparison of the molar entropy of NO⁺ and NIST experimental data^[26].

DownLoad: Full-Size Img PowerPoint

图 6 NO⁺离子约化摩尔吉布斯自由能与NIST实验数据^[26]对比

Figure 6. Comparison of the reduced molar Gibbs free energy of NO⁺ and NIST experimental data^[26].

DownLoad: Full-Size Img PowerPoint

表 1 NO⁺离子不同势能及其与RKR实验值^[36]的误差值

Table 1. Different potential energy of NO⁺ ion and its absolute error with the experimental value of RKR^[36].

r/Å	$ {E_{{\text{RKR}}}} $/cm^–1[36]	$ {E_{{\text{IHH}}}} $/cm^–1	$\left\| {\Delta {E_{ {\text{RKR–IHH} } } }} \right\|$/cm^–1	$ {E_{{\text{HH}}}} $/cm^–1	$ \left\| {\Delta {E_{{\text{RKR–HH}}}}} \right\| $/cm^–1	$ {E_{{\text{MRCI}}}} $/cm^–1[35]	$ \left\| {\Delta {E_{{\text{RKR–MRCI}}}}} \right\| $/cm^–1
0.926	16911.0	17037.50	127	16886.09	25	17113.82	203
0.934	14761.9	14825.64	64	14721.53	40	14833.44	72
0.943	12580.2	12561.20	19	12494.49	86	12417.22	163
0.953	10366.1	10382.87	17	10342.77	23	10343.47	23
0.963	8119.40	8115.441	4	8094.606	25	8111.838	8
0.977	5840.20	5839.586	1	5830.842	9	5833.200	7
0.995	3528.50	3522.741	6	3520.407	8	3526.113	2
1.022	1184.30	1161.440	23	1161.306	23	1142.913	41
1.109	1184.30	1187.712	3	1187.826	4	1160.835	23
1.147	3528.50	3505.745	23	3507.331	21	3502.362	26
1.175	5840.20	5886.843	47	5892.355	52	5887.909	48
1.199	8119.40	8269.826	150	8282.220	163	8289.580	170
1.211	10366.1	10285.10	81	10305.88	60	10179.40	187
1.241	12580.2	12581.93	2	12615.36	35	12503.09	77
1.261	14761.9	14905.62	144	14955.40	194	14955.96	194
1.280	16911.0	17187.37	276	17256.83	346	17219.91	309
RMSD			96.9		112.7		133

DownLoad: CSV

表 2 HH, IHH势模型计算得到的NO⁺离子不同热力学性质的RMSD

Table 2. RMSD of HH and IHH potential models for different thermodynamic properties of NO⁺ ions.

热力学量	HH	IHH
$ {C_{\text{m}}} $/(J·mol^–1·K^–1)	0.053912	0.021733
$ {S_{\text{m}}} $/(J·mol^–1·K^–1)	0.035233	0.022930
$\Delta H$/(kJ·mol^–1)	0.082503	0.034329
$ {G_{\text{r}}} $/(J·mol^–1·K^–1)	0.021079	0.017711

DownLoad: CSV

map

[1]	Petrou A L 2012 Chem. Geo. 308 50 Google Scholar
[2]	Ding Q C, Jia C S, Liu J Z, Li J, Du R F, Liu J Y, Peng X L, Wang C W, Tang H X 2022 Chem. Phys. Lett. 803 139844 Google Scholar
[3]	Peduzzi E, Boissonnet G, Maréchal F 2016 Fuel 181 207 Google Scholar
[4]	汪志诚 2013 热力学·统计物理 (第五版) (北京: 高等教育出版社) 第 1 页 Wang Z C 2013 Thermodynamic Statistical Physics (Vol. 5) (Beijing: Higher Education Press) p1 (in Chinese)
[5]	Kjelstrup S, Magnanelli E 2020 Trends Food Sci. Technol. 104 84 Google Scholar
[6]	Horton T E 1970 The Computation of Partition Functions and Thermo-chemistry Data for Atomic, Ionic, Diatomic, and Polyatomic Species Technical Report 32-1425, National Aeronautics and Space Administration (NASA), Jet Propulsion Laboratory, California Institute of Technology, Pasadena, CA (N-70 20647, NASA CR108970)
[7]	Bartl J, Bartlova M 1989 Contrib. Plasma Phys. 29 285 Google Scholar
[8]	Peterson K A, Flowers B A, Francisco J S 2001 J. Chem. Phys. 115 7513 Google Scholar
[9]	Capitelli M, Colonna G, Giordano D, Maraffa L, Casavola A, Minelli P, Pagano D, Pietanza L D, Taccogna F 2005 Tables of Internal Partition Functions and Thermodynamic Properties of High-Temperature Mars-Atmosphere Species from 50 K to 50000 K (Netherlands: European Space Agency Publications Division) p3
[10]	Maltsev M A, Kulikov A N, Morozov IV 2016 J. Phys. Conf. Ser. 774 012023 Google Scholar
[11]	Qin Z, Zhao J M, Liu L H 2018 J. Quant. Spectrosc. Radiat. Transfer. 210 1 Google Scholar
[12]	Liang R H, Liu Y M, Li F Y 2021 Contrib. Plasma Phys. 61 e202100036 Google Scholar
[13]	Eyube E S, Notani P P, Dikko A B 2022 Eur. Phys. J. Plus. 137 329 Google Scholar
[14]	Maltsev M A, Morozov I V, Osina E L 2019 High. Temp. 57 335 Google Scholar
[15]	Maltsev M A, Morozov I V, Osina E L 2019 High Temp. 57 37 Google Scholar
[16]	Maltsev M A, Morozov I V, Osina E L 2020 High Temp. 58 184 Google Scholar
[17]	Maltsev M A, Aksenova S A, Morozov I V, Minenkov Y, Osina E L 2023 J. Comput. Chem. 44 1189 Google Scholar
[18]	Tian H R, Fan Q C, Fan Z X, Fu J, Li H D, Ma J, Xie F 2022 Int. J. Quantum Chem. 122 e26983 Google Scholar
[19]	Partridge H, Langhoff S R, Bauschlicher C W 1990 J. Chem. Phys. 93 7179 Google Scholar
[20]	Cosby P C, Helm H 1981 J. Chem. Phys. 75 3882 Google Scholar
[21]	Werner H J, Rosmus P 1982 J. Mol. Spectrosc. 96 362 Google Scholar
[22]	Sheehan C H, Maurice J P S 2004 J. Geophys. Res. 109 A03302 Google Scholar
[23]	Liu H, Liu L H, Wei L Q, Chu B X, Qin Z Z, Jin G Z, Tong Z F, Dong L H, Li B 2020 Fuel 272 117738 Google Scholar
[24]	Smith D, Chippendale T W E, Španěl P 2014 Rapid Commun. Mass Spectrom. 28 1917 Google Scholar
[25]	Dias M, Gurusinghe R M, Suits A G 2022 J. Phys. Chem. A. 126 5354 Google Scholar
[26]	Chase M W 1998 Journal of Physical and Chemical Reference Data Monograph (Vol. 9) (New York: National Institute of Standards and Technology Gaithersburg) p1604
[27]	Dunham J L 1932 Phys. Rev. 41 721 Google Scholar
[28]	Ilieva T, Iliev I 2016 J. Mol. Spectrosc. 330 28 Google Scholar
[29]	Le Roy R J 2017 J. Quant. Spectrosc. Radiat. Transfer. 186 167 Google Scholar
[30]	Schwabl F 2006 Statistical Mechanics (2nd Ed.) (Berlin Heidelberg: Springer-Verlag) p409
[31]	Huber K P, Herzberg G 1950 Molecular Spectra and Molecular Structure: Spectra of Diatomic Molecules (New York: Van Nostrand Reinhold Company) p9
[32]	Gordillo-Vázquez F J, Kunc J A 1998 J. Appl. Phys. 84 4693 Google Scholar
[33]	Holbrook R, Kaledin L, Kunc J A 1993 Phys. Rev. E 47 1285 Google Scholar
[34]	Holbrook R, Kunc J A 1994 Phys. Plasmas. 1 1075 Google Scholar
[35]	Zhang J P, Cheng X L, Zhang H, Yang X D 2011 Chinese Phys. B. 20 060401 Google Scholar
[36]	Reddy R R, Nazeer Ahammed Y, Baba Basha D, Narasimhulu K, Siva Sankar Reddy L, Rama Gopal K 2006 J. Quant. Spectrosc. Radiat. Transfer. 97 344 Google Scholar
[37]	Barklem P S, Collet R 2016 Astron. Astrophys. 588 A96 Google Scholar

[1]	Fan Jun-Yu, Gao Nan, Wang Peng-Ju, Su Yan. Intermolecular interactions and thermodynamic properties of LLM-105. Acta Physica Sinica, 2024, 73(4): 046501. doi: 10.7498/aps.73.20231696
[2]	Zhu Cheng, Chen Xian-Hui, Wang Cheng, Song Ming, Xia Wei-Dong. Calculation of thermodynamic properties and transport coefficients of Ar-C-Si plasma. Acta Physica Sinica, 2023, 72(12): 125202. doi: 10.7498/aps.72.20222390
[3]	Jian Jun, Lei Jiao, Fan Qun-Chao, Fan Zhi-Xiang, Ma Jie, Fu Jia, Li Hui-Dong, Xu Yong-Gen. Theoretical study on thermodynamic properties of NO gas. Acta Physica Sinica, 2020, 69(5): 053301. doi: 10.7498/aps.69.20191723
[4]	Zhao Yu-Na, Cong Hong-Lu, Cheng Shuang, Yu Na, Gao Tao, Ma Jun-Gang. First-principles study of lattice dynamical and thermodynamic properties of Li₂NH. Acta Physica Sinica, 2019, 68(13): 137102. doi: 10.7498/aps.68.20190139
[5]	Deng Shi-Jie, Zhao Yu-Hong, Hou Hua, Wen Zhi-Qin, Han Pei-De. Structural, mechanical and thermodynamic properties of Ti2AlX (X= C, N) at high pressure. Acta Physica Sinica, 2017, 66(14): 146101. doi: 10.7498/aps.66.146101
[6]	Tang Wen-Hui, Xu Bin-Bin, Ran Xian-Wen, Xu Zhi-Hong. Equations of state and thermodynamic properties of hot plasma. Acta Physica Sinica, 2017, 66(3): 030505. doi: 10.7498/aps.66.030505
[7]	Wu Ruo-Xi, Liu Dai-Jun, Yu Yang, Yang Tao. First-principles investigations on structure and thermodynamic properties of CaS under high pressures. Acta Physica Sinica, 2016, 65(2): 027101. doi: 10.7498/aps.65.027101
[8]	Men Fu-Dian, Wang Bing-Fu, He Xiao-Gang, Wei Qun-Mei. Thermodynamic properties of a weakly interacting Fermi gas in a strong magnetic field. Acta Physica Sinica, 2011, 60(8): 080501. doi: 10.7498/aps.60.080501
[9]	Li Xiao-Feng, Liu Zhong-Li, Peng Wei-Min, Zhao A-Ke. Elastic and thermodynamic properties of CaPo under pressure via first-principles calculations. Acta Physica Sinica, 2011, 60(7): 076501. doi: 10.7498/aps.60.076501
[10]	Li Shi-Na, Liu Yong. First-principles calculation of elastic and thermodynamic properties of copper nitride. Acta Physica Sinica, 2010, 59(10): 6882-6888. doi: 10.7498/aps.59.6882
[11]	Xu Bu-Yi, Chen Jun-Rong, Cai Jing, Li Quan, Zhao Ke-Qing. Theoretical study on the structure，spectra and thermodynamic property of 2-（toluene-4-sulfonylamino）-benzoic. Acta Physica Sinica, 2009, 58(3): 1531-1536. doi: 10.7498/aps.58.1531
[12]	Chen Yi, Shen Jiang. Structural and thermodynamic properties of Fe based compounds with NaZn13-type. Acta Physica Sinica, 2009, 58(13): 141-S145. doi: 10.7498/aps.58.141
[13]	Liu Na-Na, Song Ren-Bo, Sun Han-Ying, Du Da-Wei. The electronic structure and thermodynamic properties of Mg2Sn from first-principles calculations. Acta Physica Sinica, 2008, 57(11): 7145-7150. doi: 10.7498/aps.57.7145
[14]	Li Quan, Zhu Zheng-He. The potential energy function and thermodynamic properties of AuZn and AuAl for the ground states and low-lying excited states. Acta Physica Sinica, 2008, 57(6): 3419-3424. doi: 10.7498/aps.57.3419
[15]	Song Hai-Feng, Liu Hai-Feng. Theoretical study of thermodynamic properties of metal Be. Acta Physica Sinica, 2007, 56(5): 2833-2837. doi: 10.7498/aps.56.2833
[16]	Yuan Du-Qi. The influence of weak interaction on thermodynamic properties and the stability of imperfect Bose gas. Acta Physica Sinica, 2006, 55(4): 1634-1638. doi: 10.7498/aps.55.1634
[17]	Men Fu-Dian. Thermodynamic properties of a weakly interacting Fermi gas in weak magnetic field. Acta Physica Sinica, 2006, 55(4): 1622-1627. doi: 10.7498/aps.55.1622
[18]	Su Guo-Zhen, Chen Li-Xuan. Thermodynamic properties of a weakly interacting Fermi gas. Acta Physica Sinica, 2004, 53(4): 984-990. doi: 10.7498/aps.53.984
[19]	Zhu Zhi-Yan, Zhu Zheng-He, Gao Tao, Jiang Gang. Statistical thermodynamics for equilibrium distribution of Au48+—Au52+ in Au plasma. Acta Physica Sinica, 2004, 53(10): 3330-3335. doi: 10.7498/aps.53.3330
[20]	Zhang Ya-Nan, Yan Shi-Lei. Thermodynamic properties of random transverse mixed Ising spin system with cryst al field. Acta Physica Sinica, 2003, 52(11): 2890-2895. doi: 10.7498/aps.52.2890

Catalog

Vol. 72, Issue 16 - 2023-08-20

Metrics

Abstract views: 2849
PDF Downloads: 50
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板

Theoretical study on macroscopic thermodynamic properties of NO⁺ ion system

Abstract

Authors and contacts

Corresponding author: Fan Qun-Chao, fanqunchao@mail.xhu.edu.cn ; Fan Zhi-Xiang, fanzhixiang@mail.xhu.edu.cn ;

FullText HTML : translate this paragraph

References

Cited By

Catalog

Metrics

Authors

Referees

About Journal

About

Search

Article

留言板

Theoretical study on macroscopic thermodynamic properties of NO+ ion system

Abstract

Authors and contacts

Corresponding author: Fan Qun-Chao, fanqunchao@mail.xhu.edu.cn ; Fan Zhi-Xiang, fanzhixiang@mail.xhu.edu.cn ;

FullText HTML : translate this paragraph

References

Cited By

Catalog

Metrics

Publishing process

Authors

Referees

About Journal

About

Theoretical study on macroscopic thermodynamic properties of NO⁺ ion system