-
The “knee” of cosmic ray spectra reflects the maximum energy accelerated by galactic cosmic ray sources or the limit to the ability of galaxy to bind cosmic rays. The measuring of individual energy spectra is a crucial tool to ascertain the origin of the knee. However, the measuring of energy and the identifying of primary nuclei are the foundation of measuring the energy spectra of individual components. The Extensive Air Shower of cosmic rays in the knee energy region is simulated via CORSIKA software. The energy resolution for different secondary components (include electron, gamma, muon, neutron and Cherenkov light) and primary nuclei identification capability are studied. The energy reconstruction by using electromagnetic particles (electron, gamma and Cherenkov light) in the energy around “knee” is better than by using other secondary particles. The resolution is 10%–19% for proton, and 4%–8% for iron. For the case of primary nuclei identification capability, the discriminability of density of muons is best both at low (~100 TeV) and high (~10 PeV) energy, the discriminability of the shape of lateral distribution of electron and gamma-rays are good at low energy and the discriminability of density of neutrons is good at high energy. The differences between the lateral distributions of secondary particles simulated by EPOS-LHC and QGSJet-Ⅱ-04 hadronic model are studied. For electron, gamma and Cherenkov light, the differences of the number of particles are within 5%; for muon, when the perpendicular distance from the shower axis is greater than 100 m, the difference of the muon number is within 5%; for neutron, the difference in neutron number between the two models is larger than 10%. The results in this work can provide important information for selecting the secondary components and detector type during energy reconstruction and identifying the primary nuclei of cosmic rays in the knee region.
-
Keywords:
- extensive air shower /
- cosmic rays /
- composition and phase identification /
- energy reconstructions
[1] Prosin V V, Berezhnev S F, Budnev N M, et al. 2014 Nucl. Instrum. Methods Phys. Res. Sect. A 756 94
Google Scholar
[2] Blümer J, Engel R, Hörandel J R 2009 Prog. Part. Nucl. Phys. 63 293
Google Scholar
[3] Rújula De A 2006 Nucl. Phys. B 151 23
Google Scholar
[4] Ahn H S, Allison P, Bagliesi M G 2009 Astrophys. J. 707 593
Google Scholar
[5] Barao F 2004 Nucl. Instruments Methods Phys. Res. Sect. A 535 134
Google Scholar
[6] Alemanno F, An Q 2021 Phys. Rev. Lett. 126 201102
Google Scholar
[7] An Q, Asfandiyarov R, Azzarello P 2019 Sci. Adv. 5 3793
Google Scholar
[8] Chang J, Ambrosi G, An Q 2017 Astropart. Phys. 95 6
Google Scholar
[9] Sparvoli R 2013 Nucl. Phys. B 239 115
Google Scholar
[10] Antoni T, Apel W D, Badea A F 2005 Astropart. Phys. 24 1
Google Scholar
[11] Bartoli B, Bernardini P, Bi X J, Cao Z 2017 Astropart. Phys. 93 46
Google Scholar
[12] Ma X H, Bi Y J, Cao Z 2022 Chin. Phys. C 46 030001
Google Scholar
[13] Abbasi R, Abdou Y, Ackermann M 2013 Nucl. Instrum. Methods. Phys. Res. Sect. A 700 188
Google Scholar
[14] Abbasi R U, Abe M, Abu-Zayyad T 2018 Astro. J. 865 74
Google Scholar
[15] Amenomori M, Bao Y W 2021 Phys. Rev. Lett. 127 031102
Google Scholar
[16] Apel W D, Arteaga-Velázquez J C 2011 Phys. Rev. Lett. 107 171104
Google Scholar
[17] Apel W D, Arteaga-Velázquez J C 2013 Phys. Rev. D 87 081101
Google Scholar
[18] Bartoli B, Bernardini P, Bi X J, Cao Z 2015 Phys. Rev. D 92 092005
Google Scholar
[19] Aartsen M G, Abbasi R 2020 Phys. Rev. D 102 122001
Google Scholar
[20] Aartsen M G, Ackermann M, Adams J 2019 Phys. Rev. D 100 082002
Google Scholar
[21] Heck D, Knapp J, Capdevielle J N, Schatz G, Thouw T 1998 CORSIKA: A Monte Carlo Code to Simulate Extensive Air Showers
[22] Capdevielle J N, Cohen F 2005 J. Phys. G 31 507
Google Scholar
[23] Apel W D, Badea A F, Bekk K 2006 Astropart. Phys. 24 467
Google Scholar
[24] Feng Y L, Zhang Y, Chen T L 2019 Chin. Phys. C 43 075002
Google Scholar
[25] Alexandru C E, Alexandru J, Lavinia-Elena G 2019 Chin. Phys. C 43 083001
Google Scholar
[26] 李骢 2018 博士学位论文 (北京: 中国科学院大学)
Li C 2018 Ph. D. Dissertation (Beijing: University of Chinese Academy of Sciences) (in Chinese)
[27] Rivera-Rangel D, Arteaga-Velázquez J C 2021 37th Inter-national Cosmic Ray Conference (ICRC 2021) Online–Berlin, Germany July 1223, 2021 p3721
[28] Aharonian F, An Q, Axikegu 2021 Chin. Phys. C 45 025002
Google Scholar
[29] Conceição R, Peres L 2021 Eur. Phys. J. C 81 1
Google Scholar
[30] Yin L Q, Zhang S S, Cao Z, Bi B Y 2019 Chin. Phys. C 43 075001
Google Scholar
[31] Jin C, Chen S Z, He H H 2020 Chin. Phys. C 44 065002
Google Scholar
-
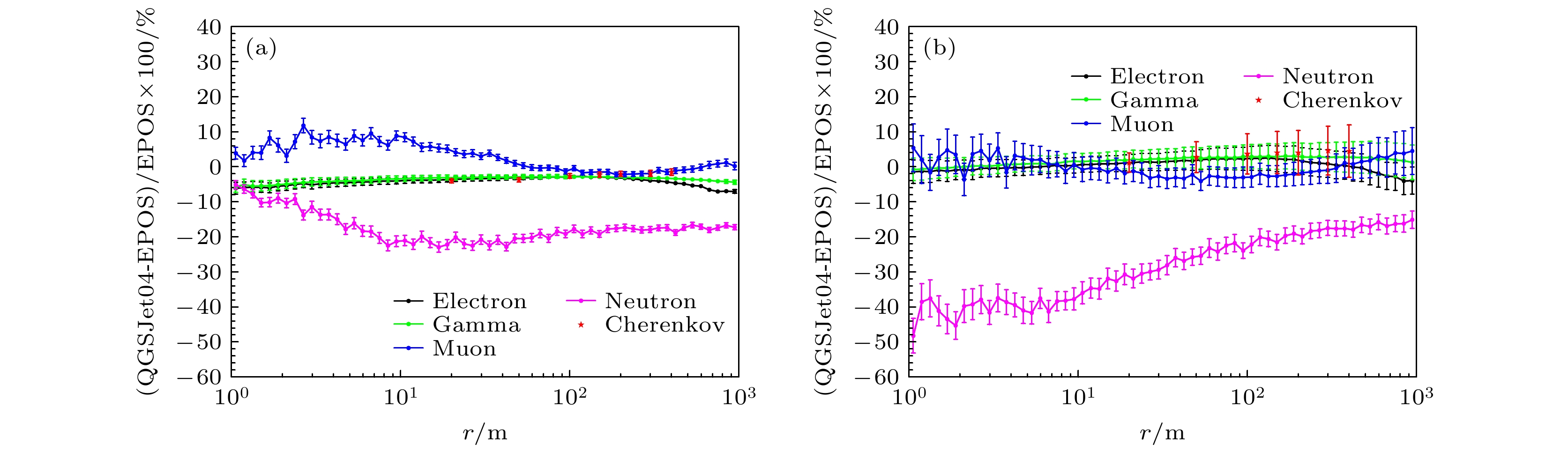
图 7 EPOS-LHC强相互作用模型和QGSJet-Ⅱ-04模型模拟的各种次级粒子横向分布的差异百分比, 其中原初粒子为不同能量的质子 (a) log10(E/GeV) = 5.1; (b) log10(E/GeV) = 6.9
Figure 7. Difference in percentage of the lateral distribution of secondary particles between EPOS-LHC and QGSJet-Ⅱ-04 hadronic interaction model, in which the primary particles are protons with different energies: (a) log10(E/GeV) = 5.1; (b) log10(E/GeV) = 6.9
图 8 EPOS-LHC强相互作用模型和QGSJet-Ⅱ-04模型模拟的各种次级粒子横向分布的差异百分比, 其中原初粒子为不同能量的铁核 (a) log10(E/GeV) = 5.1; (b) log10(E/GeV) = 6.9
Figure 8. Difference in percentage of the lateral distribution of secondary particles between EPOS-LHC and QGSJet-Ⅱ-04 hadronic interaction model, in which the primary particles are irons with different energies: (a) log10(E/GeV) = 5.1; (b) log10(E/GeV) = 6.9.
图 17 原初粒子铁核能量log10(E/GeV) = 6.1时, 垂直入射(θ = 0°)和天顶角为45° (θ = 45°)时次级成分中
$ {\rho }_{\rm{e}} $ (a),$ {s}_{\rm{\gamma }} $ (b),${\rho }_{\text{μ}}$ (c),$ {\rho }_{\rm{n}} $ (d), 以及$r=50\;{\rm{m}}\left(\rm{e}\right), r=150\;{\rm{m}}$ (f)处ρC的分布对比Figure 17. Comparison of the distribution of
$ {\rho }_{\rm{e}} $ (a),$ {s}_{\rm{\gamma }} $ (b),${\rho }_{\text{μ}}$ (c),$ {\rho }_{\rm{n}} $ (d) and$ {\rho }_{\rm{C}} $ at$r=50~\rm{m}~\left(\rm{e}\right)$ ,$r=150~\rm{m}$ (f) for θ = 0° and θ = 45°. The primary particle is iron with energy log10(E/GeV) = 6.1.
图 1 能量为log10(E/GeV) = 5.1, 原初粒子为质子(黑色)和铁核(红色)在EAS中次级成分的种类和个数
Figure 1. Type and counts of secondary components in the EAS, the primary particles are proton (black) and iron (red). Energy of the primary particle is log10(E/GeV) = 5.1.
图 2 不同原初粒子在EAS过程中产生的次级成分的横向分布 (a) 能量log10(E/GeV) = 5.1, 原初粒子为质子; (b) 能量log10(E/GeV) = 6.9, 原初粒子为铁核
Figure 2. Lateral distribution of secondary components produced by different primary particles during EAS: (a) Primary particle is proton with energy log10(E/GeV) = 5.1; (b) primary particle is iron with energy log10(E/GeV) = 6.9.
图 3 不同原初粒子在EAS过程中产生的次级成分的数量在探测平面的分布 (a) 能量log10(E/GeV) = 5.1, 原初粒子为质子; (b) 能量log10(E/GeV) = 6.9, 原初粒子为铁核
Figure 3. Distribution of the number of secondary components produced by different primary particles during EAS in the detection plane: (a) Primary particle is proton with energy log10(E/GeV) = 5.1; (b) primary particle is iron with energy log10(E/GeV) = 6.9.
图 4 次级粒子中伽马射线(a)和电子(b)的拟合参数
$ s, \varDelta$ 的相关性 (原初粒子为质子和铁核, 蓝色虚线为拟合曲线)Figure 4. Dependence between parameters
$ s $ and$\varDelta $ in gamma (a) and electron (b) lateral distribution fitting (Shower is induced by proton and iron respectively, and the blue dotted line is the fitting curve).
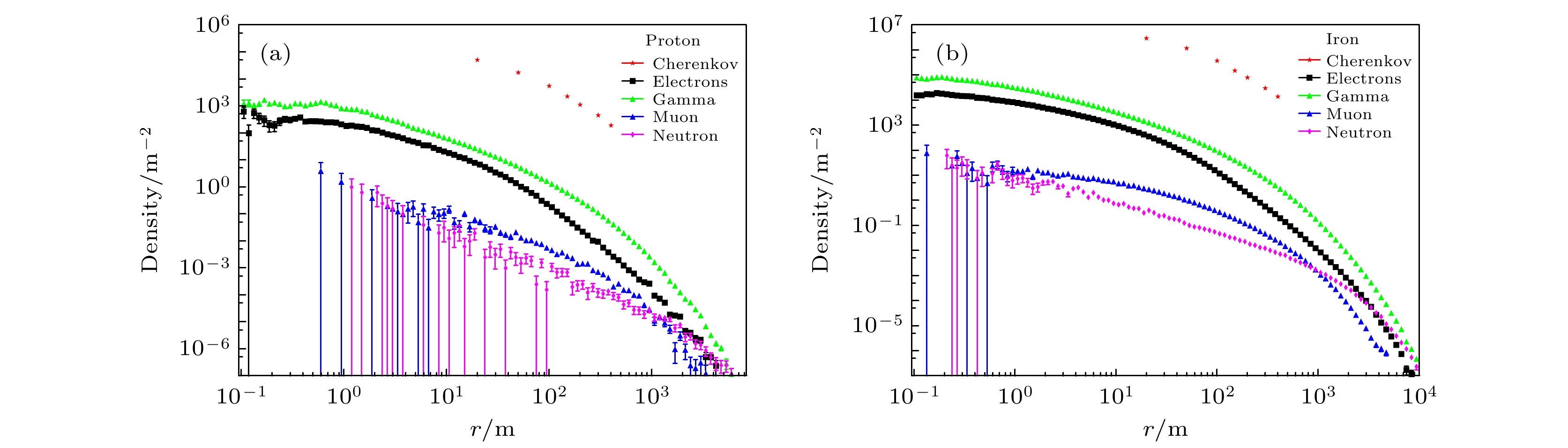
图 5 原初粒子在EAS中产生次级成分的横向分布拟合结果 (a), (b) 原初粒子为质子, 能量分别为log10(E/GeV) = 5.1 (a), log10(E/GeV) = 6.9 (b); (c), (d) 原初粒子为铁核, 能量分别为log10(E/GeV) = 5.1 (c), log10(E/GeV) = 6.9 (d). 绿色、黑色、蓝色、粉色点分别表示次级粒子中伽马射线、电子、缪子和中子, 最上端的红色五角星表示切伦科夫光, 对应颜色的实线为拟合曲线
Figure 5. Fitting of lateral distribution of secondary components: (a), (b) Primary particle is proton with log10(E/GeV) = 5.1 (a) and log10(E/GeV) = 6.9 (b); (c), (d) primary particle is iron with log10(E/GeV) = 5.1 (c) and log10(E/GeV) = 6.9 (d). The green, black, blue, and pink points represent gamma, electron, muon, and neutron respectively, the red stars at the top represent Cherenkov light. The solid lines with the same color are the fitted function.
图 6 原初粒子分别为质子(a)和铁核(b)在EAS中产生的不同次级粒子个数的统计值N与拟合值
$ {N}_{\rm{s}\rm{i}\rm{z}\rm{e}} $ 之间的偏差,Figure 6. Deviation between counted value N and fitted value
$ {N}_{\rm{s}\rm{i}\rm{z}\rm{e}} $ for different secondary particles. Shower is induced by proton (a) and iron (b).
图 9 原初粒子为不同能量的铁核产生的次级电子的粒子数密度的展宽百分比随离簇射轴垂直距离的变化
Figure 9. Resolution in percentage (sigma/mean) of the particle number density of secondary electrons varies with perpendicular distance to the shower axis. The secondary electrons are induced by iron with different energies.
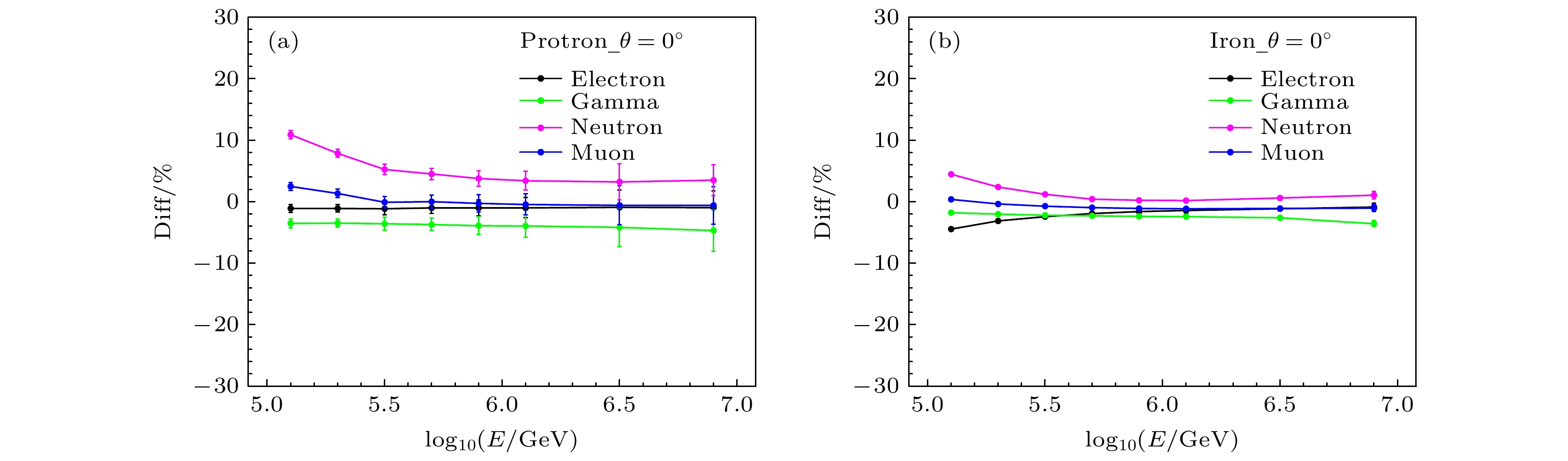
图 11 原初成分为铁核, 分别用横向分布拟合的
${N}_{\rm{s}\rm{i}\rm{z}\rm{e}}$ ,$ {N}_{\rm{s}\rm{i}\rm{z}\rm{e}2} $ 和$ \rho $ 进行能量重建的精度. 次级粒子分别为电子(a)、伽马(b)、缪子(c)和中子(d);$ {N}_{\rm{s}\rm{i}\rm{z}\rm{e}2} $ 表示修正之后的$ {N}_{\rm{s}\rm{i}\rm{z}\rm{e}} $ Figure 11. Energy resolution reconstructed by
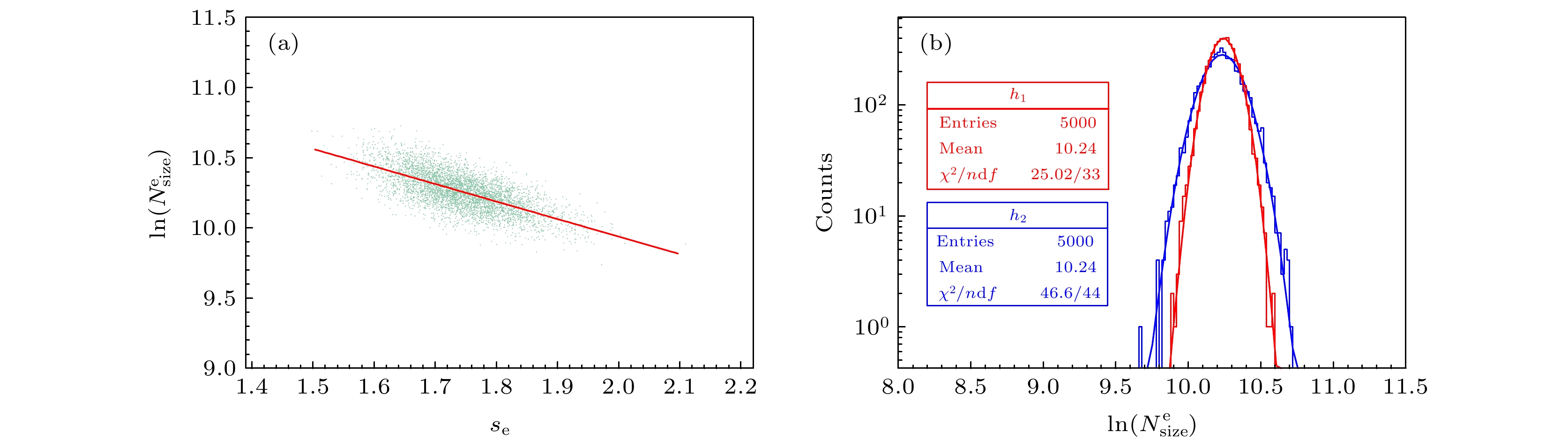
$ {N}_{\rm{s}\rm{i}\rm{z}\rm{e}} $ ,$ {N}_{\rm{s}\rm{i}\rm{z}\rm{e}2} $ , and$ \rho , $ respectively. Shower is induced by iron. The secondary particles are electron (a), gamma (b), muon (c) and neutron (d), respectively;$ {N}_{\rm{s}\rm{i}\rm{z}\rm{e}2} $ indicates the amended$ {N}_{\rm{s}\rm{i}\rm{z}\rm{e}} $ .
图 10 (a) 原初粒子铁核在能量log10(E/GeV) = 5.1时, 横向分布拟合的
${\rm{ln}}({N}_{\rm{s}\rm{i}\rm{z}\rm{e}}^{\rm{e}})$ 和$ {s}_{\rm{e}} $ 的关系 (红色实线为拟合线); (b) 用图(a)红色实线对$ {\rm{ln}}({N}_{\rm{s}\rm{i}\rm{z}\rm{e}}^{\rm{e}}) $ 进行修正, 修正前后的$ {\rm{ln}}({N}_{\rm{s}\rm{i}\rm{z}\rm{e}}^{\rm{e}}) $ 分布对比(图(b)插图中χ2/ndf可表征拟合好坏, χ2表征模型与数据点的差异, ndf指拟合的自由度, 即数据点个数减去模型的自由参数数量)Figure 10. Distribution of
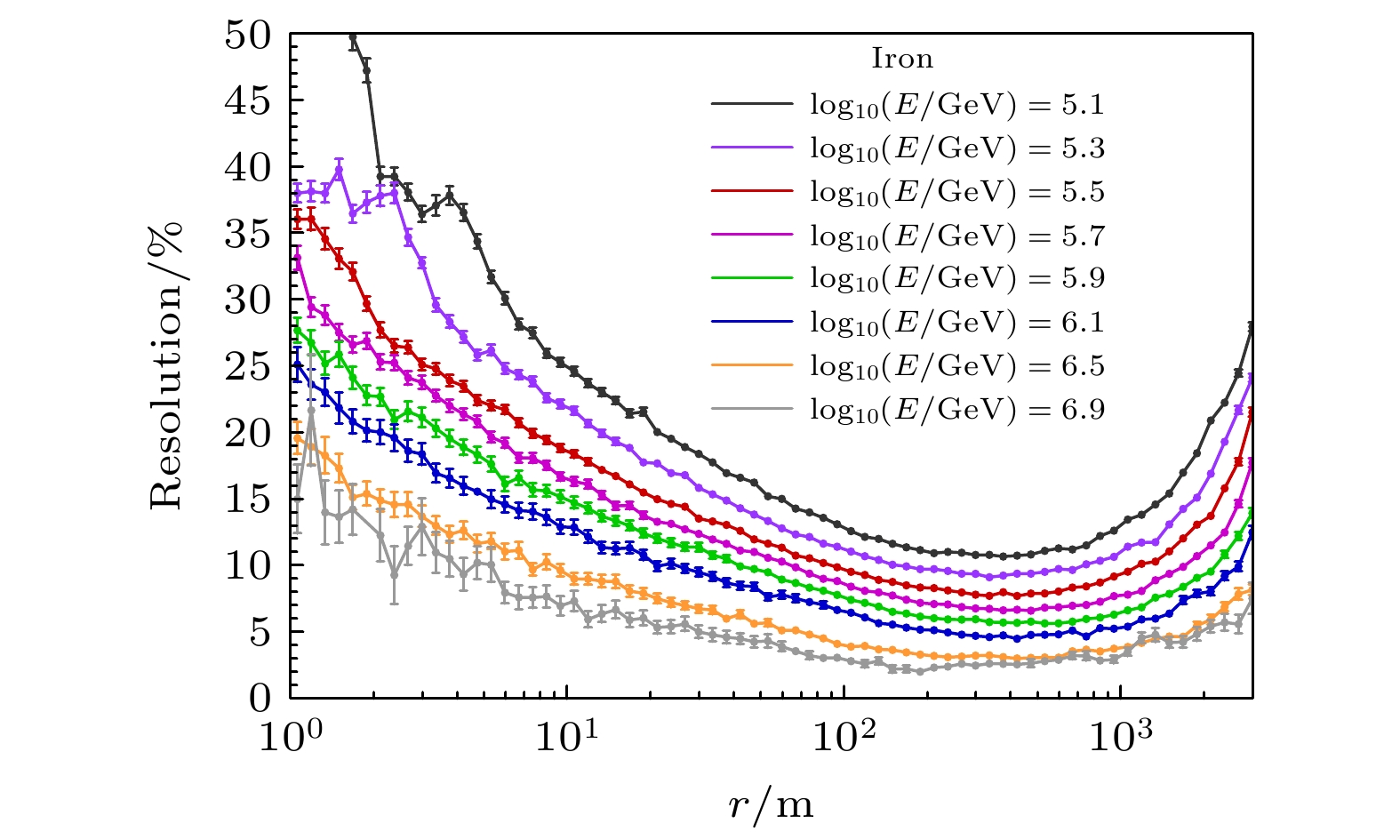
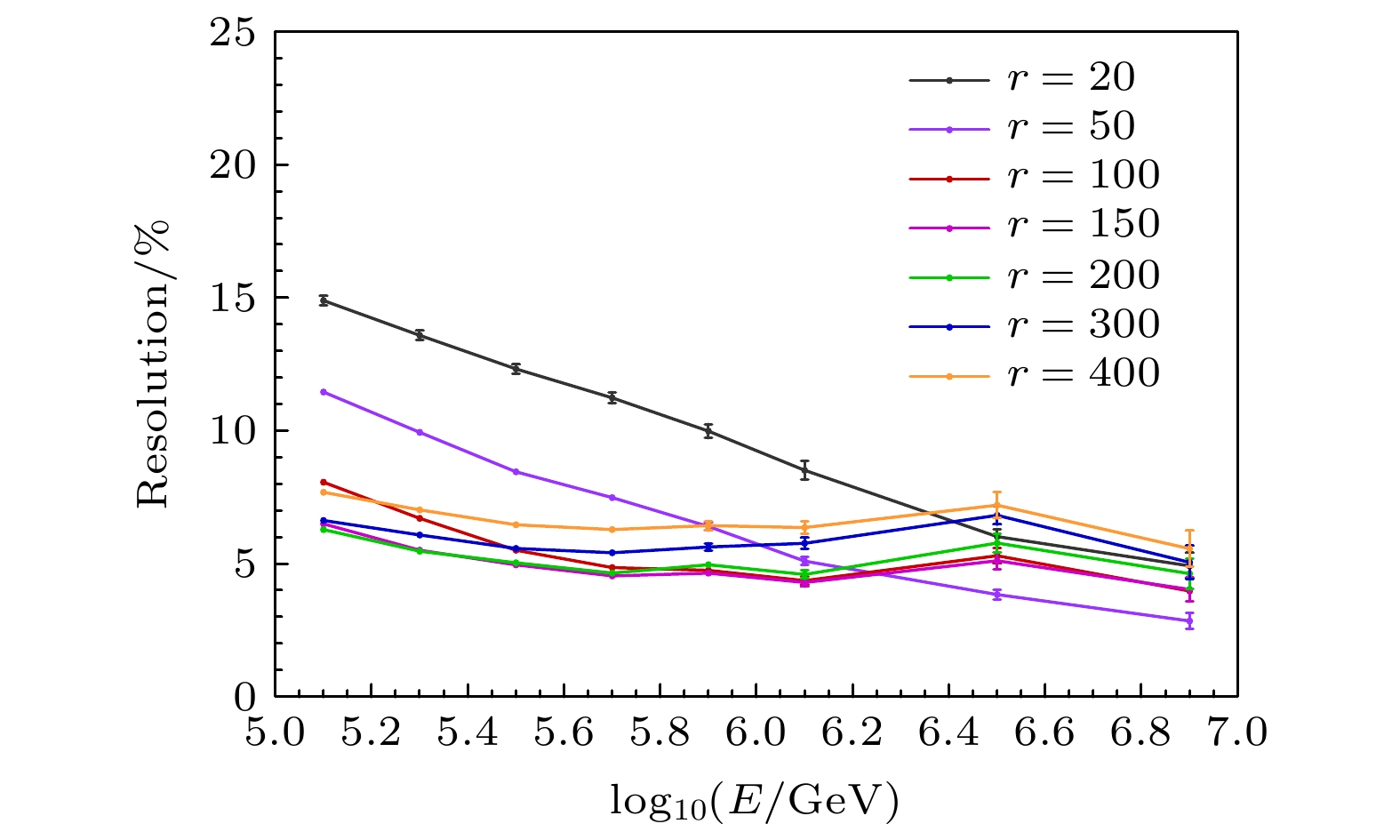
$ {\rm{ln}}({N}_{\rm{s}\rm{i}\rm{z}\rm{e}}^{\rm{e}}) $ vs. se from fitted lateral distribution function for iron with energy log10(E/GeV) = 5.1 (Red solid line is a linear fitting); (b) comparison between corrected and uncorrected$ {\rm{ln}}({N}_{\rm{s}\rm{i}\rm{z}\rm{e}}^{\rm{e}}) $ , and$ {\rm{ln}}({N}_{\rm{s}\rm{i}\rm{z}\rm{e}}^{\rm{e}}) $ corrected with the red solid line in panel (a) (In the panel (b), χ2/ndf can represent the good or bad fit, χ2 represents the difference between the model and the data point, ndf refers to the degree of freedom of fitting, that is the number of data points minus the number of free parameters of the model).
图 12 原初粒子为铁核时, 距离芯位不同垂直距离
$ r $ 处的切伦科夫光子数$ {N}^{\rm{C}} $ 分布的展宽百分比随原初粒子能量的变化关系Figure 12. Resolution in percentage (sigma/mean) of Cherenkov photon number NC varies with the energy of the primary particle at different vertical distance r from the core site. Shower is induced by iron.
图 13 不同次级成分的能量重建精度随原初粒子能量的变化关系, 原初粒子分别为质子(a), 氦核(b), 碳氮氧(c), 镁铝硅(d), 铁核(e) (彩色线表示不同的次级粒子)
Figure 13. Energy resolution from different secondary components vs. primary particle energy. The primary particles are proton (a), helium (b), CNO (c), MgAlSi (d), iron (e) (Colored lines indicate different secondary type).
图 14 对于不同的能量的原初粒子质子和铁核, ρ和s的关系 (a)
${\rho }_{\rm{e}} \text{ vs. } {s}_{\rm{e}}$ ; (b)${\rho }_{\rm{\gamma }} \text{ vs. } {s}_{\rm{\gamma }}$ ; (c)${\rho }_{\text{μ}} \text{ vs. } {s}_{\text{μ}}$ ; (d)${\rho }_{\rm{n}} \text{ vs. } {r}_{0}^{\rm{n}} $ Figure 14. Distribution of ρ vs. s when shower is induced by proton and iron respectively with different energies: (a)
${\rho }_{\rm{e}} \text{ vs. } {s}_{\rm{e}}$ ; (b)${\rho }_{\rm{\gamma }} \text{ vs. } {s}_{\rm{\gamma }}$ ; (c)${\rho }_{\text{μ}} \text{ vs. } {s}_{{\text{μ}}}$ ; (d)${\rho }_{\rm{n}} \text{ vs. } {r}_{0}^{\rm{n}} $ .
图 15 原初粒子为质子和铁核的参数 (a)
$ {s}_{\rm{e}} $ , (b)$ {s}_{\rm{\gamma }} $ , (c)$ {\rho }_{{\text{μ}}} $ , (d)$ {\rho }_{\rm{n}} $ 分布的对比(原初粒子能量均为log10(E/GeV) = 5.1)Figure 15. Comparison of the distribution of (a)
$ {s}_{\rm{e}} $ , (b)$ {s}_{\rm{\gamma }} $ , (c)$ {\rho }_{{\text{μ}}} $ , (d)$ {\rho }_{\rm{n}} $ between proton and iron with log10(E/GeV) = 5.1.
图 16 原初粒子为质子和铁核的参数 (a)
$ {s}_{\rm{e}} $ , (b)$ {s}_{\rm{\gamma }} $ , (c)${\rho }_{\text{μ}}$ , (d)$ {\rho }_{\rm{n}} $ 分布的对比(原初粒子能量均为log10(E/GeV) = 6.9Figure 16. Comparison of the distribution of (a)
$ {s}_{\rm{e}} $ , (b)$ {s}_{\rm{\gamma }} $ , (c)${\rho }_{\text{μ}}$ , (d)$ {\rho }_{\rm{n}} $ between proton and iron with log10(E/GeV) = 6.9. -
[1] Prosin V V, Berezhnev S F, Budnev N M, et al. 2014 Nucl. Instrum. Methods Phys. Res. Sect. A 756 94
Google Scholar
[2] Blümer J, Engel R, Hörandel J R 2009 Prog. Part. Nucl. Phys. 63 293
Google Scholar
[3] Rújula De A 2006 Nucl. Phys. B 151 23
Google Scholar
[4] Ahn H S, Allison P, Bagliesi M G 2009 Astrophys. J. 707 593
Google Scholar
[5] Barao F 2004 Nucl. Instruments Methods Phys. Res. Sect. A 535 134
Google Scholar
[6] Alemanno F, An Q 2021 Phys. Rev. Lett. 126 201102
Google Scholar
[7] An Q, Asfandiyarov R, Azzarello P 2019 Sci. Adv. 5 3793
Google Scholar
[8] Chang J, Ambrosi G, An Q 2017 Astropart. Phys. 95 6
Google Scholar
[9] Sparvoli R 2013 Nucl. Phys. B 239 115
Google Scholar
[10] Antoni T, Apel W D, Badea A F 2005 Astropart. Phys. 24 1
Google Scholar
[11] Bartoli B, Bernardini P, Bi X J, Cao Z 2017 Astropart. Phys. 93 46
Google Scholar
[12] Ma X H, Bi Y J, Cao Z 2022 Chin. Phys. C 46 030001
Google Scholar
[13] Abbasi R, Abdou Y, Ackermann M 2013 Nucl. Instrum. Methods. Phys. Res. Sect. A 700 188
Google Scholar
[14] Abbasi R U, Abe M, Abu-Zayyad T 2018 Astro. J. 865 74
Google Scholar
[15] Amenomori M, Bao Y W 2021 Phys. Rev. Lett. 127 031102
Google Scholar
[16] Apel W D, Arteaga-Velázquez J C 2011 Phys. Rev. Lett. 107 171104
Google Scholar
[17] Apel W D, Arteaga-Velázquez J C 2013 Phys. Rev. D 87 081101
Google Scholar
[18] Bartoli B, Bernardini P, Bi X J, Cao Z 2015 Phys. Rev. D 92 092005
Google Scholar
[19] Aartsen M G, Abbasi R 2020 Phys. Rev. D 102 122001
Google Scholar
[20] Aartsen M G, Ackermann M, Adams J 2019 Phys. Rev. D 100 082002
Google Scholar
[21] Heck D, Knapp J, Capdevielle J N, Schatz G, Thouw T 1998 CORSIKA: A Monte Carlo Code to Simulate Extensive Air Showers
[22] Capdevielle J N, Cohen F 2005 J. Phys. G 31 507
Google Scholar
[23] Apel W D, Badea A F, Bekk K 2006 Astropart. Phys. 24 467
Google Scholar
[24] Feng Y L, Zhang Y, Chen T L 2019 Chin. Phys. C 43 075002
Google Scholar
[25] Alexandru C E, Alexandru J, Lavinia-Elena G 2019 Chin. Phys. C 43 083001
Google Scholar
[26] 李骢 2018 博士学位论文 (北京: 中国科学院大学)
Li C 2018 Ph. D. Dissertation (Beijing: University of Chinese Academy of Sciences) (in Chinese)
[27] Rivera-Rangel D, Arteaga-Velázquez J C 2021 37th Inter-national Cosmic Ray Conference (ICRC 2021) Online–Berlin, Germany July 1223, 2021 p3721
[28] Aharonian F, An Q, Axikegu 2021 Chin. Phys. C 45 025002
Google Scholar
[29] Conceição R, Peres L 2021 Eur. Phys. J. C 81 1
Google Scholar
[30] Yin L Q, Zhang S S, Cao Z, Bi B Y 2019 Chin. Phys. C 43 075001
Google Scholar
[31] Jin C, Chen S Z, He H H 2020 Chin. Phys. C 44 065002
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4091
- PDF Downloads: 67
- Cited By: 0
