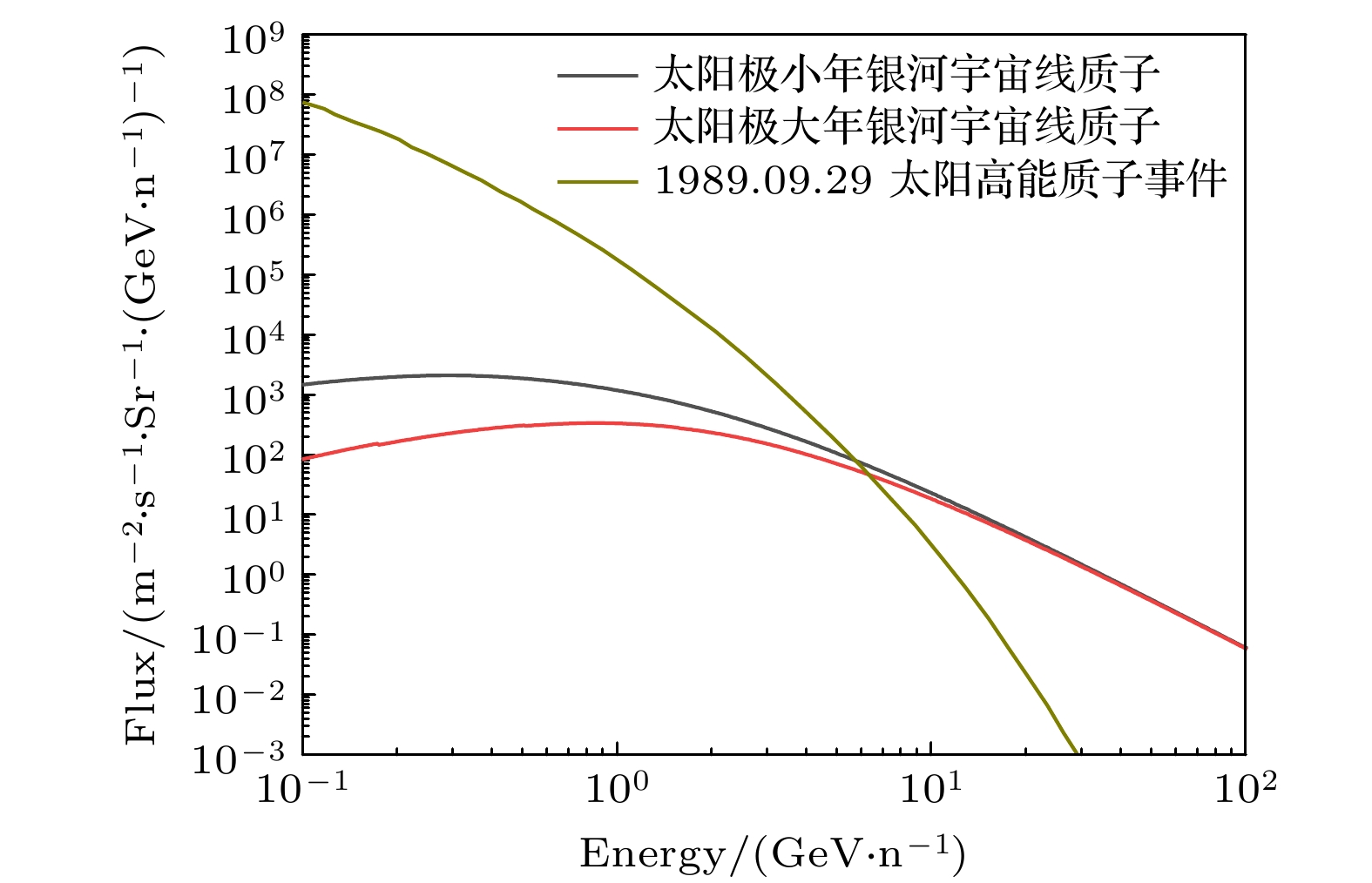
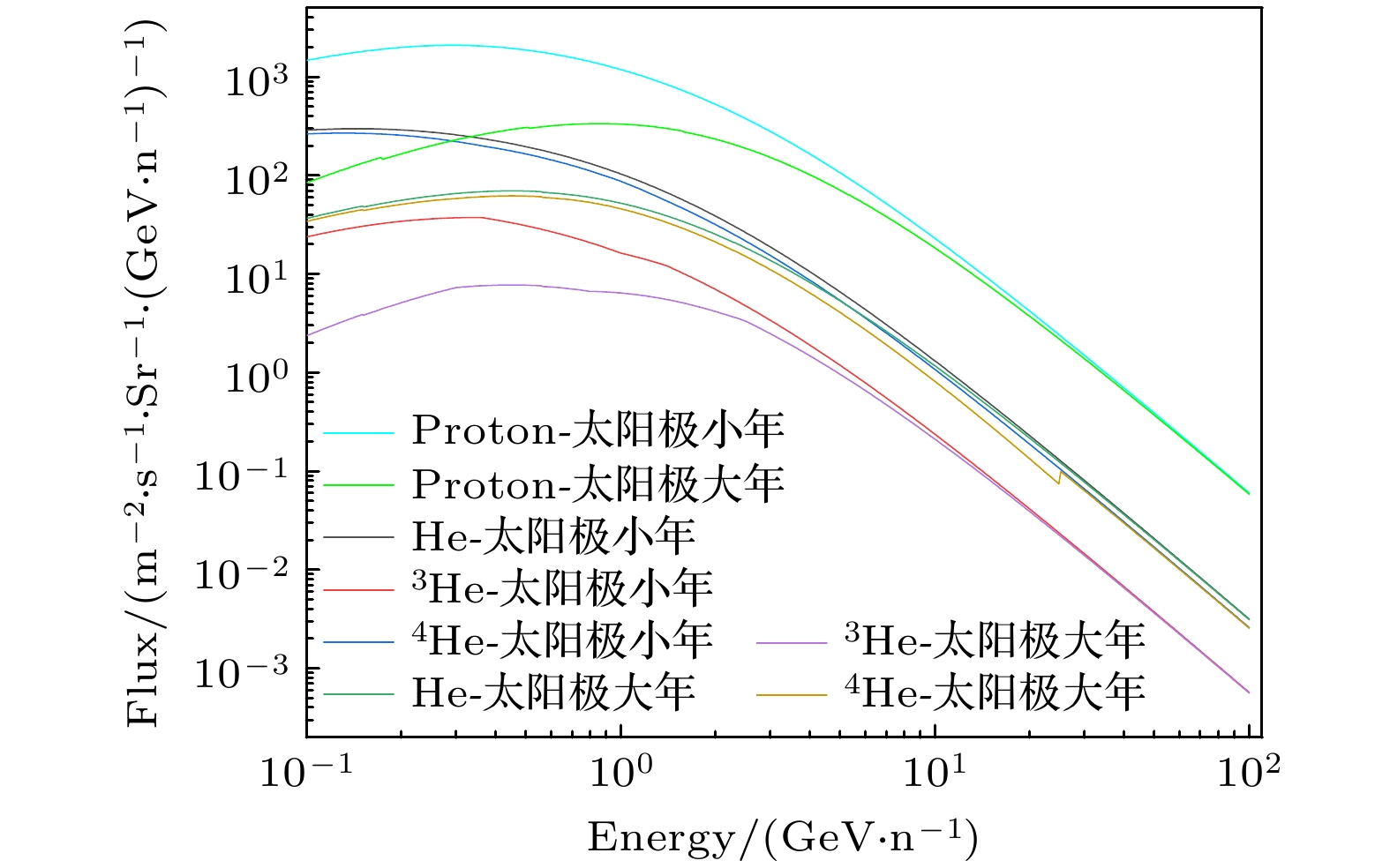
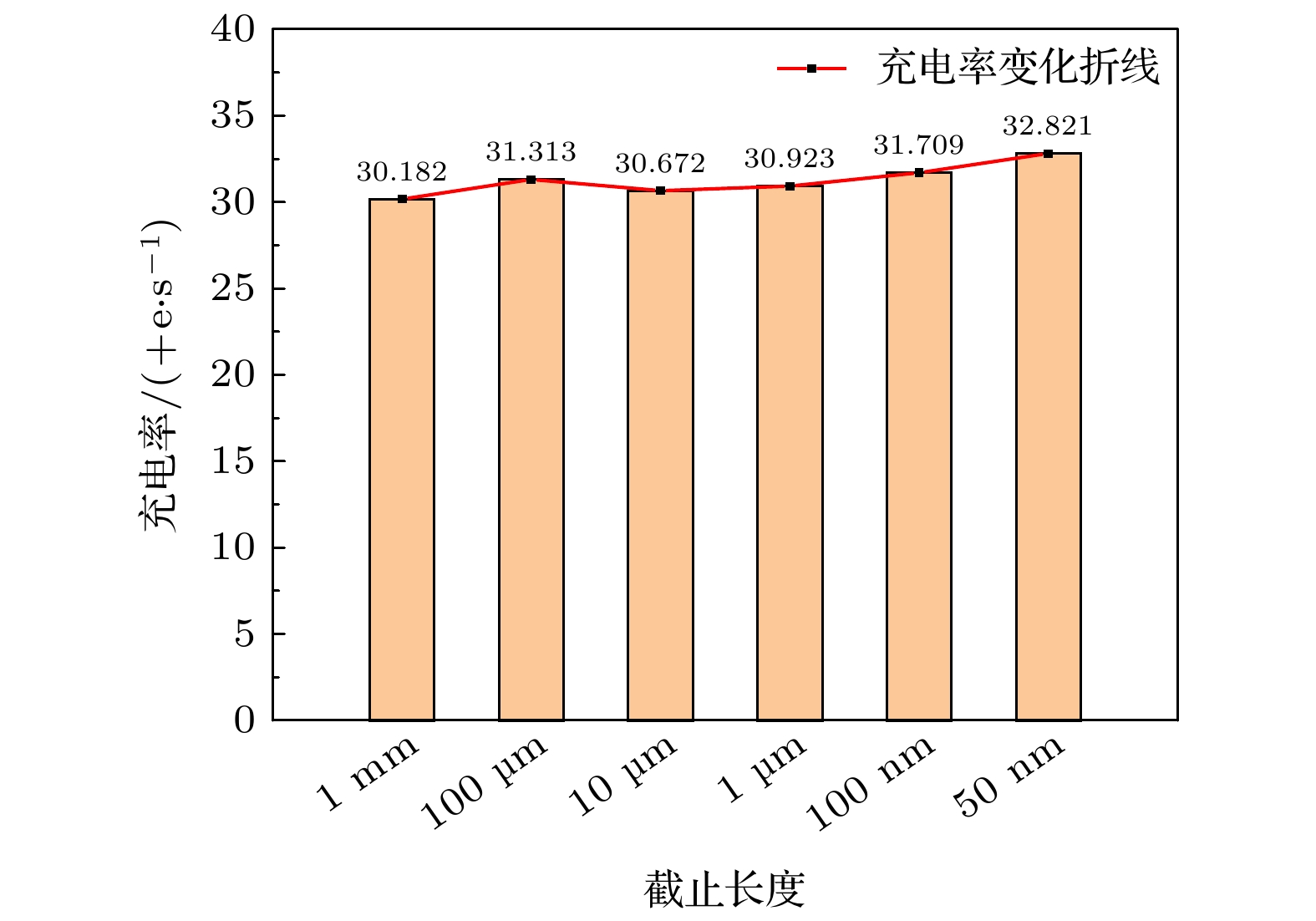
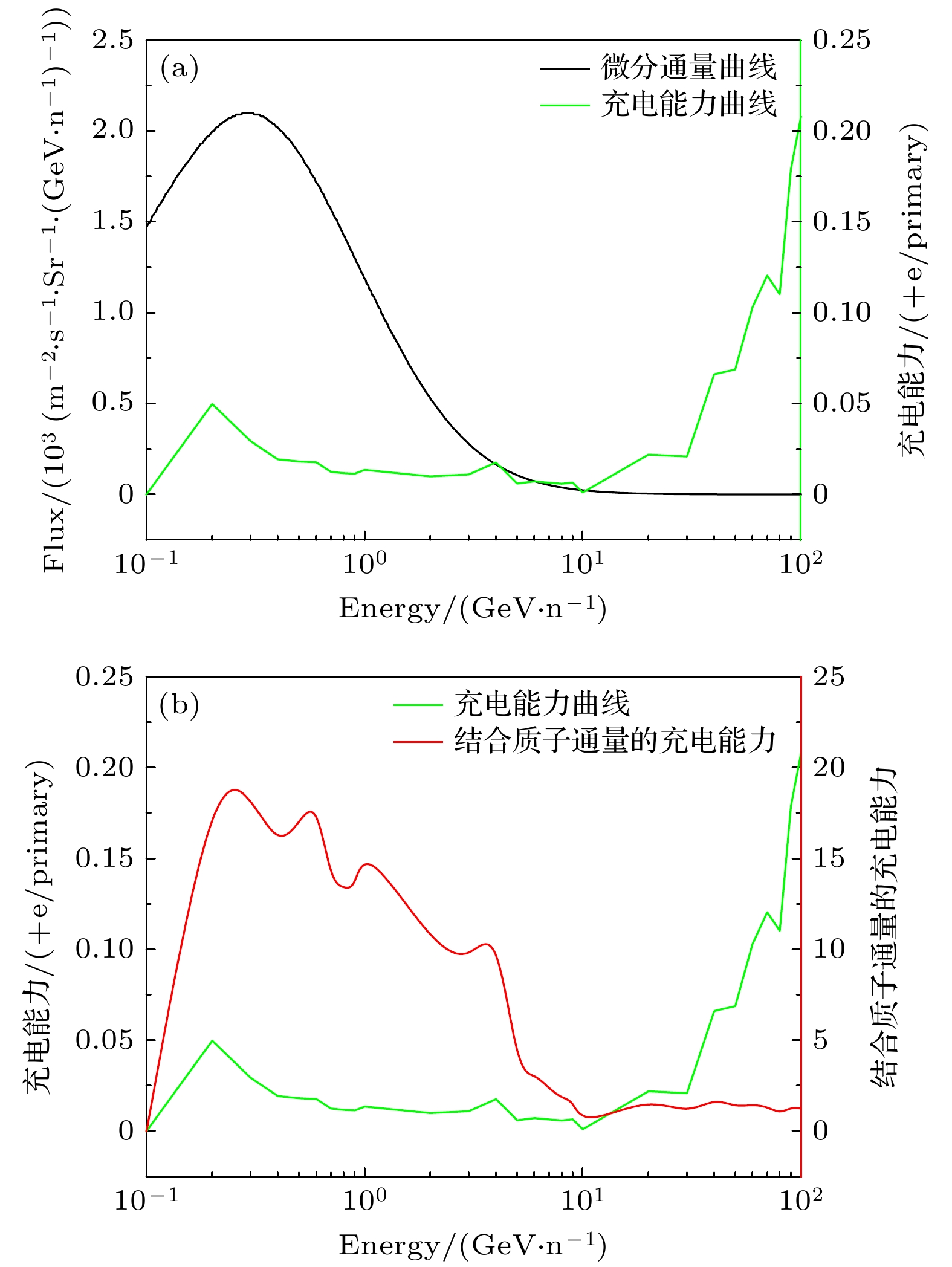
-
测试质量是空间引力波测量的核心传感器, 宇宙线高能粒子能够穿透航天器屏蔽对其造成电荷注入, 进而产生库仑力和洛伦兹力噪声对引力波科学探测造成严重影响. 本文采用蒙特卡洛仿真方法, 探究了不同宇宙线高能粒子对测试质量的充电过程和机制. 研究结果表明, 在同一能谱下随着截止能量的降低充电速率逐步增大, 充电速率变化约为9%; 太阳活动极小年时测试质量的充电速率为39.5 +e/s, 其中贡献最大的质子占比约为83.16%, 太阳活动极大年时测试质量的充电速率约为12.5 +e/s, 1989年最恶劣的太阳高能粒子事件造成测试质量的充电速率约为120700 +e/s; 在太阳活动极小年时, 银河宇宙线各成分的充电速率取决于各成分的初级粒子在测试质量中的沉积, 其中初级粒子贡献占测试质量总充电速率的73%; 太阳活动极小年时, 质子的充电贡献主要来自能量为0.1—1 GeV的区间, 占比约为65%. 研究结果可用于评估测试质量在轨充电规律, 为电荷管理的设计和在轨工作提供依据.The testing mass is the core sensor for measuring the spatial gravitational waves. The high-energy cosmic ray particles penetrating the outer structure of the spacecraft result in the electrical charges on the testing mass. The Coulomb force produced by the charges on the surrounding conducting surface and the Lorentz force generated by the motion through the interplanetary magnetic field will exert a serious influence on the geodesic motion of the testing mass. In this paper are investigated the process and mechanism of charging the testing mass by high-energy particles from different cosmic rays through using the Monte Carlo simulation method. It is concluded that the charging rate gradually increases with the decrease of cut-off energy under the same energy spectrum. The positive charging rate (elementary charges per second) in the years of minimum solar activity is predicted to be 39.5 +e/s, and the protons account for approximately 83.16% of the total quantity of galactic cosmic rays. The positive charging rate of the testing mass during the years of maximum solar activity is about 12.5 +e/s, and the charging rate of the testing mass of the worst solar energetic particle event in 1989 is about 120700 +e/s. The charging rate of the components of the galactic cosmic ray depends on the deposition of primary particles of each component in the testing mass during the years of minimum solar activity, with primary particles accounting for 73% of the total charging rate. The charging contribution of protons in years of minimum solar activity is mainly in an energy range of 0.1–1 GeV, accounting for about 65%. The research results can be used to assess the charging patterns of test quality on-orbit charges and provide a basis for designing the charge management and on-orbit work.
-
Keywords:
- gravitational waves /
- test mass /
- cosmic rays /
- GEANT4
[1] Sathyaprakash B S, Schutz B F 2009 Living Rev. Relativ. 12 1
Google Scholar
[2] 雷中华, 兰明建, 汪先友, 李建杰 2008 57 7408
Google Scholar
Lei Z H, Lan M J, Wang X Y, Li J J 2008 Acta Phys. Sin. 57 7408
Google Scholar
[3] Jafry Y, Sumner T J, Buchman S 1996 Classical Quantum Gravity 13 A97
Google Scholar
[4] Jafry Y, Sumner T J 1997 Classical Quantum Gravity 14 1567
Google Scholar
[5] Sumner T J, Jafry Y 2000 Adv. Space Res. 25 1219
Google Scholar
[6] Araújo H M, Howard A, Shaul D, Sumner T J 2003 Classical Quantum Gravity 20 S311
Google Scholar
[7] Shaul D N A, Sumner T J, Araújo H M, Rochester G K, Wass P J, Lee C G Y 2004 Classical Quantum Gravity 21 S647
Google Scholar
[8] Sumner T, Araújo H, Davidge D, Howard A, Lee C, Rochester G, Shaul D, Wass P 2004 Classical Quantum Gravity 21 S597
Google Scholar
[9] Vocca H, Grimani C, Amico P, Bosi L, Marchesoni F, Punturo M, Travasso F, Barone M, Stanga R, Vetrano F, Viceré A 2004 Classical Quantum Gravity 21 S665
Google Scholar
[10] 包纲, 倪维斗, 柳磊, Araújo H, Shaul D, Sumner T 2004 紫金山天文台台刊 23 105
Bao G, Ni W T, Liu L, Araújo H, Shaul D, Sumner T 2004 Publ. Purple Mt. Observatory 23 105
[11] Bao G, Ni W T, Shaul D N A, Araújo H M, Liu L, Sumner T J 2008 Int. J. Mod. Phys. D 17 965
Google Scholar
[12] Grimani C, Fabi M, Lobo A, Mateos I, Telloni D 2015 Classical. Quantum Gravity. 32 035001
Google Scholar
[13] Wass P J, Araújo H M, Shaul D N A, Sumner T J 2005 Classical. Quantum Gravity. 22 S311
Google Scholar
[14] Armano M, Audley H, Auger G, Baird J T, Binetruy P, Born M, Bortoluzzi D, Brandt N, Bursi A, Caleno M, Cavalleri A, Cesarini A, Cruise M, Danzmann K, Silva M, Diepholz I, Dolesi R, Dunbar N, Ferraioli L, Ferroni V, Fitzsimons E D, Flatscher R, Freschi M, Gallegos J, Marirrodriga C, Gerndt R, Gesa L, Gibert F, Giardini D, Giusteri R, Grimani C, Grzymisch J, Harrison I, Heinzel G, Hewitson M, Hollington D, Hueller M, Huesler J, Inchauspé H, Jennrich O, Jetzer P, Johlander B, Karnesis N, Kaune B, Killow C J, Korsakova N, Lloro I, Liu L, López-Zaragoza J P, Maarschalkerweerd R, Madden S, Mance D, Martín V, Martin-Polo L, Martino J, Martin-Porqueras F, Mateos I, McNamara P W, Mendes J, Mendes L, Moroni A, Nofrarias M, Paczkowski S, Perreur-Lloyd M, Petiteau A, Pivato P, Plagnol E, Prat P, Ragnit U, Ramos-Castro J, Reiche J, Perez J A, Robertson D I, Rozemeijer H, Rivas F, Russano G, Sarra P, Schleicher A, Slutsky J, Sopuerta C, Sumner T J, Texier D, Thorpe J I, Trenkel C, Vetrugno D, Vitale S, Wanner G, Ward H, Wass P J, Wealthy D, Weber W J, Wittchen A, Zanoni C, Ziegler T, Zweifel P 2017 Phys. Rev. Lett. 118 171101
Google Scholar
[15] Araújo H M, Wass P, Shaul D, Rochester G, Sumner T J 2005 Astropart. Phys. 22 451
Google Scholar
[16] 罗子人, 白姗, 边星, 陈葛瑞, 董鹏, 董玉辉, 高伟, 龚雪飞, 贺建武, 李洪银, 李向前, 李玉琼, 刘河山, 邵明学, 宋同消, 孙保三, 唐文林, 徐鹏, 徐生年, 杨然, 靳刚 2013 力学进展 43 415
Google Scholar
Luo Z R, Bai S, Bian X, Chen G R, Dong P, Dong Y H, Gao W, Gong X F, He J W, Li H Y, Li X Q, Li Y Q, Liu S H, Shao M X, Song T X, Sun B S, Tang W L, Xu P, Xu S N, Yang R, Jin G 2013 Adv. Mech. 43 415
Google Scholar
[17] Myers Z D, Seo E S, Abe K, Anraku K, Imori M, Maeno T, Makida Y, Matsumoto H, Mitchell J, Moiseev A, Nishimura J, Nozaki M, Ormes J F, Orito S, Sanuki T, Sasaki M, Shikaze Y, Streitmatter R E, Suzuki J, Tanaka K, Yamagami T, Yamamoto A, Yoshida T, Yoshimura K 2003 In International Cosmic Ray Conference Tsukuba, Japan, July 31–August 7, 2003 p1805
[18] Wang J Z, Seo E S, Anraku K, Fujikawa M, Imori M, Maeno T, Matsui N, Matsunaga H, Motoki M, Orito S, Saeki T, Sanuki T, Ueda I, Yoshimura K, Makida Y, Suzuki J, Tanaka K, Yamamoto A, Yoshida T, Mitsui T, Matsumoto H, Nozaki M, Sasaki M, Mitchell J, Moiseev A, Ormes J, Streitmatter R, Nishimura J, Yajima Y, Yamagami T 2002 Astrophys. J. 564 244
Google Scholar
[19] Casolino M, Santis C D, Simone N D, Formato V, Nikonov N, Picozza P 2011 Astrophys. Space Sci. Trans. 7 465
Google Scholar
[20] Abe K, Fuke H, Haino S, Hams T, Hasegawa M, Horikoshi A, Itazaki A, Kim K C, Kumazawa T, Kusumoto A, Lee M H, Makida Y, Matsuda S, Matsukawa Y, Matsumoto K, Mitchell J W, Moiseev A A, Nishimura J, Nozaki M, Orito R, Ormes J F, Picot-Clémente N, Sakai K, Sasaki M, Seo E S, Shikaze Y, Shinoda R, Streitmatter R E, Suzuki J, Takasugi Y, Takeuchi K, Tanaka K, Thakur N, Yamagami T, Yamamoto A, Yoshida T, Yoshimura K 2014 Adv. Space Res. 53 1426
Google Scholar
[21] Grimani C, Vocca H, Bagni G, Marconi L, Stanga R, Vetrano F, Viceré A, Amico P, Gammaitoni L, Marchesoni F 2005 Classical. Quantum Gravity. 22 S327
Google Scholar
[22] Papini P, Grimani C, Stephens S A 1996 Il Nuovo Cim. C 19 367
Google Scholar
[23] Grimani C, Vocca H, Barone M, Stanga R, Vetrano F, Viceré A, Amico P, Bosi L, Marchesoni F, Punturo M, Travasso F 2004 Classical. Quantum Gravity. 21 S629
Google Scholar
-
图 1 LISA航天器模型 (a)整体模型; (b)惯性传感器模型
Fig. 1. LISA spacecraft model: (a) Overall model; (b) inertial sensor model.
图 2 本文航天器模型图 (a) geant4模型图; (b)模型平面示意图
Fig. 2. The spacecraft model diagram in this paper: (a) Geant4 model diagram; (b) schematic diagram of the model plane.
图 3 1 AU处GCR各粒子微分能谱
Fig. 3. Differential energy spectra of each element of GCR at 1 AU.
图 5 宇宙线Proton和He(3He和4He)微分能谱
Fig. 5. Differential energy spectra of cosmic rays Proton and He (3He and 4He).
图 7 宇宙线各粒子的充电率 (a) 太阳活动极小年; (b) 太阳活动极大年
Fig. 7. The charging rate of each particle of cosmic rays: (a) Solar minimum year; (b) solar maximum year.
图 9 太阳活动极小充电能力 (a) 不同能量充电能力和质子通量; (b) 充电能力和结合质子通量的总充电比例
Fig. 9. Solar minimum charging capacity: (a) Different energy charging capacity and proton flux; (b) charging capacity and total charge ratio of combined proton flux.
表 1 航天器几何尺寸和材料构成
Table 1. Spacecraft geometric dimensions and material composition.
名称 组成成分 密度/(g·cm–3) 尺寸 厚度/mm 测试质量 Au(70%); Pt(30%) 19.837 46 mm立方体 — 钼电极 Mo 10.28 74—86 mm立方体壳层 6 钛室 Ti 4.54 75—80 mm球壳层 5 碳外壳 C 2.10 80—100 mm球壳层 20 
下载: 导出CSV
表 2 太阳极小年3He/4He的参数化比例C(m)
Table 2. The parameterized ratio C(m) of 3He/4He in solar minimum.
E/(GeV·n–1) 0.10 ≤ E ≤ 0.36 0.36 ≤ E ≤ 1.00 1.00 ≤ E ≤ 1.40 E > 1.40 C(m) 0.335 × E0.569 0.187 0.187 × E0.491 0.22
下载: 导出CSV
表 3 太阳极大年3He/4He的参数化比例C(M)
Table 3. The parameterized ratio C(M) of 3He/4He during solar maximum.
E/(GeV·n–1) 0.10 ≤ E ≤ 0.30 0.30 ≤ E ≤ 0.80 0.80 ≤ E ≤ 2.50 E > 2.50 C(M) 0.239 × E0.538 0.125 0.140 × E0.496 0.22
下载: 导出CSV
表 4 太阳极小年宇宙线主要粒子仿真参数
Table 4. The main particle simulation parameters of cosmic rays during solar minimum.
粒子种类 粒子数目/个 暴露时间/s 积分通量/(cm–2·s–1) Proton 700000 353.65 4.375 3He 100000 3563.35 0.062 4He 120000 806.26 0.329 C 100000 21052.63 0.0105 N 100000 77561.47 0.00285 O 100000 22128.79 0.00999 Ne 10000 13644.98 0.00162 Mg 10000 10476.25 0.00211 Si 10000 14542.70 0.00152 Fe 10000 19562.20 0.00113
下载: 导出CSV
表 5 太阳极大年宇宙线主要粒子仿真参数
Table 5. The main particle simulation parameters of cosmic rays during solar maximum.
粒子种类 粒子数目/个 暴露时间/s 积分通量/(cm–2·s–1) Proton 700000 353.65 4.375 3He 100000 3563.35 0.062 4He 120000 806.26 0.329
下载: 导出CSV
表 6 1989年9月29日太阳高能粒子事件仿真参数
Table 6. Simulation parameters of the SEP event on September 29, 1989.
粒子种类 粒子数目/个 暴露时间/s 积分通量/(cm–2·s–1) Proton 500000 0.150 7385.53
下载: 导出CSV
表 7 宇宙线初、次级粒子造成的充电率
Table 7. Charge rate caused by primary and secondary particles of cosmic rays.
粒子
种类初级粒子充电
率/(+e·s–1)次级粒子充电
率/(+e·s–1)初级粒子
充电率占比Proton 22.016 10.805 67.07% 3He 1.196 –0.029 100% 4He 5.125 –0.100 100% C 0.182 0.023 88.78% N 0.042 0.009 82.35% O 0.123 0.020 86.01% Ne 0.0132 0.0026 83.54% Mg 0.0137 0.0064 68.16% Si 0.0087 0.0058 60% Fe 0 0.0084 0%
下载: 导出CSV
-
[1] Sathyaprakash B S, Schutz B F 2009 Living Rev. Relativ. 12 1
Google Scholar
[2] 雷中华, 兰明建, 汪先友, 李建杰 2008 57 7408
Google Scholar
Lei Z H, Lan M J, Wang X Y, Li J J 2008 Acta Phys. Sin. 57 7408
Google Scholar
[3] Jafry Y, Sumner T J, Buchman S 1996 Classical Quantum Gravity 13 A97
Google Scholar
[4] Jafry Y, Sumner T J 1997 Classical Quantum Gravity 14 1567
Google Scholar
[5] Sumner T J, Jafry Y 2000 Adv. Space Res. 25 1219
Google Scholar
[6] Araújo H M, Howard A, Shaul D, Sumner T J 2003 Classical Quantum Gravity 20 S311
Google Scholar
[7] Shaul D N A, Sumner T J, Araújo H M, Rochester G K, Wass P J, Lee C G Y 2004 Classical Quantum Gravity 21 S647
Google Scholar
[8] Sumner T, Araújo H, Davidge D, Howard A, Lee C, Rochester G, Shaul D, Wass P 2004 Classical Quantum Gravity 21 S597
Google Scholar
[9] Vocca H, Grimani C, Amico P, Bosi L, Marchesoni F, Punturo M, Travasso F, Barone M, Stanga R, Vetrano F, Viceré A 2004 Classical Quantum Gravity 21 S665
Google Scholar
[10] 包纲, 倪维斗, 柳磊, Araújo H, Shaul D, Sumner T 2004 紫金山天文台台刊 23 105
Bao G, Ni W T, Liu L, Araújo H, Shaul D, Sumner T 2004 Publ. Purple Mt. Observatory 23 105
[11] Bao G, Ni W T, Shaul D N A, Araújo H M, Liu L, Sumner T J 2008 Int. J. Mod. Phys. D 17 965
Google Scholar
[12] Grimani C, Fabi M, Lobo A, Mateos I, Telloni D 2015 Classical. Quantum Gravity. 32 035001
Google Scholar
[13] Wass P J, Araújo H M, Shaul D N A, Sumner T J 2005 Classical. Quantum Gravity. 22 S311
Google Scholar
[14] Armano M, Audley H, Auger G, Baird J T, Binetruy P, Born M, Bortoluzzi D, Brandt N, Bursi A, Caleno M, Cavalleri A, Cesarini A, Cruise M, Danzmann K, Silva M, Diepholz I, Dolesi R, Dunbar N, Ferraioli L, Ferroni V, Fitzsimons E D, Flatscher R, Freschi M, Gallegos J, Marirrodriga C, Gerndt R, Gesa L, Gibert F, Giardini D, Giusteri R, Grimani C, Grzymisch J, Harrison I, Heinzel G, Hewitson M, Hollington D, Hueller M, Huesler J, Inchauspé H, Jennrich O, Jetzer P, Johlander B, Karnesis N, Kaune B, Killow C J, Korsakova N, Lloro I, Liu L, López-Zaragoza J P, Maarschalkerweerd R, Madden S, Mance D, Martín V, Martin-Polo L, Martino J, Martin-Porqueras F, Mateos I, McNamara P W, Mendes J, Mendes L, Moroni A, Nofrarias M, Paczkowski S, Perreur-Lloyd M, Petiteau A, Pivato P, Plagnol E, Prat P, Ragnit U, Ramos-Castro J, Reiche J, Perez J A, Robertson D I, Rozemeijer H, Rivas F, Russano G, Sarra P, Schleicher A, Slutsky J, Sopuerta C, Sumner T J, Texier D, Thorpe J I, Trenkel C, Vetrugno D, Vitale S, Wanner G, Ward H, Wass P J, Wealthy D, Weber W J, Wittchen A, Zanoni C, Ziegler T, Zweifel P 2017 Phys. Rev. Lett. 118 171101
Google Scholar
[15] Araújo H M, Wass P, Shaul D, Rochester G, Sumner T J 2005 Astropart. Phys. 22 451
Google Scholar
[16] 罗子人, 白姗, 边星, 陈葛瑞, 董鹏, 董玉辉, 高伟, 龚雪飞, 贺建武, 李洪银, 李向前, 李玉琼, 刘河山, 邵明学, 宋同消, 孙保三, 唐文林, 徐鹏, 徐生年, 杨然, 靳刚 2013 力学进展 43 415
Google Scholar
Luo Z R, Bai S, Bian X, Chen G R, Dong P, Dong Y H, Gao W, Gong X F, He J W, Li H Y, Li X Q, Li Y Q, Liu S H, Shao M X, Song T X, Sun B S, Tang W L, Xu P, Xu S N, Yang R, Jin G 2013 Adv. Mech. 43 415
Google Scholar
[17] Myers Z D, Seo E S, Abe K, Anraku K, Imori M, Maeno T, Makida Y, Matsumoto H, Mitchell J, Moiseev A, Nishimura J, Nozaki M, Ormes J F, Orito S, Sanuki T, Sasaki M, Shikaze Y, Streitmatter R E, Suzuki J, Tanaka K, Yamagami T, Yamamoto A, Yoshida T, Yoshimura K 2003 In International Cosmic Ray Conference Tsukuba, Japan, July 31–August 7, 2003 p1805
[18] Wang J Z, Seo E S, Anraku K, Fujikawa M, Imori M, Maeno T, Matsui N, Matsunaga H, Motoki M, Orito S, Saeki T, Sanuki T, Ueda I, Yoshimura K, Makida Y, Suzuki J, Tanaka K, Yamamoto A, Yoshida T, Mitsui T, Matsumoto H, Nozaki M, Sasaki M, Mitchell J, Moiseev A, Ormes J, Streitmatter R, Nishimura J, Yajima Y, Yamagami T 2002 Astrophys. J. 564 244
Google Scholar
[19] Casolino M, Santis C D, Simone N D, Formato V, Nikonov N, Picozza P 2011 Astrophys. Space Sci. Trans. 7 465
Google Scholar
[20] Abe K, Fuke H, Haino S, Hams T, Hasegawa M, Horikoshi A, Itazaki A, Kim K C, Kumazawa T, Kusumoto A, Lee M H, Makida Y, Matsuda S, Matsukawa Y, Matsumoto K, Mitchell J W, Moiseev A A, Nishimura J, Nozaki M, Orito R, Ormes J F, Picot-Clémente N, Sakai K, Sasaki M, Seo E S, Shikaze Y, Shinoda R, Streitmatter R E, Suzuki J, Takasugi Y, Takeuchi K, Tanaka K, Thakur N, Yamagami T, Yamamoto A, Yoshida T, Yoshimura K 2014 Adv. Space Res. 53 1426
Google Scholar
[21] Grimani C, Vocca H, Bagni G, Marconi L, Stanga R, Vetrano F, Viceré A, Amico P, Gammaitoni L, Marchesoni F 2005 Classical. Quantum Gravity. 22 S327
Google Scholar
[22] Papini P, Grimani C, Stephens S A 1996 Il Nuovo Cim. C 19 367
Google Scholar
[23] Grimani C, Vocca H, Barone M, Stanga R, Vetrano F, Viceré A, Amico P, Bosi L, Marchesoni F, Punturo M, Travasso F 2004 Classical. Quantum Gravity. 21 S629
Google Scholar
下载:
计量
- 文章访问数: 5690
- PDF下载量: 110
- 被引次数: 0
