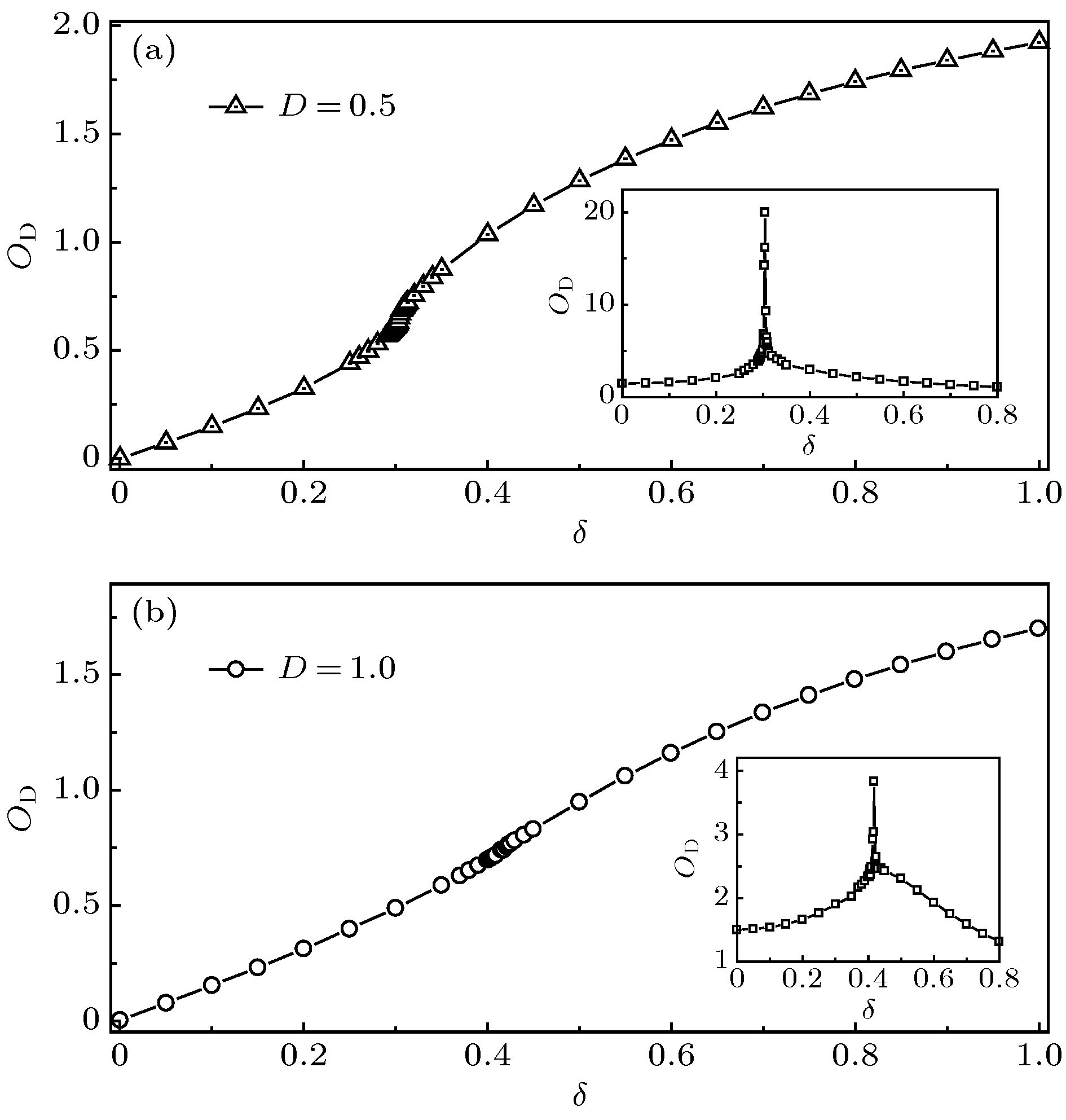
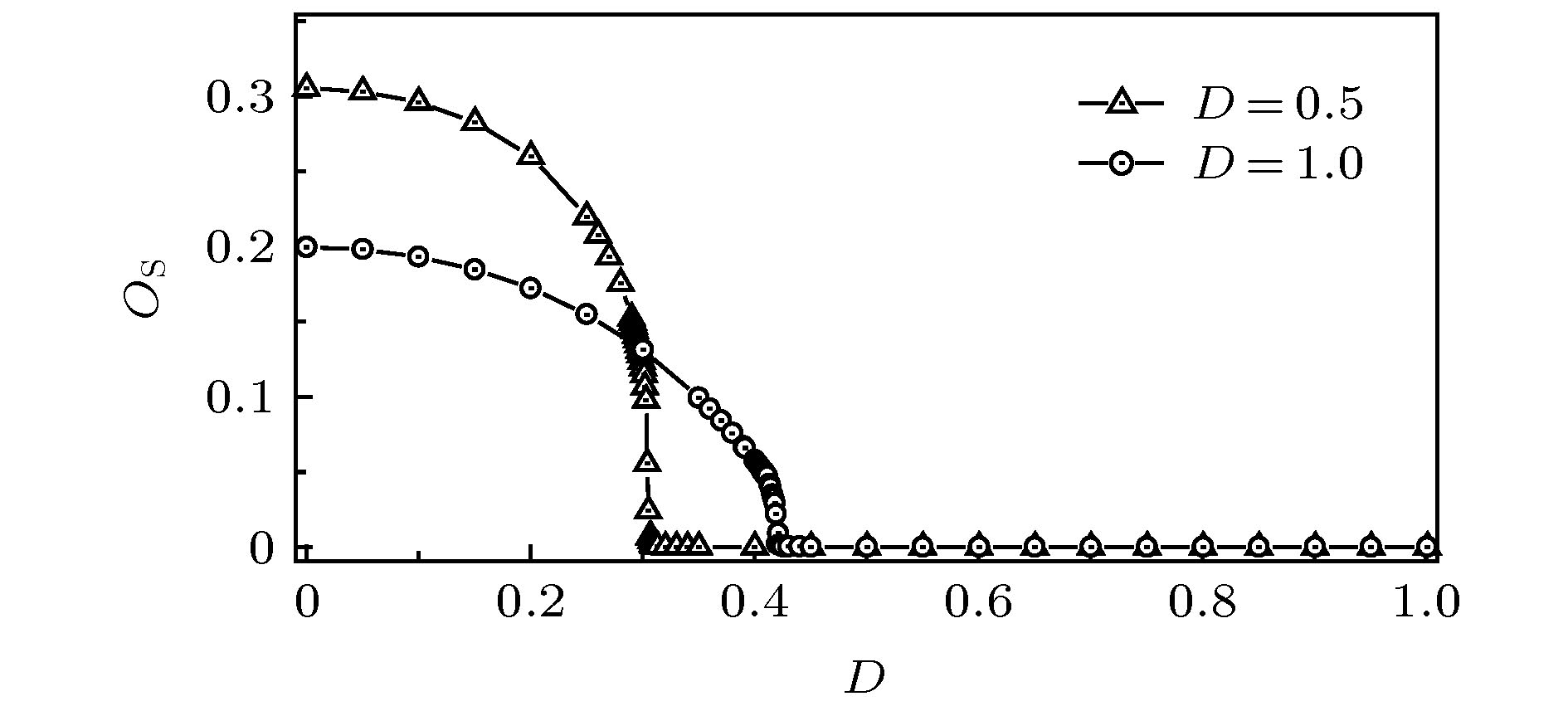
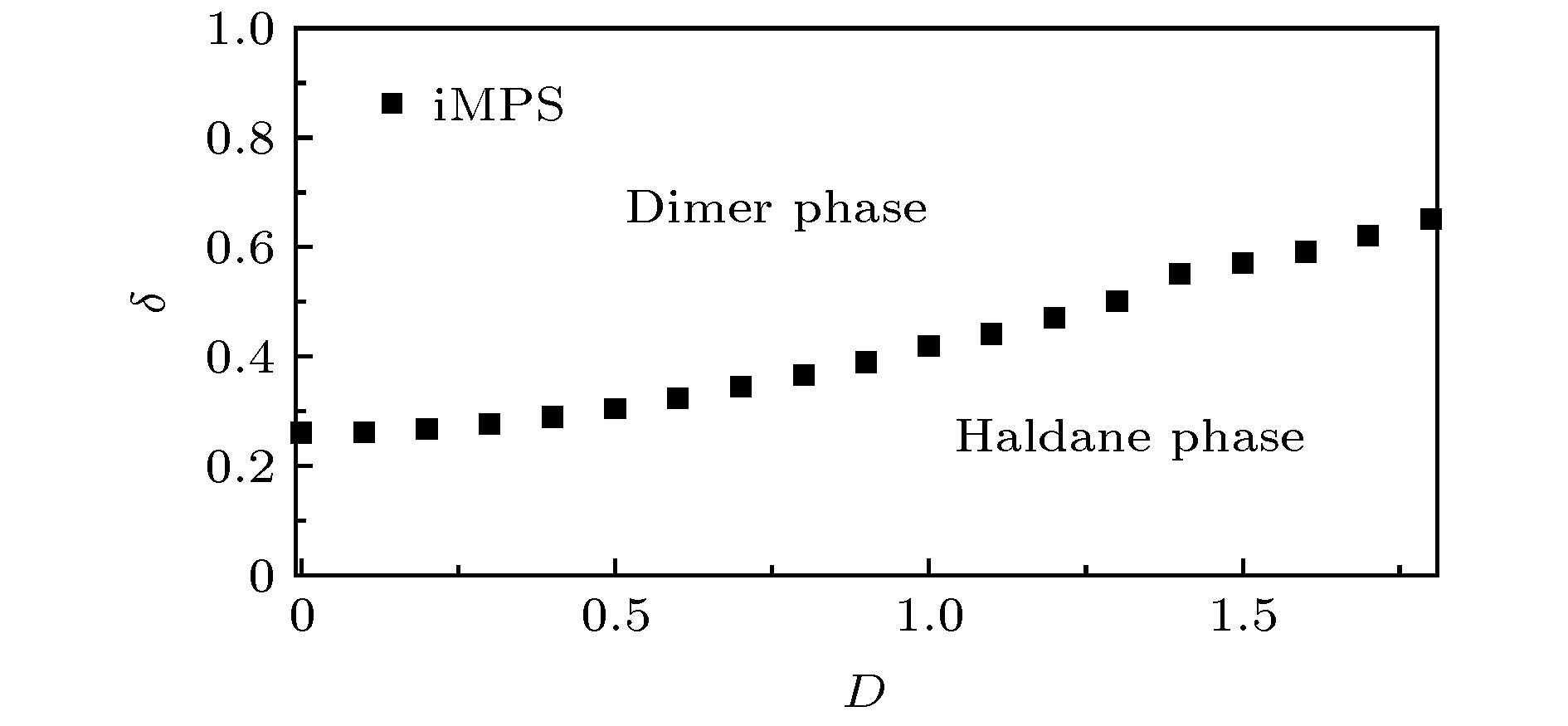
-
Quantum phase transitions are driven by quantum fluctuations due to the uncertainty principle in many-body physics. In quantum phase transitions, the ground-state changes dramatically. The quantum entanglement, specific heat, magnetization and other physical quantities diverge according to certain functions, and show specific scaling behaviors. In addition, there is a topological quantum phase transition beyond the conventional Landau-Ginzburg-Wilson paradigm, which is relevant to emergent phenomena in strongly correlated electron systems, with topological nonlocal order parameters as a salient feature. Thus, topological order is a new paradigm in the study of topological quantum phase transitions. To investigate competition mechanism of the different quantum spin interactions, in this paper, the one-dimensional spin-1 bond-alternating Heisenberg model with Dzyaloshinskii-Moriya (DM) interaction is considered. The DM interaction drives the quantum fluctuations resulting in a phase transition. By using the one-dimensional infinite matrix product state algorithm in tensor network representation, the quantum entanglement entropy and order parameters are calculated from the ground-state function. The numerical result shows that with the change of bond alternating strength, there is a quantum phase transition from the topological ordered Haldane phase to the local ordered dimer phase. Based on the von Neumann entropy and order parameter, the phase diagram of this model is obtained. There is a critical line that separates the Haldane and the dimer phase. The DM interaction inhibits the dimerization of the quantum spin system and finally breaks the fully dimerization. Due to the fact that the structurally symmetry of system is broken, the local dimer order exists in the whole parameter range when the bond-alternative strength parameter changes. The first derivative of the local dimer order behaves as a peak corresponding to the critical point. Furthermore, from the numerical scaling of the first derivative of dimer order and the non-local string order near the phase transition point, the characteristic critical exponents α and β are obtained, respectively. It shows that the characteristic critical exponent α decreases, and β increases gradually with the interaction strength of DM increasing. The resulting state i.e. the anti-symmetric anisotropic DM interaction produced by spin-orbit coupling, affects the critical properties of the system in the phase transition. This reveals that the competition mechanism of the quantum spin interaction, also provides some guidance for the future study of the critical behavior in topological quantum phase transition with the DM interaction. -
Keywords:
- quantum phase transition /
- quantum entanglement /
- Dzyaloshinskii-Moriya interaction /
- topological order /
- critical exponent
[1] Sachdev S 2011 Quantum Phase Transitions (2nd Ed.) (Cambridge: Cambridge University Press) pp1–501
[2] Rizzi M, Rossini D, Chiara G D, Montangero S, Fazio R 2005 Phys. Rev. Lett. 95 240404
Google Scholar
[3] Hu S, Normand B, Wang X, Yu L 2011 Phys. Rev. B 84 220402(R)
Google Scholar
[4] Adelhardt P, Gritsch J, Hille M, Reiss D A, Schmidt K P 2017 Phys. Rev. B 96 235123
Google Scholar
[5] Amico L, Fazio R, Osterloh A, Vedral V 2008 Rev. Mod. Phys. 80 517
Google Scholar
[6] Nehra R, Bhakuni D S, Gangadharaiah S, Sharma A 2018 Phys. Rev. B 98 045120
Google Scholar
[7] Zanardi P, Paunković N 2006 Phys. Rev. E 74 031123
Google Scholar
[8] Haldane F D M 1983 Phys. Rev. Lett. 50 1153
Google Scholar
[9] Ueda H, Nakano H, Kusakabe K 2008 Phys. Rev. B 78 224402
Google Scholar
[10] Su Y H, Cho S Y, Li B, Wang H, Zhou H 2012 J. Phys. Soc. Jpn. 81 074003
Google Scholar
[11] Dzyaloshinsky I 1958 J. Phys. Chem. Solids 4 241
Google Scholar
[12] Moriya T 1960 Phys. Rev. Lett. 4 228
Google Scholar
[13] Dmitrienko V E, Chizhikov V A 2012 Phys. Rev. Lett. 108 187203
Google Scholar
[14] Kargarian M, Jafari R, and Langari A 2009 Phys. Rev. A 79 042319
Google Scholar
[15] Thio T, Aharony A 1994 Phys. Rev. Lett. 73 894
Google Scholar
[16] Zhao J, Wang X, Xiang T, Su Z, Yu L 2003 Phys. Rev. Lett. 90 207204
Google Scholar
[17] Kohgi M, Iwasa K, Mignot J M, Fak B, Gegenwart P, Lang M, Ochiai A, Aoki H, Suzuki T 2001 Phys. Rev. Lett. 86 2439
Google Scholar
[18] Tsukada I, Takeya J T, Masuda T, Uchinokura K 2001 Phys. Rev. Lett. 87 127203
Google Scholar
[19] Stüßer N, Schotte U, Hoser A, Meschke M, Meißner M, Wosnitza J 2002 J. Phys. Condens. Matter 14 5161
Google Scholar
[20] Vidal G 2007 Phys. Rev. Lett. 98 070201
Google Scholar
[21] Suzuki M 1990 Phys. Lett. A 146 319
Google Scholar
[22] Kim J, Stamps R L, Camley R E 2016 Phys. Rev. Lett. 117 197204
Google Scholar
[23] Rózsa L, Atxitia U, Nowak U 2017 Phys. Rev. B 96 094436
Google Scholar
[24] Kenndey T, Tasaki H 1992 Commun. Math. Phys. 147 431
Google Scholar
[25] Su Y H, Chen A M, Xiang C, Wang H, Xia C, Wang J 2016 J. Stat. Mech. 2016 123102
Google Scholar
[26] Osterloh A, Amico L, Falci G, Fazio R 2002 Nature 416 608
Google Scholar
[27] Korepin V E 2004 Phys. Rev. Lett. 92 096402
Google Scholar
[28] Chung M, Landau D P 2011 Phys. Rev. B 83 113104
Google Scholar
[29] den Nijs M, Rommelse K 1989 Phys. Rev. B 40 4709
Google Scholar
[30] Wu F Y 1982 Rev. Mod. Phys. 54 235
Google Scholar
[31] Wiseman S, Domany E 1993 Phys. Rev. E 48 4080
[32] Ding C, Wang Y, Zhang W, Guo W 2013 Phys. Rev. E 88 042117
Google Scholar
-
图 1 D = 0.5和D = 1时(a)奇键von Neumann熵
${S_{\rm{o}}}$ 和(b)偶键von Neumann熵${S_{\rm{e}}}$ 随$\delta $ 的变化Figure 1. (a) Odd-bond von Neumann entropy
${S_{\rm{o}}}$ and (b) even-bond von Neumann entropy${S_{\rm{e}}}$ as a function of$\delta $ for D = 0.5 and D = 1.
图 2 二聚化序
${O_{\rm{D}}}$ 随$\delta $ 的变化(内插图为${O_{\rm{D}}}$ 随$\delta $ 的一阶导数) (a) D = 0.5, (b) D = 1Figure 2. Dimer order parameter
${O_{\rm{D}}}$ as a function of$\delta $ for (a) D = 0.5 and (b) D = 1 (first derivation of${O_{\rm{D}}}$ in the insert).
图 3 二聚化序
${O_{\rm{D}}}$ 随DM相互作用强度D的变化, 其中键交替参数选取为$\delta = 1$ Figure 3. Dimer order parameter
${O_{\rm{D}}}$ as a function of DM interaction D for$\delta =1 $ .
图 4 非局域拓扑弦序
${O_{\rm{S}}}$ 随$\delta $ 的变化Figure 4. Non-local topological string order parameter as a function of
$\delta $ .
图 5 含有DM相互作用的自旋1键交替反铁磁海森伯模型相图
Figure 5. Phase diagram of spin-1 bond-alternating Heisenberg model with DM interaction.
图 6 相互作用参数(a) D = 0, (b) D = 0.5和(c) D = 1时在对应相变点
$\delta _{\rm{c}}^{D = 0} \approx 0.260$ ,$\delta _{\rm{c}}^{D = 0.5} \approx 0.305$ 和$\delta _{\rm{c}}^{D = 1} \approx$ 0.418附近二聚化序的一阶导数$\partial {O_{\rm{D}}}/\partial \delta $ 的特征临界指数$\alpha $ 拟合Figure 6. Characteristic critical exponent
$\alpha $ from the first derivative of the dimer order$\partial {O_{\rm{D}}}/\partial \delta $ for (a) D = 0, (b) D = 0.5, and (c) D = 1 in the vicinity of the critical points$\delta _{\rm{c}}^{D = 0} \approx 0.260$ ,$\delta _{\rm{c}}^{D = 0.5} \approx 0.305$ , and$\delta _{\rm{c}}^{D = 1} \approx 0.418$ , respectively.
图 7 相互作用参数(a) D = 0.5和(b) D = 1时在对应相变点
$\delta _{\rm{c}} ^{D = 0.5} \approx 0.305$ 和$\delta _{\rm{c}}^{D = 1} \approx 0.418$ 附近非局域拓扑弦序${O_{\rm{S}}}$ 的特征临界指数$\beta $ 拟合Figure 7. Characteristic critical exponent
$\beta $ from the nonlocal topological string order${O_{\rm{S}}}$ for (a) D = 0.5 and (b) D = 1 in the vicinity of the critical points$\delta _{\rm{c}} ^{D = 0.5} \approx 0.305$ and$\delta _{\rm{c}}^{D = 1} \approx 0.418$ , respectively.表 1 不同DM相互作用强度下的临界指数
$\beta $ Table 1. Characteristic critical exponent β for different DM interactions
D 0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0 $\beta $ 0.082 0.087 0.092 0.098 0.099 0.101 0.106 0.109 0.115 0.117 0.121 
DownLoad: CSV
-
[1] Sachdev S 2011 Quantum Phase Transitions (2nd Ed.) (Cambridge: Cambridge University Press) pp1–501
[2] Rizzi M, Rossini D, Chiara G D, Montangero S, Fazio R 2005 Phys. Rev. Lett. 95 240404
Google Scholar
[3] Hu S, Normand B, Wang X, Yu L 2011 Phys. Rev. B 84 220402(R)
Google Scholar
[4] Adelhardt P, Gritsch J, Hille M, Reiss D A, Schmidt K P 2017 Phys. Rev. B 96 235123
Google Scholar
[5] Amico L, Fazio R, Osterloh A, Vedral V 2008 Rev. Mod. Phys. 80 517
Google Scholar
[6] Nehra R, Bhakuni D S, Gangadharaiah S, Sharma A 2018 Phys. Rev. B 98 045120
Google Scholar
[7] Zanardi P, Paunković N 2006 Phys. Rev. E 74 031123
Google Scholar
[8] Haldane F D M 1983 Phys. Rev. Lett. 50 1153
Google Scholar
[9] Ueda H, Nakano H, Kusakabe K 2008 Phys. Rev. B 78 224402
Google Scholar
[10] Su Y H, Cho S Y, Li B, Wang H, Zhou H 2012 J. Phys. Soc. Jpn. 81 074003
Google Scholar
[11] Dzyaloshinsky I 1958 J. Phys. Chem. Solids 4 241
Google Scholar
[12] Moriya T 1960 Phys. Rev. Lett. 4 228
Google Scholar
[13] Dmitrienko V E, Chizhikov V A 2012 Phys. Rev. Lett. 108 187203
Google Scholar
[14] Kargarian M, Jafari R, and Langari A 2009 Phys. Rev. A 79 042319
Google Scholar
[15] Thio T, Aharony A 1994 Phys. Rev. Lett. 73 894
Google Scholar
[16] Zhao J, Wang X, Xiang T, Su Z, Yu L 2003 Phys. Rev. Lett. 90 207204
Google Scholar
[17] Kohgi M, Iwasa K, Mignot J M, Fak B, Gegenwart P, Lang M, Ochiai A, Aoki H, Suzuki T 2001 Phys. Rev. Lett. 86 2439
Google Scholar
[18] Tsukada I, Takeya J T, Masuda T, Uchinokura K 2001 Phys. Rev. Lett. 87 127203
Google Scholar
[19] Stüßer N, Schotte U, Hoser A, Meschke M, Meißner M, Wosnitza J 2002 J. Phys. Condens. Matter 14 5161
Google Scholar
[20] Vidal G 2007 Phys. Rev. Lett. 98 070201
Google Scholar
[21] Suzuki M 1990 Phys. Lett. A 146 319
Google Scholar
[22] Kim J, Stamps R L, Camley R E 2016 Phys. Rev. Lett. 117 197204
Google Scholar
[23] Rózsa L, Atxitia U, Nowak U 2017 Phys. Rev. B 96 094436
Google Scholar
[24] Kenndey T, Tasaki H 1992 Commun. Math. Phys. 147 431
Google Scholar
[25] Su Y H, Chen A M, Xiang C, Wang H, Xia C, Wang J 2016 J. Stat. Mech. 2016 123102
Google Scholar
[26] Osterloh A, Amico L, Falci G, Fazio R 2002 Nature 416 608
Google Scholar
[27] Korepin V E 2004 Phys. Rev. Lett. 92 096402
Google Scholar
[28] Chung M, Landau D P 2011 Phys. Rev. B 83 113104
Google Scholar
[29] den Nijs M, Rommelse K 1989 Phys. Rev. B 40 4709
Google Scholar
[30] Wu F Y 1982 Rev. Mod. Phys. 54 235
Google Scholar
[31] Wiseman S, Domany E 1993 Phys. Rev. E 48 4080
[32] Ding C, Wang Y, Zhang W, Guo W 2013 Phys. Rev. E 88 042117
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 7712
- PDF Downloads: 144
- Cited By: 0
