Properties of vacancies and N-doping in monolayer g-ZnO: First-principles calculation and molecular orbital theory analysis

Huang Bing-Quan; Zhou Tie-Ge; Wu Dao-Xiong; Zhang Zhao-Fu; Li Bai-Kui

doi:10.7498/aps.68.20191258

Abstract

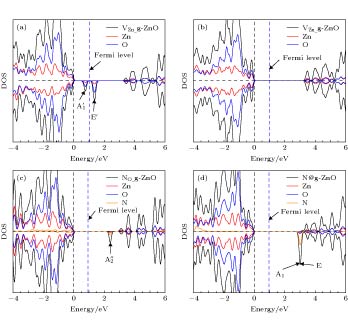
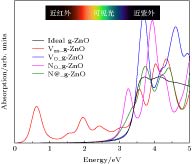
The geometric structure, electronic structure, magnetic properties and absorption spectrum of graphene-like ZnO (g-ZnO) monolayer supercell with defects are systemically studied by the first-principles calculation based on density functional theory in this work. The defect supercell model includes zinc atom vacancy (V_{Zn_}g-ZnO), oxygen atom vacancy (V_{O_}g-ZnO), nitrogen atom substituted for oxygen atom (N_{O_}g-ZnO) and nitrogen adsorbed on the g-ZnO monolayer (N@g-ZnO). The results indicate that the geometric deformation induced by N-doping in N_{O_}g-ZnO and N@g-ZnO structure is negligible, while that of supercell with vacancy is relatively large. The O atoms neighboring a Zn vacancy center in V_{Zn_}g-ZnO model move away from each other as a result of symmetry breaking. As a contrast, three N atoms around V_O center move into V_{Zn_}g-ZnO supercell. The pristine g-ZnO is non-magnetic. But the magnetic moment of V_Zn_g-ZnO is 2.00 μ_B in total as a result of symmetry breaking. The partial magnetic moment mainly results from the p-orbitals of the three neighboring O atoms. V_{O_}g-ZnO has no magnetic moment, but possesses the electronic structure with identical spin-up and spin-down. The total magnetic moment of the N-doped N_{O_}g-ZnO is 1.00 μ_B, and the total magnetic moment of N@g-ZnO is 3.00 μ_B. Their local magnetic moments are mainly contributed by the p-orbitals of N atom. The density of states and the spin density are given to analyze the magnetic properties. Based on the supercell local symmetry and molecular orbital theory, the origin of magnetic moment is well explained. The magnetic V_{Zn_}g-ZnO, N_{O_}g-ZnO and N@g-ZnO supercell are found to have a D_3h, D_3h and C_3v local symmetry, respectively, which well explains that their total magnetic moments are 2.00 μ_B, 1.00 μ_B and 3.00 μ_B, respectively. The optical absorption characteristics are also discussed. An enhancement of light absorption can be observed for the defective supercells, due to the introduction of defect states into the band gap. The optical transition between gap state and valance band leads to the below band gap absorption. These results are of insightful guidance for understanding properties of graphene-like ZnO monolayer as well as g-ZnO with vacancy and N dopant, and can be theoretically adopted for investigating the nano-electronic devices and catalytic applications based on g-ZnO monolayer.

Keywords:

Authors and contacts

1.
College of Physics and Optoelectronic Engineeing, Shenzhen University, Shenzhen 518060, China
2.
College of Electronic Information and Optical Engineering, Nankai University, Tianjin 300350, China
3.
Hefei National Laboratory of Physical Sciences at the Microscale, University of Science and Technology of China, Hefei 230026, China
4.
Department of Engineering, Cambridge University, Cambridge CB2 1PZ, United Kingdom

Corresponding author: Li Bai-Kui, libk@szu.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 61604098) and the Fundamental Research Funds for the Central Universities of Ministry of Education of China (Grant No. 63191740)

FullText HTML

References

[1]	Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsov A A 2004 Science 306 666 Google Scholar
[2]	Zhang Y, Tan Y, Stormer H L, Kim P 2005 Nature 438 201 Google Scholar
[3]	Kerelsky A, Mcgilly L J, Kennes D M, Xian L, Yankowitz M, Chen S, Watanabe K, Taniguchi T, Hone J, Dean C, Rubio A, Pasupathy A N 2019 Nature 572 95 Google Scholar
[4]	Ta H, Zhao L, Pohl D, Pang J, Trzebicka B, Rellinghaus B, Pribat D, Gemming T, Liu Z, Bachmatiuk A, Rümmeli M 2016 Crystals 6 100 Google Scholar
[5]	Weng Q, Wang X, Wang X, Bando Y, Golberg D 2016 Chem. Soc. Rev. 45 3989 Google Scholar
[6]	Zhang Z, Geng Z, Cai D, Pan T, Chen Y, Dong L, Zhou T 2015 Physica E 65 24 Google Scholar
[7]	Wang Q H, Kalantar-Zadeh K, Kis A, Coleman J N, Strano M S 2012 Nat. Nanotechnol. 7 699 Google Scholar
[8]	Gao Z, Zhou Z, Tománek D 2019 ACS Nano 13 5103 Google Scholar
[9]	Ye M, Seo H, Galli G 2019 npj Comput. Mater. 5 44 Google Scholar
[10]	Zhu C, Gao D, Ding J W, Chao D, Wang J 2018 Chem. Soc. Rev. 47 4332 Google Scholar
[11]	Liu Y, Huang Y, Duan X 2019 Nature 567 323 Google Scholar
[12]	Claeyssens F, Freeman C L, Allan N L, Sun Y, Ashfold M N R, Harding J H 2005 J. Mater. Chem. 15 139 Google Scholar
[13]	Tusche C, Meyerheim H L, Kirschner J 2007 Phys. Rev. Lett. 99 26102 Google Scholar
[14]	Topsakal M, Cahangirov S, Bekaroglu E, Ciraci S 2009 Phys. Rev. B 80 235119 Google Scholar
[15]	Zheng F B, Zhang C W, Wang P J, Luan H X 2012 J. Appl. Phys. 111 44329 Google Scholar
[16]	Peng Q, Liang C, Ji W, De S 2013 Comp. Mater. Sci. 68 320 Google Scholar
[17]	Guo H, Zhao Y, Lu N, Kan E, Zeng X C, Wu X, Yang J 2012 J. Phys. Chem. C 116 11336 Google Scholar
[18]	Chen J L, Devi N, Li N, Fu D J, Ke X W 2018 Chin. Phys. B 27 086102 Google Scholar
[19]	Tan J T, Zhang S F, Qian M C, Luo H J, Wu F, Long X M, Fang L, Wei D P, Hu B S 2018 Chin. Phys. B 27 114401 Google Scholar
[20]	Zheng S W, Fan G H, He M, Zhang T 2014 Chin. Phys. B 23 066301 Google Scholar
[21]	侯清玉, 曲灵丰, 赵春旺 2016 65 057401 Google Scholar Hou Q Y, Qu L F, Zhao C W 2016 Acta Phys. Sin. 65 057401 Google Scholar
[22]	侯清玉, 李勇, 赵春旺 2017 66 067202 Google Scholar Hou Q Y, Li Y, Zhao C 2017 Acta Phys. Sin. 66 067202 Google Scholar
[23]	张梅玲, 陈玉红, 张材荣, 李公平 2019 68 087101 Google Scholar Zhang M L, Chen Y H, Zhang C R, Li G P 2019 Acta Phys. Sin. 68 087101 Google Scholar
[24]	张丽丽, 夏桐, 刘桂安, 雷博程, 赵旭才, 王少霞, 黄以能 2019 68 017401 Google Scholar Zhang L L, Xia T, Liu G A, Lei B C, Zhao X C, Wang S X, Huang Y N 2019 Acta P hys. Sin. 68 017401 Google Scholar
[25]	Sun M, Ren Q, Zhao Y, Chou J, Yu J, Tang W 2017 Carbon 120 265 Google Scholar
[26]	张召富, 周铁戈, 左旭 2013 62 083102 Google Scholar Zhang Z F, Zhou T G, Zuo X 2013 Acta Phys. Sin. 62 083102 Google Scholar
[27]	张召富, 耿朝晖, 王鹏, 胡耀乔, 郑宇斐, 周铁戈 2013 62 246301 Google Scholar Zhang Z F, Geng Z H, Wang P, Hu Y Q, Zheng Y F, Zhou T G 2013 Acta Phys. Sin. 62 246301 Google Scholar
[28]	Zhang Z F, Zhou T G, Zhao H Y, Wei X L 2014 Chin. Phys. B 23 016801 Google Scholar
[29]	Hohenberg P, Kohn W 1964 Phys. Rev. 136 864 Google Scholar
[30]	Kresse G, Furthmüller J 1996 Phys. Rev. B 54 11169 Google Scholar
[31]	Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865 Google Scholar
[32]	Heyd J, Scuseria G E, Ernzerhof M 2003 J. Chem. Phys. 118 8207 Google Scholar
[33]	Blochl P E 1994 Phys. Rev. B 50 17953 Google Scholar
[34]	Wang V, Xu N, Liu J, Tang G, Geng W 2019 arXiv: 1908.08269 [cond-mat.mtrl-sci]
[35]	Cui J, Liang S, Sun S, Zheng X, Zhang J 2018 J. Phys.: Condens. Matter 30 175001 Google Scholar
[36]	Wang S, Ren C, Tian H, Yu J, Sun M 2018 Phys. Chem. Chem. Phys. 20 13394 Google Scholar
[37]	Niu X, Li Y, Shu H, Yao X, Wang J 2017 J. Phys. Chem. C 121 3648 Google Scholar
[38]	Liu Y, Liu H, Zhou H, Li T, Zhang L 2019 Appl. Surf. Sci. 466 133 Google Scholar
[39]	Tu Z C 2010 J. Comput. Theor. Nanosci. 7 1182

Cited By

图 1 理想g-ZnO的(a)晶体结构以及(b)能带和态密度, 其中g-ZnO价带顶对齐到0 eV

Figure 1. (a) Atomic structures, (b) band structure and density of states (DOS) of the g-ZnO primitive unit cell. The valence band maximum of g-ZnO is referred to 0 eV.

DownLoad: Full-Size Img PowerPoint

图 2 空位及掺杂超胞体系的几何结构示意图　(a) V_{Zn_}g-ZnO; (b) V_{O_}g-ZnO; (c) N_{O_}g-ZnO; (d) N原子吸附在六元环中心上方; (e) N原子吸附在Zn原子上方; (f) N原子吸附在O原子上方

Figure 2. Atomic structures of the g-ZnO supercells: (a) Ideal g-ZnO; (b) V_{O_}g-ZnO; (c) N_{O_}g-ZnO; (d) N atom at hollow site; (e) N atom on top of Zn atom; (f) N atom on top of O atom.

DownLoad: Full-Size Img PowerPoint

图 3 总态密度和分波态密度　(a) V_{Zn_}g-ZnO; (b) V_{O_}g-ZnO; (c) N_{O_}g-ZnO; (d) N@g-ZnO; 其中g-ZnO的价带顶对齐到0 eV

Figure 3. Total density of states and partial density of states: (a) V_{Zn_}g-ZnO; (b) V_{O_}g-ZnO; (c) N_{O_}g-ZnO; (d) N@g-ZnO. The valence band maximum of g-ZnO is referred to 0 eV.

DownLoad: Full-Size Img PowerPoint

图 4 能带结构　(a) V_{Zn_}g-ZnO; (b) V_{O_}g-ZnO; (c) N_{O_}g-ZnO; (d) N@g-ZnO; 其中g-ZnO的价带顶对齐到0 eV

Figure 4. Band structure of (a) V_{Zn_}g-ZnO; (b) V_{O_}g-ZnO; (c) N_{O_}g-ZnO; (d) N@g-ZnO. The valence band maximum of g-ZnO is referred to 0 eV.

DownLoad: Full-Size Img PowerPoint

图 5 分子轨道　(a) V_Zn_g-ZnO体系, O能级劈裂及电子填充示意图; (b) N_O_g-ZnO体系, p轨道分裂及电子填充示意图; (c) N@g-ZnO体系, p轨道分裂及电子填充示意图

Figure 5. Molecular orbital diagrams: (a) V_Zn_g-ZnO supercell, O energy level splitting and electron filling; (b) N_O_g-ZnO supercell, p-orbital splitting and electron filling; (c) N@g-ZnO supercell, p-orbital splitting and electron filling.

DownLoad: Full-Size Img PowerPoint

图 6 自旋密度图, 其中青色区域是自旋向上, 黄色区域是自旋向下　(a) V_{Zn_}g-ZnO体系的自旋密度; (b) V_{O_}g-ZnO体系的自旋密度; (c) N_{O_}g-ZnO体系的自旋密度; (d) N@g-ZnO体系的自旋密度

Figure 6. Spin density of (a) V_{Zn_}g-ZnO, (b) V_{O_}g-ZnO, (c) N_{O_}g-ZnO, and (d) N@g-ZnO supercells, respectively. Cyan is spin up and yellow is spin down.

DownLoad: Full-Size Img PowerPoint

图 7 理想g-ZnO及具有空位、掺杂的超胞体系的光学吸收谱

Figure 7. Optical absorption spectra of ideal g-ZnO and the defective supercell systems.

DownLoad: Full-Size Img PowerPoint

表 1 N掺杂g-ZnO单层的结构参数和结合能

Table 1. Structure parameters and binding energy of N-doped g-ZnO monolayer.

超胞模型 h_N/Å h_Zn/Å h_O/Å d_{Zn_N}/Å d_{O_N}/Å E_b/eV

N_{O_}g-ZnO 0.07 0.005 0.003 1.92 3.31 –4.12
N@g-ZnO 2.12 0.003 –0.104 2.84 2.93 –0.25

DownLoad: CSV

表 2 N掺杂g-ZnO单层的磁矩

Table 2. Magnetic moment of N-doped g-ZnO monolayer.

超胞模型 M_tot/µ_B M_N/µ_B M_Zn/µ_B M_O/µ_B

V_{O_}g-ZnO 0 — 0 0
V_{Zn_}g-ZnO 2.00 — 0.02 0.45
N_{O_}g-ZnO 1.00 0.59 0.01 0
N@g-ZnO 3.00 1.90 0 0.05

DownLoad: CSV

map

[1]	Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsov A A 2004 Science 306 666 Google Scholar
[2]	Zhang Y, Tan Y, Stormer H L, Kim P 2005 Nature 438 201 Google Scholar
[3]	Kerelsky A, Mcgilly L J, Kennes D M, Xian L, Yankowitz M, Chen S, Watanabe K, Taniguchi T, Hone J, Dean C, Rubio A, Pasupathy A N 2019 Nature 572 95 Google Scholar
[4]	Ta H, Zhao L, Pohl D, Pang J, Trzebicka B, Rellinghaus B, Pribat D, Gemming T, Liu Z, Bachmatiuk A, Rümmeli M 2016 Crystals 6 100 Google Scholar
[5]	Weng Q, Wang X, Wang X, Bando Y, Golberg D 2016 Chem. Soc. Rev. 45 3989 Google Scholar
[6]	Zhang Z, Geng Z, Cai D, Pan T, Chen Y, Dong L, Zhou T 2015 Physica E 65 24 Google Scholar
[7]	Wang Q H, Kalantar-Zadeh K, Kis A, Coleman J N, Strano M S 2012 Nat. Nanotechnol. 7 699 Google Scholar
[8]	Gao Z, Zhou Z, Tománek D 2019 ACS Nano 13 5103 Google Scholar
[9]	Ye M, Seo H, Galli G 2019 npj Comput. Mater. 5 44 Google Scholar
[10]	Zhu C, Gao D, Ding J W, Chao D, Wang J 2018 Chem. Soc. Rev. 47 4332 Google Scholar
[11]	Liu Y, Huang Y, Duan X 2019 Nature 567 323 Google Scholar
[12]	Claeyssens F, Freeman C L, Allan N L, Sun Y, Ashfold M N R, Harding J H 2005 J. Mater. Chem. 15 139 Google Scholar
[13]	Tusche C, Meyerheim H L, Kirschner J 2007 Phys. Rev. Lett. 99 26102 Google Scholar
[14]	Topsakal M, Cahangirov S, Bekaroglu E, Ciraci S 2009 Phys. Rev. B 80 235119 Google Scholar
[15]	Zheng F B, Zhang C W, Wang P J, Luan H X 2012 J. Appl. Phys. 111 44329 Google Scholar
[16]	Peng Q, Liang C, Ji W, De S 2013 Comp. Mater. Sci. 68 320 Google Scholar
[17]	Guo H, Zhao Y, Lu N, Kan E, Zeng X C, Wu X, Yang J 2012 J. Phys. Chem. C 116 11336 Google Scholar
[18]	Chen J L, Devi N, Li N, Fu D J, Ke X W 2018 Chin. Phys. B 27 086102 Google Scholar
[19]	Tan J T, Zhang S F, Qian M C, Luo H J, Wu F, Long X M, Fang L, Wei D P, Hu B S 2018 Chin. Phys. B 27 114401 Google Scholar
[20]	Zheng S W, Fan G H, He M, Zhang T 2014 Chin. Phys. B 23 066301 Google Scholar
[21]	侯清玉, 曲灵丰, 赵春旺 2016 65 057401 Google Scholar Hou Q Y, Qu L F, Zhao C W 2016 Acta Phys. Sin. 65 057401 Google Scholar
[22]	侯清玉, 李勇, 赵春旺 2017 66 067202 Google Scholar Hou Q Y, Li Y, Zhao C 2017 Acta Phys. Sin. 66 067202 Google Scholar
[23]	张梅玲, 陈玉红, 张材荣, 李公平 2019 68 087101 Google Scholar Zhang M L, Chen Y H, Zhang C R, Li G P 2019 Acta Phys. Sin. 68 087101 Google Scholar
[24]	张丽丽, 夏桐, 刘桂安, 雷博程, 赵旭才, 王少霞, 黄以能 2019 68 017401 Google Scholar Zhang L L, Xia T, Liu G A, Lei B C, Zhao X C, Wang S X, Huang Y N 2019 Acta P hys. Sin. 68 017401 Google Scholar
[25]	Sun M, Ren Q, Zhao Y, Chou J, Yu J, Tang W 2017 Carbon 120 265 Google Scholar
[26]	张召富, 周铁戈, 左旭 2013 62 083102 Google Scholar Zhang Z F, Zhou T G, Zuo X 2013 Acta Phys. Sin. 62 083102 Google Scholar
[27]	张召富, 耿朝晖, 王鹏, 胡耀乔, 郑宇斐, 周铁戈 2013 62 246301 Google Scholar Zhang Z F, Geng Z H, Wang P, Hu Y Q, Zheng Y F, Zhou T G 2013 Acta Phys. Sin. 62 246301 Google Scholar
[28]	Zhang Z F, Zhou T G, Zhao H Y, Wei X L 2014 Chin. Phys. B 23 016801 Google Scholar
[29]	Hohenberg P, Kohn W 1964 Phys. Rev. 136 864 Google Scholar
[30]	Kresse G, Furthmüller J 1996 Phys. Rev. B 54 11169 Google Scholar
[31]	Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865 Google Scholar
[32]	Heyd J, Scuseria G E, Ernzerhof M 2003 J. Chem. Phys. 118 8207 Google Scholar
[33]	Blochl P E 1994 Phys. Rev. B 50 17953 Google Scholar
[34]	Wang V, Xu N, Liu J, Tang G, Geng W 2019 arXiv: 1908.08269 [cond-mat.mtrl-sci]
[35]	Cui J, Liang S, Sun S, Zheng X, Zhang J 2018 J. Phys.: Condens. Matter 30 175001 Google Scholar
[36]	Wang S, Ren C, Tian H, Yu J, Sun M 2018 Phys. Chem. Chem. Phys. 20 13394 Google Scholar
[37]	Niu X, Li Y, Shu H, Yao X, Wang J 2017 J. Phys. Chem. C 121 3648 Google Scholar
[38]	Liu Y, Liu H, Zhou H, Li T, Zhang L 2019 Appl. Surf. Sci. 466 133 Google Scholar
[39]	Tu Z C 2010 J. Comput. Theor. Nanosci. 7 1182

[1]	Li Xin-Yue, Gao Guo-Xiang, Gao Qiang, Liu Chun-Sheng, Ye Xiao-Juan. Theoretical study of two-dimensional BeB₂ monolayer as anode material for magnesium ion batteries. Acta Physica Sinica, 2024, 73(11): 118201. doi: 10.7498/aps.73.20240134
[2]	Chen Si-Yu, Ye Xiao-Juan, Liu Chun-Sheng. Theoretical research of two-dimensional germanether in sodium-ion battery. Acta Physica Sinica, 2022, 71(22): 228202. doi: 10.7498/aps.71.20220572
[3]	Gao Xu-Dong, Yang De-Cao, Wei Wen-Jing, Li Gong-Ping. Simulation study of electron beam irradiation damage to ZnO and TiO₂. Acta Physica Sinica, 2021, 70(23): 234101. doi: 10.7498/aps.70.20211223
[4]	Liu Zi-Yuan, Pan Jin-Bo, Zhang Yu-Yang, Du Shi-Xuan. First principles calculation of two-dimensional materials at an atomic scale. Acta Physica Sinica, 2021, 70(2): 027301. doi: 10.7498/aps.70.20201636
[5]	Luo Chang-Wei, Qiu Meng-Lin, Wang Guang-Fu, Wang Ting-Shun, Zhao Guo-Qiang, Hua Qing-Song. Ions beam induced luminescence study of variation of defects in zinc oxide during ion implant and after annealing. Acta Physica Sinica, 2020, 69(10): 102901. doi: 10.7498/aps.69.20200029
[6]	Yin Yuan, Li Ling, Yin Wan-Jian. Theoretical and computational study on defects of solar cell materials. Acta Physica Sinica, 2020, 69(17): 177101. doi: 10.7498/aps.69.20200656
[7]	Zhang Li-Li, Xia Tong, Liu Gui-An, Lei Bo-Cheng, Zhao Xu-Cai, Wang Shao-Xia, Huang Yi-Neng. Electronic and optical properties of n-pr co-doped anatase TiO₂ from first-principles. Acta Physica Sinica, 2019, 68(1): 017401. doi: 10.7498/aps.68.20181531
[8]	Guo Jia-Jun, Dong Jing-Yu, Kang Xin, Chen Wei, Zhao Xu. Effect of transition metal element X (X=Mn, Fe, Co, and Ni) doping on performance of ZnO resistive memory. Acta Physica Sinica, 2018, 67(6): 063101. doi: 10.7498/aps.67.20172459
[9]	Hou Qing-Yu, Li Yong, Zhao Chun-Wang. First-principles study of Al-doped and vacancy on the magnetism of ZnO. Acta Physica Sinica, 2017, 66(6): 067202. doi: 10.7498/aps.66.067202
[10]	Wu Zi-Hua, Xie Hua-Qing, Zeng Qing-Feng. Preparation and thermoelectric properties of Ag-ZnO nanocomposites synthesized by means of sol-gel. Acta Physica Sinica, 2013, 62(9): 097301. doi: 10.7498/aps.62.097301
[11]	Li Hong-Lin, Zhang Zhong, Lü Ying-Bo, Huang Jin-Zhao, Zhang Ying, Liu Ru-Xi. First principles study on the electronic and optical properties of ZnO doped with rare earth. Acta Physica Sinica, 2013, 62(4): 047101. doi: 10.7498/aps.62.047101
[12]	Zhang Zhao-Fu, Zhou Tie-Ge, Zuo Xu. First-principles calculations of h-BN monolayers by doping with oxygen and sulfur. Acta Physica Sinica, 2013, 62(8): 083102. doi: 10.7498/aps.62.083102
[13]	Qin Jie-Ming, Tian Li-Fei, Zhao Dong-Xu, Jiang Da-Yong, Cao Jian-Ming, Ding Meng, Guo Zhen. Comprehensive Survey for the Frontier Disciplines. Acta Physica Sinica, 2011, 60(10): 107307. doi: 10.7498/aps.60.107307
[14]	Yuan Di, Huang Duo-Hui, Luo Hua-Feng, Wang Fan-Hou. First-principles study of Li-N acceptor pair codoped p-type ZnO. Acta Physica Sinica, 2010, 59(9): 6457-6465. doi: 10.7498/aps.59.6457
[15]	Cheng Xing-Wang, Li Xiang, Gao Yuan-Ling, Yu Zhou, Long Xue, Liu Ying. Synthesis and magnetic, optical properties of Co doped ZnO room-temperature ferromagnetic semiconductor. Acta Physica Sinica, 2009, 58(3): 2018-2022. doi: 10.7498/aps.58.2018
[16]	Guan Li, Li Qiang, Zhao Qing-Xun, Guo Jian-Xin, Zhou Yang, Jin Li-Tao, Geng Bo, Liu Bao-Ting. First-principles study of the optical properties of ZnO doped with Al, Ni. Acta Physica Sinica, 2009, 58(8): 5624-5631. doi: 10.7498/aps.58.5624
[17]	Yu Zhou, Li Xiang, Long Xue, Cheng Xing-Wang, Wang Jing-Yun, Liu Ying, Cao Mao-Sheng, Wang Fu-Chi. Study of synthesis and magnetic properties of Mn-doped ZnO diluted magnetic semiconductors. Acta Physica Sinica, 2008, 57(7): 4539-4544. doi: 10.7498/aps.57.4539
[18]	Bi Yan-Jun, Guo Zhi-You, Sun Hui-Qing, Lin Zhu, Dong Yu-Cheng. The electronic structure and optical properties of Co and Mn codoped ZnO from first-principle study. Acta Physica Sinica, 2008, 57(12): 7800-7805. doi: 10.7498/aps.57.7800
[19]	Duan Man-Yi, Xu Ming, Zhou Hai-Ping, Shen Yi-Bin, Chen Qing-Yun, Ding Ying-Chun, Zhu Wen-Jun. First-principles study on the electronic structure and optical properties of ZnO doped with transition metal and N. Acta Physica Sinica, 2007, 56(9): 5359-5365. doi: 10.7498/aps.56.5359
[20]	Chen Zhi-Quan, Kawasuso Atsuo. Vacancy-type defects induced by He-implantation in ZnO studied by a slow positron beam. Acta Physica Sinica, 2006, 55(8): 4353-4357. doi: 10.7498/aps.55.4353

Catalog

Vol. 68, Issue 24 - 2019-12-20

Metrics

Abstract views: 13879
PDF Downloads: 375
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板