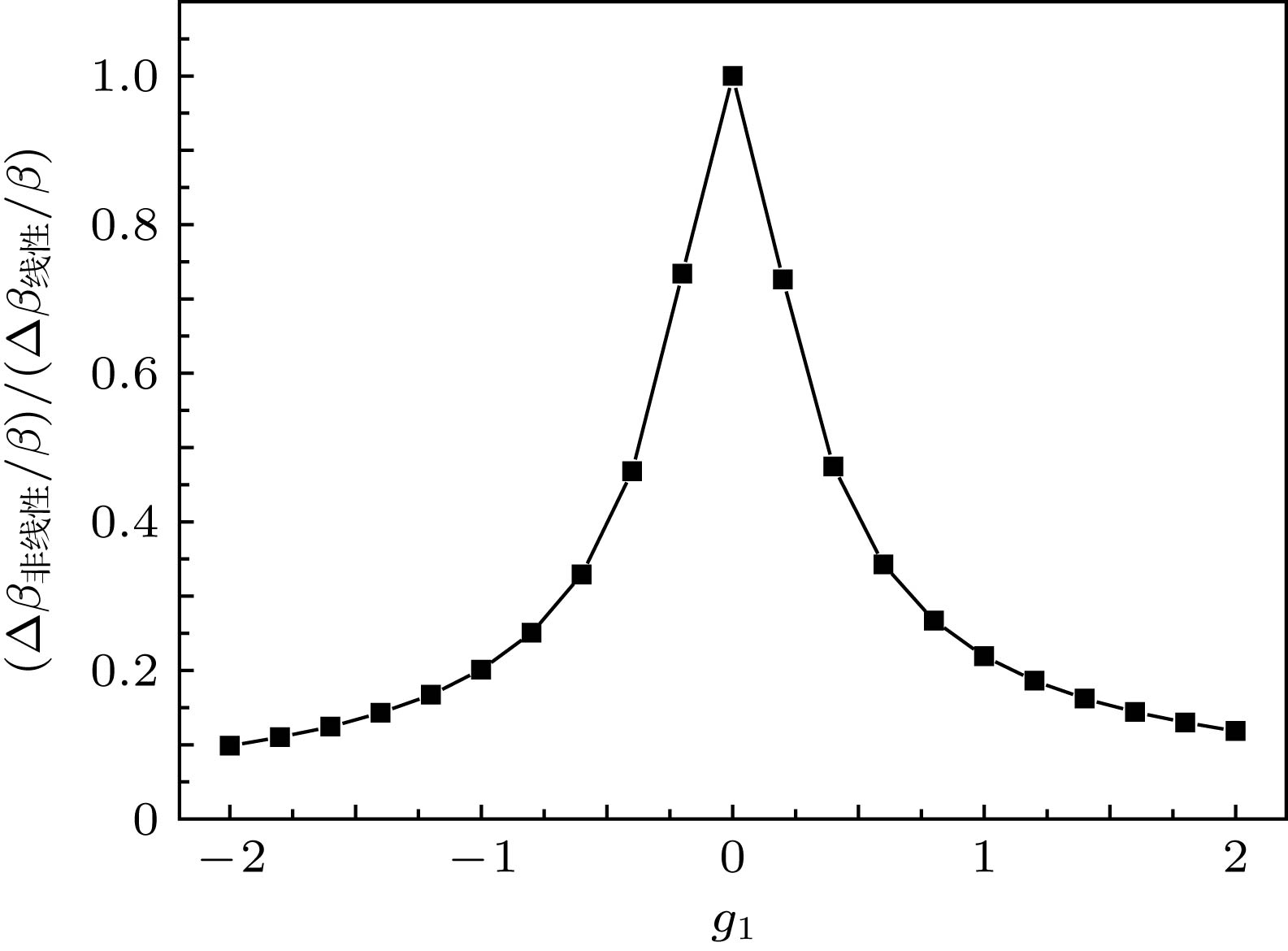
-
The interferometry of two Kapitza-Dirac (KD) pulses acting on cold atoms in a harmonic oscillator potential well is investigated by Feynman path integral method. The wave function and density distribution function of the system at a given time are calculated analytically by using the propagator under the action of an external field. The first KD pulse acts on cold atoms to produce a large number of modes in the harmonic oscillator potential well. The maximum value of wave packet of mode 0 is larger than those of other modes. These modes evolve along different paths. The external field changes the phase of each mode and makes the evolution path of the mode deviate from that without the external field. When the second KD pulse is added, it splits the mode of the first KD pulse, and thus generates more modes. These modes will evolve along different paths under the action of external field and harmonic potential well, and interfere with each other. At the moment of measurement, all the wave packets are separated without overlapping. The effect of the external field does not change the magnitude of the density distribution at the time of measurement, but makes the wave packet of each mode shift. The phase difference between adjacent modes decreases linearly with the increase of field intensity. When the external field is a gravity field, we calculate the Fisher information and the Cramer-Rao lowér bound. The Fisher information is proportional to the mass of atoms and inversely to the third power of harmonic potential well frequency. We can improve the measurement accuracy of interferometer by reducing the frequency of harmonic potential well and increasing atomic mass. When the initial state is the ground state of the harmonic potential well, the accuracy of the gravity acceleration measured by the interference device can be obtained to be 10–9 by using the experimental parameters. The initial state is the ground state of the harmonic potential well and the external field, and the calculation result indicates that the measurement accuracy will decrease. At the same time, the enhancement of inter-atomic repulsion and attraction interaction will also lead the measurement accuracy to increase.
-
Keywords:
- cold atoms /
- harmonic oscillator potential /
- propagator /
- Kapitza-Dirac pulse
[1] Fang J, Hu J, Chen X, Zhu H, Zhou L, Zhong J, Wang J, Zhan M 2018 Opt. Express 26 1586
Google Scholar
[2] Lu S B, Yao Z W, Li R B, Luo J, Barthwal S, Chen H H, Lu Z X, Wang J, Zhan M S 2018 Opt. Commun. 429 158
Google Scholar
[3] Fang B, Mielec N, Savoie D, Altorio M, Landragin A, Geiger R 2018 New J. Phys. 20 023020
Google Scholar
[4] Jaffe M, Xu V, Haslinger P, Müller H, Hamilton P 2018 Phys. Rev. Lett. 121 040402
Google Scholar
[5] Carey M, Saywell J, Elcock D, Belal M, Freegarde T 2019 Phys. Rev. A 99 023631
Google Scholar
[6] Wigley P B, Hardman K S, Freier C, Everitt P J, Legge S, Manju P, Close J D, Robins N P 2019 Phys. Rev. A 99 023615
Google Scholar
[7] Arias A, Lochead G, Wintermantel T M, Helmrich S, Whitlock S 2019 Phys. Rev. Lett. 122 053601
Google Scholar
[8] Zhou L, Xiong Z Y, Yang W, Tang B, Peng W C, Wang Y B, Xu P, Wang J, Zhan M S 2011 Chin. Phys. Lett. 28 013701
Google Scholar
[9] Imanishi Y, Sato T, Higashi T, Sun W, Okubo S 2004 Science 306 476
Google Scholar
[10] Kibble B P 1991 Proc. IEE: Science, Measurement and Technology 138 187
Google Scholar
[11] Blakely R J, Jachens R C 1991 Geological Society of America Bulletin 103 795
Google Scholar
[12] Peters A, Chung K Y, Chu S 2001 Metrologia 38 25
Google Scholar
[13] Rasel E M, Oberthaler M K, Batelaan H, Schmiedmayer J, Zeilinger A 1995 Phys. Rev. Lett. 75 2633
Google Scholar
[14] Giltner D M, McGowan R W, Lee S A 1995 Phys. Rev. Lett. 75 2638
Google Scholar
[15] 吴彬, 程冰, 付志杰, 朱栋, 周寅, 翁堪兴, 王肖隆, 林强 2018 67 190302
Google Scholar
Wu B, Cheng B, Fu Z J, Zhu D, Zhou Y, Wong K X, Wang X L, Lin Q 2018 Acta Phys. Sin. 67 190302
Google Scholar
[16] McGuirk J M, Foster G T, Fixler J B, Snadden M J, Kasevich M A 2002 Phys. Rev. A 65 033608
Google Scholar
[17] Fixler J B, Foster G T, McGuirk J M, Kasevich M A 2007 Science 315 74
Google Scholar
[18] Li W D, He T, Smerzi A 2014 Phys. Rev. Lett. 113 023003
Google Scholar
[19] 杨志安 2013 62 110302
Google Scholar
Yang Z A 2013 Acta Phys. Sin. 62 110302
Google Scholar
[20] He T C, Niu P B 2017 Phys. Lett. A 381 108
[21] Cheng R, He T, Li W, Smerzi A 2016 J. Mod. Phys. 7 2043
Google Scholar
[22] Sapiro R E, Zhang R, Raithel G 2009 Phys. Rev. A 79 043630
Google Scholar
[23] Keller C, Schmiedmayer J, Zeilinger A, Nonn T, Dürr S, Rempe G 1999 Appl. Phys. B: Lasers Opt. 69 303
Google Scholar
[24] Dong G J, Zhu J, Zhang W P, Malomed B A 2013 Phys. Rev. Lett. 110 250401
Google Scholar
[25] Zhu J, Dong G J, Shneider M N, Zhang W P 2011 Phys. Rev. Lett. 106 210403
Google Scholar
[26] Chwedenczuk J, Piazza F, Smerzi A 2013 Phys. Rev. A 87 033607
Google Scholar
[27] Shin Y, Saba M, Schirotzek A, Pasquini T A, Leanhardt A E, Pritchard D E, Ketterle W 2004 Phys. Rev. Lett. 92 150401
Google Scholar
[28] Lehtovaara L, Toivanen J, Eloranta J 2007 J. Comput. Phys. 221 148
Google Scholar
[29] Bandrauk A D, Shen H 1994 J. Phys. A 27 7147
Google Scholar
[30] Fattori M, D’Errico C, Roati G, Zaccanti M, Jona-Lasinio M, Modugno M, Inguscio M, Modugno G 2008 Phys. Rev. Lett. 100 080405
Google Scholar
-
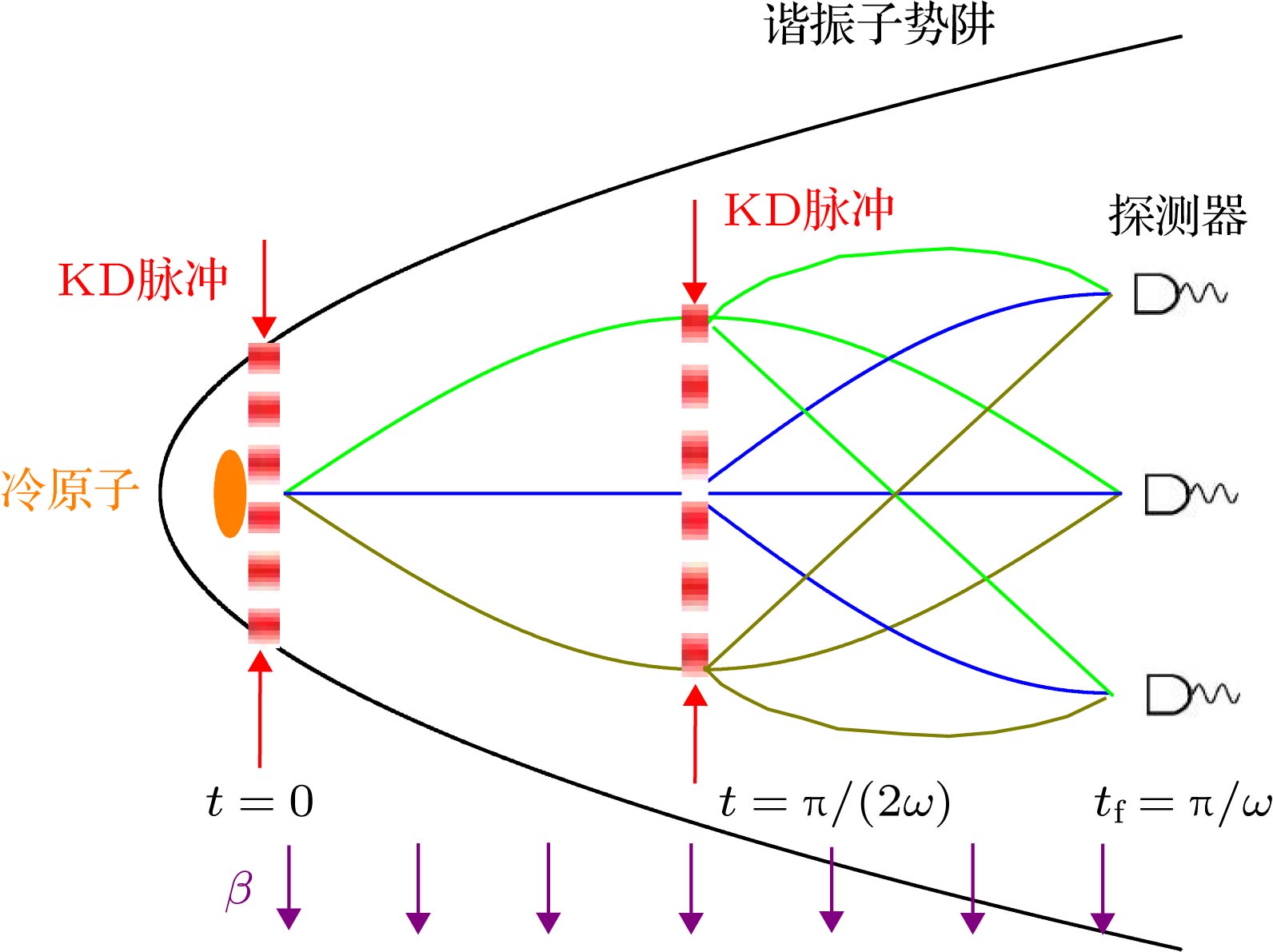
图 1 简谐势阱中冷原子受到两次KD脉冲的示意图 在测量时刻
${t_{\rm{f}}} ={\text{π}}/\omega $ , 不同模式相干叠加,$\beta $ 为外场Figure 1. Diagram of cold atoms with two KD pulses in harmonic oscillator potential. At the measurement time
${t_{\rm{f}}} = {\text{π}}/\omega $ , the coherent superposition of different modes occurs.$\beta $ is the external field.
图 2 没有外场的情况下(
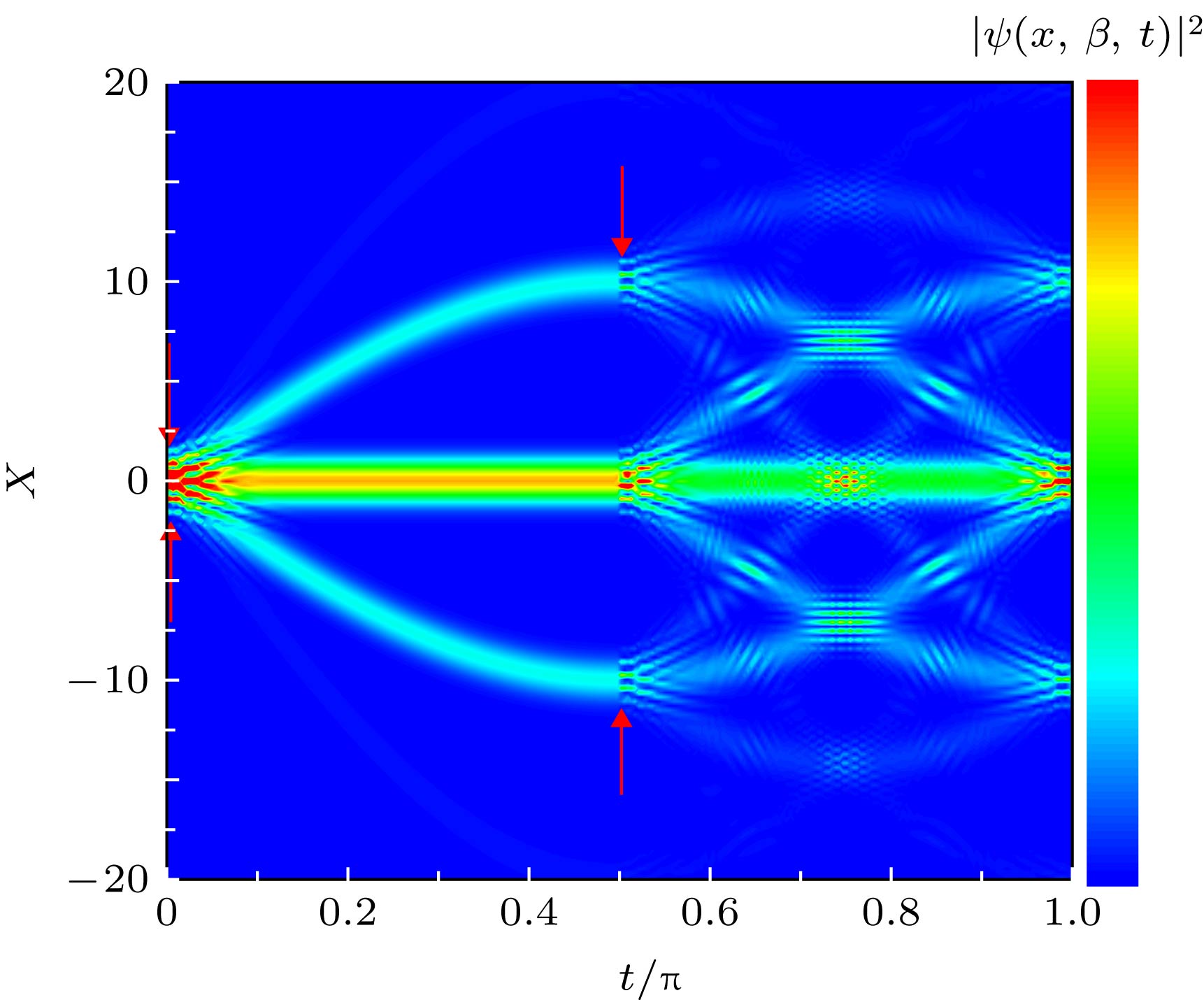
$\beta = 0$ ), 系统态密度分布函数的演化规律 无量纲参数为$V = 1,\; k = 10$ , 测量时刻在$t = {t_{\rm{f}}} = {\text{π}}$ , 两次KD脉冲都用红色箭头表示, 态密度演化图右边的彩色条表示态密度分布函数值由低(蓝色)变到高(红色)Figure 2. In the absence of an external field (
$\beta = 0$ ), the density distribution function of system varies with time. The dimensionless parameter are$V = 1,\; k = 10$ . The measurement time is at$t = {t_{\rm{f}}} ={\text{π}}$ . Both KD pulses are represented by red arrows. The color bar on the right side of the density of states evolution diagram indicates that the value of the density distribution function changes from low (blue) to high (red).
图 3 在外场
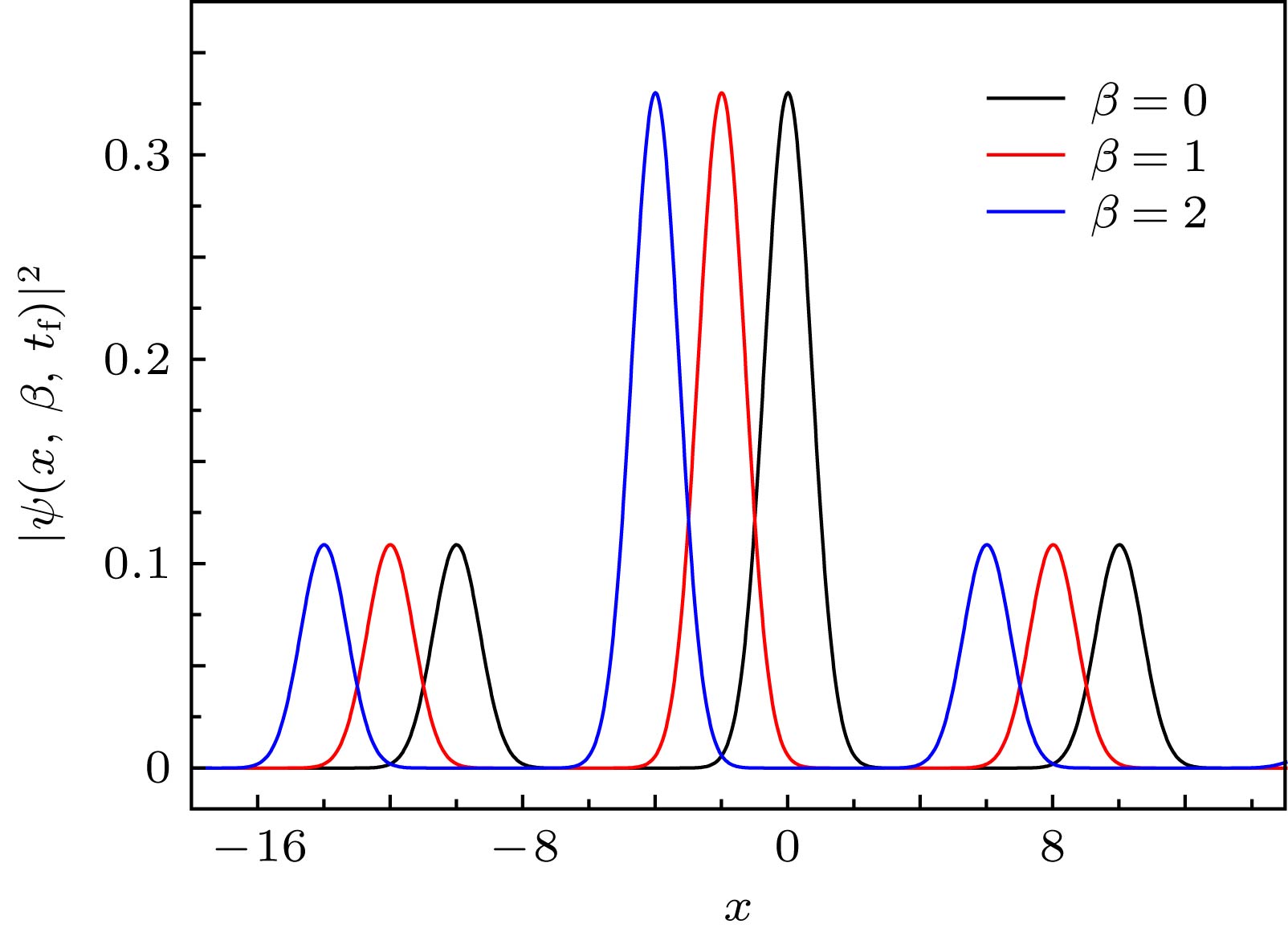
$\beta = 1$ 的情况下, 系统态密度分布函数的演化规律 图中除$\beta $ 之外的其他无量纲参数与图2相同, 红色箭头为KD脉冲, 测量时刻在$t = {t_{\rm{f}}} = {\text{π}}$ Figure 3. In the case of external field
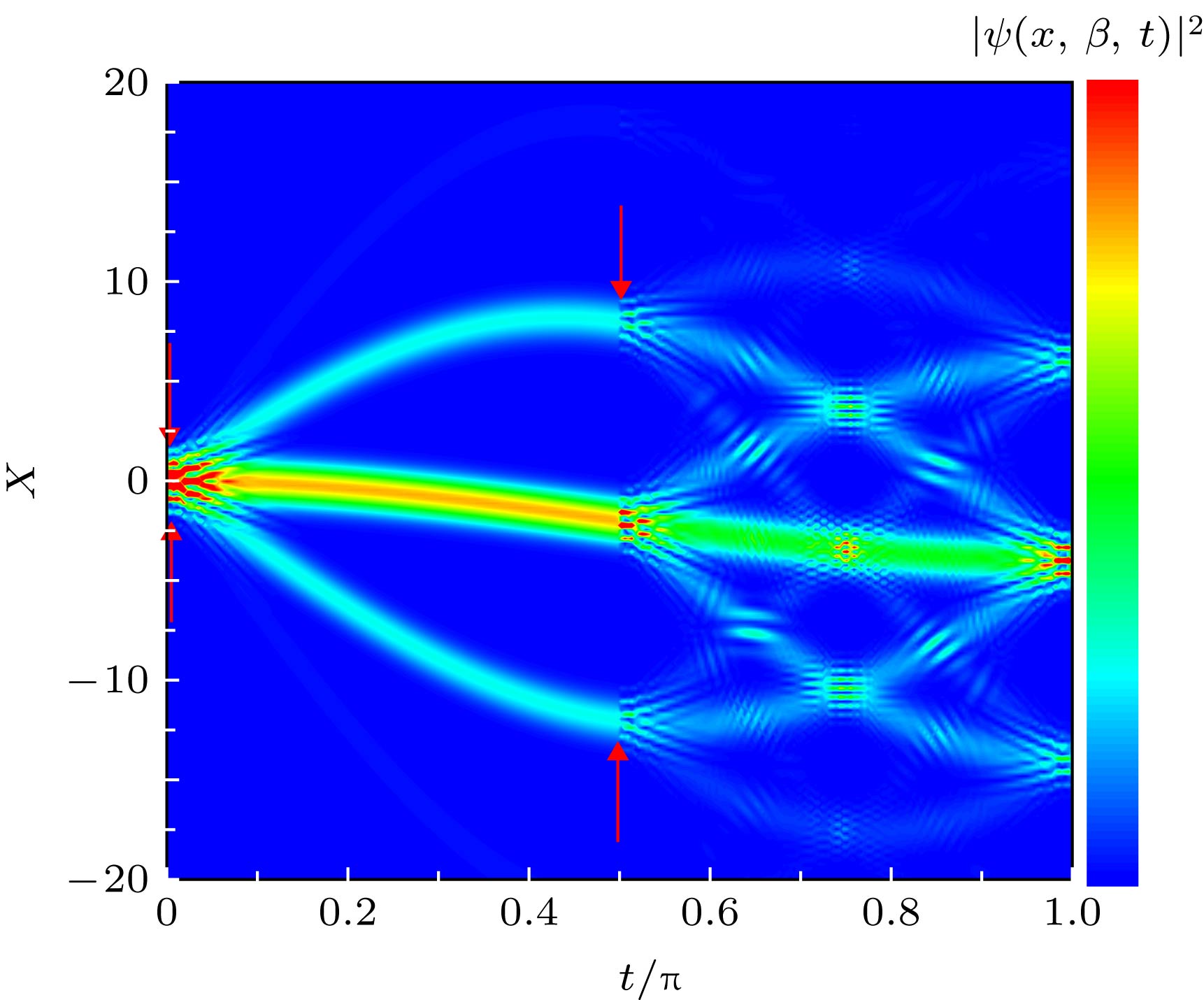
$\beta = 1$ , the evolution of density distribution function of the system is obtained. The dimensionless parameters in this figure are the same as those in Figure 2. The red arrow are KD pulses. The measurement time is at$t = {t_{\rm{f}}} = {\text{π}}$ .
图 4 在外场
$\beta = 2$ 的情况下, 系统态密度分布函数的演化规律 图中除$\beta $ 之外的其他无量纲参数与图2相同, 红色的箭头为KD脉冲, 测量时刻在$t = {t_{\rm{f}}} = {\text{π}}$ Figure 4. In the case of external field
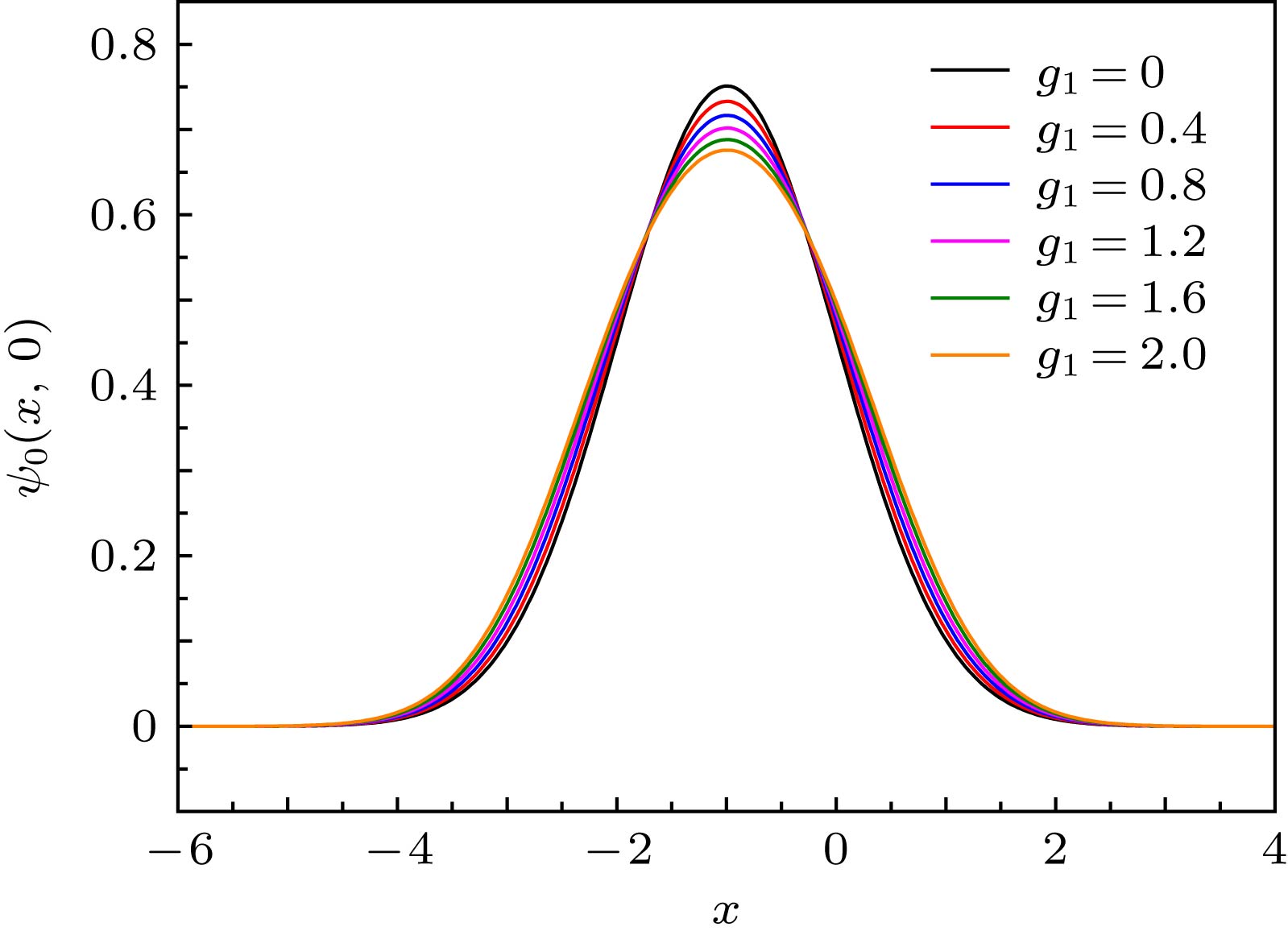
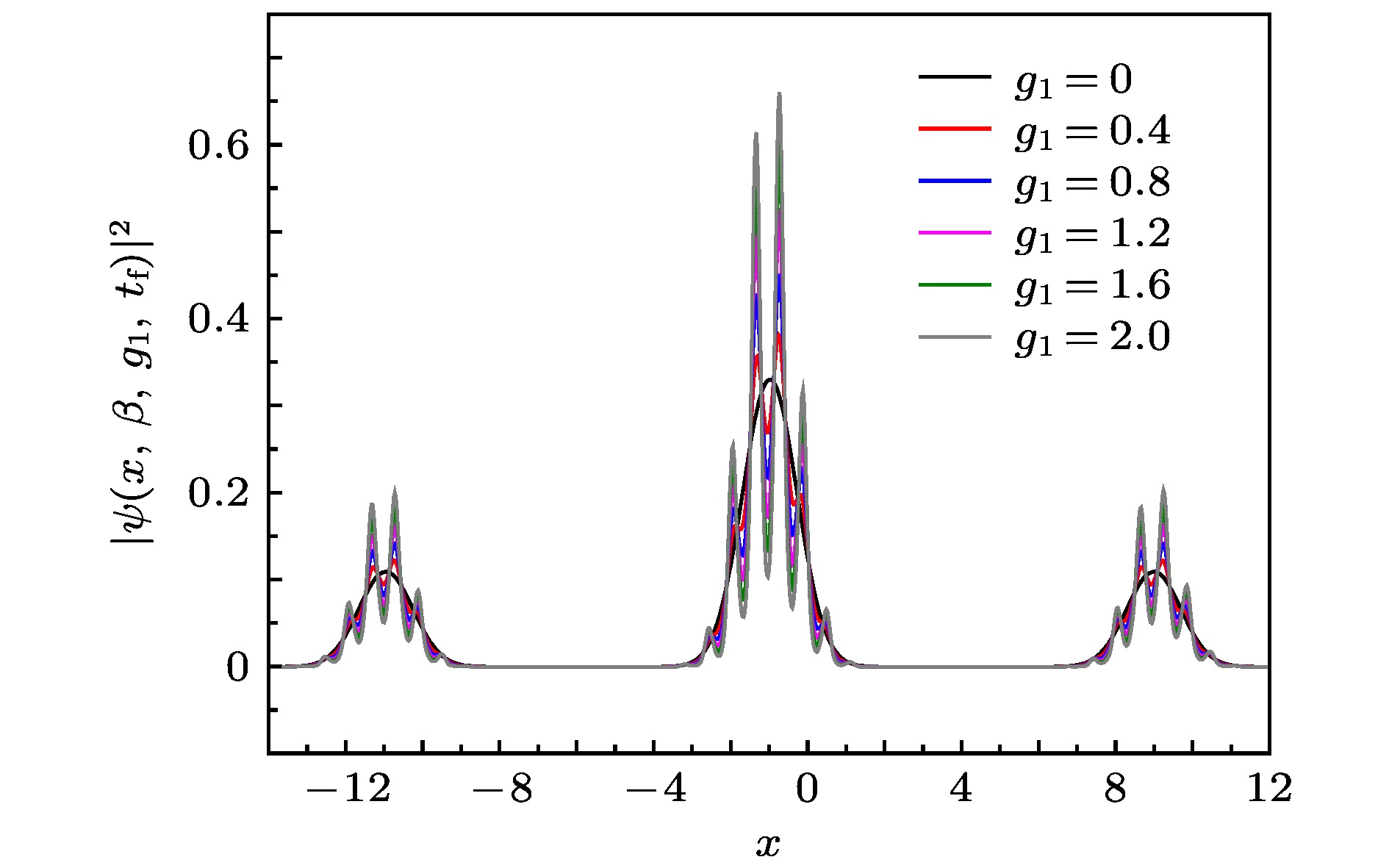
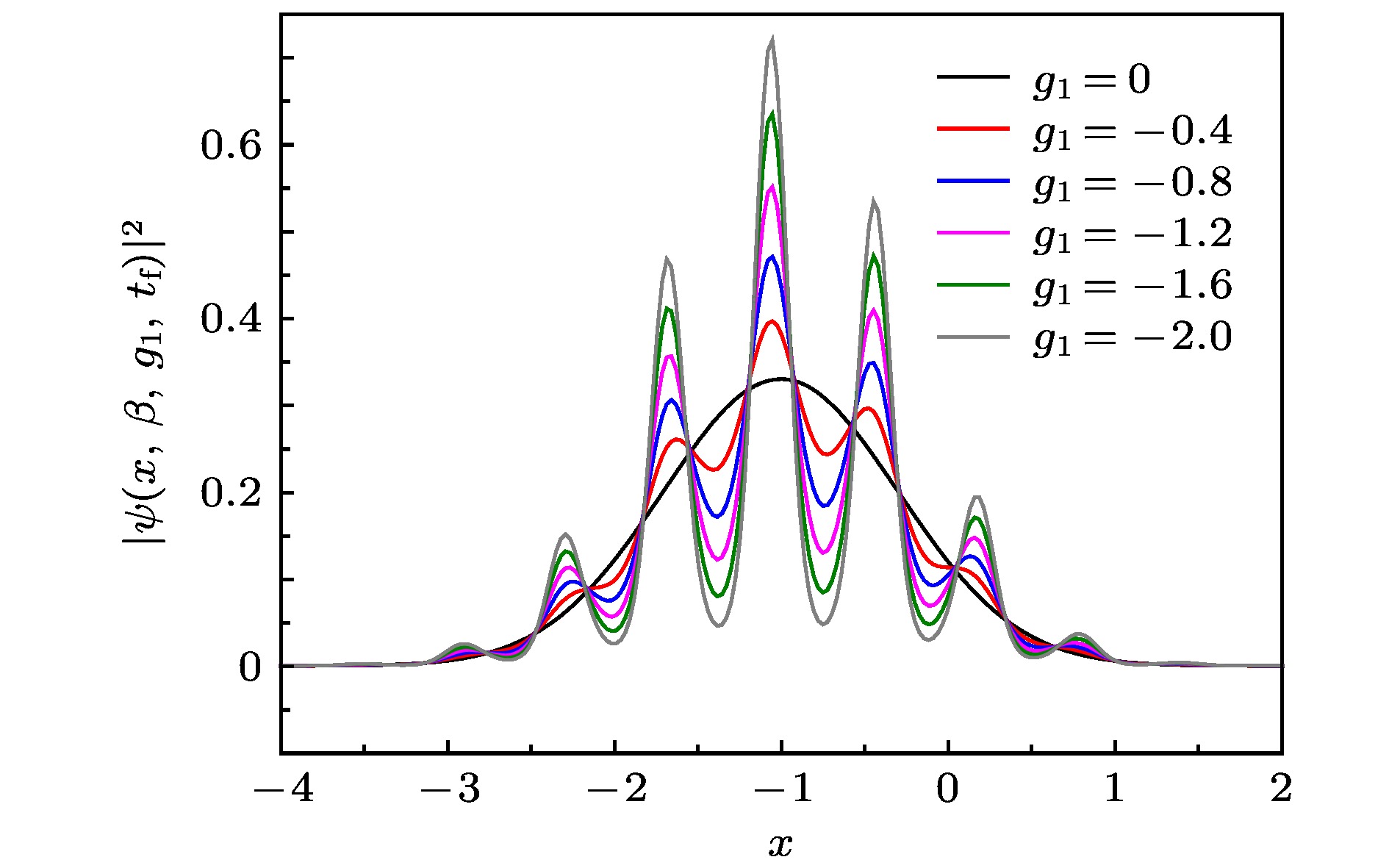
$\beta = 2$ , the evolution of density distribution function of the system is obtained. The dimensionless parameters in this figure are the same as those in Figure 2. The red arrow are KD pulses. The measurement time is at$t = {t_{\rm{f}}} = {\text{π}}$ .
图 6 外场
$\beta = 1$ 的情况下系统基态的态密度分布函数, 不同的颜色对应不同非线性相互作用下的态密度分布函数Figure 6. In the case of external field
$\beta = 1$ , the density distribution function of the ground state of the system. Different colors correspond to the density distribution function under different non-linear interactions.
图 7 在外场
$\beta = 1$ 的情况下系统基态的态密度分布函数, 不同的颜色对应不同非线性相互作用下的态密度分布函数Figure 7. In the case of external field
$\beta = 1$ , the density distribution function of the ground state of the system. Different colors correspond to the density distribution function under different non-linear interactions.
图 8 在
$V = 1,\;k = 10,\; \beta = {\rm{1}}$ 的情况下测量时刻的态密度分布函数, 不同的颜色对应不同非线性相互作用下的态密度分布函数Figure 8. In the case of
$V = 1,\;k = 10,\; \beta = {\rm{1}}$ , the density distribution functions at measuring time. Different colors correspond to the density distribution function under different non-linear interactions.
-
[1] Fang J, Hu J, Chen X, Zhu H, Zhou L, Zhong J, Wang J, Zhan M 2018 Opt. Express 26 1586
Google Scholar
[2] Lu S B, Yao Z W, Li R B, Luo J, Barthwal S, Chen H H, Lu Z X, Wang J, Zhan M S 2018 Opt. Commun. 429 158
Google Scholar
[3] Fang B, Mielec N, Savoie D, Altorio M, Landragin A, Geiger R 2018 New J. Phys. 20 023020
Google Scholar
[4] Jaffe M, Xu V, Haslinger P, Müller H, Hamilton P 2018 Phys. Rev. Lett. 121 040402
Google Scholar
[5] Carey M, Saywell J, Elcock D, Belal M, Freegarde T 2019 Phys. Rev. A 99 023631
Google Scholar
[6] Wigley P B, Hardman K S, Freier C, Everitt P J, Legge S, Manju P, Close J D, Robins N P 2019 Phys. Rev. A 99 023615
Google Scholar
[7] Arias A, Lochead G, Wintermantel T M, Helmrich S, Whitlock S 2019 Phys. Rev. Lett. 122 053601
Google Scholar
[8] Zhou L, Xiong Z Y, Yang W, Tang B, Peng W C, Wang Y B, Xu P, Wang J, Zhan M S 2011 Chin. Phys. Lett. 28 013701
Google Scholar
[9] Imanishi Y, Sato T, Higashi T, Sun W, Okubo S 2004 Science 306 476
Google Scholar
[10] Kibble B P 1991 Proc. IEE: Science, Measurement and Technology 138 187
Google Scholar
[11] Blakely R J, Jachens R C 1991 Geological Society of America Bulletin 103 795
Google Scholar
[12] Peters A, Chung K Y, Chu S 2001 Metrologia 38 25
Google Scholar
[13] Rasel E M, Oberthaler M K, Batelaan H, Schmiedmayer J, Zeilinger A 1995 Phys. Rev. Lett. 75 2633
Google Scholar
[14] Giltner D M, McGowan R W, Lee S A 1995 Phys. Rev. Lett. 75 2638
Google Scholar
[15] 吴彬, 程冰, 付志杰, 朱栋, 周寅, 翁堪兴, 王肖隆, 林强 2018 67 190302
Google Scholar
Wu B, Cheng B, Fu Z J, Zhu D, Zhou Y, Wong K X, Wang X L, Lin Q 2018 Acta Phys. Sin. 67 190302
Google Scholar
[16] McGuirk J M, Foster G T, Fixler J B, Snadden M J, Kasevich M A 2002 Phys. Rev. A 65 033608
Google Scholar
[17] Fixler J B, Foster G T, McGuirk J M, Kasevich M A 2007 Science 315 74
Google Scholar
[18] Li W D, He T, Smerzi A 2014 Phys. Rev. Lett. 113 023003
Google Scholar
[19] 杨志安 2013 62 110302
Google Scholar
Yang Z A 2013 Acta Phys. Sin. 62 110302
Google Scholar
[20] He T C, Niu P B 2017 Phys. Lett. A 381 108
[21] Cheng R, He T, Li W, Smerzi A 2016 J. Mod. Phys. 7 2043
Google Scholar
[22] Sapiro R E, Zhang R, Raithel G 2009 Phys. Rev. A 79 043630
Google Scholar
[23] Keller C, Schmiedmayer J, Zeilinger A, Nonn T, Dürr S, Rempe G 1999 Appl. Phys. B: Lasers Opt. 69 303
Google Scholar
[24] Dong G J, Zhu J, Zhang W P, Malomed B A 2013 Phys. Rev. Lett. 110 250401
Google Scholar
[25] Zhu J, Dong G J, Shneider M N, Zhang W P 2011 Phys. Rev. Lett. 106 210403
Google Scholar
[26] Chwedenczuk J, Piazza F, Smerzi A 2013 Phys. Rev. A 87 033607
Google Scholar
[27] Shin Y, Saba M, Schirotzek A, Pasquini T A, Leanhardt A E, Pritchard D E, Ketterle W 2004 Phys. Rev. Lett. 92 150401
Google Scholar
[28] Lehtovaara L, Toivanen J, Eloranta J 2007 J. Comput. Phys. 221 148
Google Scholar
[29] Bandrauk A D, Shen H 1994 J. Phys. A 27 7147
Google Scholar
[30] Fattori M, D’Errico C, Roati G, Zaccanti M, Jona-Lasinio M, Modugno M, Inguscio M, Modugno G 2008 Phys. Rev. Lett. 100 080405
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 8059
- PDF Downloads: 81
- Cited By: 0
