Search

Article

x
中国物理学会期刊
Chinese Physics Letters Chinese Physics B 物理 中国物理学会期刊网
Advance Search

留言板

尊敬的读者、作者、审稿人, 关于本刊的投稿、审稿、编辑和出版的任何问题, 您可以本页添加留言。我们将尽快给您答复。谢谢您的支持!

姓名
邮箱
手机号码
标题
留言内容
验证码

Radiative and Auger transitions of K-shell excited resonance states in boron-like sulfur ion

Sun Yan ,  Hu Feng ,  Sang Cui-Cui ,  Mei Mao-Fei ,  Liu Dong-Dong ,  Gou Bing-Cong

downloadPDF
Citation:
shu
Next Previous

Radiative and Auger transitions of K-shell excited resonance states in boron-like sulfur ion

Sun Yan, Hu Feng, Sang Cui-Cui, Mei Mao-Fei, Liu Dong-Dong, Gou Bing-Cong
Acta Phys. Sin., 2019, 68(16): 163101.
doi: 10.7498/aps.68.20190481
PDF
HTML
Get Citation

  • Abstract

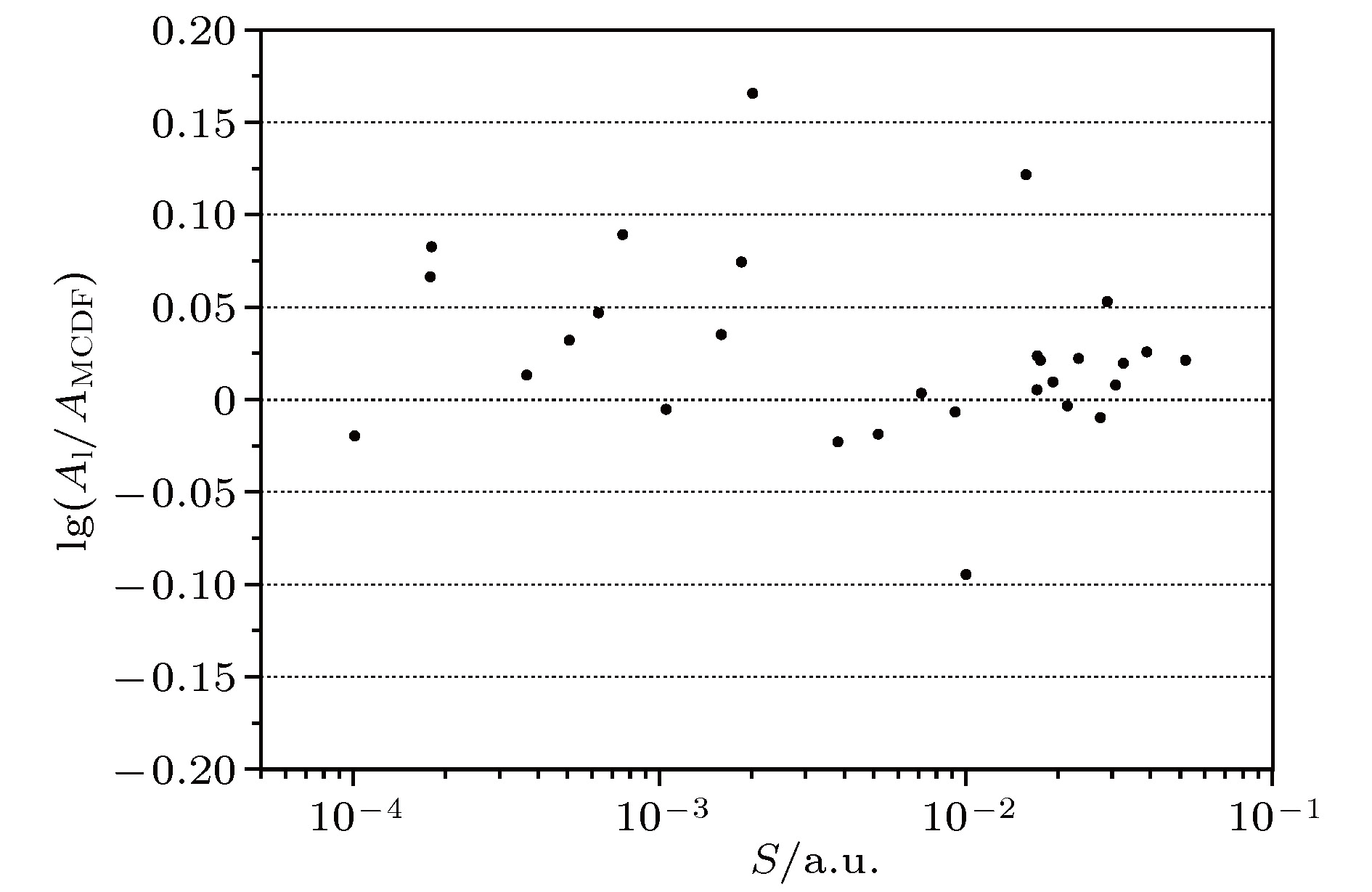
    Non-relativistic energy values and wave functions of the K-shell excited resonance states 1s2s22p2, 1s2s2p3, 1s2p4 2, 4L (L = S, P, D) in boron-like sulfur ion are calculated in the frame of multi-configuration saddle-point variation method. The electron correlation effects are considered by the expansion of configuration wave function. The wave functions are constructed and optimized by the orbital-spin angular momentum partial waves selected based on the rule of configuration interaction. To saturate the wave functional space and to improve the non-relativistic energy, the restricted variational method is used to calculate the restricted variational energy. Then, the mass polarization effect and relativistic correction are calculated by the perturbation theory. The quantum electrodynamics (QED) effect and higher-order relativistic correction are considered by the screened hydrogenic formula. Furthermore, the energy shift originating from the interaction between closed channel and open channel is also calculated. Finally, the accurate relativistic energy levels for these resonance states are obtained by adding the non-relativistic energy and all corrections.Using the optimized wave functions, the line strengths, oscillator strengths, radiative transition rates and transition wavelengths of electric-dipole transitions for the K-shell excited resonance states in boron-like sulfur ion are systematically calculated. In this work, the oscillator strengths and transition rates are given in the length, velocity, and acceleration gauges. The good agreement among the three gauges reflects that the calculated wave functions are reasonably accurate. The calculated radiative transition rates and transition wavelengths are compared with other theoretical data. Good agreement is obtained except the transition: 1s2s(3S)2p3 2Po→1s22s2p2 2D. The deviation between our theoretical result and the MCDF theoretical value is about 46%, which needs further verifying. The Auger rates, Auger branching ratios, and Auger electron energy values of the important decay channels of the K-shell excited states are calculated by the saddle-point complex-rotation method. The calculated Auger rates and Auger electron energy values are also in good agreement with the corresponding reference data. For some K-shell states, the related energy levels and Auger branching ratios are reported for the first time. The present calculations results will provide valuable theoretical data for the calibration of spectral lines and Auger electron spectra in the relevant experiments.

    Authors and contacts

    • 1.

      School of Mathematic and Physical Science, Xuzhou University of Technology, Xuzhou 221018, China

    • 2.

      College of Science, Lanzhou University of Technology, Lanzhou 730050, China

    • 3.

      School of Physics, Beijing institute of Technology, Beijing 100081, China

      • Corresponding author: Sun Yan, suenyangu@163.com
    • Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 11604284, 51506184), the Natural Science Foundation of the Jiangsu Higher Education Institutions of China (Grant No. 17KJB140025), and Sun Yan is supported by the Qinlan Project of Jiangsu Province, China.

    References

    [1]

    Altun Z, Yumak A, Badnell N R, Colgan J, Pindzol M S 2004 A&A 420 775
    [2]

    Rohringer N, Ryan D, London R A, Purvis M, Albert F, Dunn J, Bozek J D, Bostedt C, Graf A, Hill R, Hau-Riege S P, Rocca J J 2012 Nature 481 488Google Scholar

    [3]

    Martin A, Nicolas G, Jacques S 2006 Nucl. Phys. A 777 1
    [4]

    冯雷, 蒋刚 2017 66 153201Google Scholar

    Feng L, Jiang G 2017 Acta Phys. Sin. 66 153201Google Scholar

    [5]

    Rødbro M, Bruch R, Bisgaard P 1979 J. Phys. B: At. Mol. Opt. Phys. 12 2413Google Scholar

    [6]

    Schneider D, Bruch R, Butscher W, Schwarz W H E 1981 Phys. Rev. A 24 1223Google Scholar

    [7]

    Bruch R, Schneider D, Schwarz W H E, Meinhart M, Johnson B M, Taulbjerg K 1979 Phys. Rev. A 19 587Google Scholar

    [8]

    Itoh A, Schneider D, Schneider T, Zouros T J M, Nolte G, Schiwietz G, Zeitz W, Stolterfoht N 1985 Phys. Rev. A 31 684Google Scholar

    [9]

    Kádár I, Ricz S, Végh J, Sulik B, Varga D, Berényi D 1990 Phys. Rev. A 41 3518Google Scholar

    [10]

    Armour I A, Fawcett B C, Silver J D, Trabert E 1980 J. Phys. B: Atom. Molec. Phys. 13 2701Google Scholar

    [11]

    Trabertt E, Fawcett B C 1979 J. Phys. B: Atom. Molec. Phys. 12 L441
    [12]

    Faenov A Y 1994 Phys. Scr. 49 41Google Scholar

    [13]

    Schlachter A S, Sant’Anna M M, Covington A M, Aguilar A, Gharaibeh M F, Emmons E D, Scully S W J, Phaneuf R A, Hinojosa G, Álvarez I, Cisneros C, Müller A, McLaughlin B M 2004 J. Phys. B: At. Mol. Opt. Phys. 37 L103Google Scholar

    [14]

    Gharaibeh M F, Hassan N E I, Shorman M M A L, Bizau J M, Cubaynes D, Guilbaud S, Sakho I, Blancard C, McLaughlin B M 2014 J. Phys. B: At. Mol. Opt. Phys. 47 065201Google Scholar

    [15]

    Müller A, Borovik A, Buhr T, Hellhund J, Holste K, Kilcoyne A L D, Klumpp S, Martins M, Ricz S, Viefhaus J, Schippers S 2018 Phys. Rev. A 97 013409Google Scholar

    [16]

    Müller A, Borovik A, Buhr J T, Hellhund J, Holste K, Kilcoyne A L D, Klumpp S, Martins M, Ricz S, Viefhaus J, Schippers S 2015 Phys. Rev. Lett. 114 013002Google Scholar

    [17]

    Chen M H, Crasemann B 1988 At. Data Nucl. Data Tables 38 381Google Scholar

    [18]

    Chen M H, Crasemann B 1987 Phys. Rev. A 35 4579Google Scholar

    [19]

    Safronova U I, Shlyaptseva A S 1996 Phys. Scr. 54 254Google Scholar

    [20]

    Zhou F Y, Ma Y L, Qu Y Z 2016 Phys. Rev. A 93 060501Google Scholar

    [21]

    Sakho I, Sow M, Wagué A 2015 Phys. Scr. 90 045401Google Scholar

    [22]

    Sun Y, Chen F, Gou B C 2011 J. Chem. Phys. 135 124309Google Scholar

    [23]

    Sun Y, Gou B C, Chen C 2013 Phys. Rev. A 87 032509Google Scholar

    [24]

    Chung K T 1979 Phys. Rev. A 20 1743Google Scholar

    [25]

    Lin B, Berry H G, Shibata T, Livingston A E, Garnir H P, Bastin T, Désesquelles J, Savukov I 2003 J. Phys. B: At. Mol. Opt. Phys. 67 062507Google Scholar

    [26]

    Drake G W F 1982 Adv. At. Mol. Phys. 18 399Google Scholar

    [27]

    Chung K T, Zhu X W, Wang Z W 1993 Phys. Rev. A 47 1740Google Scholar

    [28]

    Drake G W F, Swainson R A 1990 Phys. Rev. A 41 1243Google Scholar

    [29]

    Chung K T, Davis B F 1982 Phys. Rev. A 26 3278Google Scholar

    [30]

    Davis B F, Chung K T 1984 Phys. Rev. A 29 1878Google Scholar

    [31]

    Kramida A, Ralchenko Yu, Reader J, NIST ASD Team 2018 NIST Atomic Spectra Database (ver. 5.6.1) [Online]. Available: https://physics.nist.gov/asd [2019, March 28]. National Institute of Standards and Technology, Gaithersburg, MD. DOI: https://doi.org/10.18434/T4W30F

  • 图 1  本文计算的电偶极跃迁振子强度的长度规范值分别与速度规范值及加速度规范值的对比

    Figure 1.  Comparison diagram of the calculated electrical dipole transition oscillator strength values in length gauge with the velocity gauge and acceleration gauge.

    DownLoad: Full-Size Img PowerPoint

    图 2  本文计算的长度规范的电偶极辐射跃迁率与MCDF理论计算的跃迁率的对比

    Figure 2.  Comparison diagram of calculated radiative transition rates in length gauge with the theoretical data from MCDF calculations.

    DownLoad: Full-Size Img PowerPoint

    表 1  类硼S离子K壳层激发共振态1s2s22p2, 1s2s2p3, 1s2p4 2, 4L (L = S, P, D)的权重中心能级(单位a.u.), 能量转化关系:1 a.u = 27.21138 eV

    Table 1.  Center of gravity levels of 1s2s22p2, 1s2s2p3, 1s2p4 2, 4L (L = S, P, D) of K-shell excited resonance states in boron-like sulfur ion (unit: a.u.). The energy conversion relationship: 1 a.u = 27.21138 eV.

    共振态${E_{{\rm{nonrel}}}}/{\rm{a.u.}}$${E_{{\rm{total}}}}/{\rm{a.u.}}$$ - {E_{{\rm{total}}}}/{\rm{eV}}$
    ${E_{\rm{b}}} + \Delta {E_{{\rm{RV}}}}$$\Delta {E_{{\rm{corr}}}}$$\Delta {E_{\rm{S}}}$本文SCUNC[21]
    1s2s22p2 4P–229.35389–0.64011–0.00245–229.996456258.52
    1s2s22p2 2S–228.66774–0.66777–0.00174–229.337256240.58
    1s2s22p2 2P–228.81110–0.66633–0.00089–229.478326244.42
    1s2s22p2 2D–228.91613–0.679420.00322–229.592336247.52
    1s2s(3S)2p3 4So–227.40768–0.608230.00018–228.015736204.62
    1s2s(1S)2p3 4So–228.21123–0.622980.00151–228.832706226.85
    1s2s(3S)2p3 4Po–228.00558–0.622150.00106–228.626676221.25
    1s2s(3S)2p3 4Do–228.30315–0.62288–0.00017–228.926206229.40
    1s2s(3S)2p3 2So–226.86291–0.625860.00070–227.488076190.26
    1s2s(3S)2p3 2Po–226.91669–0.611140.00193–227.525906191.29
    1s2s(1S)2p3 2Po–227.28245–0.616460.00620–227.892716201.28
    1s2s(3S)2p3 2Do–227.21472–0.613300.00204–227.825986199.46
    1s2s(1S)2p3 2Do–227.56290–0.620410.00280–228.180516209.11
    1s2p4 4P–226.53817–0.55727–0.00249–227.097936179.656173.07
    1s2p4 2S–225.47488–0.562200.00072–226.036366150.766145.67
    1s2p4 2P–225.94003–0.564930.00251–226.502456163.446159.02
    1s2p4 2D–226.07283–0.560740.00279–226.630786166.946163.51
    DownLoad: CSV

    表 2  S11+离子K壳层激发共振态, S11+, S12+离子低位激发态的精细结构能级($ - E$, 单位eV)

    Table 2.  Fine-structure energy levels of the K-shell excited resonance states in S11+ ion, and low-excited states in S11+, S12+ ion ($ - E$, unit eV).

    偶宇称奇宇称
    S11+离子K壳层激发态共振态
    共振态本文文献[19]共振态本文文献[19]
    1s2s22p2 4P1/26259.506265.621s2s(3S)2p3 4S3/26204.626207.16
    1s2s22p2 4P3/26258.836264.851s2s(1S)2p3 4S3/26226.856229.68
    1s2s22p2 4P5/26257.996264.051s2s(3S)2p3 4P1/26221.056223.62
    1s2s22p2 2S1/26240.586243.031s2s(3S)2p3 4P3/26221.246223.52
    1s2s22p2 2P1/26245.726248.941s2s(3S)2p3 4P5/26221.326223.54
    1s2s22p2 2P3/26243.776247.231s2s(3S)2p3 4D1/26229.216231.96
    1s2s22p2 2D3/26247.386251.291s2s(3S)2p3 4D3/26229.216232.01
    1s2s22p2 2D5/26247.626251.381s2s(3S)2p3 4D5/26229.306232.00
    1s2p4 4P1/26178.536180.771s2s(3S)2p3 4D7/26229.616231.85
    1s2p4 4P3/26179.126181.261s2s(3S)2p3 2S1/26190.266192.27
    1s2p4 4P5/26180.376182.441s2s(3S)2p3 2P1/26191.176193.98
    1s2p4 2S1/26150.766152.751s2s(3S)2p3 2P3/26191.366193.65
    1s2p4 2P1/26163.286165.341s2s(1S)2p3 2P1/26201.726204.18
    1s2p4 2P3/26163.526166.421s2s(1S)2p3 2P3/26201.056206.82
    1s2p4 2D3/26166.836169.561s2s(3S)2p3 2D3/26199.296199.03
    1s2p4 2D5/26167.006169.691s2s(3S)2p3 2D5/26199.576202.14
    1s2s(1S)2p3 2D3/26209.236212.93
    1s2s(1S)2p3 2D5/26209.036212.33
    S11+离子低位激发态
    激发态本文NIST[31]激发态本文NIST[31]
    1s22s2p2 4P1/28617.388617.291s22s22p 2P1/28641.588641.33
    1s22s2p2 4P3/28616.838616.701s22s22p 2P3/28639.788639.70
    1s22s2p2 4P5/28615.988615.861s22p3 4S3/28565.718565.69
    1s22s2p2 2S1/28586.788586.831s22p3 2P1/28545.448545.36
    1s22s2p2 2P1/28584.068583.711s22p3 2P3/28545.428545.14
    1s22s2p2 2P3/28582.998582.881s22p3 2D3/28555.978555.79
    1s22s2p2 2D3/28598.498598.351s22p3 2D5/28555.748555.72
    1s22s2p2 2D5/28598.398598.31
    S12+离子低位激发态
    激发态本文NIST[31]激发态本文NIST[31]
    1s22s2 1S08076.998076.931s22s2p 1P18028.748028.63
    1s22p2 1S07987.617987.441s22s2p 3P08052.368052.23
    1s22p2 1D28004.128003.851s22s2p 3P18051.818051.70
    1s22p2 3P08012.118012.061s22s2p 3P28050.648050.50
    1s22p2 3P18011.538011.37
    1s22p2 3P28010.438010.37
    DownLoad: CSV

    表 3  类硼S离子的K壳层激发共振态1s2s22p2, 1s2s2p3, 1s2p4 2, 4L(L = S, P, D)的电偶极辐射跃迁线强度S (a.u.)、辐射跃迁率${A_{ik}}$(s–1) (长度规范${A_{\rm{l}}}$, 速度规范${A_{\rm{v}}}$, 加速度规范${A_{\rm{a}}}$), 跃迁振子强度${f_{ki}}$(长度规范${f_{\rm{l}}}$, 速度规范${f_{\rm{v}}}$, 加速度规范${f_{\rm{a}}}$), 和跃迁波长$\lambda $(Å), 方括号的数代表10的幂次方

    Table 3.  Line strengths S (a.u.), radiative transition probabilities ${A_{ik}}$ (length gauge ${A_{\rm{l}}}$, velocity gauge${A_{\rm{v}}}$, acceleration gauge ${A_{\rm{a}}}$) (s–1), transition oscillator strengths ${f_{ki}}$ (length gauge ${f_{\rm{l}}}$, velocity gauge ${f_{\rm{v}}}$, and acceleration gauge ${f_{\rm{a}}}$), and transition wavelengths $\lambda $ (Å) of electric dipole transitions of the K-shell excited resonance states 1s2s22p2, 1s2s2p3, 1s2p4 2, 4L (L = S, P, D) in boron-like sulfur ion. The numbers in square brackets represent the power of 10.

    初态末态S/a.u.${A_{ik}}/{\rm{s}}^{-1}$${f_{ki}}$λ/Å
    ${A_{\rm{l}}}$${A_{\rm{v}}}$${A_{\rm{a}}}$文献[17]${f_{\rm{l}}}$${f_{\rm{v}}}$${f_{\rm{a}}}$本文文献[17]文献[21]
    1s2s22p2 4P1s22p3 4So5.06[–4]5.48[11]5.32[11]5.31[11]5.09[11]7.14[–3]6.94[–3]6.92[–3]5.3745.379
    1s2p4 4P1s22p3 4So2.14[–2]2.55[13]2.65[13]2.67[13]2.57[13]3.12[–1]3.23 [–1]3.26[–1]5.1965.1935.203
    1s2s22p2 2S1s22s22p 2Po3.81[–3]2.78[13]2.82[13]2.80[13]2.93[13]3.72[–2]3.78[–2]3.76[–2]5.1665.1765.167
    1s22p3 2Po1.78[–4]1.15[12]1.15[12]1.02[12]9.87[11]1.68[–3]1.67[–3]1.48[–3]5.3795.383
    1s2s22p2 2P1s22s22p 2Po3.26[–2]7.88[13]7.87[13]7.84[13]7.53[13]3.18[–1]3.18[–1]3.17[–1]5.1755.176
    1s22p3 2Po1.80[–4]3.87[11]3.88[11]3.99[12]3.20[11]1.69[–3]1.69[–3]1.74[–3]5.3885.392
    1s22p3 2Do6.30[–4]1.37[12]1.39[12]1.40[12]1.23[12]3.56[–3]3.61[–3]3.64[–3]5.3645.368
    1s2s22p2 2D1s22s22p2Po1.92[–2]2.77[13]2.67[13]2.64[13]2.71[13]1.87[–1]1.80[–1]1.78[–1]5.1815.183
    1s22p3 2Po1.01[–4]1.29[11]1.28[11]1.45[11]1.35[11]9.43[–4]9.34[–4]1.06[–3]5.3965.401
    1s22p3 2Do3.68[–4]7.98[11]8.18[11]8.25[11]7.74[11]3.46[–3]3.55[–3]3.59[–3]5.3715.375
    1s2p4 2S1s22p3 2Po7.14[–3]5.17[13]5.26[13]5.28[13]5.13[13]6.96[–2]7.08[–2]7.11[–2]5.1785.1755.191
    1s2p4 2P1s22p3 2Po1.75[–2]4.17[13]4.26[13]4.26[13]3.97[13]1.70[–1]1.74[–1]1.73[–1]5.2055.2055.217
    1s22p3 2Do2.89[–2]6.97[13]6.80[13]6.71[13]6.17[13]1.69[–1]1.65[–1]1.63[–1]5.1825.1825.191
    1s2p4 2D1s22s22p 2Po2.58[–4]4.09[11]4.42[11]4.14[11]2.59[–3]2.80[–3]2.62[–3]5.013
    1s22p3 2Po9.20[–3]1.30[13]1.32[13]1.33[13]1.32[13]8.91[–2]9.04[–2]9.11[–2]5.2135.2125.220
    1s22p3 2Do2.74[–2]3.93[13]4.10[13]4.13[13]4.02[13]1.60[–1]1.67[–1]1.68[–1]5.1905.1895.198
    1s2s(1S)2p3 4So1s22s2p2 4P3.08[–2]1.11[14]1.09[14]1.09[14]1.09[14]1.50[–1]1.48[–1]1.47[–1]5.1885.189
    1s2s(3S)2p3 4So1s22s2p2 4P7.57[–4]2.80[12]2.90[12]2.99[12]2.28[12]3.72[–3]3.85[–3]3.97[–3]5.1415.135
    1s2s(3S)2p3 4Po1s22s2p2 4P2.33[–2]2.81[13]2.79[13]2.80[13]2.67[13]1.14[–1]1.13[–1]1.13 [–1]5.1765.174
    1s2s(3S)2p3 4Do1s22s2p2 4P3.89[–2]2.79[13]2.78[13]2.77[13]2.63[13]1.89[–1]1.88[–1]1.88[–1]5.1945.192
    1s2s(3S)2p3 2So1s22s2p2 2P1.57[–2]1.13[14]1.13[14]1.13[14]8.54[13]1.53[–1]1.52[–1]1.52[–1]5.1815.180
    1s2s(1S)2p3 2Po1s22s2p2 2P1.05[–3]2.50[12]2.78[12]2.74[12]2.53[12]1.02[–2]1.13[–2]1.12[–2]5.2055.208
    1s22s2p2 2D1.71[–2]4.14[13]4.17[13]4.14[13]3.92[13]1.00[–1]1.01[–1]1.00[–1]5.1725.173
    1s2s(3S)2p3 2Po1s22s2p2 2S1.59[–3]3.85[12]3.93[12]3.50[12]3.55[12]4.66[–2]4.76[–2]4.24[–2]5.1765.174
    1s22s2p2 2P1.00[–2]2.42[13]2.42[13]2.30[13]3.01[13]9.79[–2]9.79[–2]9.31[–2]5.1835.183
    1s22s2p2 2D2.01[–3]4.95[12]4.92[12]5.19[12]3.38[12]1.18[–2]1.19[–2]1.25[–2]5.1515.149
    1s2s(1S)2p3 2Do1s22s2p2 2P1.85[–3]2.60[12]2.64[12]2.64[12]2.19[12]1.79[–2]1.81[–2]1.81[–2]5.2225.225
    1s22s2p2 2D5.21[–2]7.49[13]7.54[13]7.55[13]7.13[13]3.04[–1]3.06[–1]3.07[–1]5.1895.191
    1s2s(3S)2p3 2Do1s22s2p2 2P1.70[–2]2.43[13]2.49[13]2.50[13]2.40[13]1.65[–1]1.69[–1]1.70[–1]5.2015.201
    1s22s2p2 2D5.16[–3]7.51[12]7.72[12]7.83[12]7.84[12]3.02[–2]3.11[–2]3.15[–2]5.1685.166
    DownLoad: CSV

    表 4  类硼S离子K壳层激发态1s2s22p2, 1s2s2p3, 1s2p4 2, 4L(L = S, P, D)的俄歇跃迁率(s–1) 和俄歇分支率(BR), 方括号的数表示10的幂次方

    Table 4.  The Auger rates (s–1) and branching ratios (BR) of the K-shell excited resonance states 1s2s22p2, 1s2s2p3, 1s2p4 2, 4L (L=S, P, D) in boron-like sulfur ion. The numbers in square brackets represent the power of 10.

    俄歇跃迁通道俄歇跃迁率/s–1BR/%俄歇跃迁通道俄歇跃迁率/s–1BR(%)
    本文文献[17]本文文献[17]
    1s2s22p22S →1s22s2 1S5.05[13]8.33[13]23.31s2s(1S)2p3 2Po→1s22s2 1S6.63[11]2.33[11]0.3
    2S →1s22s2p 1Po6.35[13]6.02[13]29.32Po→1s22s2p 1Po1.18[12]1.46[13]0.5
    2S →1s22s2p 3Po1.61[13]2.06[13]7.42Po→1s22s2p 3Po1.29[14]1.22[14]59.6
    2S →1s22p2 1S7.10[13]7.85[13]32.82Po→1s22p2 1S6.50[12]5.20[12]3.0
    2S →1s22p2 1D1.53[13]1.48[13]7.12Po→1s22p2 1D5.25[12]9.54[12]2.4
    2P→1s22s2p 1Po1.82[13]2.55[13]14.72Po→1s22p2 3P7.40[13]7.54[13]34.2
    2P→1s22s2p 3Po1.05[13]7.34[12]8.52Do→1s22s2p 1Po7.30[12]8.82[12]2.8
    2P→1s22p2 1D21.97[10]7.42[12]02Do→1s22s2p 3Po1.74[14]1.74[14]66.1
    2P→1s22p2 3P9.50[13]8.68[13]76.82Do→1s22p2 1D9.55[12]1.40[13]3.6
    2D→1s22s2 1S1.24[14]1.14[14]40.32Do→1s22p2 3P7.25[13]7.66[13]27.5
    2D→1s22s2p 1Po6.80[13]6.42[13]22.14So→1s22p2 3P3.85[13]3.88[13]100
    2D→1s22s2p 3Po1.72[13]2.26[13]5.61s2s(3S)2p32So→1s22p2 3P6.55[13]4.35[13]100
    2D→1s22p2 1S3.43[12]2.82[12]1.12Po→1s22s2 1S4.33[12]2.67[12]1.6
    2D→1s22p2 1D9.15[13]9.22[13]29.82Po→1s22s2p 1Po1.28[14]1.18[14]46.9
    2D→1s22p2 3P3.37[12]4.47[12]1.12Po→1s22s2p 3Po5.40[12]7.38[12]2.0
    4P→1s22s2p 3Po1.10[14]1.18[14]54.32Po→1s22p2 1S4.54[13]4.68[13]16.6
    4P→1s22p2 3P9.25[13]9.48[13]45.72Po→1s22p2 1D6.40[13]6.23[13]23.4
    1s2p42S →1s22s2 1S2.75[12]3.04[11]0.62Po→1s22p2 3P2.58[13]3.47[13]9.5
    2S →1s22s2p 1Po4.15[12]4.73[12]1.02Do→1s22s2p 1Po1.76[14]1.71[14]54.5
    2S →1s22s2p 3Po8.57[11]1.48[12]0.22Do→1s22s2p 3Po6.85[12]1.16[13]2.1
    2S →1s22p2 1S2.43[14]3.66[13]56.32Do→1s22p2 1D1.18[14]1.19[14]36.5
    2S →1s22p2 1D1.81[14]1.87[14]41.92Do→1s22p2 3P2.22[13]1.99[13]6.9
    2P→1s22s2p 1Po2.73[11]5.19[11]0.14So →1s22p2 3P1.92[14]2.02[14]100
    2P→1s22s2p 3Po2.38[11]1.86[11]0.14Po→1s22s2p 3Po1.35[14]1.38[14]88.9
    2P→1s22p2 1D26.95[10]2.06[13]04Po→1s22p2 3P1.68[13]1.45[13]11.1
    2P→1s22p2 3P2.15[14]1.90[14]99.84Do→1s22s2p 3Po1.84[14]1.84[14]90.4
    2D→1s22s2 1S2.95[12]7.35[9]1.04Do→1s22p2 3P1.96[13]1.41[13]9.6
    2D→1s22s2p 1Po1.35[12]1.38[12]0.5
    2D→1s22s2p 3Po2.73[11]4.54[11]0.1
    2D→1s22p2 1S1.25[13]4.09[13]4.2
    2D→1s22p2 1D2.68[14]2.74[14]90.6
    2D→1s22p2 3P1.05[13]1.26[13]3.6
    4P→1s22s2p 3Po1.96[12]2.50[12]0.9
    4P→1s22p2 3P2.08[14]2.09[14]99.1
    DownLoad: CSV

    表 5  类硼S离子K壳层激发态1s2s22p2, 1s2s2p3, 1s2p4 2, 4L(L = S, P, D)的俄歇电子能量(单位: eV)

    Table 5.  The Auger electron energies of the K-shell excited resonance states 1s2s22p2, 1s2s2p3, 1s2p4 2, 4L (L = S, P, D) in boron-like sulfur ion (unit: eV).

    跃迁通道本文文献[17]跃迁通道本文文献[17]
    1s2s22p2 2S1/21s22s2 1S01836.411837.801s2s(3S)2p3 2S1/21s22p2 3P01821.851825.18
    1s22s2p 1P11788.161787.751s22p2 3P11821.271824.50
    1s22s2p 3P01811.781812.971s22p2 3P21820.171823.49
    1s22s2p 3P11811.231812.441s2s(3S)2p3 2P1/21s22s2 1S01885.821888.35
    1s22s2p 3P21810.061811.231s22s2p 1P01837.571838.30
    1s22p2 1S01747.031746.351s22s2p 3P11861.191863.52
    1s22p2 1D21763.541763.331s22s2p 3P21860.641862.99
    1s2s22p2 2P1/21s22s2p 1P11783.021782.251s22s2p 3P31859.471861.78
    1s22s2p 3P01806.641807.471s22p2 1S01796.441796.90
    1s22s2p 3P11806.091806.941s22p2 1D21812.951813.88
    1s22s2p 3P21804.921805.741s22p2 3P01820.941823.09
    1s22p2 1D21758.401757.841s22p2 3P11820.361822.41
    1s22p2 3P01766.391767.051s22p2 3P21819.261821.40
    1s22p2 3P11765.811766.371s2s(3S)2p3 2P3/21s22s2 1S01885.631888.87
    1s22p2 3P21764.711765.361s22s2p 1P01837.381838.83
    1s2s22p2 2P3/21s22s2p 1P11784.971784.031s22s2p 3P11861.001864.05
    1s22s2p 3P01808.591809.251s22s2p 3P21860.451863.51
    1s22s2p 3P11808.041808.721s22s2p 3P31859.281862.31
    1s22s2p 3P21806.871807.521s22p2 1S01796.251797.43
    1s22p2 1D21760.351759.621s22p2 1D21812.761814.41
    1s22p2 3P01768.341768.831s22p2 3P01820.751823.62
    1s22p2 3P11767.761768.151s22p2 3P11820.171822.94
    1s22p2 3P21766.661767.141s22p2 3P21819.071821.93
    1s2s22p2 2D3/21s22s2 1S01829.611830.381s2s(1S)2p3 2P1/21s22s2 1S01875.271877.33
    1s22s2p 1P11781.361780.341s22s2p 1P01827.021827.28
    1s22s2p 3P01804.981805.561s22s2p 3P11850.641852.50
    1s22s2p 3P11804.431805.021s22s2p 3P21850.091851.97
    1s22s2p 3P21803.261803.821s22s2p 3P31848.921850.77
    1s22p2 1S01740.231738.941s22p2 1S01785.891785.88
    1s22p2 1D21756.741755.921s22p2 1D21802.401802.87
    1s22p2 3P01764.731765.131s22p2 3P01810.391812.08
    1s22p2 3P11764.151764.451s22p2 3P11809.811811.40
    1s22p2 3P21763.051763.441s22p2 3P21808.711810.39
    1s2s22p2 2D5/21s22s2 1S01829.371830.331s2s(1S)2p3 2P3/21s22s2 1S01875.941877.26
    1s22s2p 1P11781.121780.291s22s2p 1P01827.691827.21
    1s22s2p 3P01804.741805.511s22s2p 3P11851.311852.43
    1s22s2p 3P11804.191804.971s22s2p 3P21850.761851.90
    1s22s2p 3P21803.021803.771s22s2p 3P31849.591850.70
    1s22p2 1S01739.991738.891s22p2 1S01786.561785.81
    1s22p2 1D21756.501755.871s22p2 1D21803.071802.80
    1s22p2 3P01764.491765.081s22p2 3P01811.061812.01
    1s22p2 3P11763.911764.401s22p2 3P11810.481811.33
    1s22p2 3P21762.811763.391s22p2 3P21809.381810.32
    1s2p4 2S1/21s22s2 1S01926.231930.571s2s(3S)2p3 2D3/21s22s2p 1P01829.451831.14
    1s22s2p 1P11877.981880.521s22s2p 3P01853.071856.36
    1s22s2p 3P01901.601905.751s22s2p 3P11852.521855.83
    1s22s2p 3P11901.051905.211s22s2p 3P21851.351854.62
    1s22s2p 3P21899.881904.011s22p2 1D21804.831806.72
    1s22p2 1S01836.851839.131s22p2 3P01812.821815.93
    1s22p2 1D21853.361856.111s22p2 3P11812.241815.25
    1s2p4 2P1/21s22s2p 1P11865.461867.171s22p2 3P21811.141814.24
    1s22s2p 3P01889.081892.391s2s(3S)2p3 2D5/21s22s2p 1P01829.171830.60
    1s22s2p 3P11888.531891.861s22s2p 3P01852.791855.82
    1s22s2p 3P21887.361890.661s22s2p 3P11852.241855.28
    1s22p2 1D21840.841842.761s22s2p 3P21851.071854.08
    1s22p2 3P01848.831851.971s22p2 1D21804.551806.18
    1s22p2 3P11848.251851.291s22p2 3P01812.541815.39
    1s22p2 3P21847.151850.281s22p2 3P11811.961814.71
    1s2p4 2P3/21s22s2p 1P11865.221866.271s22p2 3P21810.861813.70
    1s22s2p 3P01888.841891.501s2s(1S)2p3 2D3/21s22s2p 1P01819.511819.23
    1s22s2p 3P11888.291890.961s22s2p 3P01843.131844.45
    1s22s2p 3P21887.121889.761s22s2p 3P11842.581843.92
    1s22p2 1D21840.601841.861s22s2p 3P21841.411842.71
    1s22p2 3P01848.591851.071s22p2 1D21794.891794.81
    1s22p2 3P11848.011850.391s22p2 3P01802.881804.02
    1s22p2 3P21846.911849.381s22p2 3P11802.301803.35
    1s2p4 2D3/21s22s2 1S01910.161913.471s22p2 3P21801.201802.33
    1s22s2p 1P11861.911863.421s2s(1S)2p3 2D5/21s22s2p 1P01819.711819.38
    1s22s2p 3P01885.531888.641s22s2p 3P01843.331844.60
    1s22s2p 3P11884.981888.111s22s2p 3P11842.781844.07
    1s22s2p 3P21883.811886.911s22s2p 3P21841.611842.86
    1s22p2 1S01820.781822.021s22p2 1D21795.091794.96
    1s22p2 1D21837.291839.011s22p2 3P01803.081804.17
    1s22p2 3P01845.281848.221s22p2 3P11802.501803.50
    1s22p2 3P11844.701847.541s22p2 3P21801.401802.49
    1s22p2 3P21843.601846.531s2s(3S)2p3 4S3/21s22p2 3P01807.491810.50
    1s2p4 2D5/21s22s2 1S01909.991913.401s22p2 3P11806.911809.82
    1s22s2p 1P11861.741863.351s22p2 3P21805.811808.81
    1s22s2p 3P01885.361888.571s2s(1S)2p3 4S3/21s22p2 3P01785.261785.14
    1s22s2p 3P11884.811888.041s22p2 3P11784.681784.46
    1s22s2p 3P21883.641886.841s22p2 3P21783.581783.45
    1s22p2 1S01820.611821.951s2s(3S)2p 3 4P1/21s22s2p 3P01831.311832.66
    1s22p2 1D21837.121838.941s22s2p 3P11830.761832.13
    1s22p2 3P01845.111848.151s22s2p 3P21829.591830.93
    1s22p2 3P11844.531847.471s22p2 3P01791.061792.24
    1s22p2 3P21843.431846.461s22p2 3P11790.481791.56
    1s2s22p2 4P1/21s22s2p 3P01792.861791.051s22p2 3P21789.381790.55
    1s22s2p 3P11792.311790.511s2s(3S)2p3 4P3/21s22s2p 3P01831.121832.56
    1s22s2p 3P21791.141789.311s22s2p 3P11830.571832.03
    1s22p2 3P01752.611750.621s22s2p 3P21829.401830.83
    1s22p2 3P11752.031749.941s22p2 3P01790.871792.14
    1s22p2 3P21750.931748.931s22p2 3P11790.291791.46
    1s2s22p2 4P3/21s22s2p 3P01793.531791.831s22p2 3P21789.191790.45
    1s22s2p 3P11792.981791.301s2s(3S)2p3 4P5/21s22s2p 3P01831.041832.48
    1s22s2p 3P21791.811790.091s22s2p 3P11830.491831.95
    1s22p2 3P01753.281751.401s22s2p 3P21829.321830.75
    1s22p2 3P11752.701750.721s22p2 3P01790.791792.06
    1s22p2 3P21751.601749.711s22p2 3P11790.211791.38
    1s2s22p2 4P5/21s22s2p 3P01794.371792.661s22p2 3P21789.111790.37
    1s22s2p 3P11793.821792.131s2s(3S)2p3 4D1/21s22s2p 3P01823.151824.54
    1s22s2p 3P21792.651790.921s22s2p 3P11822.601824.01
    1s22p2 3P01754.121752.231s22s2p 3P21821.431822.80
    1s22p2 3P11753.541751.551s22p2 3P01782.901784.11
    1s22p2 3P21752.441750.541s22p2 3P11782.321783.43
    1s2p4 4P1/21s22s2p 3P01873.831876.311s22p2 3P21781.221782.42
    1s22s2p 3P11873.281875.781s2s(3S)2p3 4D3/21s22s2p 3P01823.151824.49
    1s22s2p 3P21872.111874.571s22s2p 3P11822.601823.96
    1s22p2 3P01833.581835.881s22s2p 3P21821.431822.75
    1s22p2 3P11833.001835.201s22p2 3P01782.901784.06
    1s22p2 3P21831.901834.191s22p2 3P11782.321783.39
    1s2p4 4P3/21s22s2p 3P01873.241875.771s22p2 3P21781.221782.38
    1s22s2p 3P11872.691875.241s2s(3S)2p3 4D5/21s22s2p 3P01823.061824.41
    1s22s2p 3P21871.521874.031s22s2p 3P11822.511823.88
    1s22p2 3P01832.991835.341s22s2p 3P21821.341822.67
    1s22p2 3P11832.411834.661s22p2 3P01782.811783.98
    1s22p2 3P21831.311833.651s22p2 3P11782.231783.30
    1s2p4 4P5/21s22s2p 3P01871.991874.501s22p2 3P21781.131782.29
    1s22s2p 3P11871.441873.961s2s(3S)2p3 4D7/21s22s2p 3P11822.201823.70
    1s22s2p 3P21870.271872.761s22s2p 3P21821.031822.50
    1s22p2 3P01831.741834.071s22p2 3P11781.921783.13
    1s22p2 3P11831.161833.391s22p2 3P21780.821782.12
    1s22p2 3P21830.061832.38
    DownLoad: CSV
    Baidu
  • [1]

    Altun Z, Yumak A, Badnell N R, Colgan J, Pindzol M S 2004 A&A 420 775
    [2]

    Rohringer N, Ryan D, London R A, Purvis M, Albert F, Dunn J, Bozek J D, Bostedt C, Graf A, Hill R, Hau-Riege S P, Rocca J J 2012 Nature 481 488Google Scholar

    [3]

    Martin A, Nicolas G, Jacques S 2006 Nucl. Phys. A 777 1
    [4]

    冯雷, 蒋刚 2017 66 153201Google Scholar

    Feng L, Jiang G 2017 Acta Phys. Sin. 66 153201Google Scholar

    [5]

    Rødbro M, Bruch R, Bisgaard P 1979 J. Phys. B: At. Mol. Opt. Phys. 12 2413Google Scholar

    [6]

    Schneider D, Bruch R, Butscher W, Schwarz W H E 1981 Phys. Rev. A 24 1223Google Scholar

    [7]

    Bruch R, Schneider D, Schwarz W H E, Meinhart M, Johnson B M, Taulbjerg K 1979 Phys. Rev. A 19 587Google Scholar

    [8]

    Itoh A, Schneider D, Schneider T, Zouros T J M, Nolte G, Schiwietz G, Zeitz W, Stolterfoht N 1985 Phys. Rev. A 31 684Google Scholar

    [9]

    Kádár I, Ricz S, Végh J, Sulik B, Varga D, Berényi D 1990 Phys. Rev. A 41 3518Google Scholar

    [10]

    Armour I A, Fawcett B C, Silver J D, Trabert E 1980 J. Phys. B: Atom. Molec. Phys. 13 2701Google Scholar

    [11]

    Trabertt E, Fawcett B C 1979 J. Phys. B: Atom. Molec. Phys. 12 L441
    [12]

    Faenov A Y 1994 Phys. Scr. 49 41Google Scholar

    [13]

    Schlachter A S, Sant’Anna M M, Covington A M, Aguilar A, Gharaibeh M F, Emmons E D, Scully S W J, Phaneuf R A, Hinojosa G, Álvarez I, Cisneros C, Müller A, McLaughlin B M 2004 J. Phys. B: At. Mol. Opt. Phys. 37 L103Google Scholar

    [14]

    Gharaibeh M F, Hassan N E I, Shorman M M A L, Bizau J M, Cubaynes D, Guilbaud S, Sakho I, Blancard C, McLaughlin B M 2014 J. Phys. B: At. Mol. Opt. Phys. 47 065201Google Scholar

    [15]

    Müller A, Borovik A, Buhr T, Hellhund J, Holste K, Kilcoyne A L D, Klumpp S, Martins M, Ricz S, Viefhaus J, Schippers S 2018 Phys. Rev. A 97 013409Google Scholar

    [16]

    Müller A, Borovik A, Buhr J T, Hellhund J, Holste K, Kilcoyne A L D, Klumpp S, Martins M, Ricz S, Viefhaus J, Schippers S 2015 Phys. Rev. Lett. 114 013002Google Scholar

    [17]

    Chen M H, Crasemann B 1988 At. Data Nucl. Data Tables 38 381Google Scholar

    [18]

    Chen M H, Crasemann B 1987 Phys. Rev. A 35 4579Google Scholar

    [19]

    Safronova U I, Shlyaptseva A S 1996 Phys. Scr. 54 254Google Scholar

    [20]

    Zhou F Y, Ma Y L, Qu Y Z 2016 Phys. Rev. A 93 060501Google Scholar

    [21]

    Sakho I, Sow M, Wagué A 2015 Phys. Scr. 90 045401Google Scholar

    [22]

    Sun Y, Chen F, Gou B C 2011 J. Chem. Phys. 135 124309Google Scholar

    [23]

    Sun Y, Gou B C, Chen C 2013 Phys. Rev. A 87 032509Google Scholar

    [24]

    Chung K T 1979 Phys. Rev. A 20 1743Google Scholar

    [25]

    Lin B, Berry H G, Shibata T, Livingston A E, Garnir H P, Bastin T, Désesquelles J, Savukov I 2003 J. Phys. B: At. Mol. Opt. Phys. 67 062507Google Scholar

    [26]

    Drake G W F 1982 Adv. At. Mol. Phys. 18 399Google Scholar

    [27]

    Chung K T, Zhu X W, Wang Z W 1993 Phys. Rev. A 47 1740Google Scholar

    [28]

    Drake G W F, Swainson R A 1990 Phys. Rev. A 41 1243Google Scholar

    [29]

    Chung K T, Davis B F 1982 Phys. Rev. A 26 3278Google Scholar

    [30]

    Davis B F, Chung K T 1984 Phys. Rev. A 29 1878Google Scholar

    [31]

    Kramida A, Ralchenko Yu, Reader J, NIST ASD Team 2018 NIST Atomic Spectra Database (ver. 5.6.1) [Online]. Available: https://physics.nist.gov/asd [2019, March 28]. National Institute of Standards and Technology, Gaithersburg, MD. DOI: https://doi.org/10.18434/T4W30F
  • [1] Qin Chao-Chao, Cui Ming-Huan, Song Di-Di, He Wei. Ultrafast multiexciton Auger recombination of CdSeS. Acta Physica Sinica, 2019, 68(10): 107801. doi: 10.7498/aps.68.20190291
    [2] Qian Xin-Yu, Sun Yan, Liu Dong-Dong, Hu Feng, Fan Qiu-Bo, Gou Bing-Cong. Energy levels and radiative transitions of the core-excited high-spin states in boron atom (ion). Acta Physica Sinica, 2017, 66(12): 123101. doi: 10.7498/aps.66.123101
    [3] Ma Xiao-Yun, Dong Chen-Zhong, Wu Zhong-Wen, Jiang Jun, Xie Lu-You. Theoretical study on electron-impact excitation processes and the relevant polarization of radiation of 2s1/2—2p3/2 in W65+ through W71+. Acta Physica Sinica, 2012, 61(21): 213401. doi: 10.7498/aps.61.213401
    [4] Sun Wei-Feng, Li Mei-Cheng, Zhao Lian-Cheng. First-principles investigation of carrier Auger lifetime and impact ionization rate in narrow-gap superlattices. Acta Physica Sinica, 2010, 59(8): 5661-5666. doi: 10.7498/aps.59.5661
    [5] Liu Shao-Ding, Cheng Mu-Tian, Zhou Hui-Jun, Li Yao-Yi, Wang Qu-Quan, Xue Qi-Kun. The effect of biexciton, wetting layer leakage and Auger capture on Rabi oscillation damping in quantum dots. Acta Physica Sinica, 2006, 55(5): 2122-2127. doi: 10.7498/aps.55.2122
    [6] Li Jie, Dong Chen-Zhong, Xie Lu-You. Effects of relaxation induced by excitation (or ionization) of inner subshell electrons on the wave functions and transition probabilities. Acta Physica Sinica, 2006, 55(2): 655-660. doi: 10.7498/aps.55.655
    [7] WANG FEI, GOU BING-CONG, HAN LI-HONG. RADIATIVE TRANSITION OF CORE-EXCITED 2smd4De STATES TO THE 2pnd4Do STATES IN LITHIUM. Acta Physica Sinica, 2001, 50(9): 1685-1688. doi: 10.7498/aps.50.1685
    [8] YUAN JIN-SHE, CHEN GUANG-DE, QI MING, LI AI-ZHEN, XU ZHUO. XPS AND AES INVESTIGATION OF GaN FILMS GROWN BY MBE. Acta Physica Sinica, 2001, 50(12): 2429-2433. doi: 10.7498/aps.50.2429
    [9] HAN LI-HONG, GOU BING-CONG, WANG FEI. RELATIVISTIC ENERGIES AND FINE STRUCTURES OF THE EXCITED STATES FOR BERYLLIUM-LIKE BⅡ. Acta Physica Sinica, 2001, 50(9): 1681-1684. doi: 10.7498/aps.50.1681
    [10] HAN LI-HONG, GOU BING-CONG, WANG FEI. RADIATIVE TRANSITION OF TRIPLY EXCITED 2p2np4So STATES TO THE ls2pmp4P STATES IN LITHIUM-LIKE IONS. Acta Physica Sinica, 2000, 49(11): 2139-2145. doi: 10.7498/aps.49.2139
    [11] CHEN WEI-DE, GUI YU-DE. DETERMINATION OF AlxGa1-xAs AUGER SENSITIVITY FACTORS. Acta Physica Sinica, 1994, 43(4): 673-677. doi: 10.7498/aps.43.673
    [12] GOU BING-CONG. RADIATIVE AND AUGER RATES OF HIGH DOUBLY EXCITED INTERSHELL STATES. Acta Physica Sinica, 1993, 42(2): 223-230. doi: 10.7498/aps.42.223
    [13] SHI YI-SHENG, ZHAO TE-XIU, LIU HONG-TU, WANG XIAO-PING. XPS AND AES STUDY FOR Pd/W/Si(lll) BILAYER INTERFACE. Acta Physica Sinica, 1992, 41(11): 1849-1855. doi: 10.7498/aps.41.1849
    [14] ZHAO RU-GUANG, YANG WEI-SHENG. TUNABLE-SAMPLING-DEPTH ELECTRON ENERGY LOSS SPECTROSCOPY AND AUGER ELECTRON SPECTROSC-OPY STUDIES OF Pb/Ni(001) INTERFACE. Acta Physica Sinica, 1992, 41(7): 1125-1131. doi: 10.7498/aps.41.1125
    [15] WEI XING, JIANG WEI-DONG, ZHOU GUO-LIANG, YU MING-REN, WANC XUN. AUGER DEPTH PROFILE ANALYSIS OF GexSi1-x/Si SUPERLATTICE. Acta Physica Sinica, 1991, 40(9): 1514-1519. doi: 10.7498/aps.40.1514
    [16] ZHONG ZHAN-TIAN, WANG DA-WEN, LIAO XIAN-BO, FAN YUE, LI CHENG-FANG, MOU SHAN-MING. XPS AND AES STUDY FOR Au/a-Si:H INTERFACE. Acta Physica Sinica, 1991, 40(2): 275-280. doi: 10.7498/aps.40.275
    [17] YU YUE-HUI, LIN CHENG-LU, ZHANG SHUN-KAI, FANG ZI-ZEI, ZOU SHI-CHANG. AUGER ELECTRON SPECTROSCOPIC STUDIES OF INTERFA CE AND BURIED LAYER OF SOI STRUCTURE FORMED BY ION IMPLANTATION. Acta Physica Sinica, 1989, 38(12): 1996-2002. doi: 10.7498/aps.38.1996
    [18] GUO YUAN-HENG, CHEN LAN-FENG. SURFACE STUDY OF SUPERCONDUCTING Nb-Ge FILM BY AUGER ELECTRON SPECTROSCOPY AND ELECTRON SPECTROSCOPY FOR CHEMICAL ANALYSIS. Acta Physica Sinica, 1988, 37(7): 1196-1102. doi: 10.7498/aps.37.1196
    [19] ZHAO ZHONG-XIN, LI JIA-MING. NON- RELATIVISTIC AND RELATIVISTIC ATOMIC CONFIGURATION INTERACTION THEORY EXCITATION ENERGY AND RADIATIVE TRANSITION PROBABILITY. Acta Physica Sinica, 1985, 34(11): 1469-1478. doi: 10.7498/aps.34.1469
    [20] WU ZI-QIN, DUAN JIAN-ZHONG. THE CALCULATION OF BACKSCATTERING FACTORS IN AUGER ELECTRON SPECTROSCOPY. Acta Physica Sinica, 1984, 33(3): 419-424. doi: 10.7498/aps.33.419
Catalog
Metrics
  • Abstract views:  7807
  • PDF Downloads:  44
  • Cited By: 0
Publishing process
  • Received Date:  02 April 2019
  • Accepted Date:  11 June 2019
  • Available Online:  01 August 2019
  • Published Online:  20 August 2019

/

返回文章
返回

Authors

Referees

About Journal

About

Copyright ©Acta Physica Sinica All Rights Reserved. 

Address： PostCode：100190

Phone：010-82649829，82649241,82649863 Email：apsoffice@iphy.ac.cn   百度统计

Baidu
map