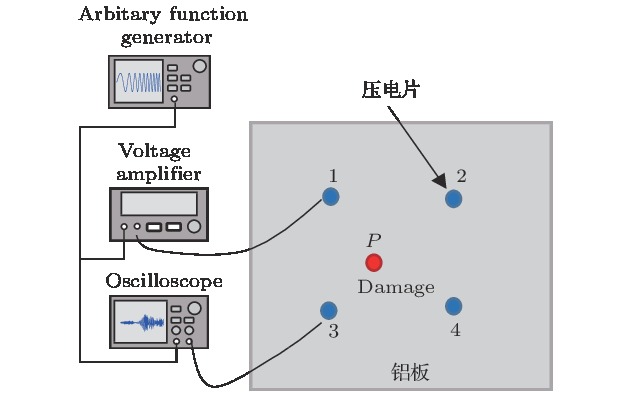
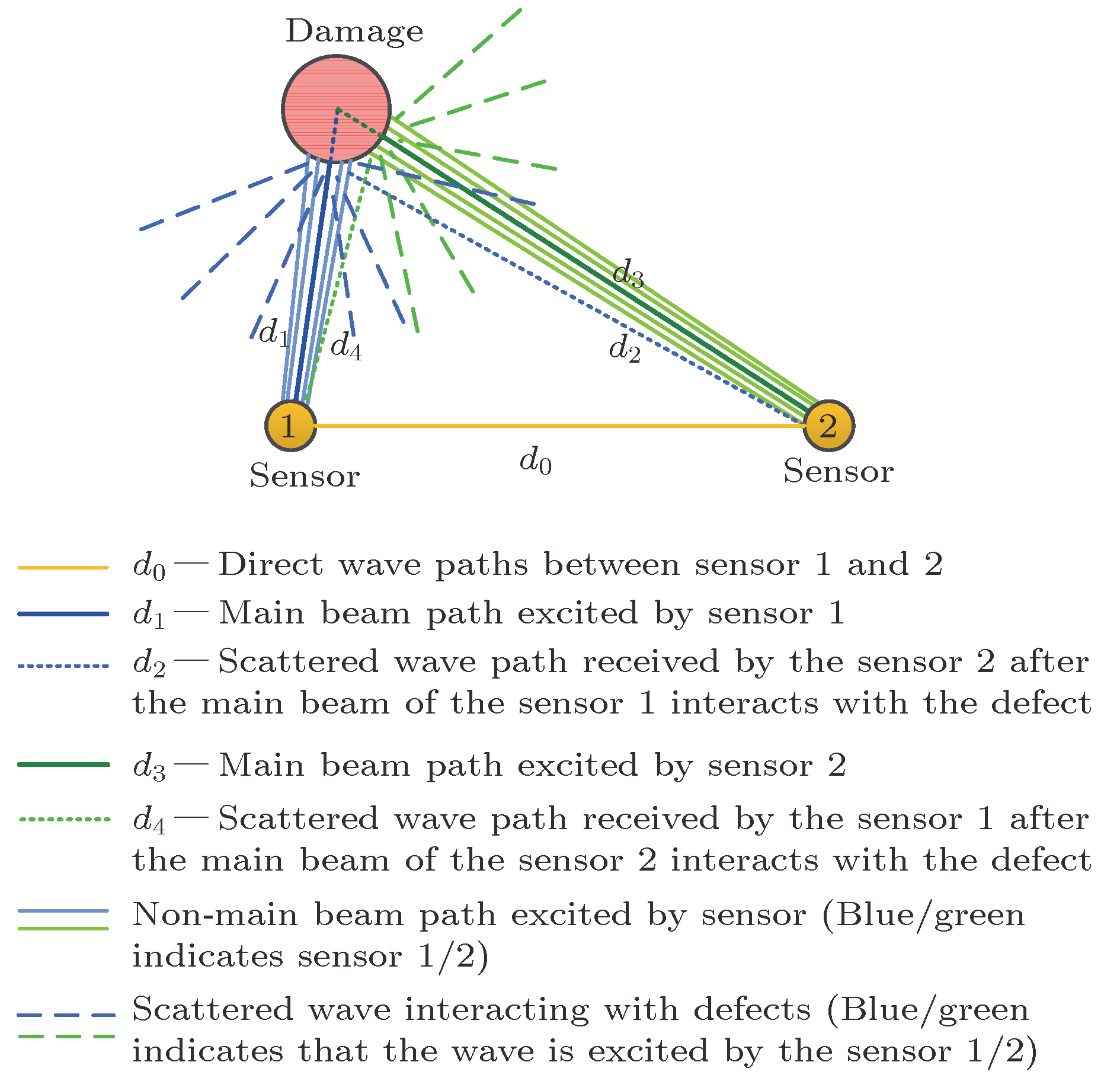
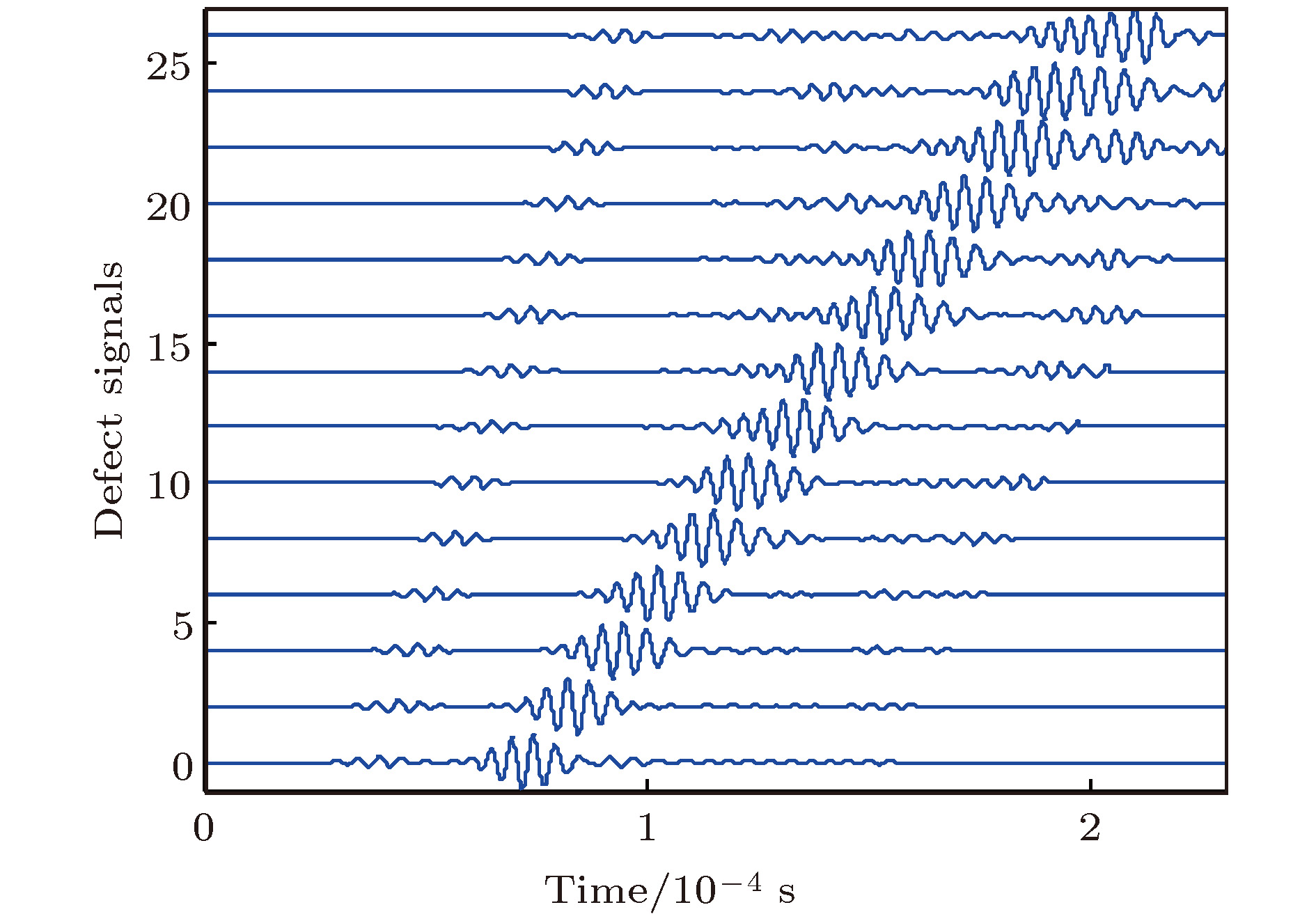
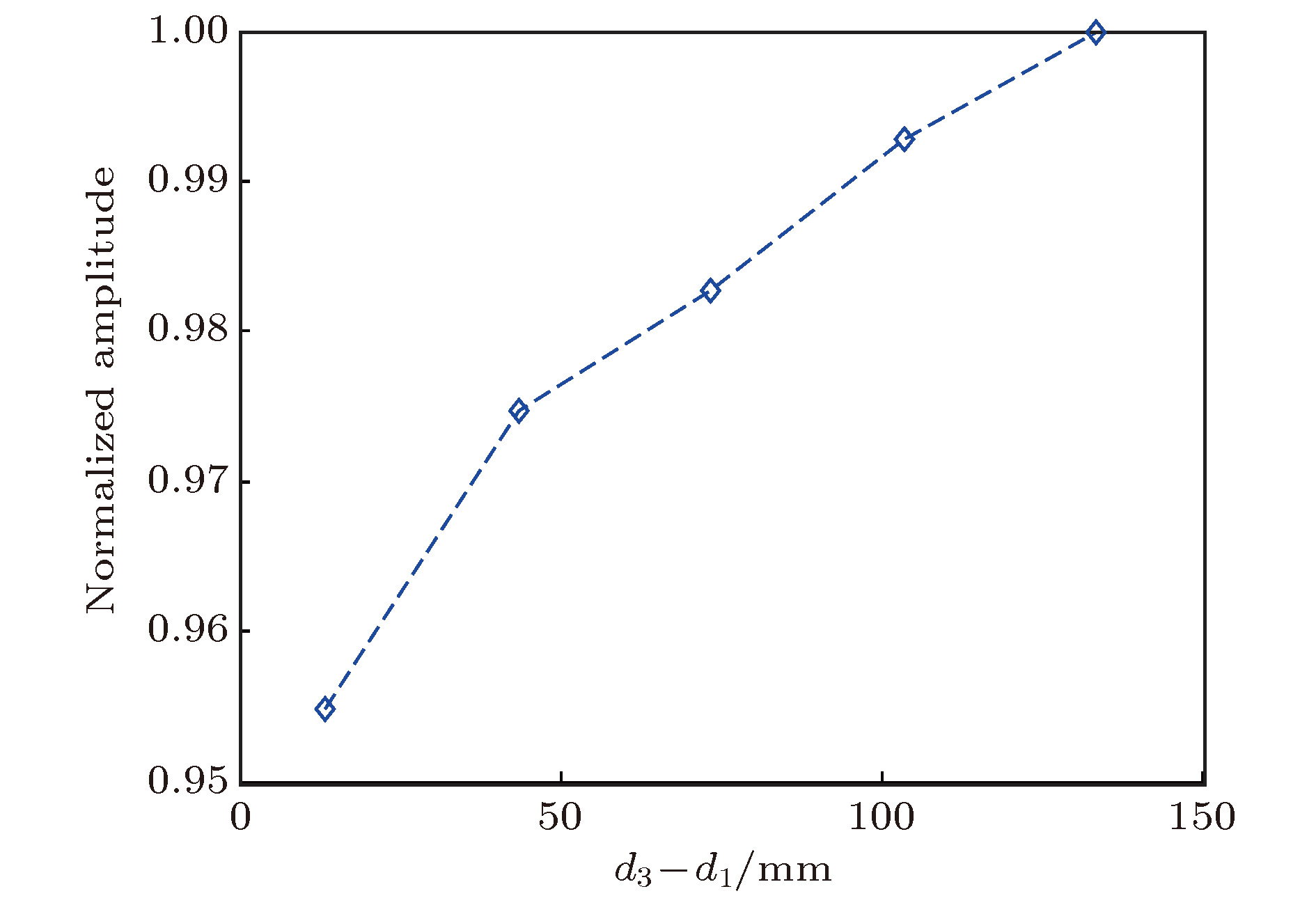
-
The traditional Lamb wave structure health monitoring imaging method based on reference signal is affected by environmental factors such as temperature change. To solve this problem, considering the difference in the scattered fields generated by the interaction between ultrasonic waves and defects in the reverse path, a Lamb wave imaging method is proposed in this paper based on the difference signal of sparse array in inverse path. Numerical simulations are carried out to determine the generation conditions of difference signal in inversion path, and the influences of the angles and distances between the defect and the two sensors on the amplitude of difference signal in inversion path. It is found that the difference signal in reverse path is much more obvious when the defect appears as asymmetric distribution towards the excitation sensor and receiving sensors; the amplitude of difference signal in inverse path is affected by distance difference of the Lamb wave propagating in reverse path and the scattering coefficient of the defect. On this basis, the effectiveness of the Lamb wave imaging method based on the difference signal in inverse path is studied numerically and experimentally. The results show that the Lamb wave imaging method based on the difference signal in inversion path can perfectly eliminate the interference between direct wave and the boundary reflection wave, and the imaging method can detect the defect at different positions in the plate. Moreover, the imaging resolution is higher and the defect location is accurate. The research work provides a new feasible scheme for the extensive health monitoring of plate structure.
-
Keywords:
- Lamb waves /
- plate structure /
- difference signal in reverse paths /
- sparse array
[1] Abbas M, Shafiee M 2018 Sensors 18 3958
Google Scholar
[2] 高广健, 邓明晰, 李明亮, 刘畅 2015 64 224301
Google Scholar
Gao G J, Deng M X, Li M L, Liu C 2015 Acta Phys. Sin. 64 224301
Google Scholar
[3] Kudela P, Radzienski M, Ostachowicz W, Yang Z 2018 Mech. Syst. Signal Proc. 108 21
Google Scholar
[4] Chen S J, Zhou S P, Li Y, Xiang Y X, Qi M X 2017 Chin. Phys. Lett. 34 044301
Google Scholar
[5] Petrone G 2018 Aerosp. Sci. Technol. 82 304
[6] Munian R K, Mahapatra D R, Gopalakrishnan S 2018 Compos. Struct. 206 484
Google Scholar
[7] Mohammadi M, Pouyan A A, Khan N A, Abolghasemi V 2018 Signal Process. 150 85
Google Scholar
[8] Kim C Y, Park K J 2015 NDT&E Int. 74 15
[9] Wilcox P D, Lowe M, Cawley P 2003 IEEE Trans. Ultrason. Ferroelectr. 50 419
Google Scholar
[10] Xu K, Ta D, Moilanen P, Wang W Q 2012 J. Acoust. Soc. Am. 131 2714
Google Scholar
[11] Agrahari J K, Kapuria S 2018 Struct. Control HLTH. 25 e2064
Google Scholar
[12] Salmanpour M S, Sharif K Z, Mhf A 2017 Sensors 17 1178
Google Scholar
[13] Muller A, Robertson-Welsh B, Gaydecki P, Gresil M, Soutis C 2017 Appl. Compos. Mater. 24 553
Google Scholar
[14] Zhao X L, Gao H D, Zhang G F, Ayhan B, Yan F, Kwan C, Rose J L 2007 Smart Mater. Struct. 16 1208
Google Scholar
[15] Chen F, Wilcox P D 2007 Ultrasonics 47 111
Google Scholar
[16] Douglass A C S, Harley J B 2018 IEEE Trans. Ultrason. Ferroelectr. 65 851
Google Scholar
[17] Lu Y, Michaels J E 2009 IEEE Sens. J. 9 1462
Google Scholar
[18] Sohn H 2007 Philos. Trans. R. Soc. A:-Math. Phys. 365 539
Google Scholar
[19] Clarke T, Cawley P, Wilcox P, Croxford A 2009 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 56 2666
Google Scholar
[20] Konstantinidis G, Wilcox P D, Drinkwater B W 2007 IEEE Sens. J. 7 905
Google Scholar
[21] Park H W, Sohn H, Law K H, Farrar C R 2007 J. Sound Vibr. 302 50
Google Scholar
[22] Jan H, Morteza T, Steven D, van Koen D A 2016 Material 9 901
Google Scholar
[23] Tabatabaeipour M, Hettler J, Delrue S, van Den Abeele K 2016 NDT&E Int. 80 23
[24] Ciampa F, Pickering S G, Scarselli G, Meo M 2017 Struct. Control HLTH. 24 e1911
Google Scholar
[25] Demetgul M, Senyurek V Y, Uyandik R, Tansel I N, Yazicioglu O 2015 Measurement 69 42
Google Scholar
[26] Nguyen L T, Kocur G K, Saenger E H 2018 Ultrasonics 90 153
Google Scholar
[27] Hongye L, Xin C, Michaels J E, Michaels T E, Cunfu H 2019 Ultrasonics 91 220
Google Scholar
[28] Zhang J, Drinkwater B W, Wilcox P D 2008 IEEE Trans. Ultrason. Ferroelectr. Freq. Control. 55 2254
Google Scholar
[29] 郑阳, 何存富, 周进节, 张也弛 2013 工程力学 30 236
Zheng Y, He C F, Zhou J J, Zhang Y C 2013 Eng. Mech. 30 236
[30] Harley J B, Moura J M 2013 J. Acoust. Soc. Am. 133 2732
Google Scholar
[31] Harley J B, José M F 2014 J. Acoust. Soc. Am. 135 1231
Google Scholar
[32] Soleimanpour R, Ng C T 2016 J. Civil Struct. Health Monit. 6 447
Google Scholar
-
图 1 无限大薄板中兰姆波传播模型示意图
Figure 1. Schematic diagram of Lamb wave propagation model in an infinite plate.
图 2 接收信号模态分析数值仿真模型
Figure 2. Numerical simulation model of modal analysis of received signals.
图 3 不同位置接收到的z方向的去参考信号
Figure 3. Dereference signals in the z direction received at different positions.
图 4 不同位置接收到x方向的去参考信号
Figure 4. Dereference signal in the x direction received at different positions.
图 5 z方向去参考信号的2D-FFT
Figure 5. 2D-FFT of the reference signal in the z direction.
图 6 x方向去参考信号的2D-FFT
Figure 6. 2D-FFT of the reference signal in the x direction.
图 7 影响因素分析数值仿真模型 (a) 双因素综合影响模型; (b) 距离差影响因素模型
Figure 7. Numerical simulation model of influencing factor analysis: (a) A model of two-factor comprehensive impact; (b) model of distance difference influencing factor.
图 8 当l = 0时接收信号及反转路径差信号
Figure 8. Received signal and reverse path delta signal when l = 0.
图 9 当l ≠ 0时接收信号及反转路径差信号
Figure 9. Received signal and reverse path delta signal when l ≠ 0.
图 10 不同传播距离差下的差信号最大幅值
Figure 10. Maximum amplitude of the delta signals under different propagation distance differences.
图 11 不同传播距离差下的
$\Delta A$ 计算结果Figure 11. Calculation results of
$\Delta A$ under different propagation distance differences.
图 12 不同缺陷位置下的差信号幅值
Figure 12. Amplitude of delta signals at different defect locations.
图 13 圆形通孔缺陷的散射系数
Figure 13. Scattering coefficient of the circular through hole defect
图 14 不同缺陷位置下的散射系数
Figure 14. Scattering coefficients at different defect locations.
图 15 不同缺陷位置下的
$\Delta A$ 值Figure 15. Values of
$\Delta A$ at different defect locations.
图 16 板结构稀疏阵列兰姆波检测仿真模型
Figure 16. Simulation model of lamb waves detection for sparse array of plate structure.
图 17 圆形通孔缺陷成像结果 (a) 序号1 (基于参考信号); (b) 序号2; (c) 序号3; (d) 序号4
Figure 17. Imaging results of circular through hole defects: (a) Number 1 (based on reference signal); (b) number 2; (c) number 3; (d) number 4.
图 18 矩形缺陷成像结果 (a) 序号; (b) 序号2
Figure 18. Imaging results of rectangular defect: (a) Number 1; (b) number 2.
图 19 典型传感器对的反转路径差信号及其对成像的贡献 (a) 1号和3号传感器对的反转路径差信号; (b) 1号和3号传感器对的反转路径差信号对成像的贡献; (c) 2号和4号传感器对的反转路径差信号; (d) 2号和4号传感器对的反转路径差信号对成像的贡献
Figure 19. Inverted path delta signal of a typical sensor pairs and its contribution to imaging: (a) Inverted path delta signal of sensor pairs of number1 and number 3; (b) contribution to imaging of inverted path delta signal of sensor pairs of number1 and number 3; (c) inverted path delta signal of sensor pairs of number 2 and number 4; (d)contribution to imaging of inverted path delta signal of sensor pairs of number 2 and number 4.
图 20 基于反转路径差信号的成像结果
Figure 20. Imaging results based on the inverted path delta signal.
图 21 实验系统示意图
Figure 21. Schematic diagram of the experimental system.
图 23 实验成像结果 (a) 4个传感器; (b) 6个传感器
Figure 23. Imaging results of the experiment: (a) Four sensors; (b) six sensors.
图 22 2号和4号传感器对的接收信号及差信号
Figure 22. Receiving signals and delta signal for sensor pairs 2 and 4.
表 1 不同位置缺陷的坐标(单位mm)以及相应的夹角α (单位(°))
Table 1. Coordinates (unit: mm) of defects at different locations and corresponding angles α (unit: (°))
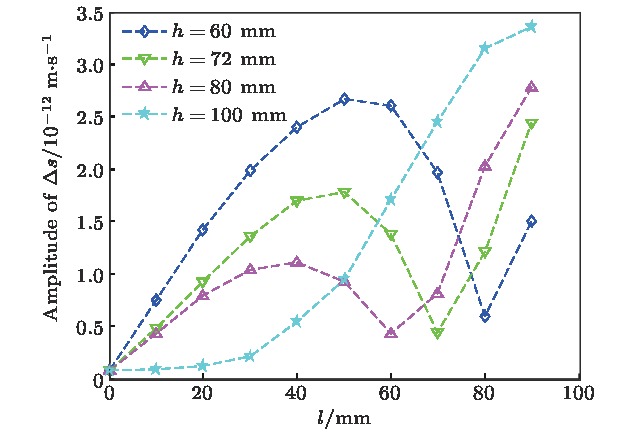
h l 0 10 20 30 40 50 60 70 80 90 60 118.1 117.7 116.6 114.62 111.8 108.0 103.1 97.1 90.0 81.93 72 102.7 102.3 101.3 99.6 97.1 93.9 90.0 85.35 80.1 74.3 80 108.5 108.1 107.0 105.2 102.6 99.1 94.8 89.6 83.7 77.2 100 90.0 89.7 88.8 87.4 85.4 82.8 79.8 76.2 72.2 68.0 
DownLoad: CSV
表 2 圆形缺陷检测时仿真参数及定位结果(单位: mm)
Table 2. Simulation parameters and positioning results for circular defect detection (unit: mm).
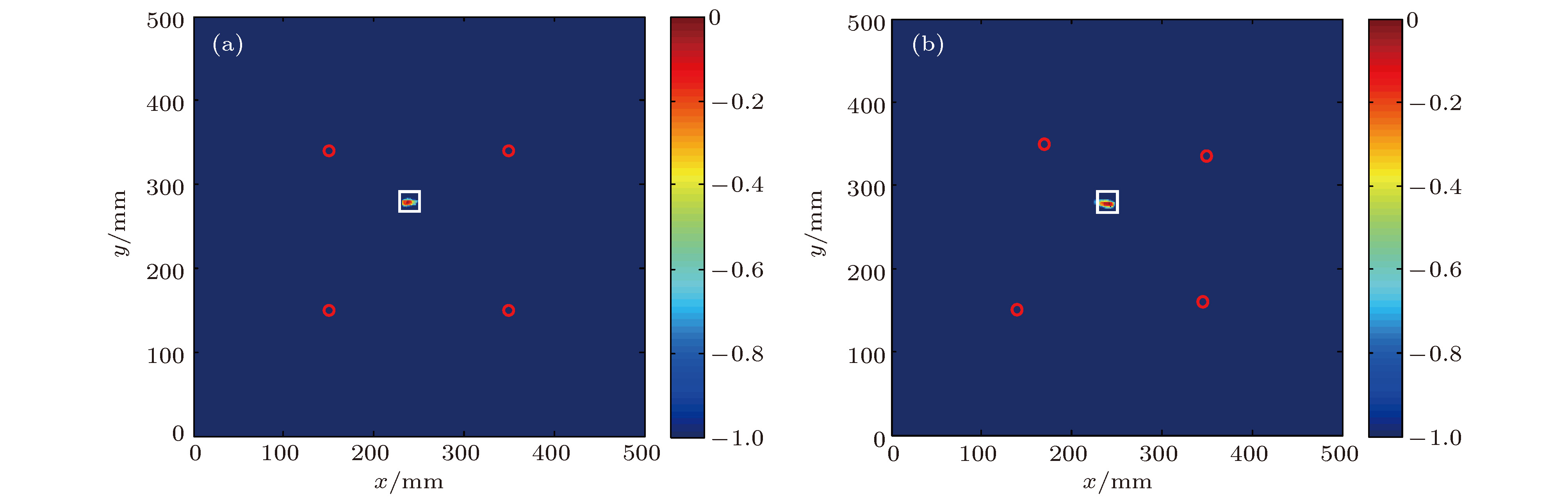
序号 传感器1 传感器2 传感器3 传感器4 缺陷P位置 定位结果 定位误差 1 (基于参考信号) (150, 350) (350, 350) (150, 150) (350, 150) (280, 280) (284, 281) 4.1 2 (150, 350) (350, 350) (150, 150) (350, 150) (280, 280) (285, 285) 7.1 3 (150, 340) (350, 340) (150, 150) (350, 150) (240, 280) (239, 282) 2.2 4 (170, 350) (350, 335) (140, 150) (345, 160) (240, 280) (239, 281) 1.4
DownLoad: CSV
表 3 矩形缺陷检测时仿真参数及定位结果(单位: mm)
Table 3. Simulation parameters and positioning results for rectangular defect detection (unit: mm).
序号 传感器1 传感器2 传感器3 传感器4 缺陷P位置 定位结果 定位误差 1 (150, 340) (350, 340) (150, 150) (350, 150) (240, 280) (239, 279) 1.4 2 (170, 350) (350, 335) (140, 150) (345, 160) (240, 280) (241, 279) 1.4
DownLoad: CSV
表 4 考虑边界影响时仿真参数及定位结果(单位: mm)
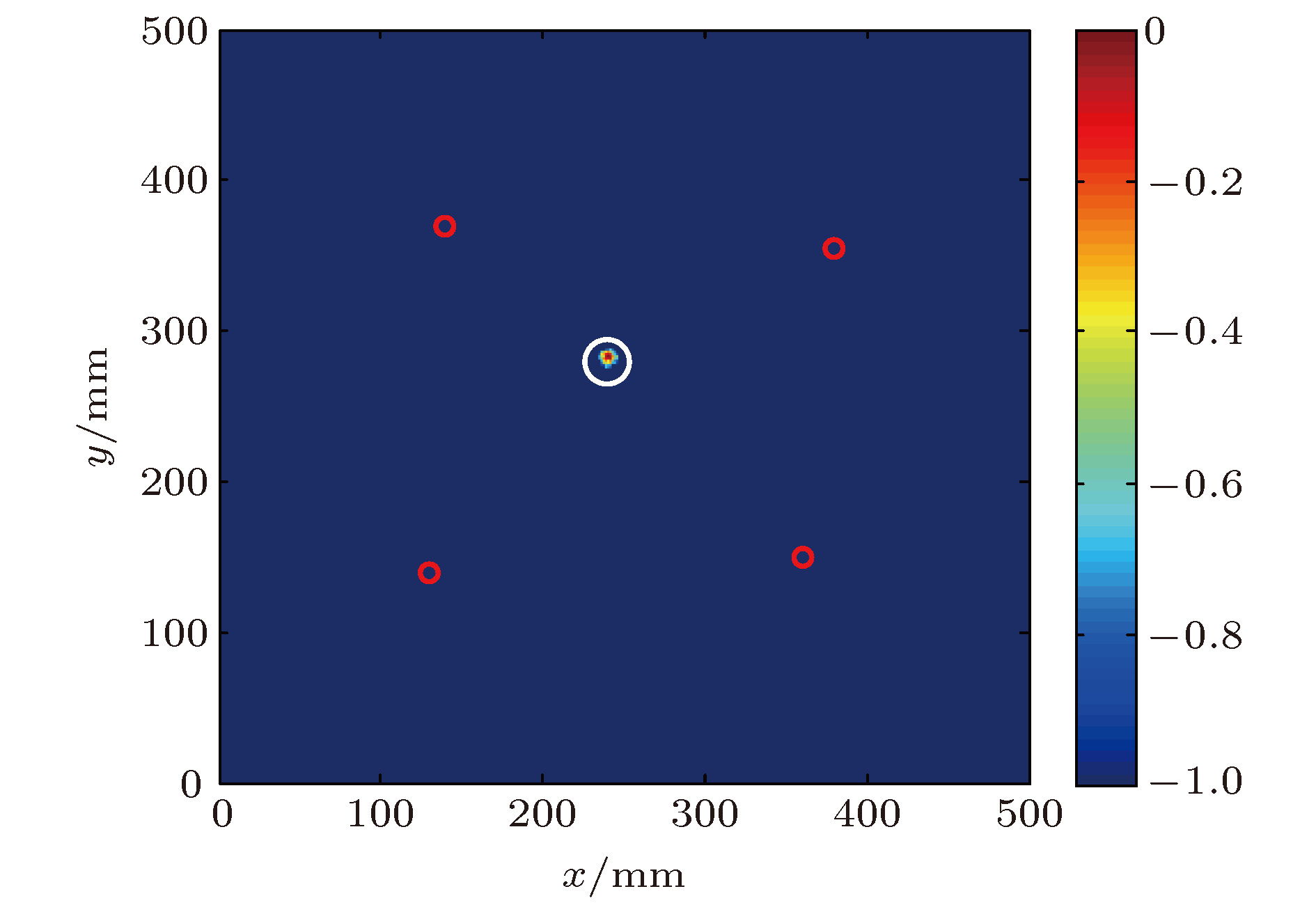
Table 4. Simulation parameters and positioning results when considering boundary effects (unit: mm).
序号 传感器1 传感器2 传感器3 传感器4 缺陷位置 定位结果 定位误差 1 (140, 370) (380, 3355) (130, 140) (360, 150) (240, 280) (240, 284) 4
DownLoad: CSV
-
[1] Abbas M, Shafiee M 2018 Sensors 18 3958
Google Scholar
[2] 高广健, 邓明晰, 李明亮, 刘畅 2015 64 224301
Google Scholar
Gao G J, Deng M X, Li M L, Liu C 2015 Acta Phys. Sin. 64 224301
Google Scholar
[3] Kudela P, Radzienski M, Ostachowicz W, Yang Z 2018 Mech. Syst. Signal Proc. 108 21
Google Scholar
[4] Chen S J, Zhou S P, Li Y, Xiang Y X, Qi M X 2017 Chin. Phys. Lett. 34 044301
Google Scholar
[5] Petrone G 2018 Aerosp. Sci. Technol. 82 304
[6] Munian R K, Mahapatra D R, Gopalakrishnan S 2018 Compos. Struct. 206 484
Google Scholar
[7] Mohammadi M, Pouyan A A, Khan N A, Abolghasemi V 2018 Signal Process. 150 85
Google Scholar
[8] Kim C Y, Park K J 2015 NDT&E Int. 74 15
[9] Wilcox P D, Lowe M, Cawley P 2003 IEEE Trans. Ultrason. Ferroelectr. 50 419
Google Scholar
[10] Xu K, Ta D, Moilanen P, Wang W Q 2012 J. Acoust. Soc. Am. 131 2714
Google Scholar
[11] Agrahari J K, Kapuria S 2018 Struct. Control HLTH. 25 e2064
Google Scholar
[12] Salmanpour M S, Sharif K Z, Mhf A 2017 Sensors 17 1178
Google Scholar
[13] Muller A, Robertson-Welsh B, Gaydecki P, Gresil M, Soutis C 2017 Appl. Compos. Mater. 24 553
Google Scholar
[14] Zhao X L, Gao H D, Zhang G F, Ayhan B, Yan F, Kwan C, Rose J L 2007 Smart Mater. Struct. 16 1208
Google Scholar
[15] Chen F, Wilcox P D 2007 Ultrasonics 47 111
Google Scholar
[16] Douglass A C S, Harley J B 2018 IEEE Trans. Ultrason. Ferroelectr. 65 851
Google Scholar
[17] Lu Y, Michaels J E 2009 IEEE Sens. J. 9 1462
Google Scholar
[18] Sohn H 2007 Philos. Trans. R. Soc. A:-Math. Phys. 365 539
Google Scholar
[19] Clarke T, Cawley P, Wilcox P, Croxford A 2009 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 56 2666
Google Scholar
[20] Konstantinidis G, Wilcox P D, Drinkwater B W 2007 IEEE Sens. J. 7 905
Google Scholar
[21] Park H W, Sohn H, Law K H, Farrar C R 2007 J. Sound Vibr. 302 50
Google Scholar
[22] Jan H, Morteza T, Steven D, van Koen D A 2016 Material 9 901
Google Scholar
[23] Tabatabaeipour M, Hettler J, Delrue S, van Den Abeele K 2016 NDT&E Int. 80 23
[24] Ciampa F, Pickering S G, Scarselli G, Meo M 2017 Struct. Control HLTH. 24 e1911
Google Scholar
[25] Demetgul M, Senyurek V Y, Uyandik R, Tansel I N, Yazicioglu O 2015 Measurement 69 42
Google Scholar
[26] Nguyen L T, Kocur G K, Saenger E H 2018 Ultrasonics 90 153
Google Scholar
[27] Hongye L, Xin C, Michaels J E, Michaels T E, Cunfu H 2019 Ultrasonics 91 220
Google Scholar
[28] Zhang J, Drinkwater B W, Wilcox P D 2008 IEEE Trans. Ultrason. Ferroelectr. Freq. Control. 55 2254
Google Scholar
[29] 郑阳, 何存富, 周进节, 张也弛 2013 工程力学 30 236
Zheng Y, He C F, Zhou J J, Zhang Y C 2013 Eng. Mech. 30 236
[30] Harley J B, Moura J M 2013 J. Acoust. Soc. Am. 133 2732
Google Scholar
[31] Harley J B, José M F 2014 J. Acoust. Soc. Am. 135 1231
Google Scholar
[32] Soleimanpour R, Ng C T 2016 J. Civil Struct. Health Monit. 6 447
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 8007
- PDF Downloads: 56
- Cited By: 0
