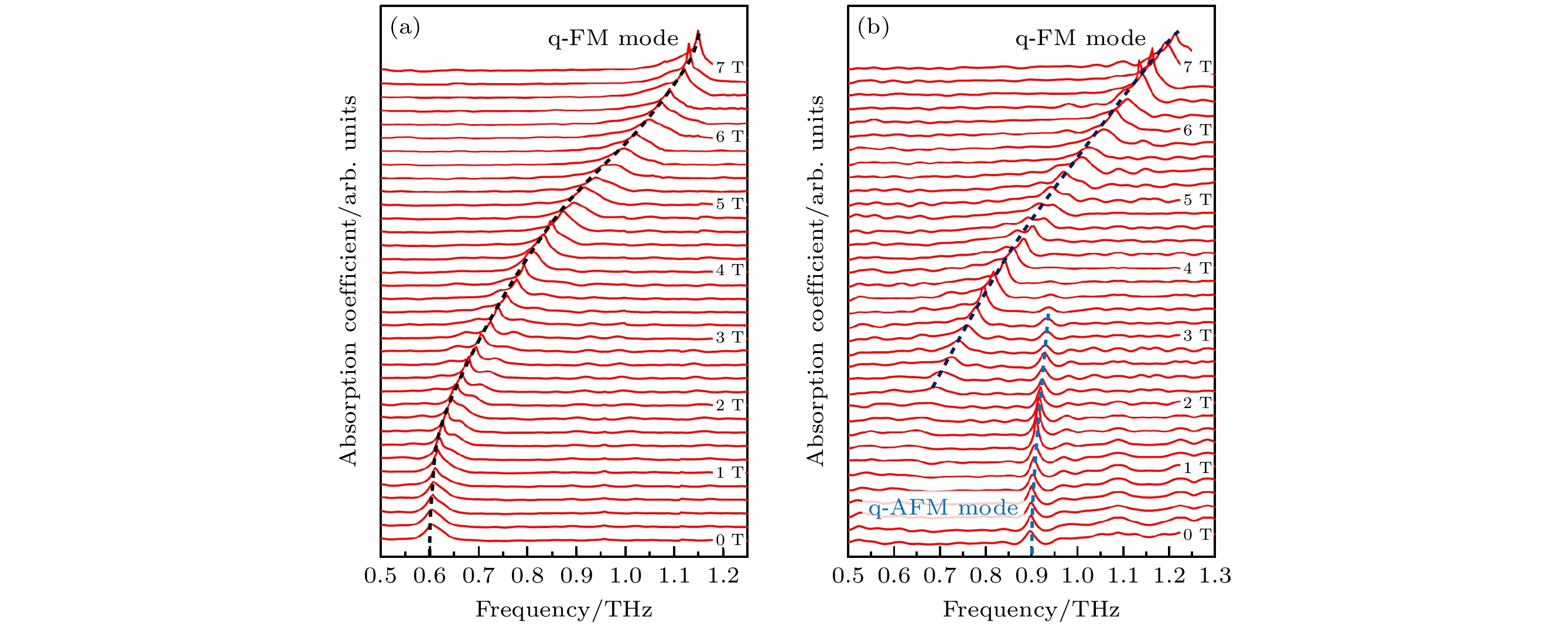
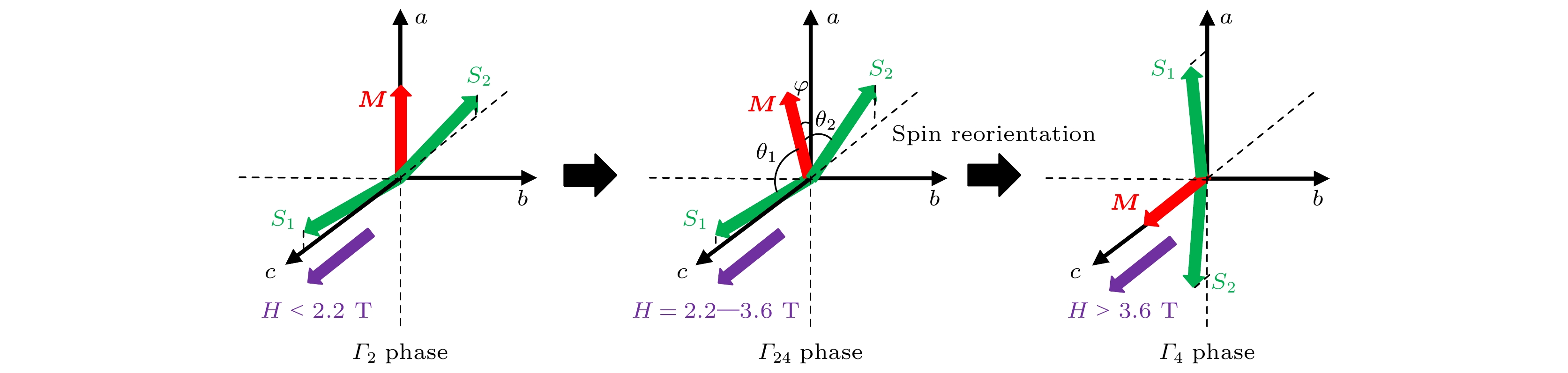
-
TmFeO3具有磁光效应、多铁性和自旋重取向等丰富的物理特性, 在凝聚态物理和材料物理领域具有重要的研究价值. 本文利用时域太赫兹低温磁光谱, 研究 TmFeO3单晶在1.6 K温度下自旋共振频率随外加磁场的变化规律, 并表征其内部复杂的相互作用. 结果表明, 随磁场增加TmFeO3单晶的准铁磁共振向高频移动, 而准反铁磁共振在临界磁场(2.2—3.6 T)转变为准铁磁共振, 通过磁结构分析和理论拟合, 证实单晶磁矩发生了磁场诱导的自旋重取向. 本研究有助于深入理解稀土正铁氧体在外磁场、温度场综合作用下, 内部磁结构的调控机制, 开发相关的自旋电子学器件.TmFeO3 exhibits rich physical properties such as magneto-optical effect, multiferroicity, and spin reorientation, making it possess significant research value in condensed matter physics and materials science. In this study, we utilize a time-domain terahertz magneto-optical spectroscopy system to investigate the changes in spin resonance frequency of TmFeO3 single crystal at T = 1.6 K under external magnetic fields in a range of 0–7 T. The TmFeO3 sample is grown in an optical floating zone furnace and its crystallographic orientation is determined by using back-reflection Laue X-ray photography with a tungsten target. The measurement setup is a self-built time-domain terahertz magneto-optical spectroscopy system, with magnetic fields in a range of 0–7 T, temperatures in a range of 1.6–300 K, and a spectral range of 0.2–2.0 THz. A pair of 1 mm-thick ZnTe nonlinear crystals is used to generate and detect terahertz signals through optical rectification and electro-optic sampling technique. The system variable temperature and magnetic field are controlled by a superconducting magnet. In experiments, a linearly polarized terahertz wave is vertically incident on the sample surface, and its magnetic component HTHz is parallel to the sample surface. By rotating the sample, the angle (θ) between macroscopic magnetic moment M and HTHz can be tuned, achieving selective excitations of the two modes, that is, θ = 0 for q-AFM mode and 90° for q-FM mode. Terahertz absorption spectrum results indicate that as the magnetic field increases, the quasi-ferromagnetic resonance (q-FM) of TmFeO3 single crystal shifts towards high frequencies, and quasi-antiferromagnetic resonance (q-AFM) transits to q-FM under low critical magnetic fields (2.2–3.6 T). Through magnetic structure analysis and theoretical fitting, it is confirmed that the magnetic moment of the single crystal undergoes magnetic field induced spin reorientation. This study is helpful in better understanding of the regulation mechanism of the internal magnetic structure of rare earth ferrite under the combined action of external magnetic field and temperature field, and also in developing related spin electronic devices.
-
Keywords:
- terahertz /
- rare earth orthoferrite /
- ferromagnetic resonance /
- spin reorientation
[1] Kimel A V, Ivanov B A, Pisarev R V, Usachev P A, Kirilyuk A, Rasing Th 2009 Nat. Phys. 5 727
Google Scholar
[2] Afanasiev D, Ivanov B A, Kirilyuk A, Rasing Th, Pisarev R V, Kimel A V 2016 Phys. Rev. Lett. 116 658270
[3] Nova T F, Cartella A, Cantaluppi A, Först M, Bossini D, Mikhaylovskiy R V, Kimel A V, Merlin R, Cavalleri A 2017 Nat. Phys. 13 132
Google Scholar
[4] White R L 1969 J. Appl. Phys. 40 1061
Google Scholar
[5] Yamaguchi T 1974 J. Phys. Chem. Solids. 35 479
Google Scholar
[6] Ma X X, Yuan N, Yang W T, Zhu S, Shi C F, Song H, Sun Z Q, Kang B J, Ren W, Cao S X 2022 Inorg. Chem. 61 14815
Google Scholar
[7] Dzyaloshinsky I 1958 J. Phys. Chem. Solids. 4 241
Google Scholar
[8] Moriya T 1960 Phys. Rev. 120 91
Google Scholar
[9] 金钻明, 阮舜逸, 李炬赓, 林贤, 任伟, 曹世勋, 马国宏, 姚建铨 2019 68 167501
Google Scholar
Jin Z M, Ruan S Y, Li J G, Lin X, Ren W, Cao S X, Ma G H, Yao J Q 2019 Acta Phys. Sin. 68 167501
Google Scholar
[10] Song H, Fan W C, Jia R R, Sun Z Q, Ma X X, Yang W T, Zhu S, Kang B J, Feng Z J, Cao S X 2023 Ceram. Int. 49 22038
Google Scholar
[11] Guo J J, Cheng L, Ren Z, Zhang W J, Lin X, Jin Z M, Cao S X, Sheng Z G, Ma G H 2020 J. Phys. Condens. Matter 32 185401
[12] Jiang J J, Song G B, Wang D Y, Jin Z M, Tian Z, Lin X, Han J G, Ma G H, Cao S X, Cheng Z X 2016 J. Phys. Condens. Matter 28 116002
[13] Shen H, Cheng Z X, Hong F, Xu J Y, Yuan S J, Cao S X, Wang X L 2013 Appl. Phys. Lett. 103 192404
Google Scholar
[14] Nikitin S E, Wu L S, Sefat A S, Shaykhutdinov K A, Lu Z, Meng S, Pomjakushina E V, Conder K, Ehlers G, Lumsden M D, Kolesnikov A I, Barilo S, Guretskii S A, Inosov D S, Podlesnyak A 2018 Phys. Rev. B 98 064424
Google Scholar
[15] Slawinski W, Przenioslo R, Sosnowska I, Suard E 2005 J. Phys. Condens. Matter 17 4605
[16] Kimel A V, Kirilyuk A, Usachev P A, Pisarev R V, Balbashov A M, Rasing Th 2005 Nature 435 655
Google Scholar
[17] Chen L, Li T W, Cao S X, Yuan S J, Hong F, Zhang J C 2012 J. Appl. Phys. 111 103905
Google Scholar
[18] Ramu N, Muralidharan R, Meera K, Jeong Y H 2016 RSC Adv. 6 72295
Google Scholar
[19] Zhao W Y, Cao S X, Huang R X, Cao Y M, Xu K, Kang B J, Zhang J C, Ren W 2015 Phys. Rev. B 91 104425
Google Scholar
[20] Long H, Cao S X, Liu M, Kang Y M, Zhang B J, Jin C 2014 Phys. Rev. B 90 144415
Google Scholar
[21] 任壮, 成龙, 谢尔盖·固瑞特斯基, 那泽亚·柳博奇科, 李江涛, 尚加敏, 谢尔盖·巴里洛, 武安华, 亚历山大·卡拉什尼科娃, 马宗伟, 周春, 盛志高 2020 69 207802
Google Scholar
Ren Z, Cheng L, Sergey G, Nadezhda L, Li J T, Shang J M, Sergey B, Wu A H, Aleksandra K, Ma Z W, Zhou C, Sheng Z G 2020 Acta Phys. Sin. 69 207802
Google Scholar
[22] Amelin K, Nagel U, Fishman R S, Yoshida Y, Sim H, Park K, Park J G, Rõõm T 2018 Phys. Rev. B 98 174417
Google Scholar
[23] Nagel U, Fishman Randy S, Katuwal T, Engelkamp H, Talbayev D, Yi H T, Cheong S W, Rõõm T 2013 Phys. Rev. Lett. 110 257201
Google Scholar
[24] Beard M C, Turner G M, Schmuttenmaer C A 2001 J. Appl. Phys. 90 5915
Google Scholar
[25] Kim T H, Kang C, Kee C S, Lee J H, Cho B K, Gruenberg P, Tokunaga Y, Tokura Y, Lee J S 2015 J. Appl. Phys. 118 233101
Google Scholar
[26] Reid A H M, Rasing Th, Pisarev R V, Duerr H A, Hoffmann M C 2015 Appl. Phys. Lett. 106 082403
Google Scholar
[27] Grishunin K, Huisman T, Li G Q, Mishina E, Rasing T, Kimel A V, Zhang K L, Jin Z M, Cao S X, Ma G H, Mikhaylovskiy R V 2018 ACS Photonics 5 1375
Google Scholar
[28] Johnson C E, Prelorendjo L A, Thomas M F 1980 J. Magn. Magn. Mater. 15 557
[29] Ju X W, Hu Z Q, Huang F, Wu H B, Wang X F 2021 Opt. Express 29 9261
Google Scholar
[30] Tsuyoshi Y, Kunirô T 1973 Phys. Rev. B 8 5187
Google Scholar
[31] Li X W, Bamba M, Yuan N, Zhang Q, Zhao Y, Xiang M L, Xu K, Jin Z M, Ren W, Ma G H, Cao S X, Turchinovich D, Kono J 2018 Science 361 794
Google Scholar
[32] Balbashov A M, Berezin A G, Gufan Y M, Kolyadko G S, Marchukov P Y, Rudashevskii E G 1987 J. Exp. Theor. Phys. 66 174
[33] Lin X, Jiang J J, Jin Z M, Wang D Y, Tian Z, Han J G, Cheng Z X, Ma G H 2015 Appl. Phys. Lett. 106 092403
Google Scholar
[34] Gorodetsky G, Shaft S, Remeika J P 1981 J. Appl. Phys. 52 7353
Google Scholar
[35] Herrmann G F 1963 J. Phys. Chem. Solids 24 597
Google Scholar
-
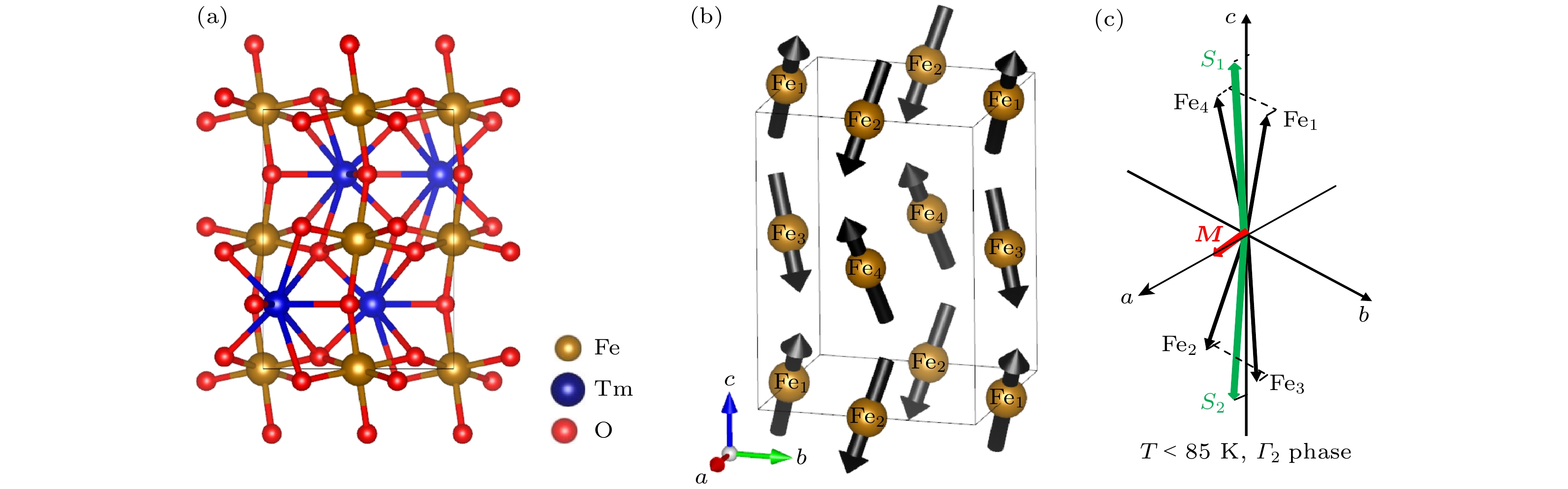
图 1 TmFeO3晶体结构及倾角反铁磁自旋构型示意图 (a)晶体结构示意图; (b)反铁磁亚晶格Fe1-4自旋方向示意图; (c) T < 85 K, Γ2相的自旋磁矩构型示意图, S1和S2表示两对合成的Fe3+亚晶格自旋
Fig. 1. Schematic diagrams of TmFeO3 crystal’s structure and canted-antiferromagnet spin configuration: (a) Crystal’s structure diagram; (b) spin directions for the Fe1-4 sites in the antiferromagnetic sublattice; (c) spin magnetic moment configuration schematic of Γ2 phase at T < 85 K, S1 and S2 stand for two pairs of spins for synthesized Fe3+ sublattices.
图 2 TmFeO3共振模式激发规律及实验构型 (a) THz波选择性激发q-FM和q-AFM两种共振模式; (b)实验构型图, 通过旋转样品改变宏观磁化矢量M 和THz磁场分量的角度
Fig. 2. Excitation rule of TmFeO3 resonance modes and experimental geometry: (a) THz wave selectively excites the two resonance modes of q-FM and q-AFM; (b) experimental geometry, the angle between the macroscopic magnetization vector M and the THz magnetic field component is changed by rotating the sample.
图 3 T = 1.6 K时, 沿晶体c轴施加0—7 T外磁场, TmFeO3在不同磁场下的THz吸收光谱 (a) HTHz//b轴; (b) HTHz//a轴
Fig. 3. THz absorption spectra of TmFeO3 at T = 1.6 K under different magnetic fields applied along crystal’s c-axis from 0–7 T: (a) HTHz//b-axis; (b) HTHz//a-axis.
图 4 1.6 K温度下TmFeO3在沿c轴外加磁场影响下磁结构变化示意图
Fig. 4. Schematic diagram of magnetic structure change of TmFeO3 under magnetic field applied along the crystal’s c-axis
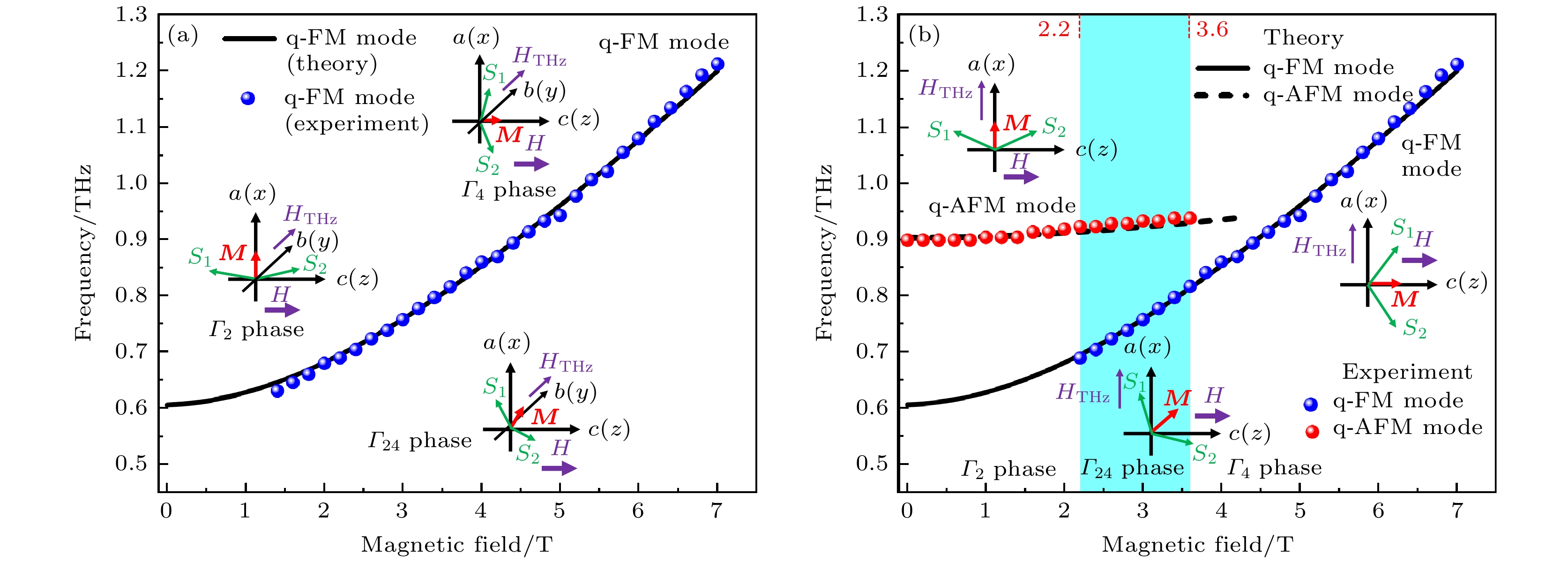
图 5 TmFeO3在1.6 K共振频率随磁场变化的理论拟合 (a)图3(a)中q-FM共振频率及其理论拟合曲线; (b)图3(b)中q-AFM和q-FM共振频率及其理论拟合曲线. 图中的插图显示了太赫兹磁分量HTHz方向, 外磁场H方向, 及Γ2相TmFeO3在不同磁场下的简化磁结构变化示意图. 黑色虚线是根据(1)式对q-AFM共振频率的拟合; 黑色实线是根据(6)式对q-FM共振频率的拟合
Fig. 5. Theoretical fittings of TmFeO3 resonance frequency varying with magnetic field at 1.6 K: (a) The q-FM resonance frequencies in Fig. 3(a) and its theoretical fitting curve; (b) the q-AFM and q-FM resonance frequencies in Fig. 3(b) and their theoretical fitting curves. The illustrations in the figure show the terahertz magnetic component HTHz, the external magnetic field H, and simplified magnetic structure changes of Γ2-phase TmFeO3 under different magnetic fields. The black dashed line is the fitting of q-AFM resonance frequency according to Eq. (1); black solid line is the fitting of q-FM resonance frequency according to Eq. (6).
-
[1] Kimel A V, Ivanov B A, Pisarev R V, Usachev P A, Kirilyuk A, Rasing Th 2009 Nat. Phys. 5 727
Google Scholar
[2] Afanasiev D, Ivanov B A, Kirilyuk A, Rasing Th, Pisarev R V, Kimel A V 2016 Phys. Rev. Lett. 116 658270
[3] Nova T F, Cartella A, Cantaluppi A, Först M, Bossini D, Mikhaylovskiy R V, Kimel A V, Merlin R, Cavalleri A 2017 Nat. Phys. 13 132
Google Scholar
[4] White R L 1969 J. Appl. Phys. 40 1061
Google Scholar
[5] Yamaguchi T 1974 J. Phys. Chem. Solids. 35 479
Google Scholar
[6] Ma X X, Yuan N, Yang W T, Zhu S, Shi C F, Song H, Sun Z Q, Kang B J, Ren W, Cao S X 2022 Inorg. Chem. 61 14815
Google Scholar
[7] Dzyaloshinsky I 1958 J. Phys. Chem. Solids. 4 241
Google Scholar
[8] Moriya T 1960 Phys. Rev. 120 91
Google Scholar
[9] 金钻明, 阮舜逸, 李炬赓, 林贤, 任伟, 曹世勋, 马国宏, 姚建铨 2019 68 167501
Google Scholar
Jin Z M, Ruan S Y, Li J G, Lin X, Ren W, Cao S X, Ma G H, Yao J Q 2019 Acta Phys. Sin. 68 167501
Google Scholar
[10] Song H, Fan W C, Jia R R, Sun Z Q, Ma X X, Yang W T, Zhu S, Kang B J, Feng Z J, Cao S X 2023 Ceram. Int. 49 22038
Google Scholar
[11] Guo J J, Cheng L, Ren Z, Zhang W J, Lin X, Jin Z M, Cao S X, Sheng Z G, Ma G H 2020 J. Phys. Condens. Matter 32 185401
[12] Jiang J J, Song G B, Wang D Y, Jin Z M, Tian Z, Lin X, Han J G, Ma G H, Cao S X, Cheng Z X 2016 J. Phys. Condens. Matter 28 116002
[13] Shen H, Cheng Z X, Hong F, Xu J Y, Yuan S J, Cao S X, Wang X L 2013 Appl. Phys. Lett. 103 192404
Google Scholar
[14] Nikitin S E, Wu L S, Sefat A S, Shaykhutdinov K A, Lu Z, Meng S, Pomjakushina E V, Conder K, Ehlers G, Lumsden M D, Kolesnikov A I, Barilo S, Guretskii S A, Inosov D S, Podlesnyak A 2018 Phys. Rev. B 98 064424
Google Scholar
[15] Slawinski W, Przenioslo R, Sosnowska I, Suard E 2005 J. Phys. Condens. Matter 17 4605
[16] Kimel A V, Kirilyuk A, Usachev P A, Pisarev R V, Balbashov A M, Rasing Th 2005 Nature 435 655
Google Scholar
[17] Chen L, Li T W, Cao S X, Yuan S J, Hong F, Zhang J C 2012 J. Appl. Phys. 111 103905
Google Scholar
[18] Ramu N, Muralidharan R, Meera K, Jeong Y H 2016 RSC Adv. 6 72295
Google Scholar
[19] Zhao W Y, Cao S X, Huang R X, Cao Y M, Xu K, Kang B J, Zhang J C, Ren W 2015 Phys. Rev. B 91 104425
Google Scholar
[20] Long H, Cao S X, Liu M, Kang Y M, Zhang B J, Jin C 2014 Phys. Rev. B 90 144415
Google Scholar
[21] 任壮, 成龙, 谢尔盖·固瑞特斯基, 那泽亚·柳博奇科, 李江涛, 尚加敏, 谢尔盖·巴里洛, 武安华, 亚历山大·卡拉什尼科娃, 马宗伟, 周春, 盛志高 2020 69 207802
Google Scholar
Ren Z, Cheng L, Sergey G, Nadezhda L, Li J T, Shang J M, Sergey B, Wu A H, Aleksandra K, Ma Z W, Zhou C, Sheng Z G 2020 Acta Phys. Sin. 69 207802
Google Scholar
[22] Amelin K, Nagel U, Fishman R S, Yoshida Y, Sim H, Park K, Park J G, Rõõm T 2018 Phys. Rev. B 98 174417
Google Scholar
[23] Nagel U, Fishman Randy S, Katuwal T, Engelkamp H, Talbayev D, Yi H T, Cheong S W, Rõõm T 2013 Phys. Rev. Lett. 110 257201
Google Scholar
[24] Beard M C, Turner G M, Schmuttenmaer C A 2001 J. Appl. Phys. 90 5915
Google Scholar
[25] Kim T H, Kang C, Kee C S, Lee J H, Cho B K, Gruenberg P, Tokunaga Y, Tokura Y, Lee J S 2015 J. Appl. Phys. 118 233101
Google Scholar
[26] Reid A H M, Rasing Th, Pisarev R V, Duerr H A, Hoffmann M C 2015 Appl. Phys. Lett. 106 082403
Google Scholar
[27] Grishunin K, Huisman T, Li G Q, Mishina E, Rasing T, Kimel A V, Zhang K L, Jin Z M, Cao S X, Ma G H, Mikhaylovskiy R V 2018 ACS Photonics 5 1375
Google Scholar
[28] Johnson C E, Prelorendjo L A, Thomas M F 1980 J. Magn. Magn. Mater. 15 557
[29] Ju X W, Hu Z Q, Huang F, Wu H B, Wang X F 2021 Opt. Express 29 9261
Google Scholar
[30] Tsuyoshi Y, Kunirô T 1973 Phys. Rev. B 8 5187
Google Scholar
[31] Li X W, Bamba M, Yuan N, Zhang Q, Zhao Y, Xiang M L, Xu K, Jin Z M, Ren W, Ma G H, Cao S X, Turchinovich D, Kono J 2018 Science 361 794
Google Scholar
[32] Balbashov A M, Berezin A G, Gufan Y M, Kolyadko G S, Marchukov P Y, Rudashevskii E G 1987 J. Exp. Theor. Phys. 66 174
[33] Lin X, Jiang J J, Jin Z M, Wang D Y, Tian Z, Han J G, Cheng Z X, Ma G H 2015 Appl. Phys. Lett. 106 092403
Google Scholar
[34] Gorodetsky G, Shaft S, Remeika J P 1981 J. Appl. Phys. 52 7353
Google Scholar
[35] Herrmann G F 1963 J. Phys. Chem. Solids 24 597
Google Scholar
下载:
计量
- 文章访问数: 2412
- PDF下载量: 85
- 被引次数: 0
