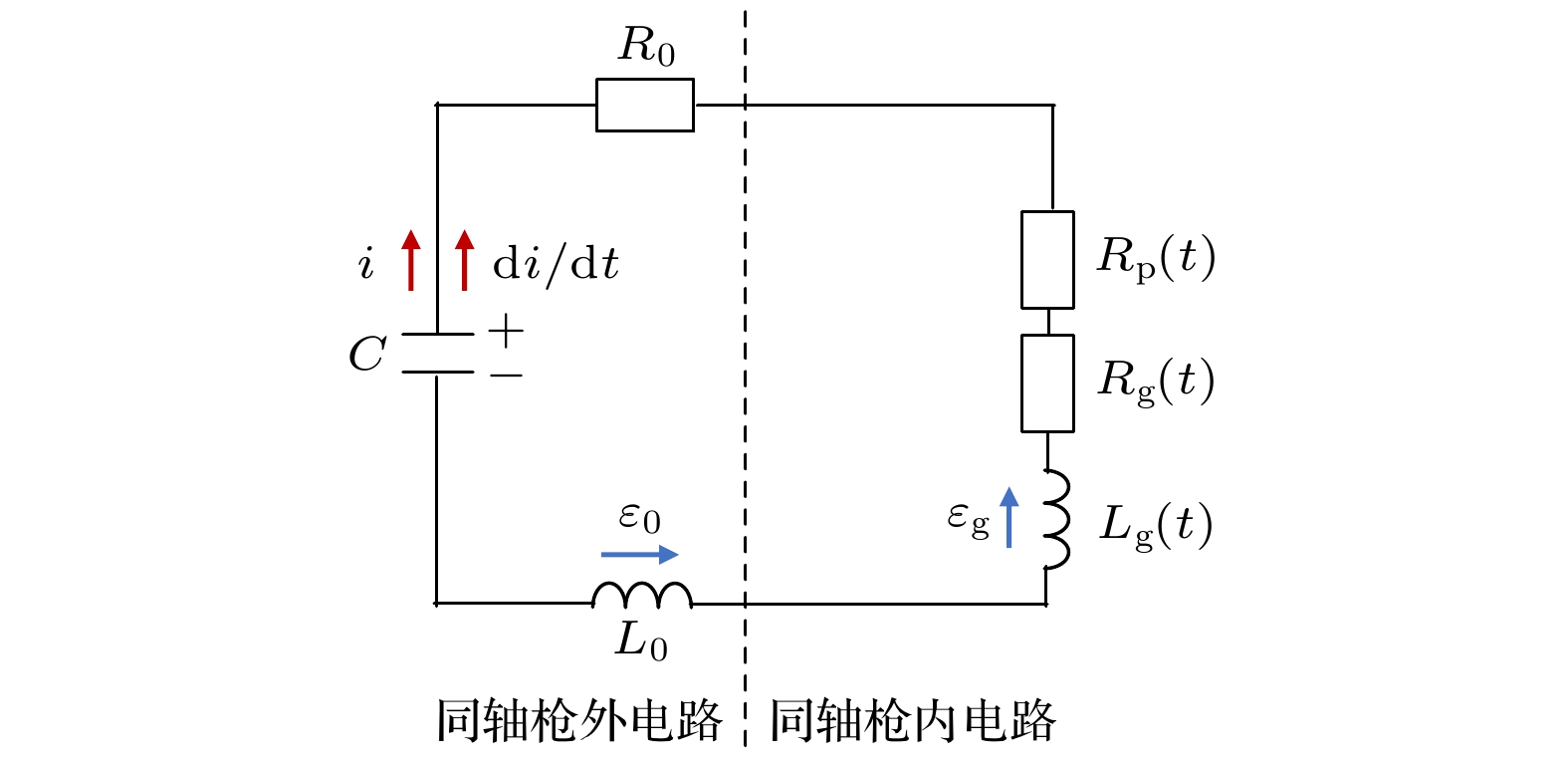
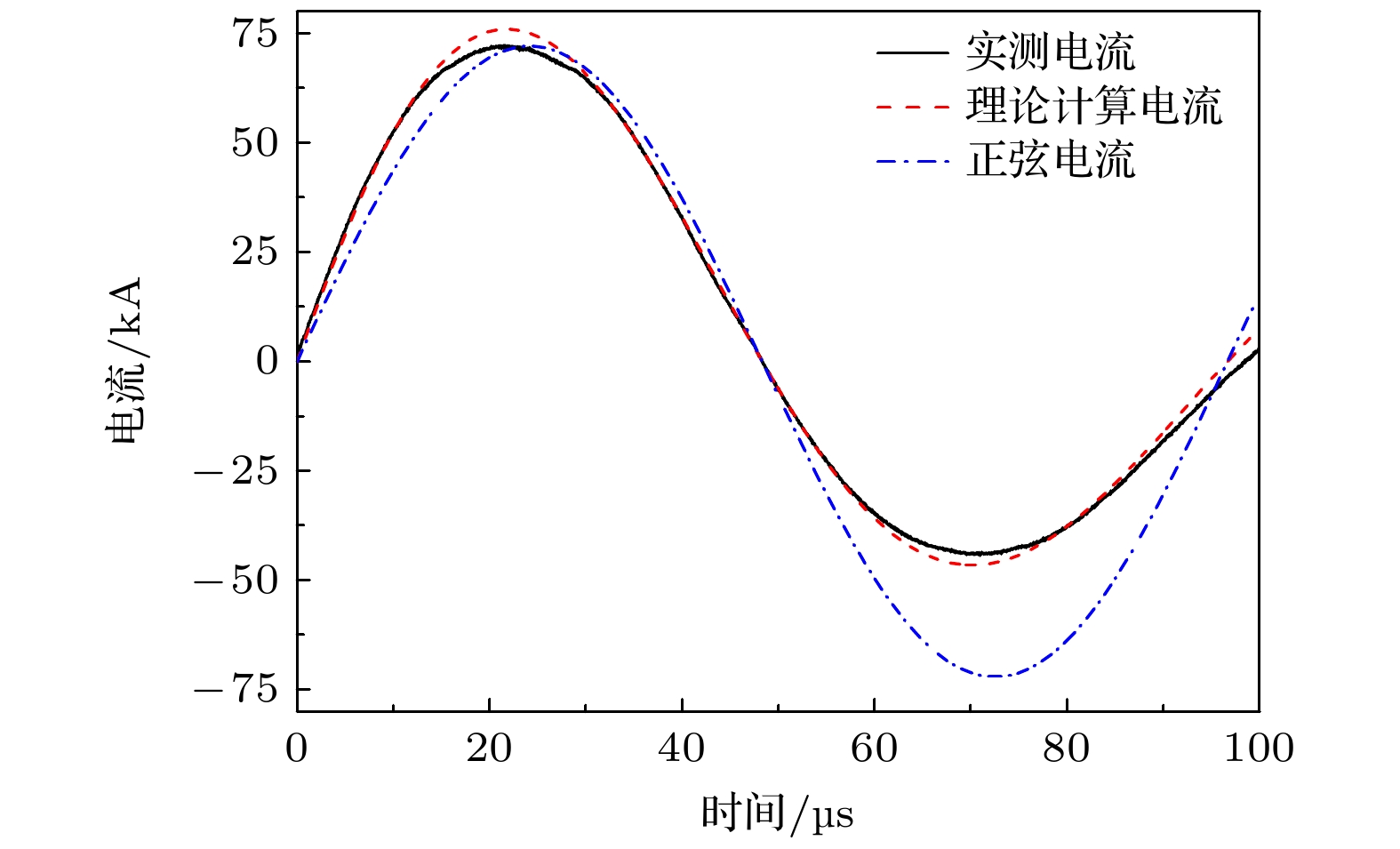
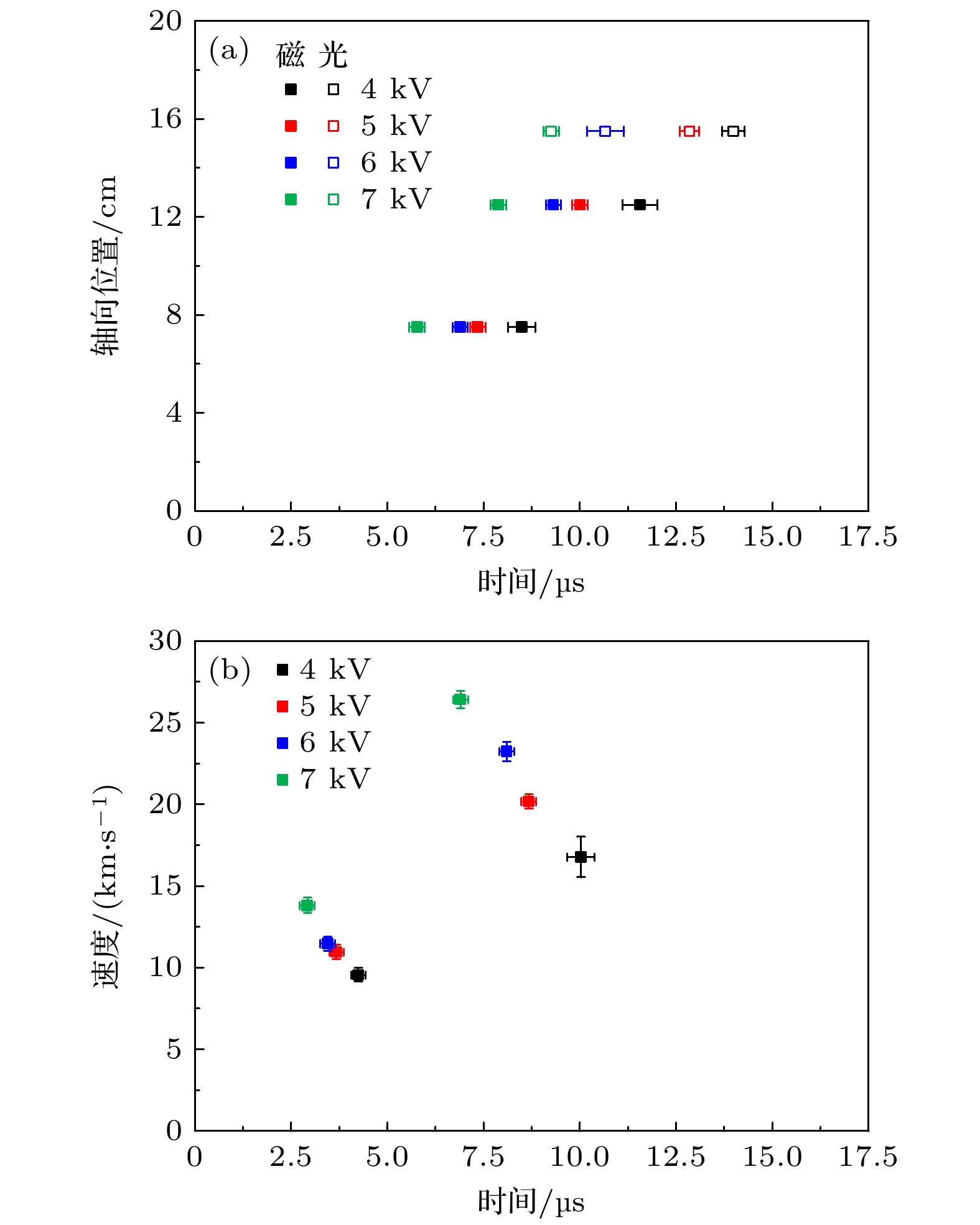
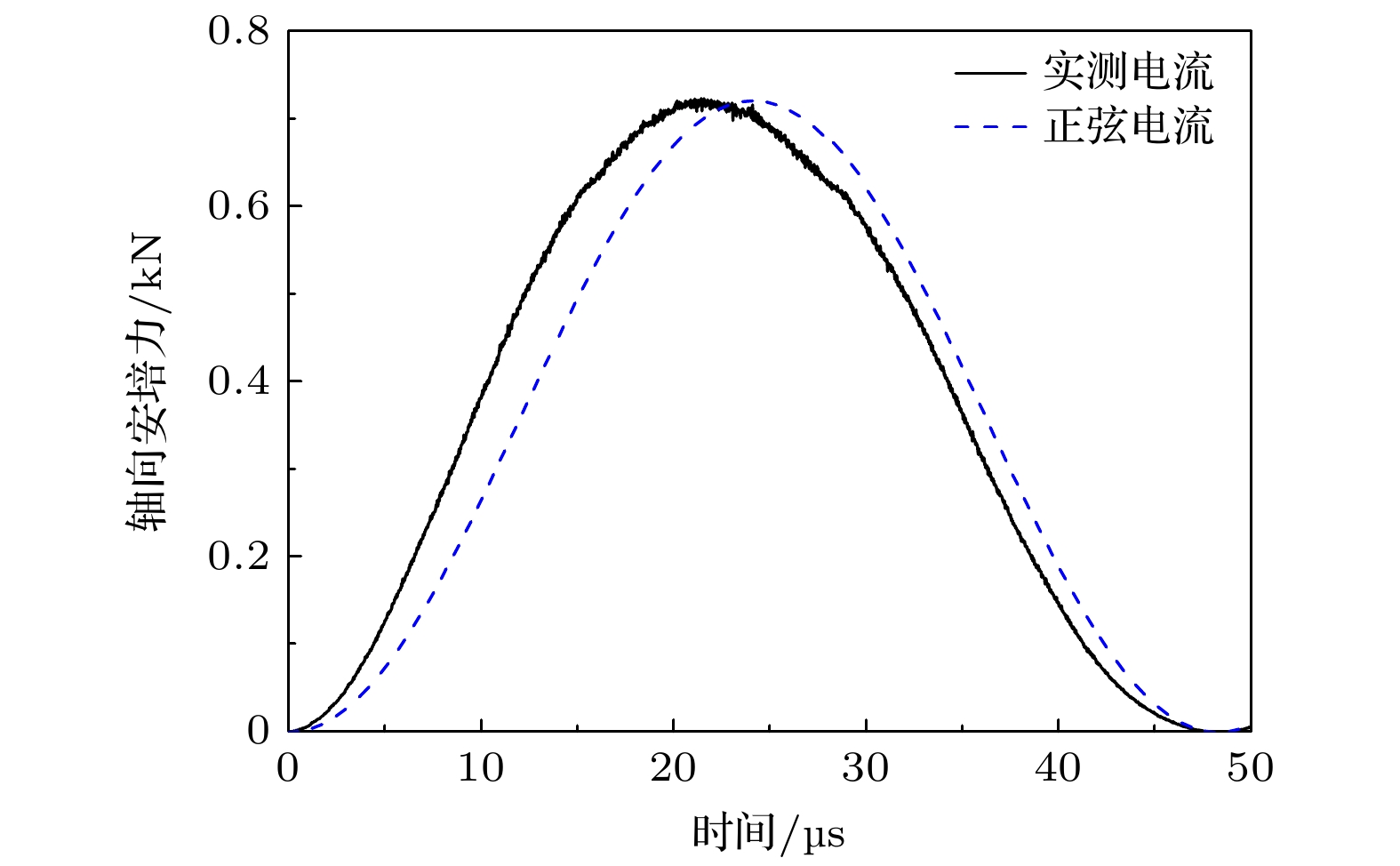
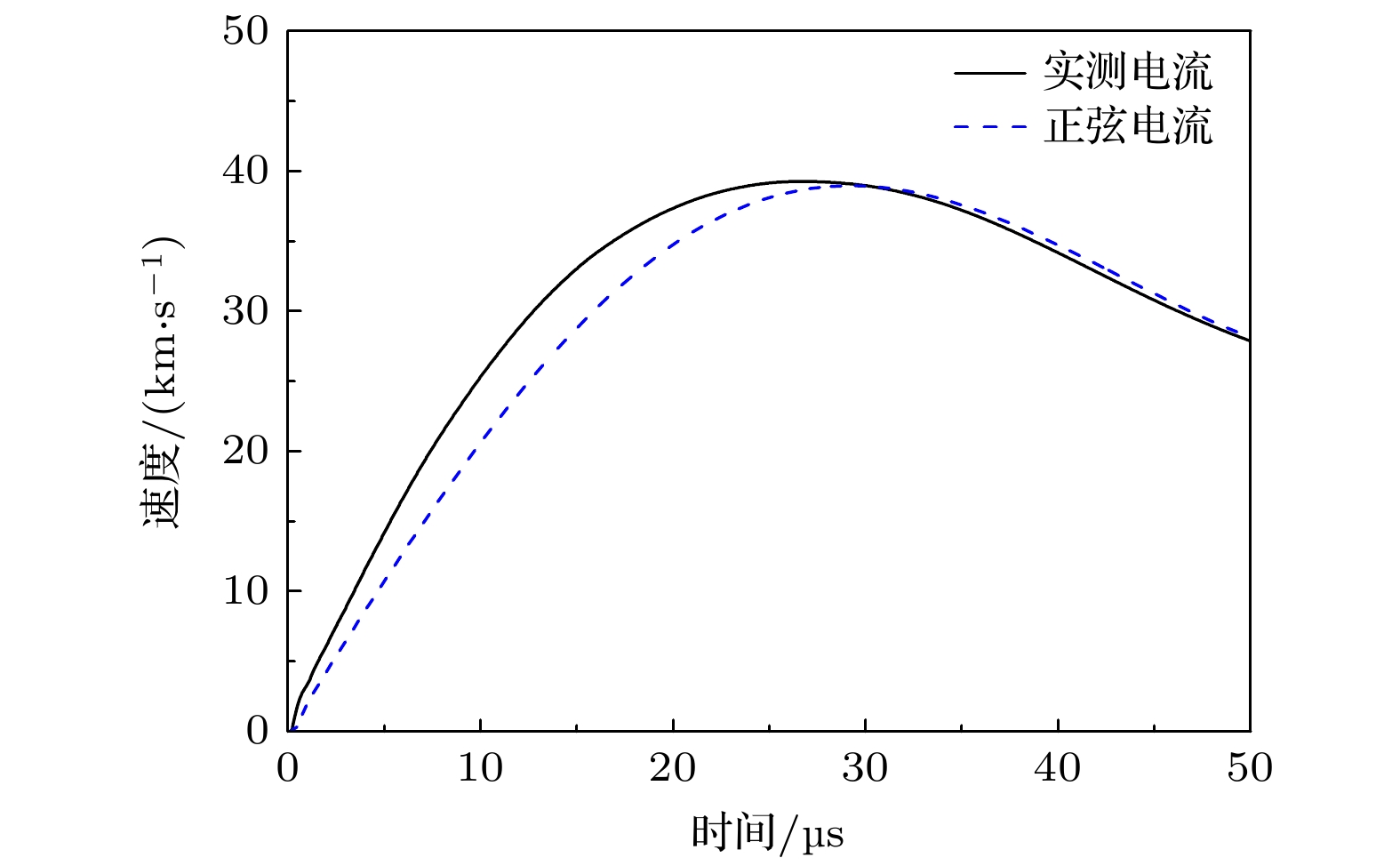
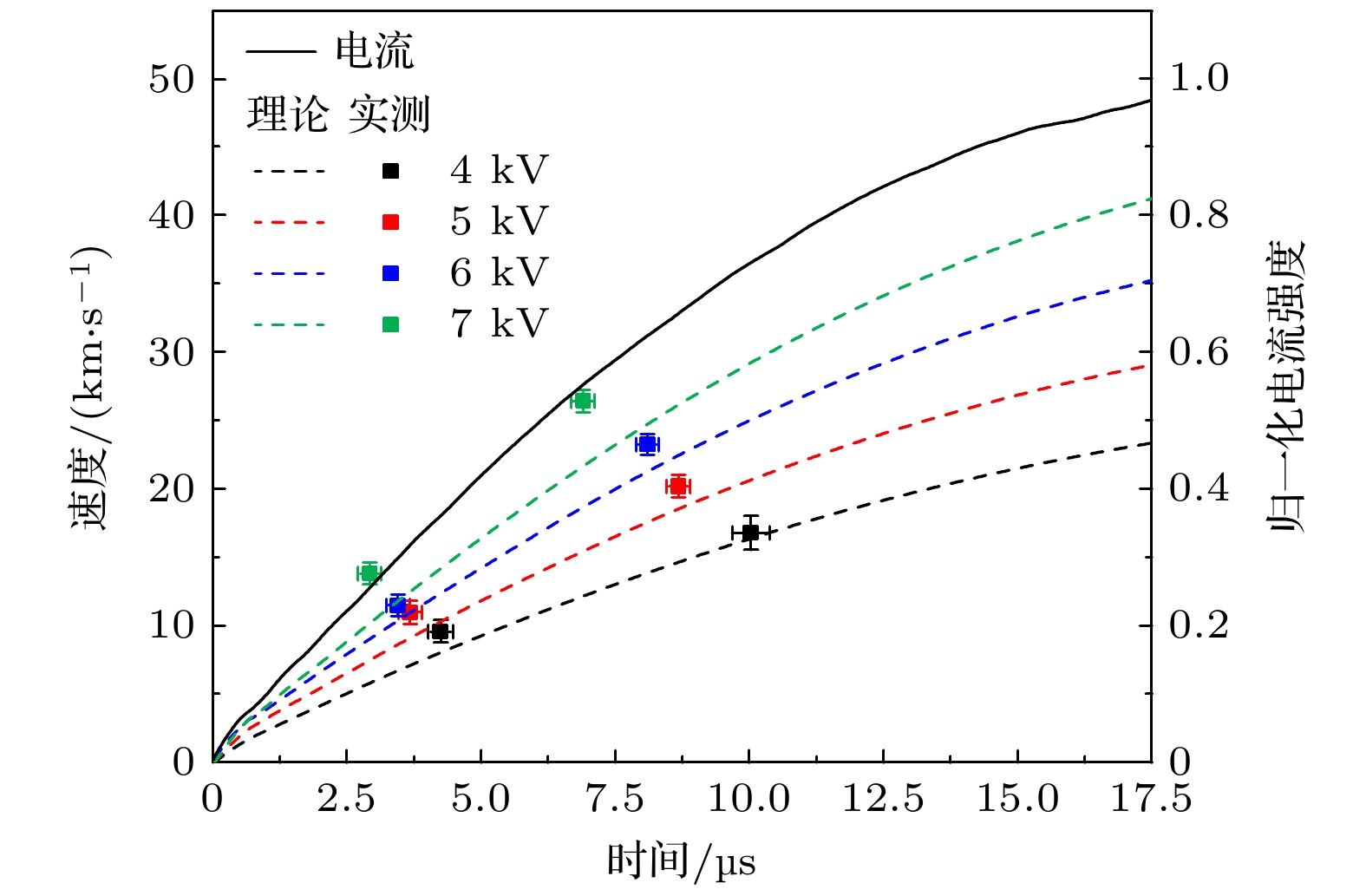
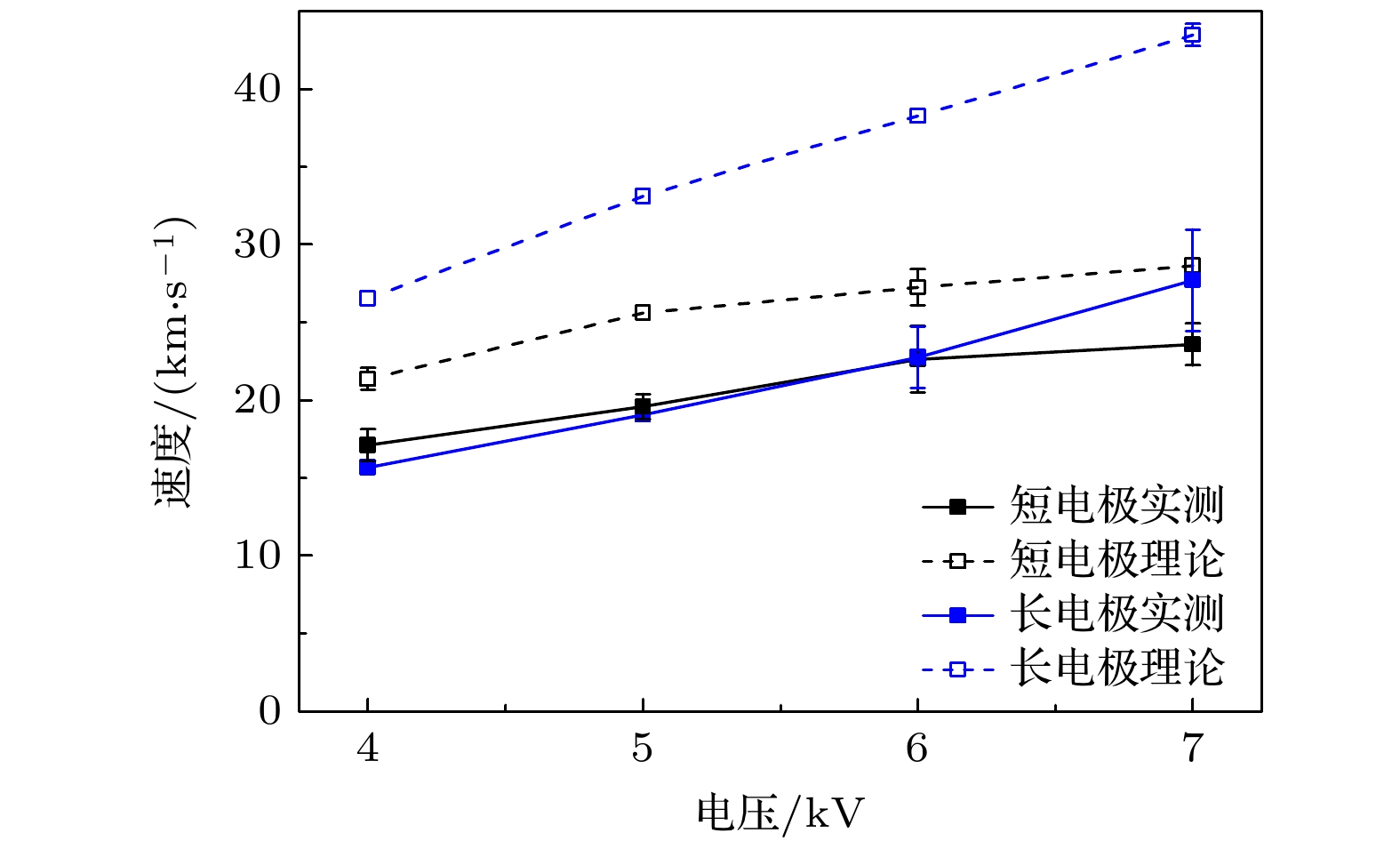
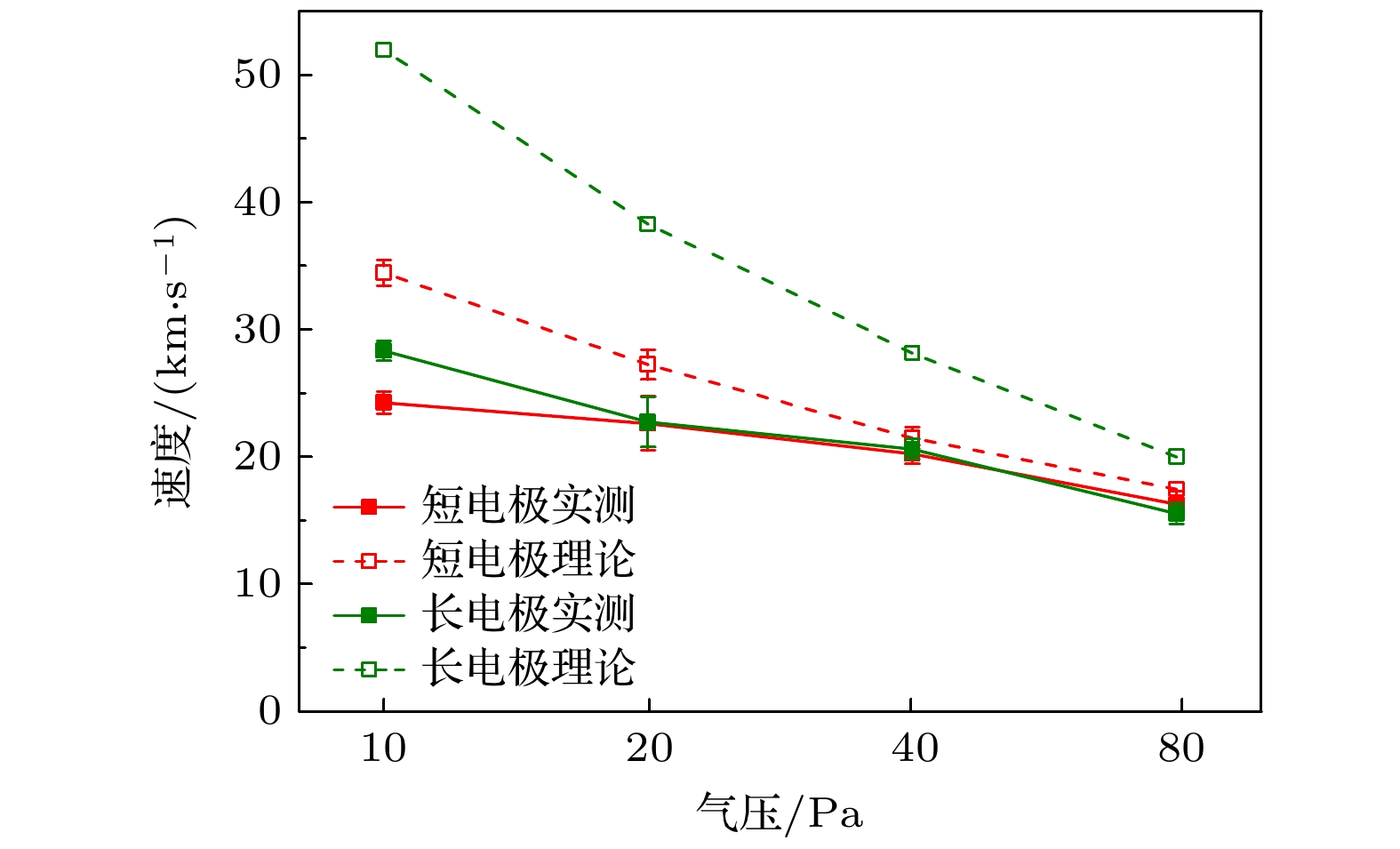
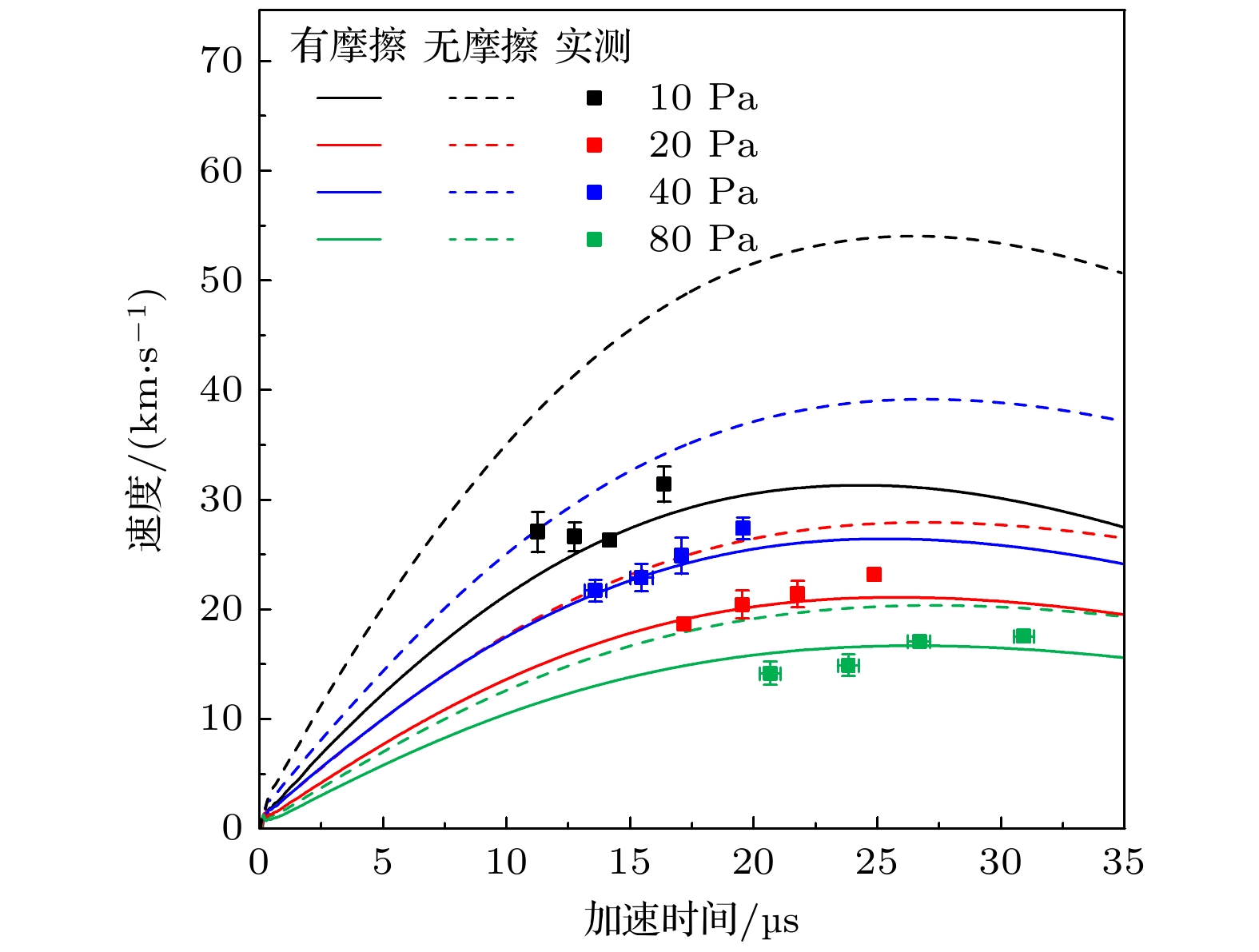
-
雪犁模型是描述预填充模式下同轴枪放电装置加速过程的主要手段, 其能够直接给出枪内等离子体轴向速度与加速时间的解析关系式, 因此在面向不同应用需求的装置结构设计中成为了重要的参考依据. 通过理论推导及光、电、磁信号的测量, 本文对比分析了不同充电电压与预填充气压条件下在加速阶段及喷口处等离子体的理论与实验速度, 并给出了雪犁模型的优化方法. 首先, 用实测电流来替代近似的正弦电流, 有效消除了磁压力计算误差对模型准确性的影响. 其次, 随着加速距离的延长, 喷口处等离子体速度的饱和现象开始出现, 摩擦阻力的存在是其主导的形成原因. 相比于现有模型25.8%—53.6%的偏差范围, 添加摩擦阻力项后不同电压和气压下的理论与实测速度的差值仅为3.1%—8.4%.Snowplow model is the main method to describe the acceleration process of coaxial plasma gun in the pre-fill mode, which can directly give the analytical expression of plasma velocity versus time. So it has become an important reference in designing the device structures satisfying the requirements for different applications. Through measuring the current, magnetic and optical signals, the characteristics of current during the discharge and the motion of plasmoid are investigated. The variation of discharge current with time is close to the damping sine curve, which is an underdamping solution of RLC equivalent discharge circuit. The measured current in lieu of the sine one is used to calculate the theoretical velocity so as to eliminate the error of magnetic pressure. The variation of plasma velocity with discharge voltage and chamber pressure are consistent with those obtained by solving the equation of plasma motion under snow plough model, but the acceleration process is another story. At the initial stage of the discharge, owing to the low sweep efficiency, the plasma is accelerated to a higher velocity than the predicted one, the increase of voltage and the decrease of pressure further enhance the effect. With the extension of the acceleration distance, owing to the friction resistance between plasma and electrodes, the acceleration slows down and the velocity starts to fall below the predicated value, the saturation of plasma velocity at the nozzle is found. The friction resistance term is added to the equation of plasma motion. Compared with the deviation range of 26.8%–53.6% of the existing model, the differences between the theoretical speed and the measured speed under different voltages and pressures are in a range of 3.1%–8.4% after adding the friction resistance term into the equation of plasma motion. The optimization of snow plow model greatly improves the accuracy of velocity prediction, which can provide an effective reference for designing device structure and calculating energy efficiency.
-
Keywords:
- coaxial gun /
- snow plough model /
- plasma velocity /
- friction resistance
[1] Marshall J 1960 Phys. Fluids 3 134
Google Scholar
[2] Ticoş C M, Wang Z, Wurden G A, Kline J L, Montgomery D S 2008 Phys. Plasmas 15 103701
Google Scholar
[3] Ticoş C M, Wang Z, Wurden G A, Kline J L, Montgomery D S, Dorf L A, Shukla P K 2008 Phys. Rev. Lett. 100 155002
Google Scholar
[4] Cheng D Y 1971 AIAA J. 9 1681
Google Scholar
[5] Lu M F, Yang S Z, Liu C Z 1997 IEEE International Conference on Plasma Science San Diego, CA, USA, May 19–22, 1997 p175
[6] Matsumoto T, Sekiguchi J, Asai T, Gota H, Garate E, Allfrey I, Valentine T, Morehouse M, Roche T, Kinley J, Aefsky S, Cordero M, Waggoner W, Binderbauer M, Tajima T 2016 Rev. Sci. Instrum. 87 053512
Google Scholar
[7] Kikuchi Y, Nakanishi R, Nakatsuka M, Fukumoto N, Nagata M 2009 IEEE Trans. Plasma Sci. 38 232
Google Scholar
[8] Federici G, Zhitlukhin A, Arkhipov N, Giniyatulin R, Klimov N, Landman I, Podkovyrov V, Safronov V, Loarte A, Merola M 2005 J. Nucl. Mater. 337 684
Google Scholar
[9] Borthakur S, Talukdar N, Neog N K, Borthakur T K 2017 Fusion Eng. Des. 122 131
Google Scholar
[10] 黄建国, 韩建伟, 李宏伟, 蔡明辉, 李小银, 张振龙, 陈赵峰, 王龙, 杨宣宗, 冯春华 2009 科学通报 02 150
Huang J G, Han J W, Lee H W, Cai M H, Lee X Y, Zhang Z L, Chen Z F, Wang L, Yang X Z, Feng C H 2009 Chin. Sci. Bull. 02 150
[11] Cassibry J T, Thio Y C F, Markusic T E, Wu S T 2006 J. Propul. Power 22 628
Google Scholar
[12] Cassibry J T 2008 IEEE Trans. Plasma Sci. 36 2180
Google Scholar
[13] 彭志坚 2004 博士学位论文 (北京: 清华大学)
Peng Z J 2004 Ph. D. Dissertation (Beijing: Tsinghua University) (in Chinese)
[14] Kikuchi Y, Nishijima D, Nakatsuka M, Ando K, Higashi T, Ueno Y, Ishihara M, Shoda K, Nagata M, Kawai T, Ueda Y, Fukumoto N, Doerner R P 2011 J. Nucl. Mater. 415 55
[15] Sakuma I, Kikuchi Y, Kitagawa Y, Asai Y, Onishi K, Fukumoto N, Nagata M 2015 J. Nucl. Mater. 463 233
Google Scholar
[16] Abdou A E, Ismail M I, Mohamed A E, Lee S, Saw S H, Verma R 2012 IEEE Trans. Plasma Sci. 40 2741
Google Scholar
[17] Raman R, Martin, F, Quirion B, St-Onge M, Lachambre J L, Michaud D, Sawatzky B, Thomas J, Hirose A, Hwang D, Richard N, Cote C, Abel G, Pinsonneault D, Gauvreau J L, Stansfield B, Decoste R, Cote A, Zuzak W, Bouche C 1994 Phys. Rev. Lett. 73 3101
Google Scholar
[18] Francis Thio Y C, Hsu S C, Witherspoon F D, Cruz E, Case A, Langendorf S, Yates K, Dunn J, Cassibry J, Samulyak R, Stoltz P, Brockington S J, Williams A, Luna M, Becker R, Cook A 2019 Fusion Sci. Technol. 75 581
Google Scholar
[19] Ticos C M, Wang Z, Wurden G A 2008 IEEE Trans. Plasma Sci. 36 2770
Google Scholar
[20] Nawaz A, Albertoni R, Auweter-Kurtz M 2010 Acta Astronaut. 67 440
Google Scholar
[21] Asai T, Matsumoto T, Roche T, Allfrey I, Gota H, Sekiguchi J, Edo T, Garate E, Takahashi T, Binderbauer M, Tajima T 2017 Nucl. Fusion 57 076018
Google Scholar
[22] Raman R 2008 Fusion Eng. Des. 83 1368
Google Scholar
[23] Hart P J 1962 Phys. Fluids. 5 38
Google Scholar
[24] Burkhardt L C, Lovberg R H 1962 Phys. Fluids. 5 341
Google Scholar
[25] Chow S P, Lee S, Tan B C 1972 J. Plasma Phys. 8 21
Google Scholar
[26] Chen Y H, Lee S 1973 Int. J. Electron. 35 341
Google Scholar
[27] Shen Z G, Liu C H, Lee C H, Wu C, Yang S 1995 J. Phys. 28 314
Google Scholar
[28] 张俊龙, 杨亮, 闫慧杰, 滑跃, 任春生 2015 64 075201
Google Scholar
Zhang J L, Yang L, Yan H J, Hua Y, Ren C S 2015 Acta Phys. Sin. 64 075201
Google Scholar
[29] 余鑫, 漆亮文, 赵崇霄, 任春生 2020 69 035202
Google Scholar
Yu X, Qi L W, Zhao C X, Ren C S 2020 Acta Phys. Sin. 69 035202
Google Scholar
[30] Dietz D 1987 J. Appl. Phys. 62 2669
Google Scholar
[31] Chen F F 2016 Introduction to Plasma Physics and Controlled Fusion (Cham: Springer) pp166–171
[32] 宋健, 李嘉雯, 白晓东, 张津硕, 闫慧杰, 肖青梅, 王德真 2021 70 105201
Google Scholar
Song J, Lee J W, Bai X D, Zhang J S, Yan H J, Xiao Q M, Wang D Z 2021 Acta Phys. Sin. 70 105201
Google Scholar
[33] Wiechula J, Hock C, Iberler M, Manegold T, Schonlein A, Jacoby J 2015 Phys. Plasmas 22 043516
Google Scholar
[34] 刘帅 2019 博士学位论文 (西安: 西安交通大学)
Liu S 2019 Ph. D. Dissertation (Xi’an: Xi’an Jiaotong University) (in Chinese)
[35] Thom K, Norwood J, Jalufka N 1964 Phys. Fluids 7 67
Google Scholar
-
图 2 同轴枪放电的典型电流、磁信号及光信号波形(充电电压6 kV, 预填充气压20 Pa)
Fig. 2. Typical electrical, magnetic and optical signals of discharge in a coaxial gun (V = 6 kV and P = 20 Pa).
图 4 电流实测波形与理论计算波形对比
Fig. 4. Comparison of the measured and the theoretical current signals.
图 5 气压20 Pa时, 不同电压下等离子体(a)轴向位置和(b)速度随加速时间的变化
Fig. 5. (a) Axial position and (b) velocity of plasma versus acceleration time with different voltage at 20 Pa.
图 6 电压6 kV时, 不同气压下等离子体(a)轴向位置和(b)速度随加速时间的变化
Fig. 6. (a) Axial position and (b) velocity of plasma versus acceleration time with different pressure at 6 kV.
图 7 实测和正弦电流下的轴向安培力对比
Fig. 7. Comparison of ampere force calculated by measured and sinusoidal current.
图 8 实测和正弦电流下的理论速度对比
Fig. 8. Comparison of theoretical velocity calculated by measured and sinusoidal current.
图 9 气压20 Pa时, 不同电压下等离子体速度随加速时间的变化
Fig. 9. Plasma velocity versus acceleration time with different voltage at 20 Pa
图 10 电压6 kV时, 不同气压下等离子体速度随加速时间的变化
Fig. 10. Plasma velocity versus acceleration time with different pressure at 6 kV.
图 11 气压20 Pa时, 等离子体枪口速度随电压的变化
Fig. 11. Muzzle velocity of plasma jet versus voltage at 20 Pa.
图 12 电压6 kV时, 等离子体枪口速度随气压的变化
Fig. 12. Muzzle velocity of plasma jet versus pressure at 6 kV.
图 13 气压20 Pa时, 加速饱和阶段等离子体速度随加速时间的变化
Fig. 13. Plasma velocity versus acceleration time in saturation stage at 20 Pa.
图 14 电压6 kV时, 加速饱和阶段等离子体速度随加速时间的变化
Fig. 14. Plasma velocity versus acceleration time in saturation stage at 6 kV.
-
[1] Marshall J 1960 Phys. Fluids 3 134
Google Scholar
[2] Ticoş C M, Wang Z, Wurden G A, Kline J L, Montgomery D S 2008 Phys. Plasmas 15 103701
Google Scholar
[3] Ticoş C M, Wang Z, Wurden G A, Kline J L, Montgomery D S, Dorf L A, Shukla P K 2008 Phys. Rev. Lett. 100 155002
Google Scholar
[4] Cheng D Y 1971 AIAA J. 9 1681
Google Scholar
[5] Lu M F, Yang S Z, Liu C Z 1997 IEEE International Conference on Plasma Science San Diego, CA, USA, May 19–22, 1997 p175
[6] Matsumoto T, Sekiguchi J, Asai T, Gota H, Garate E, Allfrey I, Valentine T, Morehouse M, Roche T, Kinley J, Aefsky S, Cordero M, Waggoner W, Binderbauer M, Tajima T 2016 Rev. Sci. Instrum. 87 053512
Google Scholar
[7] Kikuchi Y, Nakanishi R, Nakatsuka M, Fukumoto N, Nagata M 2009 IEEE Trans. Plasma Sci. 38 232
Google Scholar
[8] Federici G, Zhitlukhin A, Arkhipov N, Giniyatulin R, Klimov N, Landman I, Podkovyrov V, Safronov V, Loarte A, Merola M 2005 J. Nucl. Mater. 337 684
Google Scholar
[9] Borthakur S, Talukdar N, Neog N K, Borthakur T K 2017 Fusion Eng. Des. 122 131
Google Scholar
[10] 黄建国, 韩建伟, 李宏伟, 蔡明辉, 李小银, 张振龙, 陈赵峰, 王龙, 杨宣宗, 冯春华 2009 科学通报 02 150
Huang J G, Han J W, Lee H W, Cai M H, Lee X Y, Zhang Z L, Chen Z F, Wang L, Yang X Z, Feng C H 2009 Chin. Sci. Bull. 02 150
[11] Cassibry J T, Thio Y C F, Markusic T E, Wu S T 2006 J. Propul. Power 22 628
Google Scholar
[12] Cassibry J T 2008 IEEE Trans. Plasma Sci. 36 2180
Google Scholar
[13] 彭志坚 2004 博士学位论文 (北京: 清华大学)
Peng Z J 2004 Ph. D. Dissertation (Beijing: Tsinghua University) (in Chinese)
[14] Kikuchi Y, Nishijima D, Nakatsuka M, Ando K, Higashi T, Ueno Y, Ishihara M, Shoda K, Nagata M, Kawai T, Ueda Y, Fukumoto N, Doerner R P 2011 J. Nucl. Mater. 415 55
[15] Sakuma I, Kikuchi Y, Kitagawa Y, Asai Y, Onishi K, Fukumoto N, Nagata M 2015 J. Nucl. Mater. 463 233
Google Scholar
[16] Abdou A E, Ismail M I, Mohamed A E, Lee S, Saw S H, Verma R 2012 IEEE Trans. Plasma Sci. 40 2741
Google Scholar
[17] Raman R, Martin, F, Quirion B, St-Onge M, Lachambre J L, Michaud D, Sawatzky B, Thomas J, Hirose A, Hwang D, Richard N, Cote C, Abel G, Pinsonneault D, Gauvreau J L, Stansfield B, Decoste R, Cote A, Zuzak W, Bouche C 1994 Phys. Rev. Lett. 73 3101
Google Scholar
[18] Francis Thio Y C, Hsu S C, Witherspoon F D, Cruz E, Case A, Langendorf S, Yates K, Dunn J, Cassibry J, Samulyak R, Stoltz P, Brockington S J, Williams A, Luna M, Becker R, Cook A 2019 Fusion Sci. Technol. 75 581
Google Scholar
[19] Ticos C M, Wang Z, Wurden G A 2008 IEEE Trans. Plasma Sci. 36 2770
Google Scholar
[20] Nawaz A, Albertoni R, Auweter-Kurtz M 2010 Acta Astronaut. 67 440
Google Scholar
[21] Asai T, Matsumoto T, Roche T, Allfrey I, Gota H, Sekiguchi J, Edo T, Garate E, Takahashi T, Binderbauer M, Tajima T 2017 Nucl. Fusion 57 076018
Google Scholar
[22] Raman R 2008 Fusion Eng. Des. 83 1368
Google Scholar
[23] Hart P J 1962 Phys. Fluids. 5 38
Google Scholar
[24] Burkhardt L C, Lovberg R H 1962 Phys. Fluids. 5 341
Google Scholar
[25] Chow S P, Lee S, Tan B C 1972 J. Plasma Phys. 8 21
Google Scholar
[26] Chen Y H, Lee S 1973 Int. J. Electron. 35 341
Google Scholar
[27] Shen Z G, Liu C H, Lee C H, Wu C, Yang S 1995 J. Phys. 28 314
Google Scholar
[28] 张俊龙, 杨亮, 闫慧杰, 滑跃, 任春生 2015 64 075201
Google Scholar
Zhang J L, Yang L, Yan H J, Hua Y, Ren C S 2015 Acta Phys. Sin. 64 075201
Google Scholar
[29] 余鑫, 漆亮文, 赵崇霄, 任春生 2020 69 035202
Google Scholar
Yu X, Qi L W, Zhao C X, Ren C S 2020 Acta Phys. Sin. 69 035202
Google Scholar
[30] Dietz D 1987 J. Appl. Phys. 62 2669
Google Scholar
[31] Chen F F 2016 Introduction to Plasma Physics and Controlled Fusion (Cham: Springer) pp166–171
[32] 宋健, 李嘉雯, 白晓东, 张津硕, 闫慧杰, 肖青梅, 王德真 2021 70 105201
Google Scholar
Song J, Lee J W, Bai X D, Zhang J S, Yan H J, Xiao Q M, Wang D Z 2021 Acta Phys. Sin. 70 105201
Google Scholar
[33] Wiechula J, Hock C, Iberler M, Manegold T, Schonlein A, Jacoby J 2015 Phys. Plasmas 22 043516
Google Scholar
[34] 刘帅 2019 博士学位论文 (西安: 西安交通大学)
Liu S 2019 Ph. D. Dissertation (Xi’an: Xi’an Jiaotong University) (in Chinese)
[35] Thom K, Norwood J, Jalufka N 1964 Phys. Fluids 7 67
Google Scholar
下载:
计量
- 文章访问数: 2582
- PDF下载量: 64
- 被引次数: 0
