基于Fokker-Planck-Landau碰撞模型的非均匀尘埃等离子体目标散射特性

陈伟; 黄海; 杨利霞; 薄勇; 黄志祥

doi:10.7498/aps.72.20222113

摘要

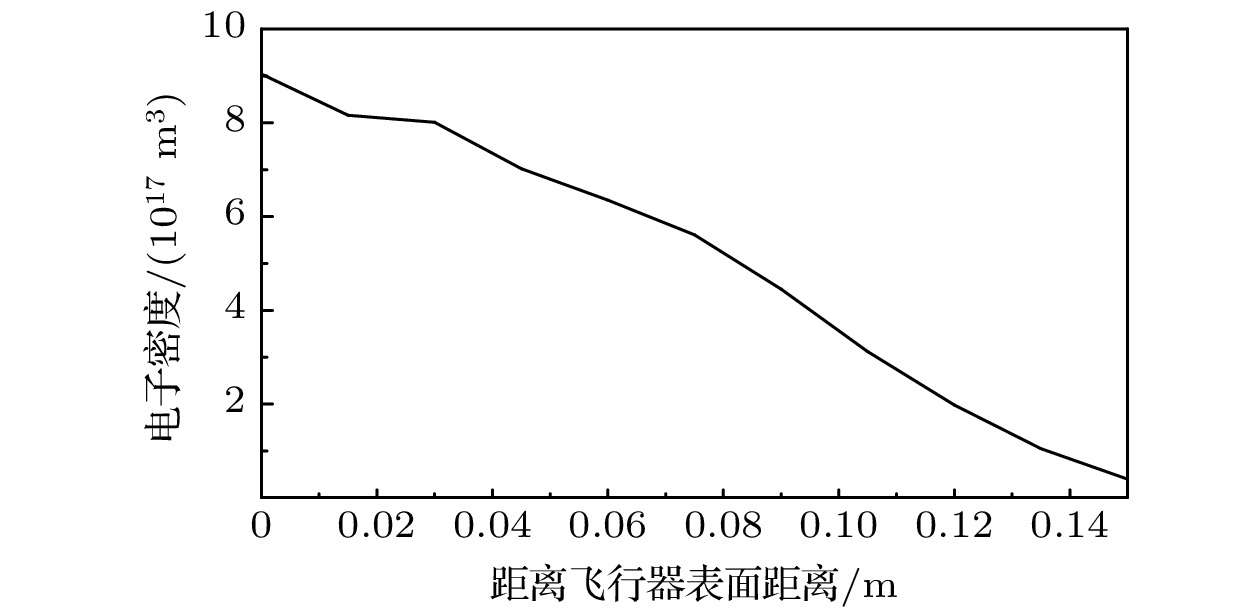
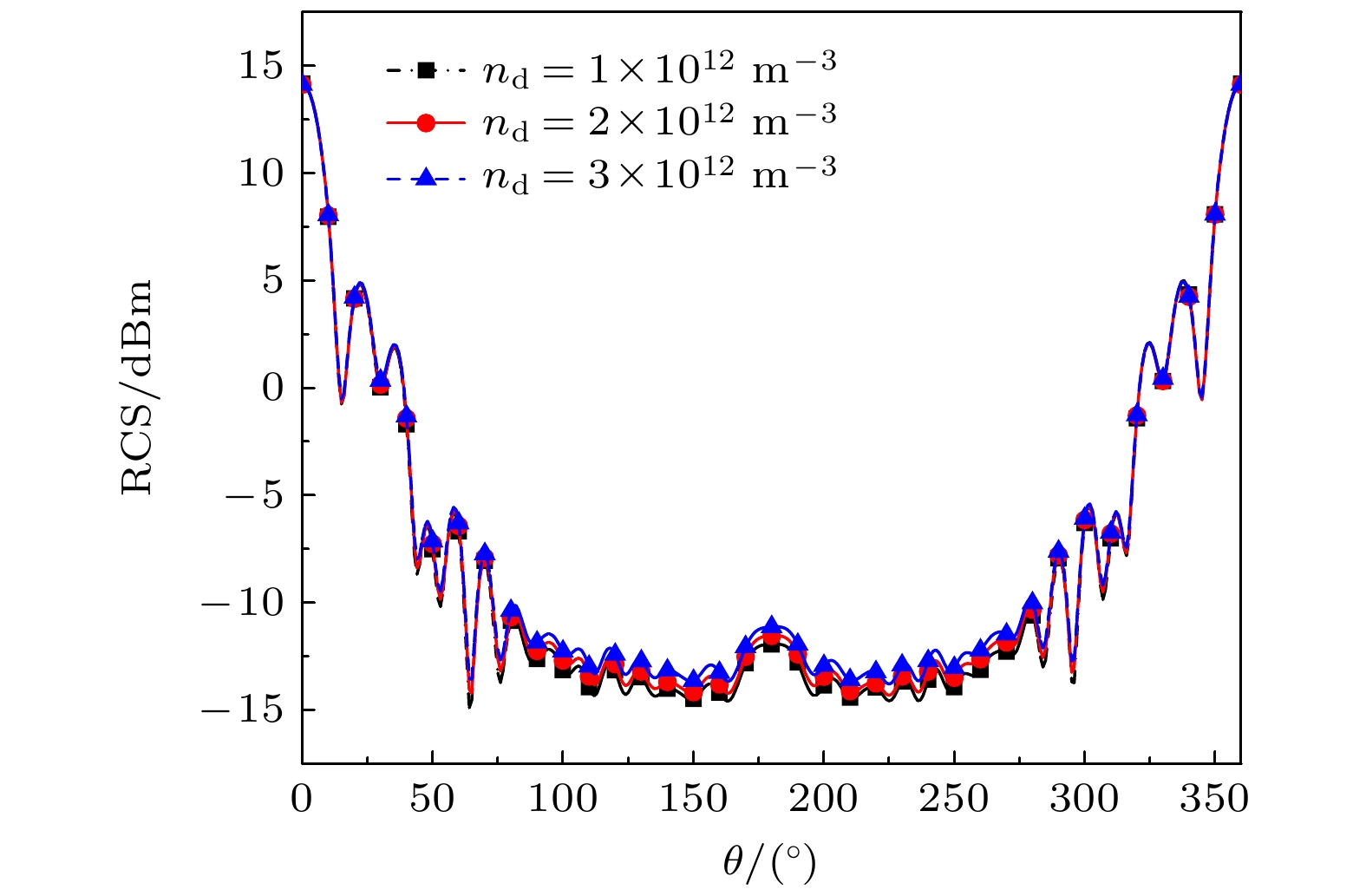
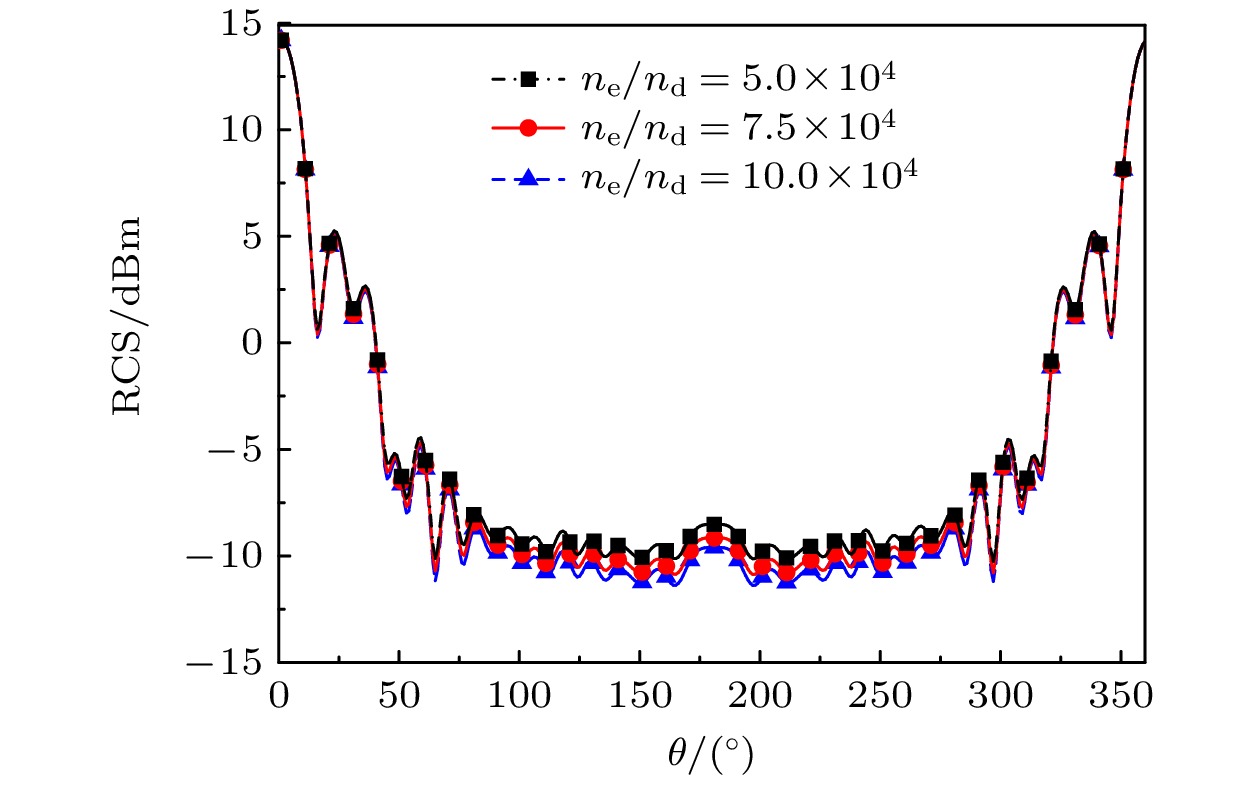
从玻尔兹曼方程出发, 推导了全电离非均匀尘埃等离子体的Fokker-Planck-Landau碰撞模型下时域有限差分的迭代表达式. 结合尘埃等离子体的碰撞效应和充电效应, 得到了Fokker-Planck-Landau碰撞模型下全电离尘埃等离子体的电导率表达式. 利用Z变换时域有限差分(Z-FDTD)法计算了二维情况下尘埃等离子体涂覆金属钝头锥的雷达散射截面. 分析了尘埃粒子密度、尘埃粒子半径、电子密度与尘埃粒子密度比值、尘埃粒子充电频率以及电磁波的入射角对钝头锥散射特性的影响. 结果表明, 在全电离空间非均匀尘埃等离子体中, 随着尘埃粒子半径的增大会削弱德拜屏蔽效应, 导致雷达散射截面增大; 此外, 受到尘埃等离子体的碰撞效应和充电效应产生影响, 会对目标的雷达散射截面有较大的影响. 这些结果对研究空间非均匀尘埃等离子体的电磁波散射特性以及近地空间的通信问题提供了理论依据.

关键词:

Abstract

Dusty plasma is a multi-particle system of dust particles suspended in plasma, which is generally composed of free electrons, ions, and dust particles. It is widely found in natural space and aerospace equipment, such as the Earth’s ionosphere, rocket tail flame, and sheath of the hypersonic vehicle. The dust particles will interact with free electrons and ions in the plasma so that the dust particles are charged. They also significantly change the characteristics of dusty plasma, showing some phenomena different from those in ordinary plasma, such as dust acoustic solitary waves and dust void. Electromagnetic (EM) waves will interact with dusty plasma, which results in the attenuation of EM wave signal and the change of phase and other serious effects, and the phenomenon of “blackout” appears. This is very unfavorable for the guidance and control system of the vehicle. The generation of “blackout” is related to the dynamics of dusty plasma and the analysis and research of EM characteristics, so studying the scattering characteristics of dusty plasma is of great significance. First, starting from the Boltzmann equation, the iterative expression of finite-difference time-domain under the Fokker-Planck-Landau (FPL) collision model of fully ionized non-uniform dusty plasma is derived. The expression of the conductivity of the fully ionized dusty plasma under the FPL collision model is obtained by combining the collision effect and charging effect of the dusty plasma. By using the Z-transform finite-difference time-domain method, the radar cross section (RCS) of the dusty plasma coated metal blunt cone in two dimensions is calculated. The effects of dust particle density, dust particle radius, ratio of electron density to dust particle density, dust particle charging frequency, and EM wave incident angle on the scattering characteristics of the mental blunt cone are analyzed. The results show that the Debye shielding effect is weakened and RCS is increased with the increase of the radius of dust particles in the fully ionized non-uniform dusty plasma. In addition, it is affected by the collision effect and charging effect of dusty plasma, which will have a great influence on the RCS of the target. These results provide a theoretical basis for studying the EM waves scattering problem of fully ionized non-uniform dusty plasma and the communication problems in near space.

Keywords:

Boltzmann equation /
dusty plasma /
Z-transform finite-difference time-domain method /
radar cross section

作者及机构信息

1.
安徽大学电子信息工程学院, 合肥　230601

2.
先进激光技术安徽省实验室, 合肥　230037

通信作者: 杨利霞, lixiayang@yeah.net

基金项目: 国家自然科学基金 (批准号: U21A20457, 61901004, 61871457, 62071003, 62071353)、先进激光技术安徽省实验室 (批准号: AHL2021KF04)、目标探测与特征提取安徽省重点实验室 (批准号: TRFE2021A001)和安徽省高校协同创新项目(批准号: GXXT-2020-050, GXXT-2021-028)资助的课题.

Authors and contacts

1.
School of Electronic and Information Engineering, Anhui University, Hefei 230601, China

2.
Advanced Laser Technology Laboratory of Anhui Province, Hefei 230037, China

Corresponding author: Yang Li-Xia, lixiayang@yeah.net

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. U21A20457, 61901004, 61871457, 62071003, 62071353), the Open Research Fund of Advanced Laser Technology Laboratory of Anhui Province, China (Grant No. AHL2021KF04), the Open Research Fund of Anhui Province Key Laboratory of Target Recognition and Feature Extraction, China (Grant No. TRFE2021A001), and the University Synergy Innovation of Program of Anhui Province, China (Grant Nos. GXXT-2020-050, GXXT-2021-028).

文章全文

参考文献

[1]	孙俊国, 张宗国, 董焕河, 杨红卫 2019 68 210201 Google Scholar Sun J G, Zhang Z G, Dong H H, Yang H W 2019 Acta Phys. Sin. 68 210201 Google Scholar
[2]	Wang Z Y, Guo L X, Li J T 2021 Chin. Phys. B 30 045203 Google Scholar
[3]	Yan J, Feng F, Liu F C, Dong L F, He Y F 2016 Chin. Phys. B 25 095202 Google Scholar
[4]	林麦麦, 付颖捷, 宋秋影, 于腾萱, 文惠珊, 蒋蕾 2022 71 095203 Google Scholar Lin M M, Fu Y J, Song Q Y, Yu T X, Wen H S, Jiang L 2022 Acta Phys. Sin. 71 095203 Google Scholar
[5]	黄峰, 叶茂福, 王龙 2004 科学通报 49 2150 Google Scholar Huang F, Ye M F, Wang L 2004 Chin. Sci. Bull. 49 2150 Google Scholar
[6]	崔平远, 窦强, 高艾 2014 宇航学报 35 1 Google Scholar Cui P Y, Dou Q, Gao A 2014 J. Astr. 35 1 Google Scholar
[7]	Tsytovich V N 2015 Contrib. Plasma Phys. 55 664 Google Scholar
[8]	Sotnikov V I, Leboeuf J N, Mudaliar S 2010 IEEE Trans. Plasma Sci. 38 2208 Google Scholar
[9]	Motie I, Bokaeeyan M 2015 Phys. Plasmas 22 023707 Google Scholar
[10]	金铭, 韦笑, 吴洋, 张羽淮, 余西龙 2015 64 205205 Google Scholar Jin M, Wei X, Wu Y, Zhang Y H, Yu X L 2015 Acta Phys. Sin. 64 205205 Google Scholar
[11]	Guo L X, Chen W, LI J T, Ren Y, Liu S H 2018 Phys. Plasmas 25 053707 Google Scholar
[12]	Dan L, Guo L X, Li J T 2018 Phys. Plasmas 25 013707 Google Scholar
[13]	Dan L, Gou L X, Li J T, Chen W, Yan X, Huang Q Q 2017 Phys. Plasmas 24 093703 Google Scholar
[14]	Mousseau V A, Knoll D A 1997 J. Comput. Phys. 136 308 Google Scholar
[15]	Li H, Wu J, Zhou Z, Yuan C 2016 Phys. Plasmas 23 073702 Google Scholar
[16]	Bendib A, Bendib-Kalache K, Cros B, Maynard G 2016 Phys. Rev. E 93 043208 Google Scholar
[17]	Bendib A, Bendib K, Sid A 1997 Phys. Rev. E 55 7522 Google Scholar
[18]	Jia J S, Yuan C X, Liu S, Yue F, Gao R L, Wang Y, Zhou Z X, Wu J, Li H 2016 Phys. Plasmas 23 043302 Google Scholar
[19]	葛德彪, 闫玉波 2011 电磁波时域有限差分方法 (第三版) (西安: 西安电子科技大学出版社) 第259页 Ge D B, Yan Y B 2011 Finite-Difference Time-Domain Method for Electromagnetic Waves (3rd Ed.) (Xi’an: Xidian University Press) p259 (in Chinese)
[20]	魏兵, 葛德彪, 王飞 2008 57 6290 Google Scholar Wei B, Ge D B, Wang F 2008 Acta Phys. Sin. 57 6290 Google Scholar
[21]	Ren Y, Guo L X, Chen W, Liu S H 2018 Phys. Plasmas 25 093515 Google Scholar
[22]	王飞, 魏兵 2013 62 084106 Google Scholar Wang F, Wei B 2013 Acta Phys. Sin. 62 084106 Google Scholar
[23]	Demarest K, Huang Z B, Plumb R 1996 IEEE Trans. Antennas Propag. 44 1150 Google Scholar
[24]	Britt C L 1989 IEEE Trans. Antennas Propag. 37 1181 Google Scholar
[25]	Zhang H F, Li J T, Bian Z, Guo L X 2022 IEEE Trans. Plasma Sci. 50 3200 Google Scholar
[26]	Liu S H, Zhu C K, Guo L X, Li J T, Dan L, Wang Z Y 2020 Phys. Plasmas 27 023701 Google Scholar

施引文献

图 1 RAM-C钝头锥飞行器模型图

Fig. 1. Model of RAM-C blunt cone vehicle.

下载: 全尺寸图片幻灯片

图 2 空间中电子密度分布

Fig. 2. Electron density distribution in space.

下载: 全尺寸图片幻灯片

图 3 未涂覆等离子体、等离子体涂覆以及尘埃等离子体涂覆的RCS

Fig. 3. RCS for without the plasma, coated with the plasma, and coated with the dusty plasma.

下载: 全尺寸图片幻灯片

图 4 不同尘埃粒子密度下的RCS

Fig. 4. RCS at different dust particle densities.

下载: 全尺寸图片幻灯片

图 5 不同尘埃粒子半径下的RCS

Fig. 5. RCS at different dust particle radii.

下载: 全尺寸图片幻灯片

图 6 不同电子密度与尘埃粒子密度比值下的RCS

Fig. 6. RCS at different ratios of electron density to dust particle density.

下载: 全尺寸图片幻灯片

图 7 不同充电频率的RCS

Fig. 7. RCS at different charging frequencies.

下载: 全尺寸图片幻灯片

图 8 不同电磁波入射角度下的RCS

Fig. 8. RCS at different incidence angles of EM waves.

下载: 全尺寸图片幻灯片

表 1 等离子体与尘埃等离子体相关参数

Table 1. Related parameters of plasma and dusty plasma.

参数等离子体尘埃等离子体

$n_{\text{d}}/(10^{12}~\text{m}^{-3})$ 0 1
$r_{\text{d}} /{\text{μ}{\rm m}}$ 0 5
$T_{\rm e}/(10^{4}~\text{K})$ 1 1
${\varphi /{\left( {^\circ } \right)} }$ 0 0

下载: 导出CSV

表 2 全电离尘埃等离子体的计算参数

Table 2. Calculated parameters of fully ionized dusty plasma.

n_d/(10¹² m^–3) r_d/μm n_e·n_d^–1/10⁴ v_ch/(10⁹ rad·s^–1)

图4 1, 2, 3 5 10 5
图5 1 3, 4, 5 10 5
图6 1 5 5.0, 7.5, 10.0 5
图7 1 5 10 5, 10, 15

下载: 导出CSV

map

[1]	孙俊国, 张宗国, 董焕河, 杨红卫 2019 68 210201 Google Scholar Sun J G, Zhang Z G, Dong H H, Yang H W 2019 Acta Phys. Sin. 68 210201 Google Scholar
[2]	Wang Z Y, Guo L X, Li J T 2021 Chin. Phys. B 30 045203 Google Scholar
[3]	Yan J, Feng F, Liu F C, Dong L F, He Y F 2016 Chin. Phys. B 25 095202 Google Scholar
[4]	林麦麦, 付颖捷, 宋秋影, 于腾萱, 文惠珊, 蒋蕾 2022 71 095203 Google Scholar Lin M M, Fu Y J, Song Q Y, Yu T X, Wen H S, Jiang L 2022 Acta Phys. Sin. 71 095203 Google Scholar
[5]	黄峰, 叶茂福, 王龙 2004 科学通报 49 2150 Google Scholar Huang F, Ye M F, Wang L 2004 Chin. Sci. Bull. 49 2150 Google Scholar
[6]	崔平远, 窦强, 高艾 2014 宇航学报 35 1 Google Scholar Cui P Y, Dou Q, Gao A 2014 J. Astr. 35 1 Google Scholar
[7]	Tsytovich V N 2015 Contrib. Plasma Phys. 55 664 Google Scholar
[8]	Sotnikov V I, Leboeuf J N, Mudaliar S 2010 IEEE Trans. Plasma Sci. 38 2208 Google Scholar
[9]	Motie I, Bokaeeyan M 2015 Phys. Plasmas 22 023707 Google Scholar
[10]	金铭, 韦笑, 吴洋, 张羽淮, 余西龙 2015 64 205205 Google Scholar Jin M, Wei X, Wu Y, Zhang Y H, Yu X L 2015 Acta Phys. Sin. 64 205205 Google Scholar
[11]	Guo L X, Chen W, LI J T, Ren Y, Liu S H 2018 Phys. Plasmas 25 053707 Google Scholar
[12]	Dan L, Guo L X, Li J T 2018 Phys. Plasmas 25 013707 Google Scholar
[13]	Dan L, Gou L X, Li J T, Chen W, Yan X, Huang Q Q 2017 Phys. Plasmas 24 093703 Google Scholar
[14]	Mousseau V A, Knoll D A 1997 J. Comput. Phys. 136 308 Google Scholar
[15]	Li H, Wu J, Zhou Z, Yuan C 2016 Phys. Plasmas 23 073702 Google Scholar
[16]	Bendib A, Bendib-Kalache K, Cros B, Maynard G 2016 Phys. Rev. E 93 043208 Google Scholar
[17]	Bendib A, Bendib K, Sid A 1997 Phys. Rev. E 55 7522 Google Scholar
[18]	Jia J S, Yuan C X, Liu S, Yue F, Gao R L, Wang Y, Zhou Z X, Wu J, Li H 2016 Phys. Plasmas 23 043302 Google Scholar
[19]	葛德彪, 闫玉波 2011 电磁波时域有限差分方法 (第三版) (西安: 西安电子科技大学出版社) 第259页 Ge D B, Yan Y B 2011 Finite-Difference Time-Domain Method for Electromagnetic Waves (3rd Ed.) (Xi’an: Xidian University Press) p259 (in Chinese)
[20]	魏兵, 葛德彪, 王飞 2008 57 6290 Google Scholar Wei B, Ge D B, Wang F 2008 Acta Phys. Sin. 57 6290 Google Scholar
[21]	Ren Y, Guo L X, Chen W, Liu S H 2018 Phys. Plasmas 25 093515 Google Scholar
[22]	王飞, 魏兵 2013 62 084106 Google Scholar Wang F, Wei B 2013 Acta Phys. Sin. 62 084106 Google Scholar
[23]	Demarest K, Huang Z B, Plumb R 1996 IEEE Trans. Antennas Propag. 44 1150 Google Scholar
[24]	Britt C L 1989 IEEE Trans. Antennas Propag. 37 1181 Google Scholar
[25]	Zhang H F, Li J T, Bian Z, Guo L X 2022 IEEE Trans. Plasma Sci. 50 3200 Google Scholar
[26]	Liu S H, Zhu C K, Guo L X, Li J T, Dan L, Wang Z Y 2020 Phys. Plasmas 27 023701 Google Scholar

计量

文章访问数: 4783
PDF下载量: 81
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

基于Fokker-Planck-Landau碰撞模型的非均匀尘埃等离子体目标散射特性