高维宇称-时间对称系统中的信息恢复与临界性

曲登科; 范毅; 薛鹏

doi:10.7498/aps.70.20220511

摘要

近期, 满足宇称-时间对称性的非厄米系统的研究取得了令人印象深刻的进展, 如物理系统拓扑性质和奇异点处临界性的观测. 宇称-时间对称的非幺正动力学的一个至关重要的方面就是系统与环境之间的信息流动. 本文利用量子态间的可区分性这一物理量, 统一量化了低维与高维宇称-时间对称的非厄米系统和环境之间的信息流动. 数值计算结果表明, 在宇称-时间对称性保持的相区域可以观测到量子态间可区分性的振荡以及完全的信息恢复. 然而在宇称时间对称性破坏的相区域, 信息处于指数衰减的状态. 奇异点处标志着信息流动的可逆与不可逆的临界性, 量子态间的可区分性表现出幂律衰减的行为. 理解非幺正量子动力学中的这些独特的现象为研究开放量子系统提供了重要视角, 并且有助于其在量子信息中的应用.

关键词:

Abstract

Recently, impressive progress has been made in the study of non-Hermitian systems with parity-time symmetry, such as observations of topological properties of physical systems and criticality at exceptional points. A crucial aspect of parity-time symmetric nonunitary dynamics is the information flow between the system and the environment. In this paper, we use the physical quantity, distinguishability between quantum states, to uniformly quantify the information flow between low-dimensional and high-dimensional parity-time symmetric non-Hermitian systems and environments. The numerical results show that the oscillation of quantum state distinguishability and complete information retrieval and can be obtained in the parity-time-unbroken phase. However, the information decays exponentially in the parity-time-broken phase. The exceptional point marks the criticality between reversibility and irreversibility of information flow, and the distinguishability between quantum states exhibits the behavior of power-law decay. Understanding these unique phenomena in nonunitary quantum dynamics provides an important perspective for the study of open quantum systems and contributes to their application in quantum information.

Keywords:

作者及机构信息

1.
东南大学物理学院, 南京　211189

2.
北京计算科学研究中心, 北京　100084

3.
中国人民解放军陆军步兵学院, 石家庄　050083

通信作者: 曲登科, dkqu@seu.edu.cn ; 薛鹏, gnep.eux@gmail.com

基金项目: 国家杰出青年科学基金(批准号: 12025401)和国家自然科学基金(批准号: U1930402, 12088101)资助的课题

Authors and contacts

1.
Department of Physics, Southeast University, Nanjing 211189, China

2.
Beijing Computational Science Research Center, Beijing 100084, China

3.
The Army Infantry Academy of PLA, Shijiazhuang 050083, China

Corresponding author: Qu Deng-Ke, dkqu@seu.edu.cn ; Xue Peng, gnep.eux@gmail.com

Funds: Project supported by the National Science Fund for Distinguished Young Scholars of China (Grant No. 12025401) and the National Natural Science Foundation of China (Grant Nos. U1930402, 12088101)

文章全文 : translate this paragraph

参考文献

[1]	Chen X Y, Zhang N N, He W T, et al. 2022 npj Quantum Inf. 8 22 Google Scholar
[2]	Zou D, Chen T, He W, et al. 2021 Nat. Commun. 12 7201 Google Scholar
[3]	Wu T, Zhang W, Zhang H, et al. 2020 Phys. Rev. Lett. 124 083901 Google Scholar
[4]	Yang Z, Zhang K, Fang C, Hu J 2020 Phys. Rev. Lett. 125 226402 Google Scholar
[5]	Zhang K, Yang Z, Fang C 2020 Phys. Rev. Lett. 125 126402 Google Scholar
[6]	Yang Z, Chiu C K, Fang C, Hu J 2020 Phys. Rev. Lett. 124 186402 Google Scholar
[7]	Yao S, Wang Z 2018 Phys. Rev. Lett. 121 086803 Google Scholar
[8]	Pan L, Chen X, Chen Y, Zhai H 2020 Nat. Phys. 16 767 Google Scholar
[9]	Zhou Z, Yu Z 2019 Phys. Rev. A 99 043412 Google Scholar
[10]	Zeng Q B, Yang Y B, Xu Y 2020 Phys. Rev. B 101 020201(R
[11]	Wang X R, Guo C X, Kou S P 2020 Phys. Rev. B 101 121116(R Google Scholar
[12]	Guo C X, Wang X R, Kou S P 2020 Phys. Rev. B 101 144439 Google Scholar
[13]	Zhang S, Jin L, Song Z 2022 Chin. Phys. B 31 010312 Google Scholar
[14]	Guo C X, Liu C H, Zhao X M, Liu Y, Chen S 2021 Phys. Rev. Lett. 127 116801 Google Scholar
[15]	Liu Y, Zhou Q, Chen S 2021 Phys. Rev. B 104 024201
[16]	Cui D, Li T, Li J, Yi X 2021 New J. Phys. 23 123037 Google Scholar
[17]	Lin G, Zhang S, Hu Y, Niu Y, Gong J, Gong S 2019 Phys. Rev. Lett. 123 033902 Google Scholar
[18]	Yang X, Cao Y, Zhai Y 2022 Chin. Phys. B 31 010308 Google Scholar
[19]	Ding P, Yi W 2022 Chin. Phys. B 31 010309 Google Scholar
[20]	Zhao X M, Guo C X, Kou S P, Zhuang L, Liu W M 2021 Phys. Rev. B 104 205131 Google Scholar
[21]	Bender C M, Boettcher S 1998 Phys. Rev. Lett. 80 5243
[22]	Bender C M, Brody D C, Jones H F 2002 Phys. Rev. Lett. 89 270401 Google Scholar
[23]	Bender C M 2007 Rep. Prog. Phys. 70 947
[24]	Heiss W D 2012 J. Phys. A 45 444016 Google Scholar
[25]	Makris K G, El-Ganainy R, Christodoulides D N, Musslimani Z H 2008 Phys. Rev. Lett. 100 103904 Google Scholar
[26]	Rüter C E, Makris K G, El-Ganainy R, Christodoulides D N, Segev M, Kip D 2010 Nat. Phys. 6 192 Google Scholar
[27]	Regensburger A, Bersch C, Miri M A, Onishchukov G, Christodoulides D N, Peschel U 2012 Nature 488 167 Google Scholar
[28]	Schindler J, Li A, Zheng M C, Ellis F M, Kottos T 2011 Phys. Rev. A 84 040101(R Google Scholar
[29]	Bender C M, Berntson B K, Parker D, Samuel E 2013 Am. J. Phys. 81 173 Google Scholar
[30]	Lin Z, Ramezani H, Eichelkraut T, Kottos T, Cao H, Christodoulides D N 2011 Phys. Rev. Lett. 106 213901 Google Scholar
[31]	Liu Z P, Zhang J, Özdemir S K, et al. 2016 Phys. Rev. Lett. 117 110802 Google Scholar
[32]	Gao T, Estrecho E, Bliokh K Y, et al. 2015 Nature 526 554 Google Scholar
[33]	Graefe E M, Korsch H J, Niederle A E 2008 Phys. Rev. Lett. 101 150408 Google Scholar
[34]	Chen S L, Chen G Y, Chen Y N 2014 Phys. Rev. A 90 054301 Google Scholar
[35]	Yin S, Huang G Y, Lo C Y, Chen P 2017 Phys. Rev. Lett. 118 065701 Google Scholar
[36]	Li J, Harter A K, Liu J, de Melo L, Joglekar Y N, Luo L 2019 Nat. Commun. 10 855 Google Scholar
[37]	Xiao L, Zhan X, Bian Z, et al. 2017 Nat. Phys. 13 1117 Google Scholar
[38]	Wang K, Qiu X, Xiao L, et al. 2019 Nat. Commun. 10 2293 Google Scholar
[39]	Xiao L, Qu D, Wang K, et al. 2021 PRX Quantum 2 020313 Google Scholar
[40]	Xiao L, Wang K, Zhan X, et al. 2019 Phys. Rev. Lett. 123 230401 Google Scholar
[41]	Bian Z, Xiao L, Wang K, et al. 2020 Phys. Rev. A 102 030201(R Google Scholar
[42]	Bian Z, Xiao L, Wang K, et al. 2020 Phys. Rev. Res. 2 022039(R Google Scholar
[43]	Xiao L, Deng T, Wang K, Wang Z, Yi W, Xue P 2021 Phys. Rev. Lett. 126 230402 Google Scholar
[44]	Zurek W H 2003 Rev. Mod. Phys 75 715 Google Scholar
[45]	de Vega I, Alonso D 2017 Rev. Mod. Phys 89 015001 Google Scholar
[46]	Kawabata K, Ashida Y, Ueda M 2017 Phys. Rev. Lett. 119 190401 Google Scholar
[47]	Misra B, Sudarshan E C G 1977 J. Math. Phys. 18 756 Google Scholar
[48]	Itano W M, Heinzen D J, Bollinger J J, Wineland D J 1990 Phys. Rev. A 41 2295 Google Scholar
[49]	Facchi P, Pascazio S 2002 Phys. Rev. Lett. 89 080401 Google Scholar
[50]	Viola L, Lloyd S 1998 Phys. Rev. A 58 2733 Google Scholar
[51]	Viola L, Knill E, Lloyd S 1999 Phys. Rev. Lett. 82 2417 Google Scholar
[52]	Viola L, Lloyd S, Knill E 1999 Phys. Rev. Lett. 83 4888 Google Scholar
[53]	Palma G M, Suominen K A, Ekert A K 1996 Proc. R. Soc. A 452 567 Google Scholar
[54]	Zanardi P, Rasetti M 1997 Phys. Rev. Lett. 79 3306 Google Scholar
[55]	Duan L M, Guo G C 1998 Phys. Rev. A 57 737 Google Scholar
[56]	Lidar D A, Chuang I L, Whaley K B 1998 Phys. Rev. Lett. 81 2594 Google Scholar
[57]	Lidar D A, Bacon D, Whaley K B 1999 Phys. Rev. Lett. 82 4556 Google Scholar
[58]	Knill E, Laflamme R, Viola L 2000 Phys. Rev. Lett. 84 2525 Google Scholar
[59]	Beige A, Braun D, Tregenna B, Knight P L 2000 Phys. Rev. Lett. 85 1762 Google Scholar
[60]	Brody D C, Graefe E M 2012 Phys. Rev. Lett. 109 230405 Google Scholar
[61]	Nielsen M A, Chuang I L 2010 Quantum Computation and Quantum Information (New York: Cambridge University Press) pp403–409
[62]	Fuchs C A, van de Graaf J 1999 IEEE Trans. Inf. Theory 45 1216 Google Scholar
[63]	Gilchrist A, Langford N K, Nielsen M A 2005 Phys. Rev. A 71 062310 Google Scholar
[64]	Ruskai M B 1994 Rev. Math. Phys. 06 1147 Google Scholar
[65]	Erez N, Gordon G, Nest M, Kurizki G 2008 Nature 452 724 Google Scholar
[66]	Wolf M M, Eisert J, Cubitt T S, Cirac J I 2008 Phys. Rev. Lett. 101 150402 Google Scholar
[67]	Breuer H P, Laine E M, Piilo J 2009 Phys. Rev. Lett. 103 210401 Google Scholar
[68]	Laine E M, Piilo J, Breuer H P 2010 Phys. Rev. A 81 062115 Google Scholar
[69]	Rivas A, Huelga S F, Plenio M B 2010 Phys. Rev. Lett. 105 050403 Google Scholar
[70]	Luo A, Fu S, Song H 2012 Phys. Rev. A 86 044101 Google Scholar
[71]	Chruściński D, Maniscalco S 2014 Phys. Rev. Lett. 112 120404 Google Scholar
[72]	Chruściński D, Macchiavello C, Maniscalco S 2017 Phys. Rev. Lett. 118 080404 Google Scholar
[73]	Breuer H P, Laine E M, Piilo J, Vacchini B 2016 Rev. Mod. Phys. 88 021002 Google Scholar
[74]	Wolf M M, Cirac J I 2008 Commun. Math. Phys. 279 147 Google Scholar
[75]	Hou S C, Yi X X, Yu S X, Oh C H 2011 Phys. Rev. A 83 062115 Google Scholar
[76]	Lu X M, Wang X, Sun C P 2010 Phys. Rev. A 82 042103 Google Scholar
[77]	Jiang M, Luo S 2013 Phys. Rev. A 88 034101 Google Scholar
[78]	Lorenzo S, Plastina F, Paternostro M 2013 Phys. Rev. A 88 020102 Google Scholar
[79]	Tang J S, Wang Y T, Yu S, et al. 2016 Nat. Photonics 10 642 Google Scholar
[80]	Hodaei H, Hassan A U, Wittek S, et al. 2017 Nature 548 187 Google Scholar
[81]	Graefe E M, Günther U, Korsch H J, Niederle A E 2008 J. Phys. A 41 255206 Google Scholar
[82]	Quiroz-Juárez M A, Perez-Leija A, Tschernig K, et al. 2019 Photonics Res. 7 862 Google Scholar
[83]	Caves C M 1982 Phys. Rev. D 26 1817 Google Scholar
[84]	Scheel S, Szameit A 2018 Europhys. Lett. 122 34001 Google Scholar
[85]	Wang K, Qiu X, Xiao L, et al. 2019 Phys. Rev. Lett. 122 020501 Google Scholar
[86]	Zhan X, Xiao L, Bian Z, et al. 2017 Phys. Rev. Lett. 119 130501 Google Scholar
[87]	Xiao L, Deng T S, Wang K, et al. 2020 Nat. Phys. 16 761 Google Scholar
[88]	Klauck F, Teuber L, Ornigotti M, Heinrich M, Scheel S, Szameit A 2019 Nat. Photonics 13 883 Google Scholar
[89]	Naghiloo M, Abbasi M, Joglekar Y N, Murch K W 2019 Nat. Phys. 19 1232
[90]	Zhan X, Wang K, Xiao L, et al. 2020 Phys. Rev. A 101 010302(R Google Scholar
[91]	Xue P 2022 Chin. Phys. B 31 010311 Google Scholar
[92]	Xue P, Sanders B C, Leibfried D 2009 Phys. Rev. Lett. 103 183602 Google Scholar
[93]	Wang K, Xiao L, Budich J C, Yi W, Xue P 2021 Phys. Rev. Lett. 127 026404 Google Scholar
[94]	Wang K, Li T, Xiao L, Han Y, Yi W, Xue P 2021 Phys. Rev. Lett. 127 270602 Google Scholar
[95]	Wang X, Xiao L, Qiu X, Wang K, Yi W, Xue P 2018 Phys. Rev. A 98 013835 Google Scholar
[96]	Xiao L, Qiu X, Wang K, et al. 2018 Phys. Rev. A 98 063847 Google Scholar

施引文献

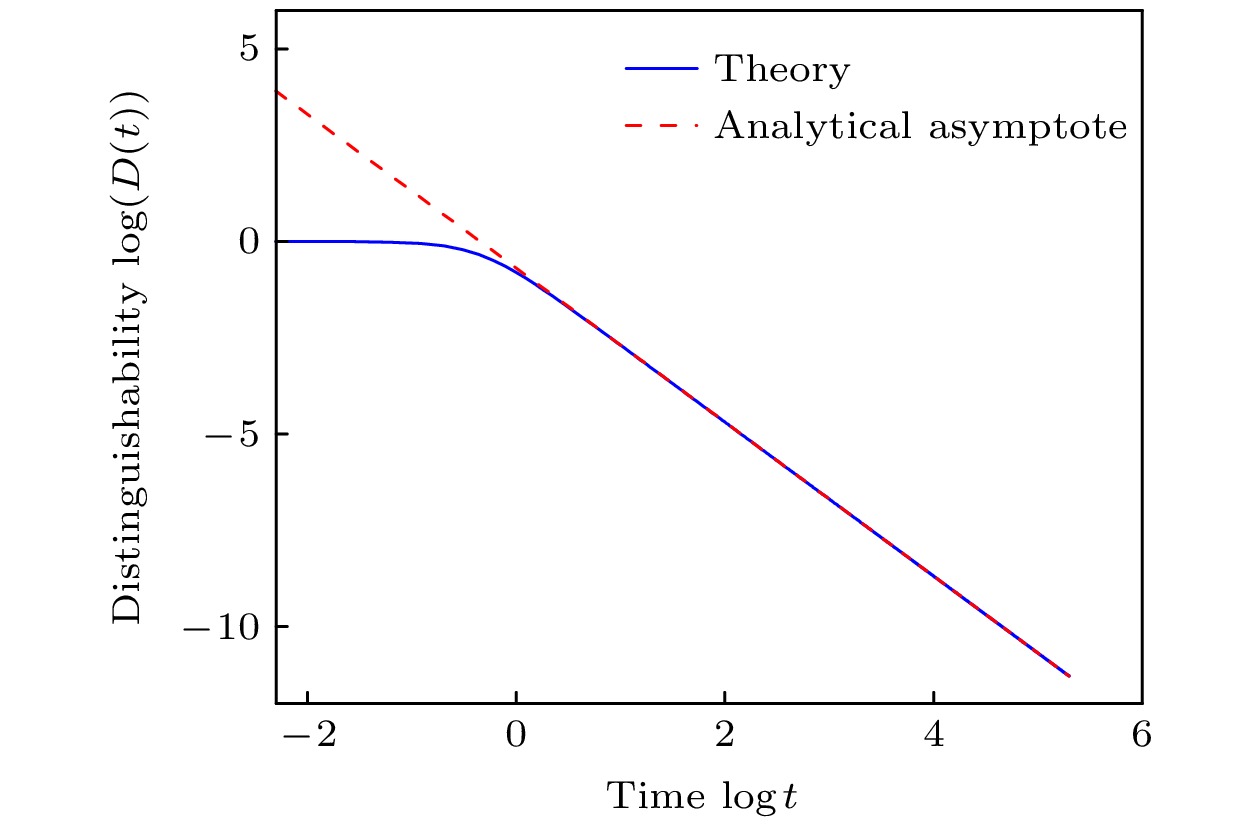
图 1 在宇称-时间对称的两能级系统的信息流动　(a), (b) 在宇称-时间对称保持的区域($0<a<1$), 量子态的可区分性表现出周期性振荡, 当逐渐靠近奇异点($a=1$)时, 信息恢复的周期会变长; (c) 在宇称-时间对称性被破坏的区域($a > $$ 1$), 量子态的可区分性在一直在衰减

Fig. 1. Information flow in the parity-time-symmetric two-level system: (a), (b) The distinguishability oscillates with period in the parity-time-unbroken phase ($0<a<1$). When approaching the exceptional point ($a=1$), the period of the information retrieval. (c) The distinguishability between quantum states is declining in the parity-time-symmetry-broken regime ($a>1$)

下载: 全尺寸图片幻灯片

图 2 在宇称-时间对称的两能级系统在奇异点处($a=1$), 量子态的可区分性的幂律行为

Fig. 2. Power-law behavior of the distinguishability of the parity-time-symmetric system at the exceptional point $a=1$

下载: 全尺寸图片幻灯片

图 3 宇称-时间对称的四能级系统的信息流动　(a), (b) 在宇称-时间对称保持的区域($\gamma<J$), 量子态的可区分性表现出周期性振荡, 当逐渐靠近奇异点($\gamma=J$)时, 信息恢复的周期会变长; (c) 在宇称-时间对称性被破坏的区域($\gamma> $$ J$), 量子态的可区分性在一直在衰减

Fig. 3. Information flow in the parity-time-symmetric four-level system: (a), (b) The distinguishability oscillates with period in the parity-time-unbroken phase ($\gamma<J$). When approaching the exceptional point ($\gamma=J$), the period of the information retrieval. (c) The distinguishability between quantum states is declining in the parity-time-symmetry-broken regime ($\gamma>J$)

下载: 全尺寸图片幻灯片

图 4 在宇称-时间对称的四能级系统在奇异点处($\gamma=J$), 量子态的可区分性的幂律行为.

Fig. 4. Power-law behavior of the distinguishability of the parity-time-symmetric system at the exceptional point $\gamma=J$.

下载: 全尺寸图片幻灯片

map

[1]	Chen X Y, Zhang N N, He W T, et al. 2022 npj Quantum Inf. 8 22 Google Scholar
[2]	Zou D, Chen T, He W, et al. 2021 Nat. Commun. 12 7201 Google Scholar
[3]	Wu T, Zhang W, Zhang H, et al. 2020 Phys. Rev. Lett. 124 083901 Google Scholar
[4]	Yang Z, Zhang K, Fang C, Hu J 2020 Phys. Rev. Lett. 125 226402 Google Scholar
[5]	Zhang K, Yang Z, Fang C 2020 Phys. Rev. Lett. 125 126402 Google Scholar
[6]	Yang Z, Chiu C K, Fang C, Hu J 2020 Phys. Rev. Lett. 124 186402 Google Scholar
[7]	Yao S, Wang Z 2018 Phys. Rev. Lett. 121 086803 Google Scholar
[8]	Pan L, Chen X, Chen Y, Zhai H 2020 Nat. Phys. 16 767 Google Scholar
[9]	Zhou Z, Yu Z 2019 Phys. Rev. A 99 043412 Google Scholar
[10]	Zeng Q B, Yang Y B, Xu Y 2020 Phys. Rev. B 101 020201(R
[11]	Wang X R, Guo C X, Kou S P 2020 Phys. Rev. B 101 121116(R Google Scholar
[12]	Guo C X, Wang X R, Kou S P 2020 Phys. Rev. B 101 144439 Google Scholar
[13]	Zhang S, Jin L, Song Z 2022 Chin. Phys. B 31 010312 Google Scholar
[14]	Guo C X, Liu C H, Zhao X M, Liu Y, Chen S 2021 Phys. Rev. Lett. 127 116801 Google Scholar
[15]	Liu Y, Zhou Q, Chen S 2021 Phys. Rev. B 104 024201
[16]	Cui D, Li T, Li J, Yi X 2021 New J. Phys. 23 123037 Google Scholar
[17]	Lin G, Zhang S, Hu Y, Niu Y, Gong J, Gong S 2019 Phys. Rev. Lett. 123 033902 Google Scholar
[18]	Yang X, Cao Y, Zhai Y 2022 Chin. Phys. B 31 010308 Google Scholar
[19]	Ding P, Yi W 2022 Chin. Phys. B 31 010309 Google Scholar
[20]	Zhao X M, Guo C X, Kou S P, Zhuang L, Liu W M 2021 Phys. Rev. B 104 205131 Google Scholar
[21]	Bender C M, Boettcher S 1998 Phys. Rev. Lett. 80 5243
[22]	Bender C M, Brody D C, Jones H F 2002 Phys. Rev. Lett. 89 270401 Google Scholar
[23]	Bender C M 2007 Rep. Prog. Phys. 70 947
[24]	Heiss W D 2012 J. Phys. A 45 444016 Google Scholar
[25]	Makris K G, El-Ganainy R, Christodoulides D N, Musslimani Z H 2008 Phys. Rev. Lett. 100 103904 Google Scholar
[26]	Rüter C E, Makris K G, El-Ganainy R, Christodoulides D N, Segev M, Kip D 2010 Nat. Phys. 6 192 Google Scholar
[27]	Regensburger A, Bersch C, Miri M A, Onishchukov G, Christodoulides D N, Peschel U 2012 Nature 488 167 Google Scholar
[28]	Schindler J, Li A, Zheng M C, Ellis F M, Kottos T 2011 Phys. Rev. A 84 040101(R Google Scholar
[29]	Bender C M, Berntson B K, Parker D, Samuel E 2013 Am. J. Phys. 81 173 Google Scholar
[30]	Lin Z, Ramezani H, Eichelkraut T, Kottos T, Cao H, Christodoulides D N 2011 Phys. Rev. Lett. 106 213901 Google Scholar
[31]	Liu Z P, Zhang J, Özdemir S K, et al. 2016 Phys. Rev. Lett. 117 110802 Google Scholar
[32]	Gao T, Estrecho E, Bliokh K Y, et al. 2015 Nature 526 554 Google Scholar
[33]	Graefe E M, Korsch H J, Niederle A E 2008 Phys. Rev. Lett. 101 150408 Google Scholar
[34]	Chen S L, Chen G Y, Chen Y N 2014 Phys. Rev. A 90 054301 Google Scholar
[35]	Yin S, Huang G Y, Lo C Y, Chen P 2017 Phys. Rev. Lett. 118 065701 Google Scholar
[36]	Li J, Harter A K, Liu J, de Melo L, Joglekar Y N, Luo L 2019 Nat. Commun. 10 855 Google Scholar
[37]	Xiao L, Zhan X, Bian Z, et al. 2017 Nat. Phys. 13 1117 Google Scholar
[38]	Wang K, Qiu X, Xiao L, et al. 2019 Nat. Commun. 10 2293 Google Scholar
[39]	Xiao L, Qu D, Wang K, et al. 2021 PRX Quantum 2 020313 Google Scholar
[40]	Xiao L, Wang K, Zhan X, et al. 2019 Phys. Rev. Lett. 123 230401 Google Scholar
[41]	Bian Z, Xiao L, Wang K, et al. 2020 Phys. Rev. A 102 030201(R Google Scholar
[42]	Bian Z, Xiao L, Wang K, et al. 2020 Phys. Rev. Res. 2 022039(R Google Scholar
[43]	Xiao L, Deng T, Wang K, Wang Z, Yi W, Xue P 2021 Phys. Rev. Lett. 126 230402 Google Scholar
[44]	Zurek W H 2003 Rev. Mod. Phys 75 715 Google Scholar
[45]	de Vega I, Alonso D 2017 Rev. Mod. Phys 89 015001 Google Scholar
[46]	Kawabata K, Ashida Y, Ueda M 2017 Phys. Rev. Lett. 119 190401 Google Scholar
[47]	Misra B, Sudarshan E C G 1977 J. Math. Phys. 18 756 Google Scholar
[48]	Itano W M, Heinzen D J, Bollinger J J, Wineland D J 1990 Phys. Rev. A 41 2295 Google Scholar
[49]	Facchi P, Pascazio S 2002 Phys. Rev. Lett. 89 080401 Google Scholar
[50]	Viola L, Lloyd S 1998 Phys. Rev. A 58 2733 Google Scholar
[51]	Viola L, Knill E, Lloyd S 1999 Phys. Rev. Lett. 82 2417 Google Scholar
[52]	Viola L, Lloyd S, Knill E 1999 Phys. Rev. Lett. 83 4888 Google Scholar
[53]	Palma G M, Suominen K A, Ekert A K 1996 Proc. R. Soc. A 452 567 Google Scholar
[54]	Zanardi P, Rasetti M 1997 Phys. Rev. Lett. 79 3306 Google Scholar
[55]	Duan L M, Guo G C 1998 Phys. Rev. A 57 737 Google Scholar
[56]	Lidar D A, Chuang I L, Whaley K B 1998 Phys. Rev. Lett. 81 2594 Google Scholar
[57]	Lidar D A, Bacon D, Whaley K B 1999 Phys. Rev. Lett. 82 4556 Google Scholar
[58]	Knill E, Laflamme R, Viola L 2000 Phys. Rev. Lett. 84 2525 Google Scholar
[59]	Beige A, Braun D, Tregenna B, Knight P L 2000 Phys. Rev. Lett. 85 1762 Google Scholar
[60]	Brody D C, Graefe E M 2012 Phys. Rev. Lett. 109 230405 Google Scholar
[61]	Nielsen M A, Chuang I L 2010 Quantum Computation and Quantum Information (New York: Cambridge University Press) pp403–409
[62]	Fuchs C A, van de Graaf J 1999 IEEE Trans. Inf. Theory 45 1216 Google Scholar
[63]	Gilchrist A, Langford N K, Nielsen M A 2005 Phys. Rev. A 71 062310 Google Scholar
[64]	Ruskai M B 1994 Rev. Math. Phys. 06 1147 Google Scholar
[65]	Erez N, Gordon G, Nest M, Kurizki G 2008 Nature 452 724 Google Scholar
[66]	Wolf M M, Eisert J, Cubitt T S, Cirac J I 2008 Phys. Rev. Lett. 101 150402 Google Scholar
[67]	Breuer H P, Laine E M, Piilo J 2009 Phys. Rev. Lett. 103 210401 Google Scholar
[68]	Laine E M, Piilo J, Breuer H P 2010 Phys. Rev. A 81 062115 Google Scholar
[69]	Rivas A, Huelga S F, Plenio M B 2010 Phys. Rev. Lett. 105 050403 Google Scholar
[70]	Luo A, Fu S, Song H 2012 Phys. Rev. A 86 044101 Google Scholar
[71]	Chruściński D, Maniscalco S 2014 Phys. Rev. Lett. 112 120404 Google Scholar
[72]	Chruściński D, Macchiavello C, Maniscalco S 2017 Phys. Rev. Lett. 118 080404 Google Scholar
[73]	Breuer H P, Laine E M, Piilo J, Vacchini B 2016 Rev. Mod. Phys. 88 021002 Google Scholar
[74]	Wolf M M, Cirac J I 2008 Commun. Math. Phys. 279 147 Google Scholar
[75]	Hou S C, Yi X X, Yu S X, Oh C H 2011 Phys. Rev. A 83 062115 Google Scholar
[76]	Lu X M, Wang X, Sun C P 2010 Phys. Rev. A 82 042103 Google Scholar
[77]	Jiang M, Luo S 2013 Phys. Rev. A 88 034101 Google Scholar
[78]	Lorenzo S, Plastina F, Paternostro M 2013 Phys. Rev. A 88 020102 Google Scholar
[79]	Tang J S, Wang Y T, Yu S, et al. 2016 Nat. Photonics 10 642 Google Scholar
[80]	Hodaei H, Hassan A U, Wittek S, et al. 2017 Nature 548 187 Google Scholar
[81]	Graefe E M, Günther U, Korsch H J, Niederle A E 2008 J. Phys. A 41 255206 Google Scholar
[82]	Quiroz-Juárez M A, Perez-Leija A, Tschernig K, et al. 2019 Photonics Res. 7 862 Google Scholar
[83]	Caves C M 1982 Phys. Rev. D 26 1817 Google Scholar
[84]	Scheel S, Szameit A 2018 Europhys. Lett. 122 34001 Google Scholar
[85]	Wang K, Qiu X, Xiao L, et al. 2019 Phys. Rev. Lett. 122 020501 Google Scholar
[86]	Zhan X, Xiao L, Bian Z, et al. 2017 Phys. Rev. Lett. 119 130501 Google Scholar
[87]	Xiao L, Deng T S, Wang K, et al. 2020 Nat. Phys. 16 761 Google Scholar
[88]	Klauck F, Teuber L, Ornigotti M, Heinrich M, Scheel S, Szameit A 2019 Nat. Photonics 13 883 Google Scholar
[89]	Naghiloo M, Abbasi M, Joglekar Y N, Murch K W 2019 Nat. Phys. 19 1232
[90]	Zhan X, Wang K, Xiao L, et al. 2020 Phys. Rev. A 101 010302(R Google Scholar
[91]	Xue P 2022 Chin. Phys. B 31 010311 Google Scholar
[92]	Xue P, Sanders B C, Leibfried D 2009 Phys. Rev. Lett. 103 183602 Google Scholar
[93]	Wang K, Xiao L, Budich J C, Yi W, Xue P 2021 Phys. Rev. Lett. 127 026404 Google Scholar
[94]	Wang K, Li T, Xiao L, Han Y, Yi W, Xue P 2021 Phys. Rev. Lett. 127 270602 Google Scholar
[95]	Wang X, Xiao L, Qiu X, Wang K, Yi W, Xue P 2018 Phys. Rev. A 98 013835 Google Scholar
[96]	Xiao L, Qiu X, Wang K, et al. 2018 Phys. Rev. A 98 063847 Google Scholar

[1]	江翠, 李家锐, 亓迪, 张莲莲. 具有宇称-时间反演对称性的虚势能对T-型石墨烯结构能谱和边缘态的影响. , 2024, 73(20): 207301. doi: 10.7498/aps.73.20240871
[2]	张慧洁, 贺衎. 哈密顿量宇称-时间对称性的刻画. , 2024, 73(4): 040302. doi: 10.7498/aps.73.20230458
[3]	古燕, 陆展鹏. 非厄米耦合链中的局域化转变. , 2024, 73(19): 197101. doi: 10.7498/aps.73.20240976
[4]	刘敬鹄, 徐志浩. 随机两体耗散诱导的非厄米多体局域化. , 2024, 73(7): 077202. doi: 10.7498/aps.73.20231987
[5]	蒋宏帆, 林机, 胡贝贝, 张肖. 非宇称时间对称耦合器中的非局域孤子. , 2023, 72(10): 104205. doi: 10.7498/aps.72.20230082
[6]	李竞, 丁海涛, 张丹伟. 非厄米哈密顿量中的量子Fisher信息与参数估计. , 2023, 72(20): 200601. doi: 10.7498/aps.72.20230862
[7]	李家锐, 王梓安, 徐彤彤, 张莲莲, 公卫江. 一维${\cal {PT}}$对称非厄米自旋轨道耦合Su-Schrieffer-Heeger模型的拓扑性质. , 2022, 71(17): 177302. doi: 10.7498/aps.71.20220796
[8]	祝可嘉, 郭志伟, 陈鸿. 实验观测非厄米系统奇异点的手性翻转现象. , 2022, 71(13): 131101. doi: 10.7498/aps.71.20220842
[9]	潘磊. 非厄米线性响应理论及其应用. , 2022, 71(17): 170305. doi: 10.7498/aps.71.20220862
[10]	唐原江, 梁超, 刘永椿. 宇称-时间对称与反对称研究进展. , 2022, 71(17): 171101. doi: 10.7498/aps.71.20221323
[11]	曲登科, 范毅, 薛鹏. 高维宇称-时间对称系统中的信息恢复与临界性. , 2022, 0(0): . doi: 10.7498/aps.71.20220511
[12]	张毅. 时间尺度上非迁移Birkhoff系统的Mei对称性定理. , 2021, 70(24): 244501. doi: 10.7498/aps.70.20210372
[13]	蔡子. 非平衡量子物态中的对称性与时间维度效应. , 2021, 70(23): 230310. doi: 10.7498/aps.70.20211741
[14]	张高见, 王逸璞. 腔光子-自旋波量子耦合系统中各向异性奇异点的实验研究. , 2020, 69(4): 047103. doi: 10.7498/aps.69.20191632
[15]	李元成, 王小明, 夏丽莉. 完整系统Nielsen方程的统一对称性与守恒量. , 2010, 59(5): 2935-2938. doi: 10.7498/aps.59.2935
[16]	李元成, 夏丽莉, 王小明. 具有非Chetaev型非完整约束的机电系统的统一对称性. , 2009, 58(10): 6732-6736. doi: 10.7498/aps.58.6732
[17]	李元成, 夏丽莉, 赵伟, 后其宝, 王静, 荆宏星. 机电系统的统一对称性. , 2007, 56(9): 5037-5040. doi: 10.7498/aps.56.5037
[18]	丁宁, 方建会, 张鹏玉, 王鹏. Poincaré-Chetaev方程的统一对称性. , 2006, 55(12): 6197-6202. doi: 10.7498/aps.55.6197
[19]	茅德强, 李名复, 任尚元. GaP中替代缺陷对的A1对称性深能级波函数. , 1984, 33(7): 897-907. doi: 10.7498/aps.33.897
[20]	刘辽, 李鉴曾. 迹反常与时间对称性反弹宇宙模型. , 1982, 31(12): 96-99. doi: 10.7498/aps.31.96

计量

文章访问数: 5220
PDF下载量: 332
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

高维宇称-时间对称系统中的信息恢复与临界性