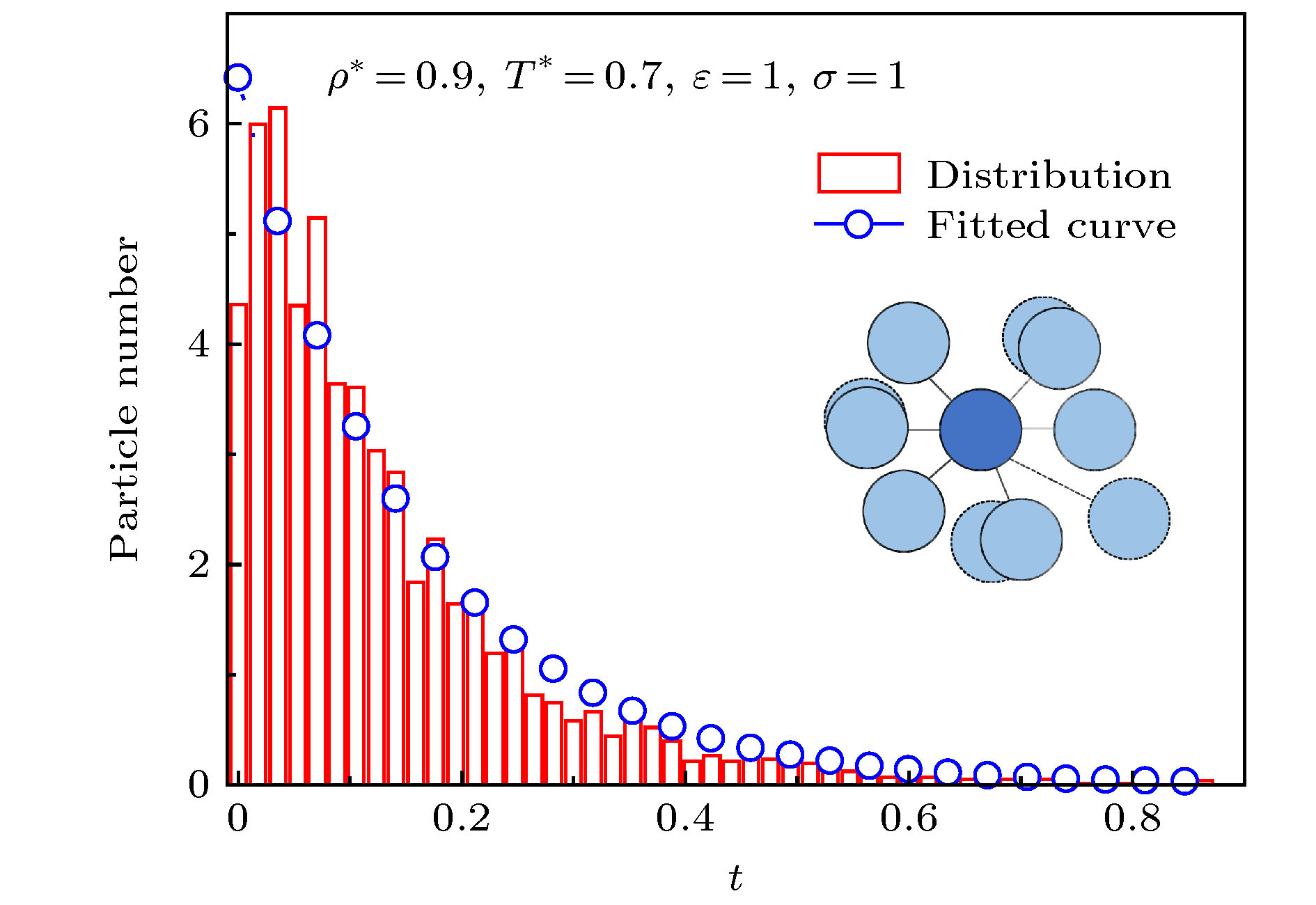
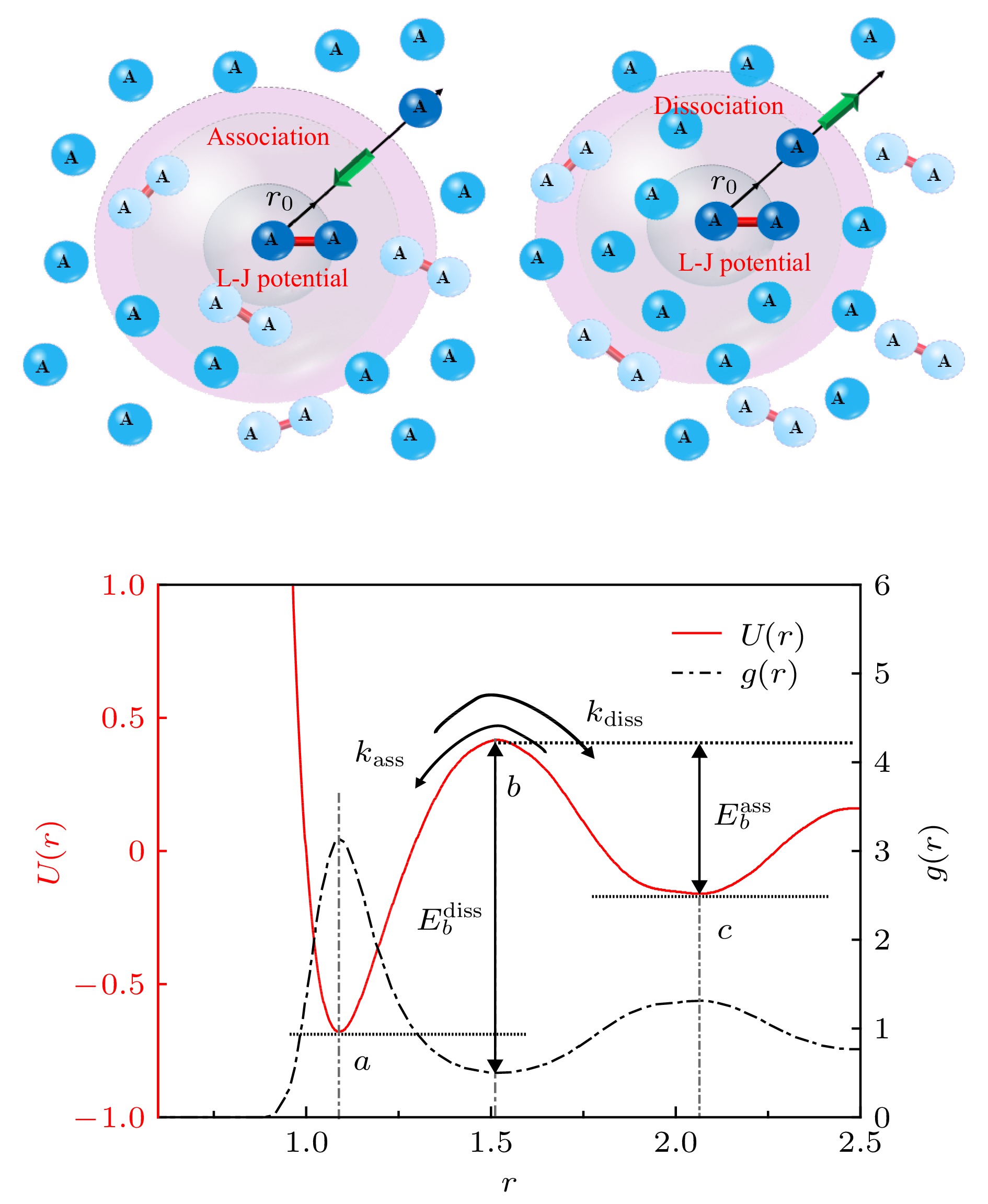
-
采用平衡态分子模拟的方法, 从微观角度对温度
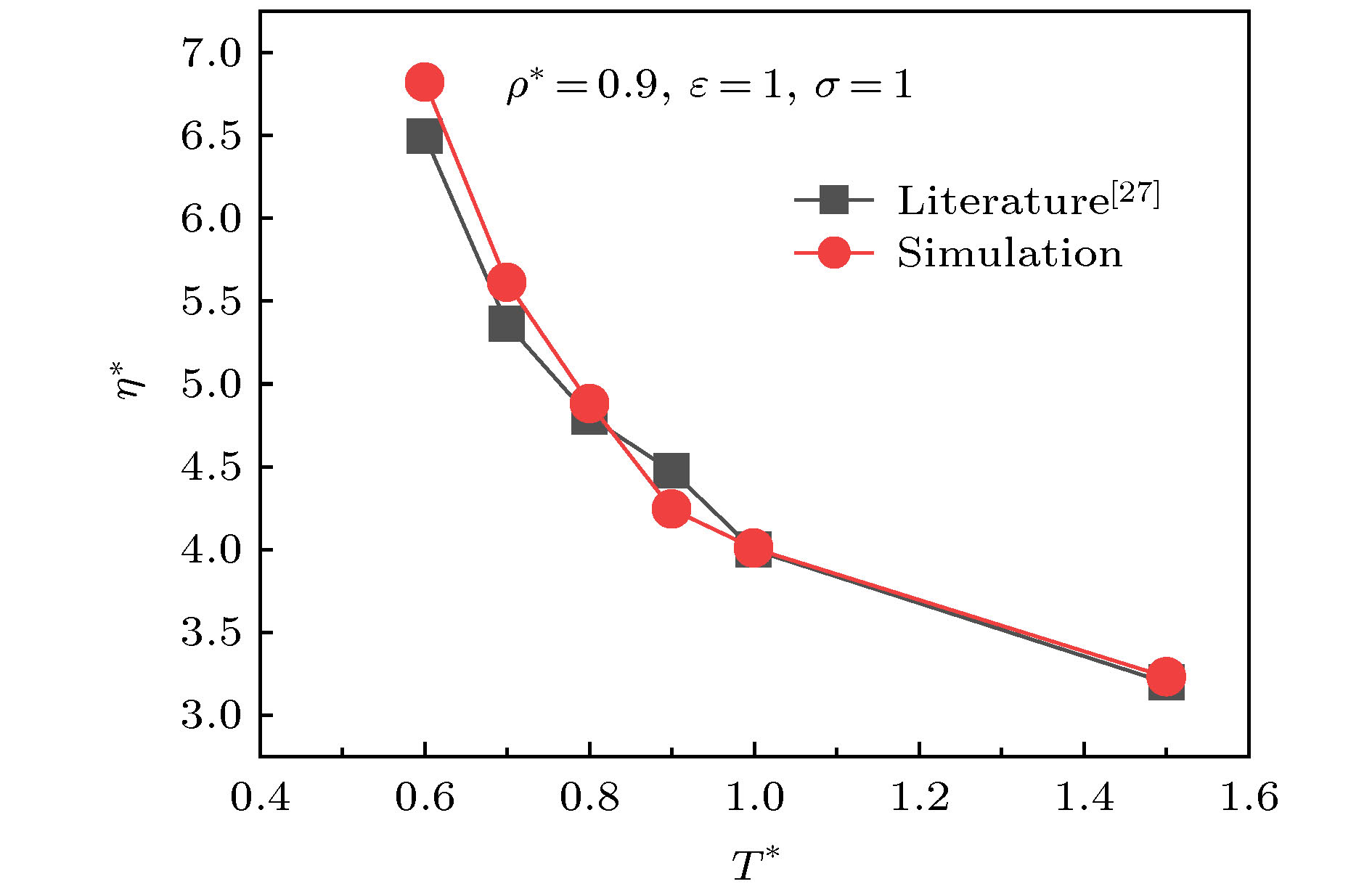
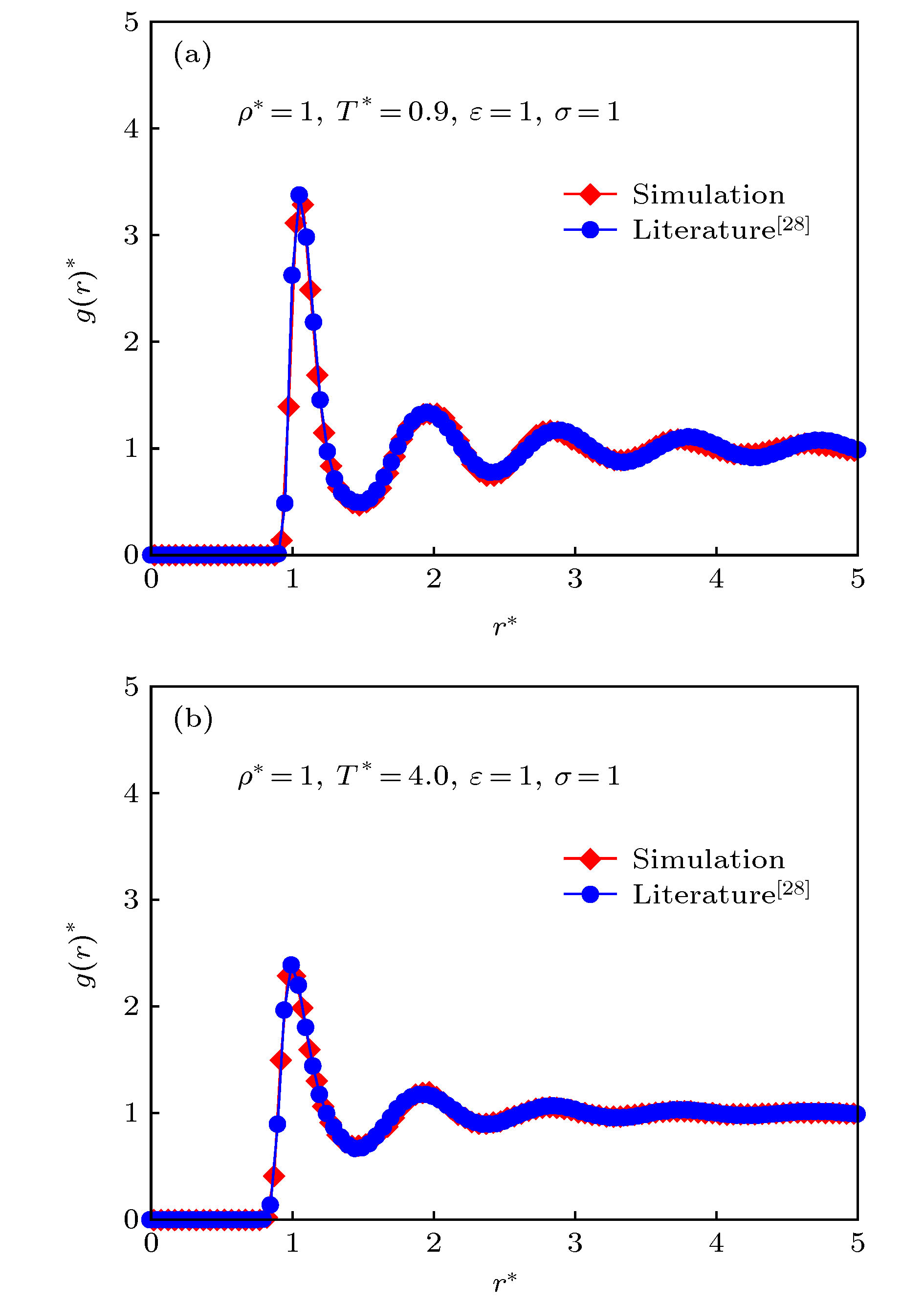
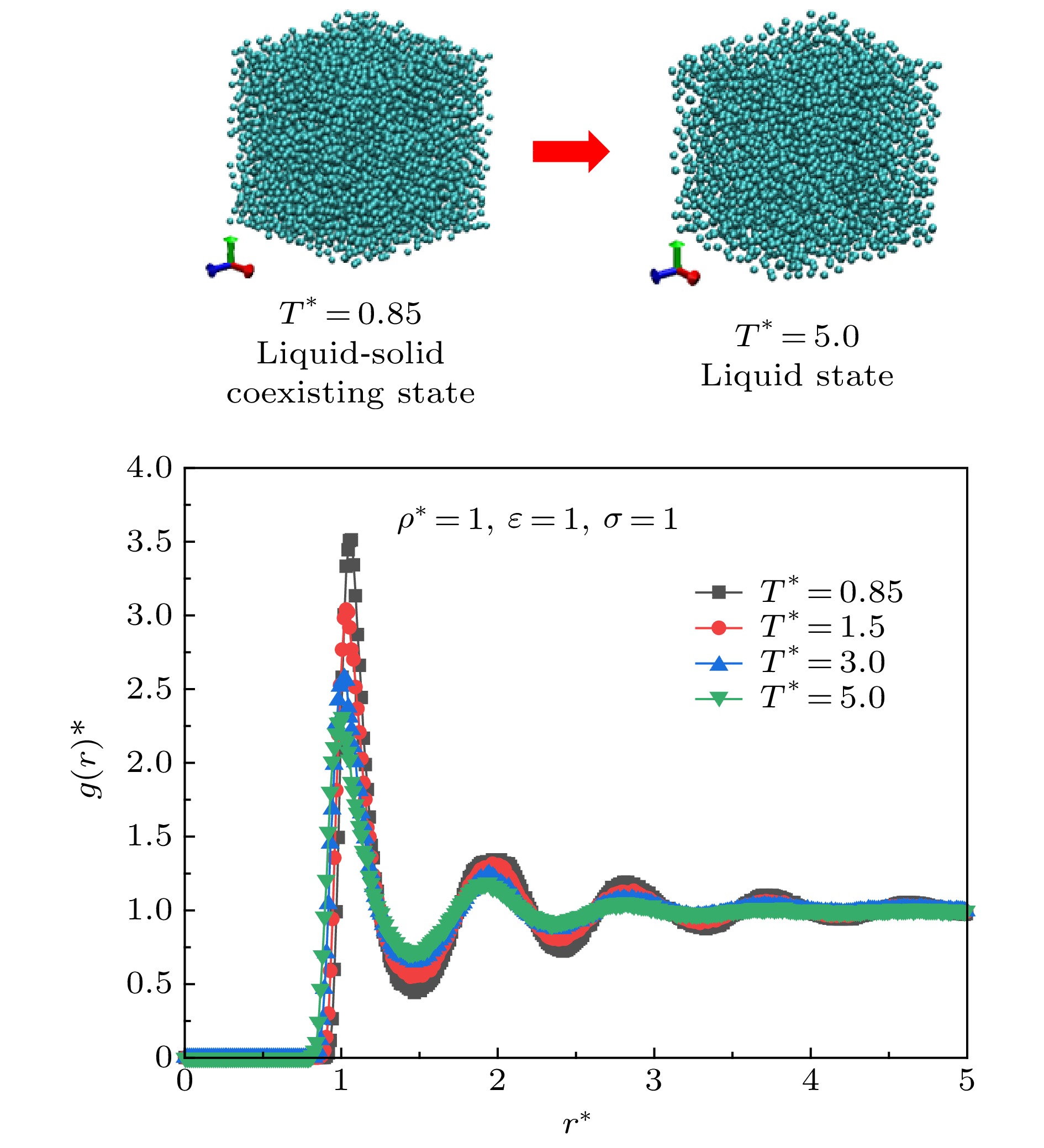
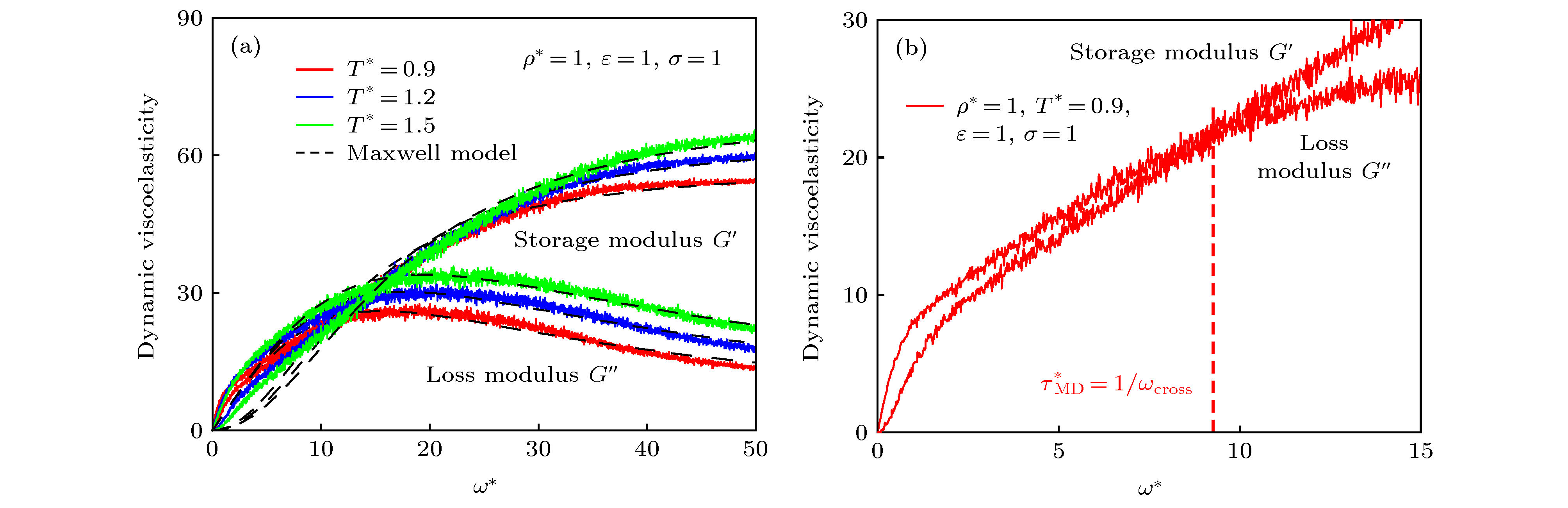
$T^* = 0.85$ —5、密度$ \rho^* $ = 0.85—1、势参数ε = 0.97—1和σ = 0.8—1.3范围内22组液固共存态及液态单原子Lennard-Jones (L-J)体的黏弹性弛豫时间进行了研究, 计算了单原子L-J体的静态黏弹性(黏度η*、无限大频率的剪切模量$ G_∞^* $ )及动态黏弹性(储能模量$ G^{\prime *} $ 、损耗模量$ G^{\prime \prime *} $ )等特性参量, 并在此基础上分析了黏弹性特征弛豫时间、Maxwell弛豫时间及原子连通弛豫时间. 此外, 本文根据系统内原子的排布情况, 应用Kramers逃逸速率理论描述原子的扩散、汇聚过程, 提出并建立了一种单原子L-J体黏弹性弛豫时间的预测方法. 结果表明: 在单原子L-J体系统中, 低温情况下, Maxwell弛豫时间与黏弹性特征弛豫时间差异明显; 原子连通弛豫时间与黏弹性特征弛豫时间结果接近, 但原子连通弛豫时间的计算过程需耗费大量时间和计算资源; 预测方法得到的弛豫时间与黏弹性特征弛豫时间的结果更为接近. 本文提出的单原子L-J体黏弹性弛豫时间的预测方法具有一定的准确性和可靠性, 可为材料黏弹性弛豫时间的研究提供一种新的思路.-
关键词:
- Lennard-Jones体 /
- 分子模拟 /
- 黏弹性 /
- 弛豫时间 /
- 预测方法
Viscoelastic relaxation time is an important concept to characterize the viscoelastic response of materials, which is directly related to the interactions among the microscopic atoms of materials. Few studies have focused on the methods of characterizing viscoelastic relaxation time. To investigate how to represent viscoelastic relaxation time effectively, the viscoelastic relaxation times of the monoatomic Lennard-Jones system on 22 conditions in a range of$ T^{ *} $ = 0.85–5, ρ* = 0.85–1, ε = 0.97–1, and σ = 0.8–1.3 are discussed from a microscopic perspective by the equilibrium molecular dynamics methods. Static viscoelasticity (viscosity η*, high-frequency shear modulus$ G_{\infty}^* $ ) is calculated by the Green-Kubo formula, and the Fourier transform is applied to the calculation of dynamic viscoelasticity (storage modulus$ G'^* $ and loss modulus$ G''^* $ ). On this basis, the viscoelastic characteristic relaxation time ($ \tau _{{\rm{MD}}}^*$ ), Maxwell relaxation time ($ \tau _{{\rm{Maxwell}}}^*$ ) and the lifetime of the state of local atomic connectivity ($ \tau _{{\rm{LC}}}^*$ ) are calculated. The viscoelastic characteristic relaxation time$ \tau _{{\rm{MD}}}^*$ , defined when the two responses crossover, is the key measure of the period of such a stimulus when the storage modulus (elasticity) equals the loss modulus (viscosity). Maxwell relaxation time$ \tau _{{\rm{Maxwell}}}^* = {\eta ^*}/G_\infty ^*$ , where η* is the static viscosity under infinitely low stimulus frequency (i.e., zero shear rate),$ G_{\infty}^* $ is the instantaneous shear modulus under infinitely high stimulus frequency, and$ \tau _{{\rm{LC}}}^*$ is the time it takes for an atom to lose or gain one nearest neighbor. The result is observed that$ \tau _{{\rm{LC}}}^*$ is closer to$ \tau _{{\rm{MD}}}^*$ than$ \tau _{{\rm{Maxwell}}}^*$ . But the calculation of$ \tau _{{\rm{LC}}}^*$ needs to take into count the trajectories of all atoms in a certain time range, which takes a lot of time and computing resources. Finally, in order to characterize viscoelastic relaxation time more easily, Kramers’ rate theory is used to describe the dissociation and association of atoms, according to the radial distribution functions. And a method of predicting the viscoelasticity of the monoatomic Lennard-Jones system is proposed and established. The comparison of all the viscoelastic relaxation times obtained above shows that$ \tau _{{\rm{Maxwell}}}^*$ is quite different from$ \tau _{{\rm{MD}}}^*$ at low temperature in the monoatomic Lennard-Jones system. Compared with$ \tau _{{\rm{Maxwell}}}^*$ ,$ \tau _{{\rm{LC}}}^*$ is close to$ \tau _{{\rm{MD}}}^*$ . But the calculation of$ \tau _{{\rm{LC}}}^*$ requires a lot of time and computing resources. Most importantly, the relaxation time calculated by our proposed method is closer to$ \tau _{{\rm{MD}}}^*$ . The method of predicting the viscoelastic relaxation time of the monoatomic Lennard-Jones system is accurate and reliable, which provides a new idea for studying the viscoelastic relaxation time of materials.-
Keywords:
- Lennard-Jones system /
- molecular simulation /
- viscoelasticity /
- relaxation time /
- prediction method
[1] 何平笙, 杨海洋, 朱平平 2004 化学通报 9 705
 Google Scholar
Google Scholar
He P S, Yang H Y, Zhu P P 2004 Chem. Bull. 9 705
 Google Scholar
Google Scholar
[2] 金日光, 华幼卿 2007 高分子物理 (北京: 化学工业出版社) 第123页
Jin R G, Hua Y Q 2007 Polymer Physics (Beijing: Chemical Industry Press) p123 (in Chinese)
[3] Winter H H 1987 Polym. Eng. Sci. 27 1698
 Google Scholar
Google Scholar
[4] Sunthar P 2010 Rheology of Complex Fluids (New York: Springer-Verlag) pp171−191
[5] Agrawal V, Holzworth K, Nantasetphong W, Amirkhizi V A, Oswald J, Nemat-Nasser 2016 J. Polym. Sci., Part B: Polym. Phys. 54 797
 Google Scholar
Google Scholar
[6] Hattkamp R, Daivids P J, Todd B D 2013 Phys. Rev. E 87 032155
 Google Scholar
Google Scholar
[7] Green M S 1954 J. Chem. Phys. 22 398
 Google Scholar
Google Scholar
[8] Kudo R, Toda M, Hashitsume N 2008 Statistical Physics II (Berlin: Springer-Verlag) pp109−145
[9] Guillaud E, Merabia S, De Ligny D, Joly L 2017 Phys. Chem. Chem. Phys. 19 2124
 Google Scholar
Google Scholar
[10] Iwashita T, Egami T 2012 Phys. Rev. Lett. 108 196001
 Google Scholar
Google Scholar
[11] Iwashita T, Nicholson D M, Egami T 2013 Phys. Rev. Lett. 110 205504
 Google Scholar
Google Scholar
[12] Ashwin J, Sen A 2015 Phys. Rev. Lett. 114 055002
 Google Scholar
Google Scholar
[13] Maxwell J C 1867 Philos. Trans. R. Soc. A 157 49
 Google Scholar
Google Scholar
[14] 陈艳 2006 硕士学位论文 (兰州: 西北师范大学)
Chen Y 2006 M. S. Thesis (Lanzhou: Northwest Normal University) (in Chines)
[15] Meier K, Laesecke A, Kabelac S 2004 J. Chem. Phys. 121 3671
 Google Scholar
Google Scholar
[16] Plimption S 1995 J. Comput. Phys. 117 1
 Google Scholar
Google Scholar
[17] Verlet L 1967 Phys. Rev. 159 98
 Google Scholar
Google Scholar
[18] Takahashi K, Yasuoka K, Narumi T 2007 J. Chem. Phys. 127 044107
 Google Scholar
Google Scholar
[19] Frenkel D, Smit B 2002 Understanding Molecular Simulation, Second Edition: From Algorithms to Applications (New York: Academic Press) p589
[20] Frantz Dale B, Plimpton S J, Shephard M S 2010 Eng. Comput. 26 205
 Google Scholar
Google Scholar
[21] Boon J P, Yip S, Percus J K 1980 Molecular Hydrodynamics (New York: Dover Publications) p285
[22] Hattemer G D, Arya G 2015 Macromol. 48 1240
 Google Scholar
Google Scholar
[23] Barnes S C, Lawless B M, Shepherd D E T, Espino D M, Bicknell G R, Bryan R T 2016 J. Mech. Behav. Biomed. 61 250
 Google Scholar
Google Scholar
[24] Heyes D M, Dini D, Brańka A C 2015 Phys. Status Solidi B 252 1514
 Google Scholar
Google Scholar
[25] Abidine Y, Laurent V M, Michel R 2015 Eur. Phys. J. Plus 130 202
 Google Scholar
Google Scholar
[26] Michalet, Xavier 2010 Phys. Rev. E 82 041914
 Google Scholar
Google Scholar
[27] Galliéro, Guillaume, Boned C, Baylaucq A 2005 Ind. Eng. Chem. Res. 44 6963
 Google Scholar
Google Scholar
[28] Morsali A, Goharshadi E K, Mansoori G A, Abbaspour M 2005 Chem. Phys. 310 11
 Google Scholar
Google Scholar
[29] Peter Hänggi, Talkner P, Borkovec M 1990 Rev. Mod. Phys. 62 251
 Google Scholar
Google Scholar
-
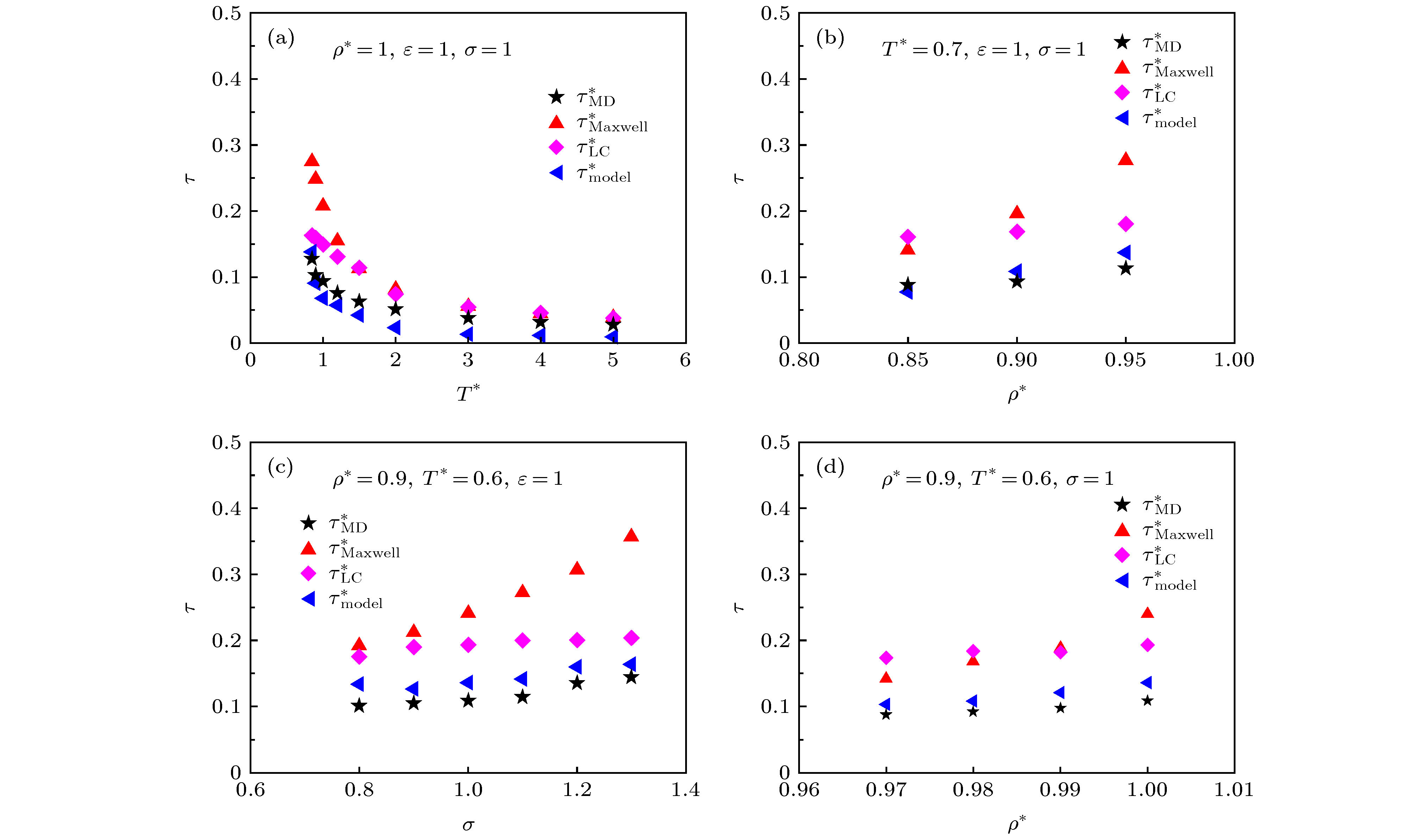
图 6 不同温度、密度以及L-J势参数下
$ {\tau }_{\rm{Maxwell}}^{*} $ ,$ {\tau }_{\rm{LC}}^{*} $ ,$ {\tau }_{\rm{MD}}^{*} $ ,$ {\tau }_{{\rm{m}}{\rm{o}}{\rm{d}}{\rm{e}}{\rm{l}}}^{*} $ (a)不同温度条件; (b)不同密度条件; (c)不同势能为0时的原子距离条件; (d)不同势能阱深度条件Fig. 6.
$ {\tau }_{\rm{Maxwell}}^{*} $ ,$ {\tau }_{\rm{LC}}^{*} $ ,$ {\tau }_{\rm{MD}}^{*} $ ,$ {\tau }_{{\rm{m}}{\rm{o}}{\rm{d}}{\rm{e}}{\rm{l}}}^{*} $ under different T,$ \rho $ , ε and σ: (a) Different temperatures; (b) different densities; (c) different distances between atoms when potential is 0; (d) different potential well depths. -
[1] 何平笙, 杨海洋, 朱平平 2004 化学通报 9 705
 Google Scholar
Google Scholar
He P S, Yang H Y, Zhu P P 2004 Chem. Bull. 9 705
 Google Scholar
Google Scholar
[2] 金日光, 华幼卿 2007 高分子物理 (北京: 化学工业出版社) 第123页
Jin R G, Hua Y Q 2007 Polymer Physics (Beijing: Chemical Industry Press) p123 (in Chinese)
[3] Winter H H 1987 Polym. Eng. Sci. 27 1698
 Google Scholar
Google Scholar
[4] Sunthar P 2010 Rheology of Complex Fluids (New York: Springer-Verlag) pp171−191
[5] Agrawal V, Holzworth K, Nantasetphong W, Amirkhizi V A, Oswald J, Nemat-Nasser 2016 J. Polym. Sci., Part B: Polym. Phys. 54 797
 Google Scholar
Google Scholar
[6] Hattkamp R, Daivids P J, Todd B D 2013 Phys. Rev. E 87 032155
 Google Scholar
Google Scholar
[7] Green M S 1954 J. Chem. Phys. 22 398
 Google Scholar
Google Scholar
[8] Kudo R, Toda M, Hashitsume N 2008 Statistical Physics II (Berlin: Springer-Verlag) pp109−145
[9] Guillaud E, Merabia S, De Ligny D, Joly L 2017 Phys. Chem. Chem. Phys. 19 2124
 Google Scholar
Google Scholar
[10] Iwashita T, Egami T 2012 Phys. Rev. Lett. 108 196001
 Google Scholar
Google Scholar
[11] Iwashita T, Nicholson D M, Egami T 2013 Phys. Rev. Lett. 110 205504
 Google Scholar
Google Scholar
[12] Ashwin J, Sen A 2015 Phys. Rev. Lett. 114 055002
 Google Scholar
Google Scholar
[13] Maxwell J C 1867 Philos. Trans. R. Soc. A 157 49
 Google Scholar
Google Scholar
[14] 陈艳 2006 硕士学位论文 (兰州: 西北师范大学)
Chen Y 2006 M. S. Thesis (Lanzhou: Northwest Normal University) (in Chines)
[15] Meier K, Laesecke A, Kabelac S 2004 J. Chem. Phys. 121 3671
 Google Scholar
Google Scholar
[16] Plimption S 1995 J. Comput. Phys. 117 1
 Google Scholar
Google Scholar
[17] Verlet L 1967 Phys. Rev. 159 98
 Google Scholar
Google Scholar
[18] Takahashi K, Yasuoka K, Narumi T 2007 J. Chem. Phys. 127 044107
 Google Scholar
Google Scholar
[19] Frenkel D, Smit B 2002 Understanding Molecular Simulation, Second Edition: From Algorithms to Applications (New York: Academic Press) p589
[20] Frantz Dale B, Plimpton S J, Shephard M S 2010 Eng. Comput. 26 205
 Google Scholar
Google Scholar
[21] Boon J P, Yip S, Percus J K 1980 Molecular Hydrodynamics (New York: Dover Publications) p285
[22] Hattemer G D, Arya G 2015 Macromol. 48 1240
 Google Scholar
Google Scholar
[23] Barnes S C, Lawless B M, Shepherd D E T, Espino D M, Bicknell G R, Bryan R T 2016 J. Mech. Behav. Biomed. 61 250
 Google Scholar
Google Scholar
[24] Heyes D M, Dini D, Brańka A C 2015 Phys. Status Solidi B 252 1514
 Google Scholar
Google Scholar
[25] Abidine Y, Laurent V M, Michel R 2015 Eur. Phys. J. Plus 130 202
 Google Scholar
Google Scholar
[26] Michalet, Xavier 2010 Phys. Rev. E 82 041914
 Google Scholar
Google Scholar
[27] Galliéro, Guillaume, Boned C, Baylaucq A 2005 Ind. Eng. Chem. Res. 44 6963
 Google Scholar
Google Scholar
[28] Morsali A, Goharshadi E K, Mansoori G A, Abbaspour M 2005 Chem. Phys. 310 11
 Google Scholar
Google Scholar
[29] Peter Hänggi, Talkner P, Borkovec M 1990 Rev. Mod. Phys. 62 251
 Google Scholar
Google Scholar
计量
- 文章访问数: 11120
- PDF下载量: 124
- 被引次数: 0


























 下载:
下载: