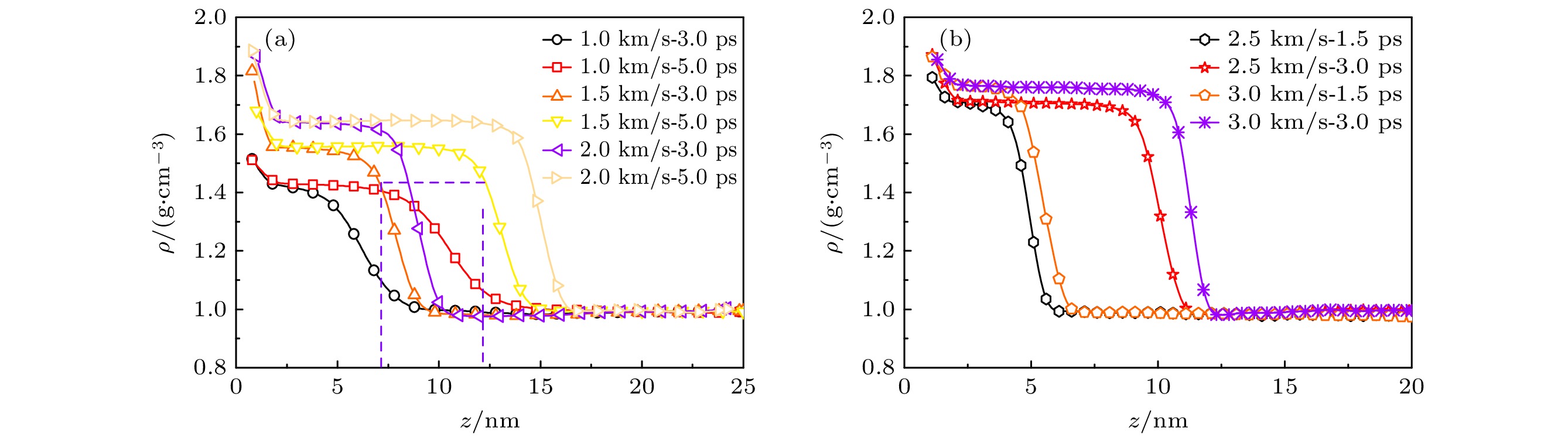
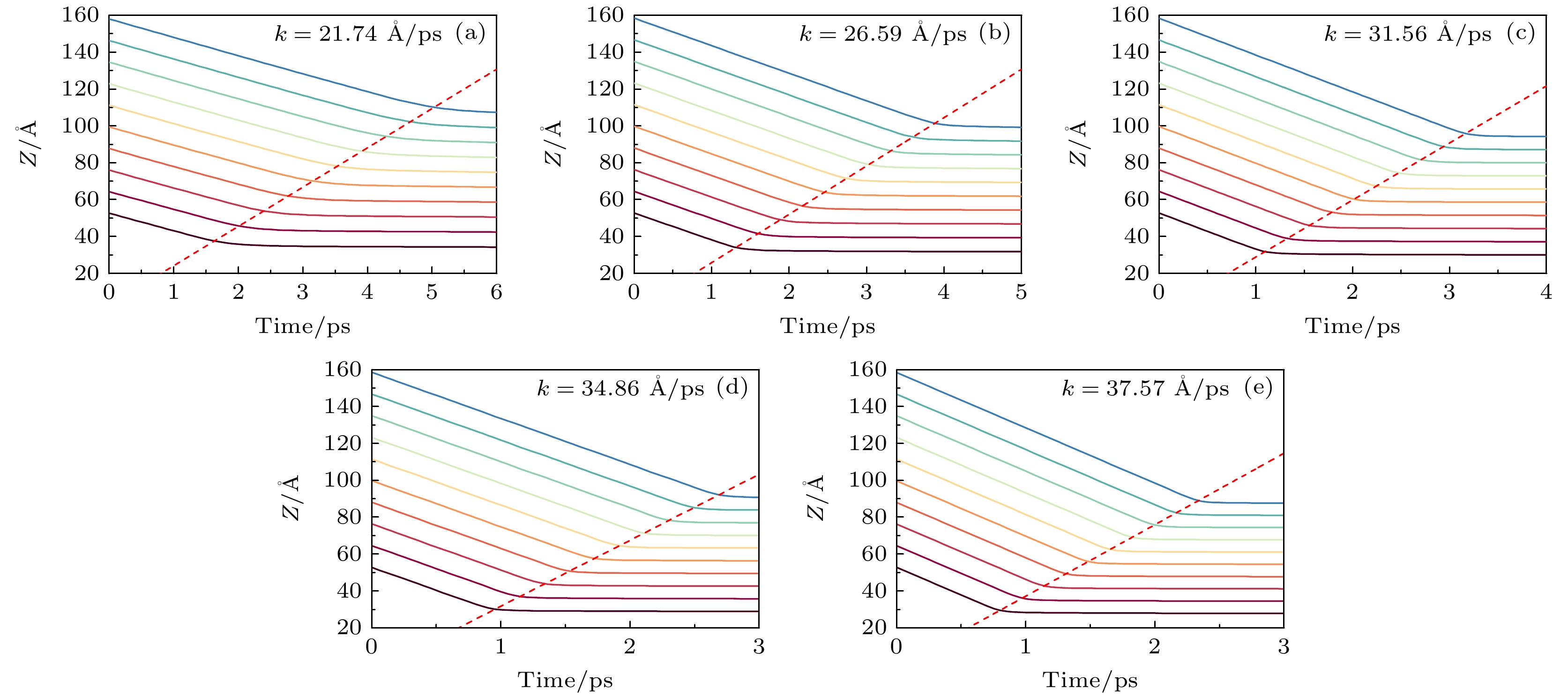
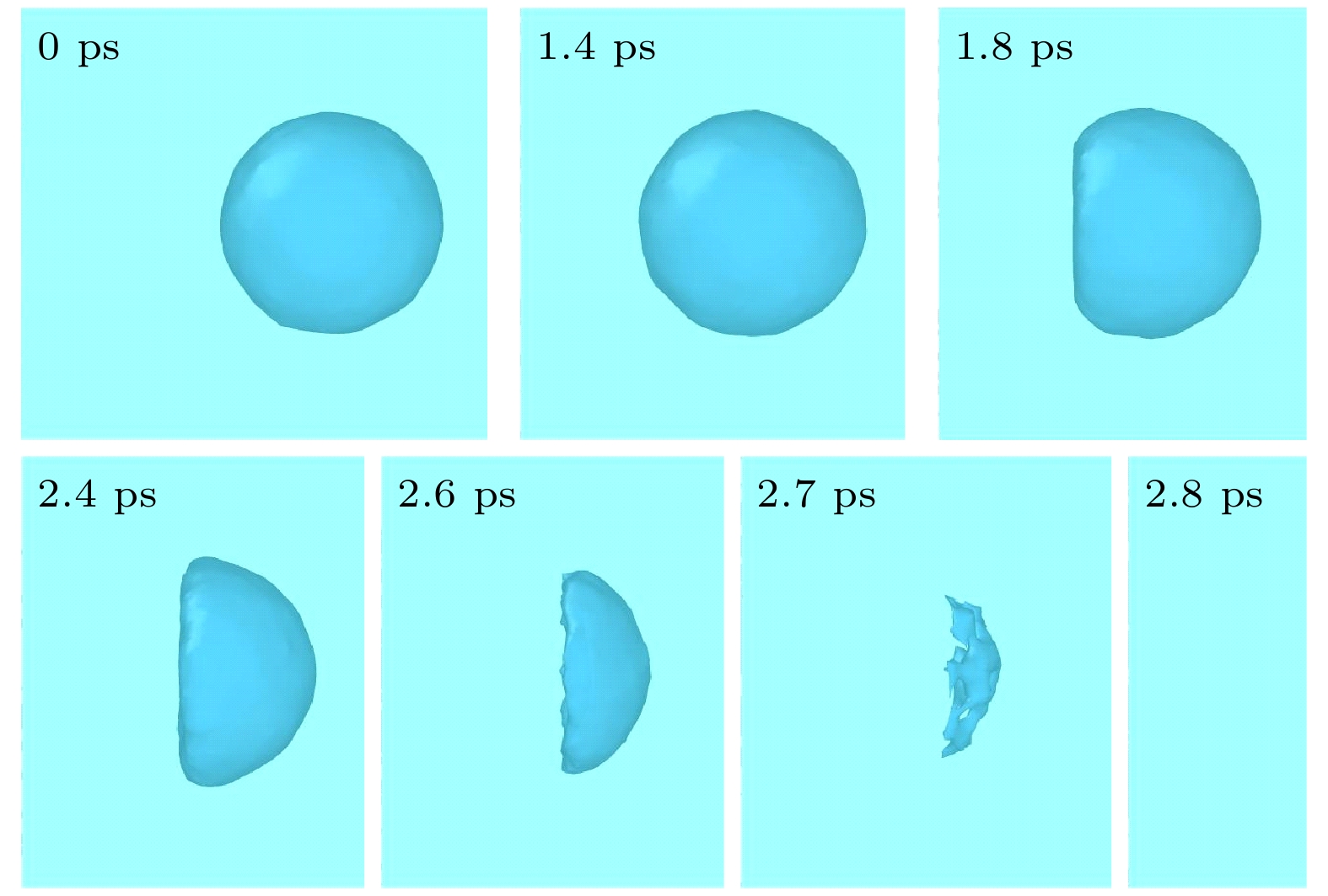
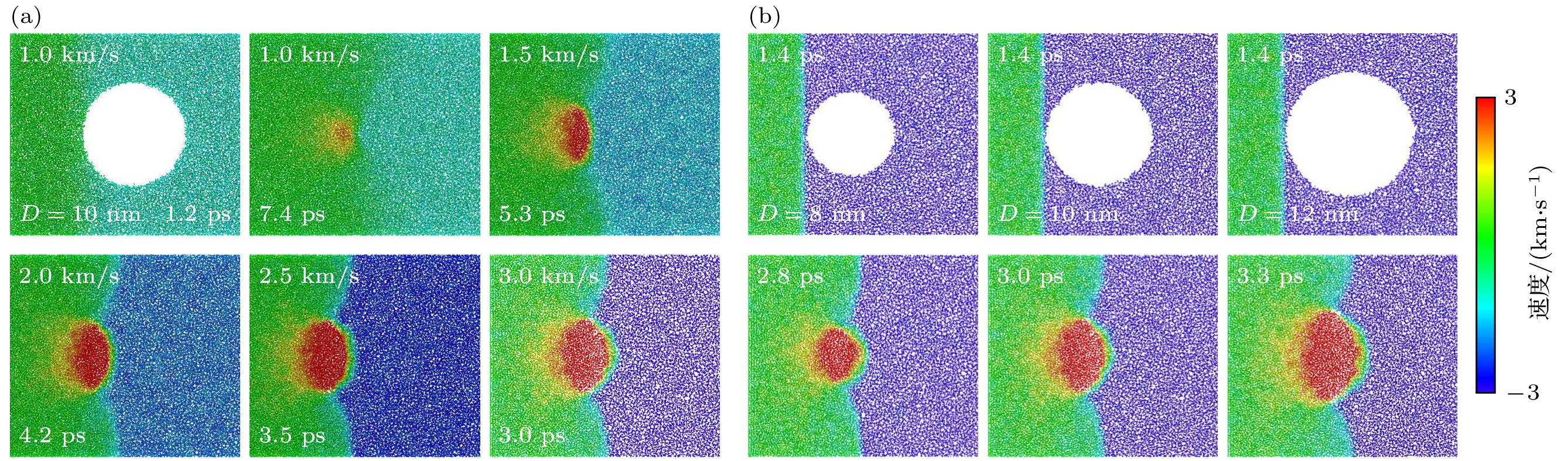
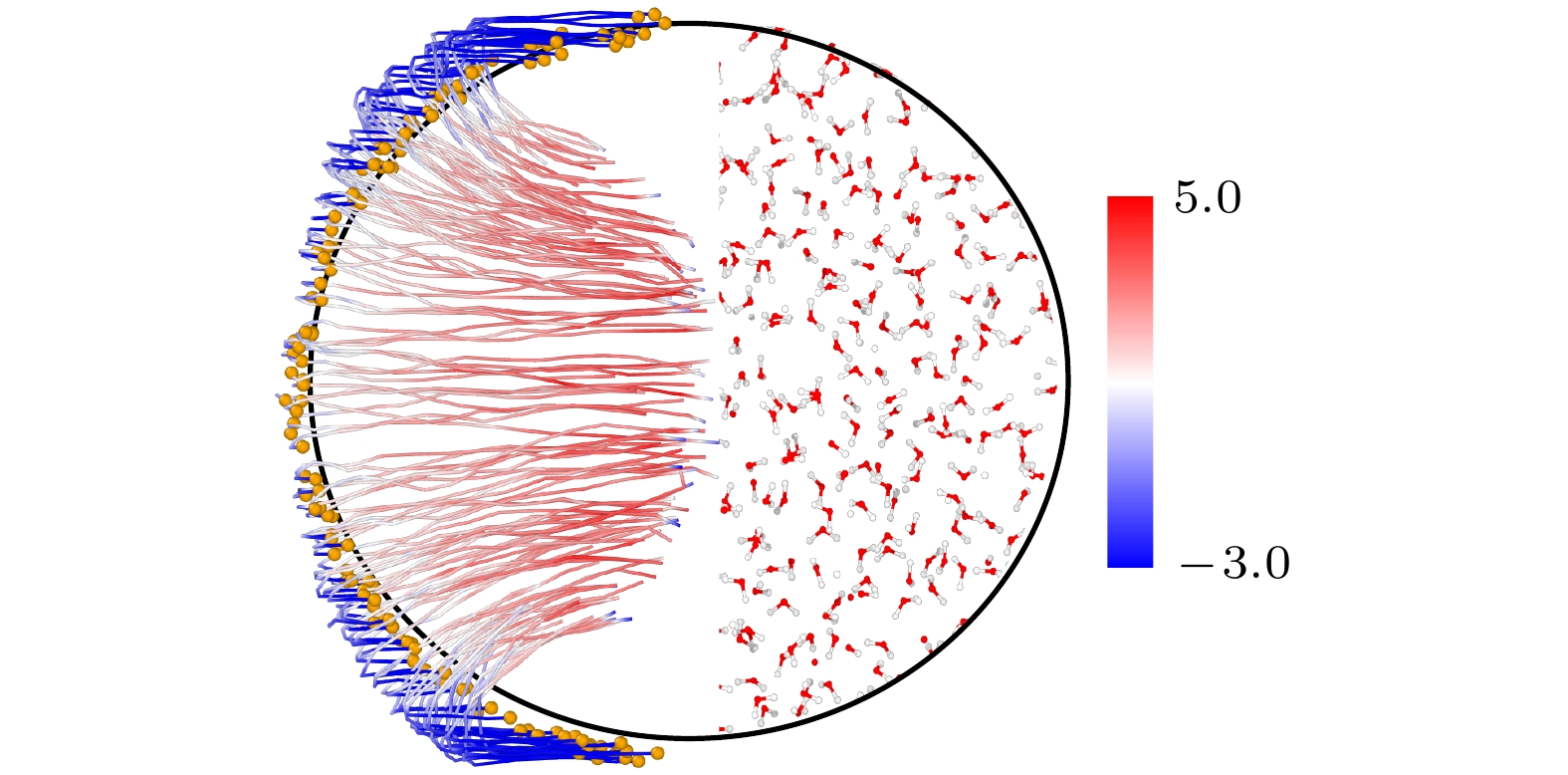
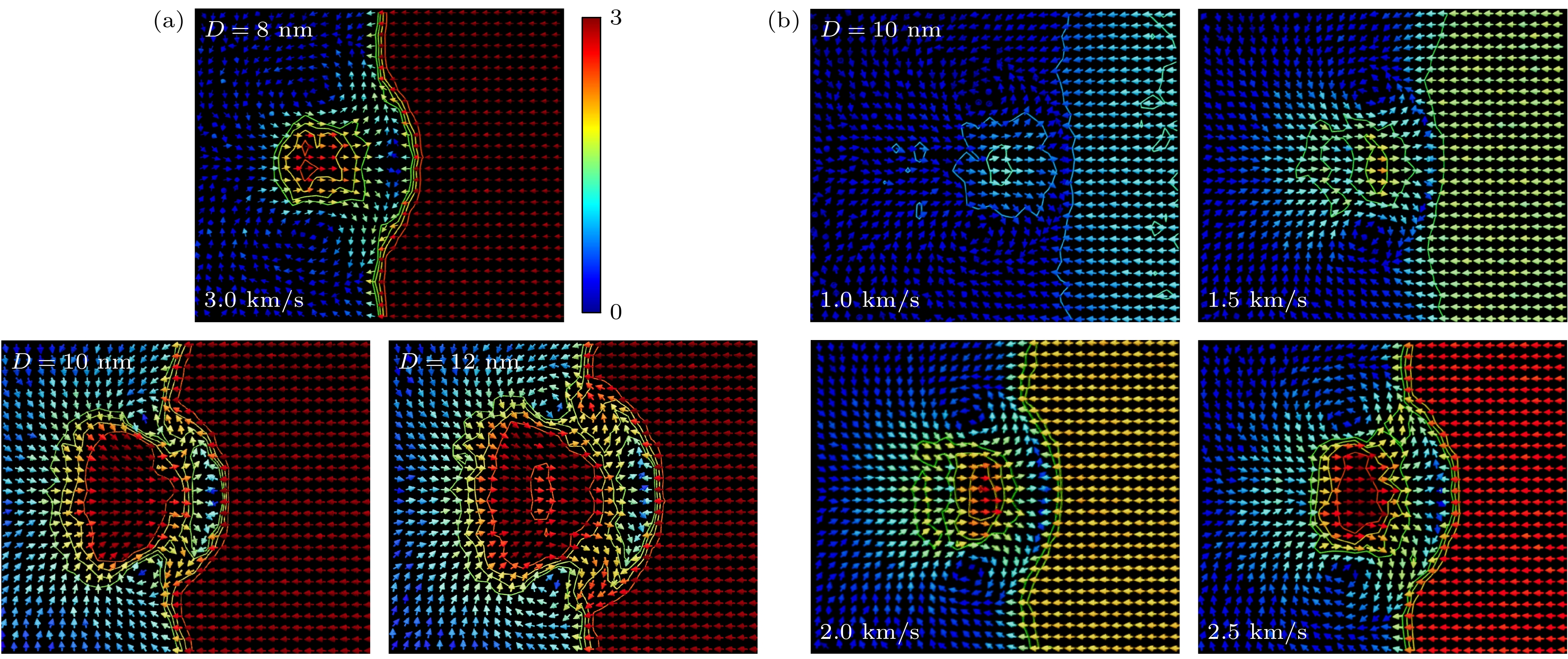
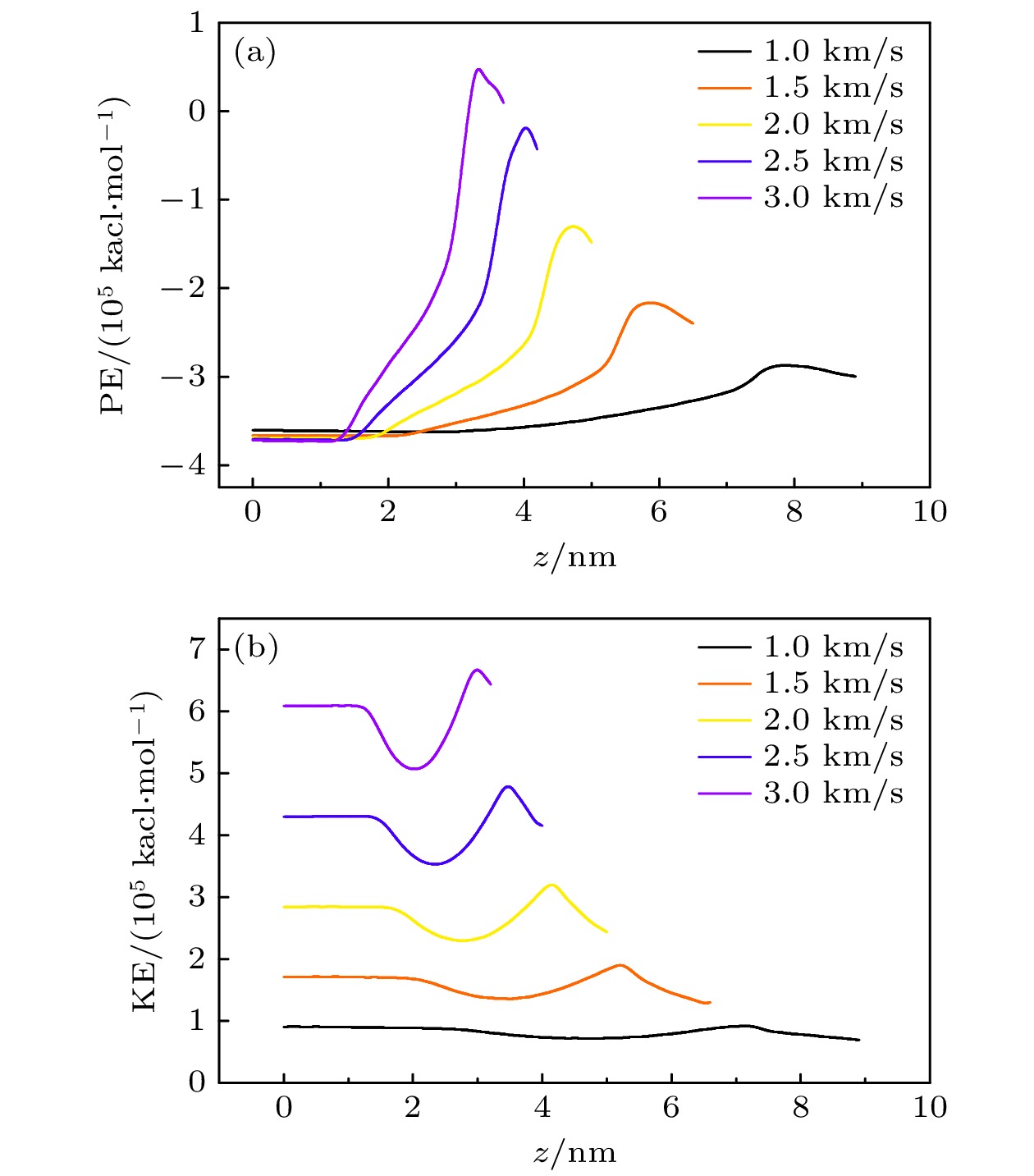
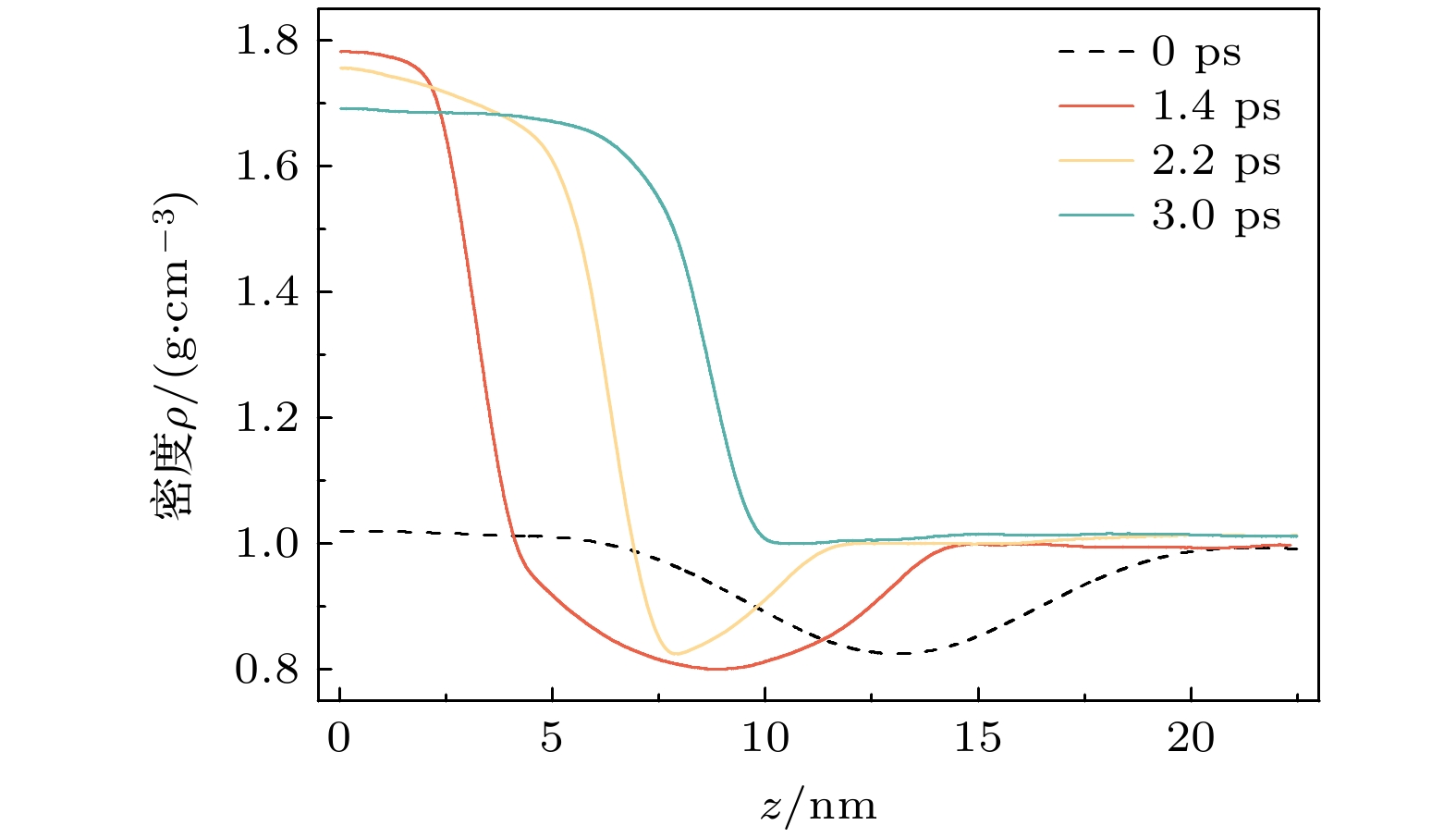
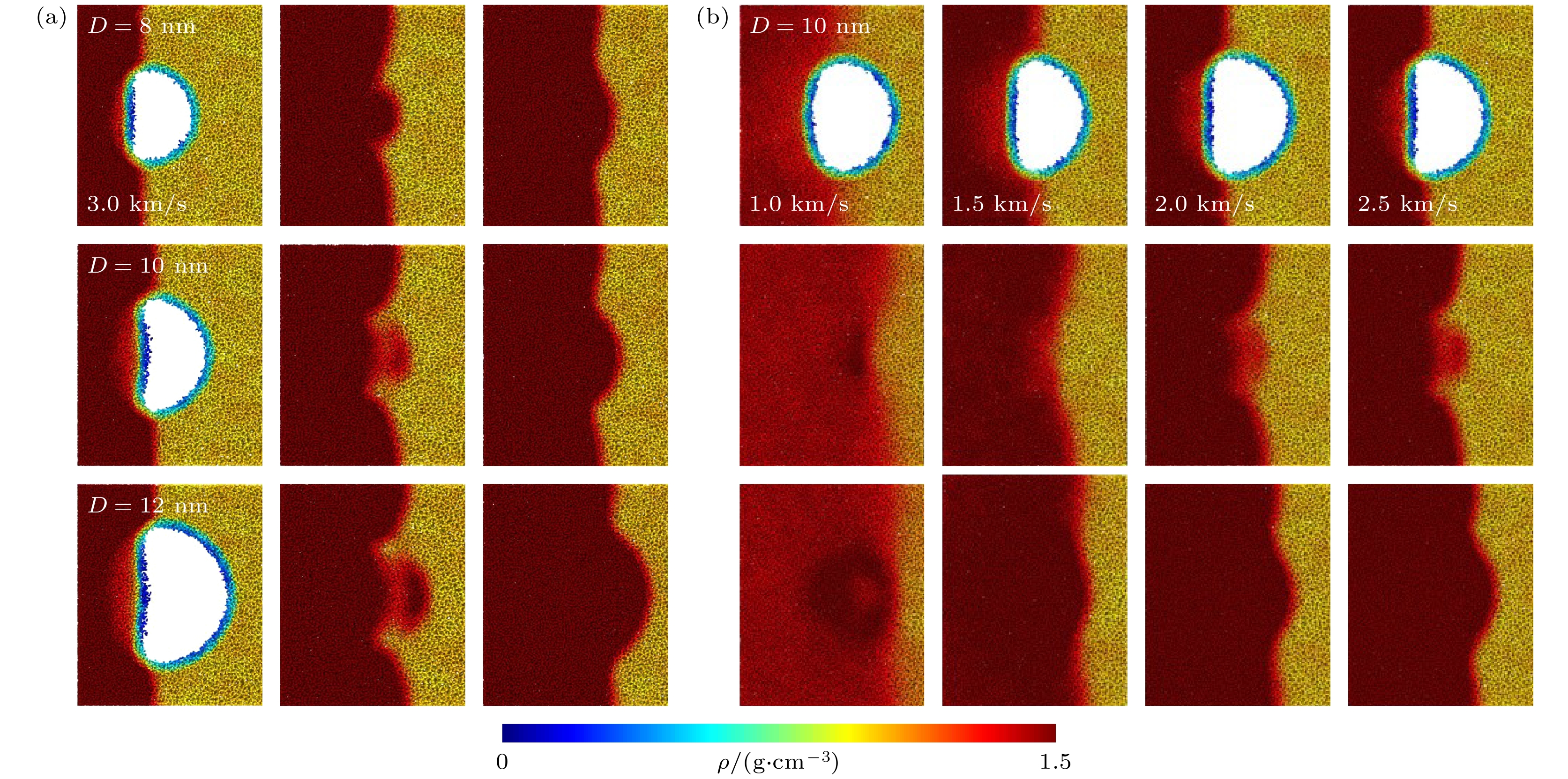
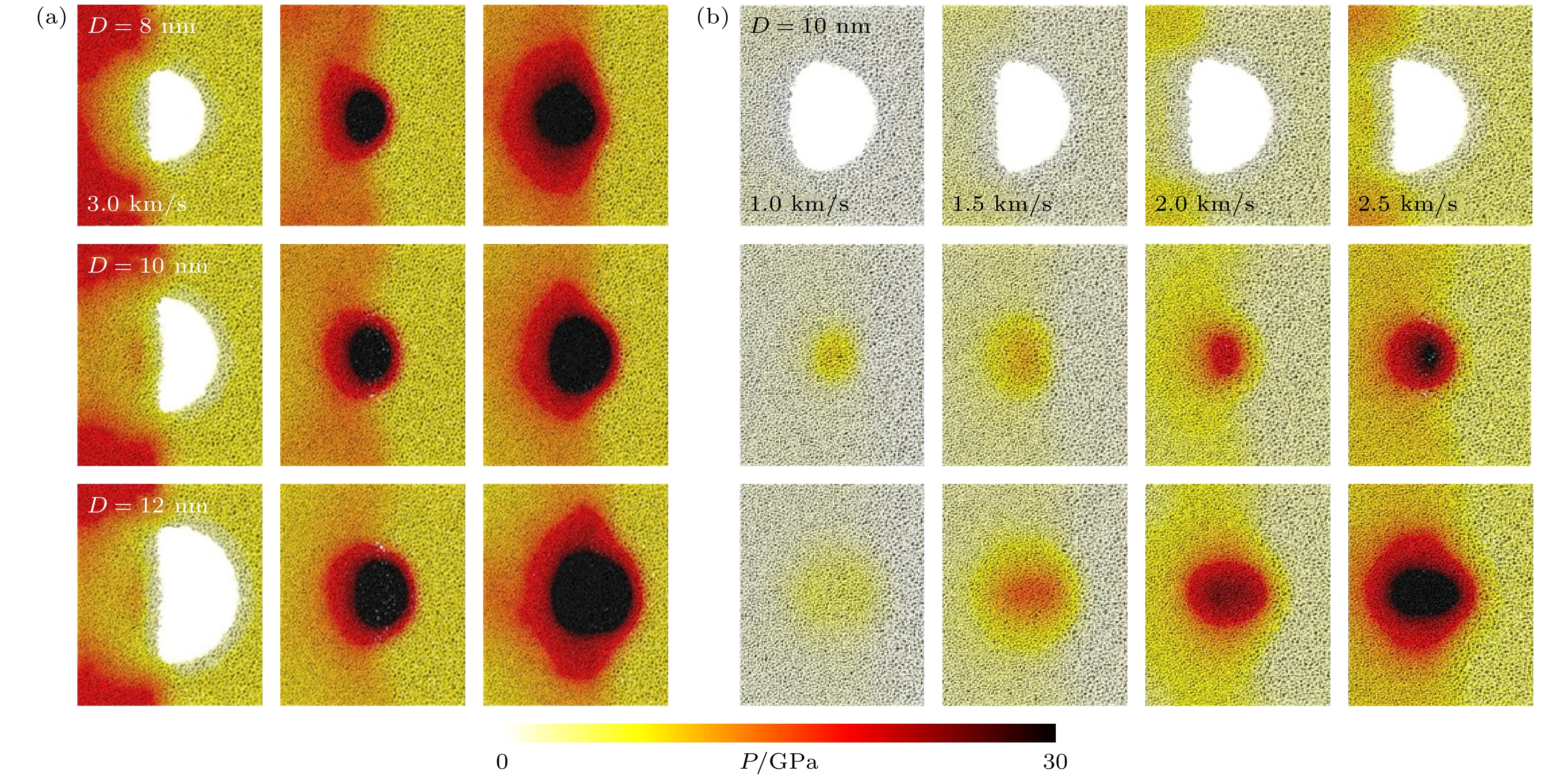
-
采用分子动力学模拟方法研究纳米气泡逐渐凹陷并发展至溃灭的过程, 本文主要研究冲击速度和气泡尺寸对纳米气泡溃灭的动力学特性影响机制. 结果表明: 纳米气泡溃灭大体上经历三个阶段. 首先是气泡外侧水分子压缩阶段, 然后是冲击波导致液膜稳定结构被破坏阶段, 最终发展至气泡完全溃灭阶段; 在冲击速度较大时, 较小尺寸气泡在更强的冲击效果作用下, 气泡溃灭时间更短; 纳米气泡溃灭后高速射流后在速度等高线右端形成凸起, 随着气泡尺寸和冲击速度增大, 凸起程度就越大, 水分子向气泡中心汇集, 在气泡上方和下方形成涡旋结构, 有效的增强了流体内部传质作用; 随着气泡尺寸和冲击速度的增大, 气泡周围密度也逐渐增大, 气泡完全时溃灭时局部密度可达1.5 g/cm3附近; 当气泡体系衰减至一半时, 出现水锤冲击效应, 随着气泡尺寸和冲击速度的增大, 水锤冲击作用愈发明显, 对于up = 3.0 km/s, D = 10 nm的纳米气泡结构塌陷后射流水锤冲击所形成的局部压强可达30 GPa.This study employs molecular dynamics simulations to investigate the process of nanobubble gradual indentation and eventual collapse. The research primarily focuses on the mechanisms by which impact velocity and bubble size influence the dynamic characteristics of nanobubble collapse. The results indicate that nanobubble collapse generally proceeds through three stages. Initially, there is a compression phase of water molecules surrounding the bubble, followed by a phase where the shock wave disrupts the stable structure of the liquid film, and finally, the complete collapse of the bubble. At higher impact velocities, smaller bubbles collapse more rapidly due to stronger shock effects. Post-collapse, a high-speed jet forms a protrusion on the right end of the velocity contour. The degree of protrusion increases with bubble size and impact velocity. Water molecules converge towards the bubble center, forming vortex structures above and below the bubble, effectively enhancing internal mass transfer. As bubble size and impact velocity increase, the density around the bubble gradually rises, reaching approximately 1.5 g/cm³ in localized areas upon complete collapse. When the bubble system decays to half its original size, a water hammer effect occurs. This effect becomes more pronounced with increasing bubble size and impact velocity. For a nanobubble structure with up = 3.0 km/s and D = 10 nm, the local pressure formed by the water hammer impact of the jet after collapse can reach 30 GPa.
-
Keywords:
- nanobubble /
- molecular dynamics simulations /
- bubble collapse /
- water hammer effect
[1] 马艳, 吴俊, 周维 2024 环境工程技术学报 14 1141
Google Scholar
Ma Y, Wu J, Zhou W 2024 J. Environ. Eng. Technol. 14 1141
Google Scholar
[2] 杨丽, 廖传华, 朱跃钊, 陈海军, 金勤芳 2012 化工进展 31 1333
Yang L, Liao C H, Zhu Y Z, Chen H J, Jin Q F 2012 Chem. Ind. Eng. Prog. 31 1333
[3] 张立娟, 郑晋, 文博, 胡钧 2024 中国科学: 化学 54 85
Google Scholar
Zhang L J, Zheng J, Wen B, Hu J 2024 Sci. Sin. Chem. 54 85
Google Scholar
[4] 张敏, 宋昭峥, 孙珊珊, 张志勇, 穆红岩, 赵立平, 李永峰, 张忠智 2016 环境工程学报 10 599
Google Scholar
Zhang M, Song Z Z, Sun S S, Zhang Z Y, Mu H Y, Zhao L P, Li Y F, Zhang Z Z 2016 Chin. J. Environ. Eng. 10 599
Google Scholar
[5] 翟伟哲, 王永刚, 王旭, 董婧, 王恒嘉 2018 环境科学与管理 43 95
Google Scholar
Zhai W Z, Wang Y G, Wang X, Dong J, Wang H J 2018 Environ. Sci. Manage. 43 95
Google Scholar
[6] 李恒震, 胡黎明, 辛鸿博 2015 岩土工程学报 37 115
Google Scholar
Li H Z, Hu L M, Xin H B 2015 Chin. J. Geotech. Eng. 37 115
Google Scholar
[7] Cook S S 1928 Proc. R. Soc. London, Ser. A 119 481
Google Scholar
[8] Obara T B, Bourne N K, Field J E 1995 Wear 186 388
[9] 詹胜鹏 2022 博士学位论文 (北京: 机械科学研究总院)
Zhan S P 2022 Ph. D Dissertation (Beijing: Academy of Machinery Science and Technology
[10] 王小峰, 陶钢, 徐宁, 王鹏, 李召, 闻鹏 2021 70 134702
Google Scholar
Wang X F, Tao G, Xu N, Wang P, Li Z, Wen P. 2021 Acta Phys. Sin. 70 134702
Google Scholar
[11] Rawat S 2023 Phys. Fluids 35 097114
Google Scholar
[12] Vedadi M H, Haas S 2011 Appl. Phys. Lett. 99 154105
Google Scholar
[13] Zhou Y, Cao D, Zhang X 2022 Nanomaterials 12 2654
Google Scholar
[14] Nan N, Si D, Hu G 2018 J. Chem. Phys. 149 074902
Google Scholar
[15] Wang X F, Tao G, Wen P, Ren B X, Pang C Q, Du C X 2020 J. Phys. Chem. B 124 9535
Google Scholar
[16] Lu X, Yuan B, Zhang X, Yang K, Ma Y 2017 Appl. Phys. Lett. 110 023701
Google Scholar
[17] Thompson A P, Aktulga H M, Berger R, Bolintineanu D S, Brown W M, Crozier P S, In ’T Veld P J, Kohlmeyer A, Moore S G, Nguyen T D, Shan R, Stevens M J, Tranchida J, Trott C, Plimpton S J 2022 Comput. Phys. Commun. 271 108171
Google Scholar
[18] Stukowski A 2010 Modell. Simul. Mater. Sci. Eng. 18 015012
Google Scholar
[19] Berendsen H J C, Grigera J R, Straatsma T P 1987 J. Phys. Chem. 91 6269
Google Scholar
[20] Zhou Y, Huang M, Tian F, Shi X, Zhang X 2024 J. Phys. Chem. 160 054109
Google Scholar
[21] Rybakov A P, Rybakov I A 1995 Eur. J. Mech. B Fluids 14 323
[22] Vedadi M, Choubey A, Nomura K, Kalia R K, Nakano A, Vashishta P, Van Duin A C T 2010 Phys. Rev. Lett. 105 014503
Google Scholar
[23] Hołyst R, Litniewski M, Garstecki P 2010 Phys. Rev. E 82 066309
Google Scholar
[24] Zhang A M, Cui P, Wang Y 2013 Exp. Fluids 54 1602
Google Scholar
[25] Zhang H, Lu Z, Zhang P, Gu J, Luo C, Tong Y, Ren X 2021 Opt. Laser Technol. 138 106606
Google Scholar
[26] Zhan S, Duan H, Pan L, Tu J, Jia D, Yang T, Li J 2021 Phys. Chem. Chem. Phys. 23 8446
Google Scholar
-
图 2 纯水体系在不同冲击速度条件下沿z轴一维密度分布 (a) 1.0—2.0 km/s; (b) 2.5—3.0 km/s
Fig. 2. One-dimensional density distribution along the z-axis in the pure water system under different impact velocities:(a) 1.0–2.0 km/s; (b) 2.5–3.0 km/s.
图 3 纯水体系在不同冲击速度条件下沿z轴一维密度分布曲线 (a) 1.0 km/s; (b) 1.5 km/s; (c) 2.0 km/s; (d) 2.5 km/s; (e) 3.0 km/s
Fig. 3. One-dimensional density distribution curves along the z-axis in the pure water system under different impact velocities: (a) 1.0 km/s; (b) 1.5 km/s; (c) 2.0 km/s; (d) 2.5 km/s; (e) 3.0 km/s.
图 5 纳米气泡体积归一化Ω(t)随冲击时间变化关系曲线 (a) 不同冲击速度; (b) 不同气泡尺寸
Fig. 5. Normalized Ω(t) curves of nanobubble volume as a function of impact time: (a) Different impact velocitys; (b) different nanobubble sizes.
图 6 不同时刻下的纳米气泡结构变化情况(D = 10 nm, up = 3.0 km/s)
Fig. 6. Changes in nanobubble structure at different times (D = 10 nm, up = 3.0 km/s).
图 7 纳米气泡溃灭前后的z 方向速度分布情况 (a) 不同冲击速度; (b) 不同气泡尺寸
Fig. 7. z-direction velocity distribution before and after the collapse of the nanobubble: (a) Different impact velocities; (b) different bubble sizes.
图 8 1.4—3.0 ps纳米气泡外层液膜的运动轨迹曲线(单位: km/s)
Fig. 8. Motion trajectory curve of outer liquid film of 1.4–3.0 ps nanobubble (unit: km/s).
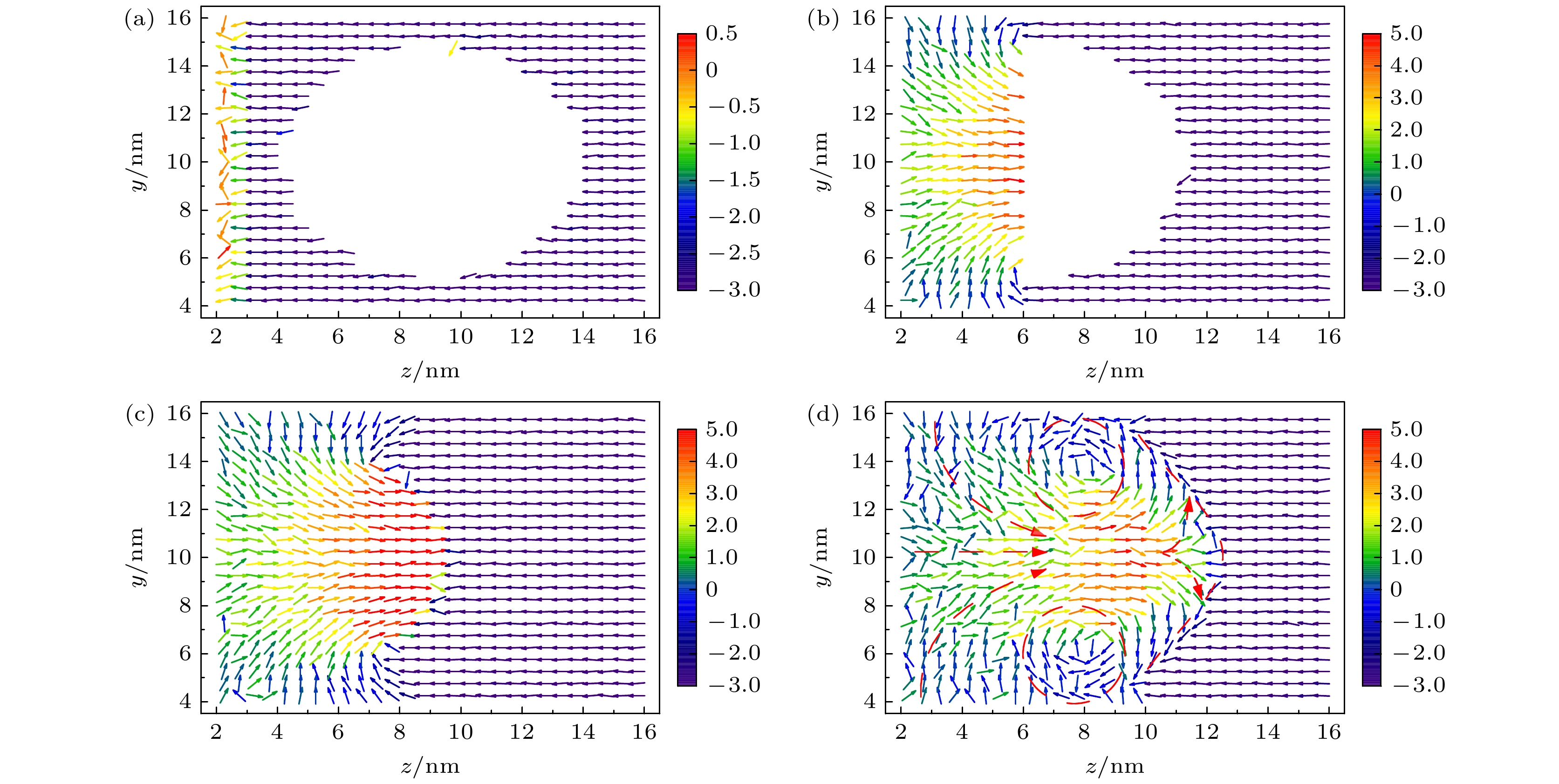
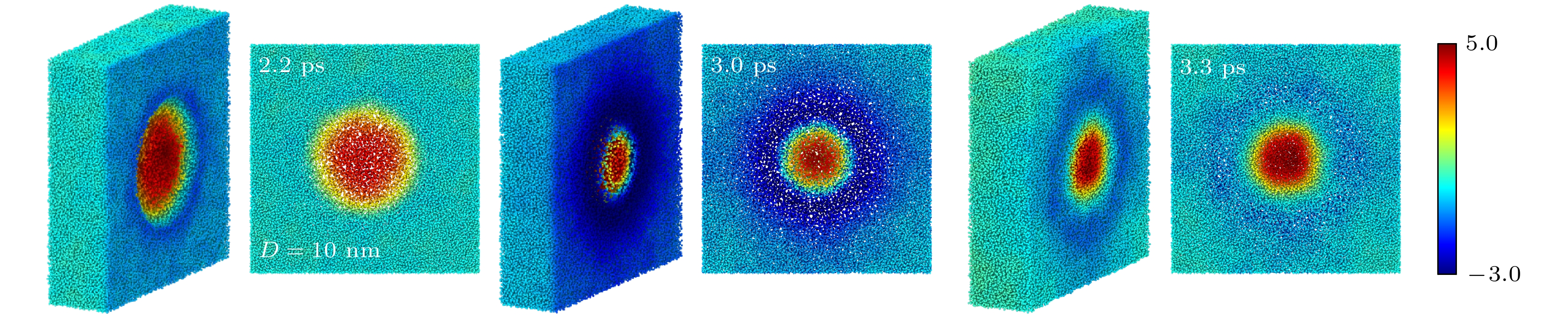
图 9 up = 3.0 km/s, D = 10 nm纳米气泡二维流场(y-z平面)速度矢量分布 (a) 1.3 ps; (b) 2.2 ps; (c) 2.8 ps; (d) 3.4 ps (单位: km/s, 由于比例问题, 气泡呈椭圆形)
Fig. 9. Velocity vector distribution of two-dimensional flow field (y-z plane) of nanobubble with up = 3.0 km/s and D = 10 nm: (a) 1.3 ps; (b) 2.2 ps; (c) 2.8 ps; (d) 3.4 ps (unit: km/s, due to proportional issues, the bubble is elliptical in shape).
图 10 不同气泡尺寸及冲击速度大小的气泡流场速度分布 (a) 不同气泡尺寸; (b) 不同冲击速度
Fig. 10. Velocity distribution of the bubble flow field at different bubble sizes and impact velocities: (a) Different bubble sizes; (b) different impact velocities.
图 11 不同冲击速度下的体系势能PE (a)和动能KE (b)沿z轴一维分布曲线(3.3 ps)
Fig. 11. One dimensional distribution curves of system potential energy PE (a) and kinetic energy KE (b) along the z-axis at different impact velocities (3.3 ps).
图 12 纳米气泡周围水分子质量密度沿z轴一维分布曲线
Fig. 12. One dimensional distribution curve of water molecule mass density around nanobubble along the z-axis.
图 13 纳米气泡周围水分子质量密度的y-z平面二维云图 (a) 不同气泡尺寸; (b) 不同冲击速度
Fig. 13. Two dimensional cloud map of y-z plane of water molecule mass density around nanobubble: (a) Different nanobubble sizes; (b) different impact velocities.
图 14 纳米气泡射流前沿x-y平面(径向)二维云图(单位: km/s)
Fig. 14. Two dimensional cloud map of the front x-y plane (radial) of the nanobubble jet (unit: km/s).
图 15 纳米气泡射流产生局部压强的y-z平面二维云图 (a) 不同气泡尺寸; (b) 不同冲击速度
Fig. 15. Two dimensional cloud map of y-z plane generated local pressure by nanobubble jet: (a) Different nanobubble size; (b) different impact velocity.
表 1 SPC/E刚性分子模型势能参数
Table 1. Potential energy parameters of SPC/E rigid molecular model.
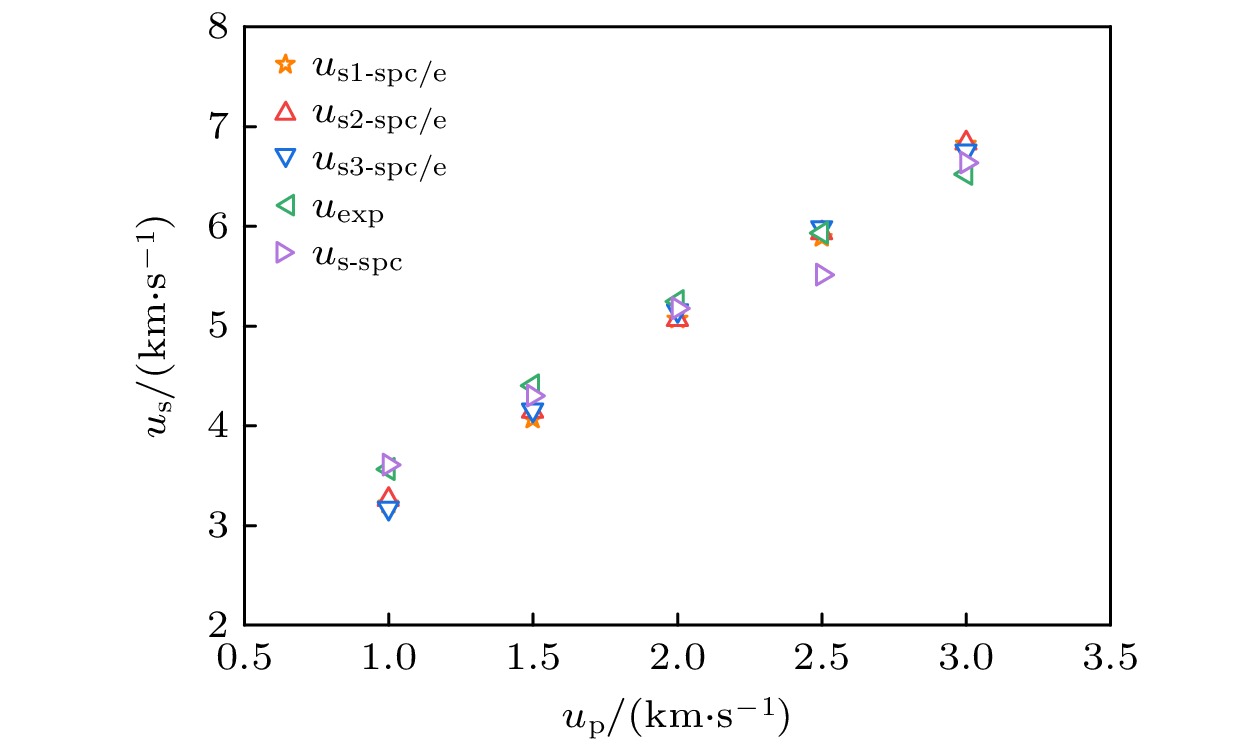
type ε/(kcal·mol–1) σ/Å q/e O 3.166 0.15535 –0.8476 H 0 0 0.4238 
下载: 导出CSV
表 2 不同条件下的粒子速度和冲击速度对应结果
Table 2. Corresponding results of particle velocity and impact velocity under different conditions.
up/(km·s–1) us1 us2 us3 uexp usim ε/% 1.0 3.21 3.26 3.17 3.57 3.61 9.8 1.5 4.06 4.14 4.16 4.40 4.30 6.4 2.0 5.07 5.06 5.16 5.25 5.18 2.9 2.5 5.89 5.93 5.99 5.93 5.51 0.6
下载: 导出CSV
表 3 不同粒子速度和尺寸下的纳米气泡破裂时间
Table 3. Breakdown time of nanobubble at different particle velocities and sizes.
粒子速度
up/(km·s–1)气泡尺寸
D/nm气泡破裂时间 τ/ps MD Rayleigh 差值 1.0 8 3.3 3.8 0.5 10 4.2 4.7 0.3 12 4.6 5.7 1.0 1.5 8 2.2 2.5 0.3 10 2.7 3.1 0.4 12 3.4 3.7 0.3 2.0 8 1.8 2.1 0.3 10 2.2 2.3 0.1 12 2.6 2.8 0.2 2.5 8 1.4 1.5 0.1 10 1.9 1.8 0.1 12 2.1 2.2 0.1 3.0 8 1.2 1.1 0.1 10 1.6 1.4 0.2 12 1.9 1.7 0.2
下载: 导出CSV
表 4 MD模拟和Rankine–Hugoniot计算冲击压力结果
Table 4. MD simulation and Rankine-Hugoniot calculation of impact pressure results.
粒子速度up/(km·s–1) 冲击速度us/(km·s–1) 冲击压力 Ps/GPa MD Rankine–
Hugoniot差值 1.0 3.22 3.09 3.20 0.11 1.5 4.12 6.16 6.18 0.02 2.0 5.09 10.19 10.18 0.01 2.5 5.93 14.96 14.82 0.14 3.0 6.80 20.63 20.40 0.23
下载: 导出CSV
-
[1] 马艳, 吴俊, 周维 2024 环境工程技术学报 14 1141
Google Scholar
Ma Y, Wu J, Zhou W 2024 J. Environ. Eng. Technol. 14 1141
Google Scholar
[2] 杨丽, 廖传华, 朱跃钊, 陈海军, 金勤芳 2012 化工进展 31 1333
Yang L, Liao C H, Zhu Y Z, Chen H J, Jin Q F 2012 Chem. Ind. Eng. Prog. 31 1333
[3] 张立娟, 郑晋, 文博, 胡钧 2024 中国科学: 化学 54 85
Google Scholar
Zhang L J, Zheng J, Wen B, Hu J 2024 Sci. Sin. Chem. 54 85
Google Scholar
[4] 张敏, 宋昭峥, 孙珊珊, 张志勇, 穆红岩, 赵立平, 李永峰, 张忠智 2016 环境工程学报 10 599
Google Scholar
Zhang M, Song Z Z, Sun S S, Zhang Z Y, Mu H Y, Zhao L P, Li Y F, Zhang Z Z 2016 Chin. J. Environ. Eng. 10 599
Google Scholar
[5] 翟伟哲, 王永刚, 王旭, 董婧, 王恒嘉 2018 环境科学与管理 43 95
Google Scholar
Zhai W Z, Wang Y G, Wang X, Dong J, Wang H J 2018 Environ. Sci. Manage. 43 95
Google Scholar
[6] 李恒震, 胡黎明, 辛鸿博 2015 岩土工程学报 37 115
Google Scholar
Li H Z, Hu L M, Xin H B 2015 Chin. J. Geotech. Eng. 37 115
Google Scholar
[7] Cook S S 1928 Proc. R. Soc. London, Ser. A 119 481
Google Scholar
[8] Obara T B, Bourne N K, Field J E 1995 Wear 186 388
[9] 詹胜鹏 2022 博士学位论文 (北京: 机械科学研究总院)
Zhan S P 2022 Ph. D Dissertation (Beijing: Academy of Machinery Science and Technology
[10] 王小峰, 陶钢, 徐宁, 王鹏, 李召, 闻鹏 2021 70 134702
Google Scholar
Wang X F, Tao G, Xu N, Wang P, Li Z, Wen P. 2021 Acta Phys. Sin. 70 134702
Google Scholar
[11] Rawat S 2023 Phys. Fluids 35 097114
Google Scholar
[12] Vedadi M H, Haas S 2011 Appl. Phys. Lett. 99 154105
Google Scholar
[13] Zhou Y, Cao D, Zhang X 2022 Nanomaterials 12 2654
Google Scholar
[14] Nan N, Si D, Hu G 2018 J. Chem. Phys. 149 074902
Google Scholar
[15] Wang X F, Tao G, Wen P, Ren B X, Pang C Q, Du C X 2020 J. Phys. Chem. B 124 9535
Google Scholar
[16] Lu X, Yuan B, Zhang X, Yang K, Ma Y 2017 Appl. Phys. Lett. 110 023701
Google Scholar
[17] Thompson A P, Aktulga H M, Berger R, Bolintineanu D S, Brown W M, Crozier P S, In ’T Veld P J, Kohlmeyer A, Moore S G, Nguyen T D, Shan R, Stevens M J, Tranchida J, Trott C, Plimpton S J 2022 Comput. Phys. Commun. 271 108171
Google Scholar
[18] Stukowski A 2010 Modell. Simul. Mater. Sci. Eng. 18 015012
Google Scholar
[19] Berendsen H J C, Grigera J R, Straatsma T P 1987 J. Phys. Chem. 91 6269
Google Scholar
[20] Zhou Y, Huang M, Tian F, Shi X, Zhang X 2024 J. Phys. Chem. 160 054109
Google Scholar
[21] Rybakov A P, Rybakov I A 1995 Eur. J. Mech. B Fluids 14 323
[22] Vedadi M, Choubey A, Nomura K, Kalia R K, Nakano A, Vashishta P, Van Duin A C T 2010 Phys. Rev. Lett. 105 014503
Google Scholar
[23] Hołyst R, Litniewski M, Garstecki P 2010 Phys. Rev. E 82 066309
Google Scholar
[24] Zhang A M, Cui P, Wang Y 2013 Exp. Fluids 54 1602
Google Scholar
[25] Zhang H, Lu Z, Zhang P, Gu J, Luo C, Tong Y, Ren X 2021 Opt. Laser Technol. 138 106606
Google Scholar
[26] Zhan S, Duan H, Pan L, Tu J, Jia D, Yang T, Li J 2021 Phys. Chem. Chem. Phys. 23 8446
Google Scholar
下载:
计量
- 文章访问数: 809
- PDF下载量: 34
- 被引次数: 0
