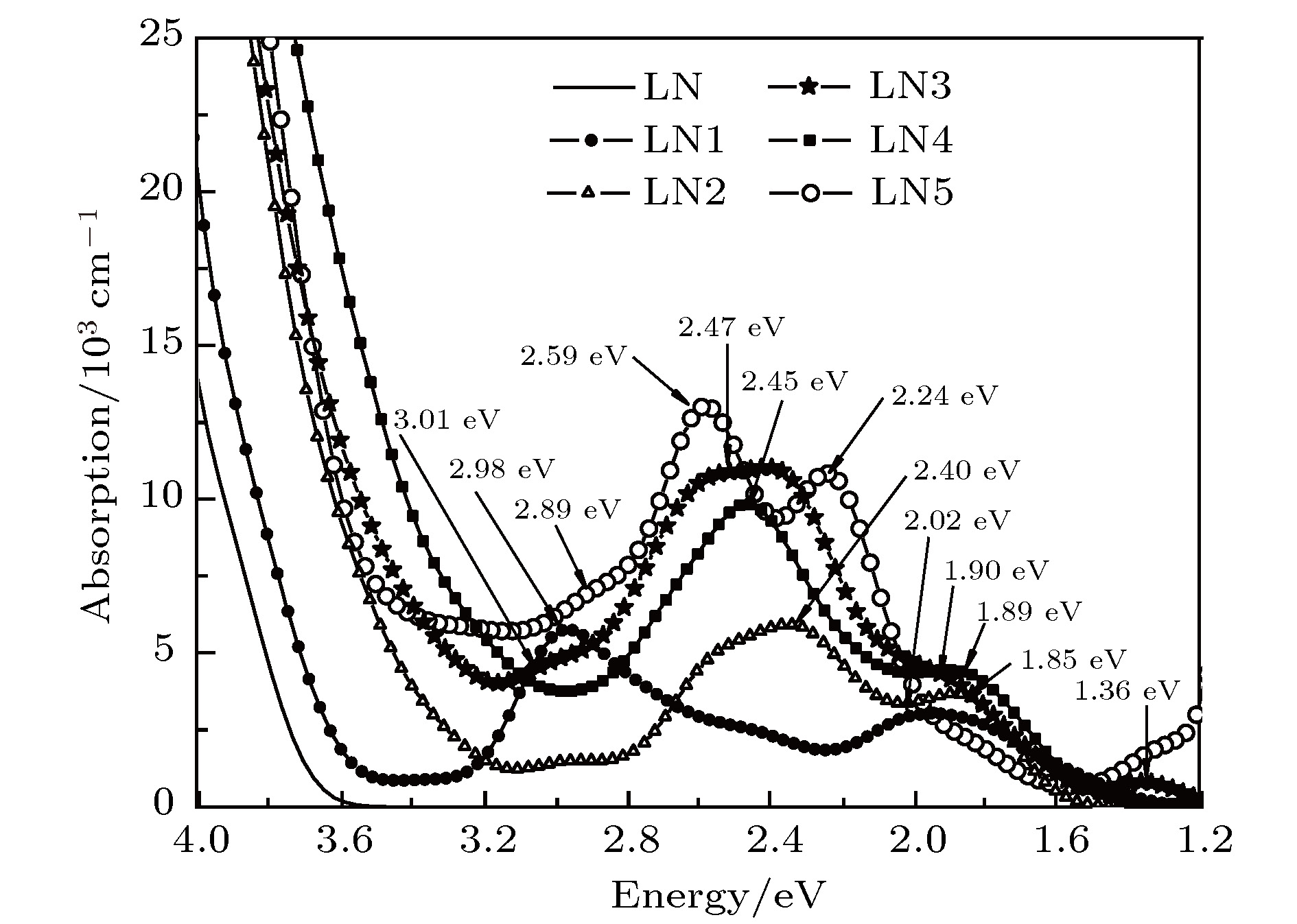
-
利用基于密度泛函理论的第一性原理, 研究了Cu:Fe:Mg:LiNbO3晶体及对比组的电子结构和光学特性. 研究显示, 单掺铜或铁铌酸锂晶体的杂质能级分别由Cu 3d轨道或Fe 3d轨道贡献, 禁带宽度分别为3.45和3.42 eV; 铜、铁共掺铌酸锂晶体杂质能级由Cu和Fe的3d轨道共同贡献, 禁带宽度为3.24 eV, 吸收峰分别在3.01, 2.53和1.36 eV处; Cu:Fe:Mg:LiNbO3晶体中Mg2+浓度低于阈值或高于阈值(阈值约为6.0 mol%)的禁带宽度分别为2.89 eV或3.30 eV, 吸收峰分别位于2.45 eV, 1.89 eV或2.89 eV, 2.59 eV, 2.24 eV. Mg2+浓度高于阈值, 会使吸收边较低于阈值情况红移; 并使得部分Fe3+占Nb位, 引起晶体场改变, 从而改变吸收峰位置和强度. 双光存储应用中可选取2.9 eV作为擦除光, 2.5 eV作为读取和写入光, 选取Mg2+浓度达到阈值的三掺晶体在增加动态范围和灵敏度等参量以及优化再现图像的质量等方面更具优势.In this paper the electronic structures and optical properties of Cu:Fe:Mg:LiNbO3 crystals and their comparative groups are investigated by first-principles based on the density functional theory to explore the characteristics of charge transfer in crystals and analyse the parameters of the two-colour holographic storage technology based on optical properties of crystals. The basic crystal model is built as a supercell structure 2 × 2 × 1 of near-stoichiometric pure LiNbO3 crystal with 120 atoms, including 24 Li atoms, 24 Nb atoms and 72 O atoms. Above that the five doped crystal models are established as follows: the copper doped LiNbO3 crystal (Cu:LiNbO3), the ferri doped LiNbO3 crystal (Fe:LiNbO3), the copper and ferri co-doped LiNbO3 crystal (Cu:Fe:LiNbO3), the copper, ferri and magnesium tri-doped LiNbO3 crystal (Cu:Fe:Mg:LiNbO3) with doping ions at Li sites, and the copper, ferri and magnesium tri-doped LiNbO3 crystal (Cu:Fe:Mg(E):LiNbO3) with ferri ions at Nb sites and magnesium ions at both Li sites and Nb sites. The last two models represent the concentration of Mg ions below the threshold (~6.0 mol%) and over the threshold respectively. The charge compensation forms are taken successively as
$\small {{\rm{Cu}}_{\rm{Li}}^+}\text-{\rm{V}}_{\rm{Li}}^-$ ,$\small {{\rm{Fe}}_{\rm{Li}}^{2+}}\text-{2\rm{V}}_{\rm{Li}}^-$ ,${{\rm{Fe}}_{\rm{Li}}^{2+}}\text-{\rm{Cu}}_{\rm{Li}}^+ \text-{3\rm{V}}_{\rm{Li}}^- $ ,${{\rm{Mg}}_{\rm{Li}}^{+} \text-{\rm{Fe}}_{\rm{Li}}^{2+}}\text- $ ${\rm{Cu}}_{\rm{Li}}^+\text -{4\rm{V}}_{\rm{Li}}^-$ and${{\rm{3Mg}}_{\rm{Li}}^{+}}\text-{\rm{Mg}}_{\rm{Nb}}^{3-}\text-{\rm{Fe}}_{\rm{Nb}}^{2-} \text-{2\rm{Cu}}_{\rm{Li}}^+$ in doped models. The results show that the extrinsic defect levels within the forbidden band of Cu:LiNbO3 crystal and Fe:LiNbO3 crystal are mainly contributed by the 3d orbits of Cu ions and the 3d orbits of Fe ions respectively. The forbidden band widths are 3.45 eV and 3.42 eV respetively in these two samples. In Cu:Fe:LiNbO3 crystal, the impurity levels are contributed by the 3d orbits of Cu and Fe ions; the forbidden band width is 3.24 eV; the absorption peaks are formed at 1.36, 2.53, and 3.01 eV. The Cu:Fe:Mg:LiNbO3 and Cu:Fe:Mg(E):LiNbO3 crystal presentthe forbidden band width of 2.89 eV and 3.30 eV respectively; the absorption peaks are formed at 2.45, 1.89 eV and 2.89, 2.59 eV, 2.24 eV, respectively. In Cu:Fe:Mg:LiNbO3 crystal, the weak absorption peak at 3.01 eV disappears, beacause of the superposition of the red-shifted absorption edge and the next bigger peak. The peak locations move slightly, which can be explained by the crystal field changing under the different doping concentrations and the different occupying positions of doping ions. In Cu:Fe:Mg(E):LiNbO3 crystal, the absorption peak near 2.5 eV is stronger than that of the other tri-doped crystal, which may be caused by the deference in occupancy among Fe ions. The peak at 2.9 eV can be chosen as erasing light, and the peak at 2.5 eV as write and read light in the two-center nonvolatile holography. The tri-doped crystal with Mg2+ concentration over the threshold shows obvious absorption peak at 2.9 eV and stronger absorption at 2.5 eV, which is beneficial for this application. The strong absorption of write light can shorten the time to reach the saturation of diffraction efficiency, then increase the dynamic range (M/#) and the sensitivity (S). Meanwhile, in this Mg doping condition, write time can be shortened, so optical damage can be weakened, and finally the image quality can be optimized.-
Keywords:
- tri-doped lithium niobate crystals /
- first-principles /
- electronic structure /
- absorption spectrum
[1] Adibi A, Buse K, Psaltis D 2001 J. Opt. Soc. Am. B: Opt. Phys. 18 584
[2] Sun X D, Luo S H, Wang J, Jiang Y Y, Shi H X 2009 J. Phys. D: Appl. Phys. 42 115413
Google Scholar
[3] Vonderlinde D, Glass A M, Rodgers K F 1974 Appl. Phys. Lett. 25 155
Google Scholar
[4] Buse K, Adibi A, Psaltis D 1998 Nature 393 665
Google Scholar
[5] Zhang X, Liang G J, Xu Z P 2019 Opt. Mater. 96 109318
Google Scholar
[6] Chen Y, Piao R Q, Zhang C Y, Zhang Z B, Xu J Q, Zhang D L 2018 Appl. Phys. B 124 206
[7] Xu C, Leng X S, Xu L, Wen A H, Xu Y H 2012 Opt. Commun. 285 3868
[8] Liu D, Liu L R, Zhou C H, Ren L Y, Li G G 2002 Appl. Opt. 41 6809
Google Scholar
[9] Chen S L, Liu H D, Kong Y F, Huang Z H, Xu J J 2006 Cryst. Res. Technol. 41 790
Google Scholar
[10] 王锐, 徐衍岭, 韦永德, 赵朝中 2001 光子学报 30 1307
Wang R, Xu Y L, Wei Y D, Zhao C Z 2001 Acta Photonica Sin. 30 1307
[11] Dai L, Liu C R, Tan C, Yan Z H, Xu Y H 2017 Chin. Phys. B 26 044207
Google Scholar
[12] Wang L P, Dai L, Liu C R, Han X B, Shao Y, Xu Y H 2019 Opt. Mater. 89 118
Google Scholar
[13] Abrahams S C, Hamilton W C, Reddy J M 1966 J. Phys. Chem. Solids 27 1013
Google Scholar
[14] 孔勇发, 许京军, 张光寅 2005 多功能光电材料-铌酸锂晶体 (北京: 科学出版社) 第42, 43页
Kong Y F, Xu J J, Zhang G Y 2005 Multi-function Photoelectric Materials LiNbO3 Crystal (Beijing: Sciences Press) pp42, 43 (in Chinese)
[15] Kuang M Q, Wu S Y, Zhang H M 2012 Optik 123 1601
Google Scholar
[16] Dai L, Han X B, Shao Y, Wang L P, Liu C R, Xu Y H 2018 Mod. Phys. Lett. B 32 1850328
[17] Zaldo C, Prieto C 1992 Ferroelectrics 134 47
Google Scholar
[18] Boker A, Donnerberg H, Schirmer O F, Feng X Q 1990 J. Phys. Condens. Matter. 2 6865
Google Scholar
[19] Vanderbilt D 1990 Phys. Rev. B 41 7892
Google Scholar
[20] Segall M D, Lindan PJ D, Probert M J, Pickard C J, Hasnip P J, Clark S J, Payne M C 2002 J. Phys. Condens. Matter. 14 2717
Google Scholar
[21] 赵佰强, 张耘, 邱晓燕, 王学维 2016 65 014212
Google Scholar
Zhao B Q, Zhang Y, Qiu X Y, Wang X W 2016 Acta Phys. Sin. 65 014212
Google Scholar
[22] Wang W W, Zheng D H, Hu M Y, Saeed S, Liu H D, Kong Y F, Zhang L X, Xu J J 2019 Materials 12 100
[23] Wang W, Wang R, Zhang W, Xing L L, Xu Y L, Wu X H 2013 Phys. Chem. Chem. Phys. 15 14347
Google Scholar
[24] Boysen H, Altorfer F 1994 Acta Cryst. B 50 405
Google Scholar
[25] 戴维, 杰尼斯著 (李建, 周勇译) 2014 密度泛函理论(北京: 国防工业出版社) 第220—224页
David S S, Janice A S (translated by Li J, Zhou Y) 2014 Density Functional Theory (Beijing: National Defense Industry Press) pp220–224 (in Chinese)
[26] Veithen M, Gonze X, Ghosez P 2004 Phys. Rev. Lett. 93 187401
Google Scholar
[27] Tsuboi T, Grinberg M, Kaczmarek S M 2002 J. Alloys Compd. 341 333
Google Scholar
[28] Kar S, Verma S, Bartwal K S 2008 Cryst. Growth Des. 8 4424
Google Scholar
[29] 潘道铠, 赵成大, 郑载兴1983 物质结构 (北京: 人民教育出版社) 第340—346页
Pan D K, Zhao C D, Zheng Z X 1983 Material Structure (Beijing: People Education Press) pp340–346 (in Chinese)
[30] 高攀, 柳清菊, 张学军 2010 59 4930
Google Scholar
Gao P, Liu Q J, Zhang X J 2010 Acta phys. Sin. 59 4930
Google Scholar
[31] Xu H X, Chernatynskiy A, Lee D, Sinnott S B, Gopalan V, Dierolf V, Phillpot S R 2010 Phys. Rev. B 82 184109
[32] Bae S I, Ichikawa J, Shimamura K, Onodera H, Fukuda T 1997 J. Cryst. Growth 180 94
Google Scholar
[33] Zhang T, Wang B, Ling F R, Fang S Q, Xu Y H 2004 Mater. Chem. Phys. 83 350
Google Scholar
[34] Pankratov V, Millers D, Grigorjeva L, Matkovskii A O, Potera P, Pracka I, Lukasiewicz T 2003 Opt. Mater. 22 257
Google Scholar
[35] Yang Y P, Psaltis D, Luennemann M, Berben D, Hartwig U, Buse K 2003 J. Opt. Soc. Am. B 20 1491
Google Scholar
[36] Dai L, Jiao S S, Xu C, Li D Y, Lin J Q, Xu Y H 2014 Mod. Phys. Lett. B 28 1450038
-
图 1 晶体结构模型 (a) LN; (b) LN1; (c) LN2; (d) LN3; (e) LN4; (f) LN5
Fig. 1. Structures of crystals: (a) LN; (b) LN1; (c) LN2; (d) LN3; (e) LN4; (f) LN5.
图 3 LN及不同掺杂LN晶体的能带结构图 (a) LN; (b) LN1; (c) LN2; (d) LN3; (e) LN4; (f) LN5
Fig. 3. Band structures of LN and different doped LN crystals: (a) LN; (b) LN1; (c) LN2; (d) LN3; (e) LN4; (f) LN5.
图 4 晶体禁带附近分态密度图 (a) LN; (b) LN1; (c) LN2; (d) LN3; (e) LN4; (f) LN5
Fig. 4. partial densities of states near the forbidden band: (a) LN; (b) LN1; (c) LN2; (d) LN3; (e) LN4; (f) LN5.
表 1 LN晶体内各原子坐标
Table 1. Coordinates of atoms within LN crystal.
Atom Oxidation state X/nm Y/nm Z/nm Li 1 0 0 0.2802 Nb 5 0 0 0 O –2 0.0477 0.3435 0.0633 
下载: 导出CSV
表 2 掺杂LN晶体样本
Table 2. Samples of doped LN crystal.
符号 LN1 LN2 LN3 LN4 LN5 晶体名称 Cu:LN Fe:LN Cu:Fe:LN Cu:Fe:Mg:LN Cu:Fe:Mg(E):LN 占位及电荷补偿 ${ {\rm{Cu} }_{\rm{Li} }^+}\text-{\rm{V} }_{\rm{Li} }^-$ ${ {\rm{Fe} }_{\rm{Li} }^{2+} }\text-{2\rm{V} }_{\rm{Li} }^-$ $ { {\rm{Fe} }_{\rm{Li} }^{2+} }\text-{\rm{Cu} }_{\rm{Li} }^+ \text-{3\rm{V} }_{\rm{Li} }^-$ $ { {\rm{Mg} }_{\rm{Li} }^{+}\text-{\rm{Fe} }_{\rm{Li} }^{2+} }\text-{\rm{Cu} }_{\rm{Li} }^+ \text-{4\rm{V} }_{\rm{Li} }^-$ $ { {\rm{3Mg} }_{\rm{Li} }^{+} }\text-{\rm{Mg} }_{\rm{Nb} }^{3-}\text-{\rm{Fe} }_{\rm{Nb} }^{2-} \text-{2\rm{Cu} }_{\rm{Li} }^+$
下载: 导出CSV
表 3 LN晶体常数的几何优化值与实验值
Table 3. Geometry optimization result and experiment values of LN crystal.
Lattice parameter a/ nm b/ nm c/ nm V/ nm3 Experimental value 1.02966 1.02966 1.38630 1.27284 Optimization result 1.04829 1.04829 1.41321 1.33821
下载: 导出CSV
-
[1] Adibi A, Buse K, Psaltis D 2001 J. Opt. Soc. Am. B: Opt. Phys. 18 584
[2] Sun X D, Luo S H, Wang J, Jiang Y Y, Shi H X 2009 J. Phys. D: Appl. Phys. 42 115413
Google Scholar
[3] Vonderlinde D, Glass A M, Rodgers K F 1974 Appl. Phys. Lett. 25 155
Google Scholar
[4] Buse K, Adibi A, Psaltis D 1998 Nature 393 665
Google Scholar
[5] Zhang X, Liang G J, Xu Z P 2019 Opt. Mater. 96 109318
Google Scholar
[6] Chen Y, Piao R Q, Zhang C Y, Zhang Z B, Xu J Q, Zhang D L 2018 Appl. Phys. B 124 206
[7] Xu C, Leng X S, Xu L, Wen A H, Xu Y H 2012 Opt. Commun. 285 3868
[8] Liu D, Liu L R, Zhou C H, Ren L Y, Li G G 2002 Appl. Opt. 41 6809
Google Scholar
[9] Chen S L, Liu H D, Kong Y F, Huang Z H, Xu J J 2006 Cryst. Res. Technol. 41 790
Google Scholar
[10] 王锐, 徐衍岭, 韦永德, 赵朝中 2001 光子学报 30 1307
Wang R, Xu Y L, Wei Y D, Zhao C Z 2001 Acta Photonica Sin. 30 1307
[11] Dai L, Liu C R, Tan C, Yan Z H, Xu Y H 2017 Chin. Phys. B 26 044207
Google Scholar
[12] Wang L P, Dai L, Liu C R, Han X B, Shao Y, Xu Y H 2019 Opt. Mater. 89 118
Google Scholar
[13] Abrahams S C, Hamilton W C, Reddy J M 1966 J. Phys. Chem. Solids 27 1013
Google Scholar
[14] 孔勇发, 许京军, 张光寅 2005 多功能光电材料-铌酸锂晶体 (北京: 科学出版社) 第42, 43页
Kong Y F, Xu J J, Zhang G Y 2005 Multi-function Photoelectric Materials LiNbO3 Crystal (Beijing: Sciences Press) pp42, 43 (in Chinese)
[15] Kuang M Q, Wu S Y, Zhang H M 2012 Optik 123 1601
Google Scholar
[16] Dai L, Han X B, Shao Y, Wang L P, Liu C R, Xu Y H 2018 Mod. Phys. Lett. B 32 1850328
[17] Zaldo C, Prieto C 1992 Ferroelectrics 134 47
Google Scholar
[18] Boker A, Donnerberg H, Schirmer O F, Feng X Q 1990 J. Phys. Condens. Matter. 2 6865
Google Scholar
[19] Vanderbilt D 1990 Phys. Rev. B 41 7892
Google Scholar
[20] Segall M D, Lindan PJ D, Probert M J, Pickard C J, Hasnip P J, Clark S J, Payne M C 2002 J. Phys. Condens. Matter. 14 2717
Google Scholar
[21] 赵佰强, 张耘, 邱晓燕, 王学维 2016 65 014212
Google Scholar
Zhao B Q, Zhang Y, Qiu X Y, Wang X W 2016 Acta Phys. Sin. 65 014212
Google Scholar
[22] Wang W W, Zheng D H, Hu M Y, Saeed S, Liu H D, Kong Y F, Zhang L X, Xu J J 2019 Materials 12 100
[23] Wang W, Wang R, Zhang W, Xing L L, Xu Y L, Wu X H 2013 Phys. Chem. Chem. Phys. 15 14347
Google Scholar
[24] Boysen H, Altorfer F 1994 Acta Cryst. B 50 405
Google Scholar
[25] 戴维, 杰尼斯著 (李建, 周勇译) 2014 密度泛函理论(北京: 国防工业出版社) 第220—224页
David S S, Janice A S (translated by Li J, Zhou Y) 2014 Density Functional Theory (Beijing: National Defense Industry Press) pp220–224 (in Chinese)
[26] Veithen M, Gonze X, Ghosez P 2004 Phys. Rev. Lett. 93 187401
Google Scholar
[27] Tsuboi T, Grinberg M, Kaczmarek S M 2002 J. Alloys Compd. 341 333
Google Scholar
[28] Kar S, Verma S, Bartwal K S 2008 Cryst. Growth Des. 8 4424
Google Scholar
[29] 潘道铠, 赵成大, 郑载兴1983 物质结构 (北京: 人民教育出版社) 第340—346页
Pan D K, Zhao C D, Zheng Z X 1983 Material Structure (Beijing: People Education Press) pp340–346 (in Chinese)
[30] 高攀, 柳清菊, 张学军 2010 59 4930
Google Scholar
Gao P, Liu Q J, Zhang X J 2010 Acta phys. Sin. 59 4930
Google Scholar
[31] Xu H X, Chernatynskiy A, Lee D, Sinnott S B, Gopalan V, Dierolf V, Phillpot S R 2010 Phys. Rev. B 82 184109
[32] Bae S I, Ichikawa J, Shimamura K, Onodera H, Fukuda T 1997 J. Cryst. Growth 180 94
Google Scholar
[33] Zhang T, Wang B, Ling F R, Fang S Q, Xu Y H 2004 Mater. Chem. Phys. 83 350
Google Scholar
[34] Pankratov V, Millers D, Grigorjeva L, Matkovskii A O, Potera P, Pracka I, Lukasiewicz T 2003 Opt. Mater. 22 257
Google Scholar
[35] Yang Y P, Psaltis D, Luennemann M, Berben D, Hartwig U, Buse K 2003 J. Opt. Soc. Am. B 20 1491
Google Scholar
[36] Dai L, Jiao S S, Xu C, Li D Y, Lin J Q, Xu Y H 2014 Mod. Phys. Lett. B 28 1450038
下载:
计量
- 文章访问数: 6752
- PDF下载量: 101
- 被引次数: 0
