-
自由空间量子通信会受到雾霾、沙尘、降雨等自然环境的干扰. 为提升环境干扰下量子通信的性能, 本文提出了基于软件定义量子通信 (software defined quantum communication, SDQC)的自由空间量子通信信道参数自适应调整策略. 该策略通过对环境状态实时监测, 根据预置在应用层的程序, 对量子初始状态及单量子态存在时间等相关参数进行自适应调整, 提高自然环境背景干扰下自由空间量子通信系统的保真度. 仿真结果表明, 在退极化、自发幅度衰变及相位阻尼三种噪声信道参数取值不同时, SDQC系统参数的最佳取值也不同. 系统根据环境变化及业务需求, 自适应地选择量子初始状态及单量子态存在时间, 使量子保真度在通信过程中始终保持在峰值, 有效提升了量子通信系统的适应能力及综合免疫力.Quantum communication in free space will be disturbed by natural environment, such as fog, dust, and rain, which is a difficult problem in the construction of quantum communication system. In order to solve this problem and improve the survivability of quantum communication system, we propose an adaptive parameter adjustment strategy for free-space quantum communication based on software-defined quantum communication (SDQC). Firstly, we propose a software-defined quantum communication model based on the idea of software defined networks. The architecture of SDQC is divided into four layers: transport layer, access layer, control layer, and management layer. The SDQC system sends the link information to the preset program at a management level through the real-time monitoring of channel state by the access layer. According to the link information, the management level issues instructions to the control layer to adjust the parameters such as the initial quantum state and the existence time of single quantum state, in order to improve the quantum entanglement and fidelity. Secondly, we analyze the relationship between quantum fidelity and parameters in SDQC system under three noise channels, i.e. depolarization channel, spontaneous amplitude decay channel, and phase damping channel. In the depolarized channel, the quantum fidelity F decreases with the increase of the error probability Pd of the qubit. When the error probability of qubit is certain, the system has the maximum quantum fidelity with the value of parameter x is 0.5. In the spontaneous amplitude decay channel, the quantum fidelity F decreases with the increase of the quantum state transition probability pt. When the transition probability of quantum state is certain, the higher the value of parameter x, the higher the fidelity will be. In the phase-damped channel, the quantum fidelity F decreases with the increase of the probability pc with which the qubit and the background interference equivalent quantum state have complete elastic scattering. When the probability is certain, the larger the value of |1/2 – x|, the higher the quantum fidelity of the system will be. Finally, we study the optimal values of SDQC system parameters under different environmental disturbances. The simulation results show that the optimal parameters of SDQC system are different when the parameters of three noise channels, namely depolarization, spontaneous amplitude decay and phase damping, are different. The system adaptively selects the initial quantum state and the existence time of single quantum state according to the environmental change and business demand, so that the quantum fidelity is always at the peak in the communication process. This strategy effectively improves the adaptability and comprehensive immunity of the quantum communication system.
-
Keywords:
- free space quantum communication /
- software defined quantum communication /
- quantum state /
- fidelity
[1] Jin X M, Ren J G, Yang B 2010 Nat. Photon. 4 376
Google Scholar
[2] Ma X S, Thomas H, Thomas S, Wang D Q, Sebastian K, William N, Bernhard W, Alexandra M, Johannes K, Elena A, Vadim M, Thomas J, Rupert U, Anton Z 2012 Nature 489 269
Google Scholar
[3] Davide E B, Timothy C, Ralph, Ivette F, Thomas J, Mohsen R 2014 Phys. Rev. D 90 045041
Google Scholar
[4] 聂敏, 任杰, 杨光, 张美玲, 裴昌幸 2015 64 150301
Google Scholar
Nie M, Ren J, Yang G, Zhang M L, Pei C X 2015 Acta Phys. Sin. 64 150301
Google Scholar
[5] 聂敏, 尚鹏钢, 杨光, 张美玲, 裴昌幸 2014 63 240303
Google Scholar
Nie M, Shang P G, Zhang M L, Pei C X 2014 Acta Phys. Sin. 63 240303
Google Scholar
[6] 聂敏, 石力, 杨光, 裴昌幸 2017 通信学报 38 2017092
Nie M, Shi L, Yang G, Pei C X 2017 J. Commun. 38 2017092
[7] Ivan C, Andrea T, Alberto D, Francesca G, Ruper U, Giuseppe V, Paolo V 2012 Phys. Rev. Lett. 109 200502
Google Scholar
[8] 聂敏, 任家明, 杨光, 张美玲, 裴昌幸 2016 光子学报 45 0927004
Nie M, Ren J M, Yang G, Zhang M L, Pei C X 2016 Acta Photon. Sin. 45 0927004
[9] 聂敏, 唐守荣, 杨光, 张美玲, 裴昌幸 2017 66 070302
Google Scholar
Nie M, Tang S R, Yang G, Zhang M L, Pei C X 2017 Acta Phys. Sin. 66 070302
Google Scholar
[10] 聂敏, 常乐, 杨光, 张美玲, 裴昌幸 2017 光子学报 46 0701002
Nie M, Chang L, Yang G, Zhang M L, Pei C X 2017 Acta Photon. Sin. 46 0701002
[11] 聂敏, 唐守荣, 杨光, 张美玲, 裴昌幸 2017 光子学报 46 1206002
Nie M, Tang S R, Yang G, Zhang M L, Pei C X 2017 Acta Photon. Sin. 46 1206002
[12] 聂敏, 任家明, 杨光, 张美玲, 裴昌幸 2016 65 190301
Google Scholar
Nie M, Ren J M, Yang G, Zhang M L, Pei C X 2016 Acta Phys. Sin. 65 190301
Google Scholar
[13] 张朝昆, 崔勇, 唐翯祎, 吴建平 2015 软件学报 26 62
Google Scholar
Zhang C K, Cui Y, Tang H Y, Wu J P 2015 J. Software 26 62
Google Scholar
[14] Mijumbi R, Serrat J, Gorricho J, Bouten N, de Truck F, Boutaba R 2016 IEEE Commun. Surv. Tut. 18 239
Google Scholar
[15] Suresh L, Schulz J, Merz R, Feldmann A 2012 Comput. Commun. Rev. 42 279
Google Scholar
[16] Kannan K, Banerjee S 2012 Proceedings of the 8th International Conference on Network and Service Management Las Vegas, USA, October 22–26, 2012 p295
[17] Jin D, Nicol D M 2013 Proceedings of the 2013 ACM SIGSIM Conference On Principles of Advanced Discrete Simulation Montreal, Canada, May 19–22, 2013 p91
[18] 尹浩, 马怀新 2006 军使量子通信概论 (北京: 军事科学出版社) 第224页
Yin H, Ma H X 2006 Introduction to Quantum Communication in Military (Beijing: Military Science Press) p224 (in Chinese)
[19] 张登玉 2013 量子逻辑门与量子退相干 (北京: 科学出版社) 第90—110页
Zhang D Y 2013 Quantum Logic Gates and Quantum Decoherence (Beijing: Science Press) pp90–110 (in Chinese)
[20] Liao X P, Fang M F, Fang J S, Zhu Q Q 2014 Chin. Phys. B 23 020304
Google Scholar
[21] 尹浩, 马怀新 2006 军使量子通信概论 (北京: 军事科学出版社) 第227页
Yin H, Ma H X 2006 Introduction to Quantum Communication in Military (Beijing: Military Science Press) p227 (in Chinese)
[22] Bennett C H 1992 Phys. Rev. Lett. 68 3121
Google Scholar
[23] Gao F, Qin S J, Huang W, Wen Q Y 2019 Sci. China: Phys. Mech. Astron. 62 070301
Google Scholar
[24] Gao F, Liu B, Wen Q Y 2012 Opt. Express 20 17411
Google Scholar
-
图 2 SDQC在退极化信道下量子保真度与量子位出错概率
${p_{\rm{d}}}$ 及参数$x$ 的关系Fig. 2. Relationship between quantum fidelity, the probability of a qubit error
${p_{\rm{d}}}$ and parameter$x$ of SDQC in depo-larization channel.
图 3 自发幅度衰变信道下量子保真度与量子态跃迁概率
${p_{\rm{t}}}$ 及参数$x$ 的关系Fig. 3. The relationship between quantum fidelity, quantum transition probability
${p_{\rm{t}}}$ and parameter$x$ of SDQC in spontaneous amplitude decay channel.
图 4 SDQC在相位阻尼信道下量子保真度与量子位与背景环境干扰等效量子态发生完全弹性散射的概率
${p_{\rm{c}}}$ 及参数$x$ 的关系Fig. 4. Relationship between quantum fidelity, the probability of a qubit having complete elastic scattering with the background
${p_{\rm{c}}}$ and parameter$x$ of SDQC in phase-damped channel.
图 5 量子位出错概率
${p_{\rm{d}}}$ 随$x$ 取值变化对量子保真度$F$ 的影响Fig. 5. Influence of the change of qubit error probability
${p_{\rm{d}}}$ with the value of$x$ on the quantum fidelity.
图 7
${p_{\rm{c}}}$ 随$x$ 取值变化对量子保真度$F$ 的影响Fig. 7. Influence of the change of the probability of a qubit having complete elastic scattering with the background
${p_{\rm{c}}}$ with the value of$x$ on the quantum fidelity.
图 6 量子态跃迁概率
${p_{\rm{t}}}$ 随$x$ 取值变化对量子保真度$F$ 的影响Fig. 6. Influence of the change of quantum transition probability
${p_{\rm{t}}}$ with the value of$x$ on the quantum fidelity.
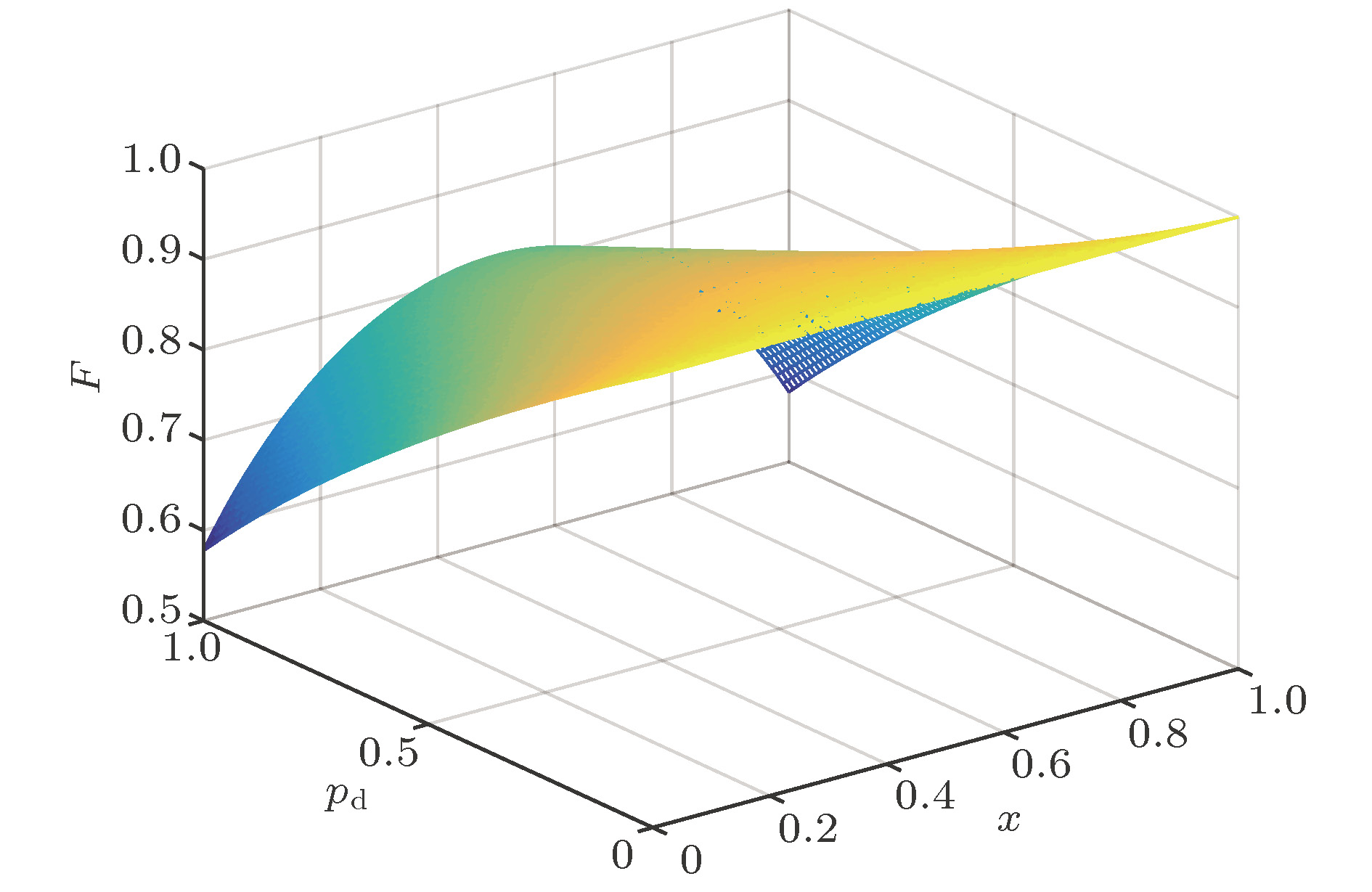
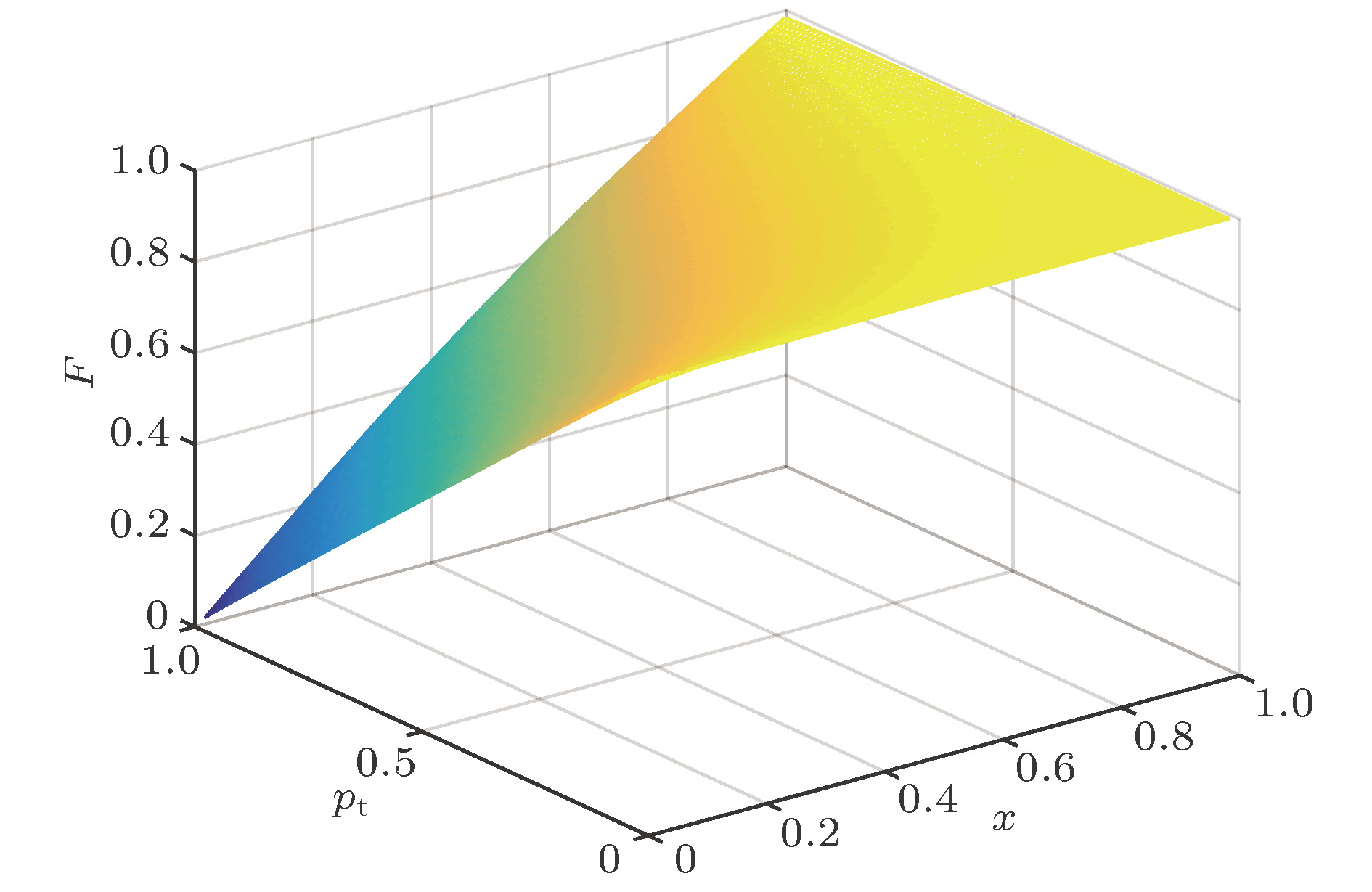
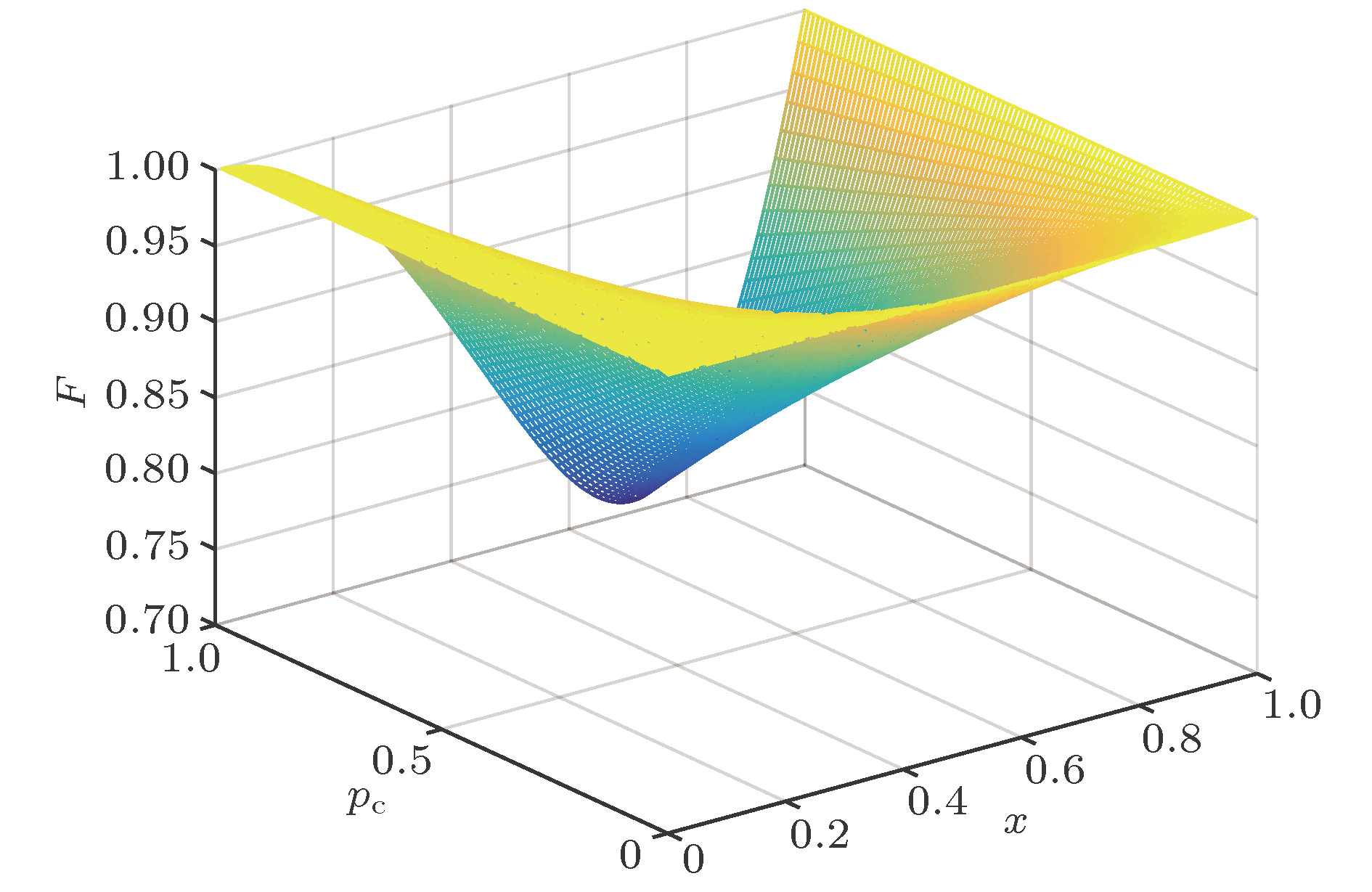
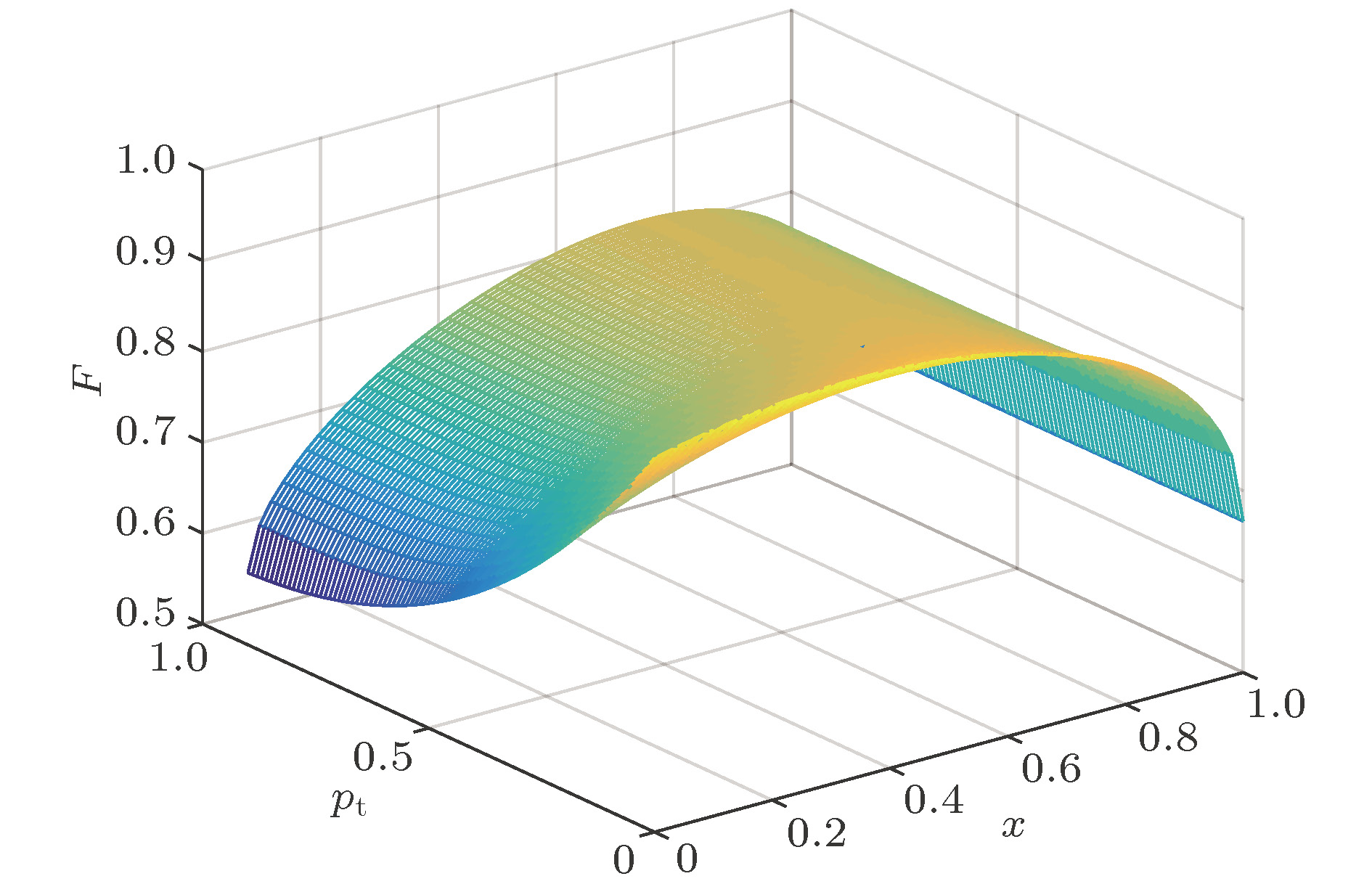
图 8 SDQC系统在不同环境干扰因素下保真度
$F$ 与参数$x$ 的取值关系随时间的演化 (a)${p_{\rm{d}}} = 0.1,\; {p_{\rm{t}}} = 0.1,\; {p_{\rm{c}}} = 0.1;$ (b)${p_{\rm{d}}} = 0.1, \;{p_{\rm{t}}} = 0.5, \;{p_{\rm{c}}} = 0.1$ ; (c)${p_{\rm{d}}} = 0.1,\; {p_{\rm{t}}} = 0.1,\; {p_{\rm{c}}} =0.5;$ (d)${p_{\rm{d}}} = 0.1,\; {p_{\rm{t}}} = 0.5,\; {p_{\rm{c}}} = 0.5$ Fig. 8. Analysis on the evolution of the relationship between fidelity
$F$ and$x$ of SDQC system under different environmental disturbance factors over time: (a)${p_{\rm{d}}} = 0.1,\; {p_{\rm{t}}} = 0.1,$ $ {p_{\rm{c}}} = 0.1;$ (b)${p_{\rm{d}}} = 0.1,\; {p_{\rm{t}}} = 0.5, \;{p_{\rm{c}}} = 0.1$ ; (c)${p_{\rm{d}}} = 0.1,\; {p_{\rm{t}}} = 0.1,$ $0.1, {p_{\rm{c}}} = 0.5$ ; (d)${p_{\rm{d}}} = 0.1, {p_{\rm{t}}} = 0.5, {p_{\rm{c}}} = 0.5$ 
图 9 保真度大于0.85时参数
$x$ 及$n$ 的集合Fig. 9. The set of parameters
$x$ and$n$ when the fidelity is greater than 0.85.表 1 不同环境干扰因素下SDQC系统参数
$x$ 的最优取值表Table 1. The optimal value of parameter
$x$ in SDQC system under different environmental disturbance factors.${p_{\rm{d}}}$ ${p_{\rm{t}}}$ ${p_{\rm{c}}}$ $n/\Delta t$ $x$ $n/\Delta t$ $x$ $n/\Delta t$ $x$ 0.1 0.1 0.1 1 0.29 5 0.49 10 0.66 0.1 0.5 0.1 1 0.43 5 0.66 10 0.74 0.1 0.1 0.5 1 0.42 5 0.73 10 0.78 0.1 0.5 0.5 1 0.56 5 0.80 10 0.81 
下载: 导出CSV
-
[1] Jin X M, Ren J G, Yang B 2010 Nat. Photon. 4 376
Google Scholar
[2] Ma X S, Thomas H, Thomas S, Wang D Q, Sebastian K, William N, Bernhard W, Alexandra M, Johannes K, Elena A, Vadim M, Thomas J, Rupert U, Anton Z 2012 Nature 489 269
Google Scholar
[3] Davide E B, Timothy C, Ralph, Ivette F, Thomas J, Mohsen R 2014 Phys. Rev. D 90 045041
Google Scholar
[4] 聂敏, 任杰, 杨光, 张美玲, 裴昌幸 2015 64 150301
Google Scholar
Nie M, Ren J, Yang G, Zhang M L, Pei C X 2015 Acta Phys. Sin. 64 150301
Google Scholar
[5] 聂敏, 尚鹏钢, 杨光, 张美玲, 裴昌幸 2014 63 240303
Google Scholar
Nie M, Shang P G, Zhang M L, Pei C X 2014 Acta Phys. Sin. 63 240303
Google Scholar
[6] 聂敏, 石力, 杨光, 裴昌幸 2017 通信学报 38 2017092
Nie M, Shi L, Yang G, Pei C X 2017 J. Commun. 38 2017092
[7] Ivan C, Andrea T, Alberto D, Francesca G, Ruper U, Giuseppe V, Paolo V 2012 Phys. Rev. Lett. 109 200502
Google Scholar
[8] 聂敏, 任家明, 杨光, 张美玲, 裴昌幸 2016 光子学报 45 0927004
Nie M, Ren J M, Yang G, Zhang M L, Pei C X 2016 Acta Photon. Sin. 45 0927004
[9] 聂敏, 唐守荣, 杨光, 张美玲, 裴昌幸 2017 66 070302
Google Scholar
Nie M, Tang S R, Yang G, Zhang M L, Pei C X 2017 Acta Phys. Sin. 66 070302
Google Scholar
[10] 聂敏, 常乐, 杨光, 张美玲, 裴昌幸 2017 光子学报 46 0701002
Nie M, Chang L, Yang G, Zhang M L, Pei C X 2017 Acta Photon. Sin. 46 0701002
[11] 聂敏, 唐守荣, 杨光, 张美玲, 裴昌幸 2017 光子学报 46 1206002
Nie M, Tang S R, Yang G, Zhang M L, Pei C X 2017 Acta Photon. Sin. 46 1206002
[12] 聂敏, 任家明, 杨光, 张美玲, 裴昌幸 2016 65 190301
Google Scholar
Nie M, Ren J M, Yang G, Zhang M L, Pei C X 2016 Acta Phys. Sin. 65 190301
Google Scholar
[13] 张朝昆, 崔勇, 唐翯祎, 吴建平 2015 软件学报 26 62
Google Scholar
Zhang C K, Cui Y, Tang H Y, Wu J P 2015 J. Software 26 62
Google Scholar
[14] Mijumbi R, Serrat J, Gorricho J, Bouten N, de Truck F, Boutaba R 2016 IEEE Commun. Surv. Tut. 18 239
Google Scholar
[15] Suresh L, Schulz J, Merz R, Feldmann A 2012 Comput. Commun. Rev. 42 279
Google Scholar
[16] Kannan K, Banerjee S 2012 Proceedings of the 8th International Conference on Network and Service Management Las Vegas, USA, October 22–26, 2012 p295
[17] Jin D, Nicol D M 2013 Proceedings of the 2013 ACM SIGSIM Conference On Principles of Advanced Discrete Simulation Montreal, Canada, May 19–22, 2013 p91
[18] 尹浩, 马怀新 2006 军使量子通信概论 (北京: 军事科学出版社) 第224页
Yin H, Ma H X 2006 Introduction to Quantum Communication in Military (Beijing: Military Science Press) p224 (in Chinese)
[19] 张登玉 2013 量子逻辑门与量子退相干 (北京: 科学出版社) 第90—110页
Zhang D Y 2013 Quantum Logic Gates and Quantum Decoherence (Beijing: Science Press) pp90–110 (in Chinese)
[20] Liao X P, Fang M F, Fang J S, Zhu Q Q 2014 Chin. Phys. B 23 020304
Google Scholar
[21] 尹浩, 马怀新 2006 军使量子通信概论 (北京: 军事科学出版社) 第227页
Yin H, Ma H X 2006 Introduction to Quantum Communication in Military (Beijing: Military Science Press) p227 (in Chinese)
[22] Bennett C H 1992 Phys. Rev. Lett. 68 3121
Google Scholar
[23] Gao F, Qin S J, Huang W, Wen Q Y 2019 Sci. China: Phys. Mech. Astron. 62 070301
Google Scholar
[24] Gao F, Liu B, Wen Q Y 2012 Opt. Express 20 17411
Google Scholar
下载:
计量
- 文章访问数: 8080
- PDF下载量: 80
- 被引次数: 0
