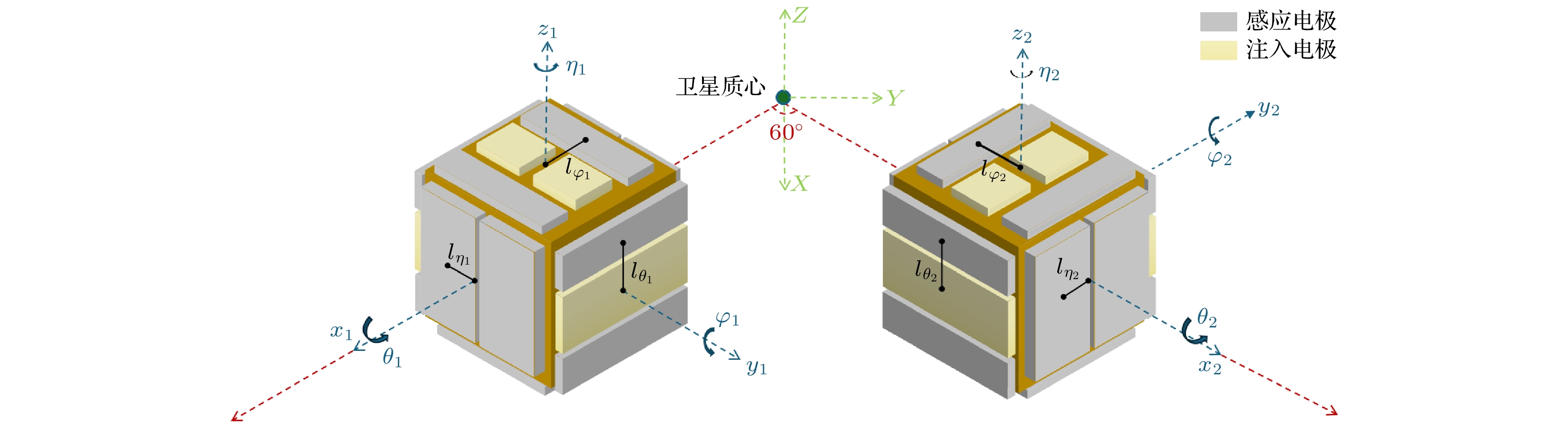
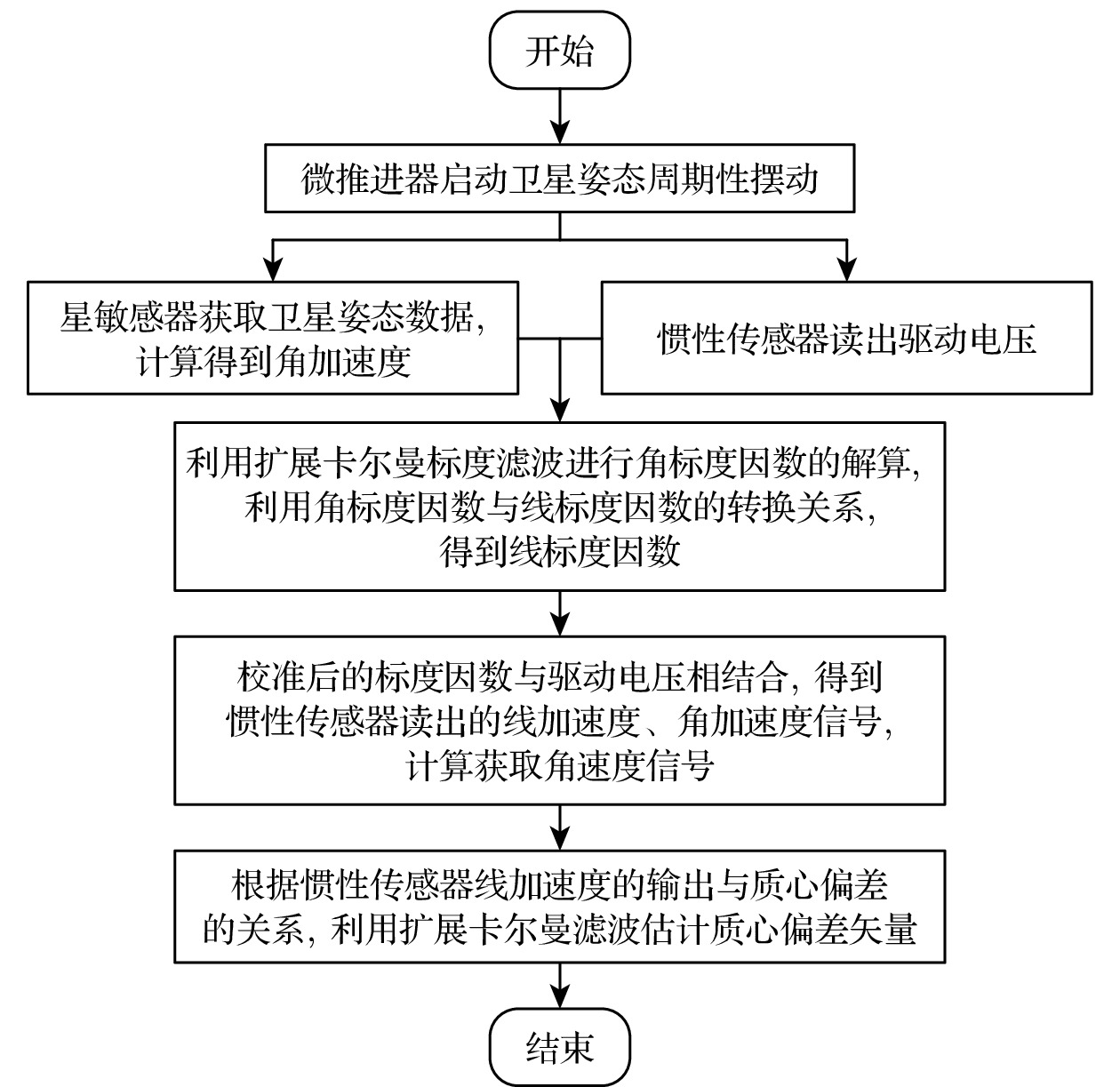
-
星载超高精度惯性传感器是空间引力波探测任务的核心载荷之一. 运行环境差异、卫星工质消耗和电子器件老化会导致惯性传感器主要在轨工作参数与地面定标结果不一致, 影响数据产品精度, 进而影响科学数据质量, 需开展惯性传感器工作参数在轨标定工作. 本文针对空间引力波探测太极计划残余加速度噪声优于3×10–15 m/(s2·Hz1/2)@3 mHz的超高精度指标要求, 结合太极计划惯性传感器设计布局以及实际噪声模型, 设计了惯性传感器标度因数和质心偏差矢量参数在轨定标方案, 并通过仿真实验验证了方案的可行性. 仿真结果表明, 标度因数的在轨定标误差小于300 × 10–6质心偏差在轨定标单轴误差$ < 75\;{\text{μm} }$, 满足太极计划惯性传感器工作参数在轨定标精度要求.
The Taiji program is a space mission designed to detect low-frequency gravitational waves. The mission's success hinges on the precise operation of its core payloads, particularly the inertial sensors, which are responsible for measuring the residual acceleration noise of the test masses. The duration of a space-based gravitational wave detection mission is 3 to 5 years. During this period, the shift in the satellite’s center of mass due to propellant consumption and other factors, as well as the drift in the scale factors caused by electronic component aging, will gradually degrade the accuracy of inertial sensor data. Therefore, it is necessary to regularly perform in-orbit calibration of inertial sensor parameters. In this work, we develop a calibration scheme, which actively applies controlled satellite oscillations and is tailored according to the installation layout of the inertial sensors in the Taiji program and the noise models. For the calibration of scale factors, high-precision star sensors are used to measure the satellite attitude signal, which is then combined with the driving voltage data from inertial sensors. By using the linear relationship between these signals, the scale factors are estimated using an extended Kalman Filter. For the calibration of center of mass (CoM) offsets, the calibrated scale factors are utilized, along with the driving voltage data from the front-end electronics of inertial sensors, to derive the test mass's angular acceleration, linear acceleration, and angular velocity. These parameters are then used to complete the CoM offset calibration according to the dynamic equation. The feasibility of the proposed calibration scheme is validated through a simulation experiment. The results demonstrate that the scale factors of the three axes can be calibrated to relative accuracies of 33 ppm, 27 ppm, and 173 ppm , respectively, meeting the requirement within 300 ppm. The CoM deviation are calibrated with accuracies of $ {\delta }_{{\boldsymbol{r}}_{1}}= $[15 μm, 31 μm, 34 μm], $ {\delta }_{{\boldsymbol{r}}_{2}}= $[5 μm, 15 μm, 13 μm], satisfying the 75 μm threshold. These results confirm that the proposed scheme can effectively maintain the inertial sensors’ performance within the required accuracy range. All in all, the calibration scheme developed in this study is crucial for maintaining the high performance of inertial sensors in the Taiji program. By achieving the precise calibration of the scale factors and deviation of center of mass within the required accuracy ranges, the scheme ensures the reliability of inertial sensor data, thereby significantly enhancing the sensitivity of space-based gravitational wave detection, which paves the way for groundbreaking discoveries in astrophysics and cosmology. -
Keywords:
- inertial sensor /
- in-orbit calibration /
- scale factor /
- center of mass offset
-
图 1 太极计划测试质量坐标系下的敏感结构
Fig. 1. Taiji inertial sensor sensitive structure in the local coordinate system.
图 2 标度因数与质心偏差的定标流程图
Fig. 2. Calibration flowchart for scale factor and mass center offset.
图 3 卫星机动效果 (a)参数定标期间推进器前500 s的力矩仿真信号; (b)参数定标期间卫星角度仿真信号
Fig. 3. Satellite maneuvering effect: (a) Propeller moment simulation signal for the first 500 s during parameter calibration; (b) satellite angle simulation signal during parameter calibration.
图 4 标度因数定标过程测量数据仿真信号 (a) 惯性传感器感应电极前500 s驱动电压; (b) 星敏感器获取角加速度前500 s仿真信号
Fig. 4. Scaling factor calibration process measurement data simulation signal: (a) Inertial sensor induction electrode drive voltage before 500 s; (b) star sensor to obtain angular acceleration simulation signal before 500 s.
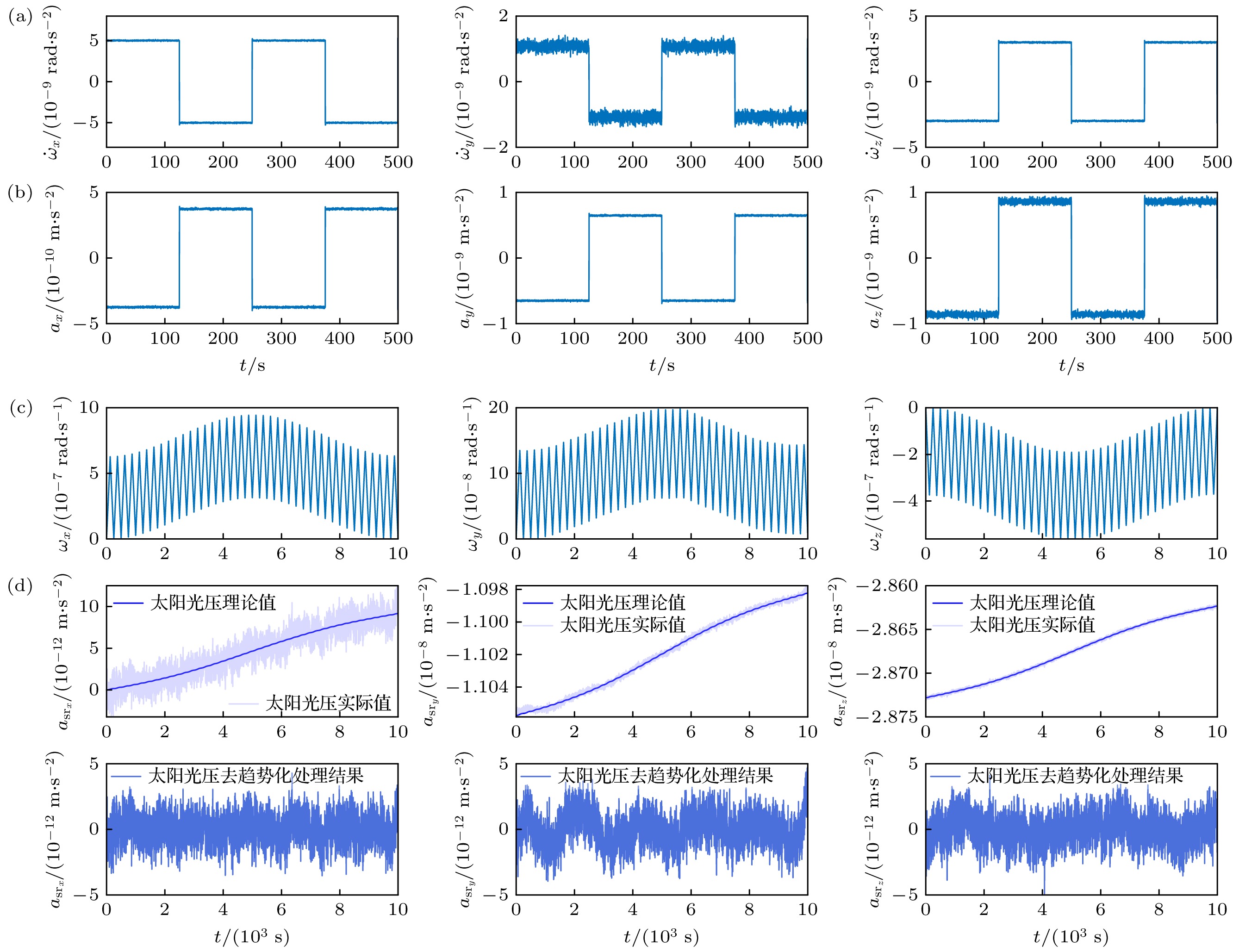
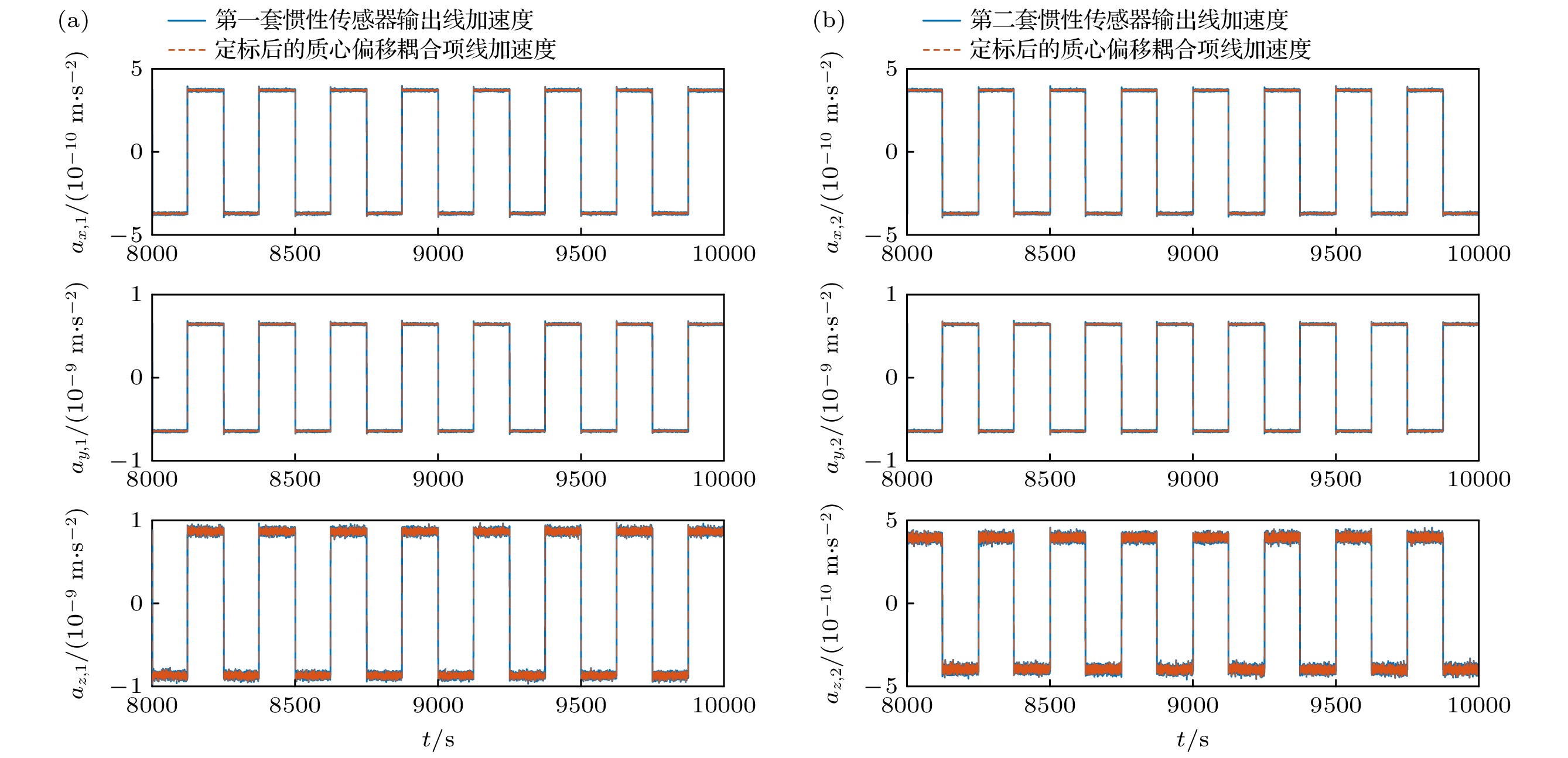
图 5 质心偏差定标期间惯性传感器测量数据模拟信号 (a) 角加速度前500 s仿真信号; (b) 线加速度前500 s仿真信号; (c) 角速度仿真信号; (d)太阳光压仿真信号及处理结果
Fig. 5. Simulation signal of measurement data during mass center offset calibration of inertial sensor: (a) Angular acceleration simulation signal before 500 s; (b) simulation signal of linear acceleration before 500 s; (c) angular velocity simulation signal; (d) simulation signal of sunlight pressure and processing results.
图 6 角标度因数定标估计收敛过程
Fig. 6. Angular scale factor calibration estimation convergence process.
图 7 质心偏差定标估计的收敛过程 (a) 质心偏差$ {\boldsymbol{r}}_{1} $ ; (b)质心偏差$ {\boldsymbol{r}}_{2} $
Fig. 7. Mass center offset calibration estimation convergence process: (a) Mass center offset $ {\boldsymbol{r}}_{1} $ ; (b) Mass center offset $ {\boldsymbol{r}}_{2} $ .
图 8 定标后质心偏差耦合加速度噪声识别效果图(8000—10000 s) (a)第一套惯性传感器系统; (b)第二套惯性传感器系统
Fig. 8. Mass center offset coupled acceleration noise recognition effect after calibration (8000–10000 s): (a) The first inertial sensor system; (b) the second inertial sensor system.
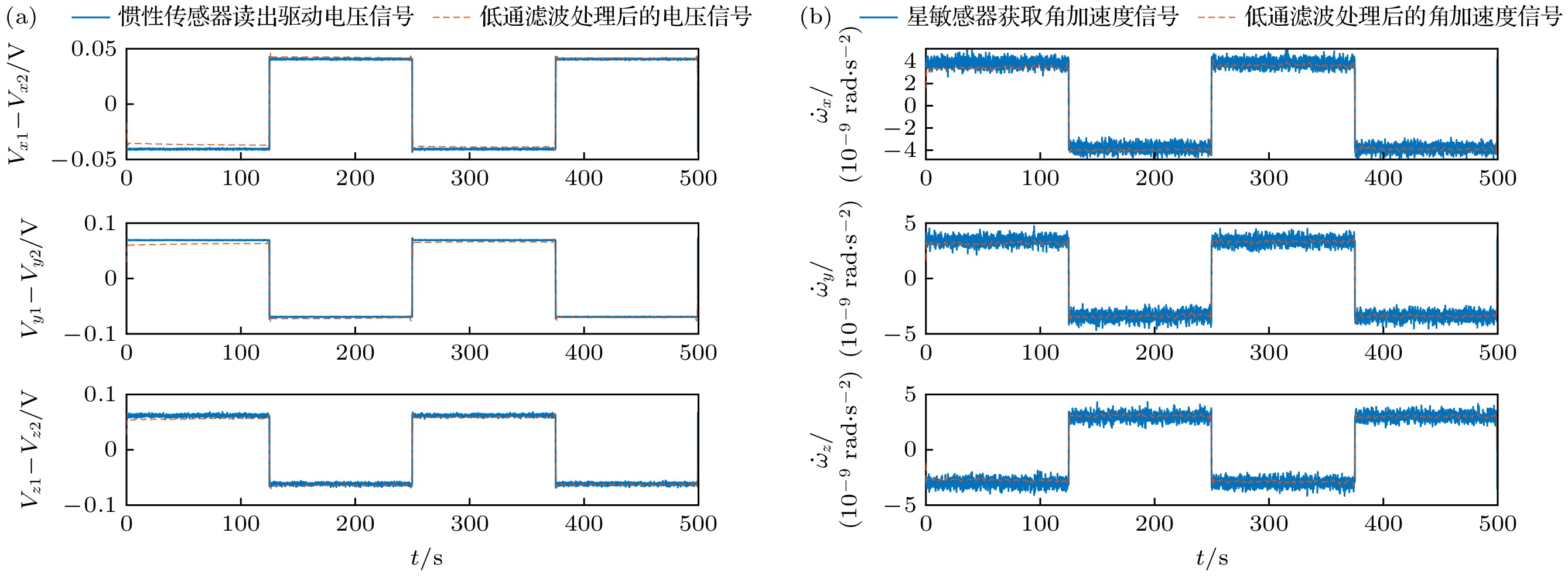
表 1 施加的加速度与驱动电压的对应关系
Table 1. Acceleration corresponds to the excitation voltage.
角加速度和线加速度 标度因数和电压读出 $ {\dot{\omega }}_{x} $ βx($ {V}_{y, 1}-{V}_{y, 2} $) $ {\dot{\omega }}_{y} $ $ {{\mathrm{\beta }}}_{{\mathrm{y}}} $($ {V}_{z, 1}-{V}_{z, 2} $) $ {\dot{\omega }}_{z} $ $ {{\mathrm{\beta }}}_{{\mathrm{z}}} $($ {V}_{x, 1}-{V}_{x, 2} $) $ {a}_{x} $ $ {{\mathrm{k}}}_{{\mathrm{x}}} $($ {V}_{x, 1}+{V}_{x, 2} $) $ {a}_{y} $ $ {{\mathrm{k}}}_{{\mathrm{y}}} $($ {V}_{y, 1}+{V}_{y, 2} $) $ {a}_{z} $ $ {{\mathrm{k}}}_{{\mathrm{z}}} $($ {V}_{z, 1}+{V}_{z, 2} $) 
下载: 导出CSV
表 2 标度因数真值与定标结果
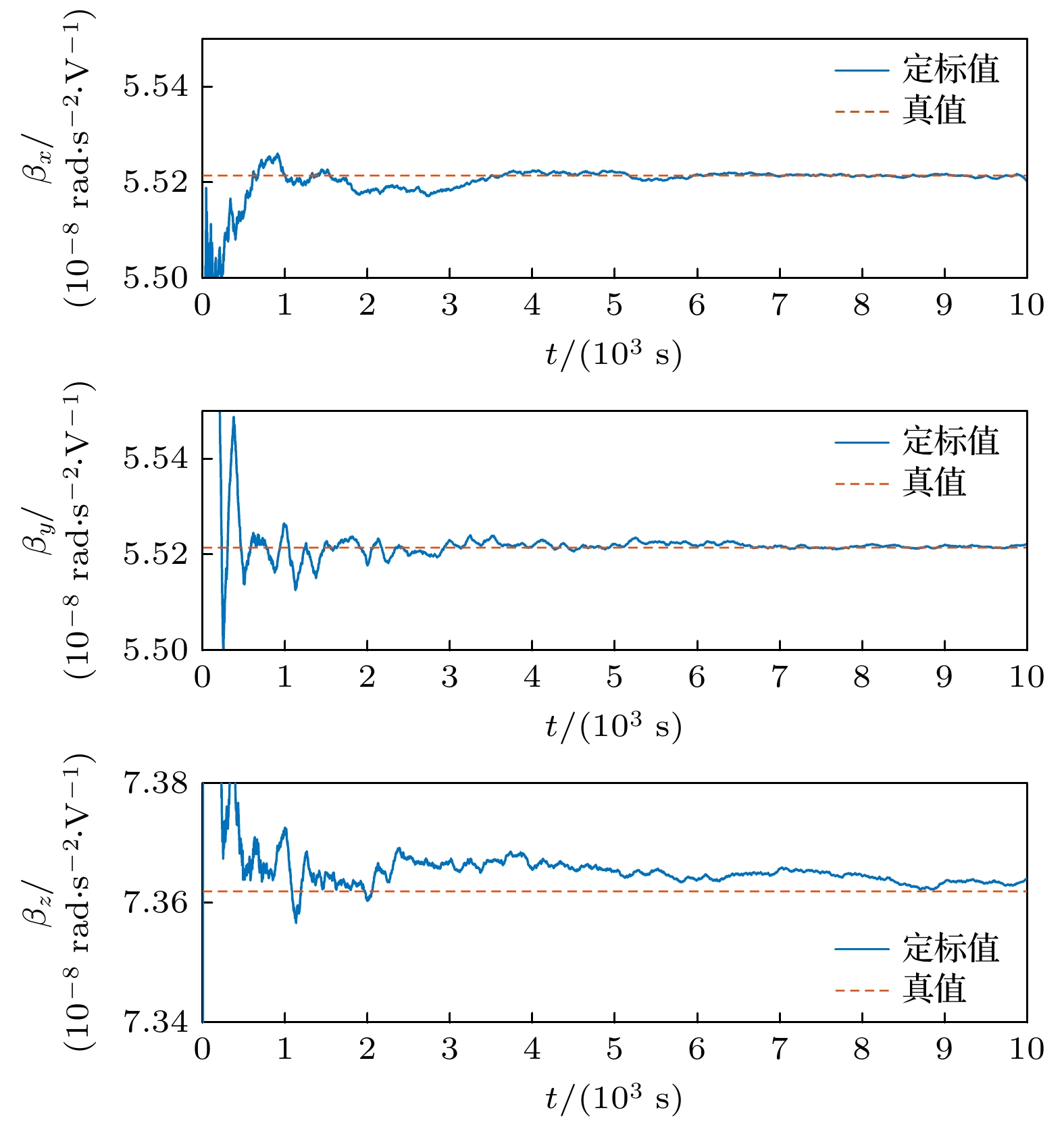
Table 2. Comparison of true scale factor values and calibration results.
标度因数 真值 定标值 βx/(10–8 rad·s–2·V–1) 5.52143 5.52125$ \pm $0.000029 Βy/(10–8 rad·s–2·V–1) 5.52143 5.52158$ \pm $0.000022 Βz/(10–8 rad·s–2·V–1) 7.36191 7.36318$ \pm $0.000022 kx/(10–9 m·s–2·V–1) 2.25765 2.25804$ \pm 0 $.000007 ky/(10–9 m·s–2·V–1) 1.12883 1.12879$ \pm 0 $.000006 kz/(10–9 m·s–2·V–1) 1.12883 1.12886$ \pm 0 $.000004
下载: 导出CSV
表 3 质心偏差真值与定标结果对比
Table 3. Comparison of true values and calibration results of mass center offset.
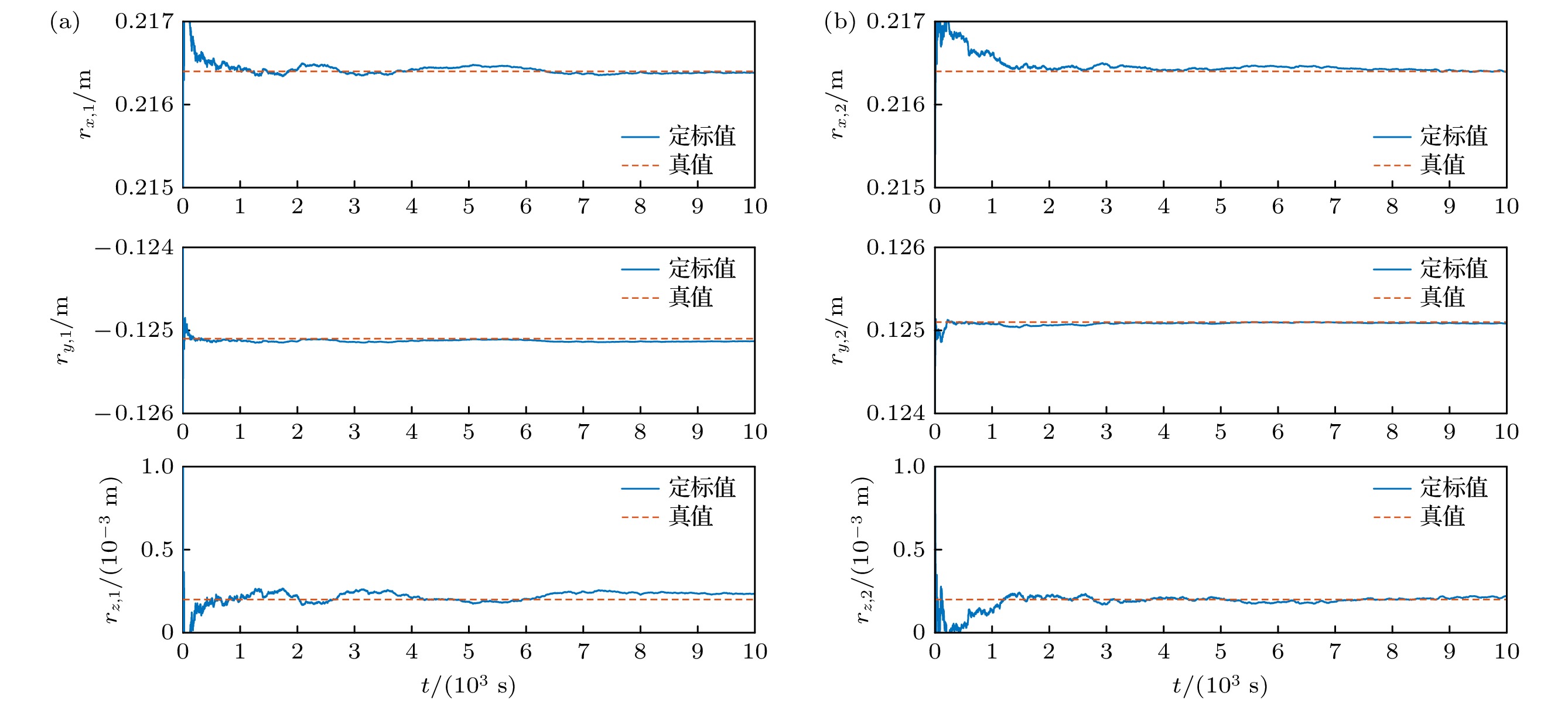
质心偏差 真值/m 定标值/m $ {\boldsymbol{r}}_{1} $ $ {r}_{x, 1} $ 0.216400 0.216385$ \pm $0.0000014 $ {r}_{y, 1} $ –0.125100 –0.125131$ \pm $0.0000005 $ {r}_{z, 1} $ 0.000200 0.000234$ \pm $0.0000009 $ {\boldsymbol{r}}_{2} $ $ {r}_{x, 2} $ 0.216400 0.216405$ \pm $0.0000067 $ {r}_{y, 2} $ 0.125100 0.125085$ \pm $0.0000022 $ {r}_{z, 2} $ 0.000200 0.000213$ \pm $0.0000046
下载: 导出CSV
-
[1] Marion F, LIGO Scientific Collaboration 2017 Nuovo Cimento C 39 310
[2] Pinard L, Michel C, Sassolas B, Balzarini L, Degallaix J, Dolique V, Flaminio R, Forest D, Granata M, Lagrange B, Straniero N, Teillon J, Cagnoli G 2017 Appl. Opt. 56 C11
Google Scholar
[3] Aso Y, Michimura Y, Somiya K, Ando M, Miyakawa O, Sekiguchi T, Tatsumi D, Yamamoto H 2013 Phys. Rev. D 88 173
[4] KAGRA Collaboration 2019 Nat. Astron. 3 35
Google Scholar
[5] Black E D, Gutenkunst R N 2003 Am. J. Phys. 71 365
Google Scholar
[6] Jani K, Shoemaker D, Cutler C 2019 Nat. Astron. 4 260
Google Scholar
[7] Jennrich O 2009 Class. Quantum Grav. 26 153001
Google Scholar
[8] Sun Q C, Wang G Q, Gong X F, Lau Y K, Xu S N, Amaro-Seoane P, Bai S, Bian X, Cao Z J, Chen G R, Chen X, Ding Y W, Dong P, Gao W, Heinzel G, Li M, Li S, Liu F K, Luo Z R, Shao M X, Spurzem R, Sun B S, Tang W L, Wang Y, Xu P, Yu P, Yuan Y F, Zhang X M, Zhou Z B 2014 J. Phys. : Conf. Ser. 610 12011
[9] Karsten D, the LISA study team 1996 Class. Quantum Grav. 3 A247
[10] Audley H, Babak S, Baker J, Barausse E, Sopuerta C F 2017 Tp. umu. se 548 411
[11] Sato S, Aso Y, Mino Y, Miyakawa O, Somiya K 2017 J. Phys. Conf. Ser. 840 012010
Google Scholar
[12] Kawamura S, Ando M, Seto N, et al. 2011 Class. Quantum Grav. 28 9
[13] Hu W R, Wu Y L 2017 Natl. Sci. Rev. 4 685
Google Scholar
[14] Wu Y L, Luo Z R, Wang J Y, Bai M, Zou Z M 2021 Commun. Phys. 4 34
Google Scholar
[15] Luo J, Chen L S, Duan H Z, Gong Y G, Hu S, Ji J, Liu Q, Mei J, Milyukov V, Sazhin M 2015 Class. Quantum Grav. 33 035010
[16] 罗子人, 张敏, 靳刚, 吴岳良, 胡文瑞 2020 深空探测学报 7 3
Luo Z R, Zhang M, Jin G, Wu Y L, Hu W R 2020 J. Deep Space Explor. 7 3
[17] Luo Z R, Zhang M, Wu Y L 2022 Chin. J. Space Sci. 42 536
Google Scholar
[18] Sun K X, Johann U, Debra1 D B, Byer R L 2007 J. Phys. Conf. Ser. 60 272
Google Scholar
[19] 王少鑫, 郭纬川, 赵平安, 王娟, 董鹏, 徐鹏, 罗子人, 齐克奇 2024 中国科学: 物理学 力学 天文学 54 91
Wang S X, Guo W C, Zhao P A, Wang J, Dong P, Xu P, Luo Z R, Qi K Q 2024 Sci. Sin. -Phy. s Mech. Astron. 54 91
[20] Wei X T, Huang L, Shen T Y, Cai Z M, He J B 2023 Phys. Rev. D 108 082001
Google Scholar
[21] Visser P N A M, Ijssel J V D 2003 Adv. Space Res. 31 1905
Google Scholar
[22] Lenoir B, Christophe B, Reynaud S 2012 Measurement 46 1411
[23] Visser P N A M 2008 J. Geod. 82 591
Google Scholar
[24] Wang F R 2003 Ph. D. Dissertation (Austin: The University of Texas at Austin
[25] Zhang H Y, Xu P, Ye Z, Ye D, Qiang L E, Luo Z R, Qi K Q, Wang S X, Cai Z M, Wang Z L, Lei J G, Wu Y L 2023 Remote Sens. 15 3817
Google Scholar
[26] 阮远东, 章志昊, 贾茳勰, 顾煜宁, 张善端, 崔旭高, 洪葳, 白彦峥, 田朋飞 2024 73 220401
Google Scholar
Ruan Y D, Zhang Z H, Jia J X, Gu Y N, Zhang S D, Cui X G, Hong W, Bai Y Z, Tian P F 2024 Acta Phys. Sin. 73 220401
Google Scholar
[27] Lupi F 2019 M. S. Thesis (Delft: Delft University of Technology
[28] Blarre L, Ouaknine J, Oddos-Marcel L, Brévannes L, Martinez P-E, Belin É 2006 AIAA Guidance, Navigation and Control Conference, Colorado, August 21–24, 2006 p6046
[29] 袁利, 王苗苗, 武延鹏, 王利, 郑然 2020 航空学报 4 17
Yuan L, Wang M M, Wu Y P, Wang L, Zheng R 2020 Chin. J. Aeronaut. 4 17
[30] Liu H, Niu X, Zeng M, Wang S, Cui K, Yu D R 2022 Acta Astronaut. 193 496510
[31] 于达仁, 牛翔, 王泰卜, 王尚胜, 曾明, 崔凯, 刘辉, 涂良成, 李祝, 黄祥青, 刘建平, 沈岩, 彭慧生, 杨铖, 宋培义, 匡双阳, 张开, 索晓晨, 黄潇博, 刘旭辉, 汪旭东, 龙军, 付新菊, 高晨光, 杨涓, 夏旭, 付瑜亮, 胡展, 康小明, 吴勤勤, 庞爱平, 周鸿博 2021 中山大学学报: 自然科学版 60 194
Yu D R, Niu X, Wang T B, Wang S S, Zeng M, Cui K, Liu H, Tu L C, Li Z, Huang X Q, Liu J P, Shen Y, Peng H S, Yang C, Song P Y, Kuang S Y, Zhang K, Suo X C, Huang X B, Liu X H, Wang X D, Long J, Fu X J, Gao C G, Yang Juan, Xia Xu, Fu Y L, Hu Z, Kang X M, Wu Q Q, Pang A P, Zhou H B 2021 Acta Sci. Nat. Univ. Sunyatseni. 60 194
[32] 张俊 2016 博士学位论文 (北京: 中国空间技术研究院, 北京控制工程研究所)
Zhang J 2016 Ph. D. Dissertation(Beijing: Beijing Institute of Control Engineering, Chinese Academy of Space Technology
[33] 门斯布吕克, 吉尔 (王家松, 祝开建, 胡小工译)2012卫星轨道: 模型、方法和应用 (北京: 国防工业出版社)第73—79页
Montenbruck O, Gill E (translated by Wang J S, Zhu K J, Hu X G) 2012 Satellite Orbits: Models, Methods and Applications (Beijing: National Defense Industry Press) pp73–79
下载:
计量
- 文章访问数: 333
- PDF下载量: 23
- 被引次数: 0
