-
双螺旋点扩散函数(double-helix point spread function, DH-PSF)显微可实现纳米尺度的三维单颗粒示踪(three-dimensional single particle tracking, 3D SPT), 被广泛应用于生命科学等领域, 但DH-PSF显微的成像景深和定位精度有限, 限制了其在活体厚样品中的应用. 为了解决此问题, 本文提出了一种基于轴向分光棱镜的多焦面DH-PSF 显微(z-splitter prism-based multifocus DH-PSF microscopy, ZPMDM)方法和系统, 通过将基于轴向分光棱镜的多焦面显微与DH-PSF相结合, 在无需扫描的情况下提高DH-PSF显微的轴向定位范围和定位精度, 解决完整活细胞内3D SPT的大景深探测难题. 通过系统标定, ZPMDM中3个通道的平均三维定位精度分别为σL(x, y, z) = (4.4 nm, 4.6 nm, 10.5 nm), σM(x, y, z) = (4.3 nm, 4.2 nm, 8.2 nm), 以及σR(x, y, z) = (4.8 nm, 4.4 nm, 10.3 nm), DH-PSF的有效景深扩展至6 μm, 实现了大景深范围内的甘油-水混合溶液中的荧光微球示踪, 并初步研究了活巨噬细胞的吞噬现象, 进一步验证了该方法的有效性, 对于3D SPT的发展和应用具有重要意义.Double-helix point spread function (DH-PSF) microscopy can realize three-dimensional single particle tracking (3D SPT) on a nanoscale, and is widely used in life sciences and other fields. However, its imaging depth-of-field (DOF) and localization accuracy are limited, which hinders its application in thick samples in vivo. To address this issue, this paper proposes a z-splitter prism-based multifocus DH-PSF microscopy (ZPMDM) method and system to improve the DOF and localization accuracy of DH-PSF microscopy without scanning. It solves the problem of large DOF detection of 3D SPT in whole living cells. By means of systematic calibration, the average 3D localization accuracies of three channels of ZPMDM are determined to be σL(x, y, z) = (4.4 nm, 4.6 nm, 10.5 nm), σM(x, y, z) = (4.3 nm, 4.2 nm, 8.2 nm), and σR(x, y, z) = (4.8 nm, 4.4 nm, 10.3 nm). And the effective DOF of the system is extended to 6 μm. Furthermore, the ZPMDM system is used to track fluorescent microspheres in a glycerol-water mixture across a large depth-of-field range. The Brownian motion of the fluorescent microspheres in the mixture solution is also investigated. The experimental results demonstrate that the errors between the experimentally obtained diffusion coefficients and the theoretically calculated diffusion coefficients are all within 10%. The reliability of the ZPMDM system in achieving single-particle 3D tracking imaging is verified in this study. The validity of the method is further verified by preliminarily investigating the phagocytosis phenomenon of live macrophages. It is of significance for the development and application of nanoscale 3D SPT. The ZPMDM system is shown in the attached figure.
[1] Dupont A, Lamb D C 2011 Nanoscale 3 4532
Google Scholar
[2] Kusumi A, Tsunoyama T A, Hirosawa K M, Kasai R S, Fujiwara T K 2014 Nat. Chem. Biol. 10 524
Google Scholar
[3] Gal N, Lechtman-Goldstein D, Weihs D 2013 Rheol. Acta 52 425
Google Scholar
[4] Cognet L, Leduc C, Lounis B 2014 Curr. Opin. Chem. Biol. 20 78
Google Scholar
[5] Kubitscheck U, Kuckmann O, Kues T, Peters R 2000 Biophys. J. 78 2170
Google Scholar
[6] Carter B C, Shubeita G T, Gross S P 2005 Phys. Biol. 2 60
Google Scholar
[7] Arhel N, Genovesio A, Kim K A, Miko S, Perret E, OlivoMarin J C, Shorte S, Charneau P 2006 Nat. Methods 3 817
Google Scholar
[8] Bacher C P, Reichenzeller M, Athale C, Herrmann H, Eils R 2004 BMC. Cell Biol. 5 45
Google Scholar
[9] Greengard A, Schechner Y, Piestun R 2006 Opt. Lett. 31 181
Google Scholar
[10] Pavani S R P, DeLuca J G, Piestun R. 2009 Opt. Express 17 19644
Google Scholar
[11] Thompson M A, Lew M D, Badieirostami M 2010 Nano Lett. 10 211
Google Scholar
[12] Thompson M A, Casolari J M, Badieirostami M 2010 PNAS 107 17864
Google Scholar
[13] Wang D P, Agrawal A, Piestun R 2017 Appl. Phys. Lett. 110 211107
Google Scholar
[14] Gahlmann A, Moerner W E 2014 Nat. Rev. Microbiol. 12 9
Google Scholar
[15] Wang D P, Wu H C, Schwartz D K 2017 Phys. Rev. Lett. 119 268001
Google Scholar
[16] Wu H C, Sarfati R, Wang D P 2020 J. Am. Chem. Soc. 142 4696
Google Scholar
[17] Lasker K, von Diezmann L, Zhou X 2020 Nat. Microbiol. 5 418
Google Scholar
[18] Rocha J M, Gahlmann A 2019 Jove-J. Vis. Exp. 151 59387
Google Scholar
[19] Li H, Chen D N, Xu G X, Yu B, Niu H B 2015 Opt. Express 23 787
Google Scholar
[20] Li H F, Wang F M, Wei T D, Miao X, Cheng Y, Pang X P, Jiang K M, Huang W, Zhang Y H 2021 Opt. Lett. 46 5088
Google Scholar
[21] Xiao S, Gritton H, Tseng H A, Zemel D, Han X, Mertz J 2020 Optica 7 1477
Google Scholar
[22] 霍英东, 曹博, 于斌, 陈丹妮, 牛憨笨 2015 64 028701
Google Scholar
Huo Y D, Cao B, Yu B, Chen D N, Niu H B 2015 Acta Phys. Sin. 64 028701
Google Scholar
[23] 林丹樱, 龚振权, 黄黎琳, 聂梦娇, 于斌, 屈军乐 2024 73 068701
Google Scholar
Lin D Y, Gong Z Q, Huang L, Nie M J, Yu B, Qu J L 2024 Acta Phys. Sin. 73 068701
Google Scholar
-
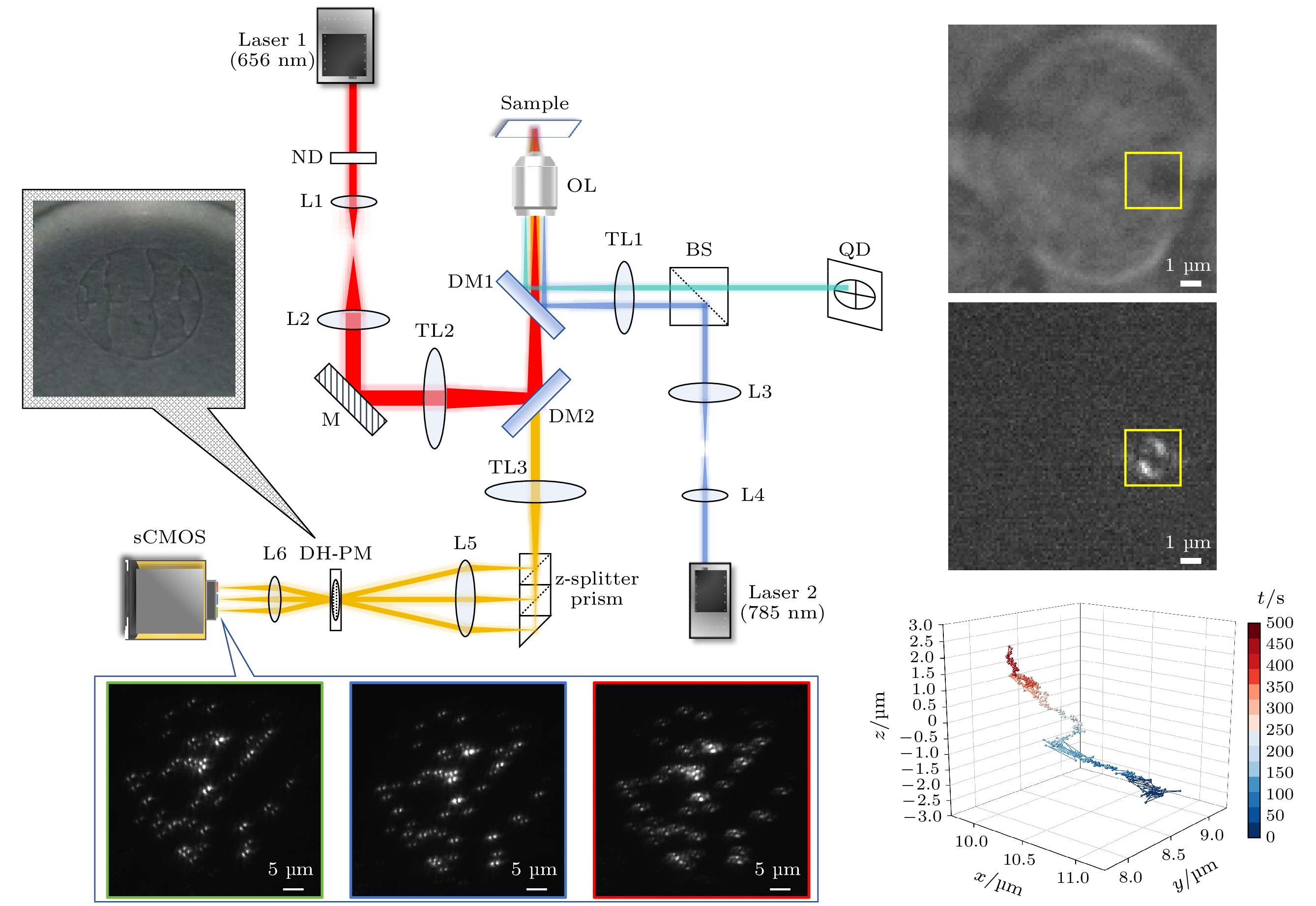
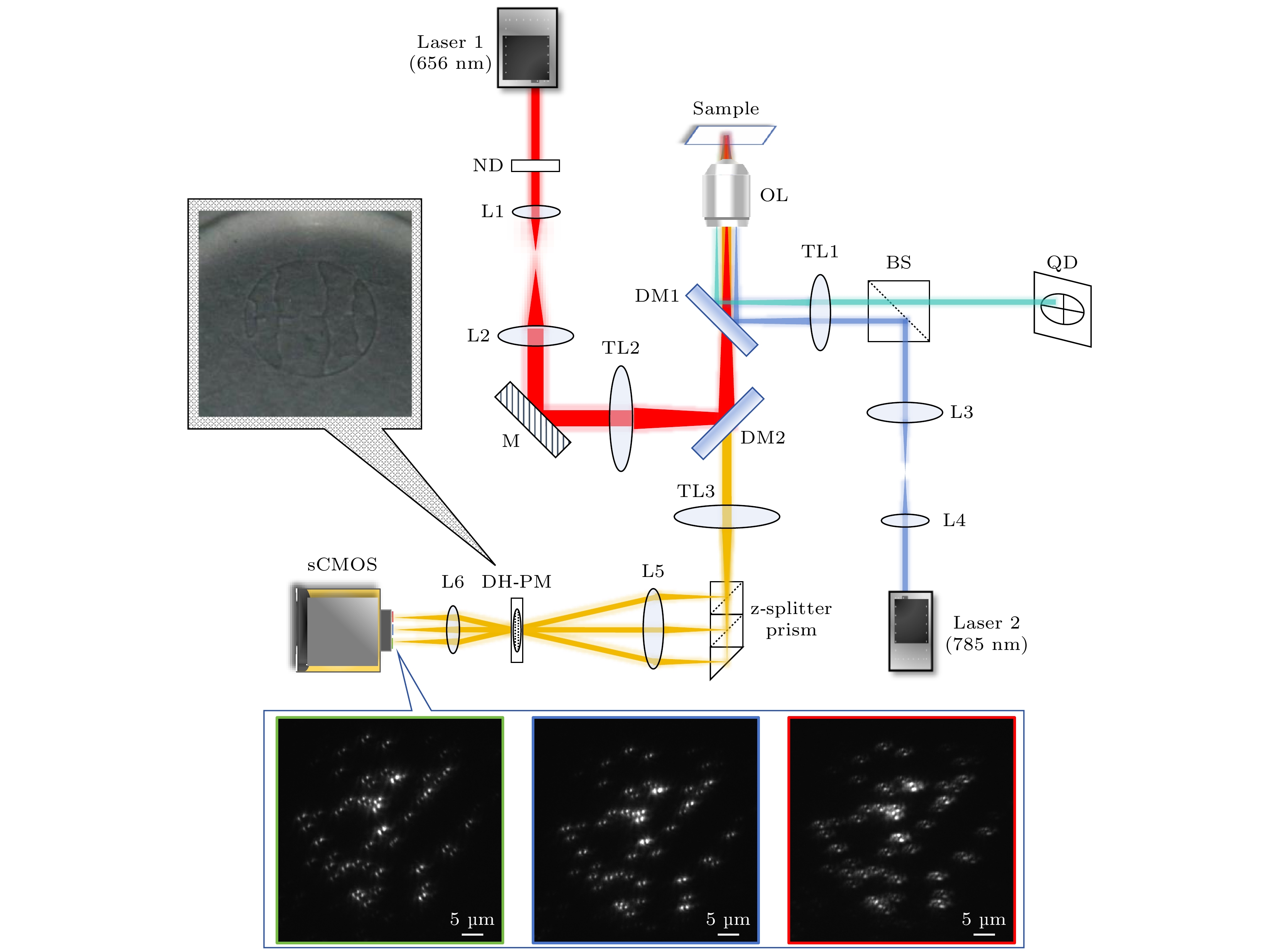
图 1 ZPMDM系统光路示意图. Laser1—Laser2, 激光器; ND, 中性密度衰减片; L1—L6, 透镜; M, 反射镜; TL1—TL3, 管镜; DM1, DM2, 二向色镜; OL, 物镜; BS, 分束棱镜; QD, 四象限探测器; DH-PM, 双螺旋相位模板; z-splitter prism, 轴向分光棱镜; sCMOS, 科研级互补金属氧化物半导体相机
Fig. 1. Schematic diagram of the ZPMDM system. Laser1–Laser2, lasers; ND, neutral density attenuator; L1–L6, lens; M, mirror; TL1–TL3, tube lens; DM1, DM2, dichroic mirrors; OL, objective lens; BS, beam splitter; QD, four-quadrant detector; DH-PM, double-helix phase mask; z-splitter prism, axial beam splitter prism; sCMOS, scientific complementary metal oxide semiconductor camera.
图 2 三通道荧光微球图像配准 (a) 左通道与中间通道荧光微球图像叠加的伪彩图; (b)配准后的左通道与中间通道荧光微球图像叠加的伪彩图; (c) 右通道与中间通道荧光微球图像叠加的伪彩图; (d)配准后的右通道与中间通道荧光微球图像叠加的伪彩图; 伪彩色: 紫色代表中间通道荧光微球图像, 绿色代表左(右)通道荧光微球图像, 两者准确重合时荧光微球图像为灰白色
Fig. 2. Three-channel fluorescent microsphere image registration: (a) Pseudo-color image superimposed with fluorescent microsphere images in the left channel and middle channels; (b) pseudo-color image superimposed with fluorescent microsphere images of the left and middle channels after registration; (c) pseudo-color image superimposed with fluorescent microsphere images in the right middle channels; (d) pseudo-color image superimposed with fluorescent microsphere images of the right channel and middle channels after registration; pseudo-color: purple represents fluorescent microsphere image in the middle channel, green represents fluorescent microsphere image in the left (right) channel, and the fluorescent microsphere image is greyish-white when the two accurately overlap.
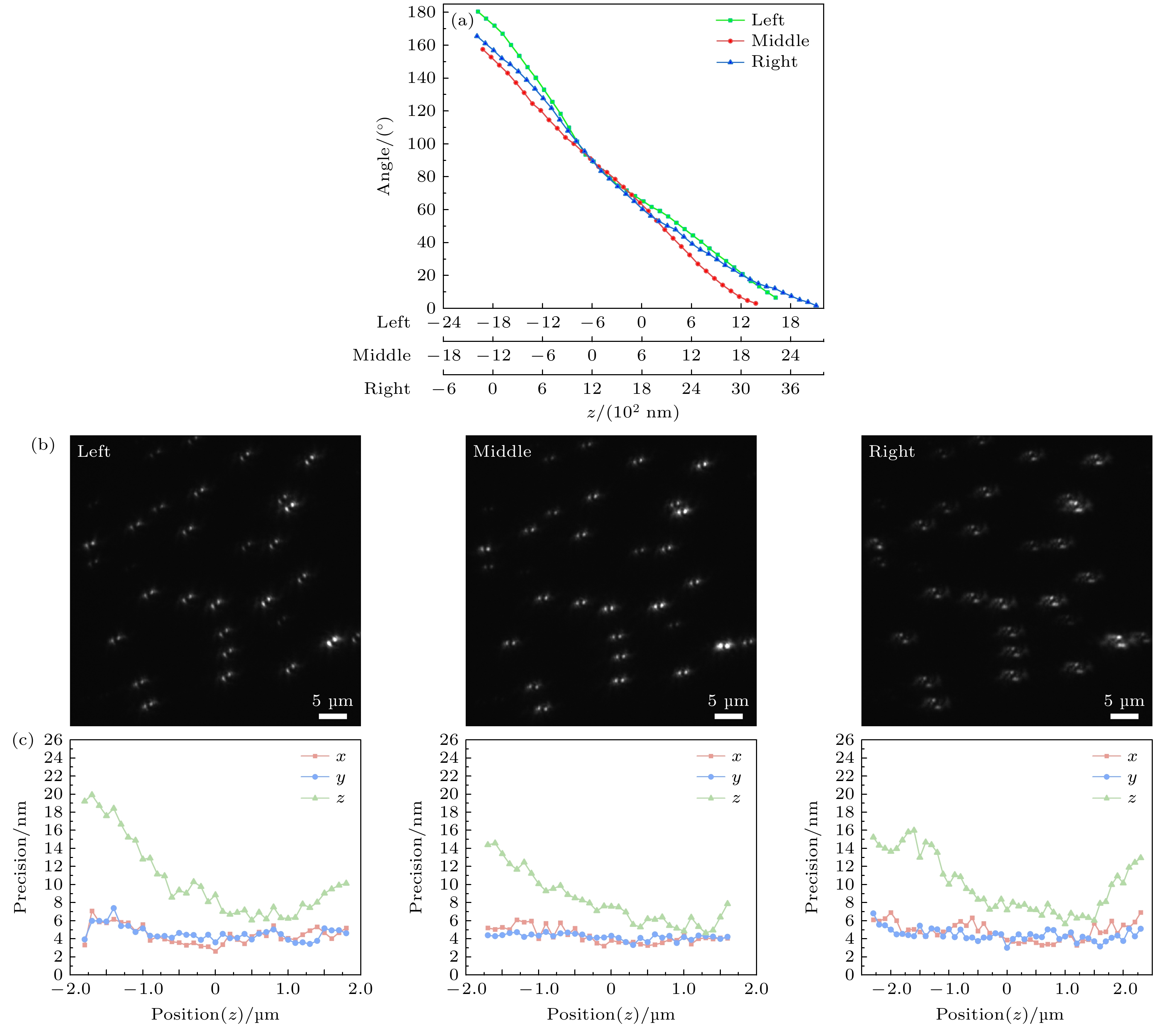
图 3 左、中、右三通道的标定 (a) 3个通道的标定曲线; (b) 同一深度下左、中、右3个通道对应的双螺旋成像结果; (c) 左、中、右3个通道的三维定位精度
Fig. 3. Calibration of the left, middle and right channels: (a) Calibration curves for the three channels; (b) the corresponding double-helix imaging results of the left, middle and right channels at the same depth; (c) 3D localization accuracy of the left, middle and right channels.
图 4 20%浓度甘油水溶液中的荧光微球的三维追踪 (a) 200 nm荧光微球在20%甘油水溶液中扩散时的动态变化图像; (b) 对图(a)红框中的荧光微球的运动轨迹进行图像重构所得到的三维追踪轨迹; (c)—(e) 图(b)中荧光微球的运动轨迹分别投射在xy面、yz面、xz面的投影
Fig. 4. 3D tracking of fluorescent beads in a 20% glycerol aqueous solution: (a) Image of the dynamics of a 200 nm fluorescent bead during diffusion in a 20% glycerol aqueous solution; (b) 3D tracking trajectory obtained by image reconstruction of the motion trajectory of the fluorescent microspheres in the red box in panel (a); (c)–(e) projections of the trajectories of the fluorescent microspheres in panel (b) onto the xy, yz and xz planes.
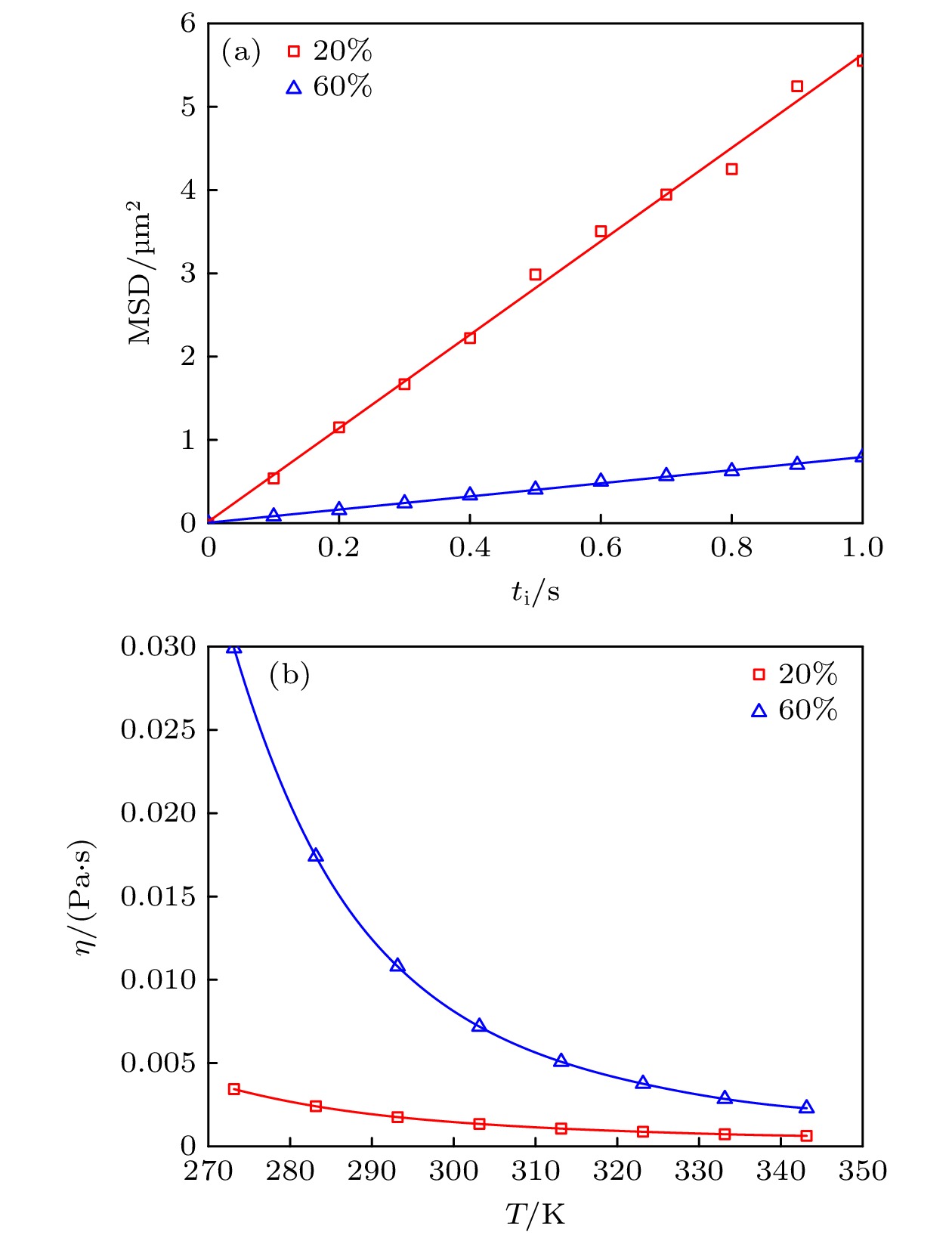
图 5 (a) 200 nm荧光微球在20%及60%浓度甘油水溶液中扩散时的均方位移曲线; (b) 20%及60%浓度下甘油水溶液的黏度随温度变化曲线图
Fig. 5. (a) Mean square displacement curves for the diffusion of 200 nm fluorescent beads in aqueous glycerol solutions at 20% and 60% concentrations; (b) viscosity versus temperature plots for aqueous glycerol solutions at 20% and 60% concentrations.
图 6 活细胞中荧光微球的三维示踪 (a) 巨噬细胞Raw-264.7的明场图像; (b) 荧光微球DH-PSF图像; (c) 巨噬细胞中荧光微球的三维运动轨迹
Fig. 6. 3D tracking of fluorescent beads in live cells: (a) White light image of the Raw-264.7 cell; (b) DH-PSF fluorescence image of a bead; (c) 3D trajectory of the bead in Raw-264.7 cell.
-
[1] Dupont A, Lamb D C 2011 Nanoscale 3 4532
Google Scholar
[2] Kusumi A, Tsunoyama T A, Hirosawa K M, Kasai R S, Fujiwara T K 2014 Nat. Chem. Biol. 10 524
Google Scholar
[3] Gal N, Lechtman-Goldstein D, Weihs D 2013 Rheol. Acta 52 425
Google Scholar
[4] Cognet L, Leduc C, Lounis B 2014 Curr. Opin. Chem. Biol. 20 78
Google Scholar
[5] Kubitscheck U, Kuckmann O, Kues T, Peters R 2000 Biophys. J. 78 2170
Google Scholar
[6] Carter B C, Shubeita G T, Gross S P 2005 Phys. Biol. 2 60
Google Scholar
[7] Arhel N, Genovesio A, Kim K A, Miko S, Perret E, OlivoMarin J C, Shorte S, Charneau P 2006 Nat. Methods 3 817
Google Scholar
[8] Bacher C P, Reichenzeller M, Athale C, Herrmann H, Eils R 2004 BMC. Cell Biol. 5 45
Google Scholar
[9] Greengard A, Schechner Y, Piestun R 2006 Opt. Lett. 31 181
Google Scholar
[10] Pavani S R P, DeLuca J G, Piestun R. 2009 Opt. Express 17 19644
Google Scholar
[11] Thompson M A, Lew M D, Badieirostami M 2010 Nano Lett. 10 211
Google Scholar
[12] Thompson M A, Casolari J M, Badieirostami M 2010 PNAS 107 17864
Google Scholar
[13] Wang D P, Agrawal A, Piestun R 2017 Appl. Phys. Lett. 110 211107
Google Scholar
[14] Gahlmann A, Moerner W E 2014 Nat. Rev. Microbiol. 12 9
Google Scholar
[15] Wang D P, Wu H C, Schwartz D K 2017 Phys. Rev. Lett. 119 268001
Google Scholar
[16] Wu H C, Sarfati R, Wang D P 2020 J. Am. Chem. Soc. 142 4696
Google Scholar
[17] Lasker K, von Diezmann L, Zhou X 2020 Nat. Microbiol. 5 418
Google Scholar
[18] Rocha J M, Gahlmann A 2019 Jove-J. Vis. Exp. 151 59387
Google Scholar
[19] Li H, Chen D N, Xu G X, Yu B, Niu H B 2015 Opt. Express 23 787
Google Scholar
[20] Li H F, Wang F M, Wei T D, Miao X, Cheng Y, Pang X P, Jiang K M, Huang W, Zhang Y H 2021 Opt. Lett. 46 5088
Google Scholar
[21] Xiao S, Gritton H, Tseng H A, Zemel D, Han X, Mertz J 2020 Optica 7 1477
Google Scholar
[22] 霍英东, 曹博, 于斌, 陈丹妮, 牛憨笨 2015 64 028701
Google Scholar
Huo Y D, Cao B, Yu B, Chen D N, Niu H B 2015 Acta Phys. Sin. 64 028701
Google Scholar
[23] 林丹樱, 龚振权, 黄黎琳, 聂梦娇, 于斌, 屈军乐 2024 73 068701
Google Scholar
Lin D Y, Gong Z Q, Huang L, Nie M J, Yu B, Qu J L 2024 Acta Phys. Sin. 73 068701
Google Scholar
下载:
计量
- 文章访问数: 1629
- PDF下载量: 94
- 被引次数: 0
