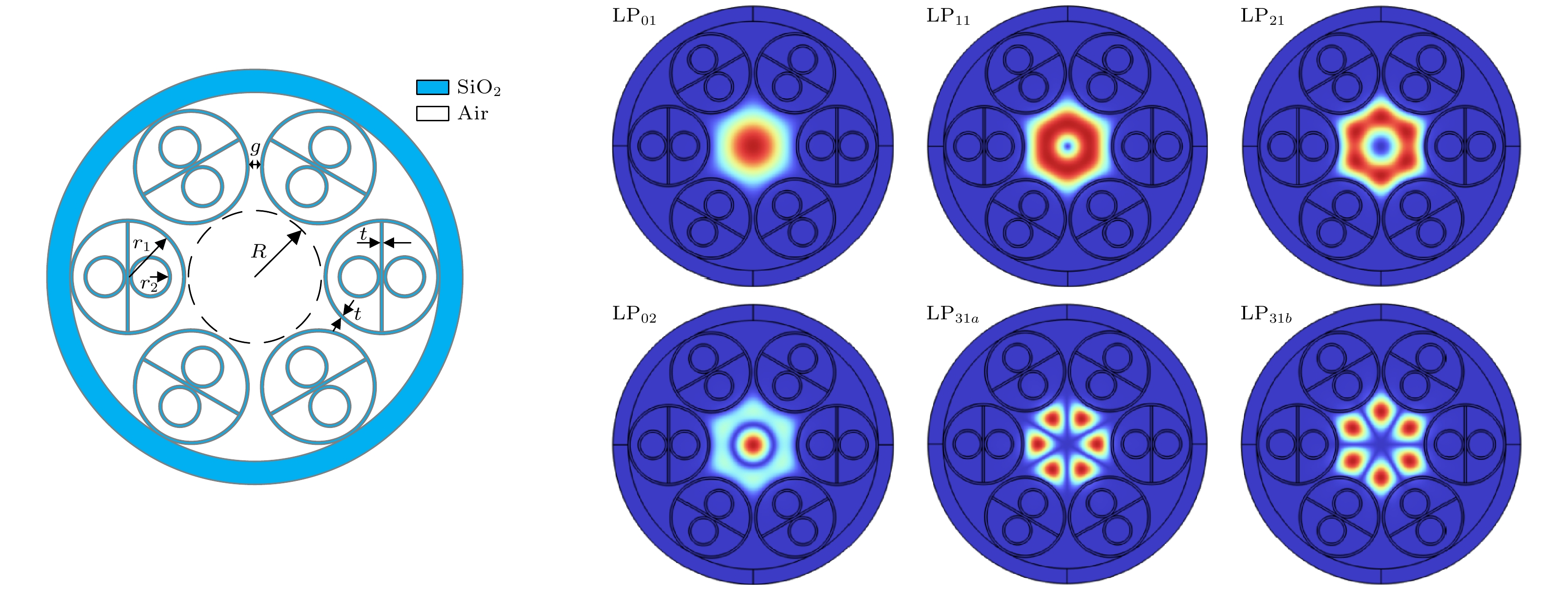
-
本文设计了一种具有对称双环嵌套管结构的新型低损耗少模空芯负曲率光纤, 该光纤支持LP01, LP11, LP21, LP02, LP31a, LP31b共6种纤芯模式. 所设计的光纤以SiO2作为基底材料, 采用特殊的对称双环嵌套结构将包层区域进行划分, 能够有效地减小纤芯模式与包层模式的耦合. 使用有限元法对该少模空芯负曲率光纤的结构参数进行优化, 并分析了纤芯各个模式的限制损耗和弯曲损耗. 仿真结果表明, 所提出的少模空芯负曲率光纤能够同时支持弱耦合的6种纤芯模式独立传输(相邻模式间的有效折射率差均大于10–4, 有效地避免了纤芯内模式间的耦合). 在400 nm带宽(1.23—1.63 μm, 覆盖O, E, S, C, L波段)范围内, 纤芯中的6个模式均保持低损耗稳定传输. 各模式限制损耗在1.4 μm处达到最低, 其中基模LP01模式的限制损耗最低, 为4.3×10–7 dB/m. 此外, 当弯曲半径为7 cm时, 各模式在一定工作波长范围内均保持低弯曲损耗传输. 公差分析表明, 当结构参数偏移±1%时, 该少模空芯负曲率光纤仍然可以保持低损耗弱耦合的传输特性.Few-mode optical fibers have played an increasingly important role in breaking through the transmission capacity limitations of single-mode optical fiber and alleviating the bandwidth crisis in optic fiber communication systems in recent years. Nevertheless, traditional solid core few-mode optical fibers usually suffer optical fiber nonlinearity and mode coupling, leading to mode crosstalk between channels. Hollow core negative curvature fibers (HC-NCF) have attracted widespread attention due to their advantages, such as low latency, low nonlinearity, low dispersion, low transmission loss, and large operating bandwidth. In this work, a novel low-loss few-mode HC-NCF with symmetrically double ring nested tube structure is designed, which supports six core modes including LP01, LP11, LP21, LP02, LP31a, and LP31b. The designed optical fiber is based on silica dioxide substrate and adopts a unique symmetrical double ring nested cladding structure, which can effectively suppress the coupling between the core mode and the cladding mode. The finite element method (FDE) is used to numerically analyze the properties of the proposed few-mode HC-NCF and optimize the structural parameters of the few-mode HC-NCF. Moreover, the confinement loss and bending loss of all core modes are investigated. The simulation results show that the proposed few-mode HC-NCF can support the independent transmission of six weakly coupled core modes (with the effective refractive index difference greater than 1×10–4 between the adjacent core modes, which greatly avoids the coupling between the adjacent modes in the fiber core). In the 400 nm bandwidth (1.23–1.63 μm, covering the O, E, S, C, and L bands), all six modes in the fiber core maintain low loss transmission. Moreover, in the range of 1.3–1.63 μm, the confinement loss (CL) of LP01, LP11 and LP21 mode are all less than 1×10–3 dB/m, and the CL of LP02 and LP31b mode are both less than 3×10–3 dB/m. The CL of each mode reaches the lowest value at 1.4 μm, and the LP01 mode has the lowest CL of 4.3×10–7 dB/m. In addition, for a bending radius of 7 cm, each mode maintains the low bending loss characteristic in a certain operating wavelength range. In the range of 1.23–1.61 μm, the BL of LP01 is less than 4.5×10–4 dB/m, and the BL of LP11 is less than 1.3×10–3 dB/m. The tolerance analysis shows that even with the deviation of structural parameters of ±1%, the few-mode HC-NCF can still maintain the characteristic of low-loss and weak coupling. The designed few-mode HC-NCF has ultra-low CL and bending-insensitive characteristics while supporting independent transmission of six modes, which will find huge potential applications in future high performance mode division multiplexing systems.
[1] Benabid F, Knight J C, Antonopoulos G, Russell P S J 2002 Science 298 399
Google Scholar
[2] Poletti F, Wheeler N V, Petrovich M N, Baddela N, Fokoua E N, Hayes J R, Gray D R, Li Z, Slavík R, Richardson D J 2013 Nat. Photonics 7 279
Google Scholar
[3] Belardi W, Knight J C 2014 Opt. Lett. 39 1853
Google Scholar
[4] Yu F, Knight J C 2016 IEEE J. Sel. Top. Quantum Electron. 22 146
Google Scholar
[5] Hasan M I, Akhmediev N, Chang W 2017 Opt. Lett. 42 703
Google Scholar
[6] Shen W, Du J, Sun L, Wang C, He Z 2020 J. Lightwave Technol. 38 3874
Google Scholar
[7] Liu Z, Karanov B, Galdino L, Hayes J R, Lavery D, Clark K, Shi K, Elson D J, Thomsen B C, Petrovich M N, Richardson D J, Poletti F, Slavik R, Bayvel P 2019 J. Lightwave Technol. 37 909
Google Scholar
[8] Michaud-Belleau V, Fokoua E R N, Bradley T, Hayes J R, Slavik R 2021 Optica 8 216
Google Scholar
[9] Zhu X, Wu D, Wang Y, Yu F, Li Q, Qi Y, Knight J, Chen S, Hu L 2021 Opt. Express 29 1492
Google Scholar
[10] Azendorf F, Schmauss B, Shi B, Fokoua E N, Radan Slavík, Eiselt M 2021 Optical Fiber Communications Conference and Exhibition (OFC) San Francisco, California United States, June 6–10, 2021 p1
[11] Liu W, Zheng Y, Wang Z, Wang Z X, Yang J, Chen M X, Qi M, Rehman S U, Shum P P, Zhu L, Wei L 2021 Adv. Mater. Interfaces 8 2001978
Google Scholar
[12] Gérôme F, Cook K T, George A K, Wadsworth W J, Knight J C 2007 Opt. Express 15 7126
Google Scholar
[13] Urich A, Maier R R, Yu F, Knight J C, Hand D P, Shephard J D 2013 Biomed. Opt. Express 4 193
Google Scholar
[14] Couch D E, Hickstein D D, Winters D G, Backus S J, Kirchner M S, Domingue S R, Ramirez J J, Durfee C G, Murnane M M, Kapteyn H C 2020 Optica 7 832
Google Scholar
[15] Poletti F 2014 Opt. Express 22 23807
Google Scholar
[16] Cregan R F, Mangan B J, Knight J C, Birks T A, Russell P S, Roberts P J, Allan D C 1999 Science 285 1537
Google Scholar
[17] Roberts P, Couny F, Sabert H, Mangan B, Williams D, Farr L, Mason M, Tomlinson A, Birks T, Knight J, Russell S J P 2005 Opt. Express 13 236
Google Scholar
[18] Luan F, George A K, Hedley T D, Pearce G J, Bird D M, Knight J C, Russell P S J 2004 Opt. Lett. 29 2369
Google Scholar
[19] Wei C, Weiblen R J, Menyuk C R, Hu J 2017 Adv. Opt. Photonics 9 562
Google Scholar
[20] Jasion G T, Bradley T, Harrington K, Sakr H, Poletti F 2020 Optical Fiber Communications Conference and Exhibition (OFC) San Diego, California United States, March 8–12, 2020
[21] Osório J H, Amrani F, Delahaye F, Dhaybi A, Vasko K, Melli F, Giovanardi F, Vandembroucq D, Tessier G, Vincetti L, Debord B, Gérôme F, Benabid F 2023 Nat. Commun. 14 1146
Google Scholar
[22] Mulvad H C H, Abokhamis Mousavi S, Zuba V, Xu L, Sakr H, Bradley T D, Hayes J R, Jasion G T, Numkam Fokoua E R, Taranta A, Alam S, Richardson D J, Poletti F 2022 Nat. Photonics 16 448
Google Scholar
[23] Ding W, Wang Y Y, Gao S, Wang M, Wang P 2020 IEEE J. Sel. Top. Quantum Electron. 26 4400312
Google Scholar
[24] Gao S F, Wang Y Y, Ding W, Jiang D, Gu S, Zhang X, Wang P 2018 Nat. Commun. 9 2828
Google Scholar
[25] Yue B, Feng J, Tao J, Zhou G, Huang X 2021 Opt. Fiber Technol. 67 102734
Google Scholar
[26] Xue L, Sheng X, Jia H, Lou S 2023 J. Lightwave Technol. 41 6043
Google Scholar
[27] Belardi W 2015 J. Lightwave Technol. 33 4497
Google Scholar
[28] Yan S B, Lou S, Wang X, Zhang W, Zhao T 2018 Opt. Fiber Technol. 46 118
Google Scholar
[29] Michieletto M, Lyngsø J K, Jakobsen C, Lægsgaard J, Bang O, Alkeskjold T T 2016 Opt. Express 24 7103
Google Scholar
[30] Zhang X, Feng Z, Marpaung D A, Fokoua E R, Sakr H, Hayes J R, Poletti F, Richardson D J, Slavík R 2022 Light Sci. Appl 11 213
Google Scholar
[31] Yao C Y, Gao S F, Wang Y Y, Wang P, Jin W, Ren W 2020 J. Lightwave Technol. 38 2067
Google Scholar
[32] Ma X X, Li J S, Guo H T, Li S G, Zhang H, Xu Y T, Meng X J, Guo Y, Chen Q, Wang C J, Cui X W 2023 Plasmonics 18 899
Google Scholar
[33] Zhang H, Chang Y J, Xu Y T, Liu C Z, Xiao X S, Li J S, Ma X X, Wang Y Y, Guo H T 2023 Opt. Express 31 7659
Google Scholar
[34] Zhou Y, Cao R, Wang S, Peng J, Li H, Chu Y, Xing Y, Dai N, Li J 2022 IEEE Photonics J. 14 1
Google Scholar
[35] Zhu Y, Wang S, Chen M, Zuo X, Wang H, Rao C, Xu Y, Ji D, Liu Y 2022 IEEE Photonics Technol. Lett. 34 283
Google Scholar
[36] Nawazuddin M B, Wheeler N V, Hayes J R, Bradley T D, Sandoghchi S R, Gouveia M A, Jasion G T, Richardson D J, Poletti F 2018 J. Lightwave Technol. 36 1213
Google Scholar
[37] Yan S, Lou S, Lian Z, Zhang W, Wang X 2019 J. Lightwave Technol. 37 5707
Google Scholar
[38] Luo L W, Ophir N, Chen C P, Gabrielli L H, Poitras C B, Bergmen K, Lipson M 2014 Nat. Commun. 5 3069
Google Scholar
[39] Chen Y X, Lin Z J, Bélanger-de Villers S, Rusch L A, Shi W 2020 IEEE J. Sel. Top. Quantum Electron. 26 6100107
Google Scholar
[40] Naghshvarianjahromi M, Kumar S, Deen M J, Iwaya T, Kimura K, Yoshida M, Hirooka T, Nakazawa M 2022 IEEE J. Sel. Top. Quantum Electron. 28 7500210
Google Scholar
[41] Richardson D J, Fini J M, Nelson L E 2013 Nat. Photonics 7 354
Google Scholar
[42] Tarighat A, Hsu R C J, Shah A, Sayed A H, Jalali B 2007 IEEE Commun. Mag. 45 57
Google Scholar
[43] Berdagué S, Facq P 1982 Appl. Opt. 21 1950
Google Scholar
[44] Habib M S, Antonio-Lopez J E, Markos C, Schülzgen A, Amezcua-Correa R 2019 Opt. Express 27 3824
Google Scholar
[45] Habib M S, Bang O, Bache M 2016 Opt. Express 24 8429
Google Scholar
[46] Wang Z, Tu J, Liu Z, Yu C, Lu C 2020 J. Lightwave Technol. 38 864
Google Scholar
[47] Goel C, Yoo S 2021 J. Lightwave Technol. 39 6592
Google Scholar
[48] Ou J, Li J P, Zheng W Q, Qin Y W, Xu O, Huang Q D, Peng D, Xiang M, Xu Y, Fu S N 2022 20th International Conference on Optical Communications and Networks (ICOCN) Shenzhen, China, August 12–15, 2022 p1
[49] Liu H, Wang Y, Zhou Y, Guan Z, Yu Z, Ling Q, Luo S, Shao J, Huang D, Chen D 2022 Opt. Express 30 21833
Google Scholar
[50] Vincetti L, Setti V 2012 Opt. Express 20 14350
Google Scholar
[51] Zhang J, Wang Z, Chen J 2014 Proc. COMSOL Conf. Shanghai, China 2014 p2
[52] Litchinitser N M, Abeeluck A K, Headley C, Eggleton B J 2002 Opt. Lett. 27 1592
Google Scholar
[53] Vincetti L 2016 Opt. Express 24 10313
Google Scholar
[54] Chen X, Hu X, Yang L, Peng J, Li H, Dai N, Li J 2019 Opt. Express 27 19548
Google Scholar
[55] Wang L, LaRochelle S 2015 Opt. Lett. 40 5846
Google Scholar
[56] Nagano K, Kawakami S, Nishida S 1978 Appl. Opt. 17 2080
Google Scholar
[57] Belardi W, Knight J C 2014 Opt. Express 22 10091
Google Scholar
[58] Pryamikov A D, Biriukov A S, Kosolapov A F, Plotnichenko V G, Semjonov S L, Dianov E M 2011 Opt. Express 19 1441
Google Scholar
[59] Yu F, Wadsworth W J, Knight J C 2012 Opt. Express 20 11153
Google Scholar
[60] Yang S, Sheng X, Zhao G, Lou S, Guo J 2021 IEEE Access 9 29599
Google Scholar
[61] Hayashi J G, Ventura A, Cimek J, Slimen F B, White N, Sakr H, Jasion G T, Wheeler N V, Poletti F 2020 22nd International Conference on Transparent Optical Networks (ICTON) Bari, Italy, July 19–23, 2020 p1
[62] Shaha K S R, Khaleque A 2021 Appl. Opt. 60 6243
Google Scholar
[63] Wei C, Weiblen R J, Menyuk C R, Hu J 2017 Adv. Opt. Photonics 9 504
Google Scholar
-
图 1 对称双环嵌套管少模HC-NCF的横截面结构图
Fig. 1. Cross sectional structure of few-mode HC-NCF with symmetrically double ring nested tube structure.
图 2 当纤芯半径R = 16 μm和k = 0.4时, 改变g对模式传输特性的影响 (a) 有效折射率; (b) CL
Fig. 2. When the core radius R = 16 μm and k = 0.4, the impact of changing g on mode transmission characteristics: (a) Effective refractive index; (b) CL.
图 3 少模HC-NCF中纤芯模式的模场分布图
Fig. 3. Mode field distribution of guided core modes in the few-mode HC-NCF.
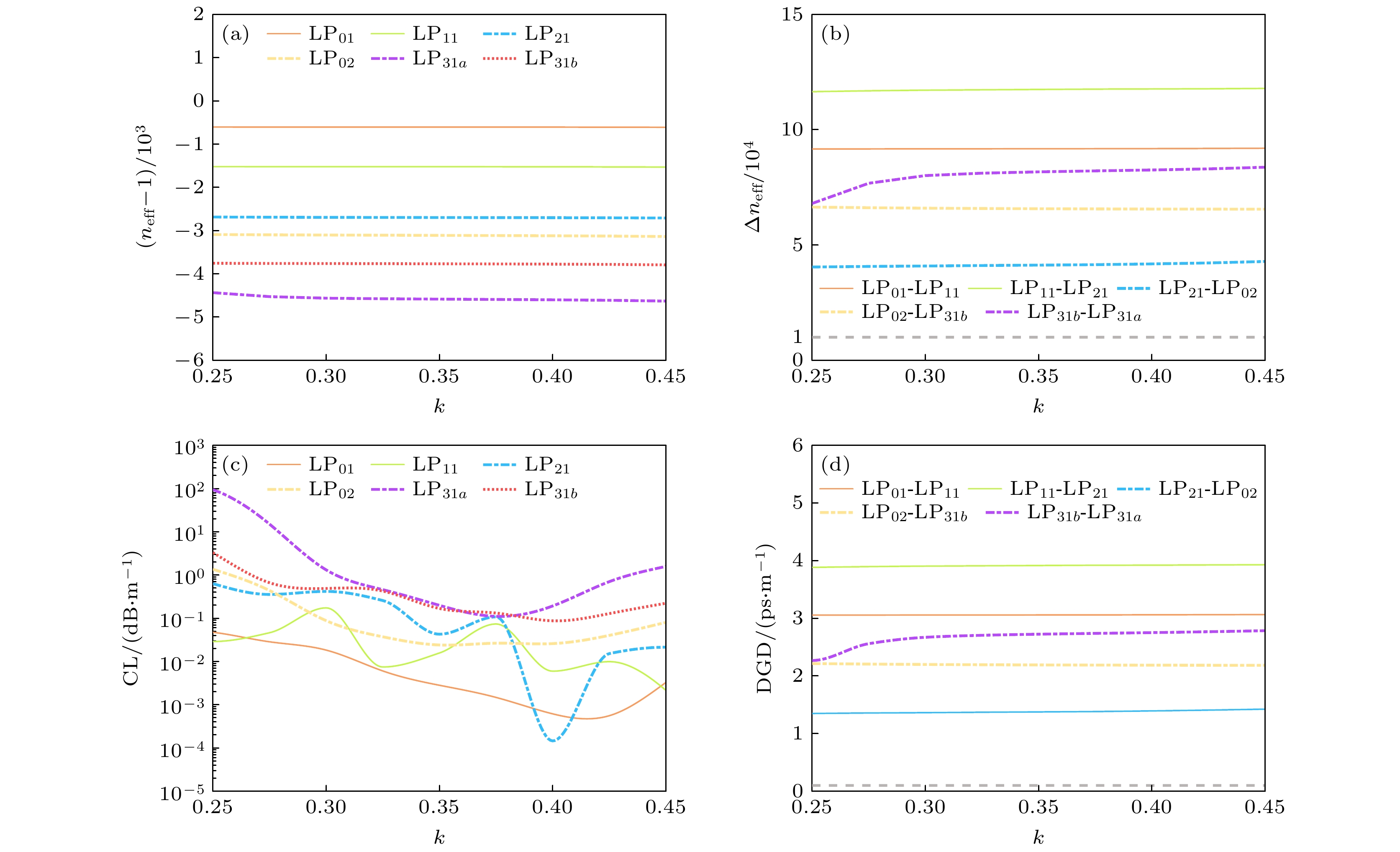
图 4 当纤芯半径R = 16 μm, g = 0.5 μm, 改变k对模式传输特性的影响 (a) 有效折射率; (b) 相邻模式有效折射率差; (c) CL; (d) 相邻模式间DGD
Fig. 4. Impact of changing k on mode transmission characteristics for R = 16 μm and g = 0.5 μm: (a) Effective refractive index; (b) difference of effective refractive index of adjacent modes; (c) CL; (d) DGD between adjacent modes.
图 5 k = 0.25, LP31a 模的模场分布 (a) 二维平面图; (b) 三维立体图
Fig. 5. Mode field distribution of LP31a modes at k = 0.25: (a) 2D plane diagram; (b) 3D stereo diagram.
图 6 当g = 0.5 μm, k = 0.4时, 改变纤芯半径R对模式传输的影响 (a) 有效折射率; (b) 相邻模式有效折射率差; (c) CL; (d) 相邻模式间的DGD
Fig. 6. Impact of changing R on mode transmission characteristics for g = 0.5 μm and k = 0.4: (a) Effective refractive index; (b) difference of effective refractive index of adjacent modes; (c) CL; (d) DGD between adjacent modes.
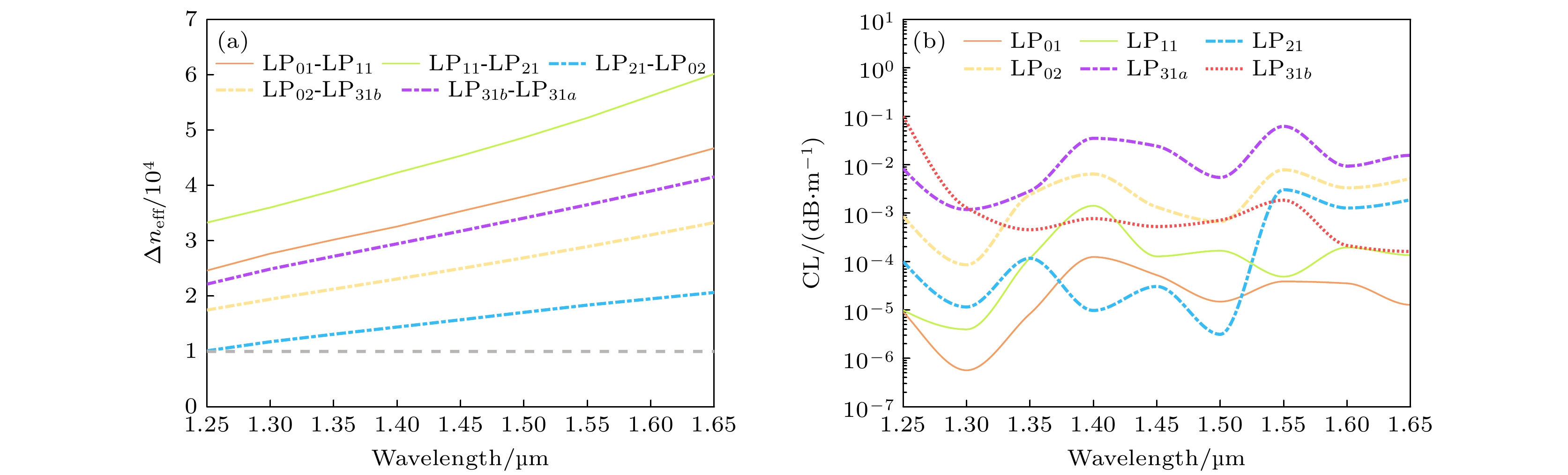
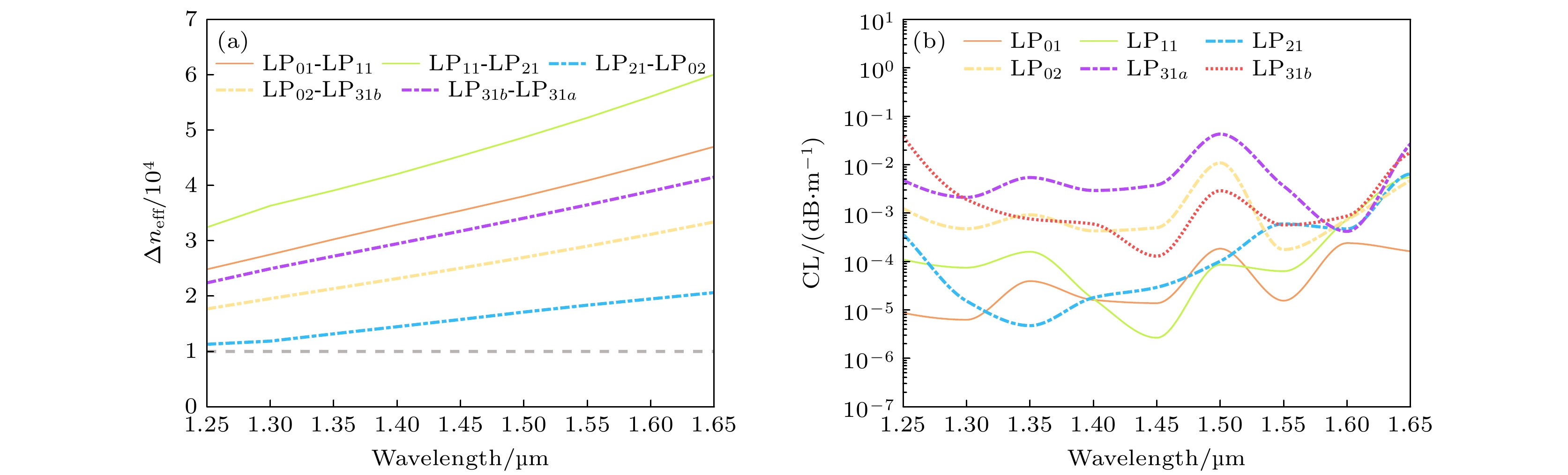
图 7 当g = 0.5 μm, k = 0.4, R = 24 μm时, 波长变化对模式传输的影响 (a) 有效折射率; (b) 相邻模式有效折射率差; (c) CL; (d) 相邻模式间的DGD
Fig. 7. Variation of changing wavelength on mode transmission characteristics for g = 0.5 μm, k = 0.4 and R = 16 μm: (a) Effective refractive index; (b) difference of effective refractive index of adjacent modes; (c) CL; (d) DGD between adjacent modes.
图 8 当g = 0.5 μm, k = 0.4, R = 24 μm时, 不同弯曲半径对模式传输的影响 (a) 预期基线; (b) 有效折射率; (c) 相邻模式有效折射率差; (d) BL
Fig. 8. Variation of changing bending radius on mode transmission characteristics for g = 0.5 μm, k = 0.4: (a) Expected baseline; (b) effective refractive index; (c) difference of effective refractive index of adjacent modes; (d) BL.
图 9 当弯曲半径Rb = 7 cm时, 不同波长对模式传输的影响 (a) 相邻模式有效折射率差; (b) BL
Fig. 9. Variation of changing wavelength on mode transmission with bending radius Rb = 7 cm: (a) Difference of effective refractive index of adjacent modes; (b) BL.
图 10 嵌套管壁厚参数t偏移+1%时, 相邻模式有效折射率差和CL的变化
Fig. 10. With nested tube wall thickness parameter t deviation +1%, the change of effective refractive index difference of adjacent mode and CL.
图 11 嵌套管壁厚参数t偏移–1%时, 相邻模式有效折射率差和CL的变化
Fig. 11. With nested tube wall thickness parameter t deviation –1%, the change of effective refractive index difference of adjacent mode and CL.
图 12 参数k偏移+1%时, 相邻模式有效折射率差和CL的变化
Fig. 12. With parameter k deviation +1%, the change of effective refractive index difference of adjacent mode and CL.
图 13 参数k偏移–1%时, 相邻模式有效折射率差和CL的变化
Fig. 13. With parameter k deviation -1%, the change of effective refractive index difference of adjacent mode and CL.
表 1 少模HC-NCF性能比较
Table 1. Performance comparison of few-mode HC-NCF
结构 中心波长/µm 支持模式数 基模最低限制损耗/(dB·m–1) 工作带宽/nm 弯曲半径/cm 弯曲损耗/(dB·m–1) Wang Z, et al. (2020)[46] 1.55 2 1.7×10–4 @1.53 µm 340 10 6.6×10–4 (200 nm) Goel C, et al. (2021)[47] 1.00 5 1.4×10–5@1 µm — 20 5×10–3 Ou J, et al. (2022)[48] 1.55 2 7.4×10–7@1.06 µm 800 — — Liu H, et al. (2022)[49] 1.55 5 3.4×10–7@1.38 µm 300 6 3×10–4 (210 nm) Our work 1.55 6 4.3×10–7@1.4 µm 330 7 4.5×10–4 (420 nm) 
下载: 导出CSV
-
[1] Benabid F, Knight J C, Antonopoulos G, Russell P S J 2002 Science 298 399
Google Scholar
[2] Poletti F, Wheeler N V, Petrovich M N, Baddela N, Fokoua E N, Hayes J R, Gray D R, Li Z, Slavík R, Richardson D J 2013 Nat. Photonics 7 279
Google Scholar
[3] Belardi W, Knight J C 2014 Opt. Lett. 39 1853
Google Scholar
[4] Yu F, Knight J C 2016 IEEE J. Sel. Top. Quantum Electron. 22 146
Google Scholar
[5] Hasan M I, Akhmediev N, Chang W 2017 Opt. Lett. 42 703
Google Scholar
[6] Shen W, Du J, Sun L, Wang C, He Z 2020 J. Lightwave Technol. 38 3874
Google Scholar
[7] Liu Z, Karanov B, Galdino L, Hayes J R, Lavery D, Clark K, Shi K, Elson D J, Thomsen B C, Petrovich M N, Richardson D J, Poletti F, Slavik R, Bayvel P 2019 J. Lightwave Technol. 37 909
Google Scholar
[8] Michaud-Belleau V, Fokoua E R N, Bradley T, Hayes J R, Slavik R 2021 Optica 8 216
Google Scholar
[9] Zhu X, Wu D, Wang Y, Yu F, Li Q, Qi Y, Knight J, Chen S, Hu L 2021 Opt. Express 29 1492
Google Scholar
[10] Azendorf F, Schmauss B, Shi B, Fokoua E N, Radan Slavík, Eiselt M 2021 Optical Fiber Communications Conference and Exhibition (OFC) San Francisco, California United States, June 6–10, 2021 p1
[11] Liu W, Zheng Y, Wang Z, Wang Z X, Yang J, Chen M X, Qi M, Rehman S U, Shum P P, Zhu L, Wei L 2021 Adv. Mater. Interfaces 8 2001978
Google Scholar
[12] Gérôme F, Cook K T, George A K, Wadsworth W J, Knight J C 2007 Opt. Express 15 7126
Google Scholar
[13] Urich A, Maier R R, Yu F, Knight J C, Hand D P, Shephard J D 2013 Biomed. Opt. Express 4 193
Google Scholar
[14] Couch D E, Hickstein D D, Winters D G, Backus S J, Kirchner M S, Domingue S R, Ramirez J J, Durfee C G, Murnane M M, Kapteyn H C 2020 Optica 7 832
Google Scholar
[15] Poletti F 2014 Opt. Express 22 23807
Google Scholar
[16] Cregan R F, Mangan B J, Knight J C, Birks T A, Russell P S, Roberts P J, Allan D C 1999 Science 285 1537
Google Scholar
[17] Roberts P, Couny F, Sabert H, Mangan B, Williams D, Farr L, Mason M, Tomlinson A, Birks T, Knight J, Russell S J P 2005 Opt. Express 13 236
Google Scholar
[18] Luan F, George A K, Hedley T D, Pearce G J, Bird D M, Knight J C, Russell P S J 2004 Opt. Lett. 29 2369
Google Scholar
[19] Wei C, Weiblen R J, Menyuk C R, Hu J 2017 Adv. Opt. Photonics 9 562
Google Scholar
[20] Jasion G T, Bradley T, Harrington K, Sakr H, Poletti F 2020 Optical Fiber Communications Conference and Exhibition (OFC) San Diego, California United States, March 8–12, 2020
[21] Osório J H, Amrani F, Delahaye F, Dhaybi A, Vasko K, Melli F, Giovanardi F, Vandembroucq D, Tessier G, Vincetti L, Debord B, Gérôme F, Benabid F 2023 Nat. Commun. 14 1146
Google Scholar
[22] Mulvad H C H, Abokhamis Mousavi S, Zuba V, Xu L, Sakr H, Bradley T D, Hayes J R, Jasion G T, Numkam Fokoua E R, Taranta A, Alam S, Richardson D J, Poletti F 2022 Nat. Photonics 16 448
Google Scholar
[23] Ding W, Wang Y Y, Gao S, Wang M, Wang P 2020 IEEE J. Sel. Top. Quantum Electron. 26 4400312
Google Scholar
[24] Gao S F, Wang Y Y, Ding W, Jiang D, Gu S, Zhang X, Wang P 2018 Nat. Commun. 9 2828
Google Scholar
[25] Yue B, Feng J, Tao J, Zhou G, Huang X 2021 Opt. Fiber Technol. 67 102734
Google Scholar
[26] Xue L, Sheng X, Jia H, Lou S 2023 J. Lightwave Technol. 41 6043
Google Scholar
[27] Belardi W 2015 J. Lightwave Technol. 33 4497
Google Scholar
[28] Yan S B, Lou S, Wang X, Zhang W, Zhao T 2018 Opt. Fiber Technol. 46 118
Google Scholar
[29] Michieletto M, Lyngsø J K, Jakobsen C, Lægsgaard J, Bang O, Alkeskjold T T 2016 Opt. Express 24 7103
Google Scholar
[30] Zhang X, Feng Z, Marpaung D A, Fokoua E R, Sakr H, Hayes J R, Poletti F, Richardson D J, Slavík R 2022 Light Sci. Appl 11 213
Google Scholar
[31] Yao C Y, Gao S F, Wang Y Y, Wang P, Jin W, Ren W 2020 J. Lightwave Technol. 38 2067
Google Scholar
[32] Ma X X, Li J S, Guo H T, Li S G, Zhang H, Xu Y T, Meng X J, Guo Y, Chen Q, Wang C J, Cui X W 2023 Plasmonics 18 899
Google Scholar
[33] Zhang H, Chang Y J, Xu Y T, Liu C Z, Xiao X S, Li J S, Ma X X, Wang Y Y, Guo H T 2023 Opt. Express 31 7659
Google Scholar
[34] Zhou Y, Cao R, Wang S, Peng J, Li H, Chu Y, Xing Y, Dai N, Li J 2022 IEEE Photonics J. 14 1
Google Scholar
[35] Zhu Y, Wang S, Chen M, Zuo X, Wang H, Rao C, Xu Y, Ji D, Liu Y 2022 IEEE Photonics Technol. Lett. 34 283
Google Scholar
[36] Nawazuddin M B, Wheeler N V, Hayes J R, Bradley T D, Sandoghchi S R, Gouveia M A, Jasion G T, Richardson D J, Poletti F 2018 J. Lightwave Technol. 36 1213
Google Scholar
[37] Yan S, Lou S, Lian Z, Zhang W, Wang X 2019 J. Lightwave Technol. 37 5707
Google Scholar
[38] Luo L W, Ophir N, Chen C P, Gabrielli L H, Poitras C B, Bergmen K, Lipson M 2014 Nat. Commun. 5 3069
Google Scholar
[39] Chen Y X, Lin Z J, Bélanger-de Villers S, Rusch L A, Shi W 2020 IEEE J. Sel. Top. Quantum Electron. 26 6100107
Google Scholar
[40] Naghshvarianjahromi M, Kumar S, Deen M J, Iwaya T, Kimura K, Yoshida M, Hirooka T, Nakazawa M 2022 IEEE J. Sel. Top. Quantum Electron. 28 7500210
Google Scholar
[41] Richardson D J, Fini J M, Nelson L E 2013 Nat. Photonics 7 354
Google Scholar
[42] Tarighat A, Hsu R C J, Shah A, Sayed A H, Jalali B 2007 IEEE Commun. Mag. 45 57
Google Scholar
[43] Berdagué S, Facq P 1982 Appl. Opt. 21 1950
Google Scholar
[44] Habib M S, Antonio-Lopez J E, Markos C, Schülzgen A, Amezcua-Correa R 2019 Opt. Express 27 3824
Google Scholar
[45] Habib M S, Bang O, Bache M 2016 Opt. Express 24 8429
Google Scholar
[46] Wang Z, Tu J, Liu Z, Yu C, Lu C 2020 J. Lightwave Technol. 38 864
Google Scholar
[47] Goel C, Yoo S 2021 J. Lightwave Technol. 39 6592
Google Scholar
[48] Ou J, Li J P, Zheng W Q, Qin Y W, Xu O, Huang Q D, Peng D, Xiang M, Xu Y, Fu S N 2022 20th International Conference on Optical Communications and Networks (ICOCN) Shenzhen, China, August 12–15, 2022 p1
[49] Liu H, Wang Y, Zhou Y, Guan Z, Yu Z, Ling Q, Luo S, Shao J, Huang D, Chen D 2022 Opt. Express 30 21833
Google Scholar
[50] Vincetti L, Setti V 2012 Opt. Express 20 14350
Google Scholar
[51] Zhang J, Wang Z, Chen J 2014 Proc. COMSOL Conf. Shanghai, China 2014 p2
[52] Litchinitser N M, Abeeluck A K, Headley C, Eggleton B J 2002 Opt. Lett. 27 1592
Google Scholar
[53] Vincetti L 2016 Opt. Express 24 10313
Google Scholar
[54] Chen X, Hu X, Yang L, Peng J, Li H, Dai N, Li J 2019 Opt. Express 27 19548
Google Scholar
[55] Wang L, LaRochelle S 2015 Opt. Lett. 40 5846
Google Scholar
[56] Nagano K, Kawakami S, Nishida S 1978 Appl. Opt. 17 2080
Google Scholar
[57] Belardi W, Knight J C 2014 Opt. Express 22 10091
Google Scholar
[58] Pryamikov A D, Biriukov A S, Kosolapov A F, Plotnichenko V G, Semjonov S L, Dianov E M 2011 Opt. Express 19 1441
Google Scholar
[59] Yu F, Wadsworth W J, Knight J C 2012 Opt. Express 20 11153
Google Scholar
[60] Yang S, Sheng X, Zhao G, Lou S, Guo J 2021 IEEE Access 9 29599
Google Scholar
[61] Hayashi J G, Ventura A, Cimek J, Slimen F B, White N, Sakr H, Jasion G T, Wheeler N V, Poletti F 2020 22nd International Conference on Transparent Optical Networks (ICTON) Bari, Italy, July 19–23, 2020 p1
[62] Shaha K S R, Khaleque A 2021 Appl. Opt. 60 6243
Google Scholar
[63] Wei C, Weiblen R J, Menyuk C R, Hu J 2017 Adv. Opt. Photonics 9 504
Google Scholar
下载:
计量
- 文章访问数: 2647
- PDF下载量: 94
- 被引次数: 0
