基于少模长周期光纤叠栅的模式转换器

薛艳茹; 田朋飞; 金娃; 赵能; 靳云; 毕卫红

doi:10.7498/aps.68.20181674

摘要

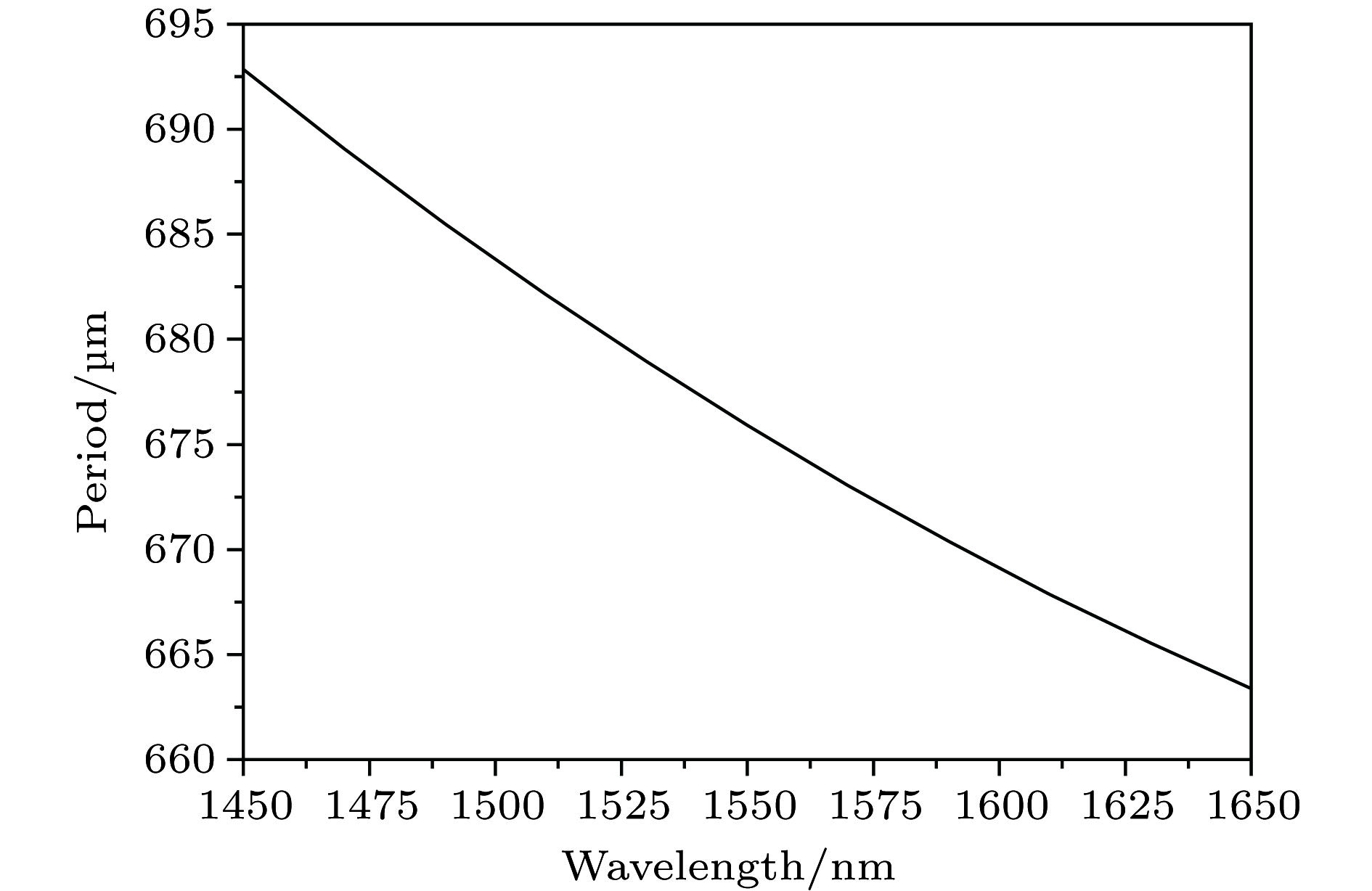
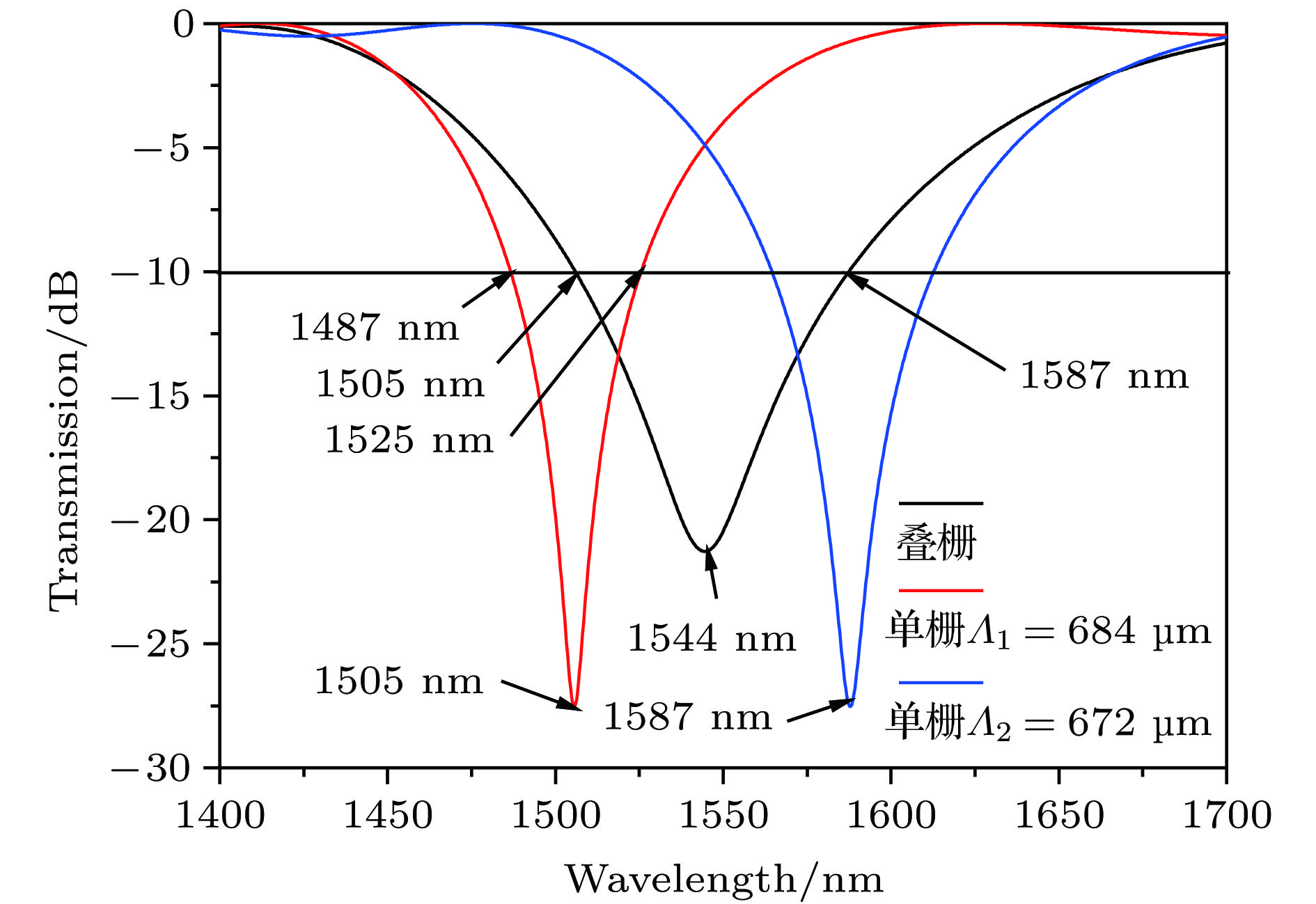
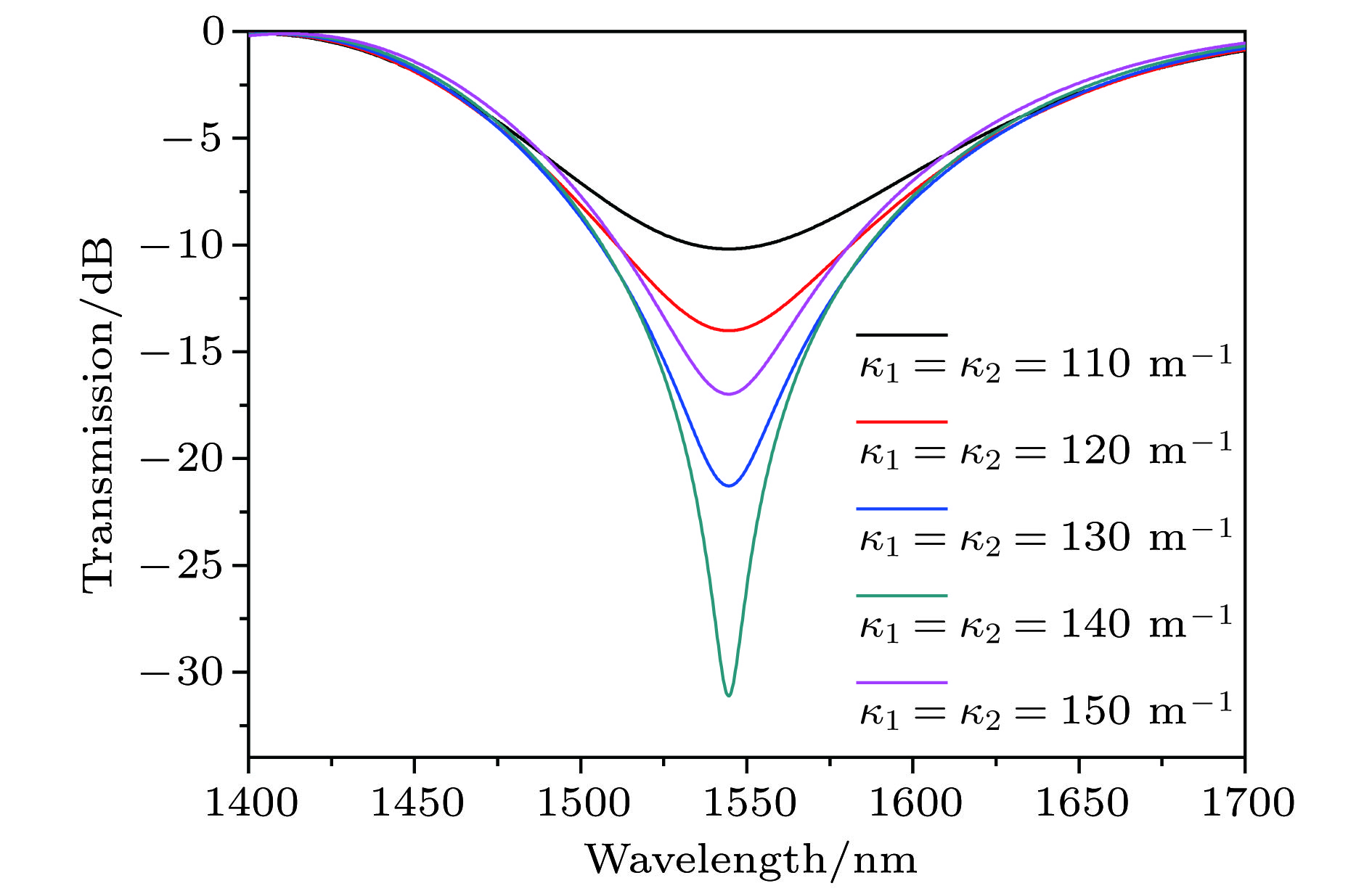
本文提出一种在同一段少模光纤上写入两个不同周期${\varLambda _1}$和${\varLambda _2}$的长周期光纤光栅构成叠栅的方法, 实现了纤芯基模LP₀₁向高阶纤芯模LP₁₁模式转换的宽带宽的新型的全光纤模式转换器. 利用有限元法和耦合模理论建立了模式转换器的理论分析模型. 数值仿真分析了叠栅中两个子光栅周期间隔、光栅长度、耦合系数等光栅参数对模式转换器的影响. 仿真分析和实验结果表明, 通过改变两个子光栅的周期间隔来改变两个损耗峰的位置, 形成一个损耗峰, 从而可以实现宽带宽的模式转换器, 其10 dB带宽约是单栅的2倍. 与传统的模式转换器相比, 该转换器带宽宽、转换效率高, 尺寸小、抗干扰能力强, 可以在模分复用系统和光通信中得到广泛的应用.

关键词:

Abstract

Mode-division multiplexing (MDM), as one of the promising techniques for overcoming current limitation of transmission capacity in single-mode fibers (SMFs), has attracted considerable attention. A key component in the MDM system is a mode converter, which makes conversion between the fundamental mode and the higher-order mode. Many mode converters have been demonstrated, such as spatial light modulators, phase plates, silicon-based asymmetrical directional couplers, fiber-based photonic lantern, and long period fiber grating (LPFG). Compared with other methods, mode converter used LPFG is a very feasible technique, which has the advantages of small size, low loss, low backward noise, high coupling efficiency and easy fabrication. However, the limitation of the mode converter is relatively narrow bandwidth. In this paper, a novel broadband all-fiber mode converter is proposed, in which two long period fiber gratings (LPFGs) with different periods are fabricated in the same spatial domain of few-mode fiber to achieve coupling from LP₀₁ to LP₁₁, thus forming superimposed long period fiber gratings (SLPFGs). The influences of grating parameters, such as the interval between two periods, the length of grating and the coupling coefficient on the mode converter, are analyzed by numerical simulation. It is found that the gap between the two resonant wavelengths becomes smaller with the periodic interval decreasing, which can form one rejection band when the gap is small enough, thus a broadband mode converter can be realized. The corresponding bandwidth at a conversion efficiency of 10 dB is about twice that of traditional LPFG. Moreover, with the increase of grating length, the conversion efficiency first increases and then decreases, because coupling efficiency experiences deficient coupling, full coupling and over coupling. The effect of coupling coefficient on converter is similar to that of grating length. According to the numerical results, grating I is fabricated with ${\varLambda _1} = 673\;{\text{μ}}{\rm m} $, 35-period. After that, the platform is rotated 180° and grating II is fabricated with ${\varLambda _2} = 688\; {\text{μ}}{\rm m}$, 35-period by CO₂ laser in tow mode fiber (TMF steped-index fiber). The bandwidths of both LPFGs at a conversion efficiency of 10 dB are about 57 nm and 67 nm respectively, while the bandwidth of SLPFG is about 153 nm. The experimental results are in pretty good agreement with the theoretical analyses. In addition, the proposed superimposed structure can also be extended to the conversion of fundamental mode into other high-order core modes. By designing the period of two sub-gratings reasonably, a wide band rejection filter with arbitrary wavelength can be realized. Compared with the traditional mode converter, the converter has the advantages of broad bandwidth, high conversion efficiency and small size, which can be widely used in the mode division multiplexing system and optical communication.

Keywords:

superimposed long period fiber gratings /
few mode fiber /
mode converter /
coupling coefficient

作者及机构信息

1.
燕山大学信息科学与工程学院, 河北省特种光纤与光纤传感重点实验室, 秦皇岛　066004

2.
河北科技师范学院机电工程学院, 秦皇岛　066004

3.
河北建材职业技术学院机电工程系, 秦皇岛　066004

通信作者: 毕卫红, whbi@ysu.edu.cn

基金项目: 国家自然科学基金(批准号: 61475133, 61575170, 61605168)、河北省自然科学基金(批准号: F2016203392)、河北省应用基础研究计划重点基础研究项目(批准号: 16961701D)、河北省高等学校科学技术研究基金(批准号: QN2016078)和燕山大学校内博士基金(批准号: B1011)资助的课题.

Authors and contacts

1.
School of Information Science and Engineering, the Key Laboratory for Special Fiber and Fiber Sensor of Hebei Province, Yanshan University, Qinhuangdao 066004, China

2.
College of Mechanical and Electrical Engineering, Hebei Normal University of Science and Technology, Qinhuangdao 066004, China

3.
Department of Mechanical and Electrical Engineering, Hebei Construction Material Vocational and Technical College, Qinhuangdao 066004, China

Corresponding author: Bi Wei-Hong, whbi@ysu.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 61475133, 61575170, 61605168), the Natural Science Foundation of Hebei Province, China (Grant No. F2016203392), the Key Basic Research Program of Hebei Province, China (Grant No. 16961701D), College and University Science and Technology Research Project of Hebei Province, China (Grant No. QN2016078), and the Intramural Doctoral Foundation of Yanshan University, China (Grant No. B1011).

文章全文 : translate this paragraph

参考文献

[1]	Goebel B, Foschini G J, Kramer G, Essiambre R, Winzer P J, Essiambre R 2010 J. Lightwave Technol. 28 662 Google Scholar
[2]	Fernandes G M, Muga N J, Pinto A N 2017 Opt. Express 25 3899 Google Scholar
[3]	Zhao Y H, Liu Y Q, Zhang L, Zhang C Y, Wen J X, Wang T Y 2016 Opt. Express 24 6186 Google Scholar
[4]	Salsi M, Koebele C, Sperti D, Tran P, Mardoyan H, Brindel P, Bigo S, Boutin A, Verluise F, Sillard P, Astruc M, Provost L, Charlet G 2012 J. Lightwave Technol. 30 618 Google Scholar
[5]	Von H J, Ryf R, Winzer P 2013 Opt. Express 21 18097 Google Scholar
[6]	Ryf R, Randel S, Gnauck A H, Bolle C, Sierra A, Mumtaz S, Esmaeelpour M, Burrows C, Essiambre R J, Winzer P J, Peckham D W, McCurdy A H, Lingle R 2012 J. Lightwave Technol. 30 521 Google Scholar
[7]	Montero-Orille C, Moreno V, Prieto-Blanco X, Mateo E F, Ip E, Crespo J, Linares J 2013 Appl. Optics 52 2332 Google Scholar
[8]	Dong J, Chiang K S, Jin W 2015 Opt. Lett. 40 3125 Google Scholar
[9]	Dong J, Chiang K S, Jin W 2015 J. Lightwave Technol. 33 4580 Google Scholar
[10]	Sai X, Li Y, Yang C, Li W, Qiu J, Hong X, Zuo Y, Guo H, Tong W, Wu J 2017 Opt. Lett. 42 4355 Google Scholar
[11]	Leon-Saval S G, Fontaine N K, Salazar-Gil J R, Ercan B, Ryf R, Bland-Hawthorn J 2014 Opt. Express. 22 1036 Google Scholar
[12]	Ali M M, Jung Y, Lim K S, Islam M R, Alam S U, Richardson D J, Ahmad H 2015 IEEE Photonics Technol. Lett. 27 1713 Google Scholar
[13]	Savin S, Digonnet M J, Kino J S, Shaw H J 2000 Opt. Lett. 25 710 Google Scholar
[14]	Ramachandran S, Wang Z Y, Yan M 2002 Opt. Lett. 27 698 Google Scholar
[15]	Liao C R, Wang Y, Wang D N, Jin L 2010 IEEE Photonics Technol. Lett. 22 425 Google Scholar
[16]	Dong J L, Chiang K S 2015 IEEE Photonics Technol. Lett. 27 1006 Google Scholar
[17]	Wang B, Zhang W G, Bai Z Y, Wang L, Zhang L Y, Zhou Q, Chen L, Yan T Y 2015 IEEE Photonics Technol. Lett. 27 145 Google Scholar
[18]	Zhao Y H, Liu Y Q, Zhang C Y, Zhang L, Zheng G J, Mou C B, Wen J X, Wang T Y 2017 Opt. Lett. 42 4708 Google Scholar
[19]	Garg R, Thyagarajan K 2013 Opt. Fiber Technol. 19 148 Google Scholar
[20]	江鹏 2016 博士学位论文(秦皇岛: 燕山大学) Jiang P 2016 Ph.D. Dissertation (Qinhuangdao: Yanshan University) (in Chinese)

施引文献

图 1 模式转换器的结构图

Fig. 1. Schematic of mode converter.

下载: 全尺寸图片幻灯片

图 2 少模光纤的模式色散特性

Fig. 2. Effective refractive indices against wavelength of two modes.

下载: 全尺寸图片幻灯片

图 3 少模光纤光栅周期与谐振波长的关系

Fig. 3. Dependence of grating period and resonant wavelength in few mode fiber.

下载: 全尺寸图片幻灯片

图 4 单栅与叠栅光谱对比

Fig. 4. Spectrum contrast between LPFG and SLPFG.

下载: 全尺寸图片幻灯片

图 5 不同周期的叠栅仿真

Fig. 5. Simulated SLPFG for different periods.

下载: 全尺寸图片幻灯片

图 6 不同光栅长度的叠栅仿真

Fig. 6. Simulated SLPFG for different grating lengths.

下载: 全尺寸图片幻灯片

图 7 不同耦合系数的叠栅仿真

Fig. 7. Simulated SLPFG for different coupling coefficient.

下载: 全尺寸图片幻灯片

图 8 少模光纤叠栅的实验制备系统

Fig. 8. Experiment setup for TMF-SLPFG.

下载: 全尺寸图片幻灯片

图 9 单栅和叠栅的实验光谱图

Fig. 9. Experimental spectrum of LPFG and SLPFG.

下载: 全尺寸图片幻灯片

图 10 模场观测装置

Fig. 10. Schematic of mode profile observation.

下载: 全尺寸图片幻灯片

图 11 不同波长处的模场图　(a) 1400 nm; (b)1486 nm; (c) 1550 nm

Fig. 11. Images of mode profiles located at different wavelength: (a) 1400 nm; (b) 1486 nm; (c) 1550 nm.

下载: 全尺寸图片幻灯片

map

[1]	Goebel B, Foschini G J, Kramer G, Essiambre R, Winzer P J, Essiambre R 2010 J. Lightwave Technol. 28 662 Google Scholar
[2]	Fernandes G M, Muga N J, Pinto A N 2017 Opt. Express 25 3899 Google Scholar
[3]	Zhao Y H, Liu Y Q, Zhang L, Zhang C Y, Wen J X, Wang T Y 2016 Opt. Express 24 6186 Google Scholar
[4]	Salsi M, Koebele C, Sperti D, Tran P, Mardoyan H, Brindel P, Bigo S, Boutin A, Verluise F, Sillard P, Astruc M, Provost L, Charlet G 2012 J. Lightwave Technol. 30 618 Google Scholar
[5]	Von H J, Ryf R, Winzer P 2013 Opt. Express 21 18097 Google Scholar
[6]	Ryf R, Randel S, Gnauck A H, Bolle C, Sierra A, Mumtaz S, Esmaeelpour M, Burrows C, Essiambre R J, Winzer P J, Peckham D W, McCurdy A H, Lingle R 2012 J. Lightwave Technol. 30 521 Google Scholar
[7]	Montero-Orille C, Moreno V, Prieto-Blanco X, Mateo E F, Ip E, Crespo J, Linares J 2013 Appl. Optics 52 2332 Google Scholar
[8]	Dong J, Chiang K S, Jin W 2015 Opt. Lett. 40 3125 Google Scholar
[9]	Dong J, Chiang K S, Jin W 2015 J. Lightwave Technol. 33 4580 Google Scholar
[10]	Sai X, Li Y, Yang C, Li W, Qiu J, Hong X, Zuo Y, Guo H, Tong W, Wu J 2017 Opt. Lett. 42 4355 Google Scholar
[11]	Leon-Saval S G, Fontaine N K, Salazar-Gil J R, Ercan B, Ryf R, Bland-Hawthorn J 2014 Opt. Express. 22 1036 Google Scholar
[12]	Ali M M, Jung Y, Lim K S, Islam M R, Alam S U, Richardson D J, Ahmad H 2015 IEEE Photonics Technol. Lett. 27 1713 Google Scholar
[13]	Savin S, Digonnet M J, Kino J S, Shaw H J 2000 Opt. Lett. 25 710 Google Scholar
[14]	Ramachandran S, Wang Z Y, Yan M 2002 Opt. Lett. 27 698 Google Scholar
[15]	Liao C R, Wang Y, Wang D N, Jin L 2010 IEEE Photonics Technol. Lett. 22 425 Google Scholar
[16]	Dong J L, Chiang K S 2015 IEEE Photonics Technol. Lett. 27 1006 Google Scholar
[17]	Wang B, Zhang W G, Bai Z Y, Wang L, Zhang L Y, Zhou Q, Chen L, Yan T Y 2015 IEEE Photonics Technol. Lett. 27 145 Google Scholar
[18]	Zhao Y H, Liu Y Q, Zhang C Y, Zhang L, Zheng G J, Mou C B, Wen J X, Wang T Y 2017 Opt. Lett. 42 4708 Google Scholar
[19]	Garg R, Thyagarajan K 2013 Opt. Fiber Technol. 19 148 Google Scholar
[20]	江鹏 2016 博士学位论文(秦皇岛: 燕山大学) Jiang P 2016 Ph.D. Dissertation (Qinhuangdao: Yanshan University) (in Chinese)

[1]	惠战强, 刘瑞华, 高黎明, 韩冬冬, 李田甜, 巩稼民. 基于对称双环嵌套管的低损耗弱耦合六模空芯负曲率光纤. , 2024, 73(7): 070703. doi: 10.7498/aps.73.20231785
[2]	陆梦佳, 恽斌峰. 基于硅基砖砌型亚波长光栅的紧凑型模式转换器. , 2023, 72(16): 164203. doi: 10.7498/aps.72.20230673
[3]	王健, 吴重庆. 低差分模式群时延少模光纤的变分法分析及优化. , 2022, 71(9): 094206. doi: 10.7498/aps.71.20212198
[4]	王瑜浩, 武保剑, 郭飚, 文峰, 邱昆. 基于非线性光纤环形镜的少模脉冲幅度调制再生器. , 2020, 69(7): 074202. doi: 10.7498/aps.69.20191858
[5]	万峰, 武保剑, 曹亚敏, 王瑜浩, 文峰, 邱昆. 空频复用光纤中四波混频过程的解析分析方法. , 2019, 68(11): 114207. doi: 10.7498/aps.68.20182129
[6]	罗雪雪, 陶汝茂, 刘志巍, 史尘, 张汉伟, 王小林, 周朴, 许晓军. 少模光纤放大器中的准静态模式不稳定实验研究. , 2018, 67(14): 144203. doi: 10.7498/aps.67.20180140
[7]	张燕君, 高浩雷, 付兴虎, 田永胜. 少模光纤的不同模式布里渊散射特性. , 2017, 66(2): 024207. doi: 10.7498/aps.66.024207
[8]	郑兴娟, 任国斌, 黄琳, 郑鹤玲. 少模光纤的弯曲损耗研究. , 2016, 65(6): 064208. doi: 10.7498/aps.65.064208
[9]	姜珊珊, 刘艳, 邢尔军. 低差分模式时延少模光纤的有限元分析及设计. , 2015, 64(6): 064212. doi: 10.7498/aps.64.064212
[10]	肖亚玲, 刘艳格, 王志, 刘晓颀, 罗明明. 基于少模光纤的全光纤熔融模式选择耦合器的设计及实验研究. , 2015, 64(20): 204207. doi: 10.7498/aps.64.204207
[11]	王冬, 徐莎, 曹延伟, 秦奋. 光子晶体高功率微波模式转换器设计. , 2014, 63(1): 018401. doi: 10.7498/aps.63.018401
[12]	王强, 周海京, 杨春, 李彪, 何晓阳. 过模波导器件的迭代设计方法. , 2013, 62(11): 115204. doi: 10.7498/aps.62.115204
[13]	林桢, 郑斯文, 任国斌, 简水生. 七芯及十九芯大模场少模光纤的特性研究和比对分析. , 2013, 62(6): 064214. doi: 10.7498/aps.62.064214
[14]	姚殊畅, 付松年, 张敏明, 唐明, 沈平, 刘德明. 基于少模光纤的模分复用系统多输入多输出均衡与解调. , 2013, 62(14): 144215. doi: 10.7498/aps.62.144215
[15]	崔健, 罗积润, 朱敏, 郭炜. 休斯结构多间隙耦合腔的稳定性分析. , 2011, 60(6): 061101. doi: 10.7498/aps.60.061101
[16]	崔健, 罗积润, 朱敏, 郭炜. 多间隙耦合腔中注-波同步与耦合. , 2011, 60(5): 051101. doi: 10.7498/aps.60.051101
[17]	李鹏, 赵建林, 张晓娟, 侯建平. 三角结构三芯光子晶体光纤中的模式耦合特性分析. , 2010, 59(12): 8625-8631. doi: 10.7498/aps.59.8625
[18]	孙旭, 赵青, 李宏福. 宽带非均匀半径渐变TE0n-TE0(n+1)模式转换器的设计研究. , 2008, 57(4): 2130-2135. doi: 10.7498/aps.57.2130
[19]	钱郁, 宋宣玉, 时伟, 陈光旨, 薛郁. 可激发介质湍流的耦合同步及控制. , 2006, 55(9): 4420-4427. doi: 10.7498/aps.55.4420
[20]	王铁邦, 覃团发, 陈光旨. 超混沌系统的耦合同步. , 2001, 50(10): 1851-1855. doi: 10.7498/aps.50.1851

计量

文章访问数: 8900
PDF下载量: 133
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

基于少模长周期光纤叠栅的模式转换器