-
具有低晶格热导率和高热电优值的二维 (2D)材料可用于热电器件的制备. 本文通过第一性原理和玻尔兹曼输运理论, 系统地预测了单层Cu2X (X = S, Se)的热电性质. 研究发现单层Cu2Se较Cu2S在室温下具有更低的晶格热导率 (1.93 W/(m·K)和3.25 W/(m·K)), 这源于其更低的德拜温度和更强的非谐性. 单层Cu2X (X = S, Se)价带顶处的能带简并效应显著增大了其载流子有效质量, 导致p型掺杂下具有高的塞贝克系数和低的电导率. 在最优掺杂浓度下, 单层Cu2S (Cu2Se) n型的功率因数16.5 mW/(m·K2) (25.9 mW/(m·K2))远高于其p型的功率因数1.1 mW/(m·K2) (6.6 mW/(m·K2)), 且随着温度的提升这一优势将更加明显. 温度为700 K时, 单层Cu2S和Cu2Se在n型最优掺杂浓度下的热电优值可以达到1.85和2.82, 高于p型最优掺杂浓度下的热电优值0.38和1.7. 单层Cu2X (X = S, Se)的优良热电性能可与近期报道的许多先进的热电材料相媲美, 特别是单层Cu2Se的热电优值高于众所周知的先进热电材料—单层SnSe (2.32). 因此, 单层Cu2X (X = S, Se)是一类具有优异性能和良好应用前景的潜在热电材料. 这些结果为后续探索2D热电材料的实验及应用提供了理论依据, 并为深入了解声子热输运对热电优值的影响提供了新的见解.Two-dimensional (2D) materials with lower lattice thermal conductivities and high figures of merit are useful for applications in thermoelectric (TE) devices. In this work, the thermoelectric properties of monolayer Cu2S and Cu2Se are systematically studied through first-principles and Boltzmann transport theory. The dynamic stability of monolayer Cu2S and Cu2Se through elastic constants and phonon dispersions are verified. The results show that monolayer Cu2S and Cu2Se have small lattice constants, resulting in lower phonon vibration modes. Phonon transport calculations confirm that monolayer Cu2Se has lower lattice thermal conductivity (1.93 W/(m·K)) than Cu2S (3.25 W/(m·K)) at room temperature, which is due to its small Debye temperature and stronger anharmonicity. Moreover, the heavier atomic mass of Se atom effectively reduces the phonon frequency, resulting in an ultra narrow phonon band gap (0.08 THz) and a lower lattice thermal conductivity for monolayer Cu2Se. The band degeneracy effect at the valence band maximum (VBM) of monolayer Cu2S and Cu2Se significantly increase their carrier effective mass, resulting in higher Seebeck coefficients and lower conductivities under p-type doping. The electric transport calculation at room temperature shows that the conductivity of monolayer Cu2S (Cu2Se) under n-type doping about 1011 cm–2 is 2.8×104 S/m (4.5×104 S/m), obviously superior to its conductivity about 2.6×102 S/m (1.6×103 S/m) under p-type doping. At the optimum doping concentration for monolayer Cu2S (Cu2Se), the n-type power factor is 16.5 mW/(m·K2) (25.9 mW/(m·K2)), which is far higher than p-type doping 1.1 mW/m·K2 (6.6 mW/(m·K2)). Through the above results, the excellent figure of merit of monolayer Cu2S (Cu2Se) under optimal n-type doping at 700 K can approach to 1.85 (2.82), which is higher than 0.38 (1.7) under optimal p-type doping. The excellent thermoelectric properties of monolayer Cu2S (Cu2Se) are comparable to those of many promising thermoelectric materials reported recently. Especially, the figure of merit of monolayer Cu2Se is larger than that of the well-known high-efficient thermoelectric monolayer SnSe (2.32). Therefore, monolayer Cu2S and Cu2Se are potential thermoelectric materials with excellent performances and good application prospects. These results provide the theoretical basis for the follow-up experiments to explore the practical applications of 2D thermoelectric semiconductor materials and provide an in-depth insight into the effect of phonon thermal transport on improvement of TE transport properties.
-
Keywords:
- first-principles /
- conductivity /
- thermal conductivity /
- thermoelectric
[1] Yang J, Stabler F R 2009 J. Electron. Mater. 38 1245
Google Scholar
[2] Sootsman J, Chung D Y, Kanatzidis M 2009 Angew. Chem. 48 8616
Google Scholar
[3] Rowe D M 1986 Appl. Energy 24 139
Google Scholar
[4] Sales B 2002 Science 295 1248
Google Scholar
[5] Zhang X, Zhao L D 2015 J. Materiomics 1 92
Google Scholar
[6] Naghavi S S, He J, Xia Y, Wolverton C 2018 Chem. Mater. 30 5639
Google Scholar
[7] Sajjad M, Singh N, Sattar S, Wolf S D, Schwingenschlögl U 2019 ACS Appl. Energy Mater. 2 3004
Google Scholar
[8] Huang H H, Xing G, Fan X, Singh D J, Zheng W T 2019 J. Mater. Chem. C 7 5094
Google Scholar
[9] Wang Y, Gao Z, Zhou J 2019 E Low dimens. Syst. Nanostruct. 108 53
Google Scholar
[10] Xu B, Xia Q, Zhang J, Ma S, Wang Y, Xu Q, Li J, Wang Y 2020 Comput. Mater. Sci. 177 109588
Google Scholar
[11] Shafique A, Samad A, Shin Y H 2017 Phys. Chem. Chem. Phys. 19 20677
Google Scholar
[12] Liu X, Zhang D, Wang H, Chen Y, Wang H, Ni Y 2021 Phys. Chem. Chem. Phys. 23 24039
Google Scholar
[13] Heremans J P, Jovovic V, Toberer E S, Saramat A, Kurosaki K, Charoenphakdee A, Yamanaka S, Snyder G J 2008 Science 321 554
Google Scholar
[14] Tan X, Shao H, Hu T, Liu G Q, Ren S F 2015 J. Phys. Condens. Matter 27 095501
Google Scholar
[15] Pei Y, Wang H, Snyder G J. 2012 Adv. Mater. 24 6125
Google Scholar
[16] Reshak A H, Khan S A 2014 J. Magn. Magn. Mater. 354 216
Google Scholar
[17] Pei Y Z, Shi X Y, Lalonde A, Wang H, Chen L D, Snyder G J 2011 Nature 473 66
Google Scholar
[18] Yu J, Li T, Nie G, Zhang B P, Sun Q 2019 Nanoscale 11 10306
Google Scholar
[19] Liu W, Shi X, Hong M, Yang L, Moshwan R, Chen Z G, Zou J 2018 J. Mater. Chem. C 6 13225
Google Scholar
[20] Kresse G, Furthmüller J 1996 Comput. Mater. Sci. 6 15
Google Scholar
[21] Wang V, Xu N, Liu J C, Tang G, Geng W T 2021 Comput. Phys. Commun. 267 108033
Google Scholar
[22] Kresse G, Joubert D 1999 Phys. Rev. B 59 1758
[23] Heyd J, Scuseria G E, Ernzerhof M 2006 J. Chem. Phys. 124 8207
[24] Madsen G K H, Carrete J, Verstraete M J 2018 Comput. Phys. Commun. 231 140
Google Scholar
[25] Togo A, Oba F, Tanaka I 2008 Phys. Rev. B 78 1341
[26] Li W, Carrete J, Katcho N A, Mingo N 2014 Comput. Phys. Commun. 185 1747
Google Scholar
[27] Li W, Mingo N, Lindsay L, Broido D A, Stewart D A, Katcho N A 2012 Phys. Rev. B 85 195436
Google Scholar
[28] Chen X, Wang D, Liu X, Li L, Sanyal B 2020 J. Phys. Chem. Lett. 11 2925
Google Scholar
[29] Born M, Huang K 1955 Am. J. Phys. 23 474
[30] Zhang F, Zhu B, Guo H, Qiu J, Zheng K, Chen X, Yu J 2021 Appl. Surf. Sci. 550 149230
Google Scholar
[31] Gao Z, Tao F, Ren J 2018 Nanoscale 10 12997
Google Scholar
[32] Zhu X L, Zhang J R, Zhou P, Xie W X, Wang G F, Tian B 2019 Nanoscale 11 19923
Google Scholar
[33] Guo S D, Wang Y H 2017 J. Appl. Phys. 121 034302
Google Scholar
[34] Peng B, Zhang H, Shao H, Xu Y, Ni G, Zhang R, Zhu H 2016 Phys. Rev. B 94 245420
Google Scholar
[35] Zhang W, Zhang X Q, Liu L, Wang Z, Li Z G 2021 Chin. Phys. B 30 526
[36] Ziman J M 1963 International Series of Monographs on Physics (Oxford: Clarendon) p168
[37] Carrete J, Li W, Lindsay L, Broido D A, Gallego L J, Mingo N 2016 Mater. Res. Lett. 4 204
Google Scholar
[38] Slack G A 1973 J. Phys. Chem. Solids 34 321
Google Scholar
[39] Broido D A, Ward A, Mingo N 2005 Phys. Rev. B 72 014308
Google Scholar
[40] Lv B, Hu X, Liu X, Zhang Z, Song J, Luo Z 2020 Phys. Chem. Chem. Phys. 22 17833
Google Scholar
[41] Morelli D T, Heremans J P 2002 Appl. Phys. Lett. 81 5126
Google Scholar
[42] Bolen E, Deligoz E, Ozisik H 2021 Solid State Commun. 327 114223
Google Scholar
[43] Mohanta M K, Sarkar A D 2020 ACS Appl. Mater. 12 18123
Google Scholar
[44] Peng B, Zhang H, Shao H, Xu K, Ni G, Li J, Zhu H, Soukoulis C M 2018 J. Mater. Chem. A 6 2018
Google Scholar
[45] 王宁 2022 博士论文 (成都: 电子科技大学)
Wang N 2022 Ph. D Dissertation (Chengdu: University of Electronic Science and Technology of China) (in Chinese)
[46] Qiu P, Agne M T, Liu Y, Zhu Y, Chen H, Mao T, Yang J, Zhang W, Haile S M, Zeier W G, Janek J, Uher C, Shi X, Chen L, Snyder G J 2018 Nat. Commun. 9 2910
Google Scholar
[47] Brown D R, Day T, Caillat T, Snyder G J 2013 J. Electron. Mater. 42 2014
Google Scholar
[48] Miyatani S Y, Suzuki Y 1953 J. Phys. Soc. Jpn. 8 680
Google Scholar
[49] Maassen J, Lundstrom M 2013 Appl. Phys. Lett. 102 093103
Google Scholar
[50] Sun Z H, Yuan K P, Chang Z, Bi S P, Zhang X L, Tang D W 2020 Nanoscale 12 3330
Google Scholar
-
图 1 单层Cu原子和X (X = S, Se)原子晶体结构的俯视图(a)和侧视图(b), 蓝色大球体表示Cu原子, 黄色小球代表X (X = S, Se)原子
Fig. 1. Top (a) and side (b) views of the crystal structure of monolayer Cu atoms and X (X = S, Se) atoms. Cu atoms are represented as blue large spheres and X (X = S, Se) atoms are represented as yellow small spheres.
图 2 单层Cu2S (a)和Cu2Se (b)的能带及分波态密度
Fig. 2. The electronic band structures and projected density of states of monolayer Cu2S (a) and Cu2Se (b).
图 3 单层Cu2S (a)和Cu2Se (b)的声子谱和态密度
Fig. 3. Phonon dispersions and phonon density of states of the monolayer Cu2S (a) and Cu2Se (b).
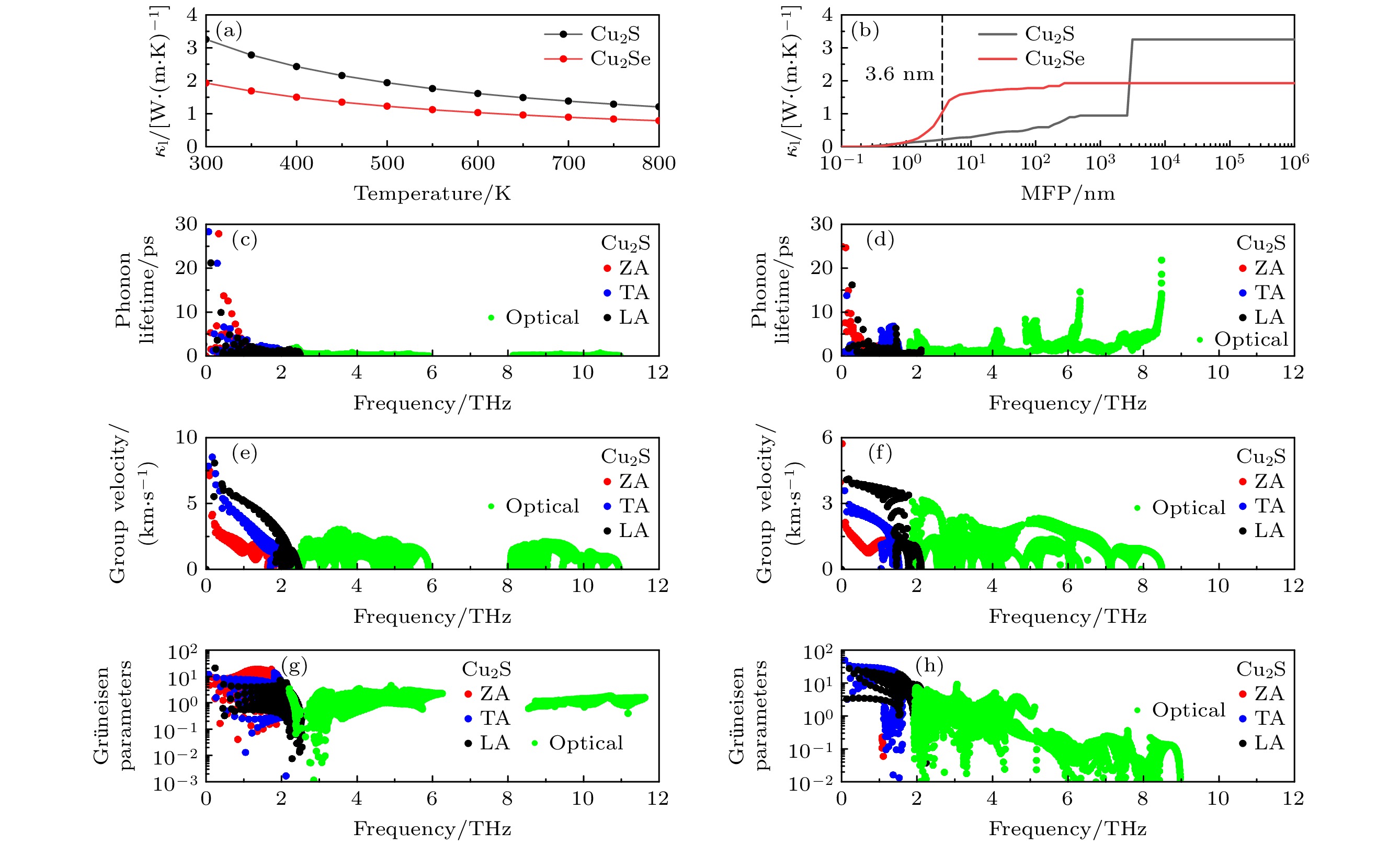
图 4 (a) 单层Cu2X (X =S, Se)的晶格热导率随温度变化趋势; (b) 300 K下单层Cu2S和Cu2Se的晶格热导率随平均自由程分布; (c), (d)声子寿命随频率分布, (e), (f) 声子群速度随频率分布; (g), (h) Grüneisen常数随频率分布
Fig. 4. (a) Lattice thermal conductivity as a function of the temperature; (b) lattice thermal conductivity of monolayer Cu2X (X = S, Se) as a function of the MFP at 300 K; (c), (d) phonon lifetime as a function of the phonon frequency; (e), (f) phonon group velocity as a function of the phonon frequency; (g), (h) the Grüneisen parameter as a function of the phonon frequency.
图 5 单层Cu2X (X = S, Se)的塞贝克系数((a), (b))、电导率((c), (d))、功率因数((e), (f))、热电优值((g), (h))与p型和n型掺杂下的载流子浓度的关系
Fig. 5. Seebeck coefficient ((a), (b)), conductivity ((c), (d)), power factor ((e), (f)), ZT ((g), (h)) as a function of carrier concentration for p-type and n-type doping of monolayer Cu2X (X = S, Se).
表 1 晶格常数α、Cu—X (X = S, Se) 键长l、Cu—X (X = S, Se)—Cu键角 θ1、Cu—X (X = S , Se)—Cu扭转角θ2、弹性张量C11, C12和C66、带隙EPBE, EHSE06由PBE和HSE06泛函计算实现
Table 1. Lattice parameters α, Cu—X (X = S, Se) bond lengths l, Cu—X (X = S, Se)—Cu bond angle θ1 , Cu—X (X = S, Se)—Cu twist angle θ2, elastic tensor C11, C12 and C66, electron bandgap EPBE, EHSE06 computed by PBE and HSE06.
α/Å l/Å θ1/(°) θ2/(°) C11 (C22)/(N·m–1) C12/(N·m–1) C66/(N·m–1) EPBE/eV EHSE06/eV Cu2S 5.02 2.22 70.78 21.38 34.7 2.3 19.6 0.23 1.15 Cu2Se 4.99 2.36 66.16 22.08 37.2 7.8 17.5 0.16 1.05 
下载: 导出CSV
表 2 室温下部分2D材料的平均声学支的德拜温度
$ {\varTheta }_{{\rm{D}}} $ 和晶格热导率κlTable 2. The Debye temperature
$ {\varTheta }_{{\rm{D}}} $ and lattice thermal conductivity$ {\kappa }_{{\rm{l}}} $ of following 2D materials at room temperature.
下载: 导出CSV
表 3 单层Cu2X (X = S, Se)的弹性模量 C2D、载流子有效质量
$ {m}^{*} $ ($ {m}_{0} $ ), 形变势常数$ {E}_{{\rm{l}}} $ , 载流子迁移率 μ和弛豫时间 τTable 3. Elastic modulus C2D, effective mass
$ {m}^{*} $ ($ {m}_{0} $ ), deformation potential constant$ {E}_{{\rm{l}}} $ , carrier mobility μ and relaxation time τ of monolayer Cu2X (X = S, Se).Type C2D/( N·m–1) $ {m}^{*} $ ${E}_{{\rm{l}}}$/eV μ/(cm2·(V·s)–1) τ/(10–14 s) Cu2S h 34.7 8.0 1.68 4.1 1.9 e 0.15 2.1 7.4×103 63.1 Cu2Se h 37.2 6.5 0.8 29.3 10.8 e 0.2 1.56 8.1×103 92.1
下载: 导出CSV
-
[1] Yang J, Stabler F R 2009 J. Electron. Mater. 38 1245
Google Scholar
[2] Sootsman J, Chung D Y, Kanatzidis M 2009 Angew. Chem. 48 8616
Google Scholar
[3] Rowe D M 1986 Appl. Energy 24 139
Google Scholar
[4] Sales B 2002 Science 295 1248
Google Scholar
[5] Zhang X, Zhao L D 2015 J. Materiomics 1 92
Google Scholar
[6] Naghavi S S, He J, Xia Y, Wolverton C 2018 Chem. Mater. 30 5639
Google Scholar
[7] Sajjad M, Singh N, Sattar S, Wolf S D, Schwingenschlögl U 2019 ACS Appl. Energy Mater. 2 3004
Google Scholar
[8] Huang H H, Xing G, Fan X, Singh D J, Zheng W T 2019 J. Mater. Chem. C 7 5094
Google Scholar
[9] Wang Y, Gao Z, Zhou J 2019 E Low dimens. Syst. Nanostruct. 108 53
Google Scholar
[10] Xu B, Xia Q, Zhang J, Ma S, Wang Y, Xu Q, Li J, Wang Y 2020 Comput. Mater. Sci. 177 109588
Google Scholar
[11] Shafique A, Samad A, Shin Y H 2017 Phys. Chem. Chem. Phys. 19 20677
Google Scholar
[12] Liu X, Zhang D, Wang H, Chen Y, Wang H, Ni Y 2021 Phys. Chem. Chem. Phys. 23 24039
Google Scholar
[13] Heremans J P, Jovovic V, Toberer E S, Saramat A, Kurosaki K, Charoenphakdee A, Yamanaka S, Snyder G J 2008 Science 321 554
Google Scholar
[14] Tan X, Shao H, Hu T, Liu G Q, Ren S F 2015 J. Phys. Condens. Matter 27 095501
Google Scholar
[15] Pei Y, Wang H, Snyder G J. 2012 Adv. Mater. 24 6125
Google Scholar
[16] Reshak A H, Khan S A 2014 J. Magn. Magn. Mater. 354 216
Google Scholar
[17] Pei Y Z, Shi X Y, Lalonde A, Wang H, Chen L D, Snyder G J 2011 Nature 473 66
Google Scholar
[18] Yu J, Li T, Nie G, Zhang B P, Sun Q 2019 Nanoscale 11 10306
Google Scholar
[19] Liu W, Shi X, Hong M, Yang L, Moshwan R, Chen Z G, Zou J 2018 J. Mater. Chem. C 6 13225
Google Scholar
[20] Kresse G, Furthmüller J 1996 Comput. Mater. Sci. 6 15
Google Scholar
[21] Wang V, Xu N, Liu J C, Tang G, Geng W T 2021 Comput. Phys. Commun. 267 108033
Google Scholar
[22] Kresse G, Joubert D 1999 Phys. Rev. B 59 1758
[23] Heyd J, Scuseria G E, Ernzerhof M 2006 J. Chem. Phys. 124 8207
[24] Madsen G K H, Carrete J, Verstraete M J 2018 Comput. Phys. Commun. 231 140
Google Scholar
[25] Togo A, Oba F, Tanaka I 2008 Phys. Rev. B 78 1341
[26] Li W, Carrete J, Katcho N A, Mingo N 2014 Comput. Phys. Commun. 185 1747
Google Scholar
[27] Li W, Mingo N, Lindsay L, Broido D A, Stewart D A, Katcho N A 2012 Phys. Rev. B 85 195436
Google Scholar
[28] Chen X, Wang D, Liu X, Li L, Sanyal B 2020 J. Phys. Chem. Lett. 11 2925
Google Scholar
[29] Born M, Huang K 1955 Am. J. Phys. 23 474
[30] Zhang F, Zhu B, Guo H, Qiu J, Zheng K, Chen X, Yu J 2021 Appl. Surf. Sci. 550 149230
Google Scholar
[31] Gao Z, Tao F, Ren J 2018 Nanoscale 10 12997
Google Scholar
[32] Zhu X L, Zhang J R, Zhou P, Xie W X, Wang G F, Tian B 2019 Nanoscale 11 19923
Google Scholar
[33] Guo S D, Wang Y H 2017 J. Appl. Phys. 121 034302
Google Scholar
[34] Peng B, Zhang H, Shao H, Xu Y, Ni G, Zhang R, Zhu H 2016 Phys. Rev. B 94 245420
Google Scholar
[35] Zhang W, Zhang X Q, Liu L, Wang Z, Li Z G 2021 Chin. Phys. B 30 526
[36] Ziman J M 1963 International Series of Monographs on Physics (Oxford: Clarendon) p168
[37] Carrete J, Li W, Lindsay L, Broido D A, Gallego L J, Mingo N 2016 Mater. Res. Lett. 4 204
Google Scholar
[38] Slack G A 1973 J. Phys. Chem. Solids 34 321
Google Scholar
[39] Broido D A, Ward A, Mingo N 2005 Phys. Rev. B 72 014308
Google Scholar
[40] Lv B, Hu X, Liu X, Zhang Z, Song J, Luo Z 2020 Phys. Chem. Chem. Phys. 22 17833
Google Scholar
[41] Morelli D T, Heremans J P 2002 Appl. Phys. Lett. 81 5126
Google Scholar
[42] Bolen E, Deligoz E, Ozisik H 2021 Solid State Commun. 327 114223
Google Scholar
[43] Mohanta M K, Sarkar A D 2020 ACS Appl. Mater. 12 18123
Google Scholar
[44] Peng B, Zhang H, Shao H, Xu K, Ni G, Li J, Zhu H, Soukoulis C M 2018 J. Mater. Chem. A 6 2018
Google Scholar
[45] 王宁 2022 博士论文 (成都: 电子科技大学)
Wang N 2022 Ph. D Dissertation (Chengdu: University of Electronic Science and Technology of China) (in Chinese)
[46] Qiu P, Agne M T, Liu Y, Zhu Y, Chen H, Mao T, Yang J, Zhang W, Haile S M, Zeier W G, Janek J, Uher C, Shi X, Chen L, Snyder G J 2018 Nat. Commun. 9 2910
Google Scholar
[47] Brown D R, Day T, Caillat T, Snyder G J 2013 J. Electron. Mater. 42 2014
Google Scholar
[48] Miyatani S Y, Suzuki Y 1953 J. Phys. Soc. Jpn. 8 680
Google Scholar
[49] Maassen J, Lundstrom M 2013 Appl. Phys. Lett. 102 093103
Google Scholar
[50] Sun Z H, Yuan K P, Chang Z, Bi S P, Zhang X L, Tang D W 2020 Nanoscale 12 3330
Google Scholar
下载:
计量
- 文章访问数: 3768
- PDF下载量: 103
- 被引次数: 0
