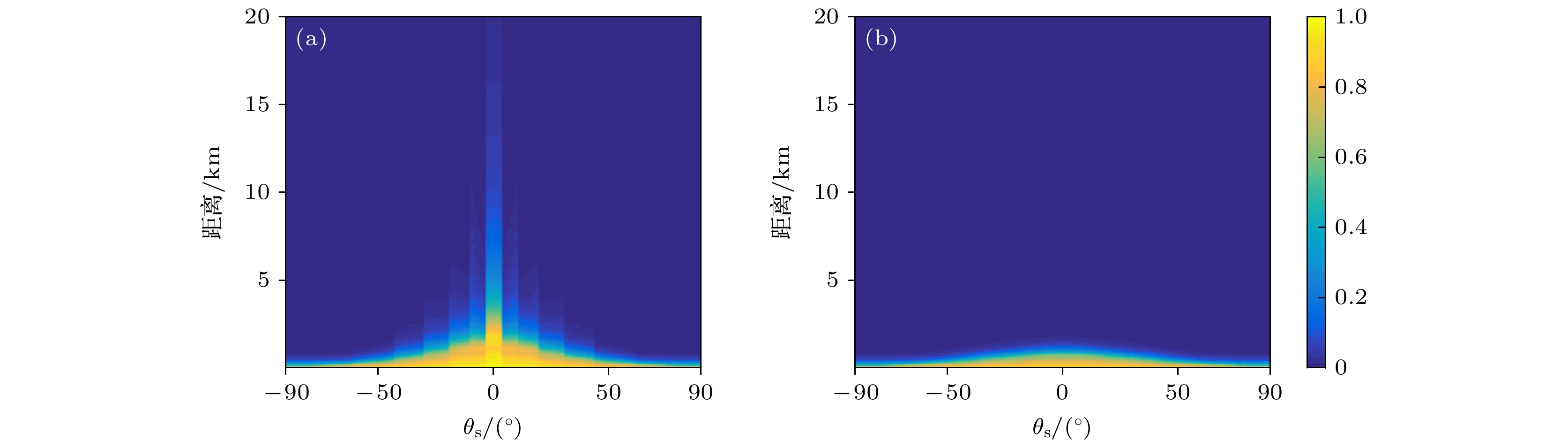
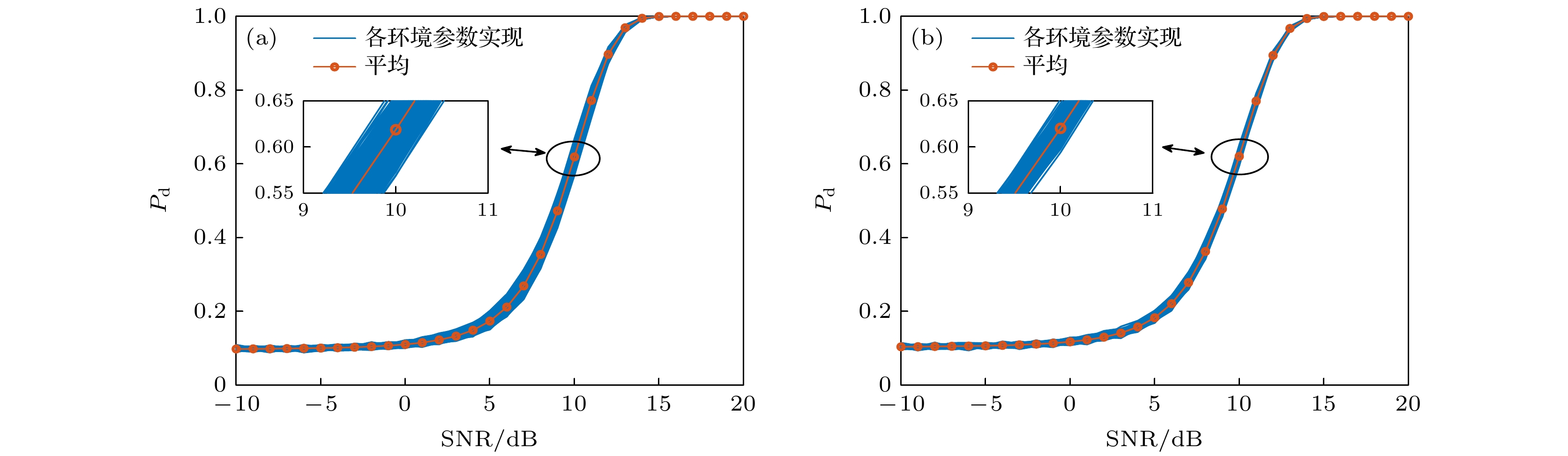
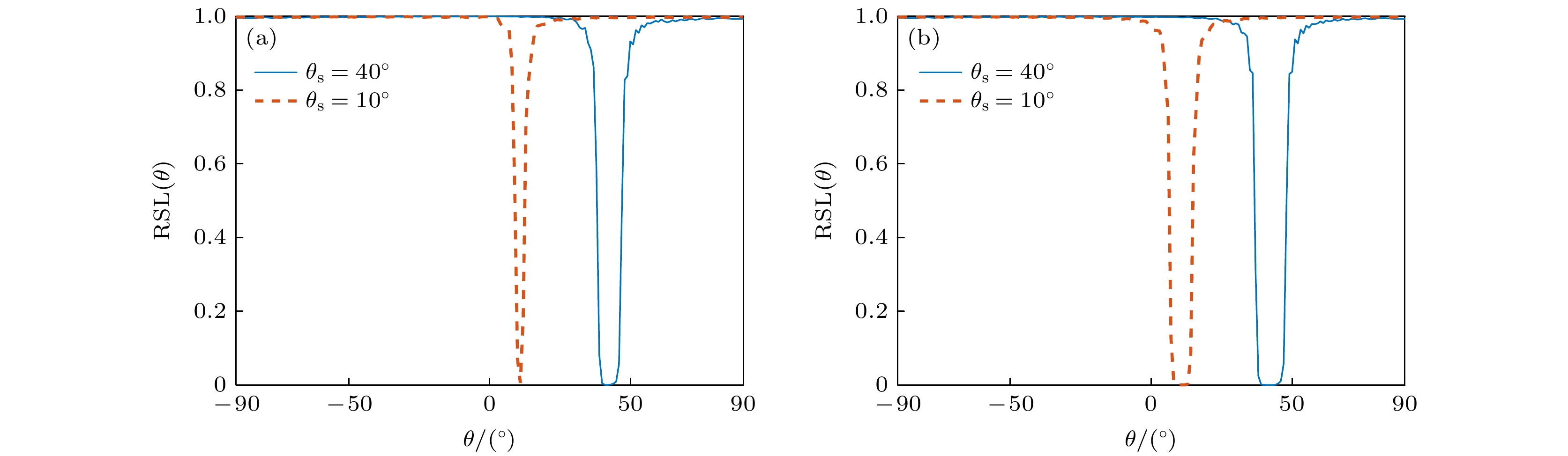
-
在复杂浅海波导中, 环境参数的不确定性影响检测的稳健性, 故稳健检测是浅海检测中的重要问题. 本文结合简正波模型, 定义和估计了不确定环境中的水平阵角度域子空间, 提出了水平阵角度域子空间检测器及其稳健形式. 角度域子空间利用了不同环境参数条件下的水平阵远场观测模型, 包含了不确定环境参数信息, 估计过程中利用了硬海底条件下传播模态的水平波数与水体、沉积层声速之间的关系, 具有较少的先验信息要求和较低的实现复杂度. 在此基础上提出的水平阵角度域子空间检测器实现简单, 在不确定环境中具有一定的稳健性, 但其检测性能随目标方位角起伏. 将角度域子空间变换到维数恒定的子空间中, 得到角度域子空间检测器的稳健形式, 即稳健水平阵角度域子空间检测器, 该检测器在不确定环境中具有一定的稳健性, 同时检测性能在各目标方位上一致. 不确定浅海环境中的仿真结果表明, 稳健水平阵角度域子空间检测器具有和能量检测器近似的稳健性, 同时提高了检测能力.The uncertainties of environmental parameters affect the robustness of detection method in complex shallow water. We define and estimate the angle-domain subspaces of horizontal linear array in a proper way under an uncertain environment. According to the angle-domain subspaces, we propose an angle-domain subspace detector and its robust form. Angle-domain subspaces contain an uncertain information by using the observation matrices in different environmental parameters. The relationship between the horizontal wave number of propagating modes and the sound speed of the bottom and sediment in hard seabed is used to estimate the angle-domain subspaces. The proposed angle-domain subspace detector is robust in an uncertain environment, but its detection performance fluctuates with target bearings. The angle domain subspace is transformed into a constant dimension subspace, robust form of the angular domain subspace detector, whose detection performance is consistent in all source bearings. The simulation results in the uncertain shallow-water environment show that the robust angle domain subspace detector has a similar robustness to the energy detector, and better detection capability.
-
Keywords:
- shallow water /
- uncertain environment /
- detection /
- horizontal array
[1] Porter M B 1994 J. Acoust. Soc. Am. 2 161
Google Scholar
[2] Li J, Stoica P, Wang Z 2004 IEEE Trans. Signal Process. 52 2407
Google Scholar
[3] 易锋, 孙超, 白晓慧 2013 中国科学: 物理学 力学 天文学 43 174
Google Scholar
Yi F, Sun C, Bai X H 2013 Sci. Sin.-Phys. Mech. Astron. 43 174
Google Scholar
[4] Jensen F B, Kuperman W A, Porter M B 2011 Computational Ocean Acoustics (New York: Springer Science and Business Media) pp337–360
[5] 张同伟, 杨坤德, 马远良, 黎雪刚 2010 59 3294
Google Scholar
Zhang T W, Yang K D, Ma Y L, Li X G 2010 Acta Phys. Sin. 59 3294
Google Scholar
[6] Conan E, Bonnel J, Nicalas B 2017 J. Acoust. Soc. Am. 142 2276
Google Scholar
[7] Sha L, Nolte L W 2005 J. Acoust. Soc. Am. 117 1942
Google Scholar
[8] 刘宗伟, 孙超, 吕连港 2015 声学学报 40 5949
Google Scholar
Liu Z W, Sun C, Lv L G 2015 Acta Acustica 40 5949
Google Scholar
[9] 李明杨, 孙超, 邵炫 2014 63 204302
Google Scholar
Li M Y, Sun C, Shao X 2014 Acta Phys. Sin. 63 204302
Google Scholar
[10] Li M, Sun C, Willett P 2018 IEEE J. Ocean Eng. 43 131
Google Scholar
[11] 李明杨 2019 博士学位论文 (西安: 西北工业大学)
Li M Y 2019 Ph. D. Dissertation (Xi’an: Northwestern Ploytechnical University) (in Chinese)
[12] 孔德智, 孙超, 李明杨 2020 69 164301
Google Scholar
Kong D Z, Sun C, Li M Y 2020 Acta Phys. Sin. 69 164301
Google Scholar
[13] 王宣, 孙超, 李明杨 2020 西北工业大学学报 38 1171
Google Scholar
Wang X, Sun C, Li M Y 2020 J. Northwestern Ploytechnical University 38 1171
Google Scholar
[14] Hari V N, Anand G V, Permkumar A B 2013 Digital Signal Process. 23 1645
Google Scholar
[15] 孔德智, 孙超, 李明杨, 卓颉, 刘雄厚 2019 68 174301
Google Scholar
Kong D Z, Sun C, Li M Y, Zhuo J, Liu X H 2019 Acta Phys. Sin. 68 174301
Google Scholar
-
图 1 目标声源与HLA空间位置关系
Fig. 1. Geometric relationship between target sound source and HLA space.
图 3 1000个环境参数实现下传播模态的水平波数 (a)传播模态阶数; (b)水平波数
Fig. 3. Horizontal wave numbers of propagating modes in 1000 environmental realizations: (a) Numbers of propagating modes; (b) wave numbers
图 4 不同水平方位角对应的角度域子空间维数
Fig. 4. Subspace dimensions of angle-domain corresponding to different horizontal azimuth angles
图 5 角度域子空间在不同空间位置处的有效性 (a)
$ \hat{W}(\theta_{\rm{s}}) $ ; (b)$ \hat{W}_{\mathrm{R}}(\theta_{\rm{s}}) $ Fig. 5. Validity of angle-domain subspaces at different spatial positions: (a)
$ \hat{W}(\theta_{\rm{s}}) $ ; (b)$ \hat{W}_{\mathrm{R}}(\theta_{\rm{s}}) $ 
图 6 两个环境实现下的水平波数和HLA接收到的归一化行波幅度 (a)水平波数; (b)归一化行波幅度
Fig. 6. The horizontal wave numbers and the amplitude of the normalized traveling wave received by HLA in two realizations: (a) Horizontal wave numbers; (b) normalized traveling wave received
图 7 两个环境实现下的
$ \mathrm{RSL}(\theta) $ 曲线 (a)$ \hat{W}(\theta) $ ; (b)$ \hat{W}_{\mathrm{R}}(\theta) $ Fig. 7.
$ \mathrm{RSL}(\theta) $ in two realizations: (a)$ \hat{W}(\theta) $ ; (b)$ \hat{W}_{\mathrm{R}}(\theta) $ 
图 8 两个环境参数实现下HASD和RHASD的检测性能 (a) HASD; (b) RHASD
Fig. 8. Detection performances of HASD and RHASD in two realizations: (a) HASD; (b) RHASD
图 9 1000个环境参数实现下HASD和RHASD的检测性能 (a) HASD; (b) RHASD
Fig. 9. Detection performances of HASD and RHASD in 1000 realizations: (a) HASD; (b) RHASD
图 10 目标分别位于
$ 40^\circ $ 和$ 10^\circ $ 的$ \mathrm{RSL}(\theta) $ 曲线 (a)$ \hat{W}(\theta) $ ; (b)$ \hat{W}_{\mathrm{R}}(\theta) $ Fig. 10.
$ \mathrm{RSL}(\theta) $ when the source bearing are at$ 40^\circ $ and$ 10^\circ $ : (a)$ \hat{W}(\theta) $ ; (b)$ \hat{W}_{\mathrm{R}}(\theta) $ 
图 11 目标分别位于
$ 40^\circ $ 和$ 10^\circ $ 时HASD和RHASD的检测性能 (a) HASD; (b) RHASDFig. 11. Detection performances of HASD and RHASD when the source bearing are at
$ 40^\circ $ and$ 10^\circ $ : (a) HASD; (b) RHASD
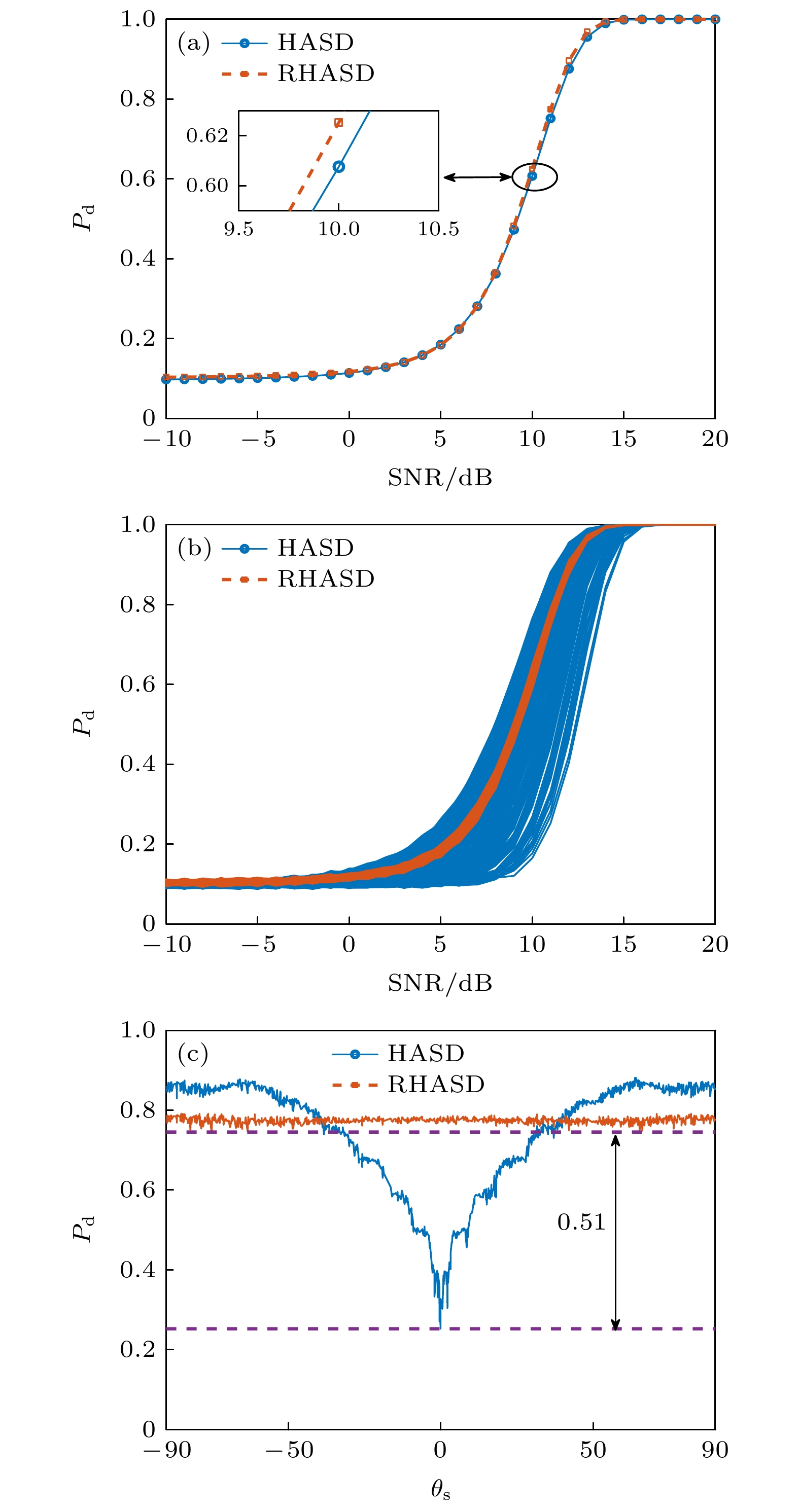
图 12 不同目标位置和环境参数条件下HASD和RHASD的检测性能 (a)平均检测性能; (b)各参数条件下检测性能; (c)检测概率随目标方位起伏
Fig. 12. Detection performances of HASD and RHASD in different environments and source positions: (a) Average detection performance; (b) detection performance in different realizations; (c) detection probability fluctuates with the target bearing
图 13 RHASD与MC-GLRD, SD, ED性能对比 (a)平均检测性能; (b)不同实现下的检测概率
Fig. 13. Detection performances of HASD, MC-GLRD, SD, ED: (a) Average detection performance; (b) detection probability in different realizations
表 1 浅海标准失配测试模型中参数意义及取值范围
Table 1. The value range of standard mismatch test model
环境参数/单位 符号 取值范围 海水深度/m $ D_1 $ $ 100\pm 2.5 $ 海面声速 /($ \rm{m\cdot s^{-1}} $) $ c_{\rm{s}} $ $ 1500\pm 2.5 $ 海底声速/($ \rm{m\cdot s^{-1}} $) $ c_{\rm{b}} $ $ 1480\pm 2.5 $ 沉积层声速/($ \rm{m\cdot s^{-1}} $) $ c_{\rm{d}} $ $ 1600\pm 50 $ 基底声速/($ \rm{m\cdot s^{-1}} $) $ c_{\rm{h}} $ $ 1750\pm 100 $ 底质密度/($ \rm{g\cdot cm^{-3}} $) $ \rho $ $ 1.7\pm 0.25 $ 底质吸收系数/($ \rm{dB\cdot }\lambda^{-1} $) $ \alpha $ $ 0.35\pm 0.25 $ 沉积层厚度/$ \rm{m} $ $ D_2 $ $ 100 $ 
下载: 导出CSV
表 2 不确定浅海波导中的两个环境实现
Table 2. Two realizations of the uncertain shallow waveguide.
环境参数 $ D_1 $/m $c_{\rm{s} } /({\rm{m}}·{\rm{s}}^{-1})$ $c_{\rm{b} } /({\rm{m} }·{\rm{s} }^{-1})$ $c_{\rm{d} } /({\rm{m}}·{\rm{s}}^{-1})$ $c_{\rm{h} } /({\rm{m}}·{\rm{s}}^{-1})$ $ \rho $/(kg·$ \mathrm{m}^{-3} $) $ \alpha $/(dB·$ \lambda^{-1} $) $ D_2 $/m 环境1 $ 98.06 $ $ 1501.33 $ $ 1478.21 $ $ 1552.63 $ $ 1716.98 $ $ 1.59 $ $ 0.25 $ $ 100 $ 环境2 $ 102.36 $ $ 1500.58 $ $ 1480.05 $ $ 1633.20 $ $ 1791.55 $ $ 1.93 $ $ 0.36 $ $ 100 $
下载: 导出CSV
-
[1] Porter M B 1994 J. Acoust. Soc. Am. 2 161
Google Scholar
[2] Li J, Stoica P, Wang Z 2004 IEEE Trans. Signal Process. 52 2407
Google Scholar
[3] 易锋, 孙超, 白晓慧 2013 中国科学: 物理学 力学 天文学 43 174
Google Scholar
Yi F, Sun C, Bai X H 2013 Sci. Sin.-Phys. Mech. Astron. 43 174
Google Scholar
[4] Jensen F B, Kuperman W A, Porter M B 2011 Computational Ocean Acoustics (New York: Springer Science and Business Media) pp337–360
[5] 张同伟, 杨坤德, 马远良, 黎雪刚 2010 59 3294
Google Scholar
Zhang T W, Yang K D, Ma Y L, Li X G 2010 Acta Phys. Sin. 59 3294
Google Scholar
[6] Conan E, Bonnel J, Nicalas B 2017 J. Acoust. Soc. Am. 142 2276
Google Scholar
[7] Sha L, Nolte L W 2005 J. Acoust. Soc. Am. 117 1942
Google Scholar
[8] 刘宗伟, 孙超, 吕连港 2015 声学学报 40 5949
Google Scholar
Liu Z W, Sun C, Lv L G 2015 Acta Acustica 40 5949
Google Scholar
[9] 李明杨, 孙超, 邵炫 2014 63 204302
Google Scholar
Li M Y, Sun C, Shao X 2014 Acta Phys. Sin. 63 204302
Google Scholar
[10] Li M, Sun C, Willett P 2018 IEEE J. Ocean Eng. 43 131
Google Scholar
[11] 李明杨 2019 博士学位论文 (西安: 西北工业大学)
Li M Y 2019 Ph. D. Dissertation (Xi’an: Northwestern Ploytechnical University) (in Chinese)
[12] 孔德智, 孙超, 李明杨 2020 69 164301
Google Scholar
Kong D Z, Sun C, Li M Y 2020 Acta Phys. Sin. 69 164301
Google Scholar
[13] 王宣, 孙超, 李明杨 2020 西北工业大学学报 38 1171
Google Scholar
Wang X, Sun C, Li M Y 2020 J. Northwestern Ploytechnical University 38 1171
Google Scholar
[14] Hari V N, Anand G V, Permkumar A B 2013 Digital Signal Process. 23 1645
Google Scholar
[15] 孔德智, 孙超, 李明杨, 卓颉, 刘雄厚 2019 68 174301
Google Scholar
Kong D Z, Sun C, Li M Y, Zhuo J, Liu X H 2019 Acta Phys. Sin. 68 174301
Google Scholar
下载:
计量
- 文章访问数: 3629
- PDF下载量: 69
- 被引次数: 0
