-
主要研究了热原子蒸气池中铯Rydberg原子nS1/2→(n + 1)S1/2微波耦合的双光子光谱. 铯原子基态(6S1/2)、第一激发态(6P3/2)、Rydberg态(69S1/2)形成阶梯型三能级系统, 弱探测光作用于基态到激发态6S1/2→6P3/2的跃迁, 强耦合光则作用于6P3/2→69S1/2的Rydberg跃迁形成电磁感应透明(EIT)效应, 实现对Rydberg原子的光学探测. 频率fMW = 11.735 GHz的微波场耦合69S1/2→70S1/2的Rydberg跃迁, 形成微波双光子光谱. 利用EIT-AT分裂光谱研究微波电场强度对双光子光谱的影响. 研究表明: 在强微波场作用时, EIT-AT分裂与微波场功率成正比, 而弱微波场时的EIT-AT分裂与微波场功率成非线性依赖关系, 理论计算与实验测量结果相一致. 本文的研究对微波电场的精密测量具有一定的指导意义.In this work, we present an nS1/2→(n + 1)S1/2 two-photon excitation EIT-AT spectrum of Rydberg atom in the vapor cell. A ground state (6S1/2), a first excited state (6P3/2) and Rydberg state (69S1/2) of cesium atoms constitute a three-level system. A weak probe laser locking to the transition of 6S1/2 (F = 4)→6P3/2 (F′ = 5) couples the ground-state transition, and the strong coupling laser drives the Rydberg transition of 6P3/2→69S1/2 to yield electromagnetically induced transparency (EIT) effect, which realizes the optical detection of Rydberg atoms. Two Rydberg 69S1/2 and 70S1/2 levels are coupled with the microwave field at a frequency of fMW = 11.735 GHz, forming a microwave two-photon spectrum. To observe the influence of microwave electric field power on two-photon spectrum, we investigate the microwave coupled Rydberg EIT-AT spectra at different microwave fields. The measurements show that the EIT-AT splitting interval is proportional to the square of the microwave electric field at strong microwave field, and indicvates a nonlinear dependence at weak microwave electric field. The theoretical calculation accords with the experimental measurement. The work here is of significance in precisely measuring the microwave electric field.
-
Keywords:
- Rydberg atom /
- microwave field /
- EIT-AT /
- two-photon spectroscopy
[1] Gallagher T F 1994 Rydberg Atoms (New York: Cambridge University Press) p38
[2] Comparat D, Pillet P 2010 J. Opt. Soc. Am. B 27 A208
Google Scholar
[3] Mohapatra A K, Jackson T R, Adams C S 2007 Phys. Rev. Lett. 98 113003
Google Scholar
[4] Autler S H, Townes C H 1955 Phys. Rev. 100 703
Google Scholar
[5] Holloway C L, Gordon J A, Jefferts S, Schwarzkopf A, Anderson D A, Miller S A, Thaicharoen N, Raithel G 2014 IEEE Trans. Antennas Propag. 62 6169
Google Scholar
[6] Sedlacek J A, Schwettmann A, Kübler H, Shaffer J P 2013 Phys. Rev. Lett. 111 063001
Google Scholar
[7] Jing M Y, Hu Y, Ma J, Zhang H, Zhang L J, Xiao L T, Jia S T 2020 Nat. Phys. 16 911
Google Scholar
[8] 樊佳蓓, 焦月春, 郝丽萍, 薛咏梅, 赵建明, 贾锁堂 2018 67 093201
Google Scholar
Fan J B, Jiao Y C, Hao L P, Xue Y M, Zhao J M, Jia S T 2018 Acta Phys. Sin. 67 093201
Google Scholar
[9] Hao L P, Xue Y M, Fan J B, Bai J X, Jiao Y C, Zhao J M, Jia S T 2020 Chin. Phys. B 29 033201
Google Scholar
[10] Gordon J A, Holloway C L, Schwarzkopf A, Anderson D A, Miller S, Thaicharoen N, Raithel G 2014 Appl. Phys. Lett. 105 024104
Google Scholar
[11] FanH Q, Kumar S, Daschner R, Kübler H, Shaffer J P 2014 Opt. Lett. 39 3030
Google Scholar
[12] Holloway C L, Gordon J A, Schwarzkopf A, Anderson D A, Miller S A, Thaicharoen N, Raithel G 2014 Appl. Phys. Lett. 104 244102
Google Scholar
[13] Cox K C, Meyer D H, Fatemi F K, Kunz P D 2018 Phys. Rev. Lett. 121 110502
Google Scholar
[14] Jiao Y C, Han X X, Fan J B, Raithel G, Zhao J M, Jia S T 2019 Appl. Phys. Exp. 12 126002
Google Scholar
[15] Song Z F, Liu H P, Liu X C, Zhang W F, Zou H Y, Zhang J, Qu J F 2019 Opt. Exp. 27 8848
Google Scholar
[16] Boyd R W 2008 Nonlinear Optics (Beijing: Academic Press) p55
[17] Jaksch D, Cirac J I, Zoller P, Rolston S L, Côte R, Lukin M D 2000 Phys. Rev. Lett. 85 2208
Google Scholar
[18] Lukin M D, Flischhauer M, Cote R, Duan L M, JakschD, Cirac J I, Zoller P 2001 Phys. Rev. Lett. 87 037901
Google Scholar
[19] Galindo A, Martín-Delgado M A 2002 Rev. Mod. Phys. 74 347
Google Scholar
[20] Isenhower L, Urban E, Zhang X L, Gill A T, Henage T, Johnson T A, Walker T G, Saffman M 2010 Phys. Rev. Lett. 104 010503
Google Scholar
[21] Dudin Y O, Kuzmich A 2012 Science 336 887
Google Scholar
[22] Peyronel T, Firstenberg O, Liang Q Y, Hofferberth S, Gorshkov A V, Pohl T, Lukin M D, Vuletić V 2012 Nature 488 57
Google Scholar
[23] Maxwell D, Szwer D J, Paredes-Barato D, Busche H, Pritchard J D, Gauguet A, Weatherill K J, Jones M P A, Adams C S 2013 Phys. Rev. Lett. 110 103001
Google Scholar
[24] Lukin M D 2003 Rev. Mod. Phys. 75 457
Google Scholar
[25] 李敬奎, 杨文广, 宋振飞, 张好, 张临杰, 赵建明, 贾锁堂 2015 64 163201
Google Scholar
Li J K, Yang W G, Song Z F, Zhang H, Zhang L J, Zhao J M, Jia S T 2015 Acta Phys. Sin. 64 163201
Google Scholar
[26] Pearman C P, Adams C S, Cox S G, Griffin P F, Smith D A, Hughes I G 2002 J. Phys. B:At. Mol. Opt. Phys. 35 5141
Google Scholar
[27] Gentile T R, Hughey B J, Kleppner D, Ducas T W 1989 Phys. Rev. A 40 5103
Google Scholar
[28] Hao L P, Jiao Y C, Xue Y M, Han X X, Bai S Y, Zhao J M, Raithel G 2018 New J. Phys. 20 073024
Google Scholar
[29] Holloway C L, Simons M T, Gordon J A, Dienstfrey A, Anderson D A, Raithel G 2017 J. Appl. Phys. 121 233106
Google Scholar
-
图 1 (a) 实验装置示意图, 其中DM为二向色镜, GP为垃圾池, Lens 1为852 nm透镜, Lens 2为510 nm透镜, PBS为偏振分光棱镜, PD为光电探测器; (b)铯原子阶梯型四能级示意图
Fig. 1. (a) Sketch of the experimental setup, where DM is dichroic mirror, Lens 1(2) is lens of 852 nm (510 nm) laser, GP is garbage pool for green laser, PBS is polarizing beam splitter, PD is photodiode detector; (b) energy-level diagram for the four-level cascade configuration.
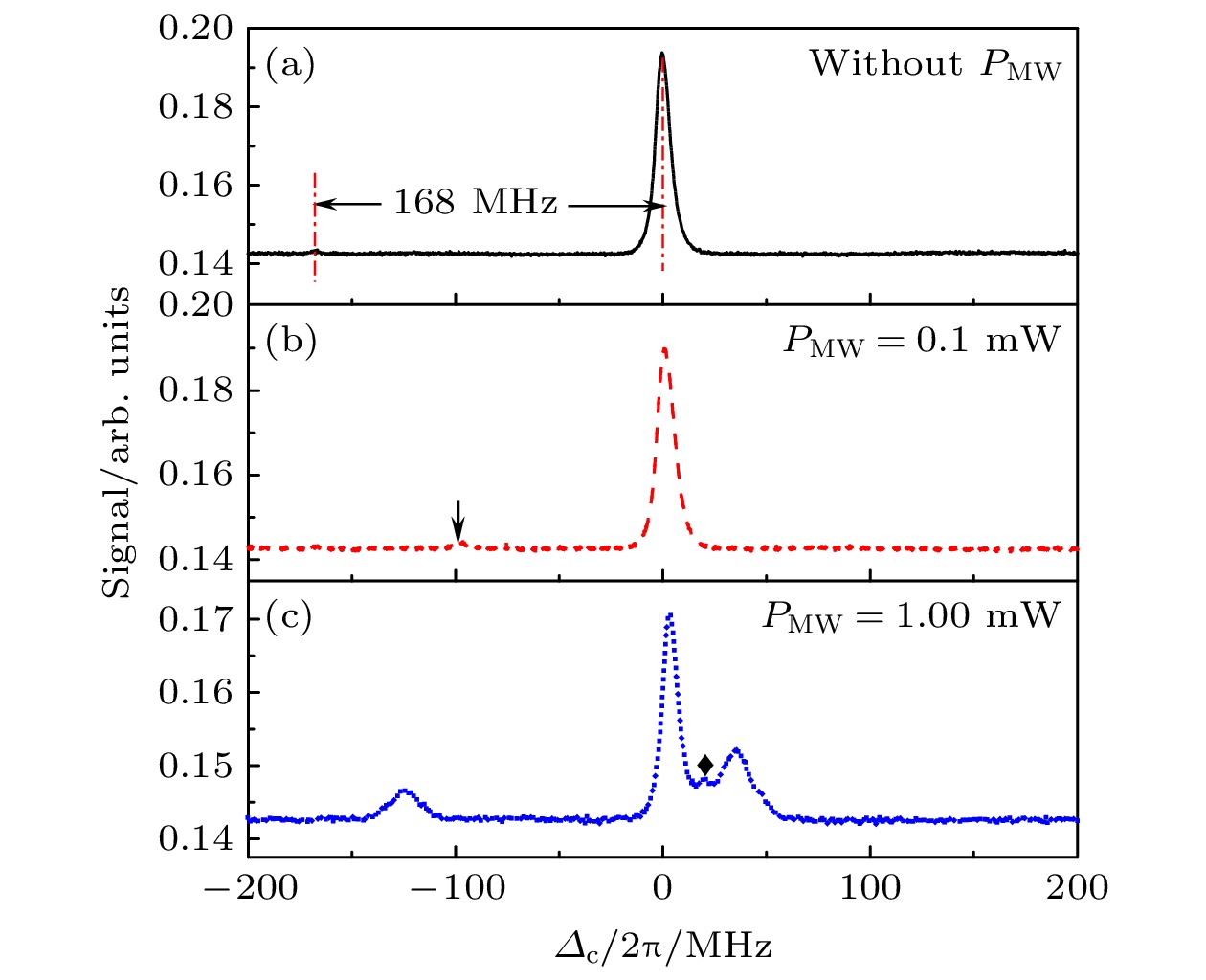
图 2 (a) 无微波场时耦合光在6P3/2 (F' = 5)→69S1/2的Rydberg跃迁附近扫描时的EIT光谱(黑色实线); (b) (c)微波场功率分别为PMW = 0.1 mW (红色虚线)和1.0 mW(蓝色点线)时的Rydberg EIT-AT双光子激发光谱
Fig. 2. (a) Rydberg EIT spectroscopy without microwave field (black solid line); (b)(Red dashed line) and (c) (blue dotted line) Rydberg-EIT-AT two-photon excitation spectrum with the microwave field power PMW = 0.1 mW and 1.0 mW, respectively.
图 3 不同微波信号源的输出功率时的Rydberg EIT-AT分裂光谱的三维图(蓝色), 红色虚线和黑色点线是理论计算微波耦合69S1/2→70S1/2时EIT-AT光谱, 蓝色方块表示中间态形成的EIT谱的频移
Fig. 3. Three-dimensional color map (blue) of the Rydberg EIT-AT spectra with different output power, the red dashed and black dotted lines are the theoretical calculations of the frequency shift and EIT-AT splitting of 69S1/2→70S1/2. The blue squares are thecalculated shift of EIT spectra due to the intermediate state.
图 4 微波耦合Rydberg原子69S1/2→70S1/2双光子EIT-AT分裂间隔与微波场功率PMW的依赖关系
Fig. 4. Dependence of the EIT-AT splitting of the Rydberg atom 69S1/2→70S1/2 transition on the microwave power PMW.
-
[1] Gallagher T F 1994 Rydberg Atoms (New York: Cambridge University Press) p38
[2] Comparat D, Pillet P 2010 J. Opt. Soc. Am. B 27 A208
Google Scholar
[3] Mohapatra A K, Jackson T R, Adams C S 2007 Phys. Rev. Lett. 98 113003
Google Scholar
[4] Autler S H, Townes C H 1955 Phys. Rev. 100 703
Google Scholar
[5] Holloway C L, Gordon J A, Jefferts S, Schwarzkopf A, Anderson D A, Miller S A, Thaicharoen N, Raithel G 2014 IEEE Trans. Antennas Propag. 62 6169
Google Scholar
[6] Sedlacek J A, Schwettmann A, Kübler H, Shaffer J P 2013 Phys. Rev. Lett. 111 063001
Google Scholar
[7] Jing M Y, Hu Y, Ma J, Zhang H, Zhang L J, Xiao L T, Jia S T 2020 Nat. Phys. 16 911
Google Scholar
[8] 樊佳蓓, 焦月春, 郝丽萍, 薛咏梅, 赵建明, 贾锁堂 2018 67 093201
Google Scholar
Fan J B, Jiao Y C, Hao L P, Xue Y M, Zhao J M, Jia S T 2018 Acta Phys. Sin. 67 093201
Google Scholar
[9] Hao L P, Xue Y M, Fan J B, Bai J X, Jiao Y C, Zhao J M, Jia S T 2020 Chin. Phys. B 29 033201
Google Scholar
[10] Gordon J A, Holloway C L, Schwarzkopf A, Anderson D A, Miller S, Thaicharoen N, Raithel G 2014 Appl. Phys. Lett. 105 024104
Google Scholar
[11] FanH Q, Kumar S, Daschner R, Kübler H, Shaffer J P 2014 Opt. Lett. 39 3030
Google Scholar
[12] Holloway C L, Gordon J A, Schwarzkopf A, Anderson D A, Miller S A, Thaicharoen N, Raithel G 2014 Appl. Phys. Lett. 104 244102
Google Scholar
[13] Cox K C, Meyer D H, Fatemi F K, Kunz P D 2018 Phys. Rev. Lett. 121 110502
Google Scholar
[14] Jiao Y C, Han X X, Fan J B, Raithel G, Zhao J M, Jia S T 2019 Appl. Phys. Exp. 12 126002
Google Scholar
[15] Song Z F, Liu H P, Liu X C, Zhang W F, Zou H Y, Zhang J, Qu J F 2019 Opt. Exp. 27 8848
Google Scholar
[16] Boyd R W 2008 Nonlinear Optics (Beijing: Academic Press) p55
[17] Jaksch D, Cirac J I, Zoller P, Rolston S L, Côte R, Lukin M D 2000 Phys. Rev. Lett. 85 2208
Google Scholar
[18] Lukin M D, Flischhauer M, Cote R, Duan L M, JakschD, Cirac J I, Zoller P 2001 Phys. Rev. Lett. 87 037901
Google Scholar
[19] Galindo A, Martín-Delgado M A 2002 Rev. Mod. Phys. 74 347
Google Scholar
[20] Isenhower L, Urban E, Zhang X L, Gill A T, Henage T, Johnson T A, Walker T G, Saffman M 2010 Phys. Rev. Lett. 104 010503
Google Scholar
[21] Dudin Y O, Kuzmich A 2012 Science 336 887
Google Scholar
[22] Peyronel T, Firstenberg O, Liang Q Y, Hofferberth S, Gorshkov A V, Pohl T, Lukin M D, Vuletić V 2012 Nature 488 57
Google Scholar
[23] Maxwell D, Szwer D J, Paredes-Barato D, Busche H, Pritchard J D, Gauguet A, Weatherill K J, Jones M P A, Adams C S 2013 Phys. Rev. Lett. 110 103001
Google Scholar
[24] Lukin M D 2003 Rev. Mod. Phys. 75 457
Google Scholar
[25] 李敬奎, 杨文广, 宋振飞, 张好, 张临杰, 赵建明, 贾锁堂 2015 64 163201
Google Scholar
Li J K, Yang W G, Song Z F, Zhang H, Zhang L J, Zhao J M, Jia S T 2015 Acta Phys. Sin. 64 163201
Google Scholar
[26] Pearman C P, Adams C S, Cox S G, Griffin P F, Smith D A, Hughes I G 2002 J. Phys. B:At. Mol. Opt. Phys. 35 5141
Google Scholar
[27] Gentile T R, Hughey B J, Kleppner D, Ducas T W 1989 Phys. Rev. A 40 5103
Google Scholar
[28] Hao L P, Jiao Y C, Xue Y M, Han X X, Bai S Y, Zhao J M, Raithel G 2018 New J. Phys. 20 073024
Google Scholar
[29] Holloway C L, Simons M T, Gordon J A, Dienstfrey A, Anderson D A, Raithel G 2017 J. Appl. Phys. 121 233106
Google Scholar
下载:
计量
- 文章访问数: 4655
- PDF下载量: 125
- 被引次数: 0
