-
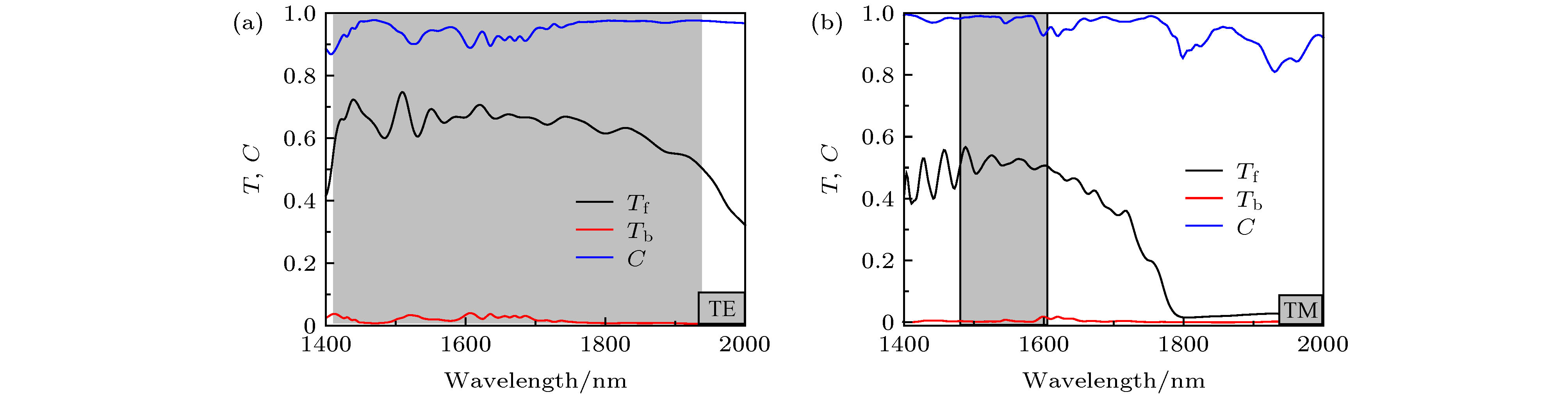
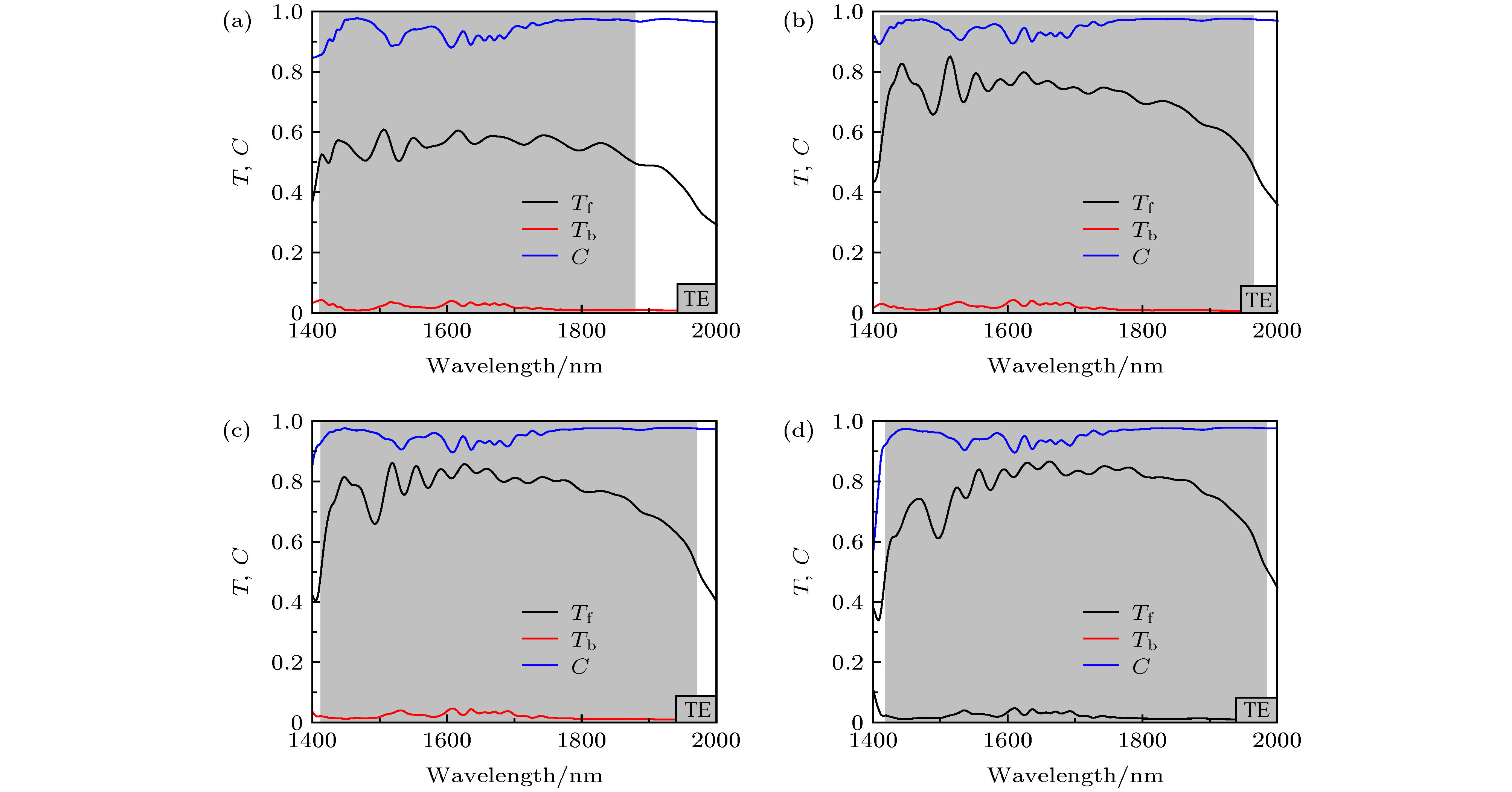
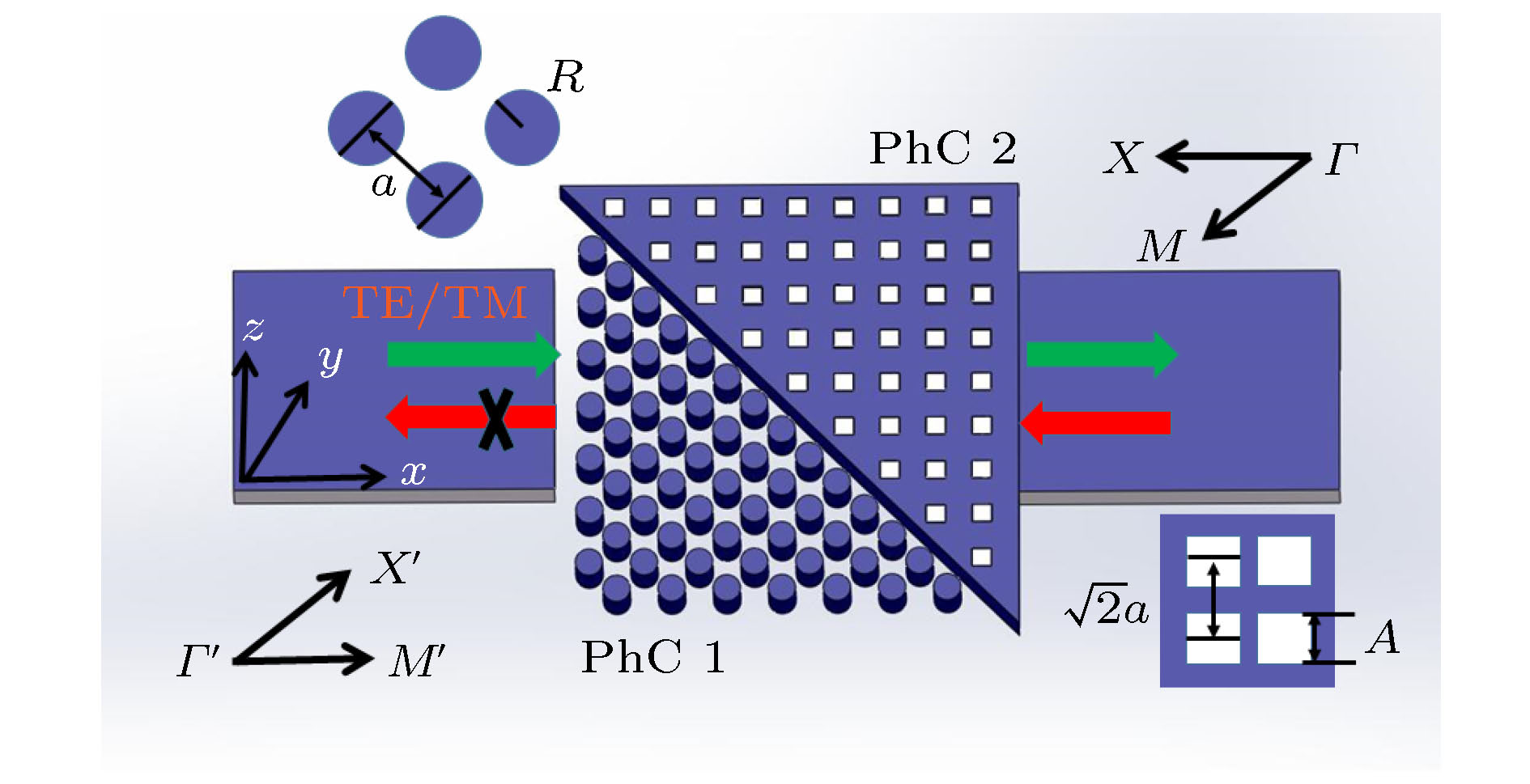
利用光子晶体的自准直效应和能带特性, 设计了一种能实现宽频带光波非对称传输的二维光子晶体异质结构. 该结构实现宽频带、高正向透射、非偏振选择的非对称传输. 横电(transverse electric, TE)偏振光非对称传输波长带宽可达532 nm, 在光通信波长1550 nm处正向透射率和透射对比度分别可达0.693和0.946; 横磁(transverse magnetic, TM)偏振光非对称传输波长带宽为128 nm, 在光通信波长1550 nm处正向透射率和透射对比度分别可达0.513和0.972; 通过进一步优化异质结界面, 在TE偏振光下非对称传输波长带宽可达562 nm.Recently, quantum computing and information processing based on photons has become one research frontier, attracting significant attentions. The optical asymmetric transmission devices (OATD), having similar function to the diode in electric circuitry, will find important applications. In particular, the OATDs based on nanophotonic structures are preferred due to their potential applications in the on-chip integration with other photonic devices. Therefore, there have been numerous applications of OATDs based on different nanostructures, including composite grating structures, metasurfaces, surface plasmon polaritons, metamaterials, photonic crystals (PhCs). However, in general, those designs show relatively low forward transmittance (< 0.5) and narrow working bandwidth (< 100 nm), and they are able to work with only one polarization state. This makes the current OATDs unsuitable for many applications. To solve this challenge, here we design a two-dimensional (2D) PhC heterostructure based on the self-collimating effect and bandgap properties. The PhC heterostructure is composed of two square lattice 2D PhCs (PhC 1 and PhC 2) on a silicon substrate with different lattice shapes and lattice constants. The PhC 1 is composed of periodically arranged silicon cylinders in air. Meanwhile, the PhC 2 is an square air hole array embedding in silicon. The two PhCs are integrated with an inclined interface with an angle of 45° with respect to the direction of incident light. The plane wave expansion method is used to calculate the band diagrams and equal frequency contours (EFCs) of the two PhCs. As the propagation directions of light waves in PhCs are determined by the gradient direction of the EFCs, we are able to control the light propagation by controlling the EFCs of PhCs. By engineering the EFCs, the PhC 2 shows strong self-collimation effect in a broad wavelength range with a central wavelength of 1550 nm for both TE and TM polarization. By self-collimating the forward incident light from different incident angles to couple to the output waveguide, we are able to significantly increase the forward transmittance to > 0.5 for both TE and TM polarized light. Meanwhile, the backward transmittance can be effectively cut off by the unique dispersion properties of the PhC heterostructures. In this way, the heterostructure is able to achieve polarization independent asymmetric transmission of light waves in a broad wavelength range. To visualize the light propagation in the PhC heterostructure, we use the finite-difference-time-domain method to calculate the electric intensity distributions of the forward and backward propagation light of both TE and TM polarization at a wavelength of 1550 nm. Strong self-collimation effect of forward propagation light and the nearly complete blockage of backward propagation light can be identified unambiguously in the intensity plots, confirming the theoretical analysis. The calculation of transmittance and contrast ratio spectra show that the asymmetric transmission wavelength bandwidth can reach 532 nm with the forward transmittance and contrast ratio being 0.693 and 0.946 at an optical communication wavelength of 1550 nm for TE polarized light. On the other hand, for the TM polarized light, the asymmetric transmission wavelength bandwidth is 128 nm, the forward transmittance and contrast ratio are 0.513 and 0.972, respectively, at 1550 nm wavelength. Thus, it is confirmed that the PhC heterostructure achieves highly efficient, broadband and polarization independent asymmetric transmission. Finally, to further improve the forward transmittance of the TE polarized light, we modulate the radius of the front row of photonic lattice of PhC 1 at the interface. It shows that the forward transmittance can be further improved to a record high value of 0.832 with a bandwidth of 562 nm for TE polarized light. Our design opens up new possibilities for designing OATDs based on PhCs, and will find broad applications, for the design can be realized by current nanofabrication techniques.
-
Keywords:
- self-collimation /
- photonic bandgap /
- heterostructure /
- asymmetric transmission
[1] Espinola R L, Izuhara T, Tsai M C, Osgoode R M 2004 Opt. Lett. 29 941
 Google Scholar
Google Scholar
[2] Zhou X, Wang Y, Leykam D, Chong Y D 2017 New J. Phys. 19 095002
 Google Scholar
Google Scholar
[3] Fan L, Wang J, Varghese L T, Shen H, Niu B, Xuan Y, Weiner A M, Qi M 2012 Science 335 447
 Google Scholar
Google Scholar
[4] Kuzmiak V, Maradudin A A 2015 Phys. Rev. A 92 013615
 Google Scholar
Google Scholar
[5] Stolarek M, Yavorskiy D, Kotyński R, Zapata R, Carlos J, Łusakowski J, Szoplik T 2013 Opt. Lett. 38 839
 Google Scholar
Google Scholar
[6] Xiao Z Y, Zou H L, Xu K K, Tang J Y 2018 J. Magn. Magn. Mater. 449 278
 Google Scholar
Google Scholar
[7] Ozer A, Kocer H, Kurt H 2018 J. Opt. Soc. Am. B 35 2111
 Google Scholar
Google Scholar
[8] Zhang L, Mei S, Huang K, Qiu C W 2016 Adv. Opt. Mater. 4 818
 Google Scholar
Google Scholar
[9] Ling Y H, Lirong H, Wei H, Tongjun L, Yali S, Jing L, Gang Y 2017 Opt. Express 25 13648
 Google Scholar
Google Scholar
[10] Peng Y X, Wang K J, He M D, Luo J H, Zhang X M, Li J B, Tan S H, Liu J Q, Hu W D, Chen X S 2018 Opt. Commun. 412 1
 Google Scholar
Google Scholar
[11] Li X F, Rui F, Ding W 2018 J. Phys. D: Appl. Phys. 51 145304
 Google Scholar
Google Scholar
[12] Bai Y, Chen Y, Zhang Y, Wang Y, Aba T, Li H, Wang L, Zhang Z 2018 J. Phys. Condens. Matter 30 114001
 Google Scholar
Google Scholar
[13] Stephen L, Yogesh N, Subramanian V 2018 J. Appl. Phys. 123 033103
 Google Scholar
Google Scholar
[14] Xu T, Lezec H J 2014 Nat. Commun. 5 4141
 Google Scholar
Google Scholar
[15] Soltani A, Ouerghi F, AbdelMalek F, Haxha S, Ademgil H, Akowuah E K 2017 Opt. Commun. 392 147
 Google Scholar
Google Scholar
[16] Wu Z, Chen J, Ji M, Huang Q, Xia J, Wu Y, Wang Y 2015 Appl. Phys. Lett. 107 221102
 Google Scholar
Google Scholar
[17] Zhang Y, Li D, Zeng C, Huang Z, Wang Y, Huang Q, Wu Y, Yu J, Xia J 2014 Opt. Lett. 39 1370
 Google Scholar
Google Scholar
[18] Zhang Y, Kan Q, Wang G P 2014 Opt. Lett. 39 4934
 Google Scholar
Google Scholar
[19] Serebryannikov A E, Alici K B, Magath T, Cakmak A O, Ozbay E 2012 Phys. Rev. A 86 053835
 Google Scholar
Google Scholar
[20] Wang C, Zhou C Z, Li Z Y 2011 Opt. Express 19 26948
 Google Scholar
Google Scholar
[21] 刘丹, 胡森, 肖明 2017 66 054209
 Google Scholar
Google Scholar
Liu D, Hu S, Xiao M 2017 Acta Phys. Sin. 66 054209
 Google Scholar
Google Scholar
[22] 费宏明, 徐婷, 刘欣, 林瀚, 陈智辉, 杨毅彪, 张明达, 曹斌照, 梁九卿 2017 66 204103
 Google Scholar
Google Scholar
Fei H M, Xu T, Liu X, Lin H, Chen Z H, Yang Y B, Zhang M D, Cao B Z, Liang J Q 2017 Acta Phys. Sin. 66 204103
 Google Scholar
Google Scholar
[23] Fei H M, Wu M, Xu T, Lin H, Yang Y B, Liu X, Zhang M D, Cao B Z 2018 J. Opt. 20 095004
 Google Scholar
Google Scholar
[24] Bourzac K 2012 Nature 483 388
 Google Scholar
Google Scholar
-
图 2 (a) PhC 1能带图; (b) PhC 2能带图, 插图为PhC 2在Γ—X方向的能带; (c) PhC 1在TE偏振模式第一条能带EFC; (d) PhC 2在TE偏振光下第四条能带EFC (蓝线表示TE偏振光1550 nm处的频带); (e) PhC 1在TM偏振光第一条能带EFC; (f) PhC 2在TM偏振光第三条能带EFC (红线表示TM模式1550 nm处的频带)
Fig. 2. (a) Photonic band diagrams of PhC 1; (b) the photonic band diagrams of PhC 2, where the insert shows the energy band of PhC 2 in Γ-X direction; (c) the first band EFC of PhC 1 under TE polarized light; (d) the fourth band EFC of PhC 2 under TE polarized light (blue lines represent TE mode at the wavelength of 1550 nm); (e) the first band EFC of PhC 1 under TM polarized light; (f) the third band EFC of PhC 2 under TM polarized light (red lines represent TM mode at 1550 nm).
图 3 1550 nm波长处正向入射场强图和反向入射场强图 (a) TE偏振光正向; (b) TE偏振光反向; (c) TM偏振光正向; (d) TM偏振光反向
Fig. 3. Electric field intensity distribution of forward transmission and backward transmission at the wavelength of 1550 nm: (a) Forward transmission of TE polarized light; (b) backward transmission of TE polarized light; (c) forward transmission of TM polarized light; (d) backward transmission of TM polarized light.
表 1 异质界面处PhC 1硅圆柱不同半径的非对称传输性能
Table 1. Asymmetric transmission performance with different radii of PhC 1 at heterostructure interface.
R/nm 1550 nm正向
透射率透射对比度 非对称传输
带宽/nm55 0.579 0.941 448 60 0.693 0.946 532 65 0.789 0.947 556 70 0.832 0.944 562 75 0.803 0.942 568 -
[1] Espinola R L, Izuhara T, Tsai M C, Osgoode R M 2004 Opt. Lett. 29 941
 Google Scholar
Google Scholar
[2] Zhou X, Wang Y, Leykam D, Chong Y D 2017 New J. Phys. 19 095002
 Google Scholar
Google Scholar
[3] Fan L, Wang J, Varghese L T, Shen H, Niu B, Xuan Y, Weiner A M, Qi M 2012 Science 335 447
 Google Scholar
Google Scholar
[4] Kuzmiak V, Maradudin A A 2015 Phys. Rev. A 92 013615
 Google Scholar
Google Scholar
[5] Stolarek M, Yavorskiy D, Kotyński R, Zapata R, Carlos J, Łusakowski J, Szoplik T 2013 Opt. Lett. 38 839
 Google Scholar
Google Scholar
[6] Xiao Z Y, Zou H L, Xu K K, Tang J Y 2018 J. Magn. Magn. Mater. 449 278
 Google Scholar
Google Scholar
[7] Ozer A, Kocer H, Kurt H 2018 J. Opt. Soc. Am. B 35 2111
 Google Scholar
Google Scholar
[8] Zhang L, Mei S, Huang K, Qiu C W 2016 Adv. Opt. Mater. 4 818
 Google Scholar
Google Scholar
[9] Ling Y H, Lirong H, Wei H, Tongjun L, Yali S, Jing L, Gang Y 2017 Opt. Express 25 13648
 Google Scholar
Google Scholar
[10] Peng Y X, Wang K J, He M D, Luo J H, Zhang X M, Li J B, Tan S H, Liu J Q, Hu W D, Chen X S 2018 Opt. Commun. 412 1
 Google Scholar
Google Scholar
[11] Li X F, Rui F, Ding W 2018 J. Phys. D: Appl. Phys. 51 145304
 Google Scholar
Google Scholar
[12] Bai Y, Chen Y, Zhang Y, Wang Y, Aba T, Li H, Wang L, Zhang Z 2018 J. Phys. Condens. Matter 30 114001
 Google Scholar
Google Scholar
[13] Stephen L, Yogesh N, Subramanian V 2018 J. Appl. Phys. 123 033103
 Google Scholar
Google Scholar
[14] Xu T, Lezec H J 2014 Nat. Commun. 5 4141
 Google Scholar
Google Scholar
[15] Soltani A, Ouerghi F, AbdelMalek F, Haxha S, Ademgil H, Akowuah E K 2017 Opt. Commun. 392 147
 Google Scholar
Google Scholar
[16] Wu Z, Chen J, Ji M, Huang Q, Xia J, Wu Y, Wang Y 2015 Appl. Phys. Lett. 107 221102
 Google Scholar
Google Scholar
[17] Zhang Y, Li D, Zeng C, Huang Z, Wang Y, Huang Q, Wu Y, Yu J, Xia J 2014 Opt. Lett. 39 1370
 Google Scholar
Google Scholar
[18] Zhang Y, Kan Q, Wang G P 2014 Opt. Lett. 39 4934
 Google Scholar
Google Scholar
[19] Serebryannikov A E, Alici K B, Magath T, Cakmak A O, Ozbay E 2012 Phys. Rev. A 86 053835
 Google Scholar
Google Scholar
[20] Wang C, Zhou C Z, Li Z Y 2011 Opt. Express 19 26948
 Google Scholar
Google Scholar
[21] 刘丹, 胡森, 肖明 2017 66 054209
 Google Scholar
Google Scholar
Liu D, Hu S, Xiao M 2017 Acta Phys. Sin. 66 054209
 Google Scholar
Google Scholar
[22] 费宏明, 徐婷, 刘欣, 林瀚, 陈智辉, 杨毅彪, 张明达, 曹斌照, 梁九卿 2017 66 204103
 Google Scholar
Google Scholar
Fei H M, Xu T, Liu X, Lin H, Chen Z H, Yang Y B, Zhang M D, Cao B Z, Liang J Q 2017 Acta Phys. Sin. 66 204103
 Google Scholar
Google Scholar
[23] Fei H M, Wu M, Xu T, Lin H, Yang Y B, Liu X, Zhang M D, Cao B Z 2018 J. Opt. 20 095004
 Google Scholar
Google Scholar
[24] Bourzac K 2012 Nature 483 388
 Google Scholar
Google Scholar
计量
- 文章访问数: 8653
- PDF下载量: 165
- 被引次数: 0













 下载:
下载: