-
不同的频率失谐会在耦合光学微腔激发出不同的工作模式. 以两个耦合光场的非线性薛定谔方程为理论模型, 分别研究了失谐参量正调谐和负调谐过程中微腔内光场的变化. 理论分析结果表明, 在正失谐区域中, 腔内光场可由多脉冲形式演变为亮孤子, 但亮孤子存在范围较小, 当失谐参量过大时, 腔内光场会演化为直流分布. 在负失谐区域, 腔内可以形成较高功率“图灵环”形式的光场. 当耦合微腔没有发生频率失谐, 或者失谐参量接近0时, 腔内只能形成混沌形式的光场分布. 当耦合微腔内激发出光孤子后, 通过选取合适的失谐参量和抽运功率, 可在腔内维持稳定的亮孤子. 此外还可通过继续调谐第一个微腔的失谐参量, 将亮孤子转变为低功率的“图灵环”. 理论分析结果对耦合微腔的实验研究具有重要意义.Different frequency detuning can excite different working mode in a dual coupled optical microcavities. Based on the nonlinear Schrödinger equations of dual coupled field, and by using the split-step Fourier method, the optical field evolution in the microcavities is analyzed under the condition of both positive and negative tuning, and various optical distributions are generated in the process of frequency tuning. Simulation results indicate that the field can develop into the bright soliton in the region of positive tuning. However, the region in which the bright soliton is maintained is small, and the field in the microcavities grows into direct current (DC) distribution because of the serious frequency detuning. In the region of negative tuning, the field of “turning pattern” with high power is generated. There is only chaos inside the microcavities without frequency detuning or the detuning parameters close to 0. In addition, under the condition of strong coupling, the bright soliton and the “turning pattern” cannot be excited. Even stronger coupling leads to optical field in the form of DC directly. After the bright soliton exciting in the microcavity, it can be preserved by selecting appropriate detuning parameters and pump power. Moreover, the bright soliton can be changed into “turning pattern” with low power by continuously changing the detuning parameter of the first microcavity. Theoretical analyses are significant for experimental research on the dual coupled microcavities.
-
Keywords:
- optical microcavities /
- frequency detuning /
- bright soliton
[1] 孟飞, 曹士英, 蔡岳, 王贵重, 曹建平, 李天初, 方占军 2011 60 100601
Google Scholar
Meng F, Cao S Y, Cai Y, Wang G Z, Cao J P, Li T C, Fang Z J 2011 Acta Phys. Sin. 60 100601
Google Scholar
[2] Del’Haye P, Coillet A, Fortier T, Beha K, Cole D C, Yang K Y, Lee H, Vahala K J, Papp S B, Diddams S A 2016 Nat. Photonics 10 516
Google Scholar
[3] Lamb E S, Carlson D R, Hickstein D D, Stone J R, Diddams S A, Papp S B 2018 Phys. Rev. Appl. 9 024030
Google Scholar
[4] Newman Z L, Maurice V, Drake T, Stone J R, Briles T C, Spencer D T, Fredrick C, Li Q, Westly D, Ilic B R, Shen B, Suh M G, Yang K Y, Johnson C, Johnson D M S, Hollberg L, Vahala K J, Srinivasan K, Diddams S A, Kitching J, Papp S, Hummon M T 2019 Optica 6 680
Google Scholar
[5] Zhang X Y, Cao Q T, Wang Z, Liu Y X, Qiu C W, Yang L, Gong Q H, Xiao Y F 2019 Nat. Photonics 13 2
Google Scholar
[6] Wang W Q, Lu Z Z, Zhang W F, Chu S T, Little B E, Wang L R, Xie X P, Liu M L, Yang Q H, Wang L, Zhao J G, Wang G X, Sun Q B, Liu Y S, Wang Y S, Zhao W 2018 Opt. Lett. 43 2002
Google Scholar
[7] Xue X X, Xuan Y, Liu Y, Wang P H, Chen S, Wang J, Leaird D E, Qi M H, Weiner A M 2015 Nat. Photonics 9 594
Google Scholar
[8] Coillet A, Balakireva I, Henriet R, Saleh K, Larger L, Dudley J M, Menyuk C R, Chembo Y K 2013 IEEE Photonics J. 5 6100409
Google Scholar
[9] Matsko A B, Liang W, Savchenkov A A, Maleki L 2013 Opt. Lett. 38 525
Google Scholar
[10] Yi X, Yang Q F, Yang K Y, Suh M G, Vahala K 2015 Optica 2 1078
Google Scholar
[11] Pasquazi A, Caspani L, Peccianti M, Clerici M, Ferrera M, Razzari L, Duchesne D, Little B E, Chu S T, Moss D J, Morandotti R 2013 Opt. Express 21 13333
Google Scholar
[12] Ferrera M, Reimer C, Pasquazi A, Peccianti M, Clerici M, Caspani L, Chu S T, Little B E, Morandotti R, Moss D J 2014 Opt. Express 22 21488
Google Scholar
[13] Riemensberger J, Hartinger K, Herr T, Brasch V, Holzwarth R, Kippenberg T J 2012 Opt. Express 20 27661
Google Scholar
[14] Xue X X, Zheng X P, Zhou B K 2019 Nat. Photonics 13 616
Google Scholar
[15] Maes B, Fiers M, Bienstman P 2009 Phys. Rev. A 80 033805
Google Scholar
[16] Maes B, Soljacic M, Joannopoulos J D 2006 Opt. Express 14 10678
Google Scholar
[17] Dumeige Y, Ghisa L, Féron P 2006 Opt. Lett. 31 2187
Google Scholar
[18] Dumeige Y, Féron P 2015 Opt. Lett. 40 3237
Google Scholar
[19] Coen S, Haelterman M 2001 Opt. Lett. 26 39
Google Scholar
[20] Akhmediev N, Pelinovsky E 2010 Eur. Phys. J. Spec. Top. 185 1
Google Scholar
[21] Xue X X, Leo F, Xuan Y, Villegas J A J, Wang P H, Leaird D E, Erkintalo M, Qi M H, Weiner A M 2017 Light: Sci. Appl. 6 e16253
Google Scholar
[22] Peng B, Ozdemir S K, Zhu J G, Yang L 2013 Opt. Lett. 37 3435
Google Scholar
[23] Bo F, Oezdemir S K, Peng B, Wang J, Zhang G Q, Xu J J, Yang L 2015 Opt. Express 23 30793
Google Scholar
[24] Kippenberg T J, Holzwarth R, Diddams S A 2011 Science 332 555
Google Scholar
-
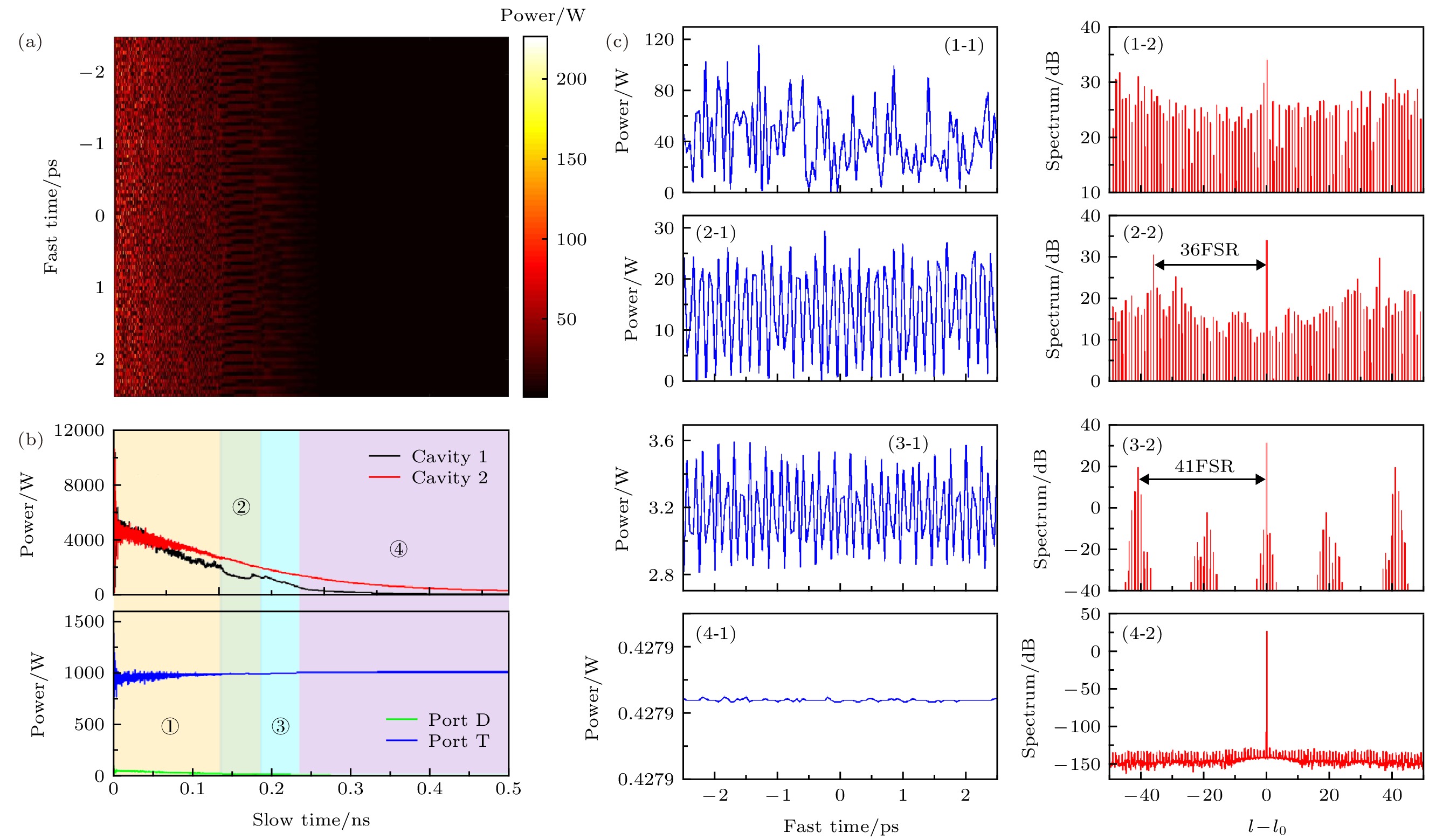
图 2 (a)失谐参量在正方向调谐过程中, 第一个光学微腔内光场分布随时间的演化; (b) 失谐参量调谐过程中, 光功率随时间的变化曲线; (c)与图(b)中各个阶段对应的光场分布和光谱图
Fig. 2. (a) Evolution of the optical field inside the first microcavity in the region of positive frequency tuning; (b) curves of the optical power variation in the process of frequency tuning; (c) field distribution and spectra corresponding to each stage in Fig. (b).
图 3 (a)失谐参量在负方向调谐过程中, 第一个光学微腔内光场分布随时间的演化; (b) 失谐参量调谐过程中, 光功率随时间的变化曲线; (c)与图(b)中各个阶段对应的光场分布和光谱图
Fig. 3. (a) Evolution of the optical field inside the first microcavity in the process of negative frequency tuning; (b) curves of the optical power variation in the process of frequency tuning; (c) field distribution and spectra corresponding to each stage in Fig. (b).
图 4 (a)强耦合光学微腔在失谐频率正调谐过程中光场的演化; (b) 强耦合光学微腔在失谐频率负调谐过程中光场的演化
Fig. 4. (a) Evolution of the optical field inside the first microcavity with strong coupling in the region of positive frequency tuning; (b) evolution of the optical field inside the first microcavity with strong coupling in the region of negative frequency tuning.
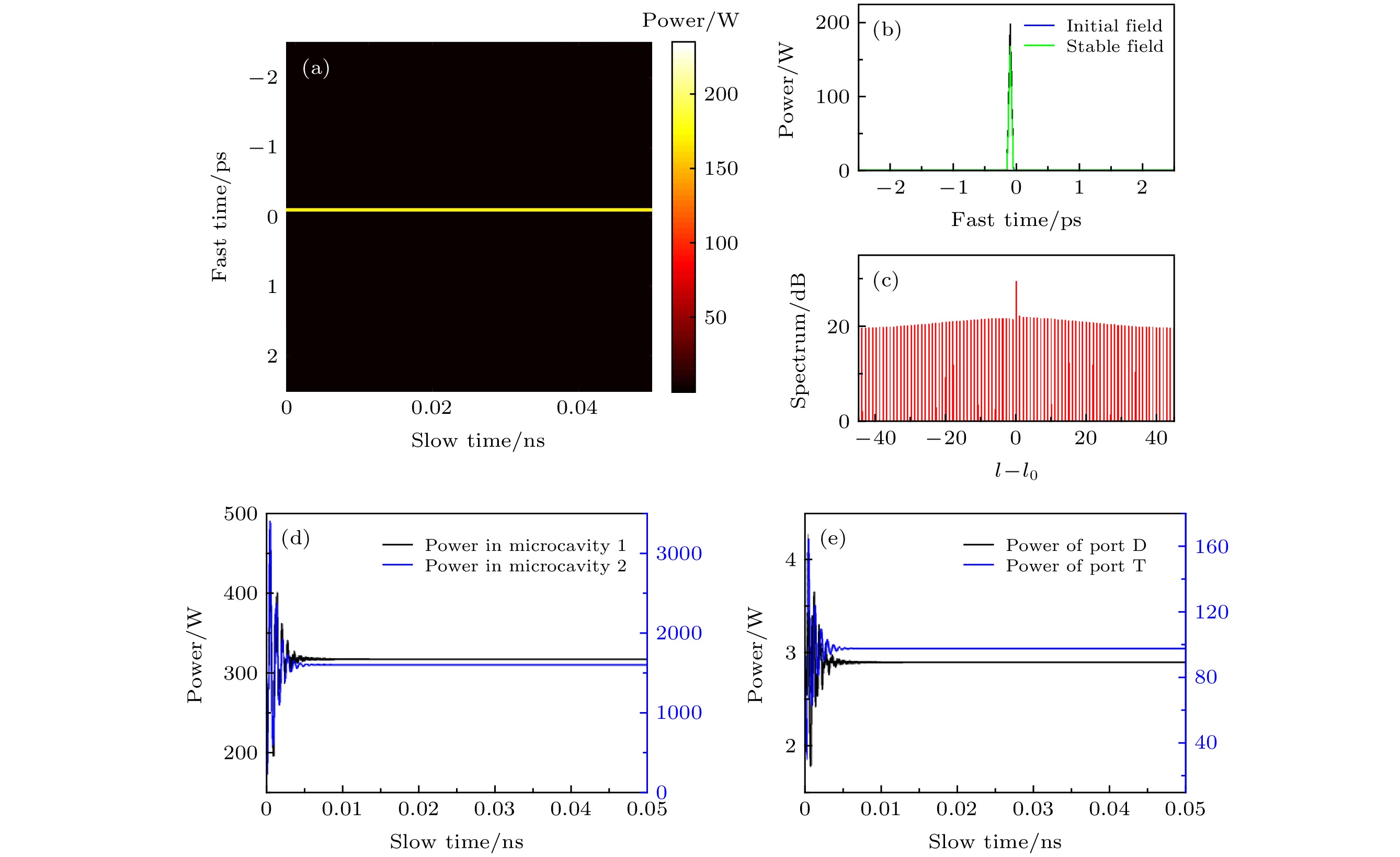
图 5 (a) 亮孤子在腔内稳定存在的演化过程(δ1 = 1.201 × 102 m–1, δ2 = 8.809 m–1, Pin = 1 W); (b) 亮孤子的光场分布; (c) 亮孤子的光谱图; (d) 两个微腔内的光功率随时间的变化曲线; (e) D端口和T端口输出功率随时间的变化曲线
Fig. 5. (a) Evolution of the stable existence of bright soliton in the microcavity (δ1 = 1.201 × 102 m–1, δ2 = 8.809 m–1, Pin = 1 W); (b) field distribution of the bright soliton; (c) spectrum of the bright soliton; (d) curves of the dual power inside the dual coupled microcavities vary with the slow time; (e) curves of the dual power of Port D and T vary with the slow time.
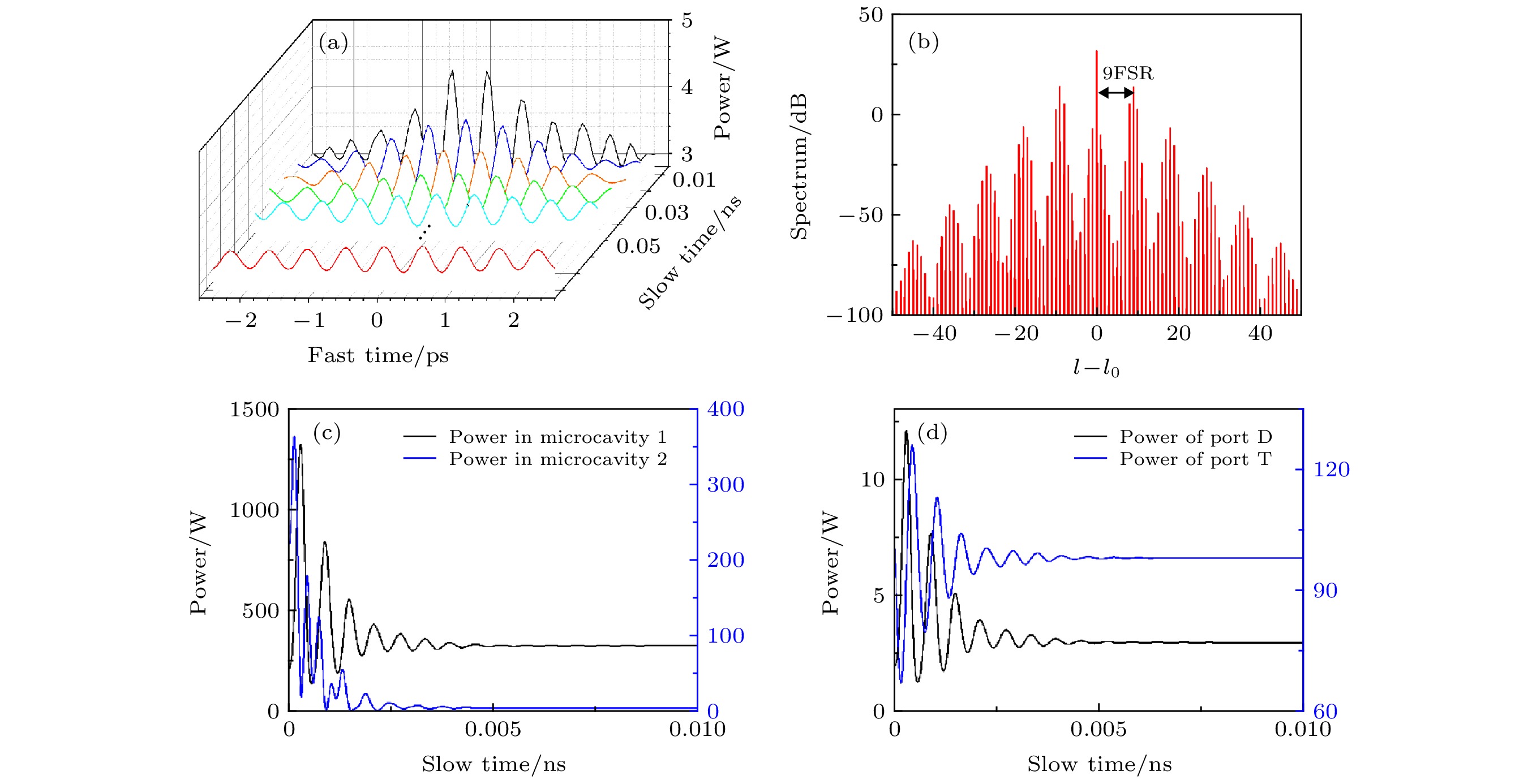
图 6 (a) 亮孤子向多脉冲光场演化的过程(δ2 = 16.1411 m–1, Δf1初始值为770 GHz, 以2.73 GHz/μs的速度变化, Pin = 1 W); (b) 多脉冲光场的光谱图; (c) 两个微腔内的光功率随时间的变化曲线; (d) D端口和T端口输出功率随时间的变化曲线
Fig. 6. (a) Evolution of a bright soliton to a multi-pulse optical field (δ2 = 16.1411 m–1, the initial value of Δf1 is 770 GHz, and the change speed of Δf1 is 2.73 GHz/μs, Pin = 1 W); (b) spectrum of the multi-pulse; (c) curves of the dual power inside the dual coupled microcavities vary with the slow time; (d) curves of the dual power of Port D and T vary with the slow time.
-
[1] 孟飞, 曹士英, 蔡岳, 王贵重, 曹建平, 李天初, 方占军 2011 60 100601
Google Scholar
Meng F, Cao S Y, Cai Y, Wang G Z, Cao J P, Li T C, Fang Z J 2011 Acta Phys. Sin. 60 100601
Google Scholar
[2] Del’Haye P, Coillet A, Fortier T, Beha K, Cole D C, Yang K Y, Lee H, Vahala K J, Papp S B, Diddams S A 2016 Nat. Photonics 10 516
Google Scholar
[3] Lamb E S, Carlson D R, Hickstein D D, Stone J R, Diddams S A, Papp S B 2018 Phys. Rev. Appl. 9 024030
Google Scholar
[4] Newman Z L, Maurice V, Drake T, Stone J R, Briles T C, Spencer D T, Fredrick C, Li Q, Westly D, Ilic B R, Shen B, Suh M G, Yang K Y, Johnson C, Johnson D M S, Hollberg L, Vahala K J, Srinivasan K, Diddams S A, Kitching J, Papp S, Hummon M T 2019 Optica 6 680
Google Scholar
[5] Zhang X Y, Cao Q T, Wang Z, Liu Y X, Qiu C W, Yang L, Gong Q H, Xiao Y F 2019 Nat. Photonics 13 2
Google Scholar
[6] Wang W Q, Lu Z Z, Zhang W F, Chu S T, Little B E, Wang L R, Xie X P, Liu M L, Yang Q H, Wang L, Zhao J G, Wang G X, Sun Q B, Liu Y S, Wang Y S, Zhao W 2018 Opt. Lett. 43 2002
Google Scholar
[7] Xue X X, Xuan Y, Liu Y, Wang P H, Chen S, Wang J, Leaird D E, Qi M H, Weiner A M 2015 Nat. Photonics 9 594
Google Scholar
[8] Coillet A, Balakireva I, Henriet R, Saleh K, Larger L, Dudley J M, Menyuk C R, Chembo Y K 2013 IEEE Photonics J. 5 6100409
Google Scholar
[9] Matsko A B, Liang W, Savchenkov A A, Maleki L 2013 Opt. Lett. 38 525
Google Scholar
[10] Yi X, Yang Q F, Yang K Y, Suh M G, Vahala K 2015 Optica 2 1078
Google Scholar
[11] Pasquazi A, Caspani L, Peccianti M, Clerici M, Ferrera M, Razzari L, Duchesne D, Little B E, Chu S T, Moss D J, Morandotti R 2013 Opt. Express 21 13333
Google Scholar
[12] Ferrera M, Reimer C, Pasquazi A, Peccianti M, Clerici M, Caspani L, Chu S T, Little B E, Morandotti R, Moss D J 2014 Opt. Express 22 21488
Google Scholar
[13] Riemensberger J, Hartinger K, Herr T, Brasch V, Holzwarth R, Kippenberg T J 2012 Opt. Express 20 27661
Google Scholar
[14] Xue X X, Zheng X P, Zhou B K 2019 Nat. Photonics 13 616
Google Scholar
[15] Maes B, Fiers M, Bienstman P 2009 Phys. Rev. A 80 033805
Google Scholar
[16] Maes B, Soljacic M, Joannopoulos J D 2006 Opt. Express 14 10678
Google Scholar
[17] Dumeige Y, Ghisa L, Féron P 2006 Opt. Lett. 31 2187
Google Scholar
[18] Dumeige Y, Féron P 2015 Opt. Lett. 40 3237
Google Scholar
[19] Coen S, Haelterman M 2001 Opt. Lett. 26 39
Google Scholar
[20] Akhmediev N, Pelinovsky E 2010 Eur. Phys. J. Spec. Top. 185 1
Google Scholar
[21] Xue X X, Leo F, Xuan Y, Villegas J A J, Wang P H, Leaird D E, Erkintalo M, Qi M H, Weiner A M 2017 Light: Sci. Appl. 6 e16253
Google Scholar
[22] Peng B, Ozdemir S K, Zhu J G, Yang L 2013 Opt. Lett. 37 3435
Google Scholar
[23] Bo F, Oezdemir S K, Peng B, Wang J, Zhang G Q, Xu J J, Yang L 2015 Opt. Express 23 30793
Google Scholar
[24] Kippenberg T J, Holzwarth R, Diddams S A 2011 Science 332 555
Google Scholar
下载:
计量
- 文章访问数: 7829
- PDF下载量: 250
- 被引次数: 0
